NCERT Exemplar Class 10 Maths Solutions Chapter 10 Constructions

Question:1

To divide a line segment AB in the ratio $5:7$, first, a ray AX is drawn so that$\angle BAX$ is an acute angle, and then at equal distances, points are marked on the ray AX such that the minimum number of these points is
(A) 8 (B) 10 (C) 11 (D) 12

Answer(D) 12
Solution
Given: $\angle BAX$ is an acute angle.
The required ratio is $5:7$
Let m = 5, n = 7

Steps of construction
1. Draw any ray AX making an acute angle with AB.
2. Locate 12 points on AX at equal distances. (Because here $m+n= 12$)
3. Join $A_{12}B$
4. Through the point $A_{5}$ draw a line parallel to $A_{12}B$ intersecting AB at the point P.
Then $AP:PB= 5:7$
$\because A_{5}P\parallel A_{12}B$
$\therefore \frac{AA_{5}}{A_{5}A_{12}}= \frac{AP}{PB}$ (By Basic Proportionality theorem)
By construction $\frac{AA_{5}}{A_{5}A_{12}}= \frac{5}{7}$
$\therefore \frac{AP}{PB}= \frac{5}{7}$
Hence, the number of points is 12.

Question:2

To divide a line segment AB in the ratio $4:7$, a ray AX is drawn first such that $\angle BAX$ is an acute angle and then points $A_{1}, A_{2 }, A_{3}\cdots$ are located at equal distances on the ray AX and the point B is joined to:
(A) A₁₂ (B) A₁₁ (C) A₁₀ (D) A₉

Answer(B) A₁₁
Solution
Given: $\angle BAX$ is an acute angle
The required ratio is $4:7$
Let m = 4, n = 7
$m+n= 4+7= 11$

Steps of construction
1. Draw any ray AX making an acute angle with AB.
2. Locate 11 points on AX at equal distances (because m + n = 11)
3. Join $A_{11}B$
4. Through the point A4 draw a line parallel to $A_{11}B$ intersecting AB at the point P.
Then $AP:PB= 4:7$
Hence, point B is joined to A₁₁.

Question:3

To divide a line segment AB in the ratio $5:6$, draw a ray AX such that $\angle BAX$ is an acute angle, then draw a ray BY parallel to AX and the points $A_{ 1}, A_ {2}, A_{3}\cdots$and $B _{1}, B_{2}, B_{3}\cdots$are located at equal distances on ray AX and BY, respectively. Then the points joined are
(A) A₅ and B₆ (B) A­ and B₅ (C) A₄ and B₅ (D) A₅ and B₄

Answer(A) A₅ and B₆

Solution
Given: $\angle BAX$ and $\angle ABY$ both are acute angles and AX parallel to BY
The required ratio is $5:6$
Let m = 5 , n = 6

Steps of construction
1. Draw any ray AX making an acute angle with AB.
2. Draw a ray $BY\parallel AX$.
3. Locate the points $A_{1},A_{2},A_{3},A_{4},A_{5}$ on AX at equal distances
4. Locate the points $B_{1}, B_{2}, B_{3 }, B_{ 4 }, B_{5}$ on BY at a distance equal to the distance between points on the AX line.
5. Join $A_{5}B_{6}$.
Let it intersect AB at a point C in the figure.
Then $AC:CB= 5:6$
Here $\bigtriangleup AA_{5}C$ is similar to $\bigtriangleup BB_{6}C$
Then $\frac{AA_{5}}{BB_{6}}= \frac{5}{6}= \frac{AC}{BC}$
$\therefore$by construction $\frac{AA_{5}}{BB_{6}}= \frac{5}{6}$
$\therefore \frac{AC}{BC}= \frac{5}{6}$
$\therefore$ Points joined one A₅ and B₆.

Question:4

To construct a triangle similar to a given $\bigtriangleup ABC$ with its sides of $\frac{3}{7}$ the corresponding sides of $\bigtriangleup ABC$, first, draw a ray BX such that CBX is an acute angle and X lies on the opposite side of A Aconcerningo BC. Then locate points B₁, B₂, B₃, ... on BX at equal distances, and the next step is to join
(A) B₁₀ to C (B) B₃ to C (C) B₇ to C (D) B₄ to C

Answer(C) B₇ to C
Solution Given: $\angle CBX$ is an acute angle.

Steps of construction
1. Draw any ray BX making an angle with BC on the side opposite vertex A.
2. Locate 7 points on BX in equidistant
3. Now join B₇ to C
4. Draw a line through B₃${C}'$ parallel to B₇C .

Question:5

To construct a triangle similar to a given $\bigtriangleup ABC$ with its sides $\frac{8}{5}$ of the corresponding sides of $\bigtriangleup ABC$ draw a ray BX such that $\angle CBX$ is an acute angle and X is on the opposite side of A concerning BC. The minimum number of points to be located at equal distances on ray BX is
(A) 5 (B) 8 (C) 13 (D) 3

Answer (B) 8
Solution
To construct a triangle similar to a triangle, with its sides $\frac{8}{5}$ of the corresponding sides of a given triangle, the minimum number of points to be located at an equal distance is equal to the greater of 8 and 5 in .$\frac{8}{5}$ Here $8> 5$
So, the minimum number of points to be located at equal distances on ray BX is 8.

Question:6

To draw a pair of tangents to a circle which are inclined to each other at an Angle of $60^{\circ}$, it is required to draw tangents at the endpoints of those two radii of the circle, and the angle between them should be
(A) $135^{\circ}$ (B) $90^{\circ}$ (C) $60^{\circ}$ (D) $120^{\circ}$

Answer(D) 120°
Solution
According to question:-

Given :$\angle QPR= 60^{\circ}$
Let $\angle QOR= x$
As we know, the angle between the agent and the radius of a circle is 90 degrees
$\angle PQO= \angle PRO= 90^{\circ}$
We know that $\angle PQO+ \angle PRO+ \angle QPR+\angle QOR= 360^{\circ}$
[$\because$ sum of interior angles of quadrilateral is $360^{\circ}$ ]
$90^{\circ}+90^{\circ}+x+60^{\circ}= 360^{\circ}$|
$240+x= 360^{\circ}$
$x= 120^{\circ}$

Question:1

Write True or False and give reasons for your answer in each of the following:
By geometrical construction, it is possible to divide a line segment in the ratio $\sqrt{3}:\frac{1}{\sqrt{3}}$

Answer [True]
Solution
We need both positive integers to divide a line segment in the ratio.
So, we can simplify it by multiplying both the terms by .$\sqrt{3}$
We obtain $\sqrt{3}\times \sqrt{3} : \sqrt{3}\times \frac{1}{\sqrt{3}}$
$\Rightarrow 3:1$
So, the required ratio is $3:1$
In geometrical construction is possible to divide a line segment in the ratio $3:1$

Question:2

Write True or False and give reasons for your answer in each of the following:
To construct a triangle similar to a given $\bigtriangleup ABC$ with its sides $\frac{7}{3}$ of the corresponding sides of $\bigtriangleup ABC$, draw a ray BX making an acute angle with BCand X lies on the opposite side of A concerning BC. The points B₁, B₂,..., B₇ are located at equal distances on BX, B3 is joined to C and then a line segment $B_6{C}'$ is drawn parallel to B₃C where ${C}'$ lies on BC produced. Finally, line segment ${A}'{C}'$ is drawn parallel to AC.

Answer: False
Solution
According to the question:-
To construct a triangle similar to a given $\bigtriangleup ABC$ with its sides $\frac{7}{3}$ of the corresponding sides of $\bigtriangleup ABC$
1. Draw a line segment BC
2. Taking B and C as centres, draw two arcs of suitable radii intersecting each other at A.
3. Join BA and CA?ABC is the required triangle.
4. From B draw any ray BX downwards making an acute angle CBX.
5. Locate seven points B₁, B₂, b₃, …. B₇ on BX such that BB₁ = B₁B₂ = B₁B₃ = B₃B₄ = B₄B₅ = B₅B₆ = B₆B₇.
6. Join B₃C and from B₇ draw a line B₇C’ ? B₃C intersecting the extended line segment BC at C’.
7. From point C’ draw C’A’? CAintersectsg the extended line segment BA at A

But as given, if we join B₃C and from B₆ draw a line B₆C’? B₃C intersecting the extended line segment BC at C’.
$BB_{3}/BB_{6}={BC}/{{BC}'} = 3/6$
${BC}/{{BC}'} = 1/2$
$BC:{BC}'= 1:2$
Hence, the sides are not in the ratio of 7:3
So, the required triangle can not be constructed in this way.
Hence, the given statement is false.

Question:3

Write True or False and give reasons for your answer in each of the following:
A pair of tangents can be constructed from a point P to a circle of radius 3.5 cm situated at a distance of 3 cm from the centre

Answer [False]
Solution
Radius, r = 3.5 cm
Point distance from centre = 3 cm
But, $r> d\Rightarrow 3.5 cm> 3cm$
So, the point P lies inside the circle.
So, a pair of tangents cannot be drawn to point P to a circle.

Question:4

Write True or False and give reasons for your answer in each of the following:
A pair of tangents can be constructed to a circle inclined at an angle of $ 170^ 70^{\circ}$.

Answer [True]
Solution
Tangent: - It is a straight line that touches the curve but does not cross it. A pair of tangents can be constructed to a circle inclined at an angle greater than $0^{\circ}$ but less than $180^{\circ}$. Here, the inclination angle is $170^{\circ}$. Hence, it is possible.

Question:1

Draw a line segment of length 7 cm. Find a point P on it which divides it in the ratio $3:5$.

Solution
Given: AB = 7cm
The required ratio is $3:5$
Let m = 3, n = 5
$m+n= 3+5= 8$

Steps of construction
1. Draw a line segment AB = 7cm.
2. Draw a ray AX making an acute angle with AB
3. Locate 8 points $A_{1},A_{2},A_{3}\cdots A_{8}$ on AX on equidistant. (because $m+n= 8$ )
4. Join $BA_{8}$
5. Through the point $A_{3}$ draw a line parallel to $BA_{8}$ which intersect line AB at P..
Here triangle AA₃P is similar to triangle $AA_{8}B$
$AA_{3}/AA_{8}= AP/AB= 3/5$ (by construction)
Therefore $AP:BP= 3:5$

Question:2

Draw a right triangle ABC in which BC = 12 cm, AB = 5 cm and $\angle B=90^ {\circ}$.Construct a triangle similar to it and of scale factor $\frac{2}{3}$. Is the new triangle also a right triangle

Solution
Given BC = 12cm, AB = 5 cm, $\angle B= 90^{\circ}$

Steps of construction
1. Draw a line $B C=12 \mathrm{~cm}$
2. From $B$ draw $A B=5 \mathrm{~cm}$ which makes an angle of $90^{\circ}$ at $B$.
3. Join AC
4. Make an acute angle at B as $<C B X$
5. On BX mark 3 points at equal distance $X_1, X_2, X_3$.
6. Join $ X_3C$
7. From $X_2$ draw $X_2 C^{\prime} \| X_3 C$ intersect AB at $C^{\prime}$
8. From point $C^{\prime}$ draw $C^{\prime} A^{\prime} \| C A$ intersect $\mathrm{AB} A^{\prime}$

Now $\triangle A^{\prime} B C^{\prime}$ is the required triangle and $\triangle A^{\prime} B C^{\prime}$ is also a right triangle.

Question:3

Draw a triangle ABC in which BC = 6 cm, CA = 5 cm, and AB = 4cm. Construct a triangle similar to it and of scale factor$\frac{5}{3}$

Solution
Given : BC = 6cm, CA = 5cm, AB = 4cm
Scale factor = 5/3
Let m = 5 and n = 3

Steps of construction

1. Draw a line BC = 6c cm

2. Taking B and C as centre mark arcs of length 4cm and 5cm respectively,y intersects each other at point A.

3. Join BA and CA

4 . Draw a ray BX making a g acute angle with BC.

5.Locate 5 Points on BX in equidistant as B₁, B₂, B₃, B₄, B₅.

6.Join B₃ to C and draw a line through B5 parallel to B₃C to intersect at BC extended at ${C}'$.

7. Draw a line through ${C}'$ parallel to AC intersect AB extended at ${A}'$ .
Now ${A}'B{C}'$ is required triangle.

Question:4

Construct a tangent to a circle of radius 4 cm from a point which is at a distance of 6 cm from its centre.

Solution
Given : Radius = 4cm

Steps of construction
1. Draw a circle at radius r = 4 cm at point O.
2. Take a point P at a distance of 6cm from point O and join PO
3. Draw a perpendicular bisector of line PO. M is the midpoint of PO.
4. Taking M as the centre, we draw another circle of radius equal to MO, and it intersects the given circle at points Q and R
5. Now, join PQ and PR.
Then PQ and PR one the required two tangents.

Question:1

Two line segments AB and AC include an angle of $60^{\circ}$ where AB = 5 cm and AC = 7 cm. Locate points P and Q on AB and AC, respectively,y such that $AP= \frac{3}{4}AB$ and $AQ= \frac{1}{4} AB$. Join P and Q and measure the length of PQ.

Solution
Given :
AB = 5 cm and AC = 7 cm
$AP= \frac{3}{4}AB\: \: \cdots 1$
$AQ= \frac{1}{4}AC\: \: \cdots 2$
From equation 1
$AP= \frac{3}{4}AB$
$AP= \frac{3}{4}\times 5= \frac{15}{4}\: \: \left [ \because AB= 5cm \right ]$
P is any point on B
$\therefore PB= AB-AP= 5-\frac{15}{4}= \frac{20-15}{4}= \frac{5}{4}cm$
$\frac{AP}{AB}= \frac{15}{4}\times \frac{4}{5}= \frac{1}{3}$
$AP:AB= 1:3$
$\therefore$scale of a line segment AB is $\frac{1}{3}$


Steps of construction
1. Draw a line segment AB = 5 cm
2. Now draw ray AO which makes an angle,
3. Which A as centre and radius equal to 7 cm, draw an arc cutting line AO at C
4. Draw ray AP with acute angle BAP
5. Along AP make 4 points $A_{1},A_{2},A_{3},A_{4}$ with equal distance.
6. Join $A_{4}B$
7. From $A_{3}$ draw $A_{3}P$ which is parallel to $A_{4}B$ which meet AB at point P.
Then P is a point which divides AB in a ratio 3: 1
AP : PB = 3 : 1
8. Now draw ray AQ, with an acute angle CAQ.
9. Along AQ mark 4 points $B_{1},B_{2},B_{3},B_{4}$ with equal distance.
10. Join $B_{4}C$
11. From $B_{1}$ draw $B_{1}Q$ which is parallel to $B_{4}C$ which meet AC at point Q.
Then Q is a point which divides AC in a ratio 1 : 3
AQ : QC = 1 : 3
12. Finally, you join PQ, and its measurement is 3.25 cm.

Question:2

Draw a parallelogram ABCD in which BC = 5 cm, AB = 3 cm, and ABC=60^ 60^{\circ}$, and divide it into triangles BCD and ABD
by the diagonal BD. Construct the triangle BD'C' similar to DBDC with scale factor $\frac{4}{3}$. Draw the line segment D'A' parallel to DA where A' lies on the extended side BA. Is A'BC'D' a parallelogram?

Solution

Steps of construction
1. Draw, A-line AB = 3 cm
2 Draw a ray by making $\angle ABP= 60^{\circ}$
3. Taking B as the centre and radius equal to 5 cm. Draw an arc which cuts BP at point C
4. Again draw ray AX making $\angle {Q}'AX= 60^{\circ}$
5. With A as the centre and radius equal to 5 cm, draw an arc which cuts AX at point D
6. Join C and D Here ABCD is a parallelogram
7. Join BD , BD is a diagonal of parallelogram ABCD
8. From B draw a ray BQ with any acute angle at po, int B, i.e., $\angle CBQ$ is the acute angle
9. Locate 4 points $B_{1},B_{2},B_{3},B_{4}$ on BQ with equal distance.
10. Join $B_{3}C$ and from $B_{4},{C}'$ parallel to $B_{3}C$ which intersect at point ${C}'$
11. From point ${C}'$ draw line ${C}'{D}'$ which is parallel to CD
12. Now draw a line segment ${D}'{A}'$ parallel to DA
Note : Here ${A}',{C}'$ and ${D}'$ are the extended sides.
13. ${A}'B{C}'{D}'$ is a parallelogram in which ${A}'{D}'= 6\cdot 5\, cm$ and ${A}'{B}= 4\, cm$ and ${A}'B{D}'= 60^{\circ}$ divide it into triangles $B{C}'{D}'$ and ${A}'{BD}'$ by the diagonal ${BD}'$

Question:3

Draw two concentric circles of radii 3 cm and 5 cm. Taking a point on the outer circle construct the pair of tangents to the other. Measure the length of a tangent and verify it by actual calculation.

Solution

Steps of construction
1. Draw two concentric circles with centre O and radii 3 cm and 5 cm
2. Taking any point P on the outer cijoin, join P and O
3. Draw a perpendicular bisector of OP let M be the midpoint of OP
4. Taking M as the centre and OM as the radius, draw a circle which cuts the inner circle at Q and R
5. Join PQ and PR. Thu, PQ and PR are required tangents
On measuring PQ and PR, we find that PQ = PR = 4 cm
Calculations
$\bigtriangleup OQP, \angle OQP= 90^{\circ}$
[usiPythagoras' theorem]
$\left ( 5 \right )^{2}= \left ( 3 \right )^{2}+\left ( PQ \right )^{2}$
$25-9= PQ^{2}$
$16= PQ^{2}$
$\sqrt{16}= PQ$
$4cm= PQ$
H, the length of both tangents is 4 cm.

Question:4

Draw an isosceles triangle ABC in which AB = AC = 6 cm and BC = 5 cm. Construct a triangle PQR similar to ABC in which PQ =8 cm. Also, justify the Construction.

Solution

Steps of construction
1. a Draw a line BC = 5 cm
2. Taking B and C as centres, draw two arcs of equal radius 6 cm intersecting each other at point A.
3. Join AB and AC DABC is required isosceles triangle
4 From B draw ray $B_{X}$ with an acute angle $CB{B}'$
6. draw $B_{1},B_{2},B_{3},B_{4}$ at $BX$ with equal distance
7. Join $B_{3}C$ and from $B_{4}$ draw line $B_{4}D\parallel B_{3}C,$ , intersect extended segment BC at point D.
8. From point D draw $DE\parallel CA$ meting BA produced at E.
Then EBD is required triangle. We can name it PQR.
Justification
$\because B_{4}D\parallel B_{3}C$
$\therefore \frac{BC}{CD}= \frac{3}{1}\Rightarrow \frac{CD}{BC}= \frac{1}{3}$
$Now\, \therefore \frac{BD}{BC}= \frac{BC+CD}{BC}= 1+\frac{CD}{BC}= 1+\frac{1}{3}= \frac{4}{3}$
$Also\, DE\parallel CA$
$\therefore \bigtriangleup ABC\sim \bigtriangleup DBE$
$\frac{EB}{AB}= \frac{DE}{CA}= \frac{BD}{BC}= \frac{4}{3}$

Question:5

Draw a triangle ABC in which AB = 5 cm, BC = 6 cm and $ ABC=60^ {\circ}$.Construct a triangle similar to ABC with scale factor $\frac{5}{7}$. Justify the construction

Solution
Given : AB = 5 cm, BC = 6 cm

Steps of construction

1. Draw a line segment AB = 5 cm

2.draw $< ABO= 60^{\circ}$ B taking as a centre draw an arc of radius BC=6cm

3. Join AC, DABC is the required triangle

4.From point A draw any ray $A{A}'$ with acute angle $\angle BA{A}'$

5.Mark 7 points $B_{1},B_{2},B_{3},B_{4},B_{5},B_{6},B_{7}$ with equal distance.

6.Join $B_{7}B$ and form $B_{5}$ draw $B_{5}X\parallel B_{7}B\, \, BY$making the angle equal From point X draw $XY\parallel BC$ intersecting AC at Y. Then, DAMN is the required triangle whose sides are equal to $\frac{5}{7}$ of the corresponding sides of the $\bigtriangleup ABC.

Justification: Here, $B_{5X}\parallel B_{7}B$ [by construction]
$\therefore \frac{AX}{XB}= \frac{5}{2}\Rightarrow \frac{XB}{AX}= \frac{2}{5}$
Now $\frac{AB}{AX}= \frac{AX+XB}{AX}$
$1+\frac{XB}{AX}= 1+\frac{2}{5}= \frac{7}{5}$
Also, $XY\parallel BC$
$\therefore \bigtriangleup AXY\sim \bigtriangleup ABC$
$\frac{AX}{AB}= \frac{AY}{AC}= \frac{YX}{BC}= \frac{5}{7}$

Question:6

Draw a circle of radius 4 cm. Construct a pair of tangents to it, the angle between which is $60^{\circ}$. Also, justify the
construction. Measure the distance between the centre of the circle and the point of intersection of tangents

Solution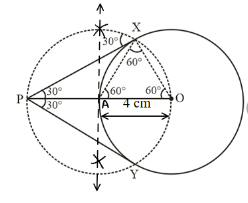

Steps of construction
1. Draw a circle of radius OA = 4 cm with centre O
2. Produce OA to P such that $OA= AP=4 cm$
3. Draw a perpendicular bisector of OP = 8 =8cm
4. Now, taking A as the centre, we draw the circle of radius AP = OA = 4 cm
5Which intersectsst the circle at x and y
6. Join PX and PY
7. PX and PY are the tangents to the circle
Justification
In $\bigtriangleup OAX$ we have
$OA= OP= 4\, cm$ (Radius)
$AX= 4\, cm$ (Radius of circle with centre A)
$\therefore OAX$ is equilateral triangle
$\angle OAX= 60^{\circ}$
$\Rightarrow \angle XAP= 120^{\circ}$
In $\bigtriangleup PAX$ we have
$PA= AX= 4cm$
$\angle XAP= 120^{\circ}$
$\angle APX= 30^{\circ}$
$\Rightarrow APY= 30^{\circ}$
Hence $\angle XPY= 60^{\circ}$

Question:7

Draw a triangle ABC in which AB = 4 cm, BC = 6 cm and AC = 9 cm. Construct a triangle similar to $\bigtriangleup ABC$ with scale factor $\frac{3}{2}$ . Justify the construction. Are the two triangles congruent? Note that all three angles and two sides of the two triangles are equal.

Solution

Steps of construction

1 . Draw a line segment BC = 6 cm

2 . Taking B and C as centres, draw an arc of radius AB 4cm and AC = 9 cm

3. Join AB and AC

4 . Triangle ABC is a required triangle. From Bd raw ray BM with acute angle $\angle XBM$

5.Make 3 points $B_{1},B_{2},B_{3}$ on BM with equal distance

6.Join $B_{2}C$ and $B_{3}$ draw $B_{3}X\parallel B_{2}C$ intersecting BC at X From point X draw XY||CA intersecting the extended line segment BA to Y Then $\bigtriangleup BXY$ is the required triangle whose sides are equal to$\frac{3}{2}$ of the $\bigtriangleup ABC$
Justification :
Here $B_{3}X\parallel B_{2}C$
$\therefore \frac{BC}{CX}= \frac{2}{1}$
$\therefore \frac{BX}{BC}= \frac{BC+CX}{BC}= 1+\frac{1}{2}= \frac{3}{2}$
Also $XY\parallel CA$
$\bigtriangleup ABC\sim \bigtriangleup YBX$
$\therefore \frac{YB}{AB}= \frac{YX}{AC}= \frac{BX}{BC}= \frac{3}{2}$.

Here are three angles that are the same, but the three sides are not the same.
$\therefore$The two triangles are not congruent because, if two triangles are congruent, then they have the same shape and size.