NCERT Solutions for Class 10 Maths Chapter 6 Triangles
We have seen clothes hangers, sandwiches, traffic signboards, etc. Do you know which shape they represent? All these things are triangular. Triangles are fundamental geometric 2-D shapes characterised by three sides, three angles and a sum of 180 degrees for their interior angles. It is a polygon with the least number of sides. In this chapter, you will learn about different kind of triangles and their amazing properties. NCERT Solutions for Class 10 can help the students understand these concepts with clarity and make them efficient in solving problems involving triangles.
This Story also Contains
- NCERT Solutions for Class 10 Maths Chapter 6 Triangles PDF Free Download
- NCERT Solutions for Class 10 Maths Chapter 6 Triangles (Exercise)
- Triangles Class 10 Solutions: Exercise Wise
- Class 10 Maths NCERT Chapter 6: Extra Question
- Triangles Class 10 Chapter 6: Topics
- NCERT Solutions for Class 10 Maths Chapter 2 Triangles: Notes
- NCERT Solutions for Class 10 Maths: Chapter Wise
NCERT Solutions for Class 10 Maths provide clear and step-by-step solutions to the exercise problems in the NCERT textbook. Students who are in need of the quadratic equation solutions will find this article very useful. It covers questions from all the topics and will help you improve your speed and accuracy. These NCERT Solutions are trustworthy and reliable, as they are created by subject matter experts at Careers360, making them an essential resource for exam preparation. Get all solved exercises, full syllabus notes, and a free PDF from this NCERT article.
NCERT Solutions for Class 10 Maths Chapter 6 Triangles PDF Free Download
Careers360 brings you NCERT Class 10 Maths Chapter 6 Triangles solutions, carefully prepared by subject experts to simplify your studies and help in exams. A downloadable PDF has been provided — click on the link below to access it.
NCERT Solutions for Class 10 Maths Chapter 6 Triangles (Exercise)
Below are the detailed NCERT Class 10 Maths Chapter 6 Triangles question answers provided in the textbook.
| Triangles Class 10 Question Answers Exercise: 6.1 Page number: 78 Total questions: 3 |
Answer:
All circles are similar.
Since all the circles have a similar shape. They may have different radii, but the shape of all circles is the same.
Therefore, all circles are similar.
Answer:
All squares are similar.
Since all the squares have a similar shape. They may have a different side, but the shape of all squares is the same.
Therefore, all squares are similar.
Answer:
All equilateral triangles are similar.
Since all the equilateral triangles have a similar shape. They may have different sides, but the shape of all equilateral triangles is the same.
Therefore, all equilateral triangles are similar.
Answer:
Two polygons of the same number of sides are similar if their corresponding angles are equal and their corresponding sides are proportional.
Thus, (a) equal
(b) proportional
Q2 (i): Give two different examples of a pair of similar figures.
Answer:
The two different examples of a pair of similar figures are :
1. Two circles with different radii.

2. Two rectangles with different breadth and length.

Q2 (ii): Give two different examples of a pair of non-similar figures.
Answer:
The two different examples of a pair of non-similar figures are :
1. Rectangle and circle

2. A circle and a triangle.

Q3: State whether the following quadrilaterals are similar or not:

Answer:
Quadrilateral PQRS and ABCD are not similar as their corresponding sides are proportional, i.e. $1:2$, but their corresponding angles are not equal.
| Triangles Class 10 Question Answers Exercise: 6.2 Page number: 84-85 Total questions: 10 |
Q1: In Fig. 6.17, (i) and (ii), DE || BC. Find EC in (i) and AD in (ii).

Answer:
(i)
Let EC be x
Given: DE || BC
By using the proportionality theorem, we get
$\frac{AD}{DB}=\frac{AE}{EC}$
$\Rightarrow \frac{1.5}{3}=\frac{1}{x}$
$\Rightarrow x=\frac{3}{1.5}=2\, cm$
$\therefore EC=2\, cm$
(ii)
Let AD be x
Given: DE || BC
By using the proportionality theorem, we get
$\frac{AD}{DB}=\frac{AE}{EC}$
$\Rightarrow \frac{x}{7.2}=\frac{1.8}{5.4}$
$\Rightarrow x=\frac{7.2}{3}=2.4\, cm$
$\therefore AD=2.4\, cm$
Answer:
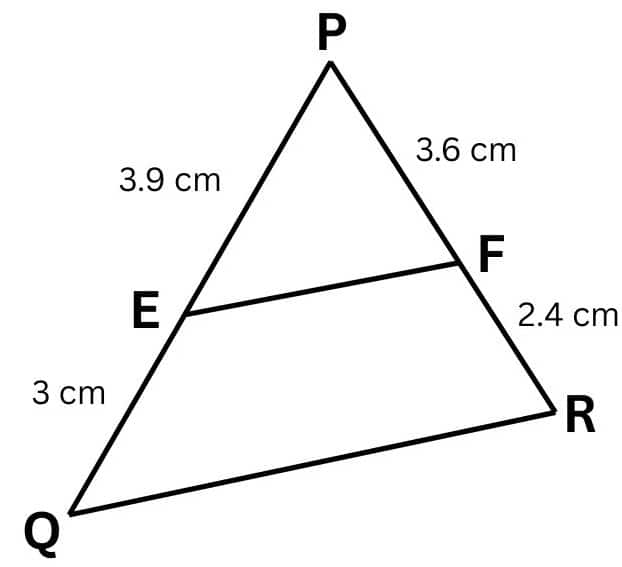
Given :
PE = 3.9 cm, EQ = 3 cm, PF = 3.6 cm and FR = 2.4 cm
$\frac{PE}{EQ}=\frac{3.9}{3}=1.3\, cm$ and $\frac{PF}{FR}=\frac{3.6}{2.4}=1.5\, cm$
We have
$\frac{PE}{EQ} \neq \frac{PF}{FR}$
Hence, EF is not parallel to QR.
Answer:
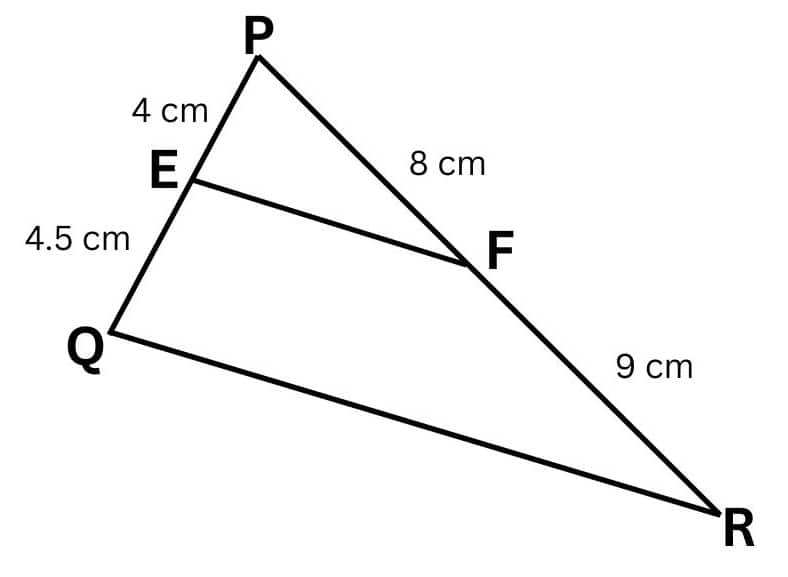
Given :
PE = 4 cm, QE = 4.5 cm, PF = 8 cm and RF = 9 cm
$\frac{PE}{EQ}=\frac{4}{4.5}=\frac{8}{9}\, cm$ and $\frac{PF}{FR}=\frac{8}{9}\, cm$
We have
$\frac{PE}{EQ} = \frac{PF}{FR}$
Hence, EF is parallel to QR.
Q2 (iii): E and F are points on the sides PQ and PR, respectively, of a triangle PQR. For each of the following cases, state whether EF || QR : PQ = 1.28 cm, PR = 2.56 cm, PE = 0.18 cm and PF = 0.36 cm
Answer:

Given :
PQ = 1.28 cm, PR = 2.56 cm, PE = 0.18 cm and PF = 0.36 cm
$\frac{PE}{PQ}=\frac{0.18}{1.28}=\frac{9}{64}\, cm$ and $\frac{PF}{PR}=\frac{0.36}{2.56}=\frac{9}{64}\, cm$
We have
$\frac{PE}{EQ} = \frac{PF}{FR}$
Hence, EF is parallel to QR.
Q3: In Fig. 6.18, if LM || CB and LN || CD, prove that $\frac{AM}{AB} = \frac{AN}{AD }$

Answer:
Given : LM || CB and LN || CD
To prove :
$\frac{AM}{AB} = \frac{AN}{AD }$
Since , LM || CB so we have
$\frac{AM}{AB}=\frac{AL}{AC}.............................................(1)$
Also, LN || CD
$\frac{AL}{AC}=\frac{AN}{AD}.............................................(2)$
From equations 1 and 2, we have
$\frac{AM}{AB} = \frac{AN}{AD }$
Hence proved.
Q4: In Fig. 6.19, DE || AC and DF || AE. Prove that BF / FE = BE / EC

Answer:
Given: DE || AC and DF || AE.
To prove :
$\frac{BF}{FE} = \frac{BE}{EC }$
Since , DE || AC so we have
$\frac{BD}{DA}=\frac{BE}{EC}.............................................1$
Also, DF || AE
$\frac{BD}{DA}=\frac{BF}{FE}.............................................2$
From equations 1 and 2, we have
$\frac{BF}{FE} = \frac{BE}{EC }$
Hence proved.
Q5: In Fig. 6.20, DE || OQ and DF || OR. Show that EF || QR.

Answer:
Given: DE || OQ and DF || OR.
To prove EF || QR.
Since DE || OQ, so we have
$\frac{PE}{EQ}=\frac{PD}{DO}.............................................1$
Also, DF || OR
$\frac{PF}{FR}=\frac{PD}{DO}.............................................2$
From equations 1 and 2, we have
$\frac{PE}{EQ} = \frac{PF}{FR }$
Thus, EF || QR. (converse of the basic proportionality theorem)
Hence proved.

Answer:
Given : AB || PQ and AC || PR
To prove: BC || QR
Since, AB || PQ so we have
$\frac{OA}{AP}=\frac{OB}{BQ}.............................................1$
Also, AC || PR
$\frac{OA}{AP}=\frac{OC}{CR}.............................................2$
From equations 1 and 2, we have
$\frac{OB}{BQ} = \frac{OC}{CR }$
Therefore, BC || QR. (converse basic proportionality theorem)
Hence proved.
Answer:
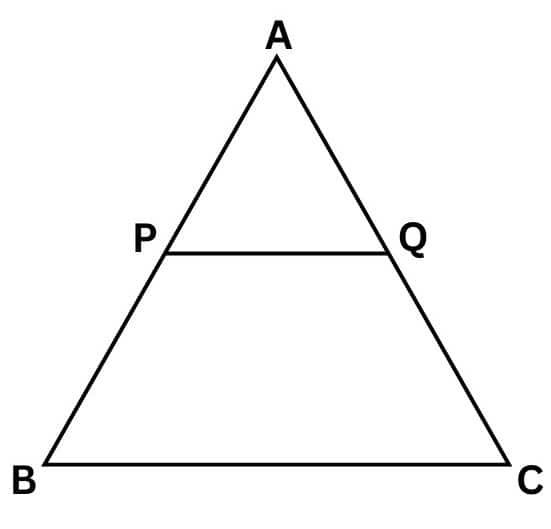
Let PQ be a line passing through the midpoint of line AB and parallel to line BC, intersecting line AC at point Q.
i.e. $PQ||BC$ and $AP=PB$ .
Using the basic proportionality theorem, we have
$\frac{AP}{PB}=\frac{AQ}{QC}..........................1$
Since $AP=PB$
$\frac{AQ}{QC}=\frac{1}{1}$
$\Rightarrow AQ=QC$
$\therefore$ Q is the midpoint of AC.
Answer:

Let P be the midpoint of line AB and Q be the midpoint of line AC.
PQ is the line joining midpoints P and Q of lines AB and AC, respectively.
i.e. $AQ=QC$ and $AP=PB$ .
We have,
$\frac{AP}{PB}=\frac{1}{1}..........................1$
$\frac{AQ}{QC}=\frac{1}{1}...................................2$
From equations 1 and 2, we get
$\frac{AQ}{QC}=\frac{AP}{PB}$
$\therefore$ By basic proportionality theorem, we have $PQ||BC$
Answer:
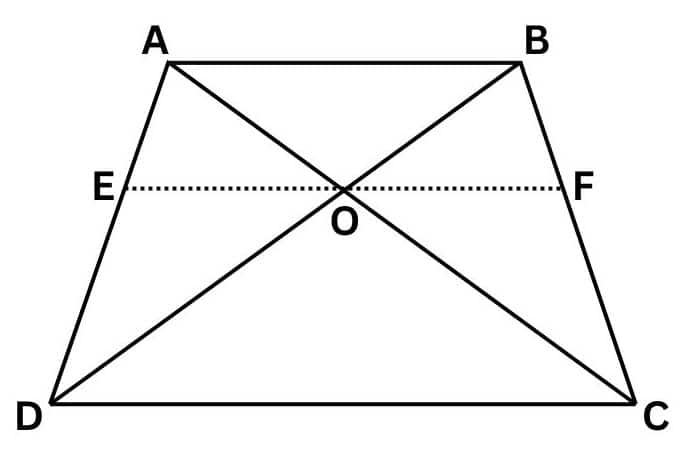
Draw a line EF passing through point O such that $EO||CD\, \, and\, \, FO||CD$
To prove :
$\frac{AO}{BO} = \frac{CO}{DO}$
In $\triangle ADC$ , we have $CD||EO$
So, by using the basic proportionality theorem,
$\frac{AE}{ED}=\frac{AO}{OC}........................................1$
In $\triangle ABD$ , we have $AB||EO$
So, by using the basic proportionality theorem,
$\frac{DE}{EA}=\frac{OD}{BO}........................................2$
Using equations 1 and 2, we get
$\frac{AO}{OC}=\frac{BO}{OD}$
$\Rightarrow \frac{AO}{BO} = \frac{CO}{DO}$
Hence proved.
Answer:

Draw a line EF passing through point O such that $EO||AB$
Given :
$\frac{AO}{BO} = \frac{CO}{DO}$
In $\triangle ABD$ , we have $AB||EO$
So, by using the basic proportionality theorem,
$\frac{AE}{ED}=\frac{BO}{DO}........................................1$
However, it is given that
$\frac{AO}{CO} = \frac{BO}{DO}..............................2$
Using equations 1 and 2, we get
$\frac{AE}{ED}=\frac{AO}{CO}$
$\Rightarrow EO||CD$ (By basic proportionality theorem)
$\Rightarrow AB||EO||CD$
$\Rightarrow AB||CD$
Therefore, ABCD is a trapezium.
| Triangles Class 10 Question Answers Exercise: 6.3 Page number: 94-97 Total questions: 16 |

Answer:
(i) $\angle A=\angle P=60 ^\circ$
$\angle B=\angle Q=80 ^\circ$
$\angle C=\angle R=40 ^\circ$
$\therefore \triangle ABC \sim \triangle PQR$ (By AAA)
So , $\frac{AB}{QR}=\frac{BC}{RP}=\frac{CA}{PQ}$
(ii) As corresponding sides of both triangles are proportional.
$\therefore \triangle ABC \sim \triangle PQR$ (By SSS)
(iii) Given triangles are not similar because corresponding sides are not proportional.
(iv) $\triangle MNL \sim \triangle PQR$ by SAS similarity criteria.
(v) Given triangles are not similar because the corresponding angle is not contained by two corresponding sides
(vi) In $\triangle DEF$ , we know that
$\angle D+\angle E+\angle F=180 ^\circ$
$\Rightarrow 70 ^\circ+80 ^\circ+\angle F=180 ^\circ$
$\Rightarrow 150 ^\circ+\angle F=180 ^\circ$
$\Rightarrow \angle F=180 ^\circ-150 ^\circ=30 ^\circ$
In $\triangle PQR$ , we know that
$\angle P+\angle Q+\angle R=180 ^\circ$
$\Rightarrow 30^{\circ}+80^{\circ}+\angle R=180^{\circ}$
$\Rightarrow 110 ^\circ+\angle R=180 ^\circ$
$\Rightarrow \angle R=180 ^\circ-110 ^\circ=70 ^\circ$
$\angle Q=\angle P=70 ^\circ$
$\angle E=\angle Q=80^{\circ}$
$\angle F=\angle R=30 ^\circ$
$\therefore \triangle DEF\sim \triangle PQR$ ( By AAA)
Q2: In Fig. 6.35, $\Delta ODC \sim \Delta OBA$ , $\angle BOC = 125 ^\circ$ and $\angle CDO = 70 ^\circ$ . Find $\angle DOC , \angle DCO , \angle OAB$

Answer:
Given : $\Delta ODC \sim \Delta OBA$ , $\angle BOC = 125 ^\circ$ and $\angle CDO = 70 ^\circ$
$\angle DOC+\angle BOC=180 ^{\circ} $ (DOB is a straight line)
$\Rightarrow \angle DOC+125 ^\circ=180 ^\circ$
$\Rightarrow \angle DOC=180 ^\circ-125 ^\circ$
$\Rightarrow \angle DOC=55 ^\circ$
In $\Delta ODC, $
$\angle DOC+\angle ODC+\angle DCO=180 ^\circ$
$\Rightarrow 55 ^\circ+ 70 ^\circ+\angle DCO=180 ^\circ$
$\Rightarrow \angle DCO+125 ^\circ=180 ^\circ$
$\Rightarrow \angle DCO=180 ^\circ-125 ^\circ$
$\Rightarrow \angle DCO=55 ^\circ$
Since, $\Delta ODC \sim \Delta OBA$, so
$\Rightarrow\angle OAB= \angle DCO=55 ^{\circ} $ (Corresponding angles are equal in similar triangles).
Answer:
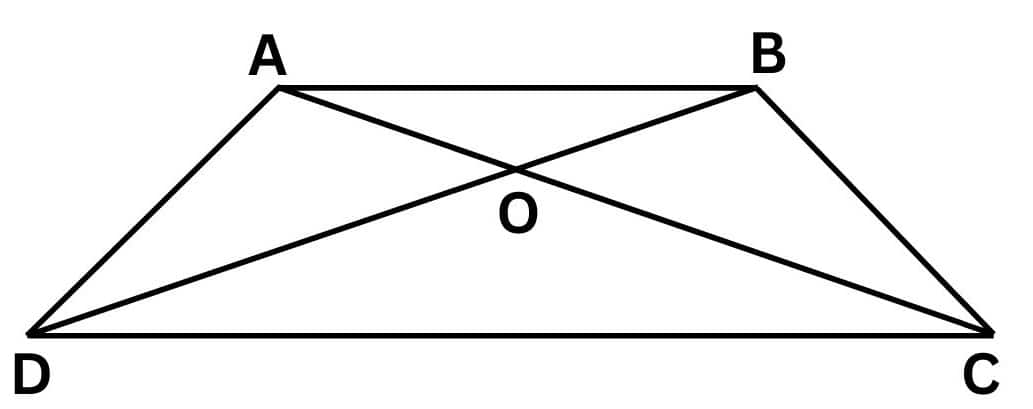
In $\triangle DOC\, and\, \triangle BOA$ , we have
$\angle CDO=\angle ABO$ ( Alternate interior angles as $AB||CD$ )
$\angle DCO=\angle BAO$ ( Alternate interior angles as $AB||CD$ )
$\angle DOC=\angle BOA$ ( Vertically opposite angles are equal)
$\therefore \triangle DOC\, \sim \, \triangle BOA$ ( By AAA)
$\therefore \frac{DO}{BO}=\frac{OC}{OA}$ ( corresponding sides are equal)
$\Rightarrow \frac{OA }{OC} = \frac{OB }{OD }$
Hence proved.

Answer:
Given : $\frac{QR }{QS } = \frac{QT}{PR}$ and $\angle 1 = \angle 2$
To prove : $\Delta PQS \sim \Delta TQR$
In $\triangle PQR$ , $\angle PQR=\angle PRQ$
$\therefore PQ=PR$
$\frac{QR }{QS } = \frac{QT}{PR}$ (Given)
$\Rightarrow \frac{QR }{QS } = \frac{QT}{PQ}$
In $\Delta PQS\, and\, \Delta TQR$,
$\Rightarrow \frac{QR }{QS } = \frac{QT}{PQ}$
$\angle Q=\angle Q$ (Common)
$\Delta PQS \sim \Delta TQR$ (By SAS)
Answer:
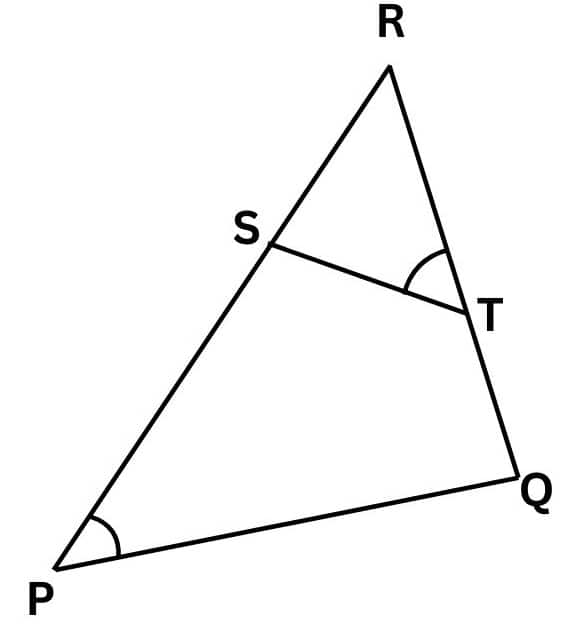
Given : $\angle$ P = $\angle$ RTS
To prove RPQ ~ $\Delta$ RTS.
In $\Delta$ RPQ and $\Delta$ RTS,
$\angle$ P = $\angle$ RTS (Given)
$\angle$ R = $\angle$ R (common)
$\Delta$ RPQ ~ $\Delta$ RTS. (By AA)
Q6: In Fig. 6.37, if $\Delta$ ABE $\equiv$ $\Delta$ ACD, show that $\Delta$ ADE ~ $\Delta$ ABC.

Answer:
Given: $\triangle ABE \cong \triangle ACD$
To prove ADE ~ $\Delta$ ABC.
Since $\triangle ABE \cong \triangle ACD$
$AB=AC$ (By CPCT)
$AD=AE$ (By CPCT)
In $\Delta$ ADE and $\Delta$ ABC,
$\angle A=\angle A$ (Common)
and
$\frac{AD}{AB}=\frac{AE}{AC}$ ( $AB=AC$ and $AD=AE$ )
Therefore, $\Delta$ ADE ~ $\Delta$ ABC. ( By SAS criteria)
Q7 (i): In Fig. 6.38, altitudes AD and CE of $\Delta ABC$ intersect each other at the point P. Show that: $\Delta AEP \sim \Delta CDP$

Answer:
To prove : $\Delta AEP \sim \Delta CDP$
In $\Delta AEP \, \, and\, \, \Delta CDP$ ,
$\angle AEP=\angle CDP$ ( Both angles are right angles)
$\angle APE=\angle CPD$ (Vertically opposite angles )
$\Delta AEP \sim \Delta CDP$ ( By AA criterion)
Q7 (ii): In Fig. 6.38, altitudes AD and CE of $\Delta ABC$ intersect each other at the point P. Show that: $\Delta ABD \sim \Delta CBE$
Answer:
To prove : $\Delta ABD \sim \Delta CBE$
In $\Delta ABD \, \, and\, \, \Delta CBE$ ,
$\angle ADB=\angle CEB$ ( Both angles are right angles)
$\angle ABD=\angle CBE$ (Common )
$\Delta ABD \sim \Delta CBE$ ( By AA criterion)
Q7 (iii): In Fig. 6.38, altitudes AD and CE of $\Delta ABC$ intersect each other at the point P. Show that: $\Delta AEP \sim \Delta ADB$
Answer:
To prove : $\Delta AEP \sim \Delta ADB$
In $\Delta AEP \, \, \, and\, \, \Delta ADB$ ,
$\angle AEP=\angle ADB$ ( Both angles are right angles)
$\angle A=\angle A$ (Common )
$\Delta AEP \sim \Delta ADB$ ( By AA criterion)
Q7 (iv): In Fig. 6.38, altitudes AD and CE of $\Delta ABC$ intersect each other at the point P. Show that: $\Delta PDC \sim \Delta BEC$
Answer:
To prove : $\Delta PDC \sim \Delta BEC$
In $\Delta PDC \, \, and\, \, \, \Delta BEC$ ,
$\angle CDP=\angle CEB$ ( Both angles are right angles)
$\angle C=\angle C$ (Common )
$\Delta PDC \sim \Delta BEC$ ( By AA criterion)
Answer:
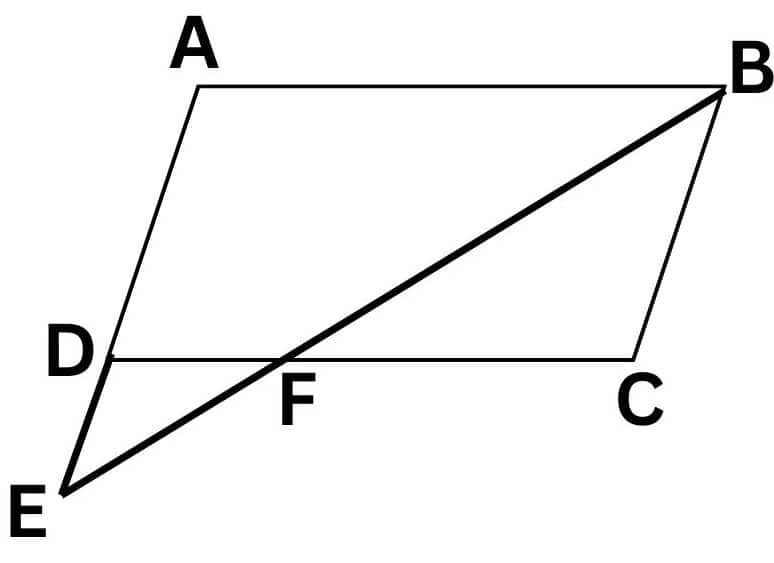
To prove : $\Delta ABE \sim \Delta CFB$
In $\Delta ABE \, \, \, and\, \, \Delta CFB$ ,
$\angle A=\angle C$ ( Opposite angles of a parallelogram are equal)
$\angle AEB=\angle CBF$ ( Alternate angles of AE||BC)
$\Delta ABE \sim \Delta CFB$ ( By AA criterion )
Q9 (i): In Fig. 6.39, ABC and AMP are two right triangles, right-angled at B and M, respectively. Prove that: $\Delta ABC \sim \Delta AMP$

Answer:
To prove : $\Delta ABC \sim \Delta AMP$
In $\Delta ABC \, \, and\, \, \Delta AMP$ ,
$\angle ABC=\angle AMP$ ( Each $90 ^\circ$ )
$\angle A=\angle A$ ( common)
$\Delta ABC \sim \Delta AMP$ ( By AA criterion )
Answer:
To prove :
$\frac{CA }{PA } = \frac{BC }{MP}$
In $\Delta ABC \, \, and\, \, \Delta AMP$ ,
$\angle ABC=\angle AMP$ ( Each $90 ^\circ$ )
$\angle A=\angle A$ ( common)
$\Delta ABC \sim \Delta AMP$ ( By AA criterion )
$\frac{CA }{PA } = \frac{BC }{MP}$ ( corresponding parts of similar triangles )
Hence proved.
Q10 (i): CD and GH are respectively the bisectors of $\angle ACB$ and $\angle EGF$ such that D and H lie on sides AB and FE of $\Delta ABC\: \: and\: \: \Delta EGF$ respectively. If $\Delta ABC \sim \Delta EGF$ , show that: $\frac{CD}{GH} = \frac{AC}{FG}$
Answer:

To prove :
$\frac{CD}{GH} = \frac{AC}{FG}$
Given : $\Delta ABC \sim \Delta EGF$
$\angle A=\angle F,\angle B=\angle E\, \, and \, \, \angle ACB=\angle FGE,\angle ACB=\angle FGE$
$\therefore \angle ACD=\angle FGH$ ( CD and GH are bisectors of equal angles)
$\therefore \angle DCB=\angle HGE$ ( CD and GH are bisectors of equal angles)
In $\Delta ACD \, \, and\, \, \Delta FGH$
$\therefore \angle ACD=\angle FGH$ ( proved above)
$\angle A=\angle F$ ( proved above)
$\Delta ACD \sim \Delta FGH$ ( By AA criterion)
$\Rightarrow \frac{CD}{GH} = \frac{AC}{FG}$
Hence proved.
Q10 (ii): CD and GH are respectively the bisectors of $\angle ABC \: \: and \: \: \angle EGF$ such that D and H lie on sides AB and FE of $\Delta ABC \: \: and \: \: \Delta EGF$ respectively. If $\Delta ABC \sim \Delta EGF$ , show that: $\Delta DCB \sim \Delta HGE$
Answer:
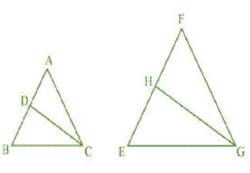
To prove : $\Delta DCB \sim \Delta HGE$
Given : $\Delta ABC \sim \Delta EGF$
In $\Delta DCB \,\, \, and\, \, \Delta HGE$ ,
$\therefore \angle DCB=\angle HGE$ ( CD and GH are bisectors of equal angles)
$\angle B=\angle E$ ( $\Delta ABC \sim \Delta EGF$ )
$\Delta DCB \sim \Delta HGE$ ( By AA criterion )
Q10 (iii): CD and GH are respectively the bisectors of $\angle ABC \: \: and \: \: \angle EGF$ such that D and H lie on sides AB and FE of $\Delta ABC \: \: and \: \: \Delta EGF$ respectively. If $\Delta ABC\sim \Delta EGF$ , show that: $\Delta DCA \sim \Delta HGF$
Answer:

To prove : $\Delta DCA \sim \Delta HGF$
Given : $\Delta ABC \sim \Delta EGF$
In $\Delta DCA \, \, \, and\, \, \Delta HGF$ ,
$\therefore \angle ACD=\angle FGH$ ( CD and GH are bisectors of equal angles)
$\angle A=\angle F$ ( $\Delta ABC \sim \Delta EGF$ )
$\Delta DCA \sim \Delta HGF$ ( By AA criterion )

Answer:
To prove : $\Delta ABD \sim \Delta ECF$
Given: ABC is an isosceles triangle.
$AB=AC \, \, and\, \, \angle B=\angle C$
In $\Delta ABD \, \, and\, \, \Delta ECF$ ,
$\angle ABD=\angle ECF$ ( $\angle ABD=\angle B=\angle C=\angle ECF$ )
$\angle ADB=\angle EFC$ ( Each $90 ^\circ$ )
$\Delta ABD \sim \Delta ECF$ ( By AA criterion)

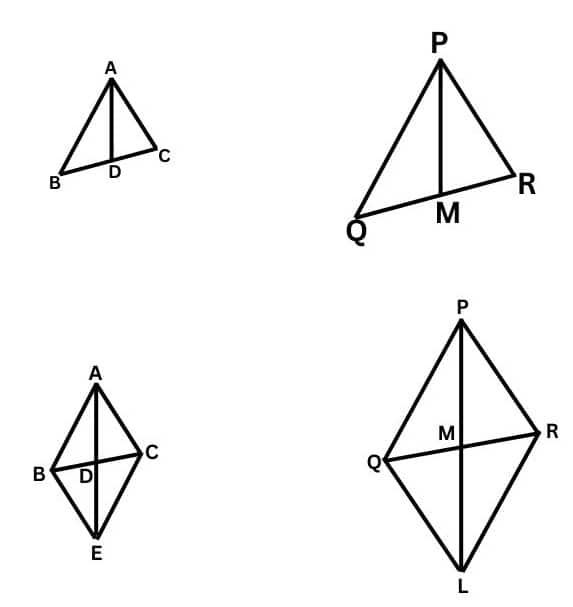
Answer:
AD and PM are medians of triangles. So,
$BD=\frac{BC}{2}\, and\, QM=\frac{QR}{2}$
Given :
$\frac{AB}{PQ}=\frac{BC}{QR}=\frac{AD}{PM}$
$\Rightarrow \frac{AB}{PQ}=\frac{\frac{1}{2}BC}{\frac{1}{2}QR}=\frac{AD}{PM}$
$\Rightarrow \frac{AB}{PQ}=\frac{BD}{QM}=\frac{AD}{PM}$
In $\triangle ABD\, and\, \triangle PQM,$
$\frac{AB}{PQ}=\frac{BD}{QM}=\frac{AD}{PM}$
$\therefore \triangle ABD\sim \triangle PQM,$ (SSS similarity)
$\Rightarrow \angle ABD=\angle PQM$ ( Corresponding angles of similar triangles )
In $\triangle ABC\, and\, \triangle PQR,$
$\Rightarrow \angle ABD=\angle PQM$ (proved above)
$\frac{AB}{PQ}=\frac{BC}{QR}$
Therefore, $\Delta ABC \sim \Delta PQR$ . ( SAS similarity)
Answer:

In, $\triangle ADC \, \, and\, \, \triangle BAC,$
$\angle ADC = \angle BAC$ ( given )
$\angle ACD = \angle BCA$ (common )
$\triangle ADC \, \, \sim \, \, \triangle BAC,$ ( By AA rule)
$\frac{CA}{CB}=\frac{CD}{CA}$ ( corresponding sides of similar triangles )
$\Rightarrow CA^2=CB\times CD$
Answer:
$\frac{AB}{PQ}=\frac{AC}{PR}=\frac{AD}{PM}$ (given)
Produce AD and PM to E and L such that AD=DE and PM=DE. Now,
join B to E, C to E, Q to L and R to L.
AD and PM are medians of a triangle; therefore
QM=MR and BD=DC
AD = DE (By construction)
PM=ML (By construction)
So, the diagonals of ABEC bisect each other at D, so ABEC is a parallelogram.
Similarly, PQLR is also a parallelogram.
Therefore, AC=BE ,AB=EC and PR=QL,PQ=LR
$\frac{AB}{PQ}=\frac{AC}{PR}=\frac{AD}{PM}$ (Given )
$\Rightarrow \frac{AB}{PQ}=\frac{BE}{QL}=\frac{2.AD}{2.PM}$
$\Rightarrow \frac{AB}{PQ}=\frac{BE}{QL}=\frac{AE}{PL}$
$\Delta ABE \sim \Delta PQL$ (SSS similarity)
$\angle BAE=\angle QPL$ ...................1 (Corresponding angles of similar triangles)
Similarity, $\triangle AEC=\triangle PLR$
$\angle CAE=\angle RPL$ ........................2
Adding equations 1 and 2,
$\angle BAE+\angle CAE=\angle QPL+\angle RPL$
$\angle CAB=\angle RPQ$ ............................3
In $\triangle ABC\, and\, \, \triangle PQR,$
$\frac{AB}{PQ}=\frac{AC}{PR}$ ( Given )
$\angle CAB=\angle RPQ$ ( From above equation 3)
$\triangle ABC\sim \triangle PQR$ ( SAS similarity)
Answer:
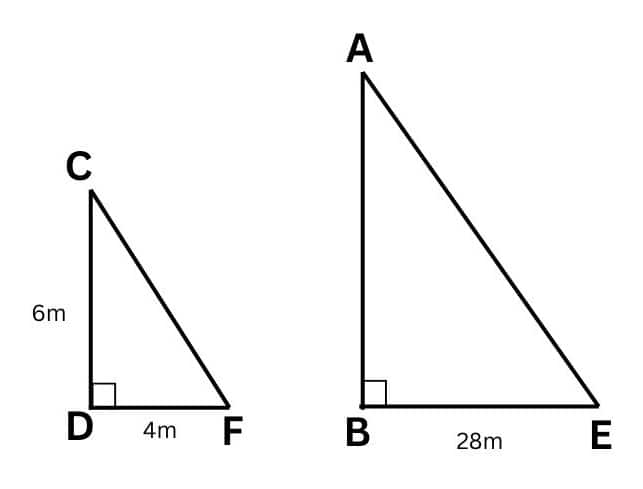
CD = pole
AB = tower
Shadow of pole = DF
Shadow of tower = BE
In $\triangle ABE\, \, and\, \triangle CDF,$
$\angle CDF=\angle ABE$ ( Each $90 ^\circ$ )
$\angle DCF=\angle BAE$ (Angle of sun at the same place )
$\triangle ABE\, \, \sim \, \triangle CDF,$ (AA similarity)
$\frac{AB}{CD}=\frac{BE}{QL}$
$\Rightarrow \frac{AB}{6}=\frac{28}{4}$
$\Rightarrow AB=42$ cm
Hence, the height of the tower is 42 cm.
Answer:
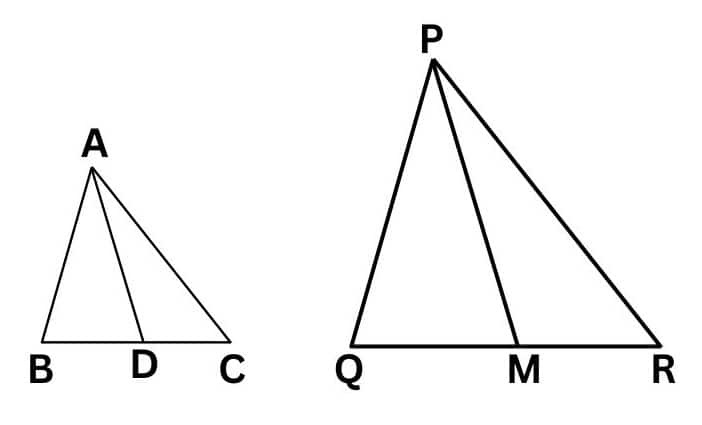
$\Delta AB C \sim \Delta PQR$ ( Given )
$\frac{AB}{PQ}=\frac{AC}{PR}=\frac{BC}{QR}$ ............... ....1( corresponding sides of similar triangles )
$\angle A=\angle P,\angle B=\angle Q,\angle C=\angle R$ ....................................2
AD and PM are medians of a triangle. So,
$BD=\frac{BC}{2}\, and\, QM=\frac{QR}{2}$ ..........................................3
From equations 1 and 3, we have
$\frac{AB}{PQ}=\frac{BD}{QM}$ ...................................................................4
In $\triangle ABD\, and\, \triangle PQM,$
$\angle B=\angle Q$ (From equation 2)
$\frac{AB}{PQ}=\frac{BD}{QM}$ (From equation 4)
$\triangle ABD\, \sim \, \triangle PQM, $ (SAS similarity)
$\frac{AB}{PQ}=\frac{BD}{QM}=\frac{AD}{PM}$
Triangles Class 10 Solutions: Exercise Wise
Exercise-wise NCERT Solutions of Triangles Class 10 Maths Chapter 6 are provided in the link below.
- Class 10 Maths Chapter 6 Exercise 6.1
- Class 10 Maths Chapter 6 Exercise 6.2
- Class 10 Maths Chapter 6 Exercise 6.3
Class 10 Maths NCERT Chapter 6: Extra Question
Question:
In $\triangle XYZ$, points P, Q, and R are points on the sides XY, YZ, and XZ, respectively. $\angle YXZ = 50^\circ$, XR = PR, ZR = QR, and $\angle RQZ = 80^\circ$. What is the value of $\angle PRQ$?
Answer:
In $\triangle XPR$
$\angle PXR = 50^\circ$
Since XR = PR, the triangle is isosceles.
$\angle$XPR = $\angle$PXR = 50$^\circ$
⇒ $\angle XRP = 180^\circ-50^\circ-50^\circ= 80^\circ$
Since ZR = QR, the triangle is isosceles.
Similarly,
$\angle RQZ = \angle RZQ = 80^\circ$
⇒ $\angle QRZ = 180^\circ- 80^\circ- 80^\circ = 20^\circ$
Now, $\angle PRQ = 180^\circ - \angle RQZ - \angle XPR$
$⇒\angle PRQ = 180^\circ-20^\circ- 80^\circ=80^\circ$
Hence, the correct answer is $80^\circ$.
Triangles Class 10 Chapter 6: Topics
Topics you will learn in NCERT Class 10 Maths Chapter 6 Triangles include:
- 6.1 Introduction
- 6.2 Similar Figures
- 6.3 Similarity of Triangles
- 6.4 Criteria for Similarity of Triangles
NCERT Solutions for Class 10 Maths Chapter 2 Triangles: Notes
Types of Triangles:
Based on the sides, we have 3 triangles:
- Equilateral Triangles: Triangles that have all 3 sides equal and all the interior angles equal are known as equilateral triangles.
- Isosceles Triangle: Triangles that have only two sides equal in length also have angles opposite to the equal sides that are equal. It is known as the Isosceles Triangle.
- Scalene Triangle: Triangles that don’t have any equal sides or angles are called scalene triangles.
Based on the measurement of the angle, we have 3 triangles:
- Acute Angle Triangle: Triangles that have all the angles measuring less than 90 degrees are called the Acute Angle Triangle.
- Right Angle Triangle: Triangles that have one angle measuring 90 degrees are called Right Angled Triangles.
- Obtuse Angle Triangle: Triangles that have one angle measuring more than 90 degrees and other angles less than 90 degrees are called Obtuse Angle Triangles.
Similarity Of a Triangle:
Two triangles are similar if they have the same ratio of corresponding sides and an equal pair of corresponding angles.
Criteria for Triangle Similarity:
- Angle-Angle-Angle (AAA) Similarity: If the three angles of one triangle are equal to the three angles of another triangle, the triangles are similar.
- Side-Angle-Side (SAS) Similarity- If one angle of a triangle is equal to one angle of another triangle and the two sides including these angles are in the same ratio, then the triangles are similar.
- Side-Side-Side (SSS) Similarity- If the corresponding sides of two triangles are in the same ratio, then the triangles are similar.
- Right Angle-Hypotenuse-Side(RHS) Similarity- If in two right-angled triangles, the hypotenuse and one corresponding side are in the same ratio, then the two triangles are similar.
Important Theorem's
Theorem 6.1: If a line is drawn parallel to one side of a triangle and it cuts the other two sides at different points, it will divide those two sides in the same ratio.
Theorem 6.2: If a line divides two sides of a triangle in the same ratio, then the line is parallel to the third side of the triangle.
Theorem 6.3: If two triangles have their corresponding angles equal, then their corresponding sides are in the same ratio, which means the two triangles are similar.
Theorem 6.4: If the sides of one triangle are in the same ratio as the sides of another triangle, then their corresponding angles are also equal, which makes the two triangles similar.
Theorem 6.5: If one angle of a triangle is equal to one angle of another triangle and the sides around these angles are in the same ratio, then the two triangles are similar.
NCERT Solutions for Class 10 Maths: Chapter Wise
We at Careers360 compiled all the NCERT class 10 Maths solutions in one place for easy student reference. The following links will allow you to access them.
NCERT Exemplar solutions - Subject-wise
After completing the NCERT textbooks, students should practice exemplar exercises to gain a better understanding of the chapters and improve clarity. The following links will help students find exemplar exercises.
NCERT Books and NCERT Syllabus here
Here are some useful links for NCERT books and the NCERT syllabus for class 10:
Frequently Asked Questions (FAQs)
To prove the Pythagorean theorem using similar triangles, we can draw an altitude from the right angle to the hypotenuse, creating two smaller triangles that are similar to each other and the original triangle, then use the proportional sides of similar triangles to derive the theorem.
Congruent triangles are identical in both shape and size, while similar triangles have the same shape but can differ in size, with corresponding sides being proportional. These concepts are discussed in class 10 maths chapter 6 solutions, practice these to command the concepts.
Similar triangles have various real-life applications, including measuring inaccessible heights or distances, understanding how zoom works on cameras, and in fields like cartography, engineering, and construction.
Thales's Theorem or Basic Proportionality Theorem (BPT) states that if a line is drawn parallel to one side of a triangle intersecting the other two sides in distinct points, then the other two sides are divided in the same ratio.
NCERT Class 10 Maths Chapter 6, "Triangles," focuses on the similarity of triangles, including criteria for similarity (AA, SSS, SAS), and theorems like the Basic Proportionality Theorem and Pythagoras Theorem.
Questions related to CBSE Class 10th
On Question asked by student community
Hrllo,
If you want to view the CM Shri School 2025-26 admission test result, visit the official website http://www.edudel.nic.in/ (http://www.edudel.nic.in/,) ; here, click on the "CM Shri Schools Admission Test - 2025." then select the "CM Shri Admission Test Result 2025 - Merit List" link. Here you need to log in with your credentials and view or download the merit list pdf.
I hope it will clear your query!!
Hello aspirant,
Fairness and equal opportunity are guaranteed by the rigorous merit-based admissions process at CM Shri Schools. The merit list will be made public on edudel.nic.in, the official DOE website. Based on how well they did on the admission exam, students will be shortlisted.
To know the result, you can visit our site through following link:
https://school.careers360.com/articles/cm-shri-school-admission-test-result-2025
Thank you
Hello
-
The CM Shri School Admission Test was conducted for students seeking quality education.
-
The result and selection list are expected to be released around 20 September 2025.
-
Students who qualified will get admission into CM Shri Model Schools across the state.
-
The official list of selected students will be available on the edudel.nic.in website.
-
Candidates can check their names in the merit list PDF once uploaded.
-
Selection is based on the student’s performance in the entrance exam.
-
Keep checking the official site or the school notice board for timely updates.
You can check CM Shri school result here: https://school.careers360.com/articles/cm-shri-school-admission-test-result-2025 Thank You.
Hello! If you are looking for the CM Shree School result for 2025, here is the link provided by Careers360 where you can check your result easily. I’ll be attaching it for your reference so you can access it directly.
https://school.careers360.com/articles/cm-shri-school-admission-test-result-2025
Popular CBSE Class 10th Questions
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters

