NCERT Solutions for Class 10 Maths Chapter 10 Circles

Edited By Ramraj Saini | Updated on Sep 07, 2023 05:18 PM IST | #CBSE Class 10th

NCERT Solutions For Class 10 Maths Chapter 10 Circles

NCERT Solutions for Class 10 Maths Chapter 10 Circles provided here. The ncert solutions have been carefully created by Maths experts at Careers360 keeping in mind of latest syllabus and pattern of CBSE 2023-24. Circles class 10 solutions are a vital resource for students preparing for the Class 10 board exams. These solutions provide step-by-step, comprehensive and detailed answers for all questions in the NCERT Class 10 Maths textbook and are arranged in an exercise-wise format to aid students' preparation and understanding. It is advisable to complete the NCERT Class 10 Maths syllabus at the earliest. To achieve high marks on the exam, students are encouraged to use these NCERT Solutions chapter 10 maths class 10 as part of their study materials. They are free to download using the link given below in this page.

Scholarship Test: Vidyamandir Intellect Quest (VIQ)

Don't Miss: JEE Main & NEET 2026 Scholarship Test (Class 10): Narayana | Aakash

This Story also Contains

NCERT Solutions For Class 10 Maths Chapter 10 Circles
NCERT Solutions for Class 10 Maths Chapter 10 Circles Free PDF Download
NCERT Solutions for Class 10 Maths Chapter 10 Circles - Important Points
NCERT Solutions for Class 10 Maths Chapter 10 Circles (Intext Questions and Exercise)
Circles Class 10 NCERT Topics
Circles Class 10 Solutions - Exercise Wise
More About Circles Class 10 NCERT Solutions
NCERT solutions for class 10 Maths - Chapter Wise
NCERT Exemplar solutions - Subject wise
NCERT Solutions for Class 10 - Subject Wise
NCERT Books and NCERT Syllabus

NCERT Solutions for Class 10 Maths Chapter 10 Circles

Also See

NCERT Solutions for Class 10 Maths Chapter 10 Circles Free PDF Download

Download PDF

NCERT Solutions for Class 10 Maths Chapter 10 Circles - Important Points

When a circle with equation x² + y² = a² is intersected by a line y = mx + c, the equation of Tangent Line to Circle: y = mx ± a √(1 + m²)

Relationship between Tangent and Radius - The tangent to a circle is perpendicular to the radius through the point of contact.

Length of Tangent from External Point: 2√(r² - d²)

Where r is the circle's radius and d is the distance from the external point to the circle's center.

Free download NCERT Solutions for Class 10 Maths Chapter 10 Circles PDF for CBSE Exam.

NCERT Solutions for Class 10 Maths Chapter 10 Circles (Intext Questions and Exercise)

Circles class 10 NCERT solutions Excercise: 10.1

Q1 How many tangents can a circle have?

Answer:

The lines that intersect the circle exactly at one single point are called tangents. In a circle, there can be infinitely many tangents.

1594292623722

Q2 Fill in the blanks :
(1) A tangent to a circle intersects it in_________ point (s).
(2) A line intersecting a circle in two points is called a _____.
(3) A circle can have ________ parallel tangents at the most.
(4) The common point of a tangent to a circle and the circle ______

Answer:

(a) one
A tangent of a circle intersects the circle exactly in one single point.

(b) secant
It is a line that intersects the circle at two points.

(d) point of contact
The common point of a tangent and a circle.

Q3 A tangent PQ at a point P of a circle of radius 5 cm meets a line through the center O at a point Q so that OQ = 12 cm. Length PQ is :

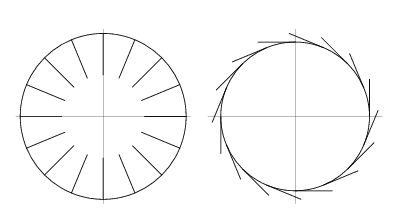
(A) 12 cm

(B) 13 cm

(D) $\sqrt { 119}$ cm.

Answer:

The correct option is (d) = $\sqrt { 119}$ cm

1594292996735
It is given that the radius of the circle is 5 cm. OQ = 12 cm
According to question,
We know that $\angle QPO=90^0$
So, triangle OPQ is a right-angle triangle. By using Pythagoras theorem,
$PQ =\sqrt{OQ^2-OP^2}=\sqrt{144-25}$
$=\sqrt{119}$ cm

Q4 Draw a circle and two lines parallel to a given line such that one is a tangent and the other, a secant to the circle.

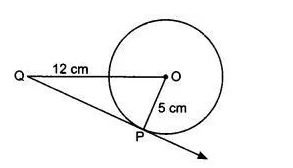
Answer:

1594293094431
AB is the given line and the line CD is the tangent to a circle at point M and parallels to the line AB. The line EF is a secant parallel to the AB

NCERT solutions circles class 10 Excercise: 10.2

Q1 From a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the center is 25 cm. The radius of the circle is

(A) 7 cm

(B) 12 cm

(D) 24.5 cm

Answer:

The correct option is (A) = 7 cm

Given that,
The length of the tangent (QT) is 24 cm and the length of OQ is 25 cm.
Suppose the length of the radius OT be $l$ cm.
We know that $\Delta OTQ$ is a right angle triangle. So, by using Pythagoras theorem-

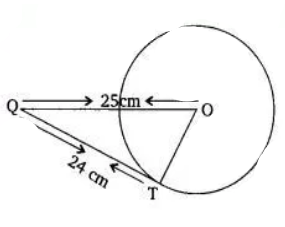
$\\OQ^2 = TQ^2+OT^2\\\\ l = \sqrt{25^2-24^2}\\\\OT = l=\sqrt{49}$ 1648791076455 OT = 7 cm

Q2 In Fig. 10.11, if TP and TQ are the two tangents to a circle with center O so that $\angle$ POQ = $11 0 \degree$ , then $\angle$ PTQ is equal to

1648791138639

(A) $60 \degree$

(B) $70 \degree$

(D) $9 0 \degree$

Answer:

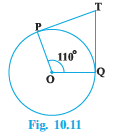
The correct option is (b)

1594293296951
In figure, $\angle POQ = 110^0$
Since POQT is quadrilateral. Therefore the sum of the opposite angles are 180

$\\\Rightarrow \angle PTQ +\angle POQ = 180^0\\\\\Rightarrow \angle PTQ = 180^0-\angle POQ$
$= 180^0-100^0$
$= 70^0$

Q3 If tangents PA and PB from a point P to a circle with center O are inclined to each other at an angle of $80 \degree$ , then $\angle$ POA is equal to

(A) 50°

(B) 60°

(D) 80°

Answer:

The correct option is (A)
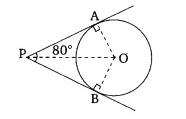
1594293381602
It is given that, tangent PA and PB from point P inclined at $\angle APB = 80^0$
In triangle $\Delta$ OAP and $\Delta$ OBP
$\angle OAP = \angle OBP = 90$
OA =OB (radii of the circle)
PA = PB (tangents of the circle)

Therefore, by SAS congruence
$\therefore \Delta OAP \cong \Delta OBP$

By CPCT, $\angle OPA = \angle OPB$
Now, $\angle$ OPA = 80/4 = 40

In $\Delta$ PAO,
$\angle P + \angle A + \angle O = 180$
$\angle O = 180 - 130$
= 50

Q4 Prove that the tangents drawn at the ends of a diameter of a circle are parallel.

Answer:

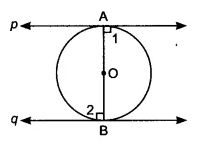
1594293543765
Let line $p$ and line $q$ are two tangents of a circle and AB is the diameter of the circle.
OA and OB are perpendicular to the tangents $p$ and $q$ respectively.
therefore,
$\angle1 = \angle2 = 90^0$

$\Rightarrow$ $p$ || $q$ { $\angle$ $\because$ 1 & $\angle$ 2 are alternate angles}

Q5 Prove that the perpendicular at the point of contact to the tangent to a circle passes through the center.

Answer:

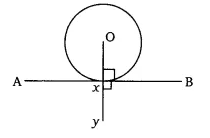
1594293656560
In the above figure, the line AXB is the tangent to a circle with center O. Here, OX is the perpendicular to the tangent AXB ( $OX\perp AXB$ ) at point of contact X.
Therefore, we have,
$\angle$ BXO + $\angle$ YXB = $90^0+90^0=180^0$

$\therefore$ OXY is a collinear
$\Rightarrow$ OX is passing through the center of the circle.

Q6 The length of a tangent from a point A at distance 5 cm from the center of the circle is 4 cm. Find the radius of the circle.

Answer:

1594293766356
Given that,
the length of the tangent from the point A (AP) is 4 cm and the length of OA is 5 cm.
Since $\angle$ APO = 90 ⁰
Therefore, $\Delta$ APO is a right-angle triangle. By using Pythagoras theorem;

$OA^2=AP^2+OP^2$
$5^2 = 4^2+OP^2$
$OP=\sqrt{25-16}=\sqrt{9}$
$OP = 3 cm$

Q7 Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.

Answer:

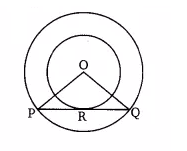
1594293901543
In the above figure, Pq is the chord to the larger circle, which is also tangent to a smaller circle at the point of contact R.
We have,
radius of the larger circle OP = OQ = 5 cm
radius of the small circle (OR) = 3 cm

OR $\perp$ PQ [since PQ is tangent to a smaller circle]

According to question,

In $\Delta$ OPR and $\Delta$ OQR
$\angle$ PRO = $\angle$ QRO {both $90^0$ }

OR = OR {common}
OP = OQ {both radii}

By RHS congruence $\Delta$ OPR $\cong$ $\Delta$ OQR
So, by CPCT
PR = RQ
Now, In $\Delta$ OPR,
by using pythagoras theorem,
$PR = \sqrt{25-9} =\sqrt{16}$
PR = 4 cm
Hence, PQ = 2.PR = 8 cm

Q8 A quadrilateral ABCD is drawn to circumscribe a circle (see Fig. 10.12). Prove that AB + CD = AD + BC

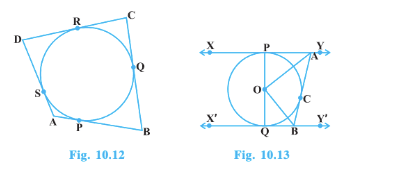
1594294016205

Answer:

1594294152195
To prove- AB + CD = AD + BC
Proof-
We have,
Since the length of the tangents drawn from an external point to a circle are equal
AP =AS .......(i)
BP = BQ.........(ii)
AS = AP...........(iii)
CR = CQ ...........(iv)

By adding all the equations, we get;

AP + BP +RD+ CR = AS +DS +BQ +CQ
$\Rightarrow$ (AP + BP) + (RD + CR) = (AS+DS)+(BQ + CQ)
$\Rightarrow$ AB + CD = AD + BC

Hence proved.

Q9 In Fig. 10.13, XY and X'Y'are two parallel tangents to a circle with center O and another tangent AB with a point of contact C intersecting XY at A and X'Y' at B. Prove that $\angle$ AOB = 90°.

Answer:

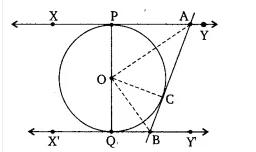
1594294251028
To prove- $\angle$ AOB = $90^0$
Proof-
In $\Delta$ AOP and $\Delta$ AOC,
OA =OA [Common]
OP = OC [Both radii]
AP =AC [tangents from external point A]
Therefore by SSS congruence, $\Delta$ AOP $\cong$ $\Delta$ AOC
and by CPCT, $\angle$ PAO = $\angle$ OAC
$\Rightarrow \angle PAC = 2\angle OAC$ ..................(i)

Similarly, from $\Delta$ OBC and $\Delta$ OBQ, we get;
$\angle$ QBC = 2. $\angle$ OBC.............(ii)

Adding eq (1) and eq (2)

$\angle$ PAC + $\angle$ QBC = 180
2( $\angle$ OBC + $\angle$ OAC) = 180
( $\angle$ OBC + $\angle$ OAC) = 90

Now, in $\Delta$ OAB,
Sum of interior angle is 180.
So, $\angle$ OBC + $\angle$ OAC + $\angle$ AOB = 180
$\therefore$ $\angle$ AOB = 90
hence proved.

Q10 Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the center.

Answer:

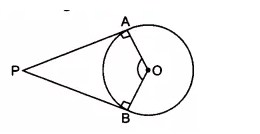
1594294380467
To prove - $\angle APB + \angle AOB = 180^0$
Proof-
We have, PA and PB are two tangents, B and A are the point of contacts of the tangent to a circle. And $OA\perp PA$ , $OB\perp PB$ (since tangents and radius are perpendiculars)

According to question,
In quadrilateral PAOB,
$\angle$ OAP + $\angle$ APB + $\angle$ PBO + $\angle$ BOA = $360^0$
90 + $\angle$ APB + 90 + $\angle$ BOA = 360
$\angle APB + \angle AOB = 180^0$
Hence proved .

Q11 Prove that the parallelogram circumscribing a circle is a rhombus.

Answer:

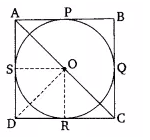
1594294663376
To prove - the parallelogram circumscribing a circle is a rhombus
Proof-
ABCD is a parallelogram that circumscribes a circle with center O.
P, Q, R, S are the points of contacts on sides AB, BC, CD, and DA respectively
AB = CD .and AD = BC...........(i)
It is known that tangents drawn from an external point are equal in length.
RD = DS ...........(ii)
RC = QC...........(iii)
BP = BQ...........(iv)
AP = AS .............(v)
By adding eq (ii) to eq (v) we get;
(RD + RC) + (BP + AP) = (DS + AS) + (BQ + QC)
CD + AB = AD + BC
$\Rightarrow$ 2AB = 2AD [from equation (i)]
$\Rightarrow$ AB = AD
Now, AB = AD and AB = CD
$\therefore$ AB = AD = CD = BC

Hence ABCD is a rhombus.

Q12 A triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 8 cm and 6 cm respectively (see Fig. 10.14). Find the sides AB and AC.

Answer:

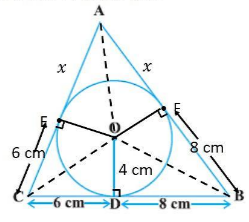
1639543596459

Consider the above figure. Assume center O touches the sides AB and AC of the triangle at point E and F respectively.

Let the length of AE is x.

Now in $\bigtriangleup ABC$ ,

$CF = CD = 6$ (tangents on the circle from point C)

$BE = BD = 6$ (tangents on the circle from point B)

$AE = AF = x$ (tangents on the circle from point A)

Now AB = AE + EB

$\\AB = AE + EB\\\\ => AB = x + 8\\\\ BC = BD + DC\\\\ => BC = 8+6 = 14\\\\ CA = CF + FA\\\\ => CA = 6 + x\\\\$

Now
$\\s = (AB + BC + CA )/2\\\\ => s = (x + 8 + 14 + 6 +x)/2\\\\ => s = (2x + 28)/2\\\\ => s = x + 14$

Area of triangle $\bigtriangleup ABC$

$\\=\sqrt{s*(s-a)*(s-b)*(s-c)}\\\\=\sqrt{(14+x)*[(14+x)-14]*[(14+x)-(6+x)]*[(14+x)-(8+x)]}\\\\=4\sqrt{3(14x+x^2)}$
Now the area of $\bigtriangleup OBC$

$\\= (1/2)*OD*BC\\\\ = (1/2)*4*14\\\\ = 56/2 = 28$
Area of $\bigtriangleup OCA$

$\\= (1/2)*OF*AC \\\\= (1/2)*4*(6+x) \\\\= 2(6+x) \\\\= 12 + 2x$

Area of $\bigtriangleup OAB$

$\\= (1/2)*OE*AB \\\\= (1/2)*4*(8+x) \\\\= 2(8+x) \\\\= 16 + 2x$

Now Area of the $\bigtriangleup ABC$ = Area of $\bigtriangleup OBC$ + Area of $\bigtriangleup OCA$ + Area of $\bigtriangleup OAB$

$\\=> 4\sqrt{3x(14+x)}= 28 + 12 + 2x + 16 + 2x \\\\=> 4\sqrt{3x(14+x)} = 56 + 4x \\\\=> 4\sqrt{3x(14+x)} = 4(14 + x) \\\\=> \sqrt{3x(14+x)}] = 14 + x$

On squaring both the side, we get

$\\3x(14 + x) = (14 + x)^2\\\\ => 3x = 14 + x \:\:\:\:\:\: (14 + x = 0 => x = -14\: is\: not\: possible) \\\\=> 3x - x = 14\\\\ => 2x = 14\\\\ => x = 14/2\\\\ => x = 7$

Hence

AB = x + 8

=> AB = 7+8

=> AB = 15

AC = 6 + x

=> AC = 6 + 7

=> AC = 13

Answer- AB = 15 and AC = 13

Q13 Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the center of the circle.

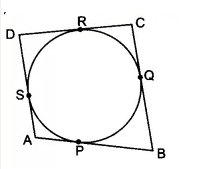
Answer:

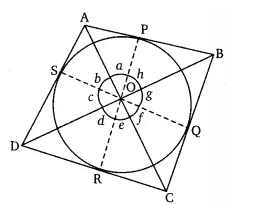
1594294831132
Given- ABCD is a quadrilateral circumscribing a circle. P, Q, R, S are the point of contact on sides AB, BC, CD, and DA respectively.

To prove-
$\\\angle AOB + \angle COD =180^0\\ \angle AOD + \angle BOC =180^0$
Proof -
Join OP, OQ, OR and OS
In triangle $\Delta$ DOS and $\Delta$ DOR,
OD =OD [common]
OS = OR [radii of same circle]
DR = DS [length of tangents drawn from an external point are equal ]
By SSS congruency, $\Delta$ DOS $\cong$ $\Delta$ DOR,
and by CPCT, $\angle$ DOS = $\angle$ DOR
$\angle c = \angle d$ .............(i)

Similarily,
$\\\angle a = \angle b\\ \angle e = \angle f\\ \angle g =\angle h$ ...............(2, 3, 4)

$\therefore 2(\angle a +\angle e +\angle h+\angle d) = 360^0$
$\\(\angle a +\angle e) +(\angle h+\angle d) = 180^0\\ \angle AOB + \angle DOC = 180^0$
SImilarily, $\angle AOD + \angle BOC = 180^0$

Hence proved.

Circles Class 10 NCERT Topics

Tangent to a Circle
Radius and Tangent Relation
Tangent From External Point to the Circle

VMC VIQ Scholarship Test

Apply

Pearson | PTE

Register now for PTE & Unlock 20% OFF : Use promo code: 'C360SPL20'. Valid till 30th NOV'24! Trusted by 3,500+ universities globally

Apply

Circles Class 10 Solutions - Exercise Wise

NCERT solutions for class 10 Maths - Chapter Wise

Chapter No.	Chapter Name
Chapter 1	Real Numbers
Chapter 2	Polynomials
Chapter 3	Pair of Linear Equations in Two Variables
Chapter 4	Quadratic Equations
Chapter 5	Arithmetic Progressions
Chapter 6	Triangles
Chapter 7	Coordinate Geometry
Chapter 8	Introduction to Trigonometry
Chapter 9	Some Applications of Trigonometry
Chapter 10	Circles
Chapter 11	Constructions
Chapter 12	Areas Related to Circles
Chapter 13	Surface Areas and Volumes
Chapter 14	Statistics
Chapter 15	Probability

NCERT Exemplar solutions - Subject wise

How To Use NCERT Solutions For Class 10 Maths Chapter 10 Circles?

Circle is the chapter, the logic and the memory both are tested equally. First, you should learn the theorems, terminologies, and concepts of circles.
Once you have done the theorems, go through some examples of the NCERT textbook.
When you have done the above-said points, then solve the practice exercises of Circles Class 10 where your understanding of concepts will be tested.
While solving the practice exercises, you can take the help of NCERT solutions for Class 10 Maths hapter 10 circles.
Lastly, solve the previous years question papers related to the particular chapter.

NCERT Solutions for Class 10 - Subject Wise

Key Features of NCERT Solutions for Class 10 Maths Chapter 10 Circles

The NCERT Solutions for class 10 maths chapter 10 Circles include several key features that help students to improve their understanding and practice of the material.

These circle class 10 solutions include answers for all exercise questions in the NCERT Class 10 Maths textbook.
These solutions help students to practice challenging questions, align with the updated CBSE syllabus and cover possible exam questions, provide guidance for important drawings.
These solutions aid students in memorizing important formulas and calculation methods.

NCERT Books and NCERT Syllabus

Happy study!

Frequently Asked Questions (FAQs)

1. What is the weightage of chapter Circles in CBSE 10 board final exam ?

Triangles, circles and constructions combined have 15 marks weightage in Class 10 final board exam. To obtain a good score in the CBSE 10th board exam follow NCERT book and the previous board papers. More questions can be practiced from NCERT exemplar.

2. What are the main concepts covered in NCERT Solutions for class 10 maths circles?

The key concepts covered in NCERT Solutions for circle chapter class 10 include the introduction to circles, the properties of tangents to a circle, the calculation of the number of tangents from a point on a circle, and a summary of the entire chapter. Students can also use chapter 10 class 10 maths solutions in PDF format.

3. How is the NCERT solution helpful in the CBSE board exams?

As most of the questions in CBSE board exam are directly asked from NCERT textbook, so must solve all the problems given in the NCERT textbook. NCERT solutions are not only important when you are stuck while solving the problems but you will get to know how to answer in the board exam in order to get good marks in the board exam.

4. Can you list the key features of circles class 10 ncert solutions?

The NCERT Solutions for chapter 10 class 10 maths includes answers to all exercise questions in the NCERT Class 10 Maths textbook. Additionally, they provide guidance for practicing challenging questions, which will enhance students' comprehension of the topics covered in the chapter.

5. Is it mandatory to study all the questions in NCERT class 10 maths chapter 10 solutions?

It is essential to study all the questions provided in NCERT Solutions for Class 10 Maths Chapter 10 as they may be present in CBSE board exams and class tests. By familiarizing yourself with these questions, you will be well-prepared for your upcoming exams. In NCERT Solutions, class 10 Maths chapter 10 question answer are comprehensively discussed. Therefore students can use these recourses to score well in the exam.

Also Read

The Human Eye and The Colorful world Class 10th Notes- Free NCERT Class 10 Science Chapter 11 Notes - Download PDF

NCERT Class 10 Science Chapter 14 Notes Sources Of Energy - Download PDF

Magnetic Effects of Electric Current Class 10th Notes - Free NCERT Class 10 Science Chapter 13 Notes - Download PDF

Electricity Class 10th Notes - Free NCERT Class 10 Science Chapter 12 Notes - Download PDF

Light- Reflection and Refraction Class 10th Notes- Free NCERT Class 10 Science Chapter 10 Notes - Download PDF

Free NCERT Class 10 Maths Chapter 2 Notes - Download PDF

NCERT Syllabus for Class 10 Maths 2024-25 - Download Free PDF

NCERT Solutions for Exercise 12.3 Class 10 Maths Chapter 12 - Areas related to circles

NCERT Solutions for Exercise 12.2 Class 10 Maths Chapter 12 - Areas related to circles

NCERT Solutions for Exercise 12.1 Class 10 Maths Chapter 12 - Areas related to circles

NCERT Solutions for Class 10 Science Chapter 6 Life Processes

NCERT Solutions For Class 10 Maths Chapter 4 Quadratic Equations

NCERT Exemplar Class 10 Maths Solutions - Chapter Wise Problems with Solutions

NCERT Exemplar Class 10 Science Solutions Chapter 1 Chemical Reactions and Equations

NCERT Exemplar Class 10 Science Solutions - Chapter Wise Problems with Solutions

Articles

NMMS West Bengal 2024-25: Application, Last Date, Eligibility, Exam Date, Admit Card

Nov 20, 2024

Maharashtra SSC Time Table 2025, Check MSBSHSE Class 10 Exam Dates @mahahsscboard.in

Nov 20, 2024

NMMS Delhi 2024-25: Application, Exam Date, Admit Card, Answer Key, Result

Nov 20, 2024

NMMS Gujarat 2024-25: Application Form, Eligibility, Syllabus, Exam Date

Nov 20, 2024

NMMS Haryana 2024-25: Admit Card (OUT), Exam Date, Answer Key, Result

Nov 20, 2024

CBSE Date Sheet 2025 Out Soon; Class 10, 12 Theory Exams Starts on February 15

Nov 20, 2024

CBSE Class 10 Date Sheet 2025 @cbse.gov.in (Soon); Download CBSE 10th Exam Time Table

Nov 17, 2024

CBSE Syllabus 2024-25 PDF (Released) -Download CBSE Syllabus for Class 9th, 10th, 11th, 12th PDF Here

Nov 14, 2024

Upcoming School Exams

National Institute of Open Schooling 12th Examination

Admit Card Date:04 October,2024 - 29 November,2024

Application Process

Exam Pattern

Admit Card

National Institute of Open Schooling 10th examination

Admit Card Date:04 October,2024 - 29 November,2024

Application Process

Exam Pattern

Mock Test

Mizoram Board of School Education 12th Examination

Application Date:07 October,2024 - 22 November,2024

Exam Pattern

Result

Mock Test

Mizoram Board 10th Examination

Application Date:07 October,2024 - 22 November,2024

Exam Pattern

Admit Card

Result

Punjab Board of Secondary Education 10th Examination

Application Correction Date:08 October,2024 - 27 November,2024

Exam Pattern

Result

Admit Card

View All School Exams

Certifications By Top Providers

Full-Stack Web Development

Via Masai School

Digital Banking Business Model

Via State Bank of India

Business Analytics Foundations

Via PW Skills

Master Data Management for Beginners

Via TCS iON Digital Learning Hub

Data Analytics Basics for Everyone

Via IBM

Banking 101 A Guide for Beginners in the Banking Sector

Via EduBridge

1113 courses

812 courses

394 courses

295 courses

Harvard University, Cambridge

98 courses

IBM

83 courses

IT & Software

Teaching and Academics

Programming and Development

Health, Fitness and Medicine

Business and Management

Engineering and Architecture

General Management

Financial Management

Environmental Studies

History

Artificial Intelligence

Psychology

Explore Top Universities Across Globe

University of Essex, Colchester

Wivenhoe Park Colchester CO4 3SQ

University College London, London

Gower Street, London, WC1E 6BT

The University of Edinburgh, Edinburgh

Old College, South Bridge, Edinburgh, Post Code EH8 9YL

University of Bristol, Bristol

Beacon House, Queens Road, Bristol, BS8 1QU

University of Delaware, Newark

Newark, DE 19716

University of Nottingham, Nottingham

University Park, Nottingham NG7 2RD

PR in UK After Study for International and Indian Students 2025

9 min ⋆ Nov 14, 2024 13:11 PM IST

Duolingo English Test Accepting Universities and Countries in 2025-26

12 min ⋆ Nov 14, 2024 13:11 PM IST

Top MBA Colleges in Dubai 2025 (with Tuition fees): List of Best Colleges

11 min ⋆ Nov 14, 2024 13:11 PM IST

Cheapest Universities in Germany for International Students 2025-26

15 min ⋆ Nov 14, 2024 13:11 PM IST

Cheapest Universities in Melbourne 2025: Discover Affordable Education

7 min ⋆ Nov 14, 2024 13:11 PM IST

Top Courses to Study in USA for Indian and International Students 2025

11 min ⋆ Nov 14, 2024 13:11 PM IST

Related E-books & Sample Papers

CBSE Class 10 Social Science Sample Paper with Solution 2024-25 PDF

395+ Downloads

CBSE Class 10 Mathematics (Standard) Sample Paper with Solution 2024-25 PDF

257+ Downloads

CBSE Class 10 Mathematics (Basic) Sample Paper with Solution 2024-25 PDF

114+ Downloads

CBSE Class 10 Hindi B Sample Paper with Solution 2024-25 PDF

39+ Downloads

CBSE Class 10 Hindi A Sample Paper with Solution 2024-25 PDF

27+ Downloads

CBSE Class 10 English (Language & Literature) Sample Paper with Solution 2024-25 PDF

45+ Downloads

Questions related to CBSE Class 10th

Have a question related to CBSE Class 10th ?

sadhu ashram ramgart road aligarh

If you're looking for directions or steps to reach Sadhu Ashram on Ramgart Road in Aligarh, here’s how you can get there:

Steps to Reach Sadhu Ashram, Ramgart Road, Aligarh:

Starting Point:
- Determine your starting point in Aligarh or the nearby area.
Use Google Maps:
- Open Google Maps on your phone or computer.
- Enter "Sadhu Ashram, Ramgart Road, Aligarh" as your destination.
- Follow the navigation instructions provided by Google Maps.
By Local Transport:
- Auto-rickshaw/Taxi: Hire an auto-rickshaw or taxi and inform the driver about your destination. Most local drivers should be familiar with Sadhu Ashram.
- Bus: Check if there are any local buses that operate on Ramgart Road. Ask the bus conductor if the bus stops near Sadhu Ashram.
Landmarks to Look For:
- As you approach Ramgart Road, look for local landmarks that might help you confirm you’re on the right path, such as known shops, temples, or schools nearby.
Ask for Directions:
- If you're unsure, ask locals for directions to Sadhu Ashram on Ramgart Road. It's a known location in the area.
Final Destination:
- Once you reach Ramgart Road, Sadhu Ashram should be easy to spot. Look for any signage or ask nearby people to guide you to the exact location.

If you need detailed directions from a specific location or more information about Sadhu Ashram, feel free to ask

Read Complete Answer

sai raja 01 September,2024

show the previous year exams ?

Hello Aspirant, Hope your doing great, your question was incomplete and regarding what exam your asking.

Read Complete Answer

SWETCHCHA MISKA 10 July,2024

as an icse student in class 10 is above 80 percent good enough to get into commerce in 11th cbse

Yes, scoring above 80% in ICSE Class 10 exams typically meets the requirements to get into the Commerce stream in Class 11th under the CBSE board . Admission criteria can vary between schools, so it is advisable to check the specific requirements of the intended CBSE school. Generally, a good academic record with a score above 80% in ICSE 10th result is considered strong for such transitions.

Read Complete Answer

Manasvini Gupta 23 July,2024

i had cleared 10th from cbse in 2022 2 years ago now i wish to apply for 12th as private candidate in 2025. can i do so?

hello Zaid,

Yes, you can apply for 12th grade as a private candidate .You will need to follow the registration process and fulfill the eligibility criteria set by CBSE for private candidates.If you haven't given the 11th grade exam ,you would be able to appear for the 12th exam directly without having passed 11th grade. you will need to give certain tests in the school you are getting addmission to prove your eligibilty.

best of luck!

Read Complete Answer

Ashvadha 03 July,2024

i had cleared 10th in 2022 from cbse can i apply for 12th as private candidate this year?

According to cbse norms candidates who have completed class 10th, class 11th, have a gap year or have failed class 12th can appear for admission in 12th class.for admission in cbse board you need to clear your 11th class first and you must have studied from CBSE board or any other recognized and equivalent board/school.

You are not eligible for cbse board but you can still do 12th from nios which allow candidates to take admission in 12th class as a private student without completing 11th.

Read Complete Answer

sadafreen356 03 July,2024

View All

Stay up-to date with CBSE Class 10th News

Download Careers360 App

All this at the convenience of your phone

Regular Exam Updates
Best College Recommendations
College & Rank predictors
Detailed Books and Sample Papers
Question and Answers

Scan and download the app

Central Board of Secondary Education Class 10th Examination

Applications Open

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

Option 1) less than 3	Option 2) more than 3 but less than 6
Option 3) more than 6 but less than 9	Option 4) more than 9

NCERT Solutions for Class 10 Maths Chapter 10 Circles

NCERT Solutions For Class 10 Maths Chapter 10 Circles

NCERT Solutions for Class 10 Maths Chapter 10 Circles Free PDF Download

NCERT Solutions for Class 10 Maths Chapter 10 Circles - Important Points

NCERT Solutions for Class 10 Maths Chapter 10 Circles (Intext Questions and Exercise)

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Circles Class 10 NCERT Topics

VMC VIQ Scholarship Test

Pearson | PTE

Circles Class 10 Solutions - Exercise Wise

More About Circles Class 10 NCERT Solutions

NCERT solutions for class 10 Maths - Chapter Wise

NCERT Exemplar solutions - Subject wise

How To Use NCERT Solutions For Class 10 Maths Chapter 10 Circles?

NCERT Solutions for Class 10 - Subject Wise

Key Features of NCERT Solutions for Class 10 Maths Chapter 10 Circles

NCERT Books and NCERT Syllabus

Frequently Asked Questions (FAQs)

Also Read

Articles

Upcoming School Exams

Certifications By Top Providers

Explore Top Universities Across Globe

Related E-books & Sample Papers

Questions related to CBSE Class 10th

Steps to Reach Sadhu Ashram, Ramgart Road, Aligarh:

Popular CBSE Class 10th Questions

Colleges After 12th

VMC VIQ Scholarship Test

JEE Main Important Physics formulas

JEE Main Important Chemistry formulas

TOEFL ® Registrations 2024

Pearson | PTE

JEE Main high scoring chapters and topics

Stay up-to date with CBSE Class 10th News

Explore on Careers360

NCERT Solutions

NCERT Exemplars

NCERT Books

NCERT Notes

CBSE

CBSE Class 10

CBSE Class 12th

CISCE Board 10th

IMO

IEO

NSO

NMMS

NVS

JNVST

Schools By Medium

By Ownership

By City

By State

Download Careers360 App

All this at the convenience of your phone

Scan and download the app