Some Applications of Trigonometry Class 10th Notes - Free NCERT Class 10 Maths Chapter 9 Notes - Download PDF

The real world uses trigonometry for measuring angles, together with distances and heights without physical access to objects. Various professional fields such as architecture, civil engineering, navigation, astronomy, together with computer graphic design, make extensive use of trigonometric methodologies. Every modern structure or astronomy operation depends on basic trigonometric equations as its foundation. The chapter enables to learn of spatial reasoning and introduces mathematical models as concepts. The revision notes present vital formulas combined with actual examples, together with essential concepts to help solve problems efficiently. The correct approach, along with clarity, turns trigonometry into a smart and organized method for addressing real-world problems in both academic settings and actual situations.

New: Get up to 90% Scholarship on NEET/JEE Coaching from top Coaching Institutes

Don't Miss: JEE Main & NEET 2026 Scholarship Test (Class 10): Narayana | Aakash

This Story also Contains

1. NCERT notes Class 10 Maths Chapter 9 Some Applications of Trigonometry
2. Line of Sight:
3. Horizontal line:
4. Angle of Elevation:
5. Angle of Depression:
6. Height and Distance Problems Using Trigonometry:
7. Class 10 Chapter Wise Notes
8. NCERT Exemplar Solutions for Class 10
9. NCERT Solutions for Class 10
10. NCERT Books and Syllabus

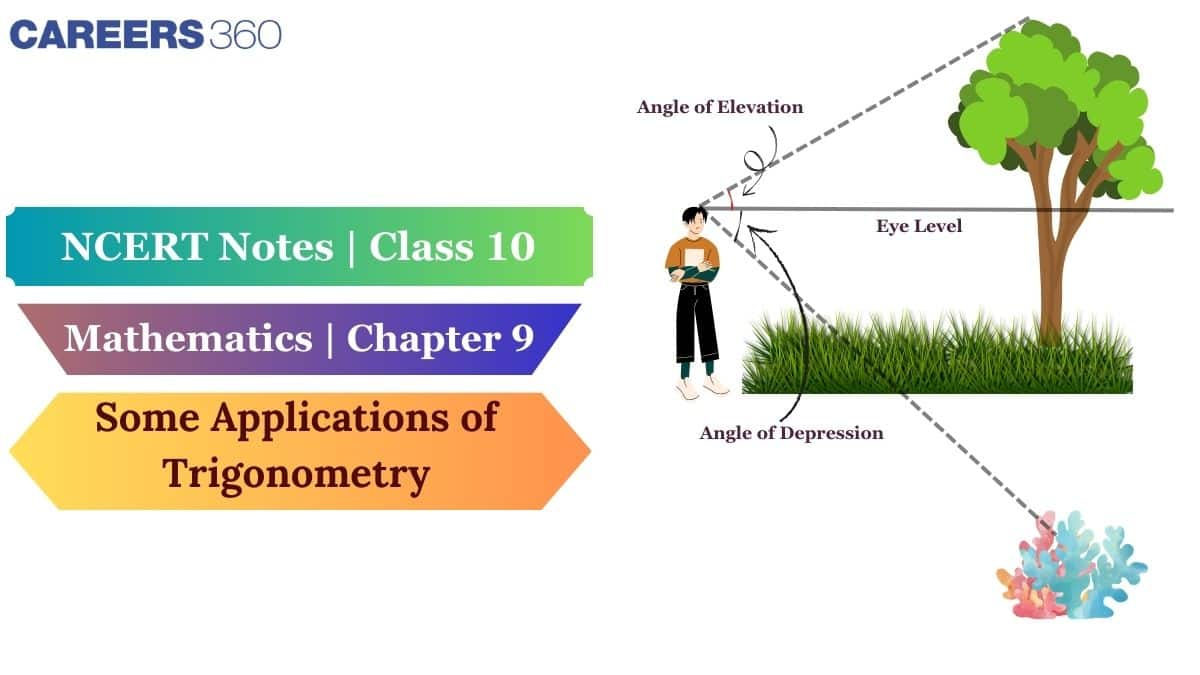
The chapter presents a study of how trigonometric principles apply in fact-based conditions. Students must practice all the topics of Trigonometry and their examples from the NCERT Exemplar Solutions for Class 10 Maths Chapter 9 Some Applications of Trigonometry. Students should use NCERT class 10th maths notes to study concepts and to access additional materials in higher-level chapters through the NCERT Notes prepared according to the latest CBSE pattern.

NCERT notes Class 10 Maths Chapter 9 Some Applications of Trigonometry

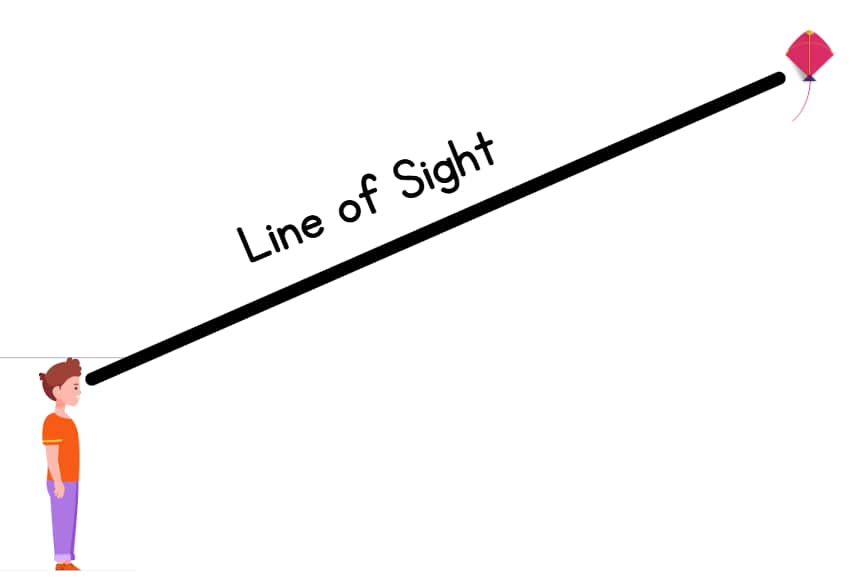
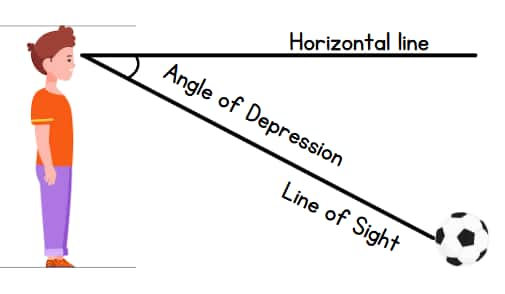
Line of Sight:

Observers create an imaginary straight line that extends from their eyes toward the viewed object. The study of trigonometry requires this tool because it enables users to identify angles during specific observational heights.

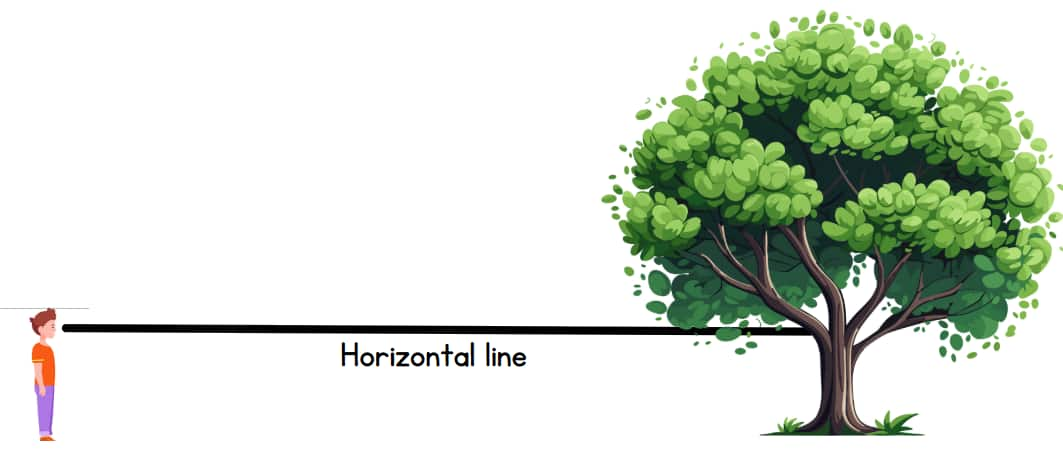
Horizontal line:

The horizontal line represents an invisible straight path which runs parallel to the ground, starting from the viewer's eye level. A horizontal line establishes a reference for trigonometry to measure the angles of elevation and depression.

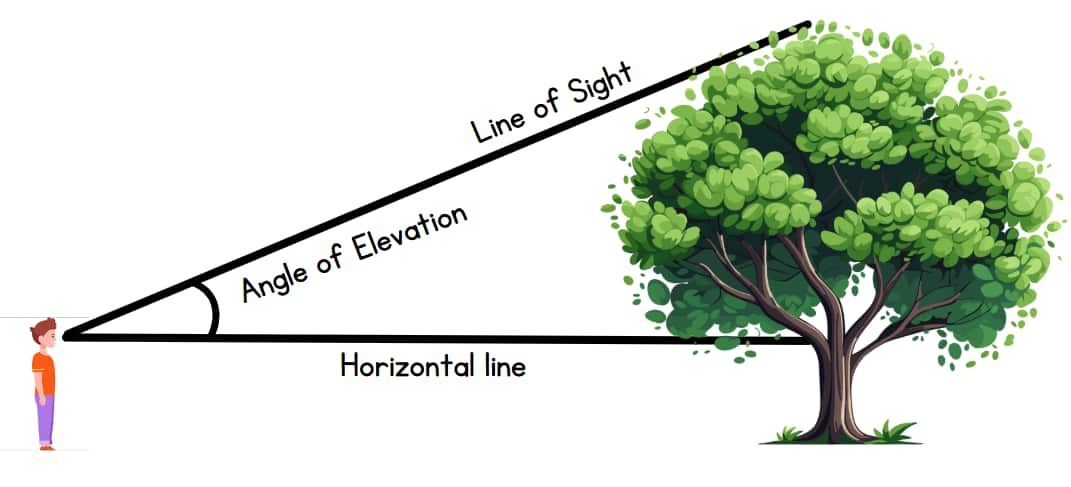
Angle of Elevation:

An observer who looks up toward an object will experience this phenomenon. To determine the measurement, the angle formed between the sightline and the eye-level horizontal line.

For Example, if a person looks at the top of a tree, the angle formed between their line of sight and the horizontal line is the angle of elevation.

Angle of Depression:

An observer who looks down toward an object will experience this phenomenon. To determine the measurement, the angle formed between the sightline and the eye-level horizontal line.

For Example, If you are standing on a cliff and looking at a boat in the sea, the angle between your line of sight and the horizontal line at your eye level is the angle of depression.

Height and Distance Problems Using Trigonometry:

The application of trigonometric ratios enables the solution of problems regarding the measurement of height and distance. The following key sequence directs a solution to these problems:

1. Spot the right-angled triangle present in the provided problem.
2. Identify known along with unknown values of height, distance and angle in this problem.
3. Select the appropriate trigonometric ratio according to the available information.
4. Solve for the unknown value.

Case 1: When the Angle of Elevation is known:

• The observer looks up towards an object.
• The height of the object and the horizontal distance form a right-angled triangle.
• Use the Trigonometric ratios accordingly.

For example, A tower is 30 meters high. The angle of elevation of the top of the tower from a point on the ground is 60°. Find the distance of the point from the base of the tower.

Solution: Given:

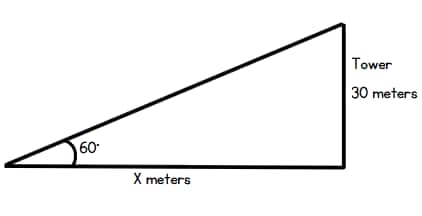
• Height of tower (Perpendicular) = 30 m
• Angle of elevation = 60°
• Distance of the point from the base (Base) = x

Using the formula:

Tan θ = $\frac{Perpendicular}{Base}$

Tan 60° = $\frac{30}{x}$

√3 = $\frac{30}{x}$

X = $\frac{30}{\surd 3}$

X = 10√3 m ≈ 17.32 m

Case 2: When the Angle of Depression is known:

• The observer looks down towards an object.
• The height of the object and the horizontal distance form a right-angled triangle.
• Use the Trigonometric ratios accordingly.

For example, A lighthouse stands on a cliff 50 meters above sea level. A boat is spotted at sea, and the angle of depression from the top of the lighthouse to the boat is 30°. Find the distance of the boat from the base of the cliff.

Solution: Given:

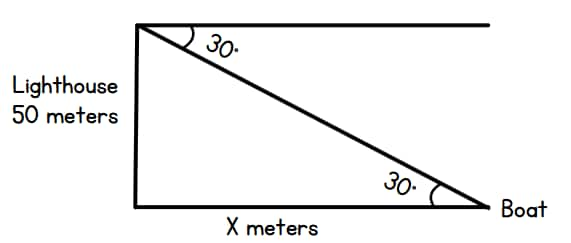
• Height of lighthouse (Perpendicular) = 50 m
• Angle of depression = 30°
• Distance of boat from the base of the cliff (Base) = x

Using the formula:

Tan θ = $\frac{Perpendicular}{Base}$

Tan 30° = $\frac{50}{x}$

$\frac{1}{\surd 3}=\frac{50}{x}$

x = 50√3 ≈ 86.6 meters

Class 10 Chapter Wise Notes

Students must download the notes below for each chapter to ace the topics.

NCERT Exemplar Solutions for Class 10

Students must check the NCERT Exemplar solutions for class 10 of Mathematics and Science Subjects.

• NCERT Exemplar Class 10 Maths solutions
• NCERT Exemplar Class 10 Science solutions

NCERT Solutions for Class 10

Students must check the NCERT solutions for class 10 of Mathematics and Science Subjects.

• NCERT Solutions for Class 10 Maths
• NCERT Solutions for Class 10 Science

NCERT Books and Syllabus

To learn about the NCERT books and syllabus, read the following articles and get a direct link to download them.

• NCERT Books for Class 10
• NCERT Syllabus for Class 10