Coordinate Geometry Class 10th Notes - Free NCERT Class 10 Maths Chapter 7 Notes - Download PDF

Coordinate Geometry is the study of geometry using coordinate points. Coordinate geometry is used to determine the distance between two points. Coordinate geometry is also called analytic geometry or Cartesian geometry. It is a part of geometry. It describes the links between two points in graphs and algebra. Coordinate geometry is used in many areas, like navigation and mapping, including map projection, GPS, air traffic control, computer graphics, interior design, building construction, art and design, etc. It deals with the geometrical figures that have points on the x-axis and y-axis to determine the exact position in a 2-dimensional plane.

New: Get up to 90% Scholarship on NEET/JEE Coaching from top Coaching Institutes

Don't Miss: JEE Main & NEET 2026 Scholarship Test (Class 10): Narayana | Aakash

This Story also Contains

1. NCERT Class 10 Maths Chapter 7 Coordinate Geometry: Notes
2. Class 10 Chapter Wise Notes
3. NCERT Exemplar Solutions for Class 10
4. NCERT Solutions for Class 10
5. NCERT Books and Syllabus

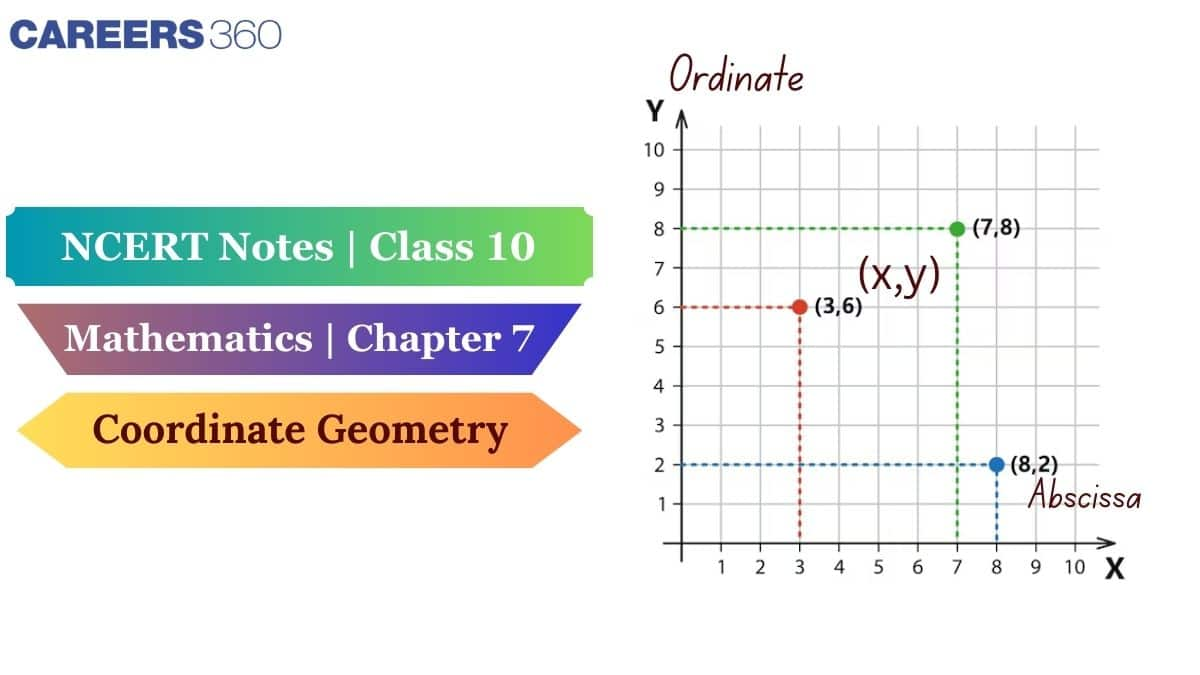
These notes include basic definitions of coordinate geometry, its subtopics like definition of points, formulae required to calculate the distance between two points, section formula, mid point theorem, what a coordinate is and a coordinate plane, equation of a line in the Cartesian plane, etc. CBSE Class 10 chapter Coordinate Geometry includes all the topics covered in these notes. Students must practice questions and check their solutions using the NCERT Solutions for Class 10 Maths Chapter 7 Coordinate Geometry. These NCERT class 10th maths notes cover the complete syllabus of class 10, all the required definitions, formulas, and examples. These NCERT notes are designed according to the latest syllabus of NCERT.

NCERT Class 10 Maths Chapter 7 Coordinate Geometry: Notes

Coordinate Geometry: Coordinate geometry is a branch of geometry that is used to determine the position of points using coordinates.

Coordinates: Coordinates are the set of values that are used to define the exact position of a point in the coordinate plane.

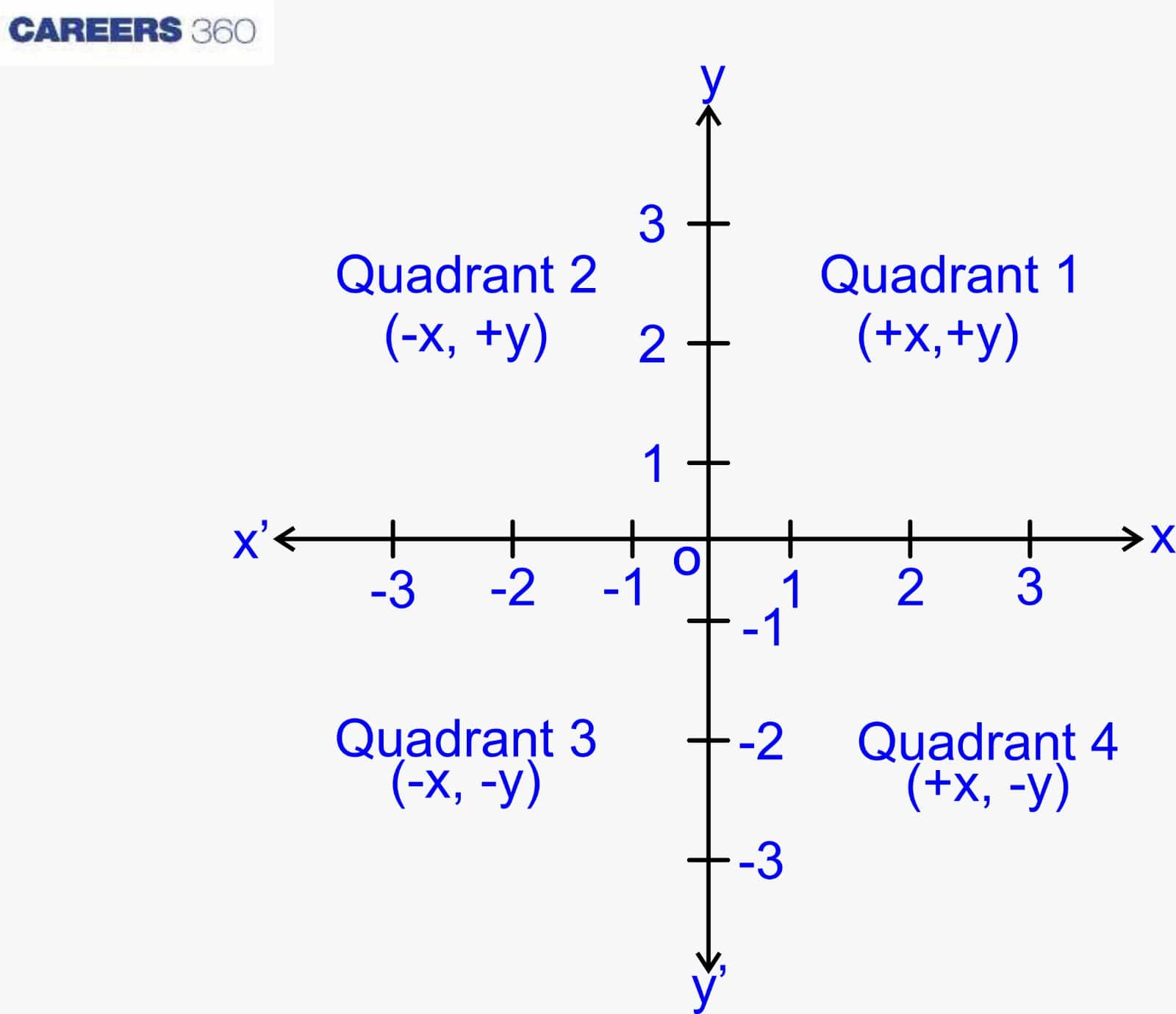
Coordinate Plane: It is a 2-dimensional plane that has two perpendicular axis called the x-axis and y-axis that intersect at a point. The point where both axis intersect is called the origin.

The 2-dimensional coordinate plane has four quadrants that are shown below:
1. Quadrant 1: (+x, +y)
2. Quadrant 2: (-x, +y)
3. Quadrant 3: (-x, -y)
4. Quadrant 4: (+x, -y)

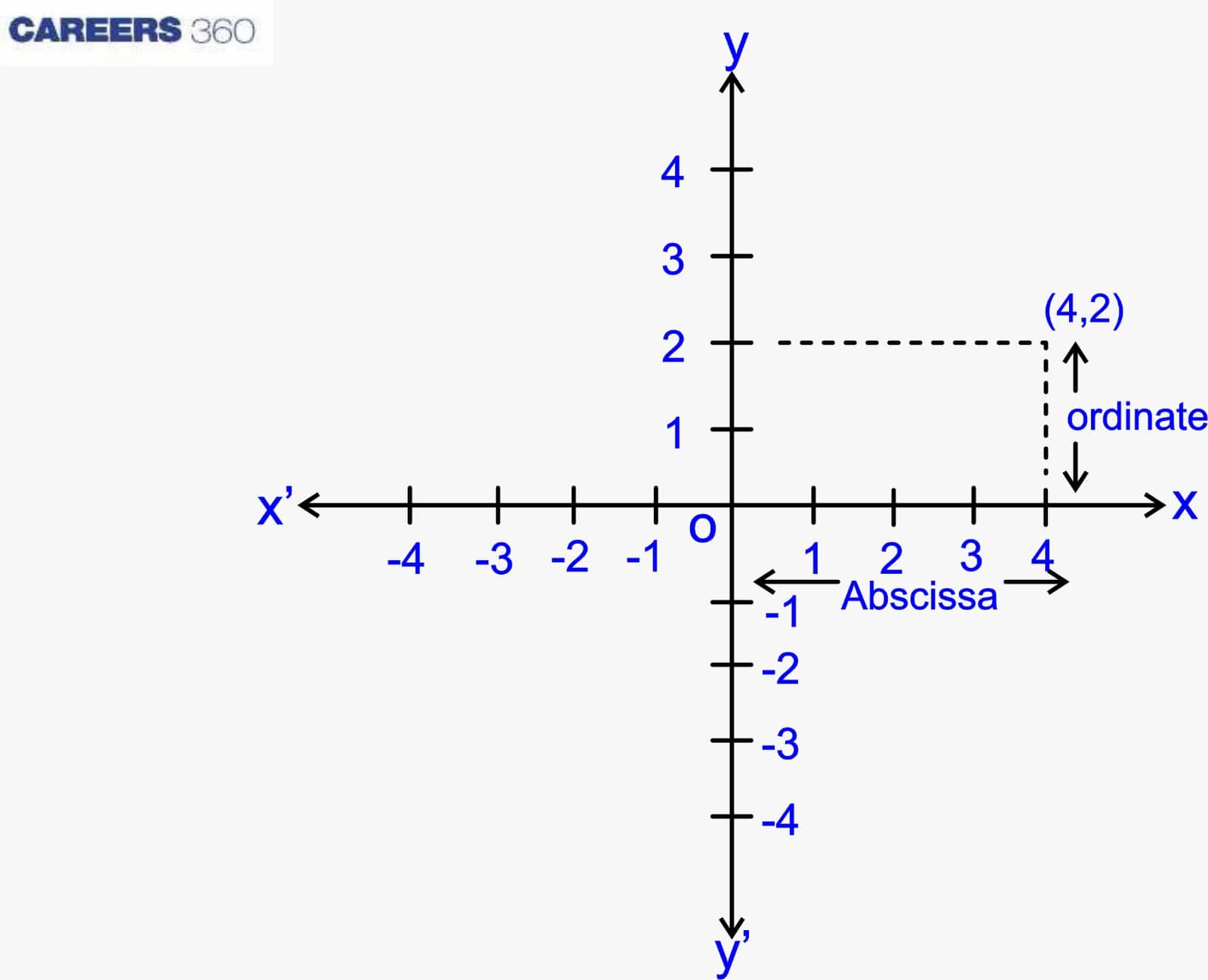
Coordinates or Points On the Cartesian or Coordinate Plane:

A pair of numbers is generally given in the terms of (x, y), and these numbers are called coordinates, and these coordinates define the position in the coordinate plane. In this plane, the distance from the point on the y-axis is called the abscissa or x-coordinate, and the distance of a point from the x-axis is called the y-coordinate or ordinate.
Example: Consider coordinate (4, 2) as shown in Figure 4 is the abscissa, and 2 is the ordinate. 4 represents the distance from the y-axis, and 2 represents the distance of a point from the x-axis.

Distance Formula:

The distance between the given points is calculated differently based on the coordinates and the position of the points.

The Distance Between Two Points on the Same Axis Coordinates:

If the two points are on the same axis, x-axis or y-axis, then the difference will be determined by subtracting the value of the x-axis from the x-axis and the y-axis from the y-axis.

Example: Consider the figure shown below:

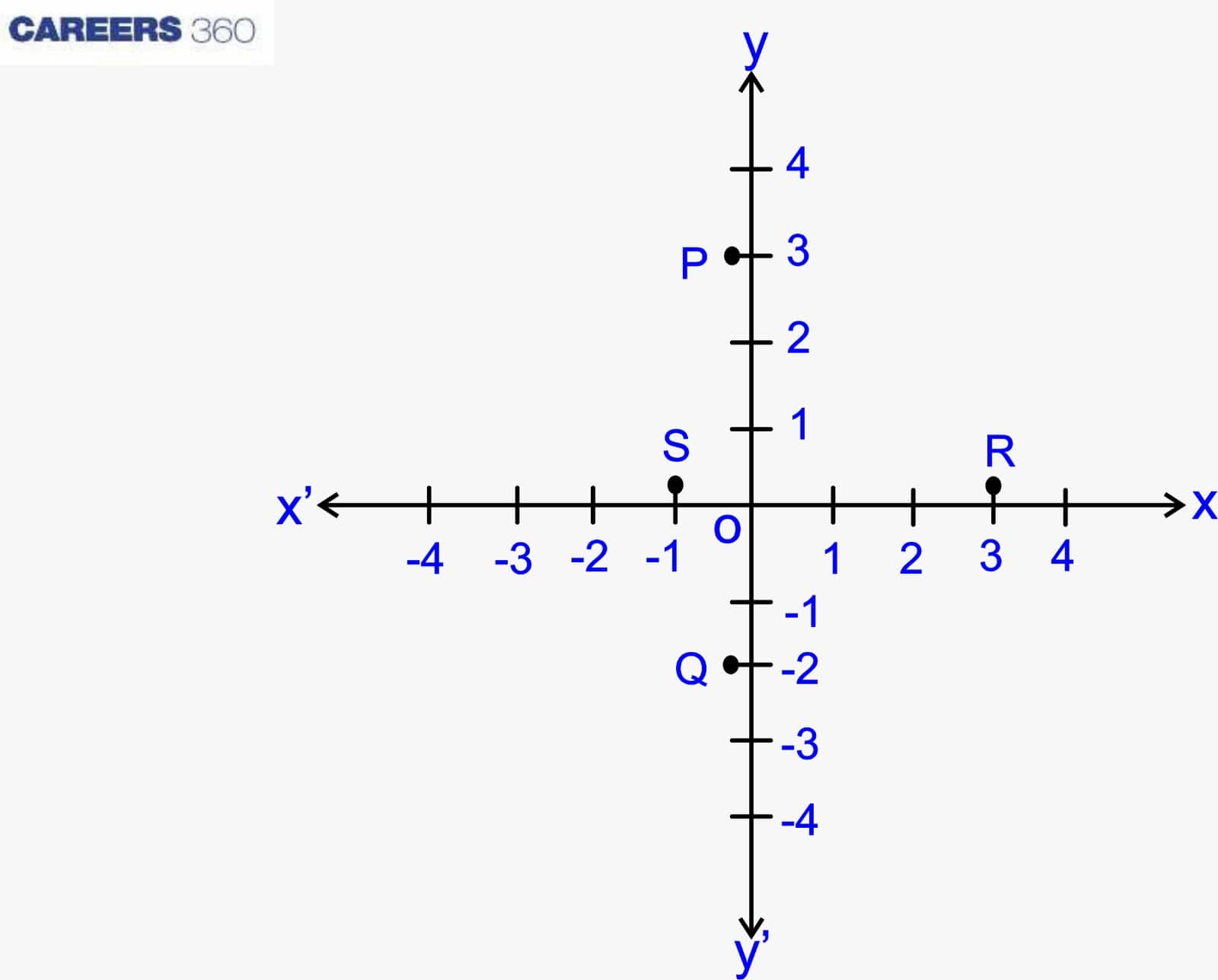
Distance between PQ = 3 - (-2) = 5
Distance between RS = 3 - (-1) = 4

Calculating Distance Between Two Points Using Pythagoras Theorem:

The distance between two points can be calculated using the Pythagorean theorem by extending the lines and creating a right-angle triangle.

Example: Consider points A(0, 3), B(4, 3), C(-4, -4), and D(-4, 0), determine the distance between C and D.
Given:
A(0, 3), B(4, 3), C(-4, -4), and D(-4, 0)
Extend the BA up to E and join C, D and E to make a right-angle triangle as shown below,

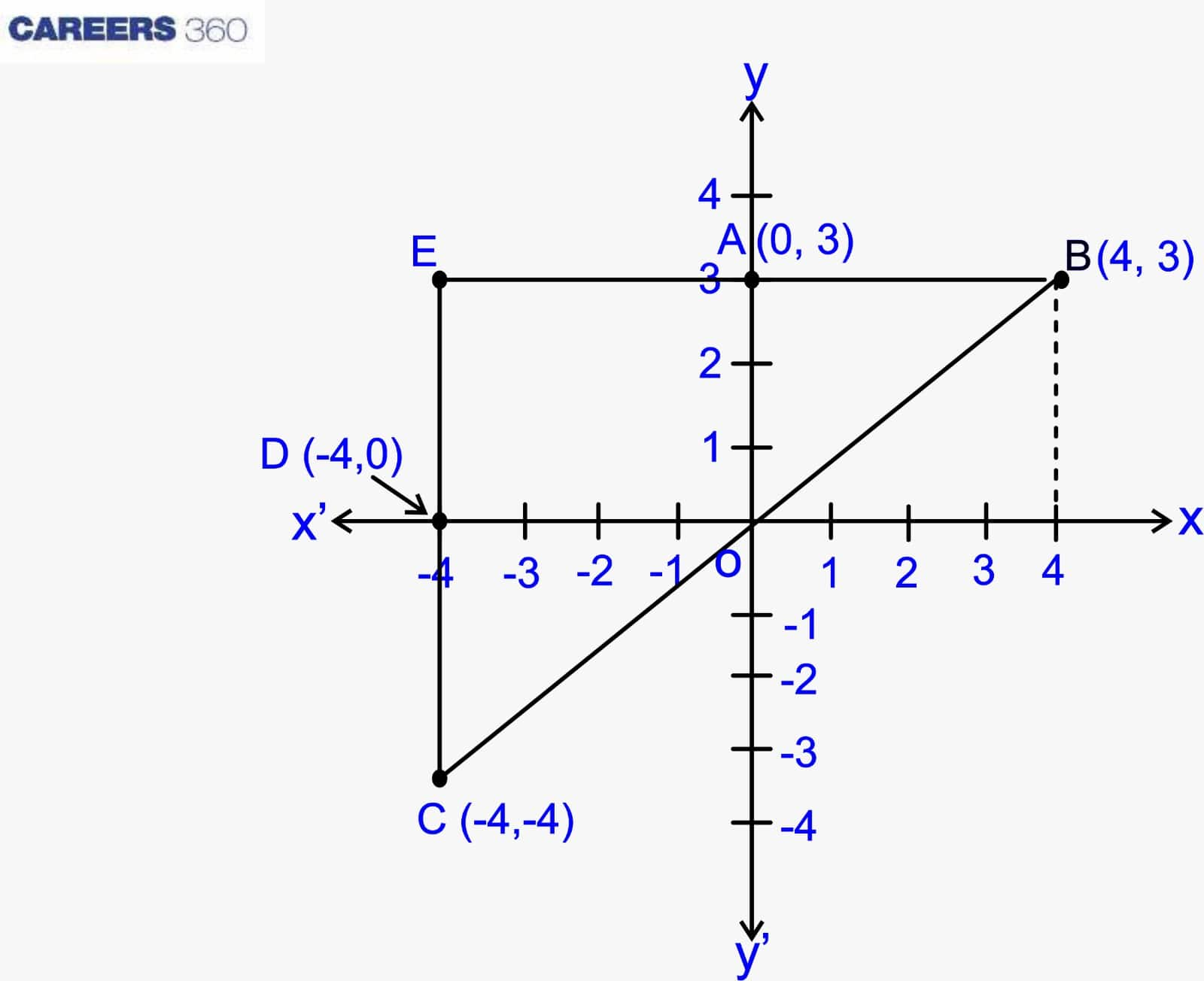
The distance between CD by using Pythagoras' Theorem is:
CD² = BE² + EC²
CD² = (4 - (-4))² + (0 - (-4))²
CD² = 8² + 4²
CD² = 64 + 16
CD = √80 units

Distance Formula:

If two points (x₁, y₁) and (x₂, y₂) then the distance can be calculated as:
d
= √[x₂ – x₁)²+(y₂ – y₁)²]

Section Formula:

If a point P divides the line segment in the ratio of m : n, then the point P can be determined by the section formula. Suppose point P(x, y) divides the line segment A(x₁, y₁) and B(x₂, y₂) in m : n ratio, then to determine the P(x, y) the section formula is as follows:

$P(x,y)$ = $(\frac{mx2+nx1}{m+n}$, $\frac{my2+ny1}{m+n})$

Example: Find the coordinates of the point which divides the line segment joining the points (3, 1) and (7, 4) in the ratio 2 : 1 internally.Given:
m : n = 2 : 1

x = $(\frac{2(7)+1(1)}{2+1})$, y = $(\frac{2(4)+1(-2)}{2+1})$

x = $(\frac{14+1}{3})$, y = $(\frac{8-2)}{3})$

x = 5, y = 2

Finding The Ratio Given Points:

If P(x, y) is the point that divides the line segments A(x1, y1) and B(x2, y2) internally, then the ratio of the line can be determined as follows:
Assume that the ratio is k : 1
Substitute the ratio in the section formula for any of the coordinates to get the value of k.

x = $(\frac{kx2+x1}{k+1})$ or y = $(\frac{ky2+y1}{k+1})$

Example: Find the ratio when point (– 3, 5) divides the line segment joining the points A(– 5, 9) and B(2, – 7)?
Given:
A(– 5, 9) and B(2, – 7)

P (– 3, 5)

m/n : 1 = k : 1

P(– 3, 5) divide the line into k : 1 segment,

x = $(\frac{kx2+x1}{k+1})$, y = $(\frac{ky2+y1}{k+1})$

After substituting the values we get,

-3 = $(\frac{2k+(-5)}{k+1})$

-3 = $(\frac{2k-5)}{k+1})$

-3k - 3 = 2k - 5

k = $(\frac{5}{2})$

Therefore, the required ratio is 5 : 2.

Midpoint:

Midpoint is a point in a line that divides the line into two equal parts or in 1 : 1 ratio. If the P is the point that divides the line A(x₁, y₁) and B(x₂, y₂). Therefore,

$P(x,y)$ = $(\frac{x1+x2}{2}$, $\frac{y1+y2}{2})$

Example: What is the midpoint of line segment AB whose coordinates are A(-4, 4) and B(2, 6), respectively?

Given:
A(-4, 4) and B(2, 6)

$P(x,y)$ = $(\frac{-4+2}{2}$, $\frac{4+6}{2})$

$P(x,y)$ = $(\frac{2}{2}$, $\frac{10}{2})$

$P(x,y)$ = $(1,5)$

Therefore, the midpoint is (1, 5)

Centroid of a Triangle

If P(x₁, y₁), Q(x₂, y₂), and R(x₃, y₃) are the vertices of a ΔPQR, then the coordinates of its centroid(A) are defined by,

$A(x,y)$ = $(\frac{x1+x2+x3}{3}$, $\frac{y1+y2+y3}{3})$

Example: Find the coordinates of the centroid of a triangle whose vertices are given as (-2, -4), (5, 1) and (9, -3)

Given:
(-2, -4), (5, 2) and (9, -3)

$A(x,y)$ = $(\frac{x1+x2+x3}{3}$, $\frac{y1+y2+y3}{3})$

After substituting the values we get,

$A(x,y)$ = $(\frac{-2+5+9}{3}$, $\frac{-4+1+(-3)}{3})$

$A(x,y)$ = $(\frac{12}{3}$, $\frac{-6)}{3})$

$A(x,y)$ = $(4,-2)$

Therefore, the centroid of the triangle is (4, -2)

Area of a Triangle Using Coordinate Geometry:

If P(x₁, y₁), Q(x₂, y₂), and R(x₃, y₃) are the vertices of a ΔPQR, then the area of ΔPQR can be defined as,

$Area$ = $\frac{1}{2}$ $[x1(y2-y3)+x2(y3-y1)+x3(y1-y2)]$

Example: Find the area of the triangle ABC whose vertices are A(2, 3), B(5, 3) and C(4, 6).
Given:
A(2, 3), B(5, 3) and C(4, 6)

$Area$ = $\frac{1}{2}$ $[x1(y2-y3)+x2(y3-y1)+x3(y1-y2)]$

After substituting the values, we get:

$Area$ = $\frac{1}{2}$ $[2(3-6)+5(6-3)+4(3-3)]$

$Area$ = $\frac{1}{2}$ $[2(-3)+5(3)+4(0)]$

$Area$ = $\frac{1}{2}$ $[-6+15+0]$

$Area$ = $\frac{1}{2}$ $[-9]$

$Area$ = $\frac{9}{2}$

Therefore, the area of the triangle is $\frac{9}{2}$ square units.

Class 10 Chapter Wise Notes

Students must download the notes below for each chapter to ace the topics.

NCERT Exemplar Solutions for Class 10

Students must check the NCERT Exemplar solutions for class 10 of Mathematics and Science Subjects.

• NCERT Exemplar Class 10 Maths solutions

• NCERT Exemplar Class 10 Science solutions

NCERT Solutions for Class 10

Students must check the NCERT solutions for class 10 of Mathematics and Science Subjects.

• NCERT Solutions for Class 10 Maths

• NCERT Solutions for Class 10 Science

NCERT Books and Syllabus

To learn about the NCERT books and syllabus, read the following articles and get a direct link to download them.

• NCERT Books for Class 10

• NCERT Syllabus for Class 10