NCERT Solutions for Exercise 10.2 Class 10 Maths Chapter 10

NCERT Solutions for Exercise 10.2 Class 10 Maths Chapter 10 - Circles

Edited By Ramraj Saini | Updated on Nov 27, 2023 01:49 PM IST | #CBSE Class 10th

Upcoming Event

CBSE Class 10th Exam Date : 15 Feb' 2025 - 15 Feb' 2025

NCERT Solutions For Class 10 Maths Chapter 10 Exercise 10.2

NCERT Solutions for Exercise 10.2 Class 10 Maths Chapter 10 Circles are discussed here. These NCERT solutions are created by subject matter expert at Careers360 considering the latest syllabus and pattern of CBSE 2023-24. Class 10 maths ex 10.2 which is an exercise followed by exercise 10.1 includes the concept of circles, there are many numerical problems with the number of tangents from a point. If we talk about tests and exams, this is an important part to cover. These concepts are easy to understand and can be worked accordingly. Students can find NCERT solutions for class 10 Maths here.

Don't Miss: JEE Main & NEET 2026 Scholarship Test (Class 10): Narayana | Aakash

This Story also Contains

NCERT Solutions For Class 10 Maths Chapter 10 Exercise 10.2
Download Free Pdf of NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2
Assess NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2
More About NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2
Benefits of NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2
NCERT Solutions Subject Wise

NCERT solutions for exercise 10.2 Class 10 Maths chapter 10 Circles covers problems on the topics like the concept of finding radius and distance from one point on the circle and other points outside the circle. 10th class Maths exercise 10.2 answers are designed as per the students demand covering comprehensive, step by step solutions of every problem. Practice these questions and answers to command the concepts, boost confidence and in depth understanding of concepts. Students can find all exercise together using the link provided below.

Circle Exercise 10.1

Pearson | PTE

Register now for PTE & Unlock 20% OFF : Use promo code: 'C360SPL20'. Valid till 31st DEC'24! Trusted by 3,500+ universities globally

Apply

Download Free Pdf of NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2

Download PDF

Assess NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2

Circles Class 10 Chapter 10 Circles Exercise: 10.2

Q1 From a point Q, the length of the tangent to a circle is 24 cm and the distance of Q from the center is 25 cm. The radius of the circle is

(A) 7 cm

(B) 12 cm

(D) 24.5 cm

Answer:

The correct option is (A) = 7 cm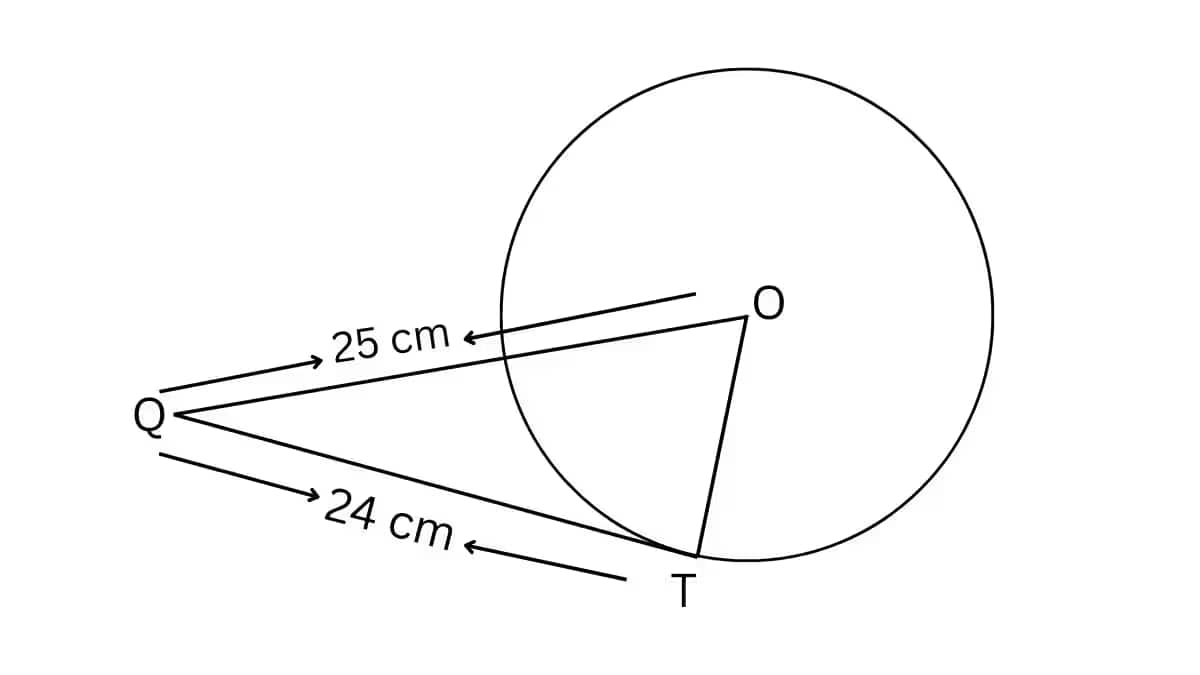
1639544843884 Given that,
The length of the tangent (QT) is 24 cm and the length of OQ is 25 cm.
Suppose the length of the radius OT be $l$ cm.
We know that $\Delta OTQ$ is a right angle triangle. So, by using Pythagoras theorem-

$\\OQ^2 = TQ^2+OT^2\\\\ l = \sqrt{25^2-24^2}\\\\OT = l=\sqrt{49}$

OT = 7 cm

Q2 In Fig. 10.11, if TP and TQ are the two tangents to a circle with center O so that $\angle$ POQ = $11 0 \degree$ , then $\angle$ PTQ is equal to

1639544819399

(A) $60 \degree$

(B) $70 \degree$

(D) $9 0 \degree$

Answer:

The correct option is (b)

1594293296951
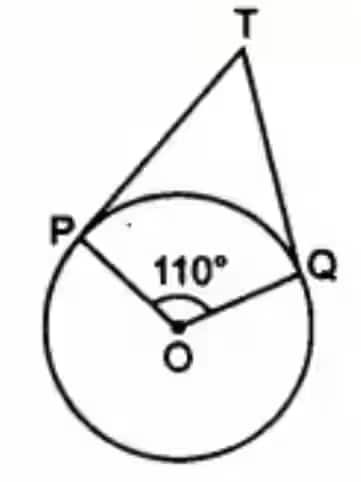
In figure, $\angle POQ = 110^0$
Since POQT is quadrilateral. Therefore the sum of the opposite angles are 180

$\\\Rightarrow \angle PTQ +\angle POQ = 180^0\\\\\Rightarrow \angle PTQ = 180^0-\angle POQ$
$= 180^0-100^0$
$= 70^0$

Q3 If tangents PA and PB from a point P to a circle with center O are inclined to each other at an angle of $80 \degree$ , then $\angle$ POA is equal to

(A) 50°

(B) 60°

(D) 80°

Answer:

The correct option is (A)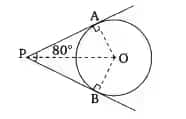
1594293381602
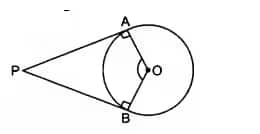
It is given that, tangent PA and PB from point P inclined at $\angle APB = 80^0$
In triangle $\Delta$ OAP and $\Delta$ OBP
$\angle OAP = \angle OBP = 90$
OA =OB (radii of the circle)
PA = PB (tangents of the circle)

Therefore, by SAS congruence
$\therefore \Delta OAP \cong \Delta OBP$

By CPCT, $\angle OPA = \angle OPB$
Now, $\angle$ OPA = 80/4 = 40

In $\Delta$ PAO,
$\angle P + \angle A + \angle O = 180$
$\angle O = 180 - 130$
= 50

Q4 Prove that the tangents drawn at the ends of a diameter of a circle are parallel.

Answer: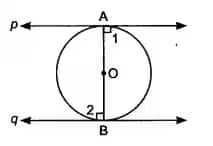

1594293543765
Let line $p$ and line $q$ are two tangents of a circle and AB is the diameter of the circle.
OA and OB are perpendicular to the tangents $p$ and $q$ respectively.
therefore,
$\angle1 = \angle2 = 90^0$

$\Rightarrow$ $p$ || $q$ { $\angle$ $\because$ 1 & $\angle$ 2 are alternate angles}

Q5 Prove that the perpendicular at the point of contact to the tangent to a circle passes through the center.

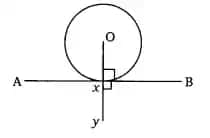
Answer:

1594293656560
In the above figure, the line AXB is the tangent to a circle with center O. Here, OX is the perpendicular to the tangent AXB ( $OX\perp AXB$ ) at point of contact X.
Therefore, we have,
$\angle$ BXO + $\angle$ YXB = $90^0+90^0=180^0$

$\therefore$ OXY is a collinear
$\Rightarrow$ OX is passing through the center of the circle.

Q6 The length of a tangent from a point A at distance 5 cm from the center of the circle is 4 cm. Find the radius of the circle.

Answer:

1594293766356
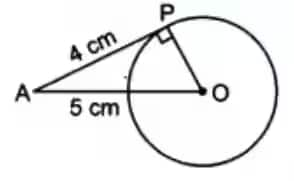
Given that,
the length of the tangent from the point A (AP) is 4 cm and the length of OA is 5 cm.
Since $\angle$ APO = 90 ⁰
Therefore, $\Delta$ APO is a right-angle triangle. By using Pythagoras theorem;

$OA^2=AP^2+OP^2$
$5^2 = 4^2+OP^2$
$OP=\sqrt{25-16}=\sqrt{9}$
$OP = 3 cm$

Q7 Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.

Answer:

1594293901543
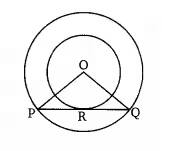
In the above figure, Pq is the chord to the larger circle, which is also tangent to a smaller circle at the point of contact R.
We have,
radius of the larger circle OP = OQ = 5 cm
radius of the small circle (OR) = 3 cm

OR $\perp$ PQ [since PQ is tangent to a smaller circle]

According to question,

In $\Delta$ OPR and $\Delta$ OQR
$\angle$ PRO = $\angle$ QRO {both $90^0$ }

OR = OR {common}
OP = OQ {both radii}

By RHS congruence $\Delta$ OPR $\cong$ $\Delta$ OQR
So, by CPCT
PR = RQ
Now, In $\Delta$ OPR,
by using pythagoras theorem,
$PR = \sqrt{25-9} =\sqrt{16}$
PR = 4 cm
Hence, PQ = 2.PR = 8 cm

Q8 A quadrilateral ABCD is drawn to circumscribe a circle (see Fig. 10.12). Prove that AB + CD = AD + BC

1594294016205

Answer:

1594294152195
To prove- AB + CD = AD + BC
Proof-
We have,
Since the length of the tangents drawn from an external point to a circle are equal
AP =AS .......(i)
BP = BQ.........(ii)
AS = AP...........(iii)
CR = CQ ...........(iv)

By adding all the equations, we get;

AP + BP +RD+ CR = AS +DS +BQ +CQ
$\Rightarrow$ (AP + BP) + (RD + CR) = (AS+DS)+(BQ + CQ)
$\Rightarrow$ AB + CD = AD + BC

Hence proved.

Q9 In Fig. 10.13, XY and X'Y'are two parallel tangents to a circle with center O and another tangent AB with a point of contact C intersecting XY at A and X'Y' at B. Prove that $\angle$ AOB = 90°.

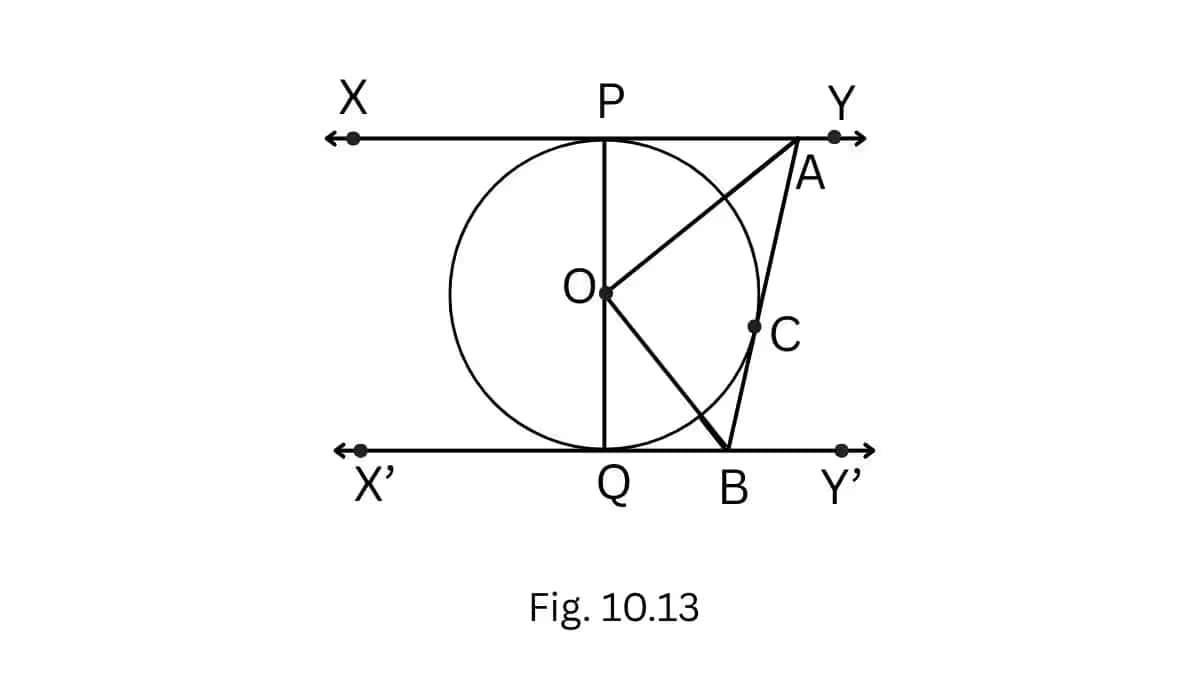
Answer:

1594294251028
To prove- $\angle$ AOB = $90^0$
Proof-
In $\Delta$ AOP and $\Delta$ AOC,
OA =OA [Common]
OP = OC [Both radii]
AP =AC [tangents from external point A]
Therefore by SSS congruence, $\Delta$ AOP $\cong$ $\Delta$ AOC
and by CPCT, $\angle$ PAO = $\angle$ OAC
$\Rightarrow \angle PAC = 2\angle OAC$ ..................(i)

Similarly, from $\Delta$ OBC and $\Delta$ OBQ, we get;
$\angle$ QBC = 2. $\angle$ OBC.............(ii)

Adding eq (1) and eq (2)

$\angle$ PAC + $\angle$ QBC = 180
2( $\angle$ OBC + $\angle$ OAC) = 180
( $\angle$ OBC + $\angle$ OAC) = 90

Now, in $\Delta$ OAB,
Sum of interior angle is 180.
So, $\angle$ OBC + $\angle$ OAC + $\angle$ AOB = 180
$\therefore$ $\angle$ AOB = 90
hence proved.

Q10 Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line-segment joining the points of contact at the center.

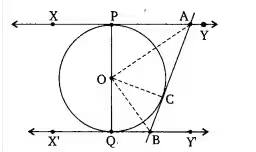
Answer:

1594294380467
To prove - $\angle APB + \angle AOB = 180^0$
Proof-
We have, PA and PB are two tangents, B and A are the point of contacts of the tangent to a circle. And $OA\perp PA$ , $OB\perp PB$ (since tangents and radius are perpendiculars)

According to question,
In quadrilateral PAOB,
$\angle$ OAP + $\angle$ APB + $\angle$ PBO + $\angle$ BOA = $360^0$
90 + $\angle$ APB + 90 + $\angle$ BOA = 360
$\angle APB + \angle AOB = 180^0$
Hence proved .

Q11 Prove that the parallelogram circumscribing a circle is a rhombus.

Answer: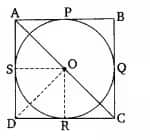

1594294663376
To prove - the parallelogram circumscribing a circle is a rhombus
Proof-
ABCD is a parallelogram that circumscribes a circle with center O.
P, Q, R, S are the points of contacts on sides AB, BC, CD, and DA respectively
AB = CD .and AD = BC...........(i)
It is known that tangents drawn from an external point are equal in length.
RD = DS ...........(ii)
RC = QC...........(iii)
BP = BQ...........(iv)
AP = AS .............(v)
By adding eq (ii) to eq (v) we get;
(RD + RC) + (BP + AP) = (DS + AS) + (BQ + QC)
CD + AB = AD + BC
$\Rightarrow$ 2AB = 2AD [from equation (i)]
$\Rightarrow$ AB = AD
Now, AB = AD and AB = CD
$\therefore$ AB = AD = CD = BC

Hence ABCD is a rhombus.

Q12 A triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 8 cm and 6 cm respectively (see Fig. 10.14). Find the sides AB and AC.

Answer:

1639543596459

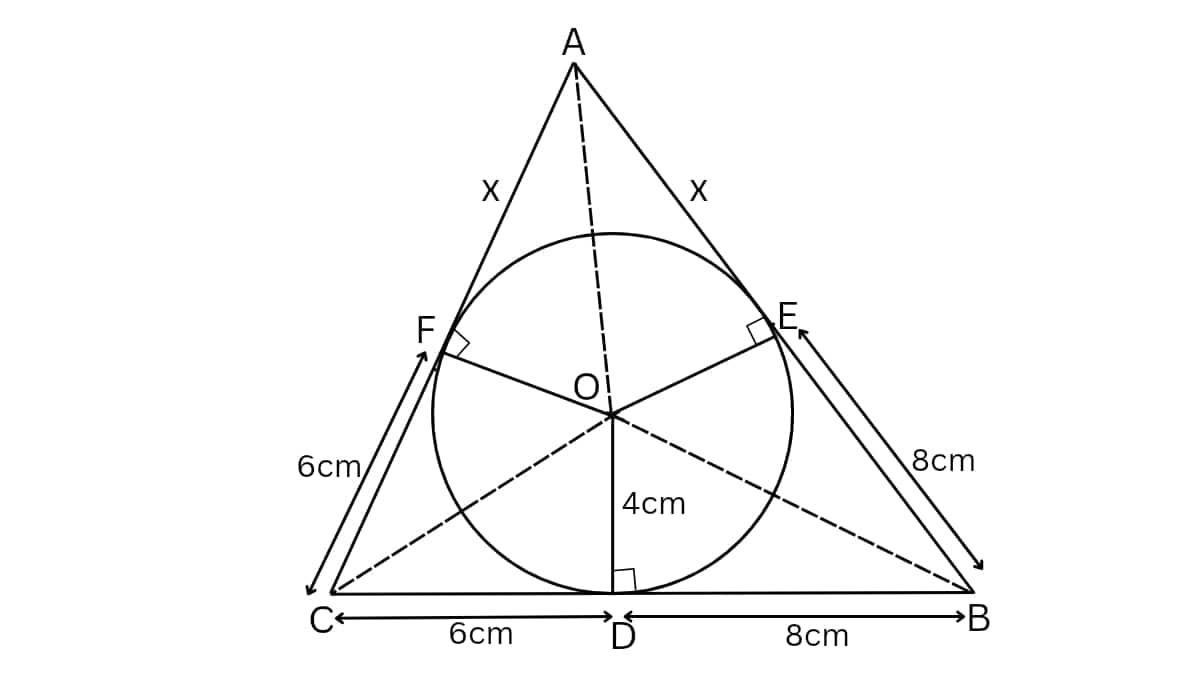
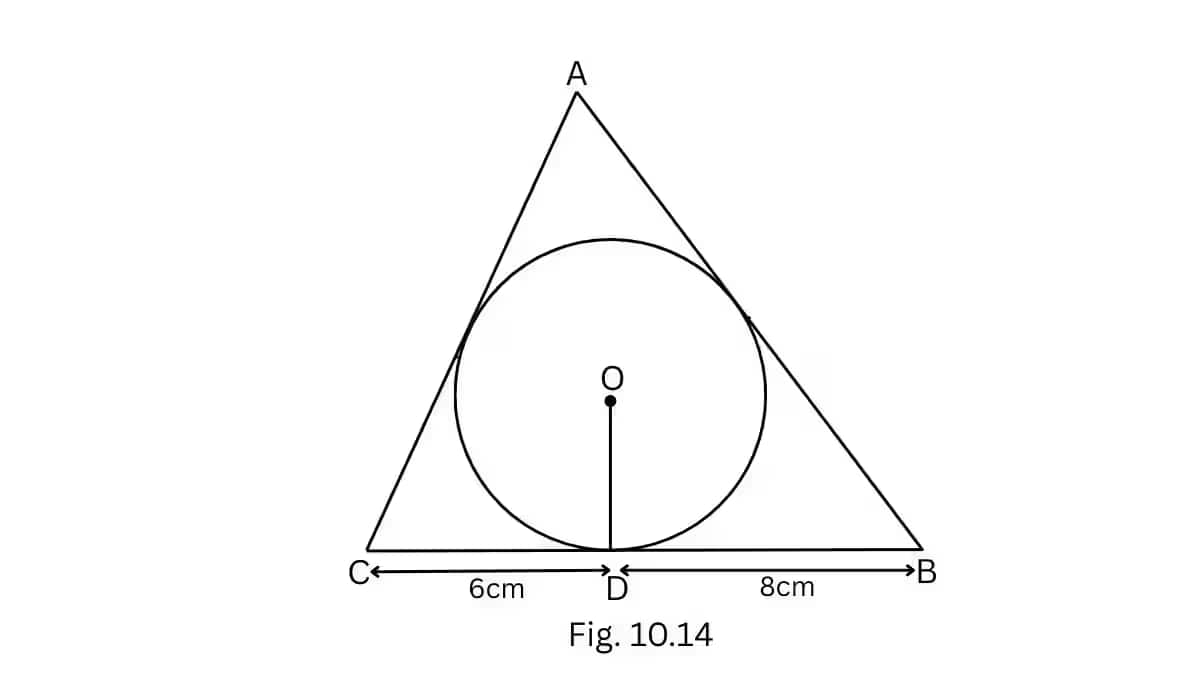
Consider the above figure. Assume center O touches the sides AB and AC of the triangle at point E and F respectively.

Let the length of AE is x.

Now in $\bigtriangleup ABC$ ,

$CF = CD = 6$ (tangents on the circle from point C)

$BE = BD = 6$ (tangents on the circle from point B)

$AE = AF = x$ (tangents on the circle from point A)

Now AB = AE + EB

$\\AB = AE + EB\\\\ => AB = x + 8\\\\ BC = BD + DC\\\\ => BC = 8+6 = 14\\\\ CA = CF + FA\\\\ => CA = 6 + x\\\\$

Now
$\\s = (AB + BC + CA )/2\\\\ => s = (x + 8 + 14 + 6 +x)/2\\\\ => s = (2x + 28)/2\\\\ => s = x + 14$

Area of triangle $\bigtriangleup ABC$

$\\=\sqrt{s*(s-a)*(s-b)*(s-c)}\\\\=\sqrt{(14+x)*[(14+x)-14]*[(14+x)-(6+x)]*[(14+x)-(8+x)]}\\\\=4\sqrt{3(14x+x^2)}$
Now the area of $\bigtriangleup OBC$

$\\= (1/2)*OD*BC\\\\ = (1/2)*4*14\\\\ = 56/2 = 28$
Area of $\bigtriangleup OCA$

$\\= (1/2)*OF*AC \\\\= (1/2)*4*(6+x) \\\\= 2(6+x) \\\\= 12 + 2x$

Area of $\bigtriangleup OAB$

$\\= (1/2)*OE*AB \\\\= (1/2)*4*(8+x) \\\\= 2(8+x) \\\\= 16 + 2x$

Now Area of the $\bigtriangleup ABC$ = Area of $\bigtriangleup OBC$ + Area of $\bigtriangleup OCA$ + Area of $\bigtriangleup OAB$

$\\=> 4\sqrt{3x(14+x)}= 28 + 12 + 2x + 16 + 2x \\\\=> 4\sqrt{3x(14+x)} = 56 + 4x \\\\=> 4\sqrt{3x(14+x)} = 4(14 + x) \\\\=> \sqrt{3x(14+x)}] = 14 + x$

On squaring both the side, we get

$\\3x(14 + x) = (14 + x)^2\\\\ => 3x = 14 + x \:\:\:\:\:\: (14 + x = 0 => x = -14\: is\: not\: possible) \\\\=> 3x - x = 14\\\\ => 2x = 14\\\\ => x = 14/2\\\\ => x = 7$

Hence

AB = x + 8

=> AB = 7+8

=> AB = 15

AC = 6 + x

=> AC = 6 + 7

=> AC = 13

Answer- AB = 15 and AC = 13

Q13 Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the center of the circle.

Answer:

1594294831132
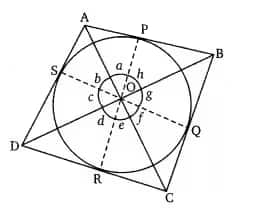
Given- ABCD is a quadrilateral circumscribing a circle. P, Q, R, S are the point of contact on sides AB, BC, CD, and DA respectively.

To prove-
$\\\angle AOB + \angle COD =180^0\\ \angle AOD + \angle BOC =180^0$
Proof -
Join OP, OQ, OR and OS
In triangle $\Delta$ DOS and $\Delta$ DOR,
OD =OD [common]
OS = OR [radii of same circle]
DR = DS [length of tangents drawn from an external point are equal ]
By SSS congruency, $\Delta$ DOS $\cong$ $\Delta$ DOR,
and by CPCT, $\angle$ DOS = $\angle$ DOR
$\angle c = \angle d$ .............(i)

Similarily,
$\\\angle a = \angle b\\ \angle e = \angle f\\ \angle g =\angle h$ ...............(2, 3, 4)

$\therefore 2(\angle a +\angle e +\angle h+\angle d) = 360^0$
$\\(\angle a +\angle e) +(\angle h+\angle d) = 180^0\\ \angle AOB + \angle DOC = 180^0$
SImilarily, $\angle AOD + \angle BOC = 180^0$

Hence proved.

Benefits of NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2

The NCERT solutions for class 10 Maths chapter 10 exercise 10.2 and the solved example before exercise 10.2 Class 10 Maths are significant since they cover questions from the basic concept of Circle and its relations.
If students can answer all of the questions in exercise 10.2 Class 10 Maths, they will have a better understanding of the notion of problem-solving as it is asked in terms of tangents or chords in chapter 10 of Class 10 Maths.
Students may receive MCQs, short answer, or long answer questions from the types discussed in Class 10 Maths chapter 10 exercise 10.2 for final exams.

Also see-

NCERT Solutions Subject Wise

Subject Wise NCERT Exemplar Solutions

Frequently Asked Questions (FAQs)

1. Define Circle?

A curved line whose ends meet and all points on the line are at the same distance from the centre or a path that revolves around a central point or a group of items arranged is called a circle.

2. What is the shortest distance between two parallel tangents of circle?

The shortest distance between two parallel tangents of the circle is Diameter i.e. twice the radius of given circle

3. From how many points a circle can be formed?

a circle can be formed by three points but the condition is the points must be non-collinear non-parallel.

4. What is the sum of Interior angles of a Quadrilateral ?

The sum of interior angles of a quadrilateral is 360 degrees.

5. What is the name of the polynomial with degree 3?

The degree 3 polynomial is referred to as Cubic polynomials is a type of polynomial in which there are

6. What is SSS Congruence in circle?

If in a circle if two tangents are parallel and another tangent from parallel tangent one cuts parallel tangent 2 forming two triangles. If these triangles have three sides in common then these triangles are equal by sss congruence.

7. What does the line intersecting circle at two points is called?

The line intersecting circle at two points is called a Secant

Also Read

NCERT Class 10 Maths Notes - Download Chapter Wise PDFs

The Human Eye and The Colorful world Class 10th Notes- Free NCERT Class 10 Science Chapter 11 Notes - Download PDF

NCERT Class 10 Science Chapter 14 Notes Sources Of Energy - Download PDF

Magnetic Effects of Electric Current Class 10th Notes - Free NCERT Class 10 Science Chapter 13 Notes - Download PDF

Electricity Class 10th Notes - Free NCERT Class 10 Science Chapter 12 Notes - Download PDF

Light- Reflection and Refraction Class 10th Notes- Free NCERT Class 10 Science Chapter 10 Notes - Download PDF

NCERT Syllabus for Class 10 Maths 2024-25 - Download Free PDF

NCERT Solutions for Exercise 12.3 Class 10 Maths Chapter 12 - Areas related to circles

NCERT Solutions for Exercise 12.2 Class 10 Maths Chapter 12 - Areas related to circles

NCERT Solutions for Exercise 12.1 Class 10 Maths Chapter 12 - Areas related to circles

NCERT Solutions for Class 10 Science Chapter 6 Life Processes

NCERT Solutions For Class 10 Maths Chapter 4 Quadratic Equations

NCERT Exemplar Class 10 Maths Solutions - Chapter Wise Problems with Solutions

NCERT Exemplar Class 10 Science Solutions Chapter 1 Chemical Reactions and Equations

NCERT Exemplar Class 10 Science Solutions - Chapter Wise Problems with Solutions

Articles

NRTS Registrations 2024: NRTS Scholarship Login, Application Window Open

Dec 13, 2024

NRTS Result 2024 Declared: Check National Rural Talent Scholarship Exam Result Link

Dec 13, 2024

NRTS Admit Card 2024 OUT: Download National Rural Talent Scholarship Exam Admit Card Here

Dec 13, 2024

Sainik School Chhingchhip Admission 2024-25: Apply for AISSEE Class 6 & 9

Dec 13, 2024

Class 10 Science Weightage 2024-25 Blueprint by CBSE, Chapter-Wise Weightage, Marking Scheme

Dec 09, 2024

CBSE Date Sheet 2025 Class 10th OUT; Download CBSE 10th Exam Time Table PDF

Dec 06, 2024

Class 10 Social Science Blueprint 2025 by CBSE: Chapter-Wise Weightage & Exam Pattern

Dec 05, 2024

CBSE Class 10 Toppers Answer Sheet 2025 - Check Here

Nov 29, 2024

Upcoming School Exams

National Institute of Open Schooling 10th examination

Application Date:21 November,2024 - 20 December,2024

Application Process

Exam Pattern

Mock Test

National Institute of Open Schooling 12th Examination

Application Date:21 November,2024 - 20 December,2024

Application Process

Exam Pattern

Admit Card

National Means Cum-Merit Scholarship

Admit Card Date:27 November,2024 - 22 December,2024

Eligibility Criteria

Application Process

Exam Pattern

Himachal Pradesh Board of Secondary Education 10th Examination

Application Date:02 December,2024 - 16 December,2024

Exam Pattern

Admit Card

Result

Himachal Pradesh Board of Secondary Education 12th Examination

Application Date:02 December,2024 - 16 December,2024

Result

Exam Pattern

View All School Exams

Certifications By Top Providers

My SQL Basics

Via Great Learning

Full-Stack Web Development

Via Masai School

Digital Banking Business Model

Via State Bank of India

Business Analytics Foundations

Via PW Skills

Full-Stack Android Development

Via Masai School

Data Science for Beginners

Via Board Infinity

1115 courses

816 courses

394 courses

295 courses

Harvard University, Cambridge

98 courses

University of Michigan, Ann Arbor

83 courses

IT & Software

Teaching and Academics

Programming and Development

Health, Fitness and Medicine

Business and Management

Engineering and Architecture

General Management

Financial Management

Environmental Studies

History

Psychology

Artificial Intelligence

Explore Top Universities Across Globe

University of Essex, Colchester

Wivenhoe Park Colchester CO4 3SQ

University College London, London

Gower Street, London, WC1E 6BT

The University of Edinburgh, Edinburgh

Old College, South Bridge, Edinburgh, Post Code EH8 9YL

University of Bristol, Bristol

Beacon House, Queens Road, Bristol, BS8 1QU

University of Delaware, Newark

Newark, DE 19716

University of Nottingham, Nottingham

University Park, Nottingham NG7 2RD

PTE Exam Pattern 2024: Format, Structure, Sections, Marking Scheme

13 min ⋆ Oct 14, 2024 09:10 AM IST

Cheapest Universities in California for International Students 2024

7 min ⋆ Sep 04, 2024 11:09 AM IST

Cheapest and Affordable Universities in France for International Students in 2025

10 min ⋆ Dec 13, 2024 12:12 PM IST

Benefits of Studying in Ireland for International Students in 2025

9 min ⋆ Dec 13, 2024 12:12 PM IST

Best Pre Law Schools in the World in 2025

3 min ⋆ Dec 13, 2024 10:12 AM IST

IELTS Result 2024 (December 13): Direct Link, Band, Exam Scorecard, How to Check

12 min ⋆ Dec 13, 2024 07:12 AM IST

Related E-books & Sample Papers

CBSE Class 10 Social Science Sample Paper with Solution 2024-25 PDF

496+ Downloads

CBSE Class 10 Mathematics (Standard) Sample Paper with Solution 2024-25 PDF

303+ Downloads

CBSE Class 10 Mathematics (Basic) Sample Paper with Solution 2024-25 PDF

165+ Downloads

CBSE Class 10 Hindi B Sample Paper with Solution 2024-25 PDF

74+ Downloads

CBSE Class 10 Hindi A Sample Paper with Solution 2024-25 PDF

42+ Downloads

CBSE Class 10 English (Language & Literature) Sample Paper with Solution 2024-25 PDF

63+ Downloads

Questions related to CBSE Class 10th

Have a question related to CBSE Class 10th ?

sadhu ashram ramgart road aligarh

If you're looking for directions or steps to reach Sadhu Ashram on Ramgart Road in Aligarh, here’s how you can get there:

Steps to Reach Sadhu Ashram, Ramgart Road, Aligarh:

Starting Point:
- Determine your starting point in Aligarh or the nearby area.
Use Google Maps:
- Open Google Maps on your phone or computer.
- Enter "Sadhu Ashram, Ramgart Road, Aligarh" as your destination.
- Follow the navigation instructions provided by Google Maps.
By Local Transport:
- Auto-rickshaw/Taxi: Hire an auto-rickshaw or taxi and inform the driver about your destination. Most local drivers should be familiar with Sadhu Ashram.
- Bus: Check if there are any local buses that operate on Ramgart Road. Ask the bus conductor if the bus stops near Sadhu Ashram.
Landmarks to Look For:
- As you approach Ramgart Road, look for local landmarks that might help you confirm you’re on the right path, such as known shops, temples, or schools nearby.
Ask for Directions:
- If you're unsure, ask locals for directions to Sadhu Ashram on Ramgart Road. It's a known location in the area.
Final Destination:
- Once you reach Ramgart Road, Sadhu Ashram should be easy to spot. Look for any signage or ask nearby people to guide you to the exact location.

If you need detailed directions from a specific location or more information about Sadhu Ashram, feel free to ask

Read Complete Answer

sai raja 01 September,2024

show the previous year exams ?

Hello Aspirant, Hope your doing great, your question was incomplete and regarding what exam your asking.

Read Complete Answer

SWETCHCHA MISKA 10 July,2024

as an icse student in class 10 is above 80 percent good enough to get into commerce in 11th cbse

Yes, scoring above 80% in ICSE Class 10 exams typically meets the requirements to get into the Commerce stream in Class 11th under the CBSE board . Admission criteria can vary between schools, so it is advisable to check the specific requirements of the intended CBSE school. Generally, a good academic record with a score above 80% in ICSE 10th result is considered strong for such transitions.

Read Complete Answer

Manasvini Gupta 23 July,2024

i had cleared 10th from cbse in 2022 2 years ago now i wish to apply for 12th as private candidate in 2025. can i do so?

hello Zaid,

Yes, you can apply for 12th grade as a private candidate .You will need to follow the registration process and fulfill the eligibility criteria set by CBSE for private candidates.If you haven't given the 11th grade exam ,you would be able to appear for the 12th exam directly without having passed 11th grade. you will need to give certain tests in the school you are getting addmission to prove your eligibilty.

best of luck!

Read Complete Answer

Ashvadha 03 July,2024

i had cleared 10th in 2022 from cbse can i apply for 12th as private candidate this year?

According to cbse norms candidates who have completed class 10th, class 11th, have a gap year or have failed class 12th can appear for admission in 12th class.for admission in cbse board you need to clear your 11th class first and you must have studied from CBSE board or any other recognized and equivalent board/school.

You are not eligible for cbse board but you can still do 12th from nios which allow candidates to take admission in 12th class as a private student without completing 11th.

Read Complete Answer

sadafreen356 03 July,2024

View All

Stay up-to date with CBSE Class 10th News

Download Careers360 App

All this at the convenience of your phone

Regular Exam Updates
Best College Recommendations
College & Rank predictors
Detailed Books and Sample Papers
Question and Answers

Scan and download the app

Central Board of Secondary Education Class 10th Examination

Applications Open

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

Option 1) less than 3	Option 2) more than 3 but less than 6
Option 3) more than 6 but less than 9	Option 4) more than 9

NCERT Solutions for Exercise 10.2 Class 10 Maths Chapter 10 - Circles

NCERT Solutions For Class 10 Maths Chapter 10 Exercise 10.2

Pearson | PTE

Download Free Pdf of NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2

Assess NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

Answer:

More About NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2

Benefits of NCERT Solutions for Class 10 Maths Chapter 10 Exercise 10.2

NCERT Solutions Subject Wise

Frequently Asked Questions (FAQs)

Also Read

Articles

Upcoming School Exams

Certifications By Top Providers

Explore Top Universities Across Globe

Related E-books & Sample Papers

Questions related to CBSE Class 10th

Steps to Reach Sadhu Ashram, Ramgart Road, Aligarh:

Popular CBSE Class 10th Questions

Colleges After 12th

JEE Main Important Physics formulas

JEE Main Important Chemistry formulas

TOEFL ® Registrations 2024

Pearson | PTE

JEE Main high scoring chapters and topics

JEE Main Important Mathematics Formulas

Stay up-to date with CBSE Class 10th News

Explore on Careers360

NCERT Solutions

NCERT Exemplars

NCERT Books

NCERT Notes

CBSE

CBSE Class 10

CBSE Class 12th

CISCE Board 10th

IMO

IEO

NSO

NMMS

NVS

JNVST

Schools By Medium

By Ownership

By City

By State

Download Careers360 App

All this at the convenience of your phone

Scan and download the app