NCERT Exemplar Class 11 Maths solutions Chapter 9 Sequence and Series

NCERT Exemplar Class 11 Maths Solutions Chapter 9 Sequence and Series

Answer:

The sum of the first p terms and the first term is given. We have to find the next q terms. So, the total terms become p+q.
Hence, the sum of all terms minus the sum of the first p terms will give the sum of the next q terms. However, the sum of the first p terms is zero, so the sum of the next q terms will be the same as the sum of all terms.
Sum of n terms is given by $S_{n}= \left( \frac{n}{2} \right) \left( 2a+ \left( n-1 \right) d \right)$
$\\ \text { required sum }=S_{p+q}-S_{p}=\frac{p+q}{2}(2 a+(p+q-1) d) \ldots \ldots(i) \\ S_{p}=\frac{p}{2}(2 a+(p-1) d \\ 0=2 a+(p-1) d \\ d=-\frac{2 a}{p-1}$

Replacing the value of d in equation (i)

$\\ \text {required sum}=\frac{p+q}{2}\left(2 a+(p+q-1)\left(-\frac{2 a}{p-1}\right)\right. \\ =\frac{p+q}{2}\left(2 a-\frac{2 a p+2 a q-2 a}{p-1}\right) \\ =\frac{p+q}{2}(2 a)\left(1-\frac{p-1}{p-1}-\frac{q}{p-1}\right) \\ \text {On simplifying,} \\ =a(p+q)\left(-\frac{q}{p-1}\right)=-\frac{a(p+q) q}{p-1}$

Question 2: A man saved Rs. 66000 in 20 years. In each succeeding year after the first year, he saved Rs 200 more than what he saved in the previous year. How much did he save in the first year?

Answer:

Total amount saved in 20 years is $S_{20}=66000$
Let the amount saved in the first year be ‘a.’
Since he increases by 200 Rs every year, d=200
Sum formula of A.P is $S_{n}= \left( \frac{n}{2} \right) \left( 2a+ \left( n-1 \right) d \right)$

$S_{20}= \left( \frac{20}{2} \right) \left( 2a+ \left( 20-1 \right) 200 \right)$
$66000=10 \left( 2a+3800 \right)$
$a=1400$

Question 3: A man accepts a position with an initial salary of Rs 5200 per month. It is understood that he will receive an automatic increase of Rs 320 in the very next month and each month thereafter.
(a) Find his salary for the tenth month
(b) What is his total earnings during the first year?

Answer:

The man’s salary in the first month is Rs. 5200 and increases every month by Rs. 320. So, we have the value of ‘a’ and ‘d’.

$t_{n}=a+ \left( n-1 \right) d$

Salary in 10 th month is given by $t_{10}=5200+ \left( 10-1 \right) 320=8080$

For total earnings in the first year, the first 12 terms of the sequence are added.

$S_{n}= \left( \frac{n}{2} \right) \left( 2a+ \left( n-1 \right) d \right)$

$S_{12}= \left( \frac{12}{2} \right) \left( 2 \left( 5200 \right) + \left( 12-1 \right) 320 \right)$

$S_{12}=6(10400+11(320)=83520$

Question 4: If the $p^{\text {th }}$ and $q^{\text {th }}$ terms of a G.P. are q and p respectively, show that its $(p+q)^{\text {th }}$ term is $\left(\frac{q^p}{p^4}\right)^{\frac{1}{p-8}}$.

Answer:

The nth term is given by $t_{n}=ar^{n-1}$
The pth term is given by $t_{p}=ar^{p-1}$
$q=ar^{p-1}$
$\frac{q}{r^{p}}=\frac{a}{r}$
$t_{q}=ar^{q-1}$
$p=ar^{q-1}$
$\\ \frac{p}{r^{q}}=\frac{a}{r}\\\\ \frac{p}{r^{q}}=\frac{q}{r^{p}}\\\\ r^{p-q}=\frac{q}{p}\\$
$r= \left( \frac{q}{p} \right) ^{\frac{1}{p-q}}$
$t_{p+q}=a \left( r \right) ^{p+q-1}$
$t_{p+q}= \left( ar^{p-1} \right) r^{q} \\\\ t_{p+q}=qr^{q} \\\\ t_{p+q}=q \left( \left( \frac{q}{p} \right) ^{\frac{1}{p-q}} \right) ^{q} \\\\$
$t_{p+q}=q \left( \frac{q^{\frac{q}{p-q}}}{p^{\frac{q}{p-q}}} \right) = \left( \frac{q^{1+\frac{q}{p-q}}}{p^{\frac{q}{p-q}}} \right) = \left( \frac{q^{\frac{p}{p-q}}}{p^{\frac{q}{p-q}}} \right) = \left( \frac{q^{p}}{p^{q}} \right) ^{\frac{1}{p-q}} \\\\$.

Question 5

A carpenter was hired to build 192 window frames. The first day he made five frames, and each day, thereafter, he made two more frames than he made the day before. How many days did it take him to finish the job?

Answer:

The first day he made 5 frames and 2 more frames each passing day. So,
a=5 and d=2
$S_{n}= \left( \frac{n}{2} \right) \left( 2a+ \left( n-1 \right) d \right)$

Let us assume that it takes ‘n’ days to build 192 window frames.

$\\ S_{n}= \left( \frac{n}{2} \right) \left( 10+ \left( n-1 \right) 2 \right) \\\\ 192= \left( \frac{n}{2} \right) \left( 8+2n \right) \\\\ 384=2n^{2}+8n \\\\ n^{2}+4n-192=0 \\\\ \left( n-12 \right) \left( n+16 \right) =0 \\\\$

Since the number of days can’t be negative, n = 12.

Question 6:

We know the sum of the interior angles of a triangle is 180°. Show that the sums of the interior angles of polygons with 3, 4, 5, 6, ... sides form an arithmetic progression. Find the sum of the interior angles for a 21-sided polygon.

Answer:

The sum of interior angles of a polygon having 'n' sides is given by $\left( n-2 \right)\times 180^{\circ}$
By this formula sum of angles with 3 sides, 4 sides, 5 sides, and 6 sides is $180^{\circ},~360^{\circ},~540^{\circ},720^{\circ}$ respectively. As the number of sides increases by 1, the sum of the interior angles increases by $180^{\circ}$
So, for the sum of angles of a polygon with 21 sides $= \left( n-2 \right) \times180^{\circ}~ \\\\$
$\\ = \left( 21-2 \right) \times 180^{\circ}=19\times 180^{\circ}~ \\\\ =3420^{\circ} \\\\$

Question 7

A side of an equilateral triangle is 20cm long. A second equilateral triangle is inscribed in it by joining the midpoints of the sides of the first triangle. The process is continued as shown in the accompanying diagram. Find the perimeter of the sixth inscribed equilateral triangle.

Answer:

Let AB=BC=AC=20cm
Let D, E, and F be midpoints of AC, CB, and AB, which are joined to form an equilateral triangle DEF. So, CD=CE=10cm. Triangle CDE is equilateral. Hence, DE = 10 cm. Similarly, GH = 5 cm
The series of sides of an equilateral triangle will be 20,10,5…..
The series is a G.P. with first term 20 and common ratio =1/2
$t_{n}=20 \left( \frac{1}{2} \right) ^{n-1}$

For the perimeter of the 6 th triangle, we first have to find the side of the 6 th triangle

$t_{6}=\frac{20}{2^{6-1}}=\frac{20}{2^{5}}=\frac{5}{8}cm \\\\$

The perimeter would be thrice the length of its side.

Perimeter of triangle= $3 \left( \frac{5}{8} \right) =\frac{15}{8}cm \\\\$

Question 8

In a potato race, 20 potatoes are placed in a line at intervals of 4 meters with the first potato 24 meters from the starting point. A contestant is required to bring the potatoes back to the starting place one at a time. How far would he run in bringing back all the potatoes?

Answer:

It is given that at the start, he has to run 24m to get the first potato, then 28 m as the next potato is 4m away, and so on.
Hence, the sequence of its running will be 24, 28, 32…..
To get potatoes from the starting point, he has to run 24+28+32+….. up to 20 terms.
$\\S_{n}= \left( \frac{n}{2} \right) \left( 2a+ \left( n-1 \right) d \right) \\\\ S_{20}= \left( \frac{20}{2} \right) \left( 2 \left( 24 \right) + \left( 20-1 \right) 4 \right) \\\\ S_{20}=10 \left( 48+76 \right) \\\\ S_{20}=1240m \\\\$

Also, he has to get the potato back to the starting point, hence the total distance will be twice.
Total Distance ran=2×1240=2480m

Question 9

In a cricket tournament, 16 school teams participated. A sum of Rs 8000 is to be awarded among themselves as prize money. If the last-placed team is awarded Rs 275 in prize money and the award increases by the same amount for successive finishing places, how much amount will the first-place team receive?

Answer:

Let the amount received by the first place be Rs. A and ‘d’ are the differences in the amount. The last team receives Rs. 275. As there are 16 teams and all teams are given prizes hence the sequence will have 16 terms because there are 16 teams.

As the total prize given is Rs 8000, hence

$a+a-d+a-2d....+275 =8000$

$ n=16$
$S_{16}=8000$
$ S_{n}= \left( \frac{n}{2} \right) \left( 2a+ \left( n-1 \right) (-d) \right)$
$8000= \left( \frac{16}{2} \right) \left( 2a+ \left( 16-1 \right) (-d) \right) =8 \left( 2a-15d \right)$
$1000=2a-15d \\\\ t_{16}=275$
$t_{16}=a+ \left( 16-1 \right) (-d)$
$275=a-15d$
$1000-275= \left( 2a-15d \right) - \left( a-15d \right)$
$ a=725$

Question 10 If $a_1, a_2, a_3, \ldots, a_{\mathrm{n}}$ are in A.P., where $a_{\mathrm{i}}>0$ for all i , show that $\frac{1}{\sqrt{a_1}+\sqrt{a_2}}+\frac{1}{\sqrt{a_2}+\sqrt{a_3}}+\cdots+\frac{1}{\sqrt{a_{n-1}}+\sqrt{a_n}}=\frac{n-1}{\sqrt{a_1}+\sqrt{a_n}}$

Answer:

$LHS=\frac{1}{\sqrt[]{a_{1}}+\sqrt[]{a_{2}}}+\frac{1}{\sqrt[]{a_{2}}+\sqrt[]{a_{3}}}+ \ldots +\frac{1}{\sqrt[]{a_{n-1}}+\sqrt[]{a_{n}}} \\\\$
$\begin{aligned} &\text {Multiplying the first term by } \frac{\sqrt{a_{1}}-\sqrt{a_{2}}}{\sqrt{a_{1}}-\sqrt{a_{2}}}, \text { the second term by } \frac{\sqrt{a_{2}}-\sqrt{a_{3}}}{\sqrt{a_{2}}-\sqrt{a_{3}}}\\ &\text {and so on rationalising each term} \end{aligned}$

$LHS= \left( \frac{1}{\sqrt[]{a_{1}}+\sqrt[]{a_{2}}} \right) \left( \frac{\sqrt[]{a_{1}}-\sqrt[]{a_{2}}}{\sqrt[]{a_{1}}-\sqrt[]{a_{2}}} \right) + \left( \frac{1}{\sqrt[]{a_{2}}+\sqrt[]{a_{3}}} \right) \left( \frac{\sqrt[]{a_{2}}-\sqrt[]{a_{3}}}{\sqrt[]{a_{2}}-\sqrt[]{a_{3}}} \right) + \ldots + \left( \frac{1}{\sqrt[]{a_{n-1}}+\sqrt[]{a_{n}}} \right) \left( \frac{\sqrt[]{a_{n-1}}-\sqrt[]{a_{n}}}{\sqrt[]{a_{n-1}}-\sqrt[]{a_{n}}} \right) \\\\$
$\\= \left( \frac{\sqrt[]{a_{1}}-\sqrt[]{a_{2}}}{a_{1}-a_{2}} \right) + \left( \frac{\sqrt[]{a_{2}}-\sqrt[]{a_{3}}}{a_{2}-a_{3}} \right) + \ldots + \left( \frac{\sqrt[]{a_{n-1}}-\sqrt[]{a_{n}}}{a_{n-1}-a_{n}} \right) \\\\ = \left( \frac{\sqrt[]{a_{1}}-\sqrt[]{a_{2}}}{-d} \right) + \left( \frac{\sqrt[]{a_{2}}-\sqrt[]{a_{3}}}{-d} \right) + \ldots + \left( \frac{\sqrt[]{a_{n-1}}-\sqrt[]{a_{n}}}{-d} \right) \\\\$

$\\ =-\frac{1}{d} \left( \sqrt[]{a_{1}}-\sqrt[]{a_{2}}+\sqrt[]{a_{2}}-\sqrt[]{a_{3}}+ \ldots +\sqrt[]{a_{n-1}}-\sqrt[]{a_{n}} \right) \\\\ =-\frac{1}{d} \left( \sqrt[]{a_{1}}-\sqrt[]{a_{n}} \right) ~ \\\\$

Rationalising again,

$\\ =-\frac{1}{d} \left( \sqrt[]{a_{1}}-\sqrt[]{a_{n}} \right) \left( \frac{ \left( \sqrt[]{a_{1}}+\sqrt[]{a_{n}} \right) }{ \left( \sqrt[]{a_{1}}+\sqrt[]{a_{n}} \right) } \right) \\\\ =-\frac{1}{d}\frac{ \left( a_{1}-a_{n} \right) }{ \left( \sqrt[]{a_{1}}+\sqrt[]{a_{n}} \right) }=-\frac{1}{d}\frac{ \left( - \left( n-1 \right) d \right) }{ \left( \sqrt[]{a_{1}}+\sqrt[]{a_{n}} \right) }=\frac{ \left( n-1 \right) }{ \left( \sqrt[]{a_{1}}+\sqrt[]{a_{n}} \right) } \\\\$

Question 11 Find the sum of the series $\left(3^3-2^3\right)+\left(5^3-4^3\right)+\left(7^3-6^3\right)+\ldots$ to

(i) n terms

(ii) 10 terms

Answer:

Generalising the series in terms of i
$\\ S= \sum _{i=1}^{n} \left[ \left( 2i+1 \right) ^{3}- \left( 2i \right) ^{3} \right] $
$ = \sum _{i=1}^{n} \left[ \left( 2i+1-2i \right) \left( \left( 2i+1 \right) ^{2}+ \left( 2i+1 \right) \left( 2i \right) + \left( 2i \right) ^{2} \right) \right] $
$ = \sum _{i=1}^{n} \left[ 4i^{2}+4i+1+4i^{2}+2i+4i^{2} \right] = \sum _{i=1}^{n} \left[ 12i^{2}+6i+1 \right] \\\\$
$\\ =12 \left[ \frac{n \left( n+1 \right) \left( 2n+1 \right) }{6} \right] +6 \left[ \frac{n \left( n+1 \right) }{2} \right] + \left[ n \right] \\\\ =2 \left[ 2n^{3}+3n^{2}+n \right] +3 \left[ n^{2}+n \right] + \left[ n \right] \\\\$
Sum up to n terms $=4n^{3}+9n^{2}+6n \\\\$
$\\ \text{Sum up to 10 terms }S_{10}=4 \left( 10 \right) ^{3}+9 \left( 10 \right) ^{2}+6 \left( 10 \right) =4960 \\\\$

Question 12: Find the rth term of an A.P. sum of whose first n terms is $2 n+3 n^2$.

$\left[\right.$ Hint : $\left.a_{\mathrm{n}}=S_{\mathrm{n}}-S_{\mathrm{n}-1}\right]$

Answer:

The sum of the first n terms is given by $S_n=2n + 3n^2$
The rth term is
$\\a_{r}=S_{r}-S_{r-1}= \left( 2r+3r^{2} \right) - \left( 2 \left( r-1 \right) +3 \left( r-1 \right) ^{2} \right) \\\\ =2r+3r^{2}- \left( 2r-2+3r^{2}-6r+3 \right) \\\\ =2r+3r^{2}- \left( 3r^{2}-4r+1 \right) \\\\ =6r-1 \\\\$

Question 13: If A is the arithmetic mean and $G_1$, and $G_2$ are two geometric means between any two numbers, then prove that.
$2\mathrm{A}=\frac{\mathrm{G}_{1}^{2}}{\mathrm{G}_{2}}+\frac{\mathrm{G}_{2}^{2}}{\mathrm{G}_{1}}$

Answer:

It is given that A is the arithmetic mean and $G_1$, and $G_2$ are two geometric means between any two numbers.
$\\ A=\frac{a+b}{2} \\\\ G=\sqrt {ab} \\\\ G_{1}=\sqrt {aG_{2}} \\\\ G_{2}=\sqrt {G_{1}b} \\\\ G_{1}^{2}=aG_{2} \\\\$
$\\ G_{1}^{2}=a\sqrt {G_{1}b} \\\\ G_{1}^{4}=a^{2}G_{1}b \\\\ G_{1}^{3}=a^{2}b \\\\ G_{1}=a^{\frac{2}{3}}b^{\frac{1}{3}} \\\\$
$\\ G_{2}= \left( a^{\frac{2}{3}}b^{\frac{1}{3}} \times b \right) ^{\frac{1}{2}}=a^{\frac{1}{3}}b^{\frac{2}{3}}~ \\\\ \frac{G_{1}^{2}}{G_{2}}= \left( \frac{ \left( a^{\frac{2}{3}}b^{\frac{1}{3}} \right) ^{2}}{a^{\frac{1}{3}}b^{\frac{2}{3}}} \right) =a \\\\ \frac{G_{2}^{2}}{G_{1}}= \left( \frac{ \left( b^{\frac{2}{3}}a^{\frac{1}{3}} \right) ^{2}}{b^{\frac{1}{3}}a^{\frac{2}{3}}} \right) =b \\\\ \frac{G_{1}^{2}}{G_{2}}+\frac{G_{2}^{2}}{G_{1}}=a+b=2A \\\\$

Question 14: If $\theta_1, \theta_2, \theta_3, \ldots, \theta_{\mathrm{n}}$ are in A.P., whose common difference is d, show that $\sec \theta_1 \sec \theta_2+\sec \theta_2 \sec \theta_3+\ldots+\sec \theta_{\mathrm{n}-1} \sec \theta_{\mathrm{n}}$ $=\frac{\tan \theta_{\mathrm{n}}-\tan \theta_1}{\sin \mathrm{~d}}$

Answer:

Given that $\theta _{1}, \theta _{2}, \ldots \theta _{n}\text{~are in A.P and common difference is d. } \\\\$

$\\ \sec \theta _{1}\sec \theta _{2}+\sec \theta _{2}\sec \theta _{3}+ \ldots +\sec \theta _{n-1}\sec \theta _{n} \\\\ =\frac{1}{\cos \theta _{1}\cos \theta _{2}}+\frac{1}{\cos \theta _{2}\cos \theta _{3}}+ \ldots +\frac{1}{\cos \theta _{n-1}\cos \theta _{n}} \\\\ =\frac{\sin d}{\sin d} \left( \frac{1}{\cos \theta _{1}\cos \theta _{2}}+\frac{1}{\cos \theta _{2}\cos \theta _{3}}+ \ldots +\frac{1}{\cos \theta _{n-1}\cos \theta _{n}} \right) \\\\$
$\\ =\frac{1}{\sin d} \left( \frac{\sin \left( \theta _{2}- \theta _{1} \right) }{\cos \theta _{1}\cos \theta _{2}}+\frac{\sin \left( \theta _{3}- \theta _{2} \right) }{\cos \theta _{2}\cos \theta _{3}}+ \ldots +\frac{\sin \left( \theta _{n}- \theta _{n-1} \right) }{\cos \theta _{n-1}\cos \theta _{n}} \right) \\\\ =\frac{1}{\sin d} \left( \frac{\sin \theta _{2}\cos \theta _{1}-\cos \theta _{2}\sin \theta _{1}}{\cos \theta _{1}\cos \theta _{2}}+\frac{\sin \theta _{3}\cos \theta _{2}-\cos \theta _{3}\sin \theta _{2}}{\cos \theta _{2}\cos \theta _{3}}+ \ldots +\frac{\sin \theta _{n}\cos \theta _{n-1}-\cos \theta _{n}\sin \theta _{n-1}}{\cos \theta _{n-1}\cos \theta _{n}} \right) \\\\$
$\\=\frac{1}{\sin d} \left( \frac{\sin \theta _{2}\cos \theta _{1}}{\cos \theta _{1}\cos \theta _{2}}-\frac{\sin \theta _{1}\cos \theta _{2}}{\cos \theta _{1}\cos \theta _{2}}+\frac{\sin \theta _{3}\cos \theta _{2}}{\cos \theta _{2}\cos \theta _{3}}-\frac{\sin \theta _{2}\cos \theta _{3}}{\cos \theta _{2}\cos \theta _{3}}+ \ldots +\frac{\sin \theta _{n}\cos \theta _{n-1}}{\cos \theta _{n-1}\cos \theta _{n}}-\frac{\sin \theta _{n}\cos \theta _{n-1}}{\cos \theta _{n-1}\cos \theta _{n}} \right) $
$ =\frac{1}{\sin d} \left( \tan \theta _{2}-\tan \theta _{1}+\tan \theta _{3}-\tan \theta _{2}+ \ldots +\tan \theta _{n}-\tan \theta _{n-1} \right) \\\\$

$\\ =\frac{ \left( \tan \theta _{n}-\tan \theta _{1} \right) }{\sin d} \\\\$

Question 15: If the sum of p terms of an A.P. is q and the sum of q terms is p, show that the sum of p + q terms is - (p + q). Also, find the sum of the first p - q terms (p > q).

Answer:

The sum of n terms is given by
$S_{n}= \left( \frac{n}{2} \right) \left( 2a+ \left( n-1 \right) d \right) \\\\$
Where ‘a’ is the first term and ’d’ is the common difference

$\\ S_{p}=q \\\\ q=\frac{p}{2} \left( 2a+ \left( p-1 \right) d \right) \\\\ \frac{2q}{p}=2a+ \left( p-1 \right) d \\\\ S_{q}=p \\\\ p=\frac{q}{2} \left( 2a+ \left( q-1 \right) d \right) \\\\$
$\\ \frac{2p}{q}=2a+ \left( q-1 \right) d \\\\ \frac{2p}{q}-\frac{2q}{p}= \left( q-1 \right) d- \left( p-1 \right) d \\\\ \frac{2p}{q}-\frac{2q}{p}= \left( q-p \right) d \\\\ d=\frac{2p^{2}-2q^{2}}{pq \left( q-p \right) }=-\frac{2 \left( p+q \right) }{pq} \\\\$
$\\ S_{p+q}=\frac{p+q}{2} \left( 2a+ \left( p+q-1 \right) d \right) \\\\ =\frac{p}{2} \left( 2a+ \left( p-1 \right) d+qd \right) +\frac{q}{2} \left( 2a+ \left( q-1 \right) d+pd \right) \\\\$
$\\ =\frac{p}{2} \left( 2a+ \left( p-1 \right) d \right) +\frac{pqd}{2}+\frac{q}{2} \left( 2a+ \left( q-1 \right) d \right) +\frac{pqd}{2} \\\\ =q+p+pqd \\\\$
$\\ =q+p+pq \left( -\frac{2 \left( p+q \right) }{pq} \right) =q+p-2p-2q \\\\ S_{p+q}=- \left( p+q \right) \\\\ S_{p-q}=\frac{p-q}{2} \left( 2a+ \left( p-q-1 \right) d \right) \\\\$
$\\ =\frac{p}{2} \left( 2a+ \left( p-1 \right) d \right) -\frac{pqd}{2}-\frac{q}{2} \left( 2a+ \left( p-1 \right) d \right) +\frac{q^{2}d}{2} $
$ =q-\frac{pqd}{2}- \left( \frac{q}{p} \right) \left( \frac{p}{2} \right) \left( 2a+ \left( p-1 \right) d \right) +\frac{q^{2}d}{2} $
$ =q-\frac{q^{2}}{p}-\frac{pqd}{2}+\frac{q^{2}d}{2} $
$ =\frac{ \left( pq-q^{2} \right) }{p}+\frac{d \left( q^{2}-pq \right) }{2} $
$ =\frac{pq-q^{2}}{p}-\frac{pq-q^{2}}{2} \left( -\frac{2 \left( p+q \right) }{pq} \right) \\\\$
$\\ =\frac{pq-q^{2}}{p}+ \left( pq-q^{2} \right) \left[ \frac{1}{q}+\frac{1}{p} \right] \\\\ =\frac{2 \left( pq-q^{2} \right) }{p}+\frac{pq-q^{2}}{q} \\\\ =\frac{2q \left( p-q \right) }{p}+p-q \\\\$

Question 16: If $p^{t h}, q^{\text {th }}$, and $r^{\text {th }}$ terms of an A.P. and G.P. are both $\mathbf{a}, \mathbf{b}$ and $\mathbf{c}$ respectively, show that $a^{\mathrm{b}-\mathrm{c}} \cdot b^{\mathrm{c}-\mathrm{a}} \cdot c^{\mathrm{a}-\mathrm{b}}=1$

Answer:

Let the first term of AP be m and the common difference as d. Let the first term of GP be I and the common ratio be s.

For AP

$\\ t_{p}=m+ \left( p-1 \right) d \\\\ a=m+ \left( p-1 \right) d \\\\ b=m+ \left( q-1 \right) d \\\\ c=m+ \left( r-1 \right) d \\\\$

For GP
$\\ t_{p}=Is^{p-1} \\\\ a=Is^{p-1} \\\\ b=Is^{q-1} \\\\ c=Is^{r-1} \\\\ b-c= \left( q-r \right) d \\\\$
$\\ c-a= \left( r-p \right) d \\\\ a-b= \left( p-q \right) d \\\\ LHS=a^{b-c}b^{c-a}c^{a-b} \\\\ = \left( Is^{p-1} \right) ^{ \left( q-r \right) d} \left( Is^{q-1} \right) ^{ \left( r-p \right) d} \left( Is^{r-1} \right) ^{ \left( p-q \right) d} \\\\ =I^{ \left( q-r+r-p+p-q \right) d}s^{ \left[ \left( pq-pr-q+r \right) + \left( qr-qp-r+p \right) + \left( rp-rq-p+q \right) \right] d} \\\\ =I^{0}s^{0}=1 \\\\$

Question 17: If the sum of $\boldsymbol{n}$ terms of an A.P. is given by $S_n=3 n+2 n^2$, then the common difference of the A.P. is

(A) 3 (B) 2 (C) 6 (D) 4

Answer:

Given that $S_{n}=3n+2n^{2}$
We know that $a_{2}=S_{2}-S_{1}$

$\\ = \left( 3 \left( 2 \right) +2 \left( 2 \right) ^{2} \right) - \left( 3 \left( 1 \right) +2 \left( 1 \right) ^{2} \right) \\\\ =14-5 \\\\ =9 \\\\ a_{1}=S_{1}=3 \left( 1 \right) +2 \left( 1 \right) ^{2}=5 \\\\ d=a_{2}-a_{1}=9-5=4 \\\\$

Hence, the correct option is d.

Question 18: The third term of G.P. is 4. The product of its first 5 terms is
(A) 43 (B) 44 (C) 45 (D) None of these

Answer:

The third term of G.P is given $T_{3}=4$

$\\ T_{n}=ar^{n-1} \\\\ ar^{2}=4 \\\\$
Product of the first 5 terms

$\\= \left( a \right) \left( ar \right) \left( ar^{2} \right) \left( ar^{3} \right) \left( ar^{4} \right) \\\\ = \left( a^{5}r^{10} \right) = \left( ar^{2} \right) ^{5} \\\\ =4^{5}=1024 \\\\$

Hence, the correct option is c.

Question 19:

If 9 times the 9th term of an A.P. is equal to 13 times the 13th term, then the 22nd term of the A.P. is A. 0 B. 22 C. 220 D. 198

Answer:

$\\ 9a_{9}=13a_{13} \\\\ 9 \left( a+ \left( 9-1 \right) d \right) =13 \left( a+ \left( 13-1 \right) d \right) \\\\ 9a+72d=13a+156d \\\\ -4a=84d \\\\ a=-21d \\\\ a_{22}=a+ \left( 22-1 \right) d \\\\ =-21d+21d=0 \\\\$

Hence, the correct option is a.

Question 20

If x, 2y, 3z are in A.P., where the distinct numbers x, y, z are in G.P. then the common ratio of the G.P. is A. 3 B. 1/3 C. 2 D. 1/2

Answer:

It is given that x, 2y, and 3z are in A.P

$\\ 2y-x=3z-2y \\\\ x=4y-3z \\\\ \frac{y}{x}=\frac{z}{y} \\\\ y^{2}=xz \\\\ y^{2}= \left( 4y-3z \right) z \\\\ y^{2}-4yz+3z^{2}=0 \\\\ \left( 3z-y \right) \left( z-y \right) =0 \\\\ y=3z \ or\ y=z \\\\ r=\frac{y}{z}=\frac{1}{3} \\\\$

Hence, the correct option is b.

Question 21

If in an A.P., Sn = qn2 and Sm = qm2, where Sr denotes the sum of r terms of the A.P., then Sq equals A. $\frac{q^3}{2}$ B. mnq C. $q^3$ D. $(m + n) q^2$

Answer:

The given series is an A.P. with the first term ‘a’ and common difference ‘d’.

$\\ S_{n}=\frac{n}{2} \left[ 2a+ \left( n-1 \right) d \right] \\\\ qn^{2}=\frac{n}{2} \left[ 2a+ \left( n-1 \right) d \right] \\\\ 2qn=2a+ \left( n-1 \right) d \\\\ 2a=2qn- \left( n-1 \right) d \\\\ S_{m}=\frac{n}{2} \left[ 2a+ \left( m-1 \right) d \right] \\\\ qm^{2}=\frac{m}{2} \left[ 2a+ \left( m-1 \right) d \right] \\\\$

$\\2qm=2a+ \left( m-1 \right) d \\\\ 2a=2qm- \left( m-1 \right) d \\\\ 2qm- \left( m-1 \right) d=2qn- \left( n-1 \right) d \\\\ 2q \left( n-m \right) = \left( n-m \right) d \\\\ d=2q \\\\ 2a=2qn- \left( n-1 \right) \left( 2q \right) \\\\$

$\\a=q \\\\ S_{q}=\frac{q}{2} \left[ 2q+ \left( q-1 \right) 2q \right] =q^{3} \\\\$

Hence, the correct option is c.

Question 22

Let $S_n$ denote the sum of the first n terms of an A.P. If $S_{2 \mathrm{n}}=3 S_{\mathrm{n}}$ then $S_{3 \mathrm{n}}: S_{\mathrm{n}}$ is equal to

A. 4

B. 6

C. 8

D. 10

Answer:

Given $S_{2n}=3S_{n} \\\\$
$\\ S_{n}=\frac{n}{2} \left[ 2a+ \left( n-1 \right) d \right] \\\\ S_{2n}=\frac{2n}{2} \left[ 2a+ \left( 2n-1 \right) d \right] \\\\ S_{2n}=3S_{n} \\\\ n \left[ 2a+ \left( 2n-1 \right) d \right] =\frac{3n}{2} \left[ 2a+ \left( n-1 \right) d \right] \\\\ 4a+4nd-2d=6a+3nd-3d \\\\ 2a=nd+d \\\\$
$\\ S_{3n}=\frac{3n}{2} \left[ 2a+ \left( 3n-1 \right) d \right] =\frac{3n}{2} \left[ \left( n+1 \right) d+ \left( 3n-1 \right) d \right] =6n^{2}d \\\\ S_{n}=\frac{n}{2} \left[ 2a+ \left( n-1 \right) d \right] =\frac{n}{2} \left[ \left( n+1 \right) d+ \left( n-1 \right) d \right] =n^{2}d \\\\ \frac{S_{3n}}{S_{n}}=\frac{6n^{2}d}{n^{2}d}=6 \\\\$

Hence, the correct option is b.

Question 23

The minimum value of $4^{\mathrm{x}}+4^{1-\mathrm{x}}, x \in R$ is

A. 2

B. 4

C. 1

D. 0

Answer:

The AM-GM inequality states that the arithmetic mean of a list of non-negative real numbers is greater than or equal to the geometric mean of the same list

$\\ \frac{x+y}{2} \geq \sqrt {xy} \\\\ \frac{4^{x}+4^{1-x}}{2} \geq \sqrt { \left( 4^{x} \right) \left( 4^{1-x} \right) } \\\\ \frac{4^{x}+4^{1-x}}{2} \geq \sqrt {4} \\\\ 4^{x}+4^{1-x} \geq 4 \\\\$

Hence, the correct option is b.

Question 24 Let $S_n$ denote the sum of the cubes of the first n natural numbers and $s_n$ denote the sum of the first n natural numbers. Then $\sum_{r=1}^n \frac{S_r}{S_r}$ equals.

A. $\frac{\mathrm{n}(\mathrm{n}+1)(\mathrm{n}+2)}{6}$

B. $\frac{\mathrm{n}(\mathrm{n}+1)}{2}$

C. $\frac{\mathrm{n}^2+3 \mathrm{n}+2}{2}$

D. None of these

Answer:

Sum of cubes of first n natural numbers $S_{n}= \sum _{i=1}^{n}i^{3}= \left( \frac{n \left( n+1 \right) }{2} \right) ^{2} \\\\$

Sum of first n natural numbers $s_{n}= \sum _{i=1}^{n}i=\frac{n \left( n+1 \right) }{2} \\\\$
$\\ \sum _{i=1}^{n}\frac{S_{n}}{s_{n}}= \sum _{i=1}^{n}\frac{n^{2}}{2}+\frac{n}{2} \\\\ =\frac{1}{2} \left[ \sum _{i=1}^{n}n^{2}+ \sum _{i=1}^{n}n \right] \\\\ =\frac{1}{2} \left[ \frac{n \left( n+1 \right) \left( 2n+1 \right) }{6}+\frac{n \left( n+1 \right) }{2} \right] \\\\ =\frac{1}{2} \left[ \frac{n \left( n+1 \right) }{2} \left( \frac{2n+1}{3}+1 \right) \right] \\\\$
$\\ =\frac{1}{2}\frac{n \left( n+1 \right) }{2}\frac{ \left( 2n+4 \right) }{3} \\\\ =\frac{n \left( n+1 \right) \left( n+2 \right) }{6} \\\\$

Hence, the correct option is (a).

Question 25

If $t_n$ denotes the $n^{\text {th }}$ term of the series $2+3+6+11+18+\ldots$ then $t_{50}$ is

A. $49^2-1$

B. $49^2$

C. $50^2+1$

D. $49^2+2$

Answer:

$S_{n}=2+3+6+11+18+ \ldots +t_{50}$(Using method of difference)
$\\ S_{n}=0+2+3+6+11+18+ \ldots +t_{50} $
$ S_{n}-S_{n}= \left( 2-0 \right) + \left( 3-2 \right) + \left( 6-3 \right) + \left( 11-6 \right) + \left( 18-11 \right) + \ldots -t_{50} $
$ t_{50}=2+ \left[ 1+3+5+7+ \ldots upto\: \: 49\: \: terms \right] $
$ t_{50}=2+\frac{49}{2} \left[ 2+ \left( 49-1 \right) 2 \right] $
$ t_{50}=2+49^{2} \\\\$
Hence, the correct option is d.

Question 26: The lengths of three unequal edges of a rectangular solid block are in G.P. The volume of the block is $216 \mathrm{~cm}^3$ and the total surface area is $252 \mathrm{~cm}^2$. The length of the longest edge is

A. 12 cm

B. 6 cm

C. 18 cm

D. 3 cm

Answer:

The volume and total S.A of a block are given
Let the length, breadth, and height of a rectangular block be given by $\frac{a}{r}, a,ar$ respectively.

$V=L*B *H=\frac{a}{r} \times a \times ar=a^{3} \\\\$
$\\216=a^{3} \\\\ a=6cm \\\\$
$S=2 \left[ L*B+B*H+H*L \right] =2 \left[ \frac{a}{r} \times a+\frac{a}{r} \times ar+a \times ar \right] =2 \left( \frac{a^{2}}{r}+a^{2}+a^{2}r \right) \\\\$
$\\ 252=2a^{2} \left( \frac{1}{r}+1+r \right) \\\\\frac{252}{72}=\frac{r^{2}+r+1}{r} \\\\ 2r^{2}-5r+2=0 \\\\ \left( 2r-1 \right) \left( r-2 \right) =0 \\\\ r=\frac{1}{2} \ or \ r=2 \\\\$

If a = 6 and r=2 then l,b, and h are $\text{3 cm, 6 cm,12 cm}$ respectively.

If a=6 and r=1/2 then l,b, and h are 12, 6, and 3 respectively.

In both cases, the longest side is 12 units.

Hence, the correct option is a.

Question 27 Fill in the blanks.
For a, b, c to be in G.P. the value of $\frac{a-b}{b-c}$ is equal to ..........

Answer:

It is given that a, b, c are in G.P
$\\ \frac{b}{a}=\frac{c}{b}=r \\\\ \frac{a-b}{b-c}=\frac{\frac{a-b}{b}}{\frac{b-c}{b}}=\frac{\frac{a}{b}-1}{1-\frac{c}{b}}=\frac{\frac{1}{r}-1}{1-r}=\frac{\frac{1-r}{r}}{1-r} \\\\ =\frac{1}{r}=\frac{a}{b}=\frac{b}{c} \\\\$

Question 28 Fill in the blanks:

The sum of terms equidistant from the beginning and end in an A.P. is equal to .............

Answer:

Taking the first and last terms of
$a_{1}+a_{n}=a+a+ \left( n-1 \right) d=2a+ \left( n-1 \right) d$
Taking k-th and (n-k+1) -th term.
$\\ a_{k}+a_{n-k+1}=a+ \left( k-1 \right) d+ \left( a+ \left( n-k+1-1 \right) d \right) \\\\ =2a+ \left( k-1+n-k+1-1 \right) d \\\\ =2a+ \left( n-1 \right) d \\\\ =a_{1}+a_{n} \\\\$

Question 29 Fill in the blanks:

The third term of a G.P. is 4, and the product of the first five terms is ................

Answer:

The third term of a G.P is given by $T_{3}=4$

Let the common ratio be ‘r’

$\\T_{1}=\frac{4}{r^{2}} , T_{2}=\frac{4}{r} , ~T_{4}=4r , T_{5}=4r^{2} \\\\$

Product of first 5 terms $= \left( \frac{4}{r^{2}} \right) \left( \frac{4}{r} \right) \left( 4 \right) \left( 4r \right) \left( 4r^{2} \right) =4^{5}=1024 \\\\$

Question 30 State whether the statement is True or False. Two sequences cannot be in both A.P. and G.P. together.

Answer:

Let the terms of A.P be $a, \left( a+d \right) , \left( a+2d \right) , \ldots$
Let the terms of G.P be $a,ar,ar^{2}$

$\\ a+d=ar \\\\ a+2d=ar^{2} \\\\ r=\frac{a+2d}{a+d}=\frac{a+d}{a} \\\\ a^{2}+2ad=a^{2}+2ad+d^{2} \\\\ d^{2}=0 \\\\ r=\frac{a+2 \left( 0 \right) }{a+ \left( 0 \right) }=1 \\\\$

So the terms of A.P and G.P are the same

Question 31 State whether the statement is True or False. Every progression is a sequence but the converse, i.e., every sequence is also a progression need not necessarily be true.

Answer:

A progression is a sequence, but a sequence is not a progression because it does not follow a specific pattern.
Hence, the given statement is TRUE.

Question 32 State whether the statement is True or False.
Any term of an A.P. (except first) is equal to half the sum of terms that are equidistant from it.

Answer:

Let us consider an A.P a, a+d, a+2d, a+3d
$\\a_{2}+a_{4}= \left( a+d \right) + \left( a+3d \right) =2 \left( a+2d \right) =2 \left( a_{3} \right) ~~~~ \\\\ ~a_{3}=\frac{a_{2}+a_{4}}{2}~ \\\\$
Hence, the given statement is TRUE.

Question 33 State whether the statement is True or False. The sum or difference of two G.P.s is again a G.P.

Answer:

Let the two G.P be $a,ar_{1},ar_{1}^{2},ar_{1}^{3}, \ldots$
And $b,br_{2},br_{2}^{2},br_{2}^{3}, \ldots \\\\$
The terms of the sum of these two G.P are

$\\T_{1}= \left( b+a \right) \\\\ T_{2}= \left( br_{2}+ar_{1} \right) \\\\ T_{3}= \left( br_{2}^{2}+ar_{1}^{2} \right) \\\\ r^{'}=\frac{T_{3}}{T_{2}}=\frac{T_{2}}{T_{1}} \\\\$
$\\ \frac{T_{3}}{T_{2}}=\frac{br_{2}^{2}+ar_{1}^{2}}{br_{2}+ar_{1}}~and~\frac{T_{2}}{T_{1}}=\frac{br_{2}+ar_{1}}{b+a} \\\\ \frac{T_{3}}{T_{2}} \neq \frac{T_{2}}{T_{1}} \\\\$

The terms of the difference between two G.P are
$\\ T_{1}= \left( b-a \right) \\\\ T_{2}= \left( br_{2}-ar_{1} \right) \\\\ T_{3}= \left( br_{2}^{2}-ar_{1}^{2} \right) \\\\ r^{'}=\frac{T_{3}}{T_{2}}=\frac{T_{2}}{T_{1}} \\\\$
$\\ \frac{br_{2}^{2}-ar_{1}^{2}}{br_{2}-ar_{1}}=\frac{br_{2}-ar_{1}}{b-a} \\\\ \frac{T_{3}}{T_{2}} \neq \frac{T_{2}}{T_{1}} \\\\$

Hence, the difference and sum of 2 G.P is not a G.P because the common ratio is not the same.

Question 34 State whether the statement is True or False. If the sum of n terms of a sequence is a quadratic expression then it always represents an A.P.

Answer:

$S_{n}=an^{2}+bn+c \\\\$
For n=1 $S_{1}=a+b+c \\\\$

$\\ S_{2}=4a+2b+c \\\\ S_{3}=9a+3b+c \\\\ a_{1}=S_{1}=a+b+c \\\\ a_{2}=S_{2}-S_{1}=3a+b \\\\ a_{3}=S_{3}-S_{2}=5a+b \\\\$
$\\d=a_{2}-a_{1}=2a-c \\\\ d=a_{3}-a_{2}=2a \\\\ a_{3}-a_{2} \neq a_{2}-a_{1} \\\\$

So, it does not represent an AP because the common difference is not the same.

Question 35

Match the questions given under Column I with their appropriate answers given under Column II.

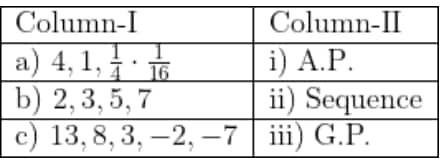
Answer:

$\left( a \right) \text{ Given 4, 1, }\frac{1}{4},\frac{1}{16}\text{~~ Here,}\frac{a_{2}}{a_{1}}=\frac{1}{4}~,\frac{a_{3}}{a_{2}}=\frac{\frac{1}{4}}{1}=\frac{1}{4}\text{~ and similarly}\frac{a_{4}}{a_{3}}=\frac{1}{16} *\frac{4}{1}=\frac{1}{4}~~ \\\\$

Hence, the given numbers are in G.P with common ratio $\frac{1}{4}~~ \\\\$
$\\~ \left( a \right) - \left( iii \right)$
$\left( b \right) \text{~Given~2, 3, 5, 7 } $
Here,$~a_{2}-a_{1}=3-2=1~~~ a_{3}-a_{2}=5-3=2~~ a_{4}-a_{3}=7-5=2$
$~a_{2}-a_{1} \neq a_{3}-a_{2}~~ \\\\$

Hence, it is not in the AP.

Now, we will check the ratio $\frac{a_{2}}{a_{1}}=\frac{3}{2},~\frac{a_{3}}{a_{2}}=\frac{5}{7}~~ $

$\\ ~So,\frac{a_{2}}{a_{1}} \neq \frac{a_{3}}{a_{2}}\text{~~Thus,~it~is not a G.P } $
$ ~ \left( b \right) - \left( ii \right) ~~ \\\\ ~ \left( c \right) ~13,8,~3, -2, -7 Here, a_{2}-a_{1}=8-13=-5 $
$\\~a_{3}-a_{2}=3-8=~-5 \\\\ ~a_{4}-a_{3}=-2-3=-5~ \\\\ ~~~~a_{2}-a_{1}=a_{3}-a_{2}$
$\text{ Hence, it is an A.P } \\\\ ~~~ \left( c \right) - \left( i \right) \\\\$

Question 36

Match the questions given under Column I with their appropriate answers given under Column II.

Answer:

Sum of ‘n’ natural numbers $\sum _{i=1}^{n}i= \left( \frac{n \left( n+1 \right) }{2} \right) \\\\$
Sum of cube of ‘n’ natural numbers $\sum _{i=1}^{n}i^{3}= \left( \frac{n \left( n+1 \right) }{2} \right) ^{2} \\\\$
Sum of square of ‘n’ natural numbers $\sum _{i=1}^{n}i^{2}= \left( \frac{n \left( n+1 \right) \left( 2n+1 \right) }{6} \right) \\\\$
Sum of twice times ‘n’ natural numbers $\sum _{i=1}^{n}2i=n \left( n+1 \right) \\\\$

So, (a) – (iii), (b)- (i), (c)- (ii), (d)- (iv)

Important Topics To Cover From NCERT Exemplar Class 11 Maths Solutions Chapter 9 Sequence and Series

Some of the important topics for students to review are as follows:

• The students should learn about different sequences, including arithmetic and geometric progressions, their properties, along their importance in mathematics.
• NCERT Exemplar Class 11 Maths Chapter 9 Solutions cover the sum of elements of any sequence referred to as a series, whether finite or infinite, and its representation through the compact form of sigma notation.
• NCERT Exemplar Class 11 Maths Solutions Chapter 9 also includes the study of arithmetic and the geometric mean of sequences.
• The students must practice the numerical and the Solutions of this Chapter from the exam point of view.
• NCERT Exemplar Solutions for Class 11 Maths Chapter 9 also derive the relation between the arithmetic mean and the geometric mean of any sequence and its formulas.

NCERT Solutions for Class 11 Maths: Chapter Wise

Students can easily find all NCERT Class 11 Maths Solutions in one place on Careers360. Simply tap the links below to get started.

Importance of Solving NCERT Questions for Class 11 Chapter 9 Sequence and Series

After accessing Class 11 Maths NCERT Solutions Chapter 9, students can study strategically at their own pace. This will boost their confidence in attempting other questions from this Chapter.

• Class 11 Maths Chapter 9 NCERT Solutions are solved by subject-matter experts and are very reliable at the same time. The Solutions provide shortcuts as well as detailed explanations with necessary formulae that will help students to understand the answers better.
• These Solutions will help students manage their time efficiently in this Chapter and understand which questions are easier to approach and which are time-consuming. This will be helpful during the exam.
• NCERT Solutions for class 11 Maths Chapter 9 Sequence and Series are designed to give the students step-by-step Solutions for a particular question.

NCERT Exemplar Class 11 Mathematics Chapter Wise

For quick and easy access, Careers360 provides all NCERT Class 11 Maths Exemplar Solutions together on one page. Use the links below to open them.

NCERT Solutions of class 11 - Subject-wise

Check out the subject-wise NCERT Solutions links for Class 11 given below.

• NCERT Solutions for Class 11 Maths
• NCERT Solutions for Class 11 Physics
• NCERT Solutions for Class 11 Chemistry
• NCERT Solutions for Class 11 Biology

NCERT Notes of class 11 - Subject Wise

Use the links below to explore NCERT Notes for Class 11 for each subject.

• NCERT Notes for Class 11 Maths
• NCERT Notes for Class 11 Physics
• NCERT Notes for Class 11 Chemistry
• NCERT Notes for Class 11 Biology

NCERT Books and NCERT Syllabus

At the beginning of the academic year, students need to consult the latest syllabus for clarity on the Chapters. Given below are the revised syllabus links and helpful reference books.

• NCERT Books Class 11 Maths
• NCERT Syllabus Class 11 Maths
• NCERT Books Class 11
• NCERT Syllabus Class 11

NCERT Exemplar Class 11 Solutions

Given below are the subject-wise Exemplar Solutions of class 11 NCERT:

• NCERT Exemplar Class 11 Biology Solutions
• NCERT Exemplar Class 11 Chemistry Solutions
• NCERT Exemplar Class 11 Physics Solutions