NCERT Solutions for Class 11 Maths Chapter 12 Limits and Derivatives

Edited By Komal Miglani | Updated on Jun 06, 2025 09:26 PM IST

Limits are considered one of the most important concepts in mathematics, and derivatives are the soul of calculus. Imagine you are climbing a mountain and wonder how steep it is at a certain point. Or, after baking a cake at a high temperature, when you bring it out to room temperature, it will cool down in time, not instantly. You want to know what temperature the cake is at after 30 minutes. Class 11 Maths chapter 12 solutions teach all the relevant concepts for these types of situations, including limits and derivatives. In simpler words, a limit helps us understand the behaviour of a function as it approaches a particular value. On the other hand, a derivative tells us the rate of change of a function. The main purpose of the NCERT class 11 Maths solutions is to strengthen the basics and provide conceptual clarity.

JEE Main Scholarship Test Kit (Class 11): Narayana | Physics Wallah | Aakash | Unacademy

Suggested: JEE Main: high scoring chapters | Past 10 year's papers

This Story also Contains

NCERT Solution for Class 11 Maths Chapter 12 Solutions: Download PDF
Limits and Derivatives Class 11 Solutions: Important Formulae
NCERT Solutions for Class 11 Maths Chapter 12: Exercise Questions
Class 11 Maths NCERT Chapter 12: Extra Question
Approach to Solve Questions of Limits and Derivatives Class 11
What Extra Should Students Study Beyond NCERT for JEE?
NCERT Solutions for Class 11 Mathematics - Chapter Wise

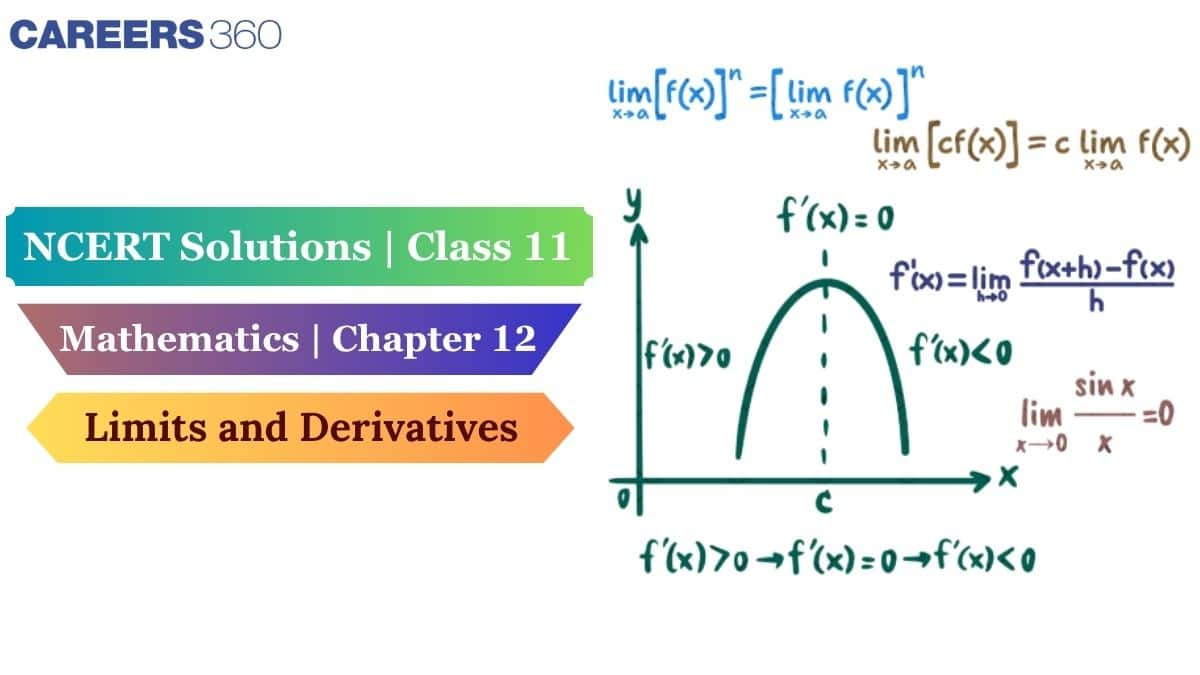
NCERT Solutions for Class 11 Maths Chapter 12 Limits and Derivatives

In class 11 Maths NCERT chapter 9 solutions, students will be introduced to calculus, which is an Important branch of mathematics, as well as study algebra of limits and derivatives with their definitions and certain standard functions. Subject matter experts at Careers360 have curated these NCERT class 11 solutions with step-by-step explanations, ensuring clarity in every step. Students can also explore the following links for syllabus, notes, and PDF: NCERT

NCERT Solution for Class 11 Maths Chapter 12 Solutions: Download PDF

Download PDF

Limits and Derivatives Class 11 Solutions: Important Formulae

Limits

The limit of a function $f (x)$ as $x$ approaches $a$ , denoted as $lim_{x \to a} f (x)$ , exists when both the left-hand limit and the right-hand limit exist and are equal:

$lim_{x \to a} f (x)$ exists if $lim_{x \to a^{-}} f (x) = lim_{x \to a^{+}} f (x)$

Left-Hand Limit:

$lim_{x \to a^{-}} f (x) = lim_{h \to 0} f (a - h)$

Right-Hand Limit:

$lim_{x \to a^{+}} f (x) = lim_{h \to 0} f (a + h)$

Properties of Limits:

If $lim_{x \to a} f (x)$ and $lim_{x \to a} g (x)$ both exist:
Sum Rule:

$lim_{x \to a} [f (x) \pm g (x)] = lim_{x \to a} f (x) \pm lim_{x \to a} g (x)$

Constant Rule:

$lim_{x \to a} [k \cdot f (x)] = k \cdot lim_{x \to a} f (x)$

Product Rule:

$lim_{x \to a} [f (x) \cdot g (x)] = lim_{x \to a} f (x) \cdot lim_{x \to a} g (x)$

Quotient Rule:

$lim_{x \to a} [\frac{f (x)}{g (x)}] = \frac{lim_{x \to a} f (x)}{lim_{x \to a} g (x)},$ provided $lim_{x \to a} g (x) \neq 0$

Standard Limits:

$lim_{x \to a} \frac{x^{n} - a^{n}}{x - a} = n a^{n - 1}$
$lim_{x \to 0} \frac{\sin x}{x} = 1$
$lim_{x \to 0} \frac{\tan x}{x} = 1$
$lim_{x \to 0} \frac{a^{x} - 1}{x} = \ln a$
$lim_{x \to 0} \frac{e^{x} - 1}{x} = 1$
$lim_{x \to 0} \frac{\ln (1 + x)}{x} = 1$

Derivatives:

The derivative of a function $f$ at point $x$ is given by:

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

Properties of Derivatives:

If $f (x)$ and $g (x)$ are differentiable:
Sum Rule:

$\frac{d}{d x} [f (x) + g (x)] = \frac{d}{d x} f (x) + \frac{d}{d x} g (x)$

Difference Rule:

$\frac{d}{d x} [f (x) - g (x)] = \frac{d}{d x} f (x) - \frac{d}{d x} g (x)$

Product Rule:

$\frac{d}{d x} [f (x) \cdot g (x)] = f^{'} (x) g (x) + f (x) g^{'} (x)$

Quotient Rule:

$\frac{d}{d x} [\frac{f (x)}{g (x)}] = \frac{f^{'} (x) g (x) - f (x) g^{'} (x)}{[g (x)]^{2}}$

Standard Derivatives:

$\frac{d}{d x} (x^{n}) = n x^{n - 1}$
$\frac{d}{d x} (\sin x) = \cos x$
$\frac{d}{d x} (\cos x) = - \sin x$
$\frac{d}{d x} (\tan x) = \sec^{2} x$
$\frac{d}{d x} (\cot x) = - \csc^{2} x$
$\frac{d}{d x} (\sec x) = \sec x \cdot \tan x$
$\frac{d}{d x} (\csc x) = - \csc x \cdot \cot x$
$\frac{d}{d x} (a^{x}) = a^{x} \cdot \ln a$
$\frac{d}{d x} (e^{x}) = e^{x}$
$\frac{d}{d x} (\ln x) = \frac{1}{x}$

NCERT Solutions for Class 11 Maths Chapter 12: Exercise Questions

Class 11 Maths chapter 12 solutions Exercise: 12.1

Page number: 237-239

Total questions: 32

Question 1: Evaluate the following limits $lim_{x \to 3} x + 3$

Answer:

$lim_{x \to 3} x + 3$

$\Rightarrow lim_{x \to 3} 3 + 3$

$\Rightarrow 6$

Question 2: Evaluate the following limits $lim_{x \to π} (x - 22 / 7)$

Answer:

Below, you can find the solution:

$lim_{x \to π} (x - 22 / 7) = π - \frac{22}{7}$

Question 3: Evaluate the following limits $lim_{r \to 1} π r^{2}$

Answer:

The limit

$lim_{r \to 1} π r^{2} = π (1)^{2} = π$

Hence, the answer is $π$

Question 4: Evaluate the following limits $lim_{x \to 4} \frac{4 x + 3}{x - 2}$

Answer:

The limit

$lim_{x \to 4} \frac{4 x + 3}{x - 2}$

$\Rightarrow \frac{4 (4) + 3}{(4) - 2}$

$\Rightarrow \frac{19}{2}$

Question 5: Evaluate the following limits $lim_{x \to - 1} \frac{x^{10} + x^{5} + 1}{x - 1}$

Answer:

The limit

$lim_{x \to 4} \frac{x^{10} + x^{5} + 1}{x - 1}$

$\Rightarrow \frac{(- 1)^{10} + (- 1)^{5} + 1}{(- 1) - 1}$

$\Rightarrow \frac{1 - 1 + 1}{- 2}$

$\Rightarrow - \frac{1}{2}$

Question 6: Evaluate the following limits $lim_{x \to 0} \frac{(x + 1)^{5} - 1}{x}$

Answer:

The limit

$lim_{x \to 0} \frac{(x + 1)^{5} - 1}{x}$

Let's put

$x + 1 = y$

Since we have changed the function, its limit will also change,

$x \to 0, y \to 0 + 1 = 1$

So our function has became

$lim_{y \to 1} \frac{y^{5} - 1}{y - 1}$

Now, as we know the property

$lim_{x \to 1} \frac{x^{5} - a^{n}}{x - a} = n a^{n - 1}$

$lim_{y \to 1} \frac{y^{5} - 1}{y - 1} = 5 (1)^{5} = 5$

Hence,

$lim_{x \to 0} \frac{(x + 1)^{5} - 1}{x} = 5$

Question 7: Evaluate the following limits $lim_{x \to 2} \frac{3 x^{2} - x - 10}{x^{2} - 4}$

Answer:

The limit

$lim_{x \to 2} \frac{3 x^{2} - x - 10}{x^{2} - 4}$

$\Rightarrow lim_{x \to 2} \frac{(x - 2) (3 x + 5)}{(x - 2) (x + 2)}$

$\Rightarrow lim_{x \to 2} \frac{(3 x + 5)}{(x + 2)}$

$\Rightarrow \frac{(3 (2) + 5)}{((2) + 2)}$

$\Rightarrow \frac{11}{4}$

Question 8: Evaluate the following limits $lim_{x \to 3} \frac{x^{4} - 81}{2 x^{2} - 5 x - 3}$

Answer:

The limit

$lim_{x \to 3} \frac{x^{4} - 81}{2 x^{2} - 5 x - 3}$

At x = 2, both the numerator and denominator become zero, so let's factorise the function

$lim_{x \to 3} \frac{(x - 3) (x + 3) (x^{2} + 9)}{(x - 3) (2 x + 1)}$

$lim_{x \to 3} \frac{(x + 3) (x^{2} + 9)}{(2 x + 1)}$

Now we can put the limit directly, so

$lim_{x \to 3} \frac{(x + 3) (x^{2} + 9)}{(2 x + 1)}$

$\Rightarrow \frac{((3) + 3) ((3)^{2} + 9)}{(2 (3) + 1)}$

$\Rightarrow \frac{6 \times 18}{7}$

$\Rightarrow \frac{108}{7}$

Question 9: Evaluate the following limits $lim_{x \to 0} \frac{a x + b}{c x + 1}$

Answer:

The limit,

$lim_{x \to 0} \frac{a x + b}{c x + 1}$

$\Rightarrow \frac{a (0) + b}{c (0) + 1}$

$\Rightarrow b$

Question 10: Evaluate the following limits $lim_{z \to 1} \frac{z^{1 / 3} - 1}{z^{1 / 6} - 1}$

Answer:

The limit

$lim_{z \to 1} \frac{z^{1 / 3} - 1}{z^{1 / 6} - 1}$

Here, on directly putting the limit, both the numerator and the denominator become zero so we factorize the function and then put the limit.

$lim_{z \to 1} \frac{z^{1 / 3} - 1}{z^{1 / 6} - 1} = lim_{z \to 1} \frac{z^{(1 / 6)^{2}} - 1^{2}}{z^{1 / 6} - 1}$

$= lim_{z \to 1} \frac{(z^{(1 / 6)} - 1) (z^{(1 / 6)} + 1)}{z^{1 / 6} - 1}$

$= lim_{z \to 1} (z^{1 / 6} + 1)$

$= (1^{1 / 6} + 1)$

$= 1 + 1$

$= 2$

Question 11: Evaluate the following limits $lim_{x \to 1} \frac{a x^{2} + b x + c}{c x^{2} + b x + a}, a + b + c \neq 0$

Answer:

The limit:

$lim_{x \to 1} \frac{a x^{2} + b x + c}{c x^{2} + b x + a}, a + b + c \neq 0$

Since the Denominator is not zero on directly putting the limit, we can directly put the limits, so,

$lim_{x \to 1} \frac{a x^{2} + b x + c}{c x^{2} + b x + a}, a + b + c \neq 0$

$= \frac{a (1)^{2} + b (1) + c}{c (1)^{2} + b (1) + a},$

$= \frac{a + b + c}{a + b + c},$

$= 1$

Question 12: Evaluate the following limits $lim_{x \to - 2} \frac{\frac{1}{x} + \frac{x}{2}}{x + 2}$

Answer:

$lim_{x \to - 2} \frac{\frac{1}{x} + \frac{x}{2}}{x + 2}$

Here, since the denominator becomes zero on putting the limit directly, we first simplify the function and then put the limit,

$lim_{x \to - 2} \frac{\frac{1}{x} + \frac{x}{2}}{x + 2}$

$= lim_{x \to - 2} \frac{\frac{x + 2}{2 x}}{x + 2}$

$= lim_{x \to - 2} \frac{1}{2 x}$

$= \frac{1}{2 (- 2)}$

$= - \frac{1}{4}$

Question 13: Evaluate the following limits $lim_{x \to 0} \frac{\sin a x}{b x}$

Answer:

The limit

$lim_{x \to 0} \frac{\sin a x}{b x}$

Here, on directly putting the limits, the function becomes $\frac{0}{0}$ form. So we try to make the function in the form of $\frac{s i n x}{x}$ . so,

$lim_{x \to 0} \frac{\sin a x}{b x}$

$= lim_{x \to 0} \frac{\sin a x (a x)}{b x (a x)}$

$= lim_{x \to 0} \frac{\sin a x}{a x} \frac{a}{b}$

As $lim_{x \to 0} \frac{s i n x}{x} = 1$

$= 1 \cdot \frac{a}{b}$

$= \frac{a}{b}$

Question 14: Evaluate the following limits $lim_{x \to 0} \frac{\sin a x}{\sin b x}, a, b \neq 0$

Answer:

The limit,

$lim_{x \to 0} \frac{\sin a x}{\sin b x}, a, b \neq 0$

On putting the limit directly, the function takes the zero by zero form. So, we convert it in the form of $\frac{s i n a}{a}$ .and then put the limit,

$\Rightarrow lim_{x \to 0} \frac{\frac{s i n a x}{a x}}{\frac{s i n b x}{b x}} \cdot \frac{a x}{b x}$

$= \frac{lim_{a x \to 0} \frac{s i n a x}{a x}}{lim_{b x \to 0} \frac{s i n b x}{b x}} \cdot \frac{a}{b}$

$= \frac{a}{b}$

Question 15: Evaluate the following limits $lim_{x \to π} \frac{\sin (π - x)}{π (π - x)}$

Answer:

The limit

$lim_{x \to π} \frac{\sin (π - x)}{π (π - x)}$

$\Rightarrow lim_{x \to π} \frac{\sin (π - x)}{(π - x)} \times \frac{1}{π}$

$= 1 \times \frac{1}{π}$

$= \frac{1}{π}$

Question 16: Evaluate the following limits $lim_{x \to 0} \frac{\cos x}{π - x}$

Answer:

The limit

$lim_{x \to 0} \frac{\cos x}{π - x}$

The function behaves well on directly putting the limit, so we put the limit directly. So.

$lim_{x \to 0} \frac{\cos x}{π - x}$

$= \frac{\cos (0)}{π - (0)}$

$= \frac{1}{π}$

Question 17: Evaluate the following limits $lim_{x \to 0}$ $\frac{\cos 2 x - 1}{\cos x - 1}$

Answer:

The limit:

$lim_{x \to 0}$ $\frac{\cos 2 x - 1}{\cos x - 1}$

The function takes the zero-by-zero form when the limit is put directly, so we simplify the function and then put the limit

$lim_{x \to 0}$ $\frac{\cos 2 x - 1}{\cos x - 1}$

$lim_{x \to 0}$ $\frac{- 2 (s i n^{2} x)}{- 2 (s i n^{2} (\frac{x}{2}))}$

$= lim_{x \to 0}$ $\frac{\frac{(s i n^{2} x)}{x^{2}}}{\frac{(s i n^{2} (\frac{x}{2}))}{(\frac{x}{2})^{2}}} \times \frac{x^{2}}{(\frac{x}{2})^{2}}$

$= \frac{1^{2}}{1^{2}} \times 4$

$= 4$

Question 18: Evaluate the following limits $lim_{x \to 0} \frac{a x + x \cos x}{b \sin x}$

Answer:

$lim_{x \to 0} \frac{a x + x \cos x}{b \sin x}$

The function takes the form zero by zero when we put the limit directly in the function Since theunction consists of the sin function and cos function, we try to make the function in the form of $\frac{s i n x}{x}$ as we know that it tends to 1 when x tends to 0.

So,

$lim_{x \to 0} \frac{a x + x \cos x}{b \sin x}$

$= \frac{1}{b} lim_{x \to 0} \frac{x (a + \cos x)}{\sin x}$

$= \frac{1}{b} lim_{x \to 0} \frac{x}{\sin x} \times (a + \cos x)$

$= \frac{1}{b} \times 1 \times (a + \cos (0))$

$= \frac{a + 1}{b}$

Question 19: Evaluate the following limits $lim_{x \to 0} x \sec x$

Answer:

$lim_{x \to 0} x \sec x$

As the function doesn't create any abnormality on putting the limit directly, we can put the limit directly. So,

$lim_{x \to 0} x \sec x$

$= (0) \times 1$

$= 0$ .

Question 20: Evaluate the following limits $lim_{x \to 0} \frac{\sin a x + b x}{a x + \sin b x} a, b, a + b \neq 0$

Answer:

$lim_{x \to 0} \frac{\sin a x + b x}{a x + \sin b x} a, b, a + b \neq 0$

The function takes the zero-by-zero form when we put the limit into the function directly, so we try to eliminate this case by simplifying the function. So

$lim_{x \to 0} \frac{\sin a x + b x}{a x + \sin b x} a, b, a + b \neq 0$

$= lim_{x \to 0} \frac{\frac{\sin a x}{a x} \cdot a x + b x}{a x + \frac{\sin b x}{b x} \cdot b x}$

$= lim_{x \to 0} \frac{\frac{\sin a x}{a x} \cdot a + b}{a + \frac{\sin b x}{b x} \cdot b}$

$= \frac{1 \cdot a + b}{a + 1 \cdot b}$

$= \frac{a + b}{a + b}$

$= 1$

Question 21: Evaluate the following limits $lim_{x \to 0} (\csc x - \cot x)$

Answer:

$lim_{x \to 0} (\csc x - \cot x)$

On putting the limit directly, the function takes infinity by infinity form, so we simplify the function and then put the limit

$lim_{x \to 0} (\csc x - \cot x)$

$= lim_{x \to 0} (\frac{1}{s i n x} - \frac{c o s x}{s i n x})$

$= lim_{x \to 0} (\frac{1 - c o s x}{s i n x})$

$= lim_{x \to 0} (\frac{2 s i n^{2} (\frac{x}{2})}{s i n x})$

$= lim_{x \to 0} (\frac{2 s i n^{2} (\frac{x}{2})}{(\frac{x}{2})^{2}}) (\frac{(\frac{x}{2})^{2}}{s i n x})$

$= lim_{x \to 0} \frac{2}{4} (\frac{s i n^{2} (\frac{x}{2})}{(\frac{x}{2})^{2}}) (\frac{(x)}{s i n x}) \cdot x$

$= \frac{2}{4} \times (1)^{2} \times 0$

$= 0$

Question 22: Evaluate the following limits $lim_{x \to π / 2} \frac{\tan 2 x}{x - π / 2}$

Answer:

$lim_{x \to π / 2} \frac{\tan 2 x}{x - π / 2}$

The function takes zero by zero form when the limit is put directly, so we simplify the function and then put the limits,

Let's put

$y = x - \frac{π}{2}$

Since we are changing the variable, the limit will also change.

$x \to \frac{π}{2}, y = x - \frac{π}{2} \to \frac{π}{2} - \frac{π}{2} = 0$

So, the function in the new variable becomes,

$lim_{y \to 0} \frac{\tan 2 (y + \frac{π}{2})}{y + π / 2 - π / 2}$

$= lim_{y \to 0} \frac{\tan (2 y + π)}{y}$

As we know that property $t a n (π + x) = t a n x$

$= lim_{y \to 0} \frac{\tan (2 y)}{y}$

$= lim_{y \to 0} \frac{s i n 2 y}{2 y} \cdot \frac{2}{c o s 2 y}$

$= 1 \times 2$

$= 2$

Question 23: Find $lim_{x \to 0} f (x) lim_{x \to 1} f (x) w h e r e f (x) = {\begin{matrix} 2 x + 3 & x \leq 0 \\ 3 (x + 1) & x > 0 \end{matrix}$

Answer:

Given Function

$f (x) = {\begin{matrix} 2 x + 3 & x \leq 0 \\ 3 (x + 1) & x > 0 \end{matrix}$

Now,

Limit at x = 0 :

$a t x = 0^{-}$

: $lim_{x \to 0^{-}} f (x) = lim_{x \to 0^{-}} (2 x + 3) = 2 (0) + 3 = 3$

$a t x = 0^{+}$

$lim_{x \to 0^{+}} f (x) = lim_{x \to 0^{+}} 3 (x + 1) = 3 (0 + 1) = 3$

Hence limit at x = 0 is 3.

Limit at x = 1

$a t x = 1^{+}$

$lim_{x \to 1^{+}} f (x) = lim_{x \to 1^{+}} 3 (x + 1) = 3 (1 + 1) = 6$

$a t x = 1^{-}$

$lim_{x \to 1^{-}} f (x) = lim_{x \to 1^{-}} 3 (x + 1) = 3 (1 + 1) = 6$

Hence limit at x = 1 is 6.

Question 24: Find $lim_{x \to 1} f (x), w h e r e f (x) = {\begin{matrix} x^{2} - 1 & x \neq 0 \\ - x^{2} - 1 & x > 1 \end{matrix}$

Answer:

$lim_{x \to 1} f (x), w h e r e f (x) = {\begin{matrix} x^{2} - 1 & x \neq 0 \\ - x^{2} - 1 & x > 1 \end{matrix}$

Limit at $x = 1^{+}$

$lim_{x \to 1^{+}} f (x) = lim_{x \to 1} (- x^{2} - 1) = - (1)^{2} - 1 = - 2$

Limit at $x = 1^{-}$

$lim_{x \to 1^{-}} f (x) = lim_{x \to 1} (x^{2} - 1) = (1)^{2} - 1 = 0$

As we can see Limit at $x = 1^{+}$ is not equal to the Limit at $x = 1^{-}$ , The limit of this function at x = 1 does not exist.

Question 25: Evaluate $lim_{x \to 0} f (x), w h e r e f (x) = {\begin{matrix} \frac{| x |}{x} & x \neq 0 \\ 0 & x = 0 \end{matrix}$

Answer:

$lim_{x \to 0} f (x), w h e r e f (x) = {\begin{matrix} \frac{| x |}{x} & x \neq 0 \\ 0 & x = 0 \end{matrix}$

The right-hand Limit or Limit at $x = 0^{+}$

$lim_{x \to 0^{+}} f (x) = lim_{x \to 0^{+}} \frac{| x |}{x} = \frac{x}{x} = 1$

The left-hand limit or Limit at $x = 0^{-}$

$lim_{x \to 0^{-}} f (x) = lim_{x \to 0^{-}} \frac{| x |}{x} = \frac{- x}{x} = - 1$

Since The left-hand limit and right-hand limit are not equal, The limit of this function at x = 0 does not exist.

Question 26: Evaluate $lim_{x \to 0} f (x), w h e r e f (x) = {\begin{matrix} \frac{x}{| x |} & x \neq 0 \\ 0 & x = 0 \end{matrix}$

Answer:

$lim_{x \to 0} f (x), w h e r e f (x) = {\begin{matrix} \frac{x}{| x |} & x \neq 0 \\ 0 & x = 0 \end{matrix}$

The right-hand Limit or Limit at $x = 0^{+}$

$lim_{x \to 0^{+}} f (x) = lim_{x \to 0^{+}} \frac{x}{| x |} = \frac{x}{x} = 1$

The left-hand limit or Limit at $x = 0^{-}$

$lim_{x \to 0^{-}} f (x) = lim_{x \to 0^{-}} \frac{x}{| x |} = \frac{x}{- x} = - 1$

Since The left-hand limit and right-hand limit are not equal, The limit of this function at x = 0 does not exist.

Question 27: Find $lim_{x \to 5} f (x), w h e r e f (x) = | x | - 5$

Answer:

$lim_{x \to 5} f (x), w h e r e f (x) = | x | - 5$

The right-hand Limit or Limit at $x = 5^{+}$

$lim_{x \to 5^{+}} f (x) = lim_{x \to 5^{+}} | x | - 5 = 5 - 5 = 0$

The left-hand limit or Limit at $x = 5^{-}$

$lim_{x \to 5^{-}} f (x) = lim_{x \to 5^{-}} | x | - 5 = 5 - 5 = 0$

Since The left-hand limit and right-hand limit are equal, the limit of this function at x = 5 is 0.

Question 28: Suppose $f (x) = {\begin{matrix} a + b x & x < 1 \\ 4 & x = 1 \\ b - a x & x > 1 \end{matrix}$ f (x) = f (1) what are possible values of a and b?

Answer:

Given,

$f (x) = {\begin{matrix} a + b x & x < 1 \\ 4 & x = 1 \\ b - a x & x > 1 \end{matrix}$

And

$lim_{x \to 1} f (x) = f (1)$

Since the limit exists,

left-hand limit = Right-hand limit = f(1).

Left-hand limit = f(1)

$lim_{x \to 1^{-}} f (x) = lim_{x \to 1} (a + b x) = a + b (1) = a + b = 4$

Right-hand limit

$lim_{x \to 1^{+}} f (x) = lim_{x \to 1} (b - a x) = b - a (1) = b - a = 4$

From both equations, we get that,

$a = 0$ and $b = 4$

Hence, the possible values of a and b are 0 and 4, respectively.

Question 29: Let a1, a2, . . ., an be fixed real numbers and define a function $f (x) = (x - a_{1}) (x - a_{2}) . . . (x - a_{n}) .$ What is $lim_{x \to a_{1}}$ f (x) ? For some $a \neq a_{1}, a_{2} . . . . a_{n}$ , compute l $lim_{x \to a} f (x)$

Answer:

Given,

$f (x) = (x - a_{1}) (x - a_{2}) . . . (x - a_{n}) .$

Now,

$lim_{x \to a_{1}} f (x) = lim_{x \to a_{1}} [(x - a_{1}) (x - a_{2}) . . . (x - a_{n})] . = [lim_{x \to a_{1}} (x - a_{1})] [lim_{x \to a_{1}} (x - a_{2})] [lim_{x \to a_{1}} (x - a_{n})] = 0$

Hence,

$lim_{x \to a_{1}} f (x) = 0$

Now,

$lim_{x \to a} f (x) = lim_{x \to a} (x - a_{1}) (x - a_{2}) . . . (x - a_{n})$

$lim_{x \to a} f (x) = (a - a_{1}) (a - a_{2}) (a - a_{3})$

Hence

$lim_{x \to a} f (x) = (a - a_{1}) (a - a_{2}) (a - a_{3})$ .

Question 30: If $f (x) = {\begin{matrix} | x | + 1 & x < 0 \\ 0 & x = 0 \\ | x | - 1 & x > 0 \end{matrix}$ For what value (s) of a does $lim_{x \to a} f (x)$ exists ?

Answer:

$f (x) = {\begin{matrix} | x | + 1 & x < 0 \\ 0 & x = 0 \\ | x | - 1 & x > 0 \end{matrix}$

The limit at x = a exists when the right-hand limit is equal to the left-hand limit. So,

Case 1: when a = 0

The right-hand Limit or Limit at $x = 0^{+}$

$lim_{x \to 0^{+}} f (x) = lim_{x \to 0^{+}} | x | - 1 = 1 - 1 = 0$

The left-hand limit or Limit at $x = 0^{-}$

$lim_{x \to 0^{-}} f (x) = lim_{x \to 0^{-}} | x | + 1 = 0 + 1 = 1$

Since the Left-hand limit and right-hand limit are not equal, The limit of this function at x = 0 does not exist.

Case 2: When a < 0

The right-hand Limit or Limit at $x = a^{+}$

$lim_{x \to a^{+}} f (x) = lim_{x \to a^{+}} | x | - 1 = a - 1$

The left-hand limit or Limit at $x = a^{-}$

$lim_{x \to a^{-}} f (x) = lim_{x \to a^{-}} | x | - 1 = a - 1$

Since LHL = RHL, the Limit exists at x = a and is equal to a-1.

Case 3: When a > 0

The right-hand Limit or Limit at $x = a^{+}$

$lim_{x \to a^{+}} f (x) = lim_{x \to a^{+}} | x | + 1 = a + 1$

The left-hand limit or Limit at $x = a^{-}$

$lim_{x \to a^{-}} f (x) = lim_{x \to a^{-}} | x | + 1 = a + 1$

Since LHL = RHL, Limit exists at x = a and is equal to a+1

Hence,

The limit exists at all points except at x=0.

Question 31:If the function f(x) satisfies $lim_{x \to 1} \frac{f (x) - 2}{x^{2} - 1} = π$ , evaluate $lim_{x \to 1} f (x)$

Answer:

Given

$lim_{x \to 1} \frac{f (x) - 2}{x^{2} - 1} = π$

Now,

$lim_{x \to 1} \frac{f (x) - 2}{x^{2} - 1} = \frac{lim_{x \to 1} (f (x) - 2)}{lim_{x \to 1} (x^{2} - 1)} = π$

$lim_{x \to 1} (f (x) - 2) = π lim_{x \to 1} (x^{2} - 1)$

$lim_{x \to 1} (f (x) - 2) = π (1 - 1)$

$lim_{x \to 1} (f (x) - 2) = 0$

$lim_{x \to 1} f (x) = 2$

Question 32: If $f (x) = [\begin{array}{cl} m x^{2} + n & ; x < 0 \\ n x + m & ; x \leq 0 \leq 1 t \\ n x^{3} + m & ; x > 1 \end{array}]$

Answer:

Given,

$f (x) = [\begin{array}{cl} m x^{2} + n & ; x < 0 \\ n x + m & ; x \leq 0 \leq 1 t \\ n x^{3} + m & ; x > 1 \end{array}]$

Case 1: Limit at x = 0

The right-hand Limit or Limit at $x = 0^{+}$

$lim_{x \to 0^{+}} f (x) = lim_{x \to 0^{+}} n x + m = n (0) + m = m$

The left-hand limit or Limit at $x = 0^{-}$

$lim_{x \to 0^{-}} f (x) = lim_{x \to 0^{-}} m x^{2} + n = m (0)^{2} + n = n$

Hence Limit will exist at x = 0 when m = n.

Case 2: Limit at x = 1

The right-hand Limit or Limit at $x = 1^{+}$

$lim_{x \to 1^{+}} f (x) = lim_{x \to 1^{+}} n x^{3} + m = n (1)^{3} + m = n + m$

The left-hand limit or Limit at $x = 1^{-}$

$lim_{x \to 1^{-}} f (x) = lim_{x \to 1^{-}} n x + m = n (1) + m = n + m$

Hence Limit at 1 exists at all integers.

Class 11 Maths chapter 12 solutions Exercise: 12.2

Page number: 248-249

Total questions: 11

Question 1: Find the derivative of $x^{2} - 2 a t x = 10$

Answer:

F(x)= $x^{2} - 2$

Now, As we know, The derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

The derivative of f(x) at x = 10:

$f^{'} (10) = lim_{h \to 0} \frac{f (10 + h) - f (10)}{h}$

$f^{'} (10) = lim_{h \to 0} \frac{(10 + h)^{2} - 2 - ((10)^{2} - 2)}{h}$

$f^{'} (10) = lim_{h \to 0} \frac{100 + 20 h + h^{2} - 2 - 100 + 2}{h}$

$f^{'} (10) = lim_{h \to 0} \frac{20 h + h^{2}}{h}$

$f^{'} (10) = lim_{h \to 0} 20 + h$

$f^{'} (10) = 20 + 0$

$f^{'} (10) = 20$

Question 2: Find the derivative of x at x = 1.

Answer:

Given

f(x)= x

Now, As we know, The derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

The derivative of f(x) at x = 1:

$f^{'} (1) = lim_{h \to 0} \frac{f (1 + h) - f (1)}{h}$

$f^{'} (1) = lim_{h \to 0} \frac{(1 + h) - (1)}{h}$

$f^{'} (1) = lim_{h \to 0} \frac{(h)}{h}$

$f^{'} (1) = 1$ (Answer)

Question 3: Find the derivative of 99x at x = l00.

Answer:

f(x)= 99x

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

The derivative of f(x) at x = 100:

$f^{'} (100) = lim_{h \to 0} \frac{f (100 + h) - f (100)}{h}$

$f^{'} (100) = lim_{h \to 0} \frac{99 (100 + h) - 99 (100)}{h}$

$f^{'} (100) = lim_{h \to 0} \frac{99 h}{h}$

$f^{'} (100) = 99$

Question 4 (i): Find the derivative of the following functions from the first principle. $x^{3} - 27$

Answer:

Given

f(x)= $x^{3} - 27$

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{(x + h)^{3} - 27 - ((x)^{3} - 27)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{x^{3} + h^{3} + 3 x^{2} h + 3 h^{2} x - 27 + x^{3} + 27}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{h^{3} + 3 x^{2} h + 3 h^{2} x}{h}$

$f^{'} (x) = lim_{h \to 0} h^{2} + 3 x^{2} + 3 h x$

$f^{'} (x) = 3 x^{2}$

Question 4(ii): Find the derivative of the following function from the first principle. $(x - 1) (x - 2)$

Answer:

f(x)= $(x - 1) (x - 2)$

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{(x + h - 1) (x + h - 2) - (x - 1) (x - 2)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{x^{2} + x h - 2 x + h x + h^{2} - 2 h - x - h + 2 - x^{2} + 2 x + x - 2}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{2 h x + h^{2} - 3 h}{h}$

$f^{'} (x) = lim_{h \to 0} 2 x + h - 3$

$f^{'} (x) = 2 x - 3$ (Answer)

Question 4 (iii): Find the derivative of the following functions from the first principle. $1 / x^{2}$

Answer:

f(x)= $1 / x^{2}$

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{1 / (x + h)^{2} - 1 / (x^{2})}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\frac{x^{2} - (x + h)^{2}}{(x + h)^{2} x^{2}}}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{x^{2} - x^{2} - 2 x h - h^{2}}{h (x + h)^{2} x^{2}}$

$f^{'} (x) = lim_{h \to 0} \frac{- 2 x h - h^{2}}{h (x + h)^{2} x^{2}}$

$f^{'} (x) = lim_{h \to 0} \frac{- 2 x - h}{(x + h)^{2} x^{2}}$

$f^{'} (x) = \frac{- 2 x - 0}{(x + 0)^{2} x^{2}}$

$f^{'} (x) = \frac{- 2}{x^{3}}$ (Answer)

Question 4 (iv): Find the derivative of the following functions from the first principle. $\frac{x + 1}{x - 1}$

Answer:

Given:

$f (x) = \frac{x + 1}{x - 1}$

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\frac{x + h + 1}{x + h - 1} - \frac{x + 1}{x - 1}}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\frac{(x + h + 1) (x - 1) - (x + 1) (x + h - 1)}{(x - 1) (x + h - 1)}}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{x^{2} - x + h x - h + x - 1 - x^{2} - x h + x - x - h + 1}{(x - 1) (x + h - 1) h}$

$f^{'} (x) = lim_{h \to 0} \frac{- 2 h}{(x - 1) (x + h - 1) h}$

$f^{'} (x) = lim_{h \to 0} \frac{- 2}{(x - 1) (x + h - 1)}$

$f^{'} (x) = \frac{- 2}{(x - 1) (x + 0 - 1)}$

$f^{'} (x) = \frac{- 2}{(x - 1)^{2}}$

Question 5: For the function $f (x) = \frac{x^{100}}{100} + \frac{x^{99}}{99} + . . . . + \frac{x^{2}}{2} + x + 1$ Prove that f '(1) =100 f '(0).

Answer:

$f (x) = \frac{x^{100}}{100} + \frac{x^{99}}{99} + . . . . + \frac{x^{2}}{2} + x + 1$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

Applying that property, we get

$f^{'} (x) = 100 \frac{x^{99}}{100} + 99 \frac{x^{98}}{99} + . . . . + 2 \frac{x}{2} + 1 + 0$

$f^{'} (x) = x^{99} + x^{98} + . . . . . . x + 1$

Now.

$f^{'} (0) = 0^{99} + 0^{98} + . . . . . .0 + 1 = 1$

$f^{'} (1) = 1^{99} + 1^{98} + . . . . . .1 + 1 = 100$

So,

Here

$1 \times 100 = 100$

$f^{'} (0) \times 100 = f^{'} (1)$

Hence Proved.

Question 6: Find the derivative of $x^{n} + a x^{n - 1} + a^{2} x^{n - 2} + . . . . + a^{n - 1} x + a^{n}$ for some fixed real number a.

Answer:

Given

$f (x) = x^{n} + a x^{n - 1} + a^{2} x^{n - 2} + . . . . + a^{n - 1} x + a^{n}$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

Applying that property, we get

$f^{'} (x) = n x^{n - 1} + a (n - 1) x^{n - 2} + a^{2} (n - 2) x^{n - 3} + . . . . + a^{n - 1} 1 + 0$

$f^{'} (x) = n x^{n - 1} + a (n - 1) x^{n - 2} + a^{2} (n - 2) x^{n - 3} + . . . . + a^{n - 1}$

Question 7(i): For some constants a and b, find the derivative of $(x - a) (x - b)$

Answer:

Given

$f (x) = (x - a) (x - b) = x^{2} - a x - b x + a b$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying that property, we get

$f^{'} (x) = 2 x - a - b$

Question 7(ii): For some constants a and b, find the derivative of $(a x^{2} + b)^{2}$

Answer:

Given

$f (x) = (a x^{2} + b)^{2} = a^{2} x^{4} + 2 a b x^{2} + b^{2}$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying those properties, we get

$f^{'} (x) = 4 a^{2} x^{3} + 2 (2) a b x + 0$

$f^{'} (x) = 4 a^{2} x^{3} + 4 a b x$

$f^{'} (x) = 4 a x (a x^{2} + b)$

Question 7(iii): For some constants a and b, find the derivative of $\frac{x - a}{x - b}$

Answer:

Given,

$f (x) = \frac{x - a}{x - b}$

Now, as we know the quotient rule of derivative,

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} \frac{d y_{1}}{d x} - y_{1} \frac{d y_{2}}{d x}}{y_{2}^{2}}$

So,, applying this rule, we get

$\frac{d (\frac{x - a}{x - b})}{d x} = \frac{(x - b) \frac{d (x - a)}{d x} - (x - a) \frac{d (x - b)}{d x}}{(x - b)^{2}}$

$\frac{d (\frac{x - a}{x - b})}{d x} = \frac{(x - b) - (x - a)}{(x - b)^{2}}$

$\frac{d (\frac{x - a}{x - b})}{d x} = \frac{a - b}{(x - b)^{2}}$

Hence

$f^{'} (x) = \frac{a - b}{(x - b)^{2}}$

Question 8: Find the derivative of $\frac{x^{n} - a^{n}}{x - a}$ for some constant a.

Answer:

Given,

$f (x) = \frac{x^{n} - a^{n}}{x - a}$

Now, as we know the quotient rule of derivative,

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} \frac{d y_{1}}{d x} - y_{1} \frac{d y_{2}}{d x}}{y_{2}^{2}}$

So, applying this rule, we get

$\frac{d (\frac{x^{n} - a^{n}}{x - a})}{d x} = \frac{(x - a) \frac{d (x^{n} - a^{n})}{d x} - (x^{n} - a^{n}) \frac{d (x - a)}{d x}}{(x - a)^{2}}$

$\frac{d (\frac{x^{n} - a^{n}}{x - a})}{d x} = \frac{(x - a) n x^{n - 1} - (x^{n} - a^{n})}{(x - a)^{2}}$

$\frac{d (\frac{x^{n} - a^{n}}{x - a})}{d x} = \frac{n x^{n} - a n x^{n - 1} - x^{n} + a^{n}}{(x - a)^{2}}$

Hence

$f^{'} (x) = \frac{n x^{n} - a n x^{n - 1} - x^{n} + a^{n}}{(x - a)^{2}}$

Question 9(i): Find the derivative of $2 x - 3 / 4$

Answer:

Given:

$f (x) = 2 x - 3 / 4$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying that property, we get

$f^{'} (x) = 2 - 0$

$f^{'} (x) = 2$

Question 9(ii): Find the derivative of $(5 x^{3} + 3 x - 1) (x - 1)$

Answer:

Given.

$f (x) = (5 x^{3} + 3 x - 1) (x - 1) = 5 x^{4} + 3 x^{2} - x - 5 x^{3} - 3 x + 1$

$f (x) = 5 x^{4} - 5 x^{3} + 3 x^{2} - 4 x + 1$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying that property, we get

$f^{'} (x) = 5 (4) x^{3} - 5 (3) x^{2} + 3 (2) x - 4 + 0$

$f^{'} (x) = 20 x^{3} - 15 x^{2} + 6 x - 4$

Question 9(iii): Find the derivative of $x^{- 3} (5 + 3 x)$

Answer:

Given

$f (x) = x^{- 3} (5 + 3 x) = 5 x^{- 3} + 3 x^{- 2}$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying that property, we get

$f^{'} (x) = (- 3) 5 x^{- 4} + 3 (- 2) x^{- 3}$

$f^{'} (x) = - 15 x^{- 4} - 6 x^{- 3}$

Question 9(iv): Find the derivative of $x^{5} (3 - 6 x^{- 9})$

Answer:

Given

$f (x) = x^{5} (3 - 6 x^{- 9}) = 3 x^{5} - 6 x^{- 4}$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying that property, we get

$f^{'} (x) = (5) 3 x^{4} - 6 (- 4) x^{- 5}$

$f^{'} (x) = 15 x^{4} + 24 x^{- 5}$

Question 9(v): Find the derivative of $x^{- 4} (3 - 4 x^{- 5})$

Answer:

Given

$f (x) = x^{- 4} (3 - 4 x^{- 5}) = 3 x^{- 4} - 4 x^{- 9}$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying that property, we get

$f^{'} (x) = (- 4) 3 x^{- 5} - (- 9) 4 x^{- 10}$

$f^{'} (x) = - 12 x^{- 5} + 36 x^{- 10}$

Question 9(vi): Find the derivative of $\frac{2}{x + 1} - \frac{x^{2}}{3 x - 1}$

Answer:

Given

$f (x) = \frac{2}{x + 1} - \frac{x^{2}}{3 x - 1}$

As we know, the quotient rule of the derivative is:

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} \frac{d y_{1}}{d x} - y_{1} \frac{d y_{2}}{d x}}{y_{2}^{2}}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

So, applying this rule, we get

$\frac{d (\frac{2}{x + 1} - \frac{x^{2}}{3 x - 1})}{d x} = \frac{(x + 1) \frac{d (2)}{d x} - 2 \frac{d (x + 1)}{d x}}{(x + 1)^{2}} - \frac{(3 x - 1) \frac{d (x^{2})}{d x} - x^{2} \frac{d (3 x - 1)}{d x}}{(3 x - 1)^{2}}$

$\frac{d (\frac{2}{x + 1} - \frac{x^{2}}{3 x - 1})}{d x} = \frac{- 2}{(x + 1)^{2}} - \frac{(3 x - 1) 2 x - x^{2} 3}{(3 x - 1)^{2}}$

$\frac{d (\frac{2}{x + 1} - \frac{x^{2}}{3 x - 1})}{d x} = \frac{- 2}{(x + 1)^{2}} - \frac{6 x^{2} - 2 x - 3 x^{2}}{(3 x - 1)^{2}}$

$\frac{d (\frac{2}{x + 1} - \frac{x^{2}}{3 x - 1})}{d x} = \frac{- 2}{(x + 1)^{2}} - \frac{3 x^{2} - 2 x}{(3 x - 1)^{2}}$

Hence

$f^{'} (x) = \frac{- 2}{(x + 1)^{2}} - \frac{3 x^{2} - 2 x}{(3 x - 1)^{2}}$

Question 10: Find the derivative of $\cos x$ from the first principle.

Answer:

Given,

f(x)= $\cos x$

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\cos (x + h) - \cos (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\cos (x) \cos (h) - \sin (x) \sin (h) - \cos (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\cos (x) \cos (h) - \cos (x)}{h} - \frac{\sin (x) \sin (h)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\cos (x) (\cos (h) - 1)}{h} - \frac{\sin (x) \sin (h)}{h}$

$f^{'} (x) = lim_{h \to 0} \cos (x) \frac{- 2 s i n^{2} (h / 2)}{h} \cdot - \sin (x) \frac{s i n h}{h}$

$f^{'} (x) = \cos (x) (0) - s i n x (1)$

$f^{'} (x) = - \sin (x)$

Question 11(i): Find the derivative of the following functions: $\sin x \cos x$

Answer:

Given,

f(x)= $\sin x \cos x$

Now, as we know the product rule of derivative,

$\frac{d (y_{1} y_{2})}{d x} = y_{1} \frac{d y_{2}}{d x} + y_{2} \frac{d y_{1}}{d x}$

So, applying the rule here,

$\frac{d (\sin x \cos x)}{d x} = \sin x \frac{d \cos x}{d x} + \cos x \frac{d \sin x}{d x}$

$\frac{d (\sin x \cos x)}{d x} = \sin x (- \sin x) + \cos x (\cos x)$

$\frac{d (\sin x \cos x)}{d x} = - \sin^{2} x + \cos^{2} x$

$\frac{d (\sin x \cos x)}{d x} = \cos 2 x$

Question 11(ii): Find the derivative of the following functions: $\sec x$

Answer:

Given

$f (x) = \sec x = \frac{1}{\cos x}$

Now, as we know the quotient rule of derivative,

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} \frac{d y_{1}}{d x} - y_{1} \frac{d y_{2}}{d x}}{y_{2}^{2}}$

So, applying this rule, we get

$\frac{d (\frac{1}{\cos x})}{d x} = \frac{\cos x \frac{d (1)}{d x} - 1 \frac{d (\cos x)}{d x}}{\cos^{2} x}$

$\frac{d (\frac{1}{\cos x})}{d x} = \frac{- 1 (- \sin x)}{\cos^{2} x}$

$\frac{d (\frac{1}{\cos x})}{d x} = \frac{\sin x}{\cos^{2} x} = \frac{\sin x}{\cos x} \frac{1}{\cos x}$

$\frac{d (\sec x)}{d x} = \tan x \sec x$

Question 11 (iii): Find the derivative of the following functions: $5 \sec x + 4 \cos x$

Answer:

Given

$f (x) = 5 \sec x + 4 \cos x$

As we know, the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying the property, we get

$\frac{d (5 \sec x + 4 \cos x)}{d x} = \frac{d (5 \sec x)}{d x} + \frac{d (4 \cos x)}{d x}$

$\frac{d (5 \sec x + 4 \cos x)}{d x} = 5 \tan x \sec x + 4 (- \sin x)$

$\frac{d (5 \sec x + 4 \cos x)}{d x} = 5 \tan x \sec x - 4 \sin x$

Question 11(iv): Find the derivative of the following functions: $\csc x$

Answer:

Given :

$f (x) = \csc x = \frac{1}{\sin x}$

Now, as we know the quotient rule of derivative,

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} \frac{d y_{1}}{d x} - y_{1} \frac{d y_{2}}{d x}}{y_{2}^{2}}$

So, applying this rule, we get

$\frac{d (\frac{1}{\sin x})}{d x} = \frac{(\sin x) \frac{d (1)}{d x} - 1 \frac{d (\sin x)}{d x}}{(\sin x)^{2}}$

$\frac{d (\frac{1}{\sin x})}{d x} = \frac{- 1 (\cos x)}{(\sin x)^{2}}$

$\frac{d (\frac{1}{\sin x})}{d x} = - \frac{(\cos x)}{(\sin x)} \frac{1}{\sin x}$

$\frac{d (\csc x)}{d x} = - \cot x \csc x$

Question 11(v): Find the derivative of the following functions: $3 \cot x + 5 \csc x$

Answer:

Given,

$f (x) = 3 \cot x + 5 \csc x$

As we know, the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying the property,

$\frac{d (3 \cot x + 5 \csc x)}{d x} = \frac{d (3 \cot x)}{d x} + \frac{d (5 \csc x)}{d x}$

$\frac{d (3 \cot x + 5 \csc x)}{d x} = 3 \frac{d (\frac{\cos x}{\sin x})}{d x} + \frac{d (5 \csc x)}{d x}$

$\frac{d (3 \cot x + 5 \csc x)}{d x} = - 5 \csc x \cot x + 3 \frac{d (\frac{\cos x}{\sin x})}{d x}$

Now, as we know the quotient rule of the derivative,

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} \frac{d y_{1}}{d x} - y_{1} \frac{d y_{2}}{d x}}{y_{2}^{2}}$

So, applying this rule, we get

$\frac{d (3 \cot x + 5 \csc x)}{d x} = - 5 \csc x \cot x + 3 [\frac{\sin x \frac{d (\cos x)}{d x} - \cos x (\frac{d (\sin x)}{d x})}{\sin^{2} x}]$

$\frac{d (3 \cot x + 5 \csc x)}{d x} = - 5 \csc x \cot x + 3 [\frac{\sin x (- \sin x) - \cos x (\cos x)}{\sin^{2} x}]$

$\frac{d (3 \cot x + 5 \csc x)}{d x} = - 5 \csc x \cot x + 3 [\frac{- \sin^{2} x - \cos^{2} x}{\sin^{2} x}]$

$\frac{d (3 \cot x + 5 \csc x)}{d x} = - 5 \csc x \cot x - 3 [\frac{\sin^{2} x + \cos^{2} x}{\sin^{2} x}]$

$\frac{d (3 \cot x + 5 \csc x)}{d x} = - 5 \csc x \cot x - 3 [\frac{1}{\sin^{2} x}]$

$\frac{d (3 \cot x + 5 \csc x)}{d x} = - 5 \csc x \cot x - 3 \csc^{2} x$

Question 11(vi): Find the derivative of the following functions: $5 \sin x - 6 \cos x + 7$

Answer:

Given,

$f (x) = 5 \sin x - 6 \cos x + 7$

Now, as we know the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

So, applying the property,

$f^{'} (x) = 5 \cos x - 6 (- \sin x) + 0$

$f^{'} (x) = 5 \cos x + 6 (\sin x)$

$f^{'} (x) = 5 \cos x + 6 \sin x$

Question 11(vii): Find the derivative of the following functions: $2 \tan x - 7 \sec x$

Answer:

Given

$f (x) = 2 \tan x - 7 \sec x$

As we know, the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying this property,

$\frac{d (2 \tan x + 7 \sec x)}{d x} = 2 \frac{d \tan x}{d x} + 7 \frac{d \sec x}{d x}$

$\frac{d (2 \tan x + 7 \sec x)}{d x} = 2 \sec^{2} x + 7 (- \sec x \tan x)$

$\frac{d (2 \tan x + 7 \sec x)}{d x} = 2 \sec^{2} x - 7 \sec x \tan x$

NCERT Limits and Derivatives Class 11 Solutions: Exercise: Miscellaneous Exercise

Page Number: 253-254

Total Questions: 30

Question 1(i): Find the derivative of the following functions from the first principle: -x

Answer:

Given.

f(x)=-x

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{- (x + h) - (- x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{- h}{h}$

$f^{'} (x) = - 1$

Question 1 (ii): Find the derivative of the following functions from the first principle: $(- x)^{- 1}$

Answer:

Given.

f(x)= $(- x)^{- 1}$

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{- (x + h)^{- 1} - (- x)^{- 1}}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{- \frac{1}{x + h} + \frac{1}{x}}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\frac{- x + x + h}{x (x + h)}}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\frac{h}{(x + h) (x)}}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{1}{x (x + h)}$

$f^{'} (x) = \frac{1}{x^{2}}$

Question 1 (iii): Find the derivative of the following functions from the first principle: $\sin (x + 1)$

Answer:

Given.

$f (x) = \sin (x + 1)$

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\sin (x + h + 1) - \sin (x + 1)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{2 \cos (\frac{x + h + 1 + x + 1}{2}) \sin (\frac{x + h + 1 - x - 1}{2})}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{2 \cos (\frac{2 x + h + 2}{2}) \sin (\frac{h}{2})}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\cos (\frac{2 x + h + 2}{2}) \sin (\frac{h}{2})}{\frac{h}{2}}$

$f^{'} (x) = \cos (\frac{2 x + 0 + 2}{2}) \times 1$

$f^{'} (x) = \cos (x + 1)$

Question 1(iv): Find the derivative of the following functions from the first principle: $\cos (x - π / 8)$

Answer:

Given.

$f (x) = \cos (x - π / 8)$

Now, as we know, the derivative of any function at x is

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{\cos (x + h - π / 8) - c o s (x - π / 8)}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{- 2 \sin (\frac{x + h - π / 8 + x - π / 8}{2}) \sin (\frac{x + h - π / 8 - x + π / 8}{2})}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{- 2 \sin (\frac{2 x + h - π / 4}{2}) \sin (\frac{h}{2})}{h}$

$f^{'} (x) = lim_{h \to 0} \frac{- \sin (\frac{2 x + h - π / 4}{2}) \sin (\frac{h}{2})}{\frac{h}{2}}$

$f^{'} (x) = \sin (\frac{2 x + 0 - π / 4}{2}) \times 1$

$f^{'} (x) = - \sin (x - π / 8)$

Question 2: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): (x+a )

Answer:

Given

f(x)= x + a

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

Applying that property, we get

$f^{'} (x) = 1 + 0$

$f^{'} (x) = 1$

Question 3: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $(p x + q) (\frac{r}{x} + s)$

Answer:

Given

$f (x) = (p x + q) (\frac{r}{x} + s)$

$f (x) = p r + p s x + \frac{q r}{x} + q s$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

Applying that property, we get

$f^{'} (x) = 0 + p s + \frac{- q r}{x^{2}} + 0$

$f^{'} (x) = p s + q (\frac{- r}{x^{2}})$

$f^{'} (x) = p s - (\frac{q r}{x^{2}})$

Question 4: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $(a x + b) (c x + d)^{2}$

Answer:

Given,

$f (x) = (a x + b) (c x + d)^{2}$

$f (x) = (a x + b) (c^{2} x^{2} + 2 c d x + d^{2})$

$f (x) = a c^{2} x^{3} + 2 a c d x^{2} + a d^{2} x + b c^{2} x^{2} + 2 b c d x + b d^{2}$

Now,

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying that property, we get

$f^{'} (x) = 3 a c^{2} x^{2} + 4 a c d x + a d^{2} + 2 b c^{2} x + 2 b c d + 0$

$f^{'} (x) = 3 a c^{2} x^{2} + 4 a c d x + a d^{2} + 2 b c^{2} x + 2 b c d$

$f^{'} (x) = 3 a c^{2} x^{2} + (4 a c d + 2 b c^{2}) x + a d^{2} + 2 b c d$

Question 5: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $a x + b / c x + d$

Answer:

Given,

$f (x) = \frac{a x + b}{c x + d}$

Now, as we know, the derivative of any function

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

Hence, the derivative of f(x) is

$\frac{d (\frac{a x + b}{c x + d})}{d x} = \frac{(c x + d) d (\frac{d (a x + b)}{d x}) - (a x + b) (\frac{d (c x + d)}{d x})}{(c x + d)^{2}}$

$\frac{d (\frac{a x + b}{c x + d})}{d x} = \frac{(c x + d) a - (a x + b) c}{(c x + d)^{2}}$

$\frac{d (\frac{a x + b}{c x + d})}{d x} = \frac{a c x + a d - a c x - b c}{(c x + d)^{2}}$

$\frac{d (\frac{a x + b}{c x + d})}{d x} = \frac{a d - b c}{(c x + d)^{2}}$

Hence, the Derivative of the function is

$\frac{a d - b c}{(c x + d)^{2}}$ .

Question 6: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{1 + \frac{1}{x}}{1 - \frac{1}{x}}$

Answer:

Given,

$f (x) = \frac{1 + \frac{1}{x}}{1 - \frac{1}{x}}$

can also be written as

$f (x) = \frac{x + 1}{x - 1}$

Now, as we know, the derivative of any function

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

Hence, the derivative of f(x) is

$\frac{d (\frac{x + 1}{x - 1})}{d x} = \frac{(x - 1) d (\frac{d (x + 1)}{d x}) - (x + 1) (\frac{d (x - 1)}{d x})}{(x - 1)^{2}}$

$\frac{d (\frac{x + 1}{x - 1})}{d x} = \frac{(x - 1) 1 - (x + 1) 1}{(x - 1)^{2}}$

$\frac{d (\frac{x + 1}{x - 1})}{d x} = \frac{x - 1 - x - 1}{(x - 1)^{2}}$

$\frac{d (\frac{x + 1}{x - 1})}{d x} = \frac{- 2}{(x - 1)^{2}}$

Hence, the Derivative of the function is

$\frac{- 2}{(x - 1)^{2}}$

Question 7: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{1}{a x^{2} + b x + c}$

Answer:

Given,

$f (x) = \frac{1}{a x^{2} + b x + c}$

Now, as we know, the derivative of any such function is given by

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

Hence, the derivative of f(x) is

$\frac{d (\frac{1}{a x^{2} + b x + c})}{d x} = \frac{(a x^{2} + b x + c) d (\frac{d (1)}{d x}) - 1 (\frac{d (a x^{2} + b x + c)}{d x})}{(a x^{2} + b x + c)^{2}}$

$\frac{d (\frac{1}{a x^{2} + b x + c})}{d x} = \frac{0 - (2 a x + b)}{(a x^{2} + b x + c)^{2}}$

$\frac{d (\frac{1}{a x^{2} + b x + c})}{d x} = - \frac{(2 a x + b)}{(a x^{2} + b x + c)^{2}}$

Question 8: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{a x + b}{p x^{2} + q x + r}$

Answer:

Given,

$f (x) = \frac{a x + b}{p x^{2} + q x + r}$

Now, as we know, the derivative of any function

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

Hence, the derivative of f(x) is

$\frac{d (\frac{a x + b}{p x^{2} + q x + r})}{d x} = \frac{(p x^{2} + q x + r) d (\frac{d (a x + b)}{d x}) - (a x + b) (\frac{d (p x^{2} + q x + r)}{d x})}{(p x^{2} + q x + r)^{2}}$

$\frac{d (\frac{a x + b}{p x^{2} + q x + r})}{d x} = \frac{(p x^{2} + q x + r) a - (a x + b) (2 p x + q)}{(p x^{2} + q x + r)^{2}}$

$\frac{d (\frac{a x + b}{p x^{2} + q x + r})}{d x} = \frac{a p x^{2} + a q x + a r - 2 a p x^{2} - a q x - 2 b p x - b q}{(p x^{2} + q x + r)^{2}}$

$\frac{d (\frac{a x + b}{p x^{2} + q x + r})}{d x} = \frac{- a p x^{2} + a r - 2 b p x - b q}{(p x^{2} + q x + r)^{2}}$

Question 9: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{p x^{2} + q x + r}{a x + b}$

Answer:

Given,

$f (x) = \frac{p x^{2} + q x + r}{a x + b}$

Now, as we know, the derivative of any function

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

Hence, the derivative of f(x) is

$\frac{d (\frac{p x^{2} + q x + r}{a x + b})}{d x} = \frac{(a x + b) d (\frac{d (p x^{2} + q x + r)}{d x}) - (p x^{2} + q x + r) (\frac{d (a x + b)}{d x})}{(a x + b)^{2}}$

$\frac{d (\frac{p x^{2} + q x + r}{a x + b})}{d x} = \frac{(a x + b) (2 p x + q) - (p x^{2} + q x + r) (a)}{(a x + b)^{2}}$

$\frac{d (\frac{p x^{2} + q x + r}{a x + b})}{d x} = \frac{2 a p x^{2} + a q x + 2 b p x + b q - a p x^{2} - a q x - a r}{(a x + b)^{2}}$

$\frac{d (\frac{p x^{2} + q x + r}{a x + b})}{d x} = \frac{a p x^{2} + 2 b p x + b q - a r}{(a x + b)^{2}}$

Question 10: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{a}{x^{4}} - \frac{b}{x^{2}} + \cos x$

Answer:

Given

$f (x) = \frac{a}{x^{4}} - \frac{b}{x^{2}} + \cos x$

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying that property, we get

$f^{'} (x) = \frac{- 4 a}{x^{5}} - (\frac{- 2 b}{x^{3}}) + (- \sin x)$

$f^{'} (x) = \frac{- 4 a}{x^{5}} + \frac{2 b}{x^{3}} - \sin x$

Question 11: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers): $4 \sqrt{x} - 2$

Answer:

Given

$f (x) = 4 \sqrt{x} - 2$

It can also be written as

$f (x) = 4 x^{\frac{1}{2}} - 2$

Now,

As we know, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

And the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying that property, we get

$f^{'} (x) = 4 (\frac{1}{2}) x^{- \frac{1}{2}} - 0$

$f^{'} (x) = 2 x^{- \frac{1}{2}}$

$f^{'} (x) = \frac{2}{\sqrt{x}}$

Question 12: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$(a x + b)^{n}$

Answer:

Given

$f (x) = (a x + b)^{n}$

Now, ass we know the chain rule of derivative,

$[f (g (x))]^{'} = f^{'} (g (x)) \times g^{'} (x)$

And, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

Also the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying those properties, we get,

$f^{'} (x) = n (a x + b)^{n - 1} \times a$

$f^{'} (x) = a n (a x + b)^{n - 1}$

Question 13: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $(a x + b)^{n} (c x + d)^{m}$

Answer:

Given

$f (x) = (a x + b)^{n} (c x + d)^{m}$

Now, ass we know the chain rule of derivative,

$[f (g (x))]^{'} = f^{'} (g (x)) \times g^{'} (x)$

And the Multiplication property of the derivative,

$\frac{d (y_{1} y_{2})}{d x} = y_{1} \frac{d y_{2}}{d x} + y_{2} \frac{d y_{1}}{d x}$

And, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

Also the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying those properties, we get,

$f^{'} (x) = (a x + b)^{n} (m (c x + d)^{m - 1}) + (c x + d) (n (a x + b)^{n - 1})$

$f^{'} (x) = m (a x + b)^{n} (c x + d)^{m - 1} + n (c x + d) (a x + b)^{n - 1}$

Question 14: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers): $\sin (x + a)$

Answer:

Given,

$f (x) = \sin (x + a)$

Now, ass we know the chain rule of derivative,

$[f (g (x))]^{'} = f^{'} (g (x)) \times g^{'} (x)$

Applying this property, we get,

$f^{'} (x) = \cos (x + a) \times 1$

$f^{'} (x) = \cos (x + a)$

Question 15: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $\csc x \cot x$

Answer:

Given,

$f (x) = \csc x \cot x$

The Multiplication Property of the derivative,

$\frac{d (y_{1} y_{2})}{d x} = y_{1} \frac{d y_{2}}{d x} + y_{2} \frac{d y_{1}}{d x}$

Applying the property

$\frac{d (\csc x) (\cot x))}{d x} = \csc x \frac{d \cot x}{d x} + \cot x \frac{d \csc x}{d x}$

$\frac{d (\csc x) (\cot x))}{d x} = \csc x (- \csc^{2} x) + \cot x (- \csc x \cot x)$

$\frac{d (\csc x) (\cot x))}{d x} = - \csc^{3} x - \cot^{2} x \csc x$

Hence, the derivative of the function is $- \csc^{3} x - \cot^{2} x \csc x$ .

Question 16: Find the derivative of the following functions (it is to be understood that a, b, c, d,p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{\cos x}{1 + \sin x}$

Answer:

Given,

$f (x) = \frac{\cos x}{1 + \sin x}$

Now, as we know, the derivative of any function

$\frac{d (\frac{\cos x}{1 + \sin x})}{d x} = \frac{(1 + \sin x) d (\frac{d \cos x}{d x}) - \cos x (\frac{d (1 + \sin x)}{d x})}{(1 + \sin x)^{2}}$

Hence, the derivative of f(x) is

$\frac{d (\frac{\cos x}{1 + \sin x})}{d x} = \frac{(1 + \sin x) (- \sin x) - \cos x (\cos x)}{(1 + \sin x)^{2}}$

$\frac{d (\frac{\cos x}{1 + \sin x})}{d x} = \frac{- \sin x - \sin^{2} x - \cos^{2} x}{(1 + \sin x)^{2}}$

$\frac{d (\frac{\cos x}{1 + \sin x})}{d x} = \frac{- \sin x - 1}{(1 + \sin x)^{2}}$

$\frac{d (\frac{\cos x}{1 + \sin x})}{d x} = - \frac{1}{(1 + \sin x)}$

Question 17: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $\frac{\sin x + \cos x}{\sin x - \cos x}$

Answer:

Given

$f (x) = \frac{\sin x + \cos x}{\sin x - \cos x}$

can also be written as

$f (x) = \frac{\tan x + 1}{\tan x - 1}$

which further can be written as

$f (x) = - \frac{\tan x + t a n (π / 4)}{1 - \tan (π / 4) \tan x}$

$f (x) = - \tan (x - π / 4)$

Now,

$f^{'} (x) = - \sec^{2} (x - π / 4)$

$f^{'} (x) = - \frac{1}{\cos^{2} (x - π / 4)}$

Question 18: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{\sec x - 1}{\sec x + 1}$

Answer:

Given,

$f (x) = \frac{\sec x - 1}{\sec x + 1}$

which also can be written as

$f (x) = \frac{1 - \cos x}{1 + \cos x}$

Now,

As we know, the derivative of such a function

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

So, the derivative of the function is,

$\frac{d (\frac{1 - \cos x}{1 + \cos x})}{d x} = \frac{(1 + \cos x) d (\frac{d (1 - \cos x)}{d x}) - (1 - \cos x) (\frac{d (1 + \cos x)}{d x})}{(1 + \cos x)^{2}}$

$\frac{d (\frac{1 - \cos x}{1 + \cos x})}{d x} = \frac{(1 + \cos x) (- (- \sin x)) - (1 - \cos x) (- \sin x)}{(1 + \cos x)^{2}}$

$\frac{d (\frac{1 - \cos x}{1 + \cos x})}{d x} = \frac{\sin x + \sin x \cos x + \sin x - \cos x \sin x}{(1 + \cos x)^{2}}$

$\frac{d (\frac{1 - \cos x}{1 + \cos x})}{d x} = \frac{2 \sin x}{(1 + \cos x)^{2}}$

This can also be written as

$\frac{d (\frac{1 - \cos x}{1 + \cos x})}{d x} = \frac{2 \sec x \tan x}{(1 + \sec x)^{2}}$ .

Question 19: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $\sin^{n} x$

Answer:

Given,

$f (x) = \sin^{n} x$

Now, as we know the chain rule of derivative,

$[f (g (x))]^{'} = f^{'} (g (x)) \times g^{'} (x)$

And, the property,

$f^{'} (x^{n}) = n x^{n - 1}$

Applying those properties, we get

$f^{'} (x) = n \sin^{n - 1} x \cos x$

Hence Derivative of the given function is $n \sin^{n - 1} x \cos x$

Question 20: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{a + b \sin x}{c + d \cos x}$

Answer:

Given Function

$f (x) = \frac{a + b \sin x}{c + d \cos x}$

Now, as we know, the derivative of any function of this type is:

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

Hence, the derivative of the given function will be:

$\frac{d (\frac{a + b \sin x}{c + d \cos x})}{d x} = \frac{(c + d \cos x) (\frac{d (a + b \sin x)}{d x}) - (a + b \sin x) (\frac{d (c + d \cos x x)}{d x})}{(c + d \cos x)^{2}}$

$\frac{d (\frac{a + b \sin x}{c + d \cos x})}{d x} = \frac{(c + d \cos x) (b \cos x) - (a + b \sin x) (d (- \sin x))}{(c + d \cos x)^{2}}$

$\frac{d (\frac{a + b \sin x}{c + d \cos x})}{d x} = \frac{c b \cos x + b d \cos^{2} x + a d \sin x + b d \sin^{2} x}{(c + d \cos x)^{2}}$

$\frac{d (\frac{a + b \sin x}{c + d \cos x})}{d x} = \frac{c b \cos x + a d \sin x + b d (\sin^{2} x + \cos^{2} x)}{(c + d \cos x)^{2}}$

$\frac{d (\frac{a + b \sin x}{c + d \cos x})}{d x} = \frac{c b \cos x + a d \sin x + b d}{(c + d \cos x)^{2}}$

Question 21: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{\sin (x + a)}{\cos x}$

Answer:

Given,

$f (x) = \frac{\sin (x + a)}{\cos x}$

Now, as we know, the derivative of any function

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

Hence, the derivative of the given function is:

$\frac{d (\frac{\sin (x + a)}{\cos x})}{d x} = \frac{(\cos x) (\frac{d (\sin (x + a))}{d x}) - \sin (x + a) (\frac{d (\cos x)}{d x})}{(\cos x)^{2}}$

$\frac{d (\frac{\sin (x + a)}{\cos x})}{d x} = \frac{(\cos x) (\cos (x + a)) - \sin (x + a) (- \sin (x))}{(\cos x)^{2}}$

$\frac{d (\frac{\sin (x + a)}{\cos x})}{d x} = \frac{(\cos x) (\cos (x + a)) + \sin (x + a) (\sin (x))}{(\cos x)^{2}}$

$\frac{d (\frac{\sin (x + a)}{\cos x})}{d x} = \frac{\cos (x + a - x)}{(\cos x)^{2}}$

$\frac{d (\frac{\sin (x + a)}{\cos x})}{d x} = \frac{\cos (a)}{(\cos x)^{2}}$

Question 22: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers): $x^{4} (5 \sin x - 3 \cos x)$

Answer:

Given

$f (x) = x^{4} (5 \sin x - 3 \cos x)$

Now, as we know, the Multiplication property of the derivative,

$\frac{d (y_{1} y_{2})}{d x} = y_{1} \frac{d y_{2}}{d x} + y_{2} \frac{d y_{1}}{d x}$

Hence, the derivative of the given function is:

$\frac{d (x^{4} (5 \sin x - 3 \cos x))}{d x} = x^{4} \frac{d (5 \sin x - 3 \cos x)}{d x} + (5 \sin x - 3 \cos x) \frac{d x^{4}}{d x}$

$\frac{d (x^{4} (5 \sin x - 3 \cos x))}{d x} = x^{4} (5 \cos x + 3 \sin x) + (5 \sin x - 3 \cos x) 4 x^{3}$

$\frac{d (x^{4} (5 \sin x - 3 \cos x))}{d x} = 5 x^{4} \cos x + 3 x^{4} \sin x + 20 x^{3} \sin x - 12 x^{3} \cos x$

Question 23: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $(x^{2} + 1) \cos x$

Answer:

Given

$f (x) = (x^{2} + 1) \cos x^{}$

Now, as we know the product rule of derivative,

$\frac{d (y_{1} y_{2})}{d x} = y_{1} \frac{d y_{2}}{d x} + y_{2} \frac{d y_{1}}{d x}$

The derivative of the given function is

$\frac{d ((x^{2} + 1) \cos x)}{d x} = (x^{2} + 1) \frac{d \cos x}{d x} + \cos x \frac{d (x^{2} + 1)}{d x}$

$\frac{d ((x^{2} + 1) \cos x)}{d x} = (x^{2} + 1) (- \sin x) + \cos x (2 x)$

$\frac{d ((x^{2} + 1) \cos x)}{d x} = - x^{2} \sin x - \sin x + 2 x \cos x$

Question 24: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $(a x^{2} + \sin x) (p + q \cos x)$

Answer:

Given,

$f (x) = (a x^{2} + \sin x) (p + q \cos x)$

Now, as we know, the Multiplication property of the derivative (the product rule)

$\frac{d (y_{1} y_{2})}{d x} = y_{1} \frac{d y_{2}}{d x} + y_{2} \frac{d y_{1}}{d x}$

And also the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying those properties, we get,

$\frac{d ((a x^{2} + \sin x) (p + q \cos x))}{d x} = (a x^{2} + \sin x) \frac{d (p + q \cos x)}{d x} + (p + q x) \frac{d (a x^{2} + s i n x)}{d x}$

$\frac{d ((a x^{2} + \sin x) (p + q \cos x))}{d x} = (a x^{2} + \sin x) (- q \sin x) + (p + q x) (2 a x + \cos x)$

$f^{'} (x) = - a q x^{2} \sin x - q \sin^{2} x + 2 a p x + p \cos x + 2 a q x^{2} + q x \cos x$

$f^{'} (x) = x^{2} (- a q \sin x + 2 a q) + x (2 a p + q \cos x) + p \cos x - q \sin^{2} x$

Question 25: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $(x + \cos x) (x - \tan x)$

Answer:

Given,

$f (x) = (x + \cos x) (x - \tan x)$

And the Multiplication property of the derivative,

$\frac{d (y_{1} y_{2})}{d x} = y_{1} \frac{d y_{2}}{d x} + y_{2} \frac{d y_{1}}{d x}$

Also the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying those properties, we get,

$\frac{d ((x + \cos x) (x - \tan x))}{d x} = (x + \cos x) \frac{d (x - \tan x)}{d x} + (x - \tan x) \frac{d (x + \cos x)}{d x}$

$= (x + \cos x) (1 - \sec^{2} x) + (x - \tan x) (1 - \sin x)$

$= (x + \cos x) (- \tan^{2} x) + (x - \tan x) (1 - \sin x)$

$= (- \tan^{2} x) (x + \cos x) + (x - \tan x) (1 - \sin x)$

Question 26: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r, and s are fixed non-zero constants and m and n are integers):

$\frac{4 x + 5 \sin x}{3 x + 7 \cos x}$

Answer:

Given,

$f (x) = \frac{4 x + 5 \sin x}{3 x + 7 \cos x}$

Now, as we know, the derivative of any function

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

Also the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying those properties, we get

$\frac{d (\frac{4 x + 5 \sin x}{3 x + 7 \cos x})}{d x} = \frac{(3 x + 7 \cos x) d (\frac{d (4 x + 5 \sin x)}{d x}) - (4 x + 5 \sin x) (\frac{d (3 x + 7 \cos x)}{d x})}{(3 x + 7 \cos x)^{2}}$

$\frac{d (\frac{4 x + 5 \sin x}{3 x + 7 \cos x})}{d x} = \frac{(3 x + 7 \cos x) (4 + 5 \cos x) - (4 x + 5 \sin x) (3 - 7 \sin x)}{(3 x + 7 \cos x)^{2}}$

$= \frac{12 x + 28 \cos x + 15 x \cos x + 35 \cos^{2} x - 12 x - 15 \sin x + 28 x \sin x + 35 \sin^{2} x}{(3 x + 7 \cos x)^{2}}$

$= \frac{12 x + 28 \cos x + 15 x \cos x - 12 x - 15 \sin x + 28 x \sin x + 35 (\sin^{2} x + \cos^{2} x)}{(3 x + 7 \cos x)^{2}}$

$= \frac{28 \cos x + 15 x \cos x - 15 \sin x + 28 x \sin x + 35}{(3 x + 7 \cos x)^{2}}$

Question 27: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q,,r, and s are fixed non-zero constants and m and n are integers):

$\frac{x^{2} \cos (π / 4)}{\sin x}$

Answer:

Given,

$f (x) = \frac{x^{2} \cos (π / 4)}{\sin x}$

As we know, the derivative of any function

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

Hence, the derivative of the given function is

$\frac{d (\frac{x^{2} \cos (π / 4)}{\sin x})}{d x} = \frac{(\sin x) d (\frac{d (x^{2} \cos (π / 4))}{d x}) - (x^{2} \cos (π / 4)) (\frac{d \sin x}{d x})}{\sin^{2} x}$

$\frac{d (\frac{x^{2} \cos (π / 4)}{\sin x})}{d x} = \frac{(\sin x) (2 x \cos (π / 4)) - (x^{2} \cos (π / 4)) (\cos x)}{\sin^{2} x}$

$\frac{d (\frac{x^{2} \cos (π / 4)}{\sin x})}{d x} = \frac{2 x \sin x \cos (π / 4) - x^{2} \cos x \cos (π / 4)}{\sin^{2} x}$

Question 28: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q,, r, and s are fixed non-zero constants and m and n are integers):

$\frac{x}{1 + \tan x}$

Answer:

Given

$f (x) = \frac{x}{1 + \tan x}$

Noas As we know, the derivative of any function

$\frac{d (\frac{x}{1 + \tan x})}{d x} = \frac{(1 + \tan x) d (\frac{d x}{d x}) - x (\frac{d (1 + \tan x)}{d x})}{(1 + \tan x)^{2}}$

$\frac{d (\frac{x}{1 + \tan x})}{d x} = \frac{(1 + \tan x) (1) - x (\sec^{2} x)}{(1 + \tan x)^{2}}$

$\frac{d (\frac{x}{1 + \tan x})}{d x} = \frac{1 + \tan x - x \sec^{2} x}{(1 + \tan x)^{2}}$

Question 29: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q, r and s are fixed non-zero constants and m and n are integers): $(x + \sec x) (x - \tan x)$

Answer:

Given

$f (x) = (x + \sec x) (x - \tan x)$

Now, ass we know the Multiplication property of the derivative,

$\frac{d (y_{1} y_{2})}{d x} = y_{1} \frac{d y_{2}}{d x} + y_{2} \frac{d y_{1}}{d x}$

Also the property

$\frac{d (y_{1} + y_{2})}{d x} = \frac{d y_{1}}{d x} + \frac{d y_{2}}{d x}$

Applying those properties, we get,

The derivative of the given function is,

$\frac{d ((x + \sec x) (x - \tan x)}{d x} = (x + \sec x) \frac{d (x - \tan x)}{d x} + (x - \tan x) \frac{d (x + \sec x)}{d x}$

$\frac{d ((x + \sec x) (x - \tan x)}{d x} = (x + \sec x) (1 - \sec^{2} x) + (x - \tan x) (1 + \sec x \tan x)$

Question 30: Find the derivative of the following functions (it is to be understood that a, b, c, d, p, q,,r, and s are fixed non-zero constants and m and n are integers):

$\frac{x}{\sin^{n} x}$

Answer:

Given,

$f (x) = \frac{x}{\sin^{n} x}$

As we know, the derivative of any function

$\frac{d (\frac{y_{1}}{y_{2}})}{d x} = \frac{y_{2} d (\frac{d y_{1}}{d x}) - y_{1} (\frac{d y_{2}}{d x})}{y_{2}^{2}}$

As the chain rule of derivatives,

$[f (g (x))]^{'} = f^{'} (g (x)) \times g^{'} (x)$

Hence, the derivative of the given function is

$\frac{d (\frac{x}{\sin^{n} x})}{d x} = \frac{\sin^{n} x d (\frac{d x}{d x}) - x (\frac{d (\sin^{n} x)}{d x})}{s i n^{2 n} x}$

$\frac{d (\frac{x}{\sin^{n} x})}{d x} = \frac{\sin^{n} x (1) - x (n \sin^{n - 1} x \times \cos x)}{s i n^{2 n} x}$

$\frac{d (\frac{x}{\sin^{n} x})}{d x} = \frac{\sin^{n} x - x \cos x n \sin^{n - 1} x}{s i n^{2 n} x}$

$\frac{d (\frac{x}{\sin^{n} x})}{d x} = \frac{\sin^{n - 1} x (\sin x - n x \cos x)}{s i n^{2 n} x}$

$\frac{d (\frac{x}{\sin^{n} x})}{d x} = \frac{(\sin x - n x \cos x)}{s i n^{n + 1} x}$

Also, read,

Class 11 Maths NCERT Chapter 12: Extra Question

Question:
$lim_{x \to 0} cosec x (\sqrt{2 \cos^{2} x + 3 \cos x} - \sqrt{\cos^{2} x + \sin x + 4})$ is

Solution:
Evaluate the limit:
$lim_{x \to 0} \csc x (\sqrt{2 \cos^{2} x + 3 \cos x} - \sqrt{\cos^{2} x + \sin x + 4})$

Rationalise the expression:
$lim_{x \to 0} \frac{\csc x ((2 \cos^{2} x + 3 \cos x) - (\cos^{2} x + \sin x + 4))}{\sqrt{2 \cos^{2} x + 3 \cos x} + \sqrt{\cos^{2} x + \sin x + 4}}$

Simplify the numerator:
$lim_{x \to 0} \frac{1}{\sin x} \cdot \frac{(\cos^{2} x + 3 \cos x - 4) - \sin x}{\sqrt{2 \cos^{2} x + 3 \cos x} + \sqrt{\cos^{2} x + \sin x + 4}}$

Rewrite the numerator:
$lim_{x \to 0} \frac{(\cos x + 4) (\cos x - 1) - \sin x}{\sin x (\sqrt{2 \cos^{2} x + 3 \cos x} + \sqrt{\cos^{2} x + \sin x + 4})}$

Using half-angle formulas:
$lim_{x \to 0} \frac{- 2 \sin^{2} \frac{x}{2} (\cos x + 4) - 2 \sin \frac{x}{2} \cos \frac{x}{2}}{2 \sin \frac{x}{2} \cos \frac{x}{2} (\sqrt{2 \cos^{2} x + 3 \cos x} + \sqrt{\cos^{2} x + \sin x + 4})}$

Cancel common terms:

$lim_{x \to 0} - \frac{\sin \frac{x}{2} (\cos x + 4) + \cos \frac{x}{2}}{\cos \frac{x}{2} (\sqrt{2 \cos^{2} x + 3 \cos x} + \sqrt{\cos^{2} x + \sin x + 4})}$

Evaluate the limit by plugging $x = 0$ :
$= - \frac{1}{2 \sqrt{5}}$

Hence, the correct answer is $- \frac{1}{2 \sqrt{5}}$ .

Approach to Solve Questions of Limits and Derivatives Class 11

Here are some approaches that students can use to approach the questions related to Limits and derivatives.

Get familiar with the properties of limits and derivatives, such as the sum rule, constant rule, product rule, quotient rule, etc.
Get clarity about the standard limits and derivatives formulas.
Check the indeterminate forms in limits first.
Before applying the rules of limits or derivatives, simplify the expression or solve it part by part.
For derivatives, decide which is better to use: the first principle of the standard rules.
In higher classes, practice using factorisation or L'Hospital's rule to resolve indeterminate forms.
Prepare a chart of all the standard derivatives in a notebook to revise from time to time for fast recall.

What Extra Should Students Study Beyond NCERT for JEE?

Concept Name	JEE	NCERT
Limit	✅	✅
Left-Hand Limits and Right-Hand Limits	✅	✅
Algebra of Limits	✅	✅
Limit of Indeterminate Form and Algebraic limit	✅	✅
Limit of Algebraic function	✅	✅
Limit Using Expansion	✅	✅
Sandwich Theorem	✅	✅
Limits of Trigonometric Functions	✅	✅
Exponential and Logarithmic Limits	✅	✅
Limits of the form (1 power infinity)	✅
L’ Hospital’s Rule	✅
Limit of the form (0 power 0 or infinity power 0)	✅	✅
Differentiation	✅
Derivative of Polynomials and Trigonometric Functions	✅	✅
Differentiation Rules	✅
Differentiation of Implicit Function	✅
Differentiation of Function in Parametric Form	✅
Differentiation of Inverse Trigonometric Function (cos/sine/tan)	✅
Differentiation Using Logarithm	✅
Differentiation of a Function wrt Another Function and Higher Order derivative of a Function	✅
Differentiation of Determinants	✅
Differentiation of Function and Relation	✅
Differentiation of Inverse Function	✅
Continuity and Discontinuity	✅
Directional Continuity and Continuity over an Interval	✅
Non - Removable, Infinite and Oscillatory Type Discontinuity	✅
Continuity of Composite Function	✅
The Intermediate Value Theorem	✅
Differentiability and Existence of Derivative	✅
Examining differentiability Using Graph of Function	✅
Continuity And Differentiability	✅
Differentiability of Composite Function	✅
Derivative as Rate Measure	✅	✅
Approximations and Errors using Derivatives	✅	✅
Tangent to the Curve at a Point	✅
Angle of Intersection between Two Curves	✅
Length of Tangent and Normal and Subtangent and subnormal	✅
Rolle’s Theorem	✅
Monotonicity and Extremum of Functions	✅
Inflection Point	✅
Maxima and Minima in Calculus	✅	✅
First Derivative Test	✅	✅
Application of Monotonicity	✅
Nature of Roots of Cubic Polynomial	✅
Application of Extremum in Plane Geometry and Solid geometry	✅
Continuity and Discontinuity obtained by Algebraic Operations	✅

NCERT Solutions for Class 11 Mathematics - Chapter Wise

NCERT Solutions for Class 11 Mathematics Chapter 1: Sets

NCERT Solutions for Class 11 Mathematics Chapter 2: Relations and Functions

NCERT Solutions for Class 11 Mathematics Chapter 3: Trigonometric Functions

NCERT Solutions for Class 11 Mathematics Chapter 4: Complex Numbers and Quadratic Equations

NCERT Solutions for Class 11 Mathematics Chapter 5: Linear Inequalities

NCERT Solutions for Class 11 Mathematics Chapter 6: Permutations and Combinations

NCERT Solutions for Class 11 Mathematics Chapter 7: Binomial Theorem

NCERT Solutions for Class 11 Mathematics Chapter 8: Sequences and Series

NCERT Solutions for Class 11 Mathematics Chapter 9: Straight Lines

NCERT Solutions for Class 11 Mathematics Chapter 10: Conic Sections

NCERT Solutions for Class 11 Mathematics Chapter 11: Introduction to Three-Dimensional Geometry

NCERT Solutions for Class 11 Mathematics Chapter 12: Limits and Derivatives

NCERT Solutions for Class 11 Mathematics Chapter 13: Statistics

NCERT Solutions for Class 11 Mathematics Chapter 14: Probability

Also, read,

NCERT Solutions for Class 11- Subject Wise

Students can check the following links for more in-depth learning.

NCERT Books and NCERT Syllabus

Here is the latest NCERT syllabus, that is useful for students before strategising their study plan. Also, it contains links to some reference books which are important for further studies.

Frequently Asked Questions (FAQs)

1. What are the important topics covered in NCERT Solutions for Class 11 Maths Chapter 12?

Important Topics in NCERT Solutions for Class 11 Maths Chapter 12 (Limits and Derivatives)

This chapter introduces limits and derivatives, forming the foundation of calculus.

1. Limits: Understanding approaching values, left-hand and right-hand limits, and standard limits like:

$lim_{x \to 0} \frac{\sin x}{x} = 1$

$lim_{x \to 0} (1 + x)^{n} = e^{x}$

2. Limit Evaluation Methods:

Direct substitution, factorization, rationalization.

3. Derivatives: First principle definition:

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$

Power rule, sum, product, and quotient rules.

2. How to solve limit problems in Class 11 Maths Chapter 12?

To solve limit problems in Class 11 Maths Chapter 12 (Limits and Derivatives), follow these steps:
1. Substitution: Directly put the given value of $x$ .
2. Factorization: Factor and cancel common terms if you get $\frac{0}{0}$ .
3. Rationalization: Multiply by the conjugate for roots.
4. Trigonometric Limits: Use identities like $lim_{x \to 0} \frac{\sin x}{x} = 1$ .
5. L'Hôpital's Rule (for indeterminate forms): Differentiate numerator and denominator separately.

3. What are the basic formulas of limits and derivatives in Class 11?

Basic Formulas of Limits and Derivatives in Class 11

Limits

1. $lim_{x \to a} c = c$

2. $lim_{x \to a} x = a$

3. $lim_{x \to a} x^{n} = a^{n}$

4. $lim_{x \to 0} \frac{\sin x}{x} = 1$

5. $lim_{x \to 0} \frac{1 - \cos x}{x} = 0$

Derivatives

1. $\frac{d}{d x} (c) = 0$

2. $\frac{d}{d x} (x^{n}) = n x^{n - 1}$

3. $\frac{d}{d x} (\sin x) = \cos x$

4. $\frac{d}{d x} (\cos x) = - \sin x$

5. $\frac{d}{d x} (e^{x}) = e^{x}$

4. How do you find derivatives using the first principle in NCERT Class 11 Maths?

To find derivatives using the first principle, we use the definition:

$f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$
Steps to Find the Derivative Using the First Principle:
1. Substitute $f (x + h)$ into the formula.
2. Find $f (x + h) - f (x)$ .
3. Simplify the expression and divide by $h$ .
4. Apply the limit $h \to 0$ .

Example: $f (x) = x^{2}$

$f^{'} (x) = lim_{h \to 0} \frac{(x + h)^{2} - x^{2}}{h} = lim_{h \to 0} \frac{x^{2} + 2 x h + h^{2} - x^{2}}{h} = lim_{h \to 0} (2 x + h) = 2 x$

5. What is the easiest way to understand limits and derivatives?

Easiest Way to Understand Limits and Derivatives
1. Limits (Approaching a Value)
Think of limits as "getting closer to a value" without necessarily reaching it.
Example: $lim_{x \to 2} (x^{2}) = 4$ , as $x^{2}$ gets closer to 4 when $x$ approaches 2 .
Use substitution first; if it gives $\frac{0}{0}$ , try factoring or rationalizing.
2. Derivatives (Rate of Change)
The derivative measures how a function changes at a point (slope of a curve).
Formula: $f^{'} (x) = lim_{h \to 0} \frac{f (x + h) - f (x)}{h}$ .
Start with simple functions like $x^{2}$ to see patterns in differentiation.

Also Read

NCERT Solutions for Class 11 Maths Chapter 2 Relations And Functions

NCERT Solutions for Class 11 Maths Chapter 5 Linear Inequalities

NCERT Solutions for Class 11 Maths Chapter 3 Trigonometric Functions

NCERT Solutions for Class 11 Maths Chapter 1 Sets

NCERT Solutions for Class 11 Biology Chapter 19 Chemical Coordination and Integration

NCERT Solutions for Class 11 Biology Chapter 16 Excretory Products and Their Elimination

Some basic concepts of Chemistry Class 11th Notes - Free NCERT Class 11 Chemistry Chapter 1 Notes - Download PDF

NCERT Class 11 Biology Chapter 13 Notes Photosynthesis In Higher Plants - Download PDF Notes

NCERT Class 11 Biology Chapter 14 Notes Respiration In Plants - Download PDF Notes

NCERT Class 11 Biology Chapter 15 Notes Plant Growth And Development- Download PDF Notes

NCERT Class 11 Biology Chapter 22 Notes Chemical Coordination And Integration- Download PDF Notes

NCERT Class 11 Biology Chapter 21 Notes Neural Control And Coordination- Download PDF Notes

NCERT Exemplar Class 11 Chemistry Solutions Chapter 9 Hydrogen

NCERT Exemplar Class 11 Chemistry Solutions Chapter 8 Redox Reactions

NCERT Exemplar Class 11 Chemistry Solutions Chapter 2 Structure of Atom

NCERT Exemplar Class 11 Solutions - Subject Wise Problems with Solutions

NCERT Exemplar Class 11 Biology Solutions Chapter 1 The Living World

NCERT Exemplar Class 11 Biology Solutions Chapter 21 Neural Control and Coordination

NCERT Books for class 11 Maths 2025-26: Download Free PDF

NCERT Book for class 11 Biology 2025-26: Download Free PDF

NCERT Books for Class 11 Physics 2025-26: Download PDF

NCERT Books for Class 11 Chemistry 2025-26: Download Free PDF

NCERT Books for Class 11 English 2025-26: Download Free PDF

NCERT Books for class 11 Hindi 2025-26: Download Free PDF

NCERT Syllabus for Class 11 Physics 2025-26 - Download Latest Syllabus Pdf

Articles

UP Scholarships for General Category Students: Eligibility & Application Guide

Jun 19, 2025

Tamil Nadu BSc Nursing Admission 2025: Fees, Eligibility Criteria, Application, Top Colleges

Jun 19, 2025

JAC Delhi Merit List 2025 – Download and Check College wise Cut offs

Jun 18, 2025

IOQM Admit Card 2025: Download Indian Olympiad Qualifier in Mathematics Hall Ticket

Jun 18, 2025

DU LLB Counselling 2025 (Started) – First Allotment (Out), Documents, Admission Process

Jun 18, 2025

Silverzone ITHO Olympiad 2025 – Exam Date, Syllabus, Eligibility & Results

Jun 17, 2025

Silverzone ISSO Result 2024-25 Out : Check International Social Studies Olympiad Results Here

Jun 17, 2025

Silverzone Commerce Olympiad Answer Key 2024: ICSI Question Paper Solution

Jun 17, 2025

Upcoming School Exams

Bihar Open Board 10th Examination

Application Date:16 May,2025 - 30 June,2025

Result

Admit Card

Bihar Open Board 12th Examination

Application Date:16 May,2025 - 30 June,2025

Result

Admit Card

National Institute of Open Schooling 10th examination

Application Date:24 May,2025 - 23 June,2025

Result

Admit Card

Mock Test

National Institute of Open Schooling 12th Examination

Application Date:24 May,2025 - 23 June,2025

Result

Mock Test

Admit Card

Chattisgarh State Open School 12th Examination

Application Date:01 June,2025 - 30 June,2025

Result

Admit Card

View All School Exams

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

Option 1) less than 3	Option 2) more than 3 but less than 6
Option 3) more than 6 but less than 9	Option 4) more than 9

Exams

Top Schools

Products & Resources

NCERT Study Material

Exams

Colleges

Predictors

Resources

Exams

Colleges & Courses

Predictors

Resources

Exams

Colleges

Predictors

Resources

Exams

Colleges

Predictors & E-Books

Resources

Exams

Predictors & Articles

Colleges

Resources

Exams

Colleges

Resources

Exams

Resources

Top Courses & Careers

Colleges

Exams

Colleges

Resources

Quick Links

Exams

Colleges

Resources

Exams

Colleges

Resources

Diploma Colleges

Exams

Resources

Upcoming Events

Other Exams

Exams

Colleges

Top Countries

Resources

Exams

Colleges

Upcoming Events

Resources

Engineering Preparation

Medical Preparation

Law Preparation

MBA Preparation

Top Streams

Specializations

Resources

Top Providers

NCERT Solutions for Class 11 Maths Chapter 12 Limits and Derivatives

NCERT Solution for Class 11 Maths Chapter 12 Solutions: Download PDF

Limits and Derivatives Class 11 Solutions: Important Formulae

Properties of Limits:

Standard Limits:

Derivatives:

Standard Derivatives:

NCERT Solutions for Class 11 Maths Chapter 12: Exercise Questions

Class 11 Maths NCERT Chapter 12: Extra Question

Approach to Solve Questions of Limits and Derivatives Class 11

What Extra Should Students Study Beyond NCERT for JEE?

NCERT Solutions for Class 11 Mathematics - Chapter Wise

NCERT Solutions for Class 11- Subject Wise

NCERT Books and NCERT Syllabus

Frequently Asked Questions (FAQs)

Also Read

Articles

Upcoming School Exams