NCERT Exemplar Class 11 Maths Solutions Chapter 10 Straight Lines

Edited By Ravindra Pindel | Updated on Sep 12, 2022 06:05 PM IST

NCERT Exemplar Class 11 Maths solutions chapter 10 are prepared by our experts for helping students to understand and grasp the very basics of coordinate geometry. NCERT Exemplar solutions for Class 11 Maths chapter 10 focuses on the representation of a straight line in coordinate geometry. This is done through the determination of the slope of the straight line with reference to the previously studied concept of algebra and geometry.

JEE Main Scholarship Test Kit (Class 11): Narayana | Physics Wallah | Aakash | Unacademy

Suggested: JEE Main: high scoring chapters | Past 10 year's papers

This Story also Contains

NCERT Exemplar Class 11 Maths Solutions Chapter 10: Exercise: 10.3
More About NCERT Exemplar Class 11 Maths Chapter 10
Topics and Subtopics in NCERT Exemplar Class 11 Maths Solutions Chapter 10
What will the students learn from NCERT Exemplar Class 11 Maths Solutions Chapter 10?
NCERT Solutions for Class 11 Mathematics Chapters
Important Topics To Cover in NCERT Exemplar Class 11 Maths Solutions Chapter 10

NCERT Exemplar Class 11 Maths solutions chapter 10 provide detailed and simple explanations for NCERT problems that help students in their competitive exams as well. The solutions are drafted very carefully by a detailed study of the concepts and exam pattern of CBSE, keeping the important topics in mind for better understanding and preparation.

Also, check - NCERT Solutions for Class 11

NCERT Exemplar Class 11 Maths Solutions Chapter 10: Exercise: 10.3

Question:1

Find the equation of the straight line which passes through the point (1, – 2) and cuts off equal intercepts from axes.

Answer:

Equation of line in intercept form= $\frac{x}{a}+\frac{y}{b}=1$
Given that $a=b; \frac{x}{a}+\frac{y}{b}=1$
$\frac{x+y}{a}=1$
x+y=a……(1)
If equation (1) passes through (1,-2) we get 1+(-2) =a
1-2=a
a=-1 Putting the value of'a'in equation 1we get x+y=-1
Equation of straight line is x+y+1=0

Question:2

Find the equation of the line passing through the point (5, 2) and perpendicular to the line joining the points (2, 3) and (3, – 1).

Answer:

Given points are A(5,2) B(2,3) and C(3,-1).
Slopeof the line joining points B and C = $\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{-1-3}{3-2}=-\frac{4}{1}=-4$
It is given that line passing through the point A is perpendicular to BC
m₁*m₂= -1
-4*m₂=-1
m₂=1/4
Equation of line passing through point A
Equation of line: y-y₁= m(x-x₁₎
y-2=1/4 (x-5)
4y-8=x-5
x-5-4y+8=0
Equation of straight line passing through point A is x-4y+3=0

Question:3

Find the angle between the lines $y=\left ( 2-\sqrt{3} \right )\left ( x+5 \right )$ and $y=\left ( 2+\sqrt{3} \right )\left ( x-7 \right )$

Answer:

Given equations are $y=\left ( 2-\sqrt{3} \right )\left ( x+5 \right )$
$y=\left ( 2-\sqrt{3} \right )x+\left ( 2-\sqrt{3} \right )5$ ................(1)
and $y=\left ( 2+\sqrt{3} \right )\left ( x-7 \right )$
$y=\left ( 2+\sqrt{3} \right ) x-7 \left ( 2+\sqrt{3} \right )$ ............(2)
In equation 1 the slope is $\left ( 2-\sqrt{3} \right )$ as it is in the form of y=mx+b and in equation 2 it is $2+\sqrt{3}$
Let θ be the angle between the given m₁ and m₂ two lines $\tan \theta =\left |\frac{\left ( m_{1}-m_{2} \right )}{1+m_{1}m_{2}} \right |$
Putting the values of m₁and m₂in above equation we get
$\tan \theta =\left |\frac{2-\sqrt{3}-\left ( 2+\sqrt{3} \right )}{1+\left ( 2-\sqrt{3} \right )\left (2+\sqrt{3} \right )} \right |$
$=\left | -\frac{2\sqrt{3}}{1+\left ( 4-3 \right )} \right |$
$=\left | -\frac{2\sqrt{3}}{2} \right |$
$= \sqrt{3}$
$\theta =\tan ^{-1}\sqrt{3}$
θ=60⁰

Question:4

Find the equation of the lines which passes through the point (3, 4) and cuts off intercepts from the coordinate axes such that their sum is 14.

Answer:

Equation of line in intercept form = $\frac{x}{a}+\frac{y}{b}=1$
Given that a+b=14 b=14-a
So equation of line $\frac{x}{a}+\frac{y}{14-a}=1$
$\frac{x\left ( 14-a \right )+ay}{a\left ( 14-a \right )} =1$
$14x-ax+ay=14a-a^{2}$ ........................(1)
If equation 1 passes through (3,4) then $14 * 3 - a*3+a*4=14a-a^{2}$
42-3a+4a-14a+a²=0
a²-13a+42=0
a²-7a-6a+42=0
(a-6)(a-7)=0
a=6 or a=7
If a=6 then 6+b=14 b=8
If a=7 the 7+b=14 b=7
If a=6 and b=8 then equation of line is $\frac{x}{6}+\frac{y}{8}=1$
4x+3y=24
If a=7 and b=7 then equation of line $\frac{x}{7}+\frac{x}{7}=1$
x+y=7

Question:5

Find the points on the line x + y = 4 which lie at a unit distance from the line 4x + 3y = 10.

Answer:

Let x₁, y₁be any point lying inthe equation x+y=4
x₁+y₁=4…......(1)
Distance of point x₁,y₁from the equation 4x+3y=10
$d=\frac{\left | Ax+By+C \right |}{\sqrt{A^{2}+B^{2}}}$
$1=\frac{4x_{1}+3y_{1}-10}{\sqrt{\left ( 4 \right )^{2}+\left ( 3 \right )^{2}}}=\left | \frac{4x_{1}+3y_{1}-10}{5} \right |$
4x₁+3y₁-10=±5
4x₁+3y₁-10=5 or 4x₁+3y₁-10=-5
4x₁+3y₁=(15)...….(2) or 4x₁+3y₁=5….....(3)
From equation 1 we have y₁=4-x₁….. (4)
Putting the values of y₁in equation 2 we get 4x₁+3(4-x₁)=15
4x₁+12-3x₁=15
x₁=15-12 =3
Putting the value of x₁in equation 4 we get y₁=1
Now, 4x1+34-x1=5
4x₁+12-3x₁=5
x₁=5-12 x₁=-7
Putting the value in equation 4 we get y₁=4--7=4+7=11
Hence, the required points on the given line are (3,1) and (-7,11)

Question:6

Show that the tangent of an angle between the lines $\frac{x}{a}+\frac{y}{b}=1$ and $\frac{x}{a}-\frac{y}{b}=1$ is $\frac{2ab}{a^{2}-b^{2}}$

Answer:

Equation of line in intercept form $\frac{x}{a}+\frac{y}{b}=1$ ............(i) and $\frac{x}{a}-\frac{y}{b}=1$ ..............(ii)
$\frac{x}{a}+\frac{y}{b}=1$
$\frac{y}{b}=1-\frac{x}{a}$
$y=b-\frac{b}{a}x$
$y=\left (-\frac{b}{a} \right )x+b$
y=mx+b Slope of equation 1 is $m_{1}=-\frac{b}{a}$
Similarly for equation 2 , $-\frac{y}{b}=1-\frac{x}{a}$
$-y=b-\frac{b}{a}x$
$y=\left (\frac{b}{a} \right )x-b$
$y=\left (\frac{b}{a} \right )x+\left (-1 \right )b$
Since, the above equation is in y=mx+b form
Slope of the equation 2 is $m_{2}=\frac{b}{a}$
Let θ be the angle between the given two lines $\tan \theta =\left | \frac{\left ( m_{1}-m_{2} \right )}{1+m_{1}m_{2}} \right |$
Putting the values of m₁and m₂in above equation we get
$\tan \theta =\left | \frac{-\frac{b}{a} -\frac{b}{a}}{1+\left ( -\frac{b}{a} \right )\left ( \frac{b}{a} \right )} \right |=\left | \frac{-2\left (\frac{b}{a} \right )}{1-\left (\frac{b^{2}}{a^{2}} \right )} \right |$
$=\left | -\frac{2ab}{a^{2}-b^{2}} \right |=\frac{2ab}{a^{2}-b^{2}}$

Question:7

Find the equation of lines passing through (1, 2) and making angle 30° with y-axis.

Answer:

Given that line passes through (1,2) making an angle of 30⁰with y axis.
Angle made by line with x axis is 60⁰ Slope of the line, m= $\sqrt{3}$
Equation of line passing through x₁,y₁and having slope 'm' is y-y₁=m(x-x₁₎
Here, (x₁, y₁)=(1,2) and m= $\sqrt{3}$
$y-2= \sqrt{3}\left ( x-1 \right )$
$y-2= \sqrt{3}x- \sqrt{3}$
$\sqrt{3}x-y-\sqrt{3}+2=0$

Question:8

Find the equation of the line passing through the point of intersection of 2x + y = 5 and x + 3y + 8 = 0 and parallel to the line 3x + 4y = 7.

Answer:

. Given 2x+y=5…. (1) and x+3y=-8….(2)
Firstly, we find the point of intersection of both equations we get $y=-\frac{21}{5}$ and $x=\frac{23}{5}$
Hence, the point of intersection is $\left (\frac{23}{5},-\frac{21}{5} \right )$
Now the slope of the equation 3x+4y=7 m= $-\frac{3}{4}$
Then the equation of the line passing through the point $\left (\frac{23}{5},-\frac{21}{5} \right )$ having slope $-\frac{3}{4}$ is

y-y₁=m(x-x₁)
$y-\left ( -\frac{21}{5} \right )=-\frac{3}{4}\left ( x- \frac{23}{5} \right )$
$y+\frac{21}{5} =-\frac{3}{4} x+\frac{69}{20}$
$\frac{3}{4}x+y =\frac{69}{20} -\frac{21}{5}$
$\frac{3x+4y}{4} = -\frac{15}{5}$
$3x+4y+3=0$

Question:9

For what values of a and b the intercepts cut off on the coordinate axes by the line ax + by + 8 = 0 are equal in length but opposite in signs to those cut off by the line 2x – 3y + 6 = 0 on the axes.

Answer:

Given equation is ax+by+8=0 or ax+by= -8
Now dividing by-8 to both sides $\frac{a}{-8}x+\frac{b}{-8}y = 1$ $\frac{x}{\left (-\frac{8}{a} \right )}+\frac{y}{\left (-\frac{8}{b} \right )} = 1$
So the intercepts are $-\frac{8}{a}$ and $-\frac{8}{b}$
Now, the second equation which is given is 2x-3y+6=0 or 2x-3y=-6
Dividing by-6 on both sides $-\frac{2}{-6}x-\frac{3}{-6}y=1$
$\frac{x}{-3}+\frac{y}{2}=1$
So, the intercepts are-3 and 2
Now, according to the question $-\frac{8}{a}=3$ and $-\frac{8}{b}=-2$
$a=-\frac{8}{3}$ and $b=4$

Question:10

If the intercept of a line between the coordinate axes is divided by the point (–5, 4) in the ratio 1:2, then find the equation of the line.

Answer:

. Let a and b be the intercepts on the given line
Coordinates of A and B are (a,0)and (0,b) respectiively.
Using the section formula we find the value of a and b
$\left ( x,y \right )=\left ( \frac{m_{1}x_{2}+m_{2}x_{1}}{m_{1}+m_{2}}, \frac{m_{1}y_{2}+m_{2}y_{1}}{m_{1}+m_{2}}\right )$
$\left ( -5,4\right )=\left ( \frac{1*0+2*a}{1+2}, \frac{1*b+2*0}{1+2}\right )=\left (\frac{2a}{3},\frac{b}{3} \right )$
$-5=\frac{2a}{3}$ and $4=\frac{b}{3}$
$-15=2a$ and $b=12$
$a=-\frac{15}{2}$ and $b=12$
Coordinates of A and B are $\left ( -\frac{15}{2},0 \right )$ and $\left ( 0 , 12 \right )$
a10
Equation of line AB $y-0 = \frac{12-0}{0-\left ( -\frac{15}{2} \right )}\left ( x- \left ( -\frac{15}{2} \right ) \right )$
$y = \frac{12}{\frac{15}{2}}\left ( x+\frac{15}{2} \right )$
$y =\frac{24}{15}\left ( x+\frac{15}{2} \right )$
$y =\frac{8}{5}x+15$
$5y=8x+60$

Hence, trequired equation is 8x-5y+60=0

Question:11

Find the equation of a straight line on which length of perpendicular from the origin is four units and the line makes an angle of 120° with the positive direction of x-axis.

Answer:

a11
Given that length of the perpendicular from the origin is 4 units and line makes an angle with positive direction of x-axis
∠BAX=120
∠BA0=180-120=60
∠MAO=60
Now in triangle AMO ∠MAO+∠AOM+∠OMA=180⁰
60⁰+θ+ 90⁰=180⁰
θ=30⁰ ∠AOM= 30⁰
x cosθ+ysinθ=p
xcos 30⁰+ysin30⁰=4
$x\left ( \frac{\sqrt{3}}{2} \right )+y\left ( \frac{\sqrt{1}}{2} \right )=4$
$\sqrt{3}x+y=8$

Question:12

Find the equation of one of the sides of an isosceles right angled triangle whose hypotenuse is given by 3x + 4y = 4 and the opposite vertex of the hypotenuse is (2, 2).

Answer:

Equation of hypotenuse is 3x+4y=4 and opposite vertex is (2,2)
Slope of the equation of hypotenuse is $-\frac{3}{4}$
Now let the slope of AC be m $\tan \theta =\left | \frac{\left ( m_{1}-m_{2} \right )}{1+m_{1}m_{2}} \right |$
Putting the values of m₁and m₂in the equation $\tan 45^{0} =\left | \frac{\left ( m-\left ( -\frac{3}{4} \right ) \right )}{1-\frac{3}{4}m} \right |$
$1=\left | \frac{m+\frac{3}{4}}{1-\frac{3}{4}m} \right |=\left | \frac{4m+3}{4-3m} \right |$
$1=\pm \left | \frac{4m+3}{4-3m} \right |$
$\frac{4m+3}{4-3m} =1$
4m+3=4-3m
4m+3m=4-3
7m=1 m= $\frac{1}{7}$
OR $-\frac{4m+3}{4-3m}=1$
$4m+3=-\left ( 4-3m \right )$
4m+3=-4+3m
4m-3m=-4-3
m= -7
If m=1/7
equation of AC is y-y₁=m(x-x₁)
y-2=1/7(x-2)
7y-14=x-2
x-7y-2+14=0
x-7y+12=0
If m= -7 then equation of AC is y-2=-(7)(x-2)
y-2=-7x+14
7x+y=16
The required equations are x-7y+12=0 and 7x+y=16

Question:13

If the equation of the base of an equilateral triangle is x + y = 2 and the vertex is (2, – 1), then find the length of the side of the triangle.

Answer:

Let ABC be an equilateral triangle, BC is base, and altitude from A on BC meets at mid-point D.
Given:Equation of the base BC is x+y=2
$\sin 60^{0}=\frac{AD}{AB}$
$\frac{\sqrt{3}}{2}=\frac{AD}{AB}$
$AD=\frac{\sqrt{3}}{2}AB$
Distance of point (x₁,y₁) from the equation Ax+By+C=0
$d=\frac{\left | Ax+By+C \right |}{\sqrt{A^{2}+B^{2}}}$
Now, length of perpendicular from vertex A(2,-1) to the line x+y=2
$AD=\frac{\left | 1*2+1\left ( -1 \right ) -1 \right |}{\sqrt{\left ( 1 \right )^{2} +\left ( 1 \right )^{2}}}$
$\frac{\sqrt{3}}{2}AB=\left | \frac{2-1-2}{\sqrt{2}} \right |=\frac{1}{\sqrt{2}}$
Squaring both the sides, we get $\frac{3}{4}AB^{2}=\frac{1}{2}$
$AB^{2}=\frac{4}{3}*\frac{1}{2}=\frac{2}{3}$
$AB=\sqrt{\frac{2}{3}}$

Question:14

A variable line passes through a fixed point P. The algebraic sum of the perpendiculars drawn from the points (2, 0), (0, 2) and (1, 1) on the line is zero. Find the coordinates of the point P.

Answer:

Let the variable line be ax+by=1
Length of perpendicular from (2,0) to the line ax+by-1=0
$d=\frac{\left | 2*a+0*b-1 \right |}{\sqrt{a^{2}+b^{2}}}=\frac{2a-1}{\sqrt{a^{2}+b^{2}}}$
Now perpendicular distance from B(0,2) = $\left | \frac{0*a+2*b-1}{\sqrt{a^{2}+b^{2}}} \right |$
Now, perpendicular distance from C(1,1)= $\left | \frac{1*a+1*b-1}{\sqrt{a^{2}+b^{2}}} \right |$
The algebraic sum of the perpendicular from the given points (2,0), (0,2)
and (1,1) to this line is zero.
d₁+d₂+d₃=0
$\frac{2a-1}{\sqrt{a^{2}+b^{2}}}+\frac{2b-1}{\sqrt{a^{2}+b^{2}}}+\frac{a+b-1}{\sqrt{a^{2}+b^{2}}}=0$
2a-1+2b-1+a+b-1=0
3a+3b-3=0
a+b-1=0
a+b=1
So, the equation ax+by=1 represents a family of straight lines passing
through a fixed point .
Comparing equation ax+by=1 and a+b=1, we get x=1 and y=1.
Coordinates of fixed point is (1,1)

Question:15

In what direction should a line be drawn through the point (1, 2) so that its point of intersection with the line x + y = 4 is at a distance √6/3 from the given point.

Answer:

Let the given line x+y=4 and the required line'l'intersect at B (a,b)
Slope of line'l'is $m=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{b-2}{a-1}$
$m=\tan \theta =\frac{b-2}{a-1}$
Given that $AB=\frac{\sqrt{6}}{3}$
So, by distance formula for point A(1,2) and B(a,b) we get d
$=\sqrt{\left ( x_{2}-x_{1} \right )^{2}+\left ( y_{2}-y_{1} \right )^{2}}$
$\frac{\sqrt{6}}{3}=\sqrt{\left ( a-1 \right )^{2}+\left (b-2 \right )^{2}}$
$\frac{6}{9}=\left ( a-1 \right )^{2}+\left (b-2 \right )^{2}$
$\frac{2}{3}=a^{2}+1-2a+b^{2}+4-4b$
$2=3a^{2}+3-6a+3b^{2}+12-12b$
$3a^{2}+3b^{2}+3-6a-12b+13=0..........(i))$
Point B(a,b)also satisfies x+y=4
a+b=4; b=4-a
Putting the value of b in equation (i )we get 3a²+3(4-a)²-6a-12(4-a)+13=0
3a²+48+3a²-24a-6a-48+12a+13=0
6a²-18a+13=0
Using the formula $x=\frac{-b\pm \sqrt{b^{2}-4ac}}{2a}$
$a=\frac{-\left ( -18 \right )\pm \sqrt{\left ( -18 \right )^{2}-4*6*13}}{2*6}=\frac{18\pm \sqrt{324-312}}{12}$
$=\frac{18\pm \sqrt{12}}{12}=\frac{9\pm \sqrt{3}}{6}$
Putting the value of a in the equation we get
$b=4-\frac{\left (9\pm \sqrt{3} \right )}{6} =\frac{15\pm \sqrt{3}}{6}$

Now putting the value of a and b in equation $\tan \theta =\frac{b-2}{a-1}$
$=\frac{\frac{\left (15 \pm \sqrt{3}\right )}{6}-2} {\frac{9\pm \sqrt{3} }{6}-1}=\frac{3 \pm \sqrt{3}}{3\pm \sqrt{3}}$
$\tan \theta =\frac{\sqrt{3}+1}{\sqrt{3}-1} or \frac{\sqrt{3}-1}{\sqrt{3}+1}$
$\theta =\tan ^{-1}\left (\frac{\sqrt{3}-1}{\sqrt{3}+1} \right )$
$\theta =\tan ^{-1}\left (\sqrt{3} \right )-\tan ^{-1}\left (1 \right )$
θ= 60⁰-45⁰=15⁰
Similarly, taking $\theta =\tan ^{-1}\left (\frac{\sqrt{3}+1}{\sqrt{3}-1} \right )$
$\theta =\tan ^{-1}\left (\sqrt{3} \right )+\tan ^{-1}\left (1 \right )$
θ= 60⁰+45⁰=105⁰

Question:16

A straight line moves so that the sum of the reciprocals of its intercepts made on axes is constant. Show that the line passes through a fixed point.

Answer:

Equation of line in intercept form=xa+yb=1
where a and b are intercepts on the axes
Given that $\frac{1}{a}+\frac{1}{b}=\frac{1}{k}$
Then, $\frac{k}{a}+\frac{k}{b}=1$
This shows that the line is passing through the fixed point that is (k,k)

Question:17

Find the equation of the line which passes through the point minus 4, 3 and the portion of the line intercepted between the axes is divided internally in the ratio 5 ratio 3 by this point?

Answer:

Let the line cut the x axis at (a,0) and Y axis at (0,b) Therefore
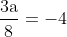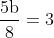
It is given that the point (-4,3) divides the line internally in 5: 3 ratio. Hence applying the formula for internal division of line segment, we get $\frac{3 \mathrm{a}}{8}=-4$ and $\frac{5 \mathrm{b}}{8}=3$

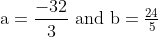Hence $\mathrm{a}=\frac{-32}{3}$ \text{and} $\mathrm{b}=\frac{24}{5}$ Using slope intercept form we get
$\frac{x}{\frac{-32}{3}}+\frac{y}{\frac{24}{5}}=1\\ \\ 9 x-20 y+96=0$

Question:18

Find the equations of the lines through the point of intersection of the lines x – y + 1 = 0 and 2x – 3y + 5 = 0 and whose distance from the point (3, 2) is 7/5.

Answer:

Given two lines are: x-y+1=0 and 2x-3y+5=0
Solving these two equations gives us points of intersection we get y=3 and x=2
(x,y)=(2,3)
Let m be the slope of the required line
Then, equation of the line is y-3=mx-2
y-3=mx-2m
mx-y-2m+3=0….(1)
Since, the perpendicular distance from the point 3,2to the line is75then
$d=\frac{\left | m*\left ( 3 \right ) -2 +3-2m \right |}{\sqrt{m^{2}+1^{2}}}$
$\frac{7}{5}=\frac{\left | 3m+1-2m \right |}{\sqrt{m^{2}+1^{1}}}=\frac{m+1}{\sqrt{m^{2}+1^{2}}}$
Squaring both the sides, we get $\frac{49}{25}=\frac{\left ( m+1 \right )^{2}}{m^{2}+1}$
49m²+1=25(m+1)²
49m²+49=25m²+25+50m
25m²+25+50m-49m²-49=0
-24m²+50m-24=0
-12m²+25m-12=0
Factorising, we get (3m-4)(4m-3)=0
3m-4=0 or 4m-3=0
3m=4 or 4m=3
m=4/3 or 3/4
Putting the value of m=4/3 in equation 1 we get 4x/3-y-2(4/3)+3=0
4x/3-y-8/3+3=0
4x/3-y=-1/3
4x-3y+1=0
Putting the value of m=3/4 in the equation we get 3x/4-y-2(3/4)+3=0
3x/4-y-3/2+3=0
3x/4-y+3/2=0
3x-4y+6=0
Hence, the required equation are 4x-3y+1=0 and 3x-4y+6=0

Question:19

If the sum of the distances of a moving point in a plane from the axes is 1, then find the locus of the point

Answer:

a-19
Let the coordinates of a moving point P be (a,b)
It is given that the sum of the distance from the axes to the point is always 1 |x|+|y|=1
±x±y=1 -x-y=1, x+y=1, -x+y=1 and x-y=1
Hence, these equations gives us the locus of the point P which is a square.

Question:20

P₁, P₂ are points on either of the two lines $y-\sqrt{3}\left | x \right |=2$ at a distance of 5 units from their point of intersection. Find the coordinates of the foot of perpendiculars drawn from P₁, P₂ on the bisector of the angle between the given lines.

Answer:

Given lines are $y-\sqrt{3}\left | x \right |=2$
If x≥0, then $y-\sqrt{3} x =2$ ..............(i)
If x<0 then $y+\sqrt{3}x=2$ .............(ii)
On adding both the equations we get $y-\sqrt{3}x +y+\sqrt{3}x=2+2$
2y=4
y=2
Putting the value of y=2 in equation (ii), we get $2+\sqrt{3}x=2$
$\sqrt{3}x=2-2=0$
Point of intersection of given lines is (0,2 )
Now we find the slopes of given lines Slope of equation (i) is $y+\sqrt{3}x=2$
Comparing the above equation with y=mx+b, we get $m=\sqrt{3}$
and we know that $m=\tan \theta =\sqrt{3}$
θ= 60⁰
Slope of equation (ii) is $y=-\sqrt{3}x+2$ , we get $m=-\sqrt{3}$
$\tan \theta =-\sqrt{3}$
θ=180⁰- 60⁰= 120⁰
a-20
In ACB,
$\cos 30^{\circ}=\frac{BA}{AC}$
$\frac{\sqrt{3}}{2}=\frac{BA}{5}$
$BA=\frac{5\sqrt{3}}{2}$
$OB=OA+AB=2+\frac{5\sqrt{3}}{2}$
Hence, the coordinates of the foot of perpendicular $=\left (0,2+\frac{5\sqrt{3}}{2} \right )$

Question:21

If p is the length of the perpendicular from the origin on the line $\frac{x}{a}+\frac{y}{b}=1$ and a², p², b² are in A.P, then show that a⁴ + b⁴ = 0.

Answer:

Equation of line in intercept form= $\frac{x}{a}+\frac{y}{b}=1$
Since, p is the length of perpendicular drawn from the origin to the gievn line p= $\left | \frac{\frac{0}{a}+\frac{0}{b}-1}{\sqrt{\frac{1}{a^{2}}+\frac{1}{b^{2}}}} \right |$
Squaring both the sides, we have $p= \left | \frac{1}{\sqrt{\frac{1}{a^{2}}+\frac{1}{b^{2}}}} \right |$
$\frac{1}{p^{2}}=\frac{1}{a^{2}}+\frac{1}{b^{2}}........(i)$
Since, a^2,b²and p²are in AP
2p²=a²+b²
$p^{2}=\frac{\left ( a^{2}+b^{2} \right )}{2}$
$\frac{1}{p^{2}}=\frac{2}{a^{2}+b^{2}}..........(ii)$
From equation (i) and (ii) we get $\frac{1}{a^{2}}+\frac{1}{b^{2}}=\frac{2}{a^{2}+b^{2}}$
$\frac{b^{2}+a^{2}}{a^{2}b^{2}}=\frac{2}{a^{2}+b^{2}}$
$\left ( a^{2}+b^{2} \right )\left ( a^{2}+b^{2} \right )=2\left ( a^{2}b^{2} \right )$
$a^{4}+b^{4}=0$
Hence, proved.

Question:22

A line cutting off intercept – 3 from the y-axis and the tangent at angle to the x-axis is 3/5, its equation is
A. 5y – 3x + 15 = 0
B. 3y – 5x + 15 = 0
C. 5y – 3x – 15 = 0
D. None of these

Answer:

. Given that tanθ=3/5
We know that slope of a line, m=tanθ Slope of line, m=3/5
Since, the lines cut off intercepts -3 on y axis so the line is passing
through the point (0,-3)
So, the equation of line is y-y₁=m(x-x₁)
y-(-3)=3/5(x-0)
y+3=3x/5
5y+15=3x
5y-3x+15=0
Hence, the correct option is (a)

Question:23

Slope of a line which cuts off intercepts of equal lengths on the axes is
A. – 1
B. – 0
C. 2
D. $\sqrt{3}$

Answer:

Equation of line in intercept form= $\frac{x}{a}+\frac{y}{b}=1$
Given that a=b ; $\frac{x}{a}+\frac{y}{a}=1$
$\frac{x+y}{a} =1$
x+y=a……(1)
y=-x+a
y=(-1)x+a
Since, the above equation is in y=mx+b form
So, the slope of the line is (-1) .

Question:24

The equation of the straight line passing through the point (3, 2) and perpendicular to the line y = x is
A. x – y = 5
B. x + y = 5
C. x + y = 1
D. x – y = 1

Answer:

Given that straight line passing through the point (3,2) and is perpendicular to
line y=x
Let the equation of line L is (y-y₁) =m(x-x₁)
Since, L is passing through the point (3,2)
y-2=m(x-3)..…(i)
Now, given equation is y=x
Comparing it with y=mx+b
we get slope of the equation as 1.
It is also given that line L and y=x are perpendicular to each other.
We know that when two lies are perpendicular then m₁*m₂= -1 m*1=-1
m=-1
Putting this value of m in equation (i) we get y-2=-(1)(x-3)
y-2=-x+3
x+y=5
Hence, the correct option is b

Question:25

The equation of the line passing through the point (1, 2) and perpendicular to the line x + y + 1 = 0 is
A. y – x + 1 = 0
B. y – x – 1 = 0
C. y – x + 2 = 0
D. y – x – 2 = 0

Answer:

Given that line passing through the point (1,2) and perpendicular to the line
x+y+1=0
Let the equation of line L is x-y+k=0…..(i)
Since, L is passing through the point (1,2)
1-2+k=0
k=1
Putting this value of k in equation )i) we get x-y+1=0 or y-x-1=0
Hence, the correct option is b

Question:26

The tangent of angle between the lines whose intercepts on the axes are a, – b and b, – a, respectively, is
A. $\frac{a^{2}-b^{2}}{ab}$
B. $\frac{b^{2}-a^{2}}{2}$
C. $\frac{b^{2}-a^{2}}{2ab}$
D. None of these

Answer:

First equation of line in intercept form=
$\frac{x}{a}+\frac{y}{-b}=1$
$\frac{x}{a}-\frac{y}{b}=1$
bx-ay=ab……. (i)
Let the second equation of line having intercepts on the axes b, -a is $\frac{x}{b}+\frac{y}{-a}=1$
$\frac{x}{b}-\frac{y}{a}=1$
ax-by=ab…..(ii)
Now we find the slope in the first equation bx-ay=ab
ay=bx-ab
$y=\frac{b}{a}x-b$
Slope of the equation $m_{1}=\frac{b}{a}$
Now, we find the slope of equation (ii) ax-by=ab
by=ax-ab
$y=\frac{a}{b}x-a$
Slope of the equation $m_{2}=\frac{a}{b}$
Let θ be the angle between the given two lines $\tan \theta =\left |\frac{\left ( m_{1}-m_{2} \right )}{1+m_{1}m_{2} } \right |$
Putting the values of m1 and m2 in above equation, we get $\tan \theta =\left |\frac{\left ( \frac{b}{a}-\frac{a}{b} \right )}{1+\left ( \frac{b}{a} \right )\left (\frac{a}{b} \right )} \right |$
$\tan \theta =\left | \frac{\frac{b^{2}-a^{2}}{ab}}{1+1} \right |=\left | \frac{\left (b^{2}-a^{2} \right )}{2ab} \right |=\left | \frac{b^{2}-a^{2} }{2ab} \right |$ is the required angle.
Hence, the correct option is (c)

Question:27

If the line $\frac{x}{a}+\frac{y}{b}=1$ passes through the points (2, –3) and (4, –5), then (a, b) is
A. (1, 1)
B. (– 1, 1)
C. (1, – 1)
D. (– 1, –1)

Answer:

Given points are (2, -3) and (4, -5)
Firstly the equation of line is found
We know that the equation of line when two points are given is y-y₁= $\frac{y_{2}-y_{1}}{x_{2}-x_{1}}\left ( x-x_{1} \right )$
Putting the values we get $y-\left ( -3 \right )=\frac{-5-\left ( -3 \right )}{4-2}\left ( x-2 \right )$
$y+3=\frac{-5+3 }{2}\left ( x-2 \right )$
$y+3=-\frac{2 }{2}\left ( x-2 \right )$
y+3= -1( x-2)
y+3= -x+2
x+y=2-3
x+y=-1
$\frac{x}{-1}+\frac{y}{-1}=1$ in intercept form
Comparing the equaton with intercept form of equation that is $\frac{x}{a}+\frac{y}{b}=1$
the value of a=-1 and b=-1
Hence, the correct option is (d)

Question:28

The distance of the point of intersection of the lines 2x – 3y + 5 = 0 and 3x + 4y = 0 from the line 5x – 2y = 0 is
A. $\frac{130}{17\sqrt{29}}$
B. $\frac{13}{7\sqrt{29}}$
C. $\frac{130}{7}$
D. None of these

Answer:

(a) Given lines are:
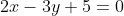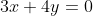
2 x-3 y+5=0 and 3 x+4 y=0

Solving these lines, we get point of intersection as $\left(\frac{-20}{17}, \frac{15}{17}\right)$
therefore Distance of this point from the line 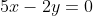5 x-2 y=0

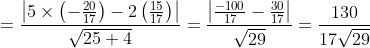
$=\frac{\left|5 \times\left(-\frac{20}{17}\right)-2\left(\frac{15}{17}\right)\right|}{\sqrt{25+4}}=\frac{\left|\frac{-100}{17}-\frac{30}{17}\right|}{\sqrt{29}}=\frac{130}{17 \sqrt{29}}$

Question:29

The equations of the lines which pass through the point (3, –2) and are inclined at 60° to the line $\sqrt{3}x+y=1$ is
A. y + 2 = 0, $\sqrt{3}x-y-2-3\sqrt{3}=0$
B. x – 2 = 0, $\sqrt{3}x-y+2+3\sqrt{3}=0$
C. $\sqrt{3}x-y-2-3\sqrt{3}=0$
D. None of these

Answer:

Given equation is $\sqrt{3}x+y=1$ and θ= 60⁰
Slope of the equation $\sqrt{3}x+y=1$
$y=1-\sqrt{3}x$
Slope of the equation $m_{1}=-\sqrt{3}$
Let slope of the required line be m₂
Then $\tan \theta = \left | \frac{\left ( m_{1}-m_{2} \right )}{1+m_{1}m_{2}} \right |$
Putting the values in the above equation we gettan $60^{\circ}=\left | \frac{-\sqrt{3}-m_{2}}{1+\left ( -\sqrt{3} \right )*m_{2}} \right |$
$\sqrt{3}=\left | \frac{-\sqrt{3}-m_{2}}{1+\left ( -\sqrt{3} \right )*m_{2}} \right |$
$\sqrt{3}= \pm\left ( \frac{-\sqrt{3}-m_{2}}{1+\left ( -\sqrt{3} \right )*m_{2}} \right )$
Taking+sign we get $-\sqrt{3}-m_{2}=\sqrt{3}\left ( 1-\sqrt{3}m_{2} \right )$
$-\sqrt{3}-m_{2}=\sqrt{3}-3m_{2}$
$3m_{2}-m_{2}=\sqrt{3}+\sqrt{3}$
$2m_{2}=2\sqrt{3}$
$m_{2}=\sqrt{3}$
Taking -ve sign we get $\sqrt{3}+m_{2}=\sqrt{3}\left ( 1-\sqrt{3}m_{2} \right )$
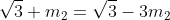
$\sqrt{3}+m_{2}=\sqrt{3}-3m_{2}$

$3m_{2}+m_{2} =0$
$4m_{2} =0$
m₂=0
Equation of line passing through (3,-2) with slope $\sqrt{3}$ is y-y₁=m(x-x₁)
$y-\left ( -2 \right )=\sqrt{3}\left ( x-3 \right )$
$y+2=\sqrt{3}x-3\sqrt{3}$
$\sqrt{3}x-y-3\sqrt{3}-2=0$
and equation of line passing through (3, -2) with slope 0 is y-y₁=m(x-x₁)
y-(-2)=0(x-3)
y+2=0
Hence, the required equations are $\sqrt{3}x-y-\left ( 3\sqrt{3}+2 \right )=0$ and y+2=0
Hence, the correct option is (a)

Question:30

The equations of the lines passing through the point (1, 0) and at a distance $\frac{\sqrt{3}}{2}$ from the origin, are
A. $\sqrt{3}x+y-\sqrt{3}=0,\sqrt{3}x-y-\sqrt{3}=0$
B. $\sqrt{3}x+y+\sqrt{3}=0,\sqrt{3}x-y+\sqrt{3}=0$
C. $x+\sqrt{3}y-\sqrt{3}=0,x-\sqrt{3}y-\sqrt{3}=0$
D. None of these.

Answer:

Let the equation of any line passing through the point (1,0) is y-y₁=m(x-x₁)
y-0=m(x-1)
y=mx-m
mx-m-y=0….........(i)
Distance from the origin of the line is $\frac{\sqrt{3}}{2}$ Distance of point (x₁,y₁) from the equation Ax+By+C=0
$d=\frac{\left | Ax+By+C \right |}{\sqrt{A^{2}+B^{2}}}$
$\frac{\sqrt{3}}{2}=\frac{\left | m*0+\left ( -1 \right )*0+\left ( -m \right ) \right |}{\sqrt{m^{2}+\left ( -1 \right )^{2}}}=\left |- \frac{m}{\sqrt{m^{2}+1}} \right |$
Squaring both the sides we get $\frac{3}{4}=\frac{m^{2}}{m^{2}+1}$
3(m²+1)=4m²
3m²+3=4m²
4m²-3m²=3
m²=3
$m = \pm \sqrt{3}$
Putting the value of m= $\sqrt{3}$ in equation (i) we get $\sqrt{3}$ x-y- $\sqrt{3}$ =0
Now, putting the value of m= - $\sqrt{3}$ in the same equation we get- $\sqrt{3}$ x-y+ $\sqrt{3}$ =0
Hence, the correct option is (a)

Question:31

The distance between the lines y = mx + c₁ and y = mx + c₂ is
A. $\frac{c_{1}-c_{2}}{\sqrt{m^{2}+1}}$

B. $\frac{\left |c_{1}-c_{2} \right |}{\sqrt{1+m^{2}}}$

C. $\frac{c_{2}-c_{1} }{\sqrt{1+m^{2}}}$

D. 0

Answer:

Given equations are y=mx+c₁….(i) and y=mx+c₂…….(ii)
Firstly, we find the slope of both the equations
Since, both of them have the same slope they are parallel lines.
We know that distance between two parallel lines Ax+By+C₁=0 and Ax+By+C₂=0
$d=\frac{\left | c_{1}-c_{2} \right |}{\sqrt{A^{2}+B^{2}}}=\frac{\left | c_{1}-c_{2} \right |}{\sqrt{m^{2}+\left ( -1 \right )^{2}}}=\frac{\left | c_{1}-c_{2} \right |}{\sqrt{m^{2}+1}}$
Hence, the correct option is b.

Question:32

The coordinates of the foot of perpendiculars from the point (2, 3) on the line y = 3x + 4 is given by
A. $\frac{37}{10},\frac{-1}{10}$
B. $\frac{-1}{10},\frac{37}{10}$
C. $\frac{10}{37},-10$
D. $\frac{2}{3},-\frac{1}{3}$

Answer:

Given equations are y=3x+4….(i)
Comparing this equation with y=mx+b form , the slope of the equation is 3.
Let the equation of any line passing through the point (2,3) is y-y₁=m(x-x₁)
y-3=m(x-2)……(ii)
Given that equation (i) is perpendicular to equation (ii)
And we know that, if two lines are perpendicular then m₁m₂= -1
3*m₂=-1
m₂=-1/3 which is the slope of the required line
Putting the value of slope in equation ii we get y-3=-1/3(x-2)
3y-9=-x+2
x+3y-9-2=0
x+3y-11=0……(iii)
Now we have to find the coordinates of foot of the perpendicular
Solving equation (i) and (iii) we get x+3(3x+4)-11=0
x+9x+12-11=0
10x+1=0
x=-1/10
Putting the value of x in equation i , we get y=3(-1/10)+4
y= -3/10+4
y=37/10
So the required coordinates are (-1/10,37/10)
Hence, the correct option is (b)

Question:33

If the coordinates of the middle point of the portion of a line intercepted between the coordinate axes is (3, 2), then the equation of the line will be
A. 2x + 3y = 12
B. 3x + 2y = 12
C. 4x – 3y = 6
D. 5x – 2y = 10
Answer:

Let the given line meets the axes at A(a,0) and B(0,b)
Given that (3,2) is the midpoint $3=\frac{0+a}{2}$
a=6 and $2=\frac{0+b}{2}$
b=4
Intercept form of the line AB is $\frac{x}{a}+\frac{y}{b}=1$
Putting the value of a and b in above equation, we get $\frac{x}{6}+\frac{y}{4}=1$
$\frac{2x+3y}{12}=1$
2x+3y=12
Hence, the correct option is (a)

Question:34

Equation of the line passing through (1, 2) and parallel to the line y = 3x – 1 is
A. y + 2 = x + 1
B. y + 2 = 3 (x + 1)
C. y – 2 = 3 (x – 1)
D. y – 2 = x – 1

Answer:

Given equation of the line is y=3x-1
Now we find the slope of the above equation by comparing it with y=mx+b form
So m=3
Now, we find the equation of line passing through the point (1,2) and parallel to the given line
with slope=3
y-y₁=m(x-x₁)
y-2=3(x-1)
Hence, the correct option is (c)

Question:35

Equations of diagonals of the square formed by the lines x = 0, y = 0, x = 1 and y = 1 are
A. y = x, y + x = 1
B. y = x, x + y = 2
C. 2y = x, y + x = 1/3
D. y = 2x, y + 2x = 1

Answer:

It is given that the lines x=0, y=0 , x=1 and y=1 form a square of side 1 unit
Let us form a square OABC having corners O(0,0) from the given lines
with A(1,0), B(1,1) and C(0,1)
lkjhfdfglk
Now we have to find the equation of the diagonal AC
Equation of a line is found out by $y-y_{1}=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}\left ( x-x_{1} \right )$
$y-0=\frac{1-0}{0-1}\left ( x-1 \right )$
y=-1(x-1)
y=-x+1
x+y=1
Equation of diagonal OB is $y-0=\frac{1-0}{1-0}\left ( x-0 \right )$
y=x
y=x
Hence, the correct option is (a)

Question: 36

For specifying a straight line, how many geometrical parameters should be known?
A. 1
B. 2
C. 4
D. 3

Answer:

Equation of straight line in intercept form= $\frac{x}{a}+\frac{y}{b}=1$
where a and b are the intercepts on the axis.
In intercept form we need 2 parameters a and b to specify a straight line
Slope-Intercept Form y=mx+c where m=tanθ
and θ is the angle made with positive x axis and c is the intercept on y axis
So, we need two parameters 'm' and 'c' to specify a straight line
Hence, the correct option is b

Question:37

The point (4, 1) undergoes the following two successive transformations:
(i) Reflection about the line y = x
(ii) Translation through a distance 2 units along the positive x-axis. Then the final coordinates of the point are
A. (4, 3)
B. (3, 4)
C. (1, 4)
D. $\frac{7}{2}, \frac{7}{2}$

Answer:

Let Q(x,y) be the reflection of P(4,1) about the line y=x, then
midpoint of PQ $\left ( \frac{4+x}{2},\frac{1+y}{2} \right )$
which lies on y=x $\frac{4+x}{2}=\frac{1+y}{2}$
4+x=1+y
x-y+3=0……(i)
Now, we find the slope of given equation y=x
Since this equation is in y=mx+b form
So the slope=m=1
Slope of $PQ=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{y-1}{x-4}$
Since, PQ is perpendicular to y=x
And when two lines rae perpendicular then m₁m₂=-1
$1*\left ( \frac{y-1}{x-4} \right )=-1$
y-1=-(x-4)
x+y-5=0…..(ii)
On adding equation i and equation ii we get x-y+3+x+y-5=0
2x-2=0
x-1=0
x=1
Putting the value of x=1 in equation i we get 1-y+3=0 -y+4=0 y=4
It is given that translation through a distance of 2 units along the positive x axis takes place
The point after translaton is (1+2,4)=(3 ,4)
Hence, the correct option is (b)

Question:38

A point equidistant from the lines 4x + 3y + 10 = 0, 5x – 12y + 26 = 0 and 7x + 24y – 50 = 0 is
A. (1, –1)
B. (1, 1)
C. (0, 0)
D. (0, 1)

Answer:

Given equations are 4x+3+10=0 …..(i)
5x-12y+26=0…......(ii)
and 7x+24y-50=0
Let p,qbe the point which is equidistant from the givenlines
Now, we find the distance of (p,q) from the given lines
Distance of point (x₁,y₁) from the equation Ax+By+C=0
$d=\frac{\left | Ax+By+C \right |}{\sqrt{A^{2}+B^{2}}}$
Distance of (p,q) from equation i is $d=\frac{\left | 4p+4q+10 \right |}{\sqrt{4^{2}+3^{2}}}=\frac{\left | 4p+4q+10 \right |}{5}$
Distance of (p,q) from equation ii is $d=\frac{\left | 5p-12q+26 \right |}{\sqrt{5^{2}+\left (-12 \right )^{2}}}=\frac{\left | 5p-12q+26 \right |}{13}$
Distance of (p,q) from equation iii is $d=\frac{\left | 7p-24q+50 \right |}{\sqrt{7^{2}+24^{2}}}=\frac{\left | 7p-24q+50\right |}{25}$
Given that (p,q) is equidistant from the given lines $\frac{\left | 4p-3q+10 \right |}{5}$ = $\frac{\left | 5p-12q+26 \right |}{13}=\frac{\left | 7p-24q+50\right |}{25}$
On putting the value of (p,q) as (0,0) we get $\left | \frac{10}{5} \right |=\left | \frac{26}{13} \right |=\left | -\frac{50}{25} \right |$
Hence, the correct option is c

Question:39

A line passes through (2, 2) and is perpendicular to the line 3x + y = 3. Its y-intercept is
A. 1/3
B. 2/3
C. 1
D. 4/3

Answer:

Given line is 3x+y=3 It can be re written as y=-3x+3
Comparing it with the y=mx+b form of equation we get the slope as m= -3
So slope of the perpendicular line will be1/3
The line passes through (2,2) and has a slope1/3 is $y-2=\frac{1}{3}\left ( x-2 \right )$
3y-6=x-2
3y=x+4
$y=\frac{1}{3}x + \frac{4}{3}$
So, the y intercept is $\frac{4}{3}$
Hence, the correct option is (d)

Question:40

The ratio in which the line 3x + 4y + 2 = 0 divides the distance between the lines 3x + 4y + 5 = 0 and 3x + 4y – 5 = 0 is
A. 1 : 2
B. 3 : 7
C. 2 : 3
D. 2 : 5

Answer:

Given lines are 3x+4y+5=0…....(i)
3x+4y-5=0…....(ii)
and 3x+4y+2=0….......(iii)
Since the coefficient of x and y are same equation i, ii and iii are parallel to each other
We know that in case of i and iii
distance between two parallel lines is $d=\frac{\left | c_{1}-c_{2} \right |}{\sqrt{A^{2}+B^{2}}}=\frac{\left | 5-2 \right |}{\sqrt{3^{2}+(4)^{2}}}=\frac{\left | 3 \right |}{\sqrt{9+16}}=\left | \frac{3}{5} \right |$
Similarly in case of ii and iii distance between two parallel lines is $d=\frac{\left | c_{1}-c_{2} \right |}{\sqrt{A^{2}+B^{2}}}=\frac{\left | -5-2 \right |}{\sqrt{3^{2}+(4)^{2}}}=\frac{\left | -7 \right |}{\sqrt{9+16}}=\left |- \frac{7}{5} \right |$
Ratio between the distance is $\frac{3}{5}:\frac{7}{5}=3:7$
Hence, the correct option is b

Question:41

One vertex of the equilateral triangle with centroid at the origin and one side as x + y – 2 = 0 is
A. (–1, –1)
B. (2, 2)
C. (–2, –2)
D. (2, –2)

Answer:

a-41
Let ABC be an equilateral triangle with vertex A (a,b)
Let AD be perpendicular to BC and let (p,q) be the coordinates of D
Given that the centroid P lies at the origin (0,0)
We know that, the centroid of a triangle divides the median in the ratio 1:2
Now, using the section formula, we get
$\left ( x,y \right )=\left ( \frac{m_{1}x_{2}+m_{2}x_{1}}{m_{1}+m_{2}} , \frac{m_{1}y_{2}+m_{2}y_{1}}{m_{1}+m_{2}}\right )$
$\left ( 0,0 \right )=\left ( \frac{1*a+2*p}{1+2} , \frac{1*b+2*q}{1+2}\right )$
$\left ( 0,0 \right )=\left ( \frac{a+2p}{3} , \frac{b+2q}{3}\right )$
$\frac{a+2p}{3}=0$ and $\frac{b+2q}{3}=0$
a+2p=0 and b+2q=0…A…
a+2p=b+2q
2p-2q=b-a …i
It is given that BC=x+y-2=0
Since, the above equation passes through p,q
p+q-2=0
Now we find the slope of line AP
$m=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{b-0}{a-0}=\frac{b}{a}$
Equation of line BC is x+y-2=0
y= -x+2
y=-(1)x+2
Since the above equation is in y=mx+b form
So, slope of line BC is m_BC=-1
Since both lines rae perpendicular $\frac{b}{a}*\left ( -1 \right )=-1$
b=a
Putting this value in equation (i )we get 2p-q=b-b=0
p=q
Now putting this value in equation (i) we get p+q-2=0
2p=2
p=1
q=1
Putting the value of p and q in equation A , we get a+2*1=0 and b+2*1=0
a=-2 and b=-2
So, the coordinates of vertex A (a,b) is (-2,-2)
Hence, the correct option is c

Question:42

Fill in the blanks
If a, b, c are in A.P., then the straight lines ax + by + c = 0 will always pass through ____.
Answer:

Given that a,b,c are in AP $b=\frac{a+c}{2}$
2b=a+c
a-2b+c=0….i
Now comparing equation iwith the given equation ax+by+c=0 we get x=1, y=-2
So, the line will pass through (1,-2 )

Question:43

Fill in the blanks
The line which cuts off equal intercept from the axes and pass through the point (1, –2) is ____.

Answer:

Equation of straight line in intercept form= $\frac{x}{a}+\frac{y}{b}=1$ where a and b are intercepts
Given that a=b $\frac{x}{a}+\frac{y}{a}=1$
$\frac{x+y}{a}=1$
x+y=a….(i)
If equation ipasses through the point (1, -2) we get 1+(-2)=a
1-2=a
a=-1
Putting the value of a in equation i we get x+y=-1
x+y+1=0

Question:44

Fill in the blanks
Equations of the lines through the point (3, 2) and making an angle of 45° with the line x – 2y = 3 are ____.

Answer:

Given equation is x-2y=3
x-3=2y
$y=\frac{1}{2}x+\left ( -\frac{3}{2} \right ).................(i)$
The slope of the equation can be found by comparing with y=mx+b form
So, $m_{1}=\frac{1}{2}$
We have to find an equation which is passing through the point (3,2)
A line passing through the point x₁,y₁ has an equation y-y₁=m(x-x₁)
So, here x₁=3 and y₁=2
y-2=m(x-3)....…(ii)
Now, it is given that the angle between the given two lines is 45⁰
$\tan \theta =\left | \frac{\left ( m_{1}-m_{2} \right )}{1+m_{1}m_{2}} \right |$
Putting the values of m₁and m₂ in above equation we get tan45^{0 =}
$1=\left | \frac{ 2m-1 }{2+m} \right |$
$1=\pm \frac{ 2m-1 }{2+m}$
2m-1=2+m or-(2m-1)=2+m
2m-m=2+1 or -2m+1-m=2
m=3 or-3m=1 or m=-1/3
Putting the value of m=3 in equation (ii) we get y-2=3(x-3)
y-2=3x-9
3x-y-9+2=0
3x-y-7=0
Putting the value of m=-1/3 in equation (ii) we get y-2= -1/3(x-3)
3(y-2)=3-x
3y-6=3-x
x+3y-6-3=0
x+3y-9=0

Question:45

Fill in the blanks
The points (3, 4) and (2, – 6) are situated on the ____ of the line 3x – 4y – 8 = 0.

Answer:

Given line 3x-4y-8=0 and given points are (3,4) and (2,-6)
For point (3,4)
3(3)-4(4)-8=9-16-8
=9-24= -15<0
Forpoint (2, -6) 3(2)-4(-6)-8 =6+24-8
=30-8=22>0
So the points (3,4)and (2,-6) are situated on the opposite sides of 3x-4y-8=0

Question:46

Fill in the blanks
A point moves so that square of its distance from the point (3, –2) is numerically equal to its distance from the line 5x – 12y = 3. The equation of its locus is ____.

Answer:

Given point is (3, -2) and equation of line is 5x-12y=3
Let (p,q) be any moving point
Distance between them (p,q) and (3,-2) $d_{1}=\sqrt{\left ( p-3 \right )^{3}+\left ( q-\left ( -2 \right ) \right )^{2}}$
(d₁)²=(p-3)²+(q+2)²
Now, distance of the point (p,q) from the given line 5x-12y-3=0
$d=\frac{\left | Ax+By+C \right |}{\sqrt{A^{2}+B^{2}}}$
$d_{2}=\frac{\left | 5p-12q-3 \right |}{\sqrt{(5)^{2}+(12)^{2}}}= \frac{\left | 5p-12q-3 \right |}{\sqrt{25+144}}=\frac{\left | 5p-12q-3 \right |}{\sqrt{169}}$
= $=\frac{\left | 5p-12q-3 \right |}{13}$
Taking numerical values only, we have (p-3)²+(q-2)²= $=\frac{5p-12q-3 }{13}$
13[(p-3)²+(q+2)²]=5p-12q-3
13[p²+9-6p+q²+4+4q]=5p-12q-3
On solving we get 13p²+13q²-83p+64q+172=0
A point moves so that square of its distance from the point (3,-2) is numerically equal to its distance from the line 5x-12y=3 .
The equation of its locus is 13p²+13q²-83p+64q+172=0

Question:47

Fill in the blanks
Locus of the mid-points of the portion of the line x sin θ + y cos θ = p intercepted between the axes is ____.

Answer:

Given equation of the line is x sinθ+y cosθ=p…..(i)
Let Ph,kbe the midpoint of the given line where it meets the two axis at a,0and 0,b.
Since (a,0) lies on equation (i) then asinθ+0=p a= $\frac{p}{\sin \theta }$ …..(ii)
(0,b) also lies on the equation i then 0+bcosθ=p
$b=\frac{p}{\cos \theta }$ ……(iii)
Since, P (h,k) is the midpoint of the given line $h=\frac{a+0}{2}=\frac{a}{2}$
2h=a and $k=\frac{0+b}{2}=\frac{b}{2}$
2k=b
Putting the value of a=2h in equation (ii) we get $2h=\frac{p}{\sin \theta }$
$\sin \theta =\frac{p}{2k }$ …..(iv)
Putting the value of b=2k in equation (ii) we get $2k =\frac{p}{\cos \theta }$
$\cos \theta =\frac{p}{2k }$ ….(v)
Squaring and adding equation (iv) and (v), we get $\sin^{2}\theta + \cos^{2}\theta=\left ( \frac{p}{2h} \right )^{2}+\left ( \frac{p}{2k} \right )^{2}$
$1= \frac{p^{2}}{4h^{2}}+ \frac{p^{2}}{4k^{2}}$ or $1= \frac{p^{2}}{4x^{2}}+ \frac{p^{2}}{4y^{2}}$
or 4x²y²=p²y²+p²x²
or 4x²y²=p²(x²+y²) is the locus of the mid-points of the portion of the line intercepted between the axes

Question:48

State whether the statements are true or false.
If the vertices of a triangle have integral coordinates, then the triangle can’t be equilateral.

Answer:

Let ABC be a triangle with vertices A(x₁,y₁), B (x₂,y₂) and C (x₃, y₃) where x_i, y_i, i=1,2,3 are integers
Then area of $ABC=\frac{1}{2}\left [ x_{1}\left ( y_{2}-y_{3} \right ) +x_{2}\left ( y_{3}-y_{1} \right ) +x_{3}\left ( y_{1}-y_{2} \right ) \right ]$
Since, xi and yi all are integers but $\frac{1}{2}$ is a rational number.
So, the result comes out to be a rational number. i.e . Area of ABC=a rational number
Suppose, ABC be an equilateral triangle, then area of ABC is= $\frac{\sqrt{3}}{4}\left ( AB \right )^{2}$
It is given that vertices are integral coordinates, it means the value of coordinates is in whole
number. Therefore, the value of (AB)² is also an integer.
$\frac{\sqrt{3}}{4}$ (positive integer)
But, $\sqrt{3}$ is an irrational number
Area of triangle ABC=an ir-rational number
This contradicts the fact that the area is a rational number
Hence, the given statement is true.

Question:49

State whether the statements are true or false.
The points A (– 2, 1), B (0, 5), C (– 1, 2) are collinear.

Answer:

Given points are A(-2,1), B (0,5) and C(-1,2)
There are two ways to find that given points are collinear or not .
The first is if 3 points are collinear, then slope of any two pairs of points will be equal.
Second way is that if the value of area of triangle formed by the 3 points is zero, then the points
are collinear.
Slope of AB $m=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}=\frac{5-1}{0-\left ( -2 \right )}=2$
Slope of BC $m==\frac{2-5}{-1-0}=\frac{3}{-1}=3$
Slope of CA is $m==\frac{1-2}{-2-\left ( -1 \right )}=\left (-\frac{1}{-2+1} \right )=1$
Since, the slopes are different.
So, the given points are not collinear
Hence, the given statement is False

Question:50

State whether the statements are true or false.
Equation of the line passing through the point (a cos3θ, a sin3θ) and perpendicular to the line x sec θ + y cosec θ = a is x cos θ – y sin θ = a sin 2θ.

Answer:

Let the equation of line y=mx+c….(i)
So, slope of the above equation is m
Given equation of line is x secθ+y cosecθ=a sin 2θ.
$\frac{\sec \theta x }{cosec \theta }+y=-\frac{a \sin 2 \theta}{cosec \theta }$
Since the slope of the equation is m'= $y=-\frac{\sec \theta }{cosec \theta }$
Given that equation (i) is perpendicular to x secθ+y cosec θ=a sin 2θ
m*m'= -1
$m*\left (-\frac{\sec \theta }{cosec \theta } \right )=-1$
$m=\frac{cosec \theta }{\sec \theta }$
Putting the value of m in equation (i)we get $y=\frac{cosec \theta }{\sec \theta }x+c$
$y=\frac{cosec \theta +c\left ( sec \theta \right ) }{\sec \theta }$
ysecθ=x cosec θ+c secθ
x cosec θ-y secθ=k….(ii)
If equation (ii) passes through the point (a cos³θ, a sin³θ)
(a cos³θ )cosecθ-(a sin³θ) secθ=k
$\frac{a\cos ^{3} \theta}{\sin \theta}- \frac{a\sin ^{3} \theta}{\cos \theta}=x \, cosec\, \, \theta -y\sec \theta$
$\frac{a\cos ^{4} \theta -a\sin ^{4}\theta}{\sin \theta \cos \theta}=\frac{x}{\sin \theta }-\frac{x}{\cos \theta }$
a[(cos²θ-sin²θ)(cos²θ+sin²θ)]=x cosθ-ysinθ
a[cos²θ-sin²θ]=xcosθ-ysinθ
a[cos2θ]=xcos θ-y sinθ
The given statement is FALSE

Question:51

State whether the statements are true or false.
The straight line 5x + 4y = 0 passes through the point of intersection of the straight lines x + 2y – 10 = 0 and 2x + y + 5 = 0.

Answer:

Given equations are x+2y-10=0….(i) and 2x+y+5=0…..(ii)
The point of intersection is obtained by solving them together i.e. $\left ( -\frac{20}{3} ,\frac{25}{3}\right )$
If the given line 5x+4y=0 passes through the point $\left ( -\frac{20}{3} ,\frac{25}{3}\right )$ then $5\left ( -\frac{20}{3} \right )+4\left ( \frac{25}{3} \right )=0$
$-\frac{100}{3}+ \frac{100}{3} =0$
0=0
So, the given line passes through the point of intersection of the given lines.
Hence, the given statement is True.

Question:52

State whether the statements are true or false.
The vertex of an equilateral triangle is (2, 3) and the equation of the opposite side is x + y = 2. Then the other two sides are $y-3=\left ( 2\pm \sqrt{3} \right )\left ( x-2 \right )$ .

Answer:

Let ABC be an equilateral triangle with vertex (2,3) and the equation of the opposite side is x+y=2
In the case of equilateral triangle θ=60
Let the slope of line AB is m and the slope of the given equation x+y=2 is m₂=-1
We know that $\tan \theta =\left | \frac{\left ( m_{1}-m_{2} \right )}{1+m_{1}m_{2}} \right |$
Putting the values of m₁ and m₂ in the above equation, we get $\tan 60^{\circ}=\left | \frac{\left ( m-\left ( -1 \right ) \right )}{1+m\left ( -1 \right )} \right |$
$\sqrt{3}=\left | \frac{m+1}{1-m}\right |$
$\sqrt{3}=\pm \left (\frac{m+1}{1-m} \right )$
$\sqrt{3}= \left (\frac{m+1}{1-m} \right ) 0r -\left (\frac{m+1}{1-m} \right )$
$\left ( 1-m \right )\sqrt{3}=1+m \, \, or \, \, \left ( 1-m \right )\left (-\sqrt{3} \right )=-1-m$
$\sqrt{3}-\sqrt{3}m=1+m \, \, or\, \, -\sqrt{3}+\sqrt{3}m=-1-m$
$\sqrt{3}-1=m \left ( 1+\sqrt{3} \right )\, \, or\, \, -\left (\sqrt{3}-1 \right )=-\left ( \sqrt{3} +1\right )$
$m=\frac{\sqrt{3}+1}{\sqrt{3}-1}...........(i)\, \, \, or\, \, m=\frac{\sqrt{3}-1}{\sqrt{3}+1}...........(ii)$
Rationalizing both the equations we get $m=2+ \sqrt{3} \, \, \, or\, \, m=2- \sqrt{3}$
So, the slope of line AB is $2\pm + \sqrt{3}$
Thus, the equations of the other two lines joining the point (2, 3) are $y-3=2\pm \sqrt{3}\left ( x-2 \right )$
Hence, the given statement is True.

Question:53

State whether the statements are true or false.
The equation of the line joining the point (3, 5) to the point of intersection of the lines 4x + y – 1 = 0 and 7x – 3y – 35 = 0 is equidistant from the points (0, 0) and (8, 34).

Answer:

Given two lines are 4x+y-1=0…(i ) and 7x-3y-35=0…..(ii)
Now, point of intersection of these lines can be find out as x=2 and y=-7
To find the equation of the line joining the point (3,5) and (2,-7)
$y-y_{1}=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}\left ( x-x_{1} \right )$
$y-5=\frac{-7-5}{2-3}\left ( x-3 \right )$
$y-=-\frac{12}{-1}\left ( x-3 \right )$
y-5=12x-3
y-5=12x-36
12x-y-31=0……iv
Now, the distance of equation (iv) from the point (0,0) is d= $\frac{\left |Ax+By+C \right |}{\sqrt{A^{2}+B^{2}}}$
$d=\frac{\left |12(8)-34-31 \right |}{\sqrt{12^{2}+(-1)}}$

$d=\frac{\left | 31\right |}{\sqrt {145}}$
Now the distance of
equation iv from the point (8,34) is $\frac{\left |Ax+By+C \right |}{\sqrt{A^{2}+B^{2}}}$
$d=\frac{\left |12(8)-34-31 \right |}{\sqrt{12^{2}+(-1)}}=\frac{\left | 31\right |}{\sqrt {145}}$
Hence, the equation of line 12x-y-31=0 is equidistant from (0,0)and (8,34)
Hence, the given statement is True.

Question:54

State whether the statements are true or false.

The line $\frac{x}{a}+\frac{y}{b}=1$ moves in such a way that $\frac{1}{a^{2}}+\frac{1}{b^{2}}=\frac{1}{c^{2}}$ , where c is a constant. The locus of the foot of the perpendicular from the origin on the given line is $x^{2}+y^{2}=c^{2}$

Answer:

Equation of line $\frac{x}{a}+\frac{y}{b}=1$ ……i
Equation of line passing through the origin and perpendicular to the given line $\frac{x}{a}-\frac{y}{b}=0$ ….ii
Now the foot of perpendicular from origin on the line (i) is the point of intersection of lines (i) and ii
So, to find its locus we have to eliminate the variable a and b
Squaring and adding both the equations we get $\left (\frac{x}{a}+\frac{y}{b} \right )^{2}+\left (\frac{x}{a}-\frac{y}{b} \right )^{2}=1+0$
$\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}+\frac{2xy}{ab}+\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}-\frac{2xy}{ab}=1$
$\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}+\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1$
$x^{2}\left (\frac{1}{a^{2}}+\frac{1}{b^{2}} \right )+y^{2}\left (\frac{1}{a^{2}}+\frac{1}{b^{2}} \right )=1$
$\left (x^{2}+y^{2} \right )+\left (\frac{1}{a^{2}}+\frac{1}{b^{2}} \right )=1$
$\left (x^{2}+y^{2} \right )+\left (\frac{1}{c^{2}} \right )=1$
x²+y²=c²
Hence, the given statement is true.

Question:55

State whether the statements are true or false.
The lines ax + 2y + 1 = 0, bx + 3y + 1 = 0 and cx + 4y + 1 = 0 are concurrent if a, b, c are in G.P.

Answer:

Given that ax+2y+1=0 bx+3y+1=0 and cx+4y+1=0 are concurrent .
For the lines to be concurrent $\begin{vmatrix} a & 2 & 1 \\ b & 3 & 1 \\ c & 4 & 1 \end{vmatrix}=1$
Now expanding along first column we get
a[3-4]-b[2-4]+c[2-3]=0
-a+2b-c=0
2b=a+c and we know that if a, b ,c are in AP then $b=\frac{a+c}{2}$
This means given lines are in AP not in GP
Hence, the given statement is False.

Question:56

State whether the statements are true or false.
Line joining the points (3, – 4) and (– 2, 6) is perpendicular to the line joining the points (–3, 6) and (9, –18).

Answer:

Given points are (3,-4), (-2,6), (-3,6) and (9,-18)
Now we find the slope since the lines are perpendicular, the product of the slopes is -1 i.e. m₁m₂= -1
Slope of the line joining the points (3,-4) and (-2,6)
$m=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}$
Here, x₁=3, x₂=-2 , y₁=-4 and y₂=6
$m_{1}=\frac{6-\left ( -4 \right )}{-2-3}=\frac{6+4}{-5}=\frac{10}{-5}=-2$
Now, slope of the line joining the points -(3,6) and (9,-18)
$m=\frac{y_{2}-y_{1}}{x_{2}-x_{1}}$
Here, x₁=-3, x₂=9, y₁=6 and y₂=-18
$m_{2}=\frac{-18-6}{9-(-3)}=\frac{24}{9+3}=-\frac{24}{12}=-2$
m₁=m₂=-2
and m₁m₂= -2*(-2)=-4 ≠-1
So, the lines are parallel and not perpendicular
Hence, the given statement is False

Question: 57

Match the questions given under Column C1 with their appropriate answers given under the Column C2.

Column C₁	Column C₂
a) The coordinates of the points P and Q on the line x+5y=13 which are at a distance of 2 units from the line 12x-5y+26=0 are	i) (3,1), (-7,11)
b) The coordinates of the points on the line x+y=4 which are at a unit distance from the line 4x-3y+10=0 are	ii) -1/3, 11/3, 4/3, 7/3
c) The coordinates of the points on the line joining A (-2,5) and B(3,1) such that AP=+Q=QB are	iii) 1, 12/5,-3,16/5

Answer:

Let P (x₁,y₁) be any point on the given line x+5y=13
x₁+5y₁=13
5y₁=13-x₁….(i)
Distance of the point P(x₁,y₁)from the equation 12x-5y+26=0
$d=\frac{\left | Ax+By+C \right |}{\sqrt{A^{2}+B ^{2}}}$
$2=\frac{\left | 12x_{1}+5y_{1}+26 \right |}{\sqrt{\left ( 12 \right )^{2}+\left ( -5 \right )^{2}}}$
$2=\frac{\left | 12x_{1}-\left ( 13-x_{1} \right )+26 \right |}{\sqrt{144+25}} = \frac{\left | 12x_{1}-13+x_{1} +26 \right |}{13}$
$2=\frac{\left | 13x_{1}+13 \right |}{13}$
2=|x₁+1|
2= ±(x₁+1)
So x₁=1….(ii) or x₁=-3…. (iii)
Putting the value in equation (i) we get 5y₁=13-1=12
$y_{1}=\frac{12}{5}$
Putting the value of x₁=-3 in the same equation we get 5y₁=13-(-3)=16
$y_{1}=\frac{16}{5}$
Hence, the required points on the given line are $\left ( 1,\frac{12}{5} \right ) \, \, and\, \, \left ( -3,\frac{16}{5} \right )$ and -3,165
Hence, (a)-(iii)
bLet P x₁, y₁ be any point lying in the equation x+y=4
x₁+y₁=4…. i
Now, the distance of the point from the equation is $d=\frac{\left | Ax+By+C \right |}{\sqrt{A^{2}+ B ^{2}}}$
$1=\frac{\left | 4x_{1}+3y_{1}-10 \right |}{\sqrt{(4)^{2}+(3)2}}=\frac{\left | 4x_{1}+3y_{1}-10 \right |}{\sqrt{16-9}}$
$1=\left |\frac{ 4x_{1}+3y_{1}-10 }{5}\right |$
4x₁+3y₁-10= ±5
either 4x₁+3y₁-10=5 or 4x₁+3y₁-10=-5
4x₁+3y₁=15 ….(ii) or 4x₁+3y₁=5…..(iii)
From equation i we have y₁=4-x₁….(iv)
Putting the value of y₁ in equation ii we get 4x₁+34-x₁=15
4x₁+12-3x₁=15
x₁=3 Putting the value of x₁in equation (iv) we get y₁=4-3=1
Putting the value of y₁in equation iii we get 4x₁+34-x₁=5
4x₁+12-3x₁=5
x₁=5-12=-7
Putting the value of x₁ in equation (iv) , we get y₁=4-(-7)
y₁=4+7=11
Hence, the required points on the given line are (3,1) and (-7,11)
Hence, b-i
c Given that AP=PQ=QB and given points are A(-2,5) and B(3,1)
Firstly, we find the slope of the line joining the points (-2,5) and (3,1)
Slope of line joining two points= $\frac{y_{2}-y_{1}}{x_{2}-x_{1}}$
$m_{AB}=\frac{1-5}{3-\left ( -2 \right )}=-\frac{4}{3+2}=-\frac{4}{5}$
Now equation of line passing through the point (-2, 5) y-5=-4/5[x-(-2)]
5y-25=-4(x+2)
4x+5y-17=0 Let P (x_1,y₁) and Q (x₂,y₂) be any two points on the AB
P(x₁,y₁) divides the line AB in the ratio 1:2
$x_{1}=\frac{1*3+2*(-2)}{1+2}=\frac{3-4}{3}=\frac{1}{3}$
$y_{1}=\frac{1*1+2*5}{1+2}=\frac{1+10}{3}=\frac{11}{3}$
Now, Q (x₂,y₂) is the midpoint of PB $x_{2}=\frac{3+\left (-\frac{1}{3} \right )}{2}=\frac{8}{6}=\frac{4}{3}$
$y_{2}=\frac{1+{11}{3}}{2}=\frac{3+11}{6}=\frac{14}{6}=\frac{7}{3}$
Hence, the coordinates of Q (x₂,y₂) is (4/3,7/3)
Hence, c-ii

Question:58

The value of the λ, if the lines (2x + 3y + 4) + λ (6x – y + 12) = 0 are

Column C₁	Column C₂
a) parallel to y-axis is	i) $\lambda =-\frac{3}{4}$
b) perpendicular to 7x+y-4=0	ii) $\lambda =-\frac{1}{3}$
c) Passes through (1,2) is	iii) $\lambda =-\frac{17}{41}$
d) parallel to x-axis is	iv) $\lambda =3$

Answer:

a ) Given equation is (2x+3y+4)+ λ(6x-y+12)=0
2x+3y+4+6λx-λy+12λ=0
(2+6λ)x+(3-λ)y+4+12λ=0…. (i)
If equation i is parallel to y-axis, then 3-λ=0
λ=3
Hence, iv.
b) Given equation is 2x+3y+4+λ6x-y+12=0
2x+3y+4+6λx-λy+12λ=0
(3-λ)y= -4-12λ-(2+6λ)x
$y=-\left (\frac{2+6\lambda }{3-\lambda } \right )x+\left ( -1 \right )\left (\frac{4+12\lambda }{3-\lambda } \right )$
Since, the above equation is in y=mx+b form
So the slope of equation (i) is $m_{1}=-\left (\frac{2+6\lambda }{3-\lambda } \right )$
Now the second equation is 7x+y-4=0…..(ii)
y=-7x+4
So, the slope of equation (ii) is m₂=-7
Now equation i is perpendicular to equation (ii)
m₁m₂=-1
$-\left (\frac{2+6\lambda }{3-\lambda } \right )*\left ( -7 \right )=-1$
(2+6λ)*7=-(3-λ)
On solving we get λ=-17/41
Hence, (b)-(iii)
c) Given equation is (2x+3y+4)+λ(6x-y+12)=0
If the above equation passes through the point (1,2) then [2*1+3*2+4]+λ[6*1-2+12]=0
2+6+4+λ(6+10)=0
12+16λ=0
12=-16λ
λ=-12/16=-3/4
Hence, (c)-(i)
d) Given equation is (2x+3y+4)+λ(6x-y+12)=0
2x+3y+4+6λx-λy+12λ=0
(2+6λ)x+(3-λ)y+4+12λ=0…..(i)
If equation (i) is parallel to x axis, then 2+6λ=0 6λ=-2
λ=-1/3
(d)-(ii)

Question:59

The equation of the line through the intersection of the lines 2x – 3y = 0 and 4x – 5y = 2 and

Column C₁	Column C₂
a) through the point (2,1) is	i) 2x-y =4
b)perpendicular to the line x+2y+1=0 is	ii)x+y-5=0
c)parallel to the line 3x+4y+5=0 is	iii)x-y-1=0
d) equally inclined to the axes is	iv) 3x-4y-1=0

Answer:

.a) Given equations are 2x-3y=0….(i) and 4x-5y=2….(ii )
Equation of line passing through equation iand (ii), we get 2x-3y+λ4x-5y-2=0….(iii)
If the above equation passes through the point (2,1) we get (2*2-3*1)+λ(4*2-5*1-2)=0
(4-3)+λ(8-5-2)=0
1+λ=0
λ=-1
Putting the value of λ in equation iii we get (2x-3y)+(-1)(4x-5y-2)=0
2x-3y-4x+5y+2=0
-2x+2y+2=0
x-y-1=0
Hence, (a)-(iii)
b) Given equations are 2x-3y=0….(i) and 4x-5y=2….(ii)
Equation of line passing through equation i and ii, we get 2x-3y+λ4x-5y-2=0…(iii)
2x-3y+4λx-5λy-2λ=0
x(2+4λ)-y(3+5λ)-2λ=0
-y(3+5λ)= -(2+4λ)+2λ
$y=\left ( \frac{2+4\lambda }{3+5\lambda } \right )+\left ( -\frac{2}{3+5\lambda } \right )$
Slope of equation iii is m₁= $\left ( \frac{2+4\lambda }{3+5\lambda } \right )$
Now, we find the slope of thegiven line x+2y+1=0…..(iv)
2y=-x-1 y=-1/2x+(-1/2)
So slope of equation (iv) is m₂=(-1/2)
We know that, if two lines are perpendicular to each other then the product of their slopes is equal to-1
So, m₁*m₂=-1
$\left ( \frac{2+4\lambda }{3+5\lambda } \right )*\left (-\frac{1}{2} \right )=-1$
2+4λ=2*(3+5λ)
2+4λ=6+10λ
-6λ=4
λ=-4/6= -2/3
Putting the value of λ in equation (iii) we get (2x-3y)+(-2/3)(4x-5y-2)=0
6x-9y-8x+10y+4=0
-2x+y+4=0
2x-y=4
b-i
c) Given equations are 2x-3y=0….(i) and 4x-5y=2…..(ii)
Equation of line passing through equation iand (ii), we get (2x-3y)+ λ(4x-5y-2)=0….iii
2x-3y+4λx-5λy-2λ=0
x(2+4λ)-y(3+5λ)-2λ=0
$y=\left ( \frac{2+4\lambda }{3+5\lambda } \right )x+\left ( -\frac{2}{3+5\lambda } \right )$
So slope of equation iii is m₁= $\left ( \frac{2+4\lambda }{3+5\lambda } \right )$
Now, we find the slope of the given line 3x-4y+5=0
3x+5=4y y=3/4x+5/4
So slope of equation is 3/4
If 2 lines are parallel then their slopes are also equal So $\left ( \frac{2+4\lambda }{3+5\lambda } \right )=\frac{3}{4}$
4(2+4λ)=3(3+5λ)
8+16λ=9+15λ
λ=1
Putting the values of λ in equaaion iii we get (2x-3y)+(4x-5y-2)=0
2x-3y+4x-5y-2=0
6x-8y-2=0
3x-4y-1=0
Hence, c-iv
d) Given equations are 2x-3y=0…(i) and 4x-5y=2….(ii)
Equation of line passing through equation (i) and (ii), we get (2x-3y)+λ(4x-5y-2)=0
x(2+4λ)-y(3+5λ)-2λ=0
-y(3+5λ)= -(2+4λ)+2λ
$y=\left ( \frac{2+4\lambda }{3+5\lambda } \right )x+\left (\frac{-2 }{3+5\lambda } \right )$
Slope of equation iii is $\left ( \frac{2+4\lambda }{3+5\lambda } \right )$
Since the equation is equally inclined with axes, it means that the line makes equal angles with both the
coordinate axes.
It will make an angle of 45⁰ or 135⁰
m₂=tan45⁰ andtan135⁰
= 1 and-1
$y=\left ( \frac{2+4\lambda }{3+5\lambda } \right )=-1 \, \, or\, \, \left (\frac{2+4\lambda }{3+5\lambda } \right )=1$
2+4λ=-3+5λ or 2+4λ=3+5λ
So λ=-5/9 or-1
Putting the value in equation iii we get (2x-3y)+(-5/9)(4x-5y-)2=0
18x-27y-20x+25y+10=0
-2x-2y+10=0
x+y-5=0
If λ=-1, then the required equation is (2x-3y)+(-1)( 4x-5y-2)=0
2x-3y-4x+5y+2=0
-2x+2y+2=0
x-y-1=0
d-ii, iii

More About NCERT Exemplar Class 11 Maths Chapter 10

The students trying to ace their examinations can access NCERT Exemplar Class 11 Maths solutions chapter 10 PDF download from here. This will help them in getting a helpful approach towards the preparation of your exams that are fabricated by our experts through thorough study.

The NCERT Exemplar solutions for Class 11 Maths chapter 10 tries to aid students with properly examined solutions from the perspective of exams and its usage in various other fields.

Also read - NCERT Solutions for Class 11 Maths Chapter 10

Topics and Subtopics in NCERT Exemplar Class 11 Maths Solutions Chapter 10

Introduction
Slope of a line
Slope of a line when coordinates of any two points on the line are given
Conditions for parallelism and perpendicularity of lines in terms of their slopes
Angle between two lines
Collinearity of three points
Various forms of the equation of the line
Horizontal and vertical lines
Point-slope form
Two-point form
Slope-intercept form
Intercept-form
Normal form
General Equation of a line
Different forms of Ax + By + C = 0
Distance of a point from a line
Distance between two parallel lines

What will the students learn from NCERT Exemplar Class 11 Maths Solutions Chapter 10?

The students will get a brief introduction of coordinate geometry in two dimensions along with the main focus on one of the simplest figures, which is a straight line. NCERT Exemplar solutions for Class 11 Maths chapter 10 covers different concepts relating to a straight line that will teach students about the calculation of angle between two lines, the distance between two parallel lines, determining the slope of a line and other forms of the equation of a line. A straight line is the simplest shape of geometry, but it is also the most important part as it acts as the base for the introduction of other shapes such as curves that will help students in the further lessons. The learners will also benefit from NCERT Exemplar Class 11 Maths solutions chapter 10 through its use and application in different fields, including physics, architecture, geometry, and much more.

NCERT Solutions for Class 11 Mathematics Chapters

Chapter 1 Sets

Chapter 2 Relations and Functions

Chapter 3 Trigonometric Functions

Chapter 4 Principle of Mathematical Induction

Chapter 5 Complex Numbers and Quadratic Equations

Chapter 6 Linear Inequalities

Chapter 7 Permutations and Combinations

Chapter 8 Binomial Theorem

Chapter 9 Sequences and Series

Chapter 11 Conic Sections

Chapter 12 Introduction to Three Dimensional Geometry

Chapter 13 Limits and Derivatives

Chapter 14 Mathematical Reasoning

Chapter 15 Statistics

Chapter 16 Probability

Also, check -

JEE Main Highest Scoring Chapters & Topics

Just Study 40% Syllabus and Score upto 100%

Download EBook

Important Topics To Cover in NCERT Exemplar Class 11 Maths Solutions Chapter 10

Some of the important topics for students to review from this chapter are:

The learners should revise all the basics and concepts of coordinate geometry learned previously for better understanding.
NCERT Exemplar Class 11 Maths chapter 10 solutions covers the calculation of inclination of the line along with gradient or slope of a line in two-dimensional planes.
The students should study all the solutions of NCERT books along with examples to get a better understanding of concepts.
The students should cover various forms of the equation of lines from an examination perspective.

Check Chapter-Wise NCERT Solutions of Book

Chapter-1	Sets
Chapter-2	Relations and Functions
Chapter-3	Trigonometric Functions
Chapter-4	Principle of Mathematical Induction
Chapter-5	Complex Numbers and Quadratic equations
Chapter-6	Linear Inequalities
Chapter-7	Permutation and Combinations
Chapter-8	Binomial Theorem
Chapter-9	Sequences and Series
Chapter-10	Straight Lines
Chapter-11	Conic Section
Chapter-12	Introduction to Three Dimensional Geometry
Chapter-13	Limits and Derivatives
Chapter-14	Mathematical Reasoning
Chapter-15	Statistics
Chapter-16	Probability

NCERT Exemplar Class 11 Solutions

NCERT Exemplar Class 11 Biology Solutions

NCERT Exemplar Class 11 Chemistry Solutions

NCERT Exemplar Class 11 Physics Solutions

Read more NCERT Solution subject wise -

Also, read NCERT Notes subject wise -

Also Check NCERT Books and NCERT Syllabus here:

Frequently Asked Questions (FAQs)

1. Are these solutions helpful from an exam point of view?

Yes, NCERT Exemplar Class 11 Maths chapter 10 solutions are very important from the perspective of exams including Board exams as well as other competitive exams.

2. Is the chapter important for the Board examination?

Yes, NCERT Exemplar Class 11 Maths solutions chapter 10 are very important for the Board examination as it has the relevant weightage of marks along with its importance in other competitive exams.

3. What are some of the important topics from this chapter that have practical application in other fields?

The important topics to be covered in this chapter are the slope of a line, general equation of a line, the distance of a point from a line and various forms of the equation of a line in coordinate geometry.

Also Read

NCERT Class 11 Chemistry Chapter 4 Notes Chemical Bonding and Molecular Structure - Download PDF

Hydrogen Class 11th Notes - Free NCERT Class 11 Chemistry Chapter 9 Notes - Download PDF

The S-Block Elements Class 11th Notes - Free NCERT Class 11 Chemistry Chapter 10 Notes - Download PDF

The P-Block Elements Class 11th Notes - Free NCERT Class 11 Chemistry Chapter 11 Notes - Download PDF

Environmental Chemistry Class 11th Notes - Free NCERT Class 11 Chemistry Chapter 14 Notes - Download PDF

NCERT Class 11 Chemistry Chapter 3 Notes - Download PDF

NCERT Solutions for Class 11 Physics Chapter 6 Work Energy and Power

NCERT Solutions for Class 11 Chemistry Chapter 5 States of Matter

NCERT Class 11 Physics Chapter 5 Notes Laws of Motion - Download PDF

NCERT Class 11 Physics Chapter 3 Notes Motion in a straight Line - Download PDF

NCERT Solutions for Exercise 10.1 Class 11 Maths Chapter 10 - Straight Lines

NCERT Solutions for Miscellaneous Exercise Chapter 16 Class 11 - Probability

NCERT Exemplar Class 11 Maths Solutions Chapter 12 Introduction to Three Dimensional Geometry

NCERT Exemplar Class 11 Maths Solutions Chapter 10 Straight Lines

NCERT Exemplar Class 11 Maths Solutions Chapter 6 Linear Inequalities

NCERT Exemplar Class 11 Maths Solutions Chapter 16 Probability

NCERT Exemplar Class 11 Maths Solutions Chapter 14 Mathematical Reasoning

NCERT Exemplar Class 11 Maths Solutions Chapter 7 Permutations and Combinations

Articles

MVPP Scholarship Exam 2024: Mukhyamantri Vigyan Pratibha Pariksha Registration, Exam Date

Dec 16, 2024

Silverzone ISSO Answer Key 2024-25: Download PDF

Dec 16, 2024

Silverzone IOEL Answer Key 2024 – Download International Olympiad of English Language Solutions

Dec 16, 2024

Silverzone Olympiad IOM Answer Key 2024-25: Qualifying Marks, Score

Dec 16, 2024

Silverzone STEM Olympiad Answer Key 2024-25: Check Answer Key for all Classes

Dec 16, 2024

Silverzone ABHO Answer Key 2024 | Download Akhil Bhartiya Hindi Olympiad Solutions

Dec 16, 2024

Silverzone SKGKO Answer Key 2024-25: Download Smart Kid GK Olympiad Answer Key @ silverzone.org

Dec 16, 2024

IGNOU BEd Answer Key 2025: Download OMR Sheet Here

Dec 13, 2024

Upcoming School Exams

National Institute of Open Schooling 10th examination

Application Date:21 November,2024 - 20 December,2024

Application Process

Exam Pattern

Mock Test

National Institute of Open Schooling 12th Examination

Application Date:21 November,2024 - 20 December,2024

Application Process

Exam Pattern

Admit Card

National Means Cum-Merit Scholarship

Admit Card Date:27 November,2024 - 22 December,2024

Eligibility Criteria

Application Process

Exam Pattern

West Bengal Board 12th Examination

Exam Date:02 December,2024 - 20 December,2024

Result

Exam Pattern

Admit Card

Gujarat Board Secondary School Certificate Examination

Late Fee Application Date:11 December,2024 - 20 December,2024

Exam Pattern

Admit Card

Result

View All School Exams

Get answers from students and experts

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

Option 1) less than 3	Option 2) more than 3 but less than 6
Option 3) more than 6 but less than 9	Option 4) more than 9

NCERT Exemplar Class 11 Maths Solutions Chapter 10 Straight Lines

NCERT Exemplar Class 11 Maths Solutions Chapter 10: Exercise: 10.3

More About NCERT Exemplar Class 11 Maths Chapter 10

Topics and Subtopics in NCERT Exemplar Class 11 Maths Solutions Chapter 10

What will the students learn from NCERT Exemplar Class 11 Maths Solutions Chapter 10?

NCERT Solutions for Class 11 Mathematics Chapters

Important Topics To Cover in NCERT Exemplar Class 11 Maths Solutions Chapter 10

NCERT Exemplar Class 11 Solutions

Frequently Asked Questions (FAQs)

Also Read

Articles

Upcoming School Exams

National Institute of Open Schooling 10th examination

National Institute of Open Schooling 12th Examination

National Means Cum-Merit Scholarship

West Bengal Board 12th Examination

Gujarat Board Secondary School Certificate Examination

Popular Questions

Colleges After 12th

Popular Course After 12th

Applications for Admissions are open.

JEE Main Important Physics formulas

JEE Main Important Chemistry formulas

TOEFL ® Registrations 2024

Pearson | PTE

JEE Main high scoring chapters and topics

JEE Main Important Mathematics Formulas

Explore on Careers360

NCERT Solutions

NCERT Exemplars

NCERT Books

NCERT Notes

CBSE

CBSE Class 10

CBSE Class 12th

CISCE Board 10th

IMO

IEO

NSO

NMMS

Schools By Medium

By Ownership

By City

By State

NVS

JNVST

Download Careers360 App

All this at the convenience of your phone

Scan and download the app