NCERT Exemplar Class 12 Physics Solutions Chapter 2 Electrostatic Potential and Capacitance
CBSE Class 12th Exam Date:17 Feb' 26 - 17 Feb' 26
Have you ever thought how the lightning on the thunderstorm can illuminate the entire sky? This spectacular phenomenon is a strong consequence of electrostatic problems-- huge electric charges accumulating and then exploding. This natural phenomenon is discussed in Class 12 Physics Chapter 2: Electrostatic Potential and Capacitance which deals with the interaction between charged particles and storage and transfer of energy in electric fields.
While registering for JEE Mains 2026, students will be required to upload photograph, signature, along with Aadhaar Card, category certificate etc. NTA has asked candidates to keep their certificates updated and authenticated to avoid discrepancies in the application process.
This Story also Contains
- NCERT Exemplar Class 11 Physics Solutions Chapter 2: MCQI
- NCERT Exemplar Class 11 Physics Solutions Chapter 2: MCQII
- NCERT Exemplar Class 11 Physics Solutions Chapter 2: Very Short Answer
- NCERT Exemplar Class 11 Physics Solutions Chapter 2: Short Answer
- NCERT Exemplar Class 11 Physics Solutions Chapter 2: Long Answer
- Main Topics of NCERT Exemplar Class 12 Physics Solutions Chapter 2
- NCERT Exemplar Class 12 Physics Solutions Chapter-Wise

NCERT Exemplar Solutions Class 12 Physics Chapter 2 will assist you in mastering these concepts by providing clear step-by-step solutions to the questions on electric potential, work and electric fields, properties of equipotential surfaces, and capacitor behavior. Such solutions are particularly handy since they have a lot of varieties of questions e.g. MCQs, short answers, long answers as well as very long answers, as is the case in competitive exams or board papers. As you gear up to take CBSE exams, JEE or NEET, in these exemplar solutions you will learn ways to solve problems and understand the most basic concepts of physics in a practical manner by applying logic.
Also check - NCERT Solutions for Class 12 Physics
NCERT Exemplar Class 11 Physics Solutions Chapter 2: MCQI
Question:2.1
A capacitor of $4\mu F$ is connected as shown in the circuit. The internal resistance of the battery is $0.5\Omega$. The amount of charge on the capacitor plates will be
$a) 0$
$b) 4\mu C$
$c) 16\; \mu C$
$d) 8\; \mu C$
Answer:
The answer is the option (d) $8\mu C$Current through the $2\Omega$ resistance considering internal resistance of the battery $1\Omega$,
$I=\frac{2.5V}{\left ( 2\Omega +0.5\Omega \right )}=1A$
The voltage across the internal resistance of the battery
$=\left ( 0.5\Omega \right )\left ( 1A \right )=0.5V$
The voltage across the $4\mu F$ capacitor
$2.5V-0.5V=2V$
Therefore, charge on the capacitor plates
$Q=CV=\left ( 4\mu F \right )\left ( 2V \right )=8\mu C$
Question:2.2
A positively charged particle is released from rest in a uniform electric field. The electric potential energy of the charge
a) remains a constant because the electric field is uniform
b) increases because the charge moves along the electric field
c) decreases because the charge moves along the electric field
d) decreases because the charge moves opposite to the electric field
Answer:
The answer is the option (c)
The direction of electric field is always perpendicular to one equipotential surface maintained at high electrostatic potential to other equipotential surface maintained at low electrostatic potential. The positively charged particle experiences electrostatic force along the direction of electric field i.e., from high electrostatic potential to low electrostatic potential. Thus, the work is done by the electric field on the positive charge, hence electrostatic potential energy of the positive charge decreases.
Question:2.3
Figure shows some equipotential lines distributed in space. A charged object is moved from point A to point B.
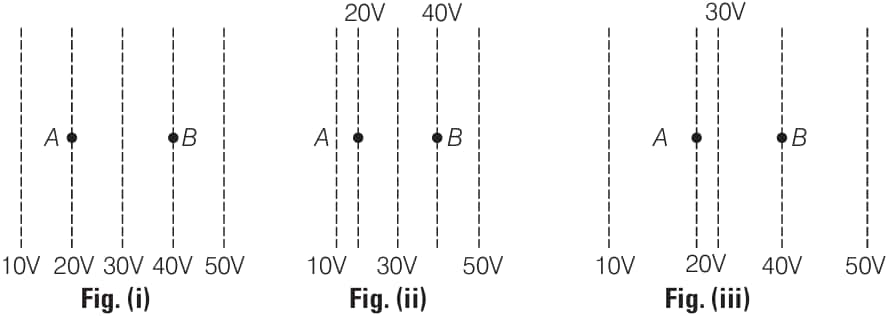
a) the work is done in fig (ii) is the least
b) the work is done in fig (i) is the greatest
c) the work is done in fig (iii) is greater than fig (ii) but equal to that in fig (i)
d) the work done is the same in fig (i), fig (ii), and fig (iii)
Answer:
The answer is the option (d)
The work done by a electrostatic force is given by $W_{12}=q\left(V_2-V_1\right)$. Here initial and final potentials are same in all three cases and same charge is moved, so work done is same in all three cases.
Question:2.4
The electrostatic potential on the surface of a charged conducting sphere is 100V. Two statements are made in this regard:
S1: At any point inside the sphere, the electric intensity is zero
S2: At any point inside the sphere, the electrostatic potential is 100V
Which of the following is a correct statement?
a) S1 and S2, both are false
b) S1 is true, S2 is false
c) S1 and S2, both are true but each of the statements is independent
d) S1 and S2, both are true and S1 is the cause of S2
Answer:
The answer is the option (d) S1 and S2, both are true, and S1 is the cause of S2
In this problem, the electric field intensity $E$ and electric potential $V$ are related as
$
E=-\frac{d V}{d r}
$
Electric field intensity $E=0$ suggest that $\frac{d V}{d r}=0$
This imply that $V=$ constant.
Thus, $E=0$ inside the charged conducting sphere causes, the same electrostatic potential 100 V at any point inside the sphere.
Question:2/5
Equipotential at a great distance from a collection of charges whose total sum is not zero are approximately
a) Ellipsoids
b) Spheres
c) Paraboloids
d) planes
Answer:
The correct answer is (b) spheres
In this problem, the collection of charges, whose total sum is not zero, with regard to great distance can be considered as a point charge. The equipotentials due to point charge are spherical in shape as electric potential due to point charge $q$ is given by
$
V=k \frac{q}{r}
$
This suggest that electric potentials due to point charge is same for all equidistant points. The locus of these equidistant points, which are at same potential, form spherical surface.
Question:2.6
A parallel plate capacitor is made of two dielectric blocks in series. One of the blocks has thickness $d_{1}$ and dielectric constant $K_{1}$ and the other has thickness $d_{2}$ and dielectric constant $K_{2}$ as shown in the figure. This arrangement can be thought of as a dielectric slab of thickness $d=d_{1}+d_{2}$ and effective dielectric constant K. The K is
$a) \frac{2K_{1}K_{2}}{\left ( K_{1}+K_{2} \right )}$
$b) \frac{K_{1}d_{1}+K_{2}d_{2}}{\left ( d_{1}+d_{2} \right )}$
$c) \frac{\left ( K_{1}d_{1}+K_{2}d_{2} \right )}{\left ( K_{1}+K_{2} \right )}$
$d)\frac{K_{1}K_{2}\left ( d_{1}+d_{2} \right )}{\left ( K_{1}d_{1}+K_{2}d_{2} \right )}$
Answer:
The answer is the option (d)${{C}_{eq}}=\dfrac{{{K}_{1}}{{K}_{2}}{{\varepsilon }_{0}}A}{{{K}_{1}}{{d}_{2}}+{{K}_{2}}{{d}_{1}}}.............(1)$
$C=\dfrac{K{{\varepsilon }_{0}}A}{{{d}_{1}}+{{d}_{2}}}.........(2)$
From (1) and (2)
$\dfrac{{{K}_{1}}{{K}_{2}}{{\varepsilon }_{0}}A}{{{K}_{1}}{{d}_{2}}+{{K}_{2}}{{d}_{1}}}=\dfrac{K{{\varepsilon }_{0}}A}{{{d}_{1}}+{{d}_{2}}}$
$K=\frac{K_{1}K_{2}\left ( d_{1}+d_{2} \right )}{\left ( K_{1}d_{1}+K_{2}d_{2} \right )}$
NCERT Exemplar Class 11 Physics Solutions Chapter 2: MCQII
Question:2.7
Consider a uniform electric field in the $\hat{z}$ direction. The potential is a constant
a) in all space
b) on the x-y plane for a given z
c) for any y for a given z
d) for any x for a given z
Answer:
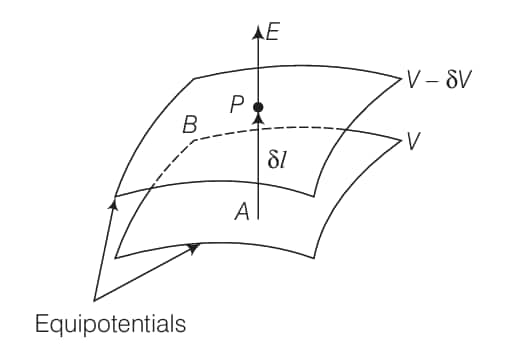
Here, the figure electric field is always remain in the direction in which the potential decreases steepest. Its magnitude is given by the change in the magnitude of potential per unit displacement normal to the equipotential surface at the point.
The electric field in z-direction suggest that equipotential surfaces are in $x-y$ plane. Therefore the potential is a constant for any $x$ for a given $z$, for any $y$ for a given $z$ and on the $x-y$ plane for a given $z$.
Question:2.8
Equipotential surfaces
a) will always be equally spaced
b) are closer in regions of large electric fields compared to regions of lower electric fields
c) will be more crowded near regions of large charge densities
d) will be more crowded near sharp edges of a conductor
Answer:
The electric field intensity $E$ is inversely proportional to the separation between equipotential surfaces. So, equipotential surfaces are closer in regions of large electric fields.
Since, the electric field intensities is large near sharp edges of charged conductor and near regions of large charge densities. Therefore, equipotential surfaces are closer at such places.
b) are closer in regions of large electric fields compared to regions of lower electric fields
c) will be more crowded near sharp edges of a conductor
d) will be more crowded near regions of large charge densities
Question:2.9
The work done to move a charge along an equipotential from A to B
a) is zero
b) cannot be defined as $-\int_{A}^{B}E.dl$
c) must be defined as $-\int_{A}^{B}E.dl$
d) can have a non-zero value
Answer:
Work done in displacing a charge particle is given by $W_{12}=q\left(V_2-V_1\right)$ and the line integral of electrical field from point 1 to 2 gives potential difference $V_2-V_1=-\int_1^2 E . d$ For equipotential surface, $V_2-V_1=0$ and $W=0$.
Note If displaced charged particle is $+1 C$, then and only then option (b) is correct.
Question:2.10
In a region of constant potential
(a) the electric field is uniform
(b) the electric field is zero
(c) there can be no charge inside the region.
(d) the electric field shall necessarily change if a charge is placed outside the region.
Answer:
The electric field intensity $E$ and electric potential $V$ are related as $E=0$ and for $V=$ constant, $\frac{d V}{d r}=0$
This implies that electric field intensity $E=0$.
c) in the inside region there can be no charge
d) zero electric field will be there
Question:2.11
In the circuit shown in the figure, initially key $K_{1}$ is closed and $K_{2}$ is open. Then $K_{1}$ is opened and $K_{2}$ is closed. Then
a) charge on $C_{1}$ gets redistributed i.e, $V_{1}=V_{2}$
b) charge on $C_{1}$ gets redistributed i.e,$\left ( Q_{1}+Q_{2} \right )=Q$
c) charge on $C_{1}$ gets redistributed such that $Q_{1}=Q_{2}$
d) charge on $C_{1}$ gets redistributed such that $C_{1}V_{1}+C_{2}V_{2}=C_{1}E$
Answer:
The charge stored by capacitor $C_1$ gets redistributed between $C_1$ and $C_2$ till their potentials become same i.e., $V_2=V_1$. By law of conservation of charge, the charge stored in capacitor $C_1$ when key $K_1$ is closed and key $K_2$ is open is equal to sum of charges on capacitors $C_1$ and $C_2$ when $K_1$ is opened and $K_2$ is closed i.e.,
$
Q_1^{\prime}+Q_2^{\prime}=Q
$
a) charge on $C_{1}$ gets redistributed such that $V_{1}=V_{2}$
d) charge on $C_{1}$ gets redistributed such that $\left ( Q_{1}+Q_{2} \right )=Q$
Question: 2.12
If a conductor has a potential $V\neq 0$ and there are no charges anywhere else outside, then
a) the charges must on the surface or inside itself
b) no charge will be in the body of the conductor
c) the charges must be only on the surface
d) the charges must be inside the surface
Answer:
The charge resides on the outer surface of a closed charged conductor.
The answer are the options,a) the charges must on the surface or inside itself
b) no charge will be in the body of the conductor
Question:2.13
(a) In A : Q remains same but C changes.
(b) In B : V remains same but C changes.
(c) In A : V remains same and hence Q changes.
(d) In B : Q remains same and hence V changes.
Answer:
Case A When key $K$ is kept closed and plates of capacitors are moved apart using insulating handle, the separation between two plates increases which in turn decreases its capacitance $\left(C=\frac{K \varepsilon_0 A}{d}\right)$ and hence, the charge stored decreases as $Q=C V$ ( potential continue to be the same as capacitor is still connected with cell).
Case B When key $K$ is opened and plates of capacitors are moved apart using insulating handle, charge stored by disconnected charged capacitor remains conserved and with the decreases of capacitance, potential difference $V$ increases as $V=Q / C$.
NCERT Exemplar Class 11 Physics Solutions Chapter 2: Very Short Answer
Question:2.14
Answer:
Here, $\sigma _{1}R_{1}=\sigma _{2}R_{2}$Hence, $\frac{\sigma _{1}}{\sigma _{2}}=\frac{R_{2}}{R_{1}}$
If we consider $R_{2}>R_{1}$, therefore, $\sigma _{1}>\sigma _{2}$
Therefore, it is clear from the above statement that the charge density of the smaller sphere is greater than the charge density of the larger sphere.
Question:2.15
Do free electrons travel to a region of higher potential or lower potential?
Answer:
We know that the charged particle in the electric field has a force, that can be expressed as $F=qE$Here, the direction of the electrostatic force experienced by the free electrons is exactly in the opposite direction of the electric field. The electrons travel from a lower potential region to a higher potential because the direction of the electric field is higher than the potential.
Question:2.16
Can there be a potential difference between two adjacent conductors carrying the same charge?
Answer:
Yes, it is possible and there may be a potential difference between two same charges carrying adjacent conductors. This is because the sizes of the conductors might be differentQuestion:2.17
Can the potential function have a maximum or minimum in free space?
Answer:
Because the atmosphere around the conductor that prevents the electric discharge is absent, therefore, the potential function cannot be maximum or minimum in free space.Question: 2.18
Answer:
Here we can observe that the work done in both of the cases is zero. The work done due to the electric force on the charge is in the closed-loop and the value of it is equal to zero.
NCERT Exemplar Class 11 Physics Solutions Chapter 2: Short Answer
Question:2.19
Answer:
Let's assume contradicting statement that the potential is not same inside the closed equipotential surface. Let the potential just inside the surface is different to that of the surface causing in a potential gradient $\left(\frac{d V}{d r}\right)$. Consequently electric field comes into existence, which is given by as $E=-\frac{d V}{d r}$.Consequently field lines pointing inwards or outwards from the surface. These lines cannot be again on the surface, as the surface is equipotential. It is possible only when the other end of the field lines are originated from the charges inside.
This contradict the original assumption. Hence, the entire volume inside must be equipotential.
Question:2.20
Answer:
|
Quantity
|
Battery is removed
|
Battery remains connected
|
|
|
 |
 |
|
Capacity
|
$C'=KC$
|
$C'=KC$
|
|
Charge
|
$Q'=Q$
|
$Q'=KQ$
|
|
Potential
|
$V'=\frac{V}{K}$
|
$V'V$
|
|
Intensity
|
$E'=\frac{E}{K}$
|
$E'=E$
|
|
Energy
|
$U'=\frac{U}{K}$
|
$U'=UK$
|
Question:2.21
Answer:
The electric potential will decrease in the direction of the electric field. The potential$E=-\frac{dV}{dr}$
The electric potential will also decrease again when the path from the charged conductor is taken to the uncharged conductor in the direction of the electric field.
This process will continue when another uncharged conductor is considered to the infinity lowering the potential even further.
As a result, the uncharged body is at intermediate potential and the charged body is at infinity potential.
Question:2.22
Answer:
The potential energy of a point charge q is U and this point is placed at potential V, $U=qV$
A negatively charged particle is placed at the axis of the ring with charge Q
Let a be the radius of the ring
The electric potential at the axial distance is given as
$V=\frac{1}{4\pi \epsilon _{0}}\frac{Q}{\sqrt{z^{2}+a^{2}}}$
The potential energy, U is given as
$U=\frac{1}{4\pi \epsilon _{0}}\frac{Qq}{\sqrt{1+\left ( \frac{z}{a} \right )^{2}}}$
When z=infinity, U=0.
When z=0, U is given as
$U=\frac{1}{4\pi \epsilon _{0}}\frac{Qq}{a}$
Question:2.23
Answer:

Here, in the above image, point P is perpendicular to O and is at a distance of z from point O, which is the centre of the ring.
The charge dq is at a distance z from the point P.
Therefore, V can be written as:
$V=\frac{1}{4\pi \epsilon _{0}}\int \frac{dq}{r}=\frac{1}{4\pi \epsilon _{0}}\int \frac{dq}{\sqrt{z^{2}+a^{2}}}$
Therefore, the net potential will be :
$V=\frac{1}{4\pi \epsilon _{0}}\frac{Q}{\sqrt{z^{2}+a^{2}}}$
NCERT Exemplar Class 11 Physics Solutions Chapter 2: Long Answer
Question:2.24
Answer:
Let the field lines must be radically outward. Draw a cylindrical Gaussian surface of radius $r$ and length $l$. Then, applying Gauss' theorem
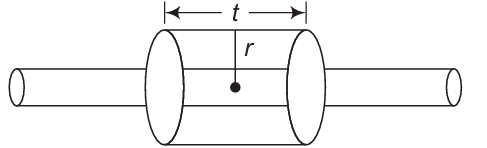
$
\begin{aligned}
& \int \mathrm{E} . \mathrm{dS}=\frac{1}{\varepsilon_0} \lambda l \\
& E_r 2 \pi r l=\frac{1}{\varepsilon_0} \lambda l \Rightarrow E_r=\frac{\lambda}{2 \pi \varepsilon_0 r}
\end{aligned}
$
Hence, if $r_0$ is the radius,
$
V(r)-V\left(r_0\right)=-\int_{r_0}^r \mathrm{E} \cdot \mathrm{dl}=\frac{\lambda}{2 \pi \varepsilon_0} \ln \frac{r_0}{r}
$
Since,
$
\int_{r_0}^r \frac{\lambda}{2 \pi \varepsilon_0 r} d r=\frac{\lambda}{2 \pi \varepsilon_0} \int_{r_0}^r \frac{1}{r} d r=\frac{\lambda}{2 \pi \varepsilon_0} \ln \frac{r}{r_0}
$
For a given $V$,
$
\Rightarrow \quad \begin{aligned}
r & =r_0 e^{-2 \pi \varepsilon_0 V r_0 / \lambda} e+2 \pi \varepsilon_0 V(r) / \lambda \\
r & =r_0 e^{-2 \pi \varepsilon_0\left[V_{(r)}-V_{\left(r_0\right)}\right] \lambda}
\end{aligned}
$
Question:2.25
Answer:

Here, the potential due to charges at the point P is
$V_{p}=\frac{1}{4\pi\varepsilon _{0}}\frac{q}{r_{1}}+\frac{1}{4\pi\varepsilon _{0}}\frac{(-q)}{r_{2}}$
The net electric potential at this point is zero,
Therefore, $r_{1}=r_{2}$
We know that
$r_{1}=\sqrt{\left ( \frac{x+d}{2} \right )^{2}+h^{2}}$
$r_{2}=\sqrt{\left ( \frac{x-d}{2} \right )^{2}+h^{2}}$
Solving the two equations, we the required equation in-plane x = 0 which is a y-z plane.
Question:2.26
Answer:
Here, the capacitors are connected in parallel. Therefore, the voltage across the capacitors is the same. Let us assume the final voltage is U. Here, C is the capacitance of the capacitor without the dielectric. At this point, the charge is $Q_{1}=CU$If the initial charge is $Q_{0}$ given by $Q_{0}=CU_{0}$
The conversion of charges is
$Q_{1}=Q_{1}+Q_{2}$
$CU_{0}=CU+\alpha CU_{2}$
$\alpha U_{2}+U-U_{o}=0$
Solving the equation, we get $U=6V$
Question:2.27
Answer:
Assuming initially the disc is in touch with the bottom plate, so the entire plate is a equipotential.
The electric field on the disc, when potential difference $V$ is applied across it, given by
$
E=\frac{V}{d}
$
Let charge $q$ ' is transferred to the disc during the process,
Therefore by Gauss' theorem,
$
\therefore \quad q^{\prime}=-\varepsilon_0 \frac{V}{d} \pi r^2
$
Since, Gauss theorem states that
$
\begin{aligned}
\phi & =\frac{q}{\varepsilon_0} \text { or } q=\frac{\varepsilon_0}{\phi} \\
& =\varepsilon E A=\frac{\varepsilon_0 V}{d} A
\end{aligned}
$
The force acting on the disc is
$
-\frac{V}{d} \times q^{\prime}=\varepsilon_0 \frac{V^2}{d^2} \pi r^2
$
If the disc is to be lifted, then
$
\varepsilon_0 \frac{V^2}{d^2} \pi r^2=m g \Rightarrow V=\sqrt{\frac{m g d^2}{\pi \varepsilon_0 r^2}}
$
This is the required expression.
Question:2.28
Answer:
This system is made up of three charges. The potential energy of the system is equal to the algebraic sum of PE of each pair. So,
$
\begin{aligned}
U & =\frac{1}{4 \pi \varepsilon_0}\left\{\frac{q_d q_d}{r}-\frac{q_u q_d}{r}-\frac{q_u q_d}{r}\right\} \\
& =\frac{9 \times 10^9}{10^{-15}}\left(1.6 \times 10^{-19}\right)^2\left[\left\{(1 / 3)^2-(2 / 3)(1 / 3)-(2 / 3)(1 / 3)\right\}\right] \\
& =2.304 \times 0^{-13}\left\{\frac{1}{9}-\frac{4}{9}\right\}=-7.68 \times 10^{-14} \mathrm{~J} \\
& =4.8 \times 10^5 \mathrm{eV}=0.48 \mathrm{meV}=5.11 \times 10^{-4}\left(m_n c^2\right)
\end{aligned}
$
Question:2.29
Answer:
Let us say, the charge stored on the first and second metal spheres before contact is $Q_{1}$ and $Q_{2}$ respectively.Therefore,
$Q_{1}=\sigma .4\pi R^2$
$Q_{2}=\sigma .4\pi (2R)^2=4\; Q_{1}$
Again assume, the charge stored on the first and second metal spheres are $Q{_{1}}^{'}$ and $Q{_{2}}^{'}$ respectively.
Therefore,
$\left ( Q{_{1}}^{'}+Q{_{2}}^{'} \right )=\left ( Q{_{1}}+Q{_{2}} \right )=5\; Q_{1}$
When the metal spheres are in contact, the following is the potentials acquired by them
$Q{_{1}}^{'} = \frac{Q{_{2}}^{'}}{2}$
By solving the above equations, we get,
$\sigma_1=5\frac{\sigma }{3}$
$\sigma_2=5\frac{\sigma }{6}$
Question:2.30
Answer:

When key $K_{1}$ is closed and key $K_{2}$ is open, then capacitors $C_{1}$ and $C_{2}$ are connected in series with the battery.
Therefore, the charge stored in the capacitors $C_{1}$ and $C_{2}$ will be same as $Q_{1}=Q_{2}$
Therefore, $Q_{1}=Q_{2}=q=\left ( \frac{C_{1}}{\left ( C_{1}+C_{2} \right )} \right )E=18\mu C$

Assuming only capacitors $C_{2}$ and $C_{3}$ are placed in parallel,
$C_{2}V'+ C_{3}V'=Q_{2}$
$V'=\frac{Q_{2}}{C_{2}+C_{3}}=3V$
Therefore,
$Q{_{2}}^{'}=3CV'=9\; \mu C$
$Q{_{3}}=3CV'= 9\mu C$
$Q{_{1}}^{'}=18\; \mu C$
Question:2.31
Answer:

In the above figure, we can see that the disc is divided into several charged rings. Let P be the point on the axis of the disc at a distance x from the centre of the disc.
The radius of the ring is r and the width is dr. dq is the charge on the ring which is given as
$dq=\sigma dA=\sigma 2\pi rdr$
The potential is given as
$dV=\frac{1}{4\pi\varepsilon _{0}}\frac{dq}{\sqrt{r^{2}+x^{2}}}=\frac{1}{4\pi \varepsilon _{0}}\frac{\left ( \sigma 2\pi rdr \right )}{\sqrt{r^{2}+x^{2}}}$
The total potential at P is given as
$\frac{Q}{2\pi \varepsilon _{0}R^2}\left ( \sqrt{R_{2}+x_{2}-x} \right )$
Question:2.32
Answer:

We know that the potential at point P is $V=\sum Vi$
Where $Vi=\frac{qi}{4\pi \varepsilon _{0}}$, ri is the magnitude of the position vector P
$V=\frac{1}{4 \pi \varepsilon _{0}}\sum \frac{qi}{rpi}$
When the (x,y,z) plane is considered, the two charges lie on the z-axis and are separated by 2d. The potential is given as
$\frac{q_{1}}{\sqrt{x^{2}+y^{2}+\left ( z-d \right )^{2}}}+\frac{q_{2}}{\sqrt{x^{2}+y^{2}+\left ( z+d \right )^{2}}}=0$
Squaring the equation, we get
$x^{2}+y^{2}+z^{2}+\left [ \left ( \frac{q_{1}^2+q_2^2}{q_{1}^2-q_2^2} \right )^2 \right ]\left ( 2zd \right )+d^2=0$
The Centre of the sphere is
$\left ( 0,0,-d\left [ \frac{q_{1}^{2}+q_{2}^{2}}{q_{1}^{2}-q_{2}^{2}} \right ] \right )$
And radius is
$r=\frac{2q_{1}q_{2}d}{q_{1}^{2}-q_{2}^{2}}$
Question:2.33
Answer:
In the above figure, $+q$ charge is at a point away from O towards $\left ( -d,0 \right )$.
This can be written as
$U=q\left ( V_{1}+V_{2} \right )=q\frac{1}{4\pi\; \varepsilon _{0}}[\frac{-q}{\left ( d-x \right )}+\frac{-q}{d+x}]$
$U=\frac{1}{2\pi\varepsilon _{0}}\frac{-q^{2}d}{d^{2}-x^{2}}$
At x=0;
$U=\frac{1}{2\pi\varepsilon _{0}}\frac{q^{2}}{d}$
If we differentiate both sides of the equation with respect to x, we get
$\frac{dU}{dx}>0,$ when $x<0$
and $\frac{dU}{dx}<0,$ when $x>0$
With the help of this two, we can assume that the charge on the particle to be
$F=\frac{-dU}{dx}$
Hence, $F=\frac{-dU}{dx}=0$
When
a) $\frac{d^2U}{dx^{2}}$ = positive, equilibrium is stable
b) $\frac{d^2U}{dx^{2}}$ = negative, equilibrium is unstable
c) $\frac{d^2U}{dx^{2}}=0$, equilibrium is neutral
Therefore, when
$x=0,\frac{d^2U}{dx^{2}}=\left ( \frac{-2dq2}{4\pi\varepsilon _{0}} \right )\left ( \frac{1}{d^{6}} \right )\left ( 2d^2 \right )<0$
Which shows that the system is an unstable equilibrium.
Main Topics of NCERT Exemplar Class 12 Physics Solutions Chapter 2
- Introduction
- Electrostatic Potential
- Potential due to a point charge
- Electric Potential Of A Dipole And System Of Charges
- Equipotential Surfaces
- Relation Between Field And Potential
- Potential Energy Of A System Of Charges
- Potential Energy In An External Field
- Electrostatics Of Conductors
- Dielectrics And Polarization
- Capacitors And Capacitance
- The Parallel Plate Capacitor
- Effect Of Dielectrics On Capacitance
- Combination Of Capacitors
- Energy Stored In A Capacitor
NCERT Exemplar Class 12 Physics Solutions Chapter-Wise
NCERT Exemplar Class 12 Solutions
- NCERT Exemplar Class for 12 Physics
- NCERT Exemplar Class 12 Chemistry Solutions
- NCERT Exemplar Class 12 Mathematics Solutions
- NCERT Exemplar Class 12 Biology Solutions
Check Class 12 Physics Chapter-wise Solutions
Also, Read NCERT Solution subject-wise
- NCERT Solution for Class 12 Physics
- NCERT Solution for Class 12 Chemistry
- NCERT Solution for Class 12 Maths
- NCERT Solution for Class 12 Biology
Must read NCERT Notes subject-wise
- NCERT Notes for Class 12 Physics
- NCERT Notes for Class 12 Chemistry
- NCERT Notes for Class 12 Maths
- NCERT Notes for Class 12 Biology
Also, Check NCERT Books and NCERT Syllabus
Frequently Asked Questions (FAQs)
All these questions given in the chapter are solved exhaustively, with proper explanation, steps, diagrams and conclusion.
In all 33 questions from main exercise is solved in detail in the NCERT exemplar Class 12 Physics solutions chapter 2 Electrostatic Potential and Capacitance
Our very own highly experienced teachers of physics have solved these questions as per the CBSE pattern
Questions related to CBSE Class 12th
On Question asked by student community
Hello,
The date of 12 exam is depends on which board you belongs to . You should check the exact date of your exam by visiting the official website of your respective board.
Hope this information is useful to you.
Hello,
Class 12 biology questions papers 2023-2025 are available on cbseacademic.nic.in , and other educational website. You can download PDFs of questions papers with solution for practice. For state boards, visit the official board site or trusted education portal.
Hope this information is useful to you.
Hello Pruthvi,
Taking a drop year to reappear for the Karnataka Common Entrance Test (KCET) is a well-defined process. As a repeater, you are fully eligible to take the exam again to improve your score and secure a better rank for admissions.
The main procedure involves submitting a new application for the KCET through the official Karnataka Examinations Authority (KEA) website when registrations open for the next academic session. You must pay the required application fee and complete all formalities just like any other candidate. A significant advantage for you is that you do not need to retake your 12th board exams. Your previously secured board marks in the qualifying subjects will be used again. Your new KCET rank will be calculated by combining these existing board marks with your new score from the KCET exam. Therefore, your entire focus during this year should be on preparing thoroughly for the KCET to achieve a higher score.
For more details about the KCET Exam preparation,
CLICK HERE.
I hope this answer helps you. If you have more queries, feel free to share your questions with us, and we will be happy to assist you.
Thank you, and I wish you all the best in your bright future.
Yes, you can switch from Science in Karnataka State Board to Commerce in CBSE for 12th. You will need a Transfer Certificate from your current school and meet the CBSE school’s admission requirements. Since you haven’t studied Commerce subjects like Accountancy, Economics, and Business Studies, you may need to catch up before or during 12th. Not all CBSE schools accept direct admission to 12th from another board, so some may ask you to join Class 11 first. Make sure to check the school’s rules and plan your subject preparation.
Hello
For the 12th CBSE Hindi Medium board exam, important questions usually come from core chapters like “Madhushala”, “Jhansi ki Rani”, and “Bharat ki Khoj”.
Questions often include essay writing, letter writing, and comprehension passages. Grammar topics like Tenses, Voice Change, and Direct-Indirect Speech are frequently asked.
Students should practice poetry questions on themes and meanings. Important questions also cover summary writing and translation from Hindi to English or vice versa.
Previous years’ question papers help identify commonly asked questions.
Focus on writing practice to improve handwriting and presentation. Time management during exams is key to answering all questions effectively.
Popular CBSE Class 12th Questions
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters








