NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei
CBSE Class 12th Exam Date:17 Feb' 26 - 17 Feb' 26
Have you ever asked yourself how the stars manage to generate such a great amount of energy, or how nuclear power stations manage to produce electricity? The solution is in the minute nucleus of atoms, which, in spite of being almost a thousand times smaller than the atom itself, contains nearly all of its weight. Class 12 Physics Chapter 13 Nuclei presents the students with the fascinating field of nuclear physics, which includes such topics as atomic masses, nuclear binding energy, nuclear forces, radioactivity, and nuclear energy, which is fundamental to the field in both theory and practice.
The National Testing Agency (NTA) has announced that the registration window for JEE Main 2026 will open in October 2025. The exam is the first stage in the IIT admission process, where only around 33% of qualified candidates secure a seat. Candidates will have to submit the application form through the official website jeemain.nta.nic.in.
This Story also Contains
- NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei: Download PDF
- NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei: Exercise Questions
- NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei: Additional Questions
- NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei: Higher Order Thinking Skills (HOTS) Questions
- Nuclei NCERT Solutions: Topics
- Class 12 Physics Chapter 13 - Nuclei: Important Formulas
- Approach to Solve Questions of Class 12 Physics Chapter 13 - Nuclei
- What Extra Should Students Study Beyond NCERT for JEE/NEET?
- NCERT Solutions for Class 12 Physics Chapter-wise

NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei are specifically developed by the experts of the subject to explain all the exercises given in the textbook, the other practice questions, and the HOTS problems step by step. These NCERT solutions have made complex nuclear concepts simple to understand, and at the same time, derivations and solving of numerical problems are easy to understand. These NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei are available in free PDF and are highly useful in the preparation of the board exams of Class 12 of the CBSE, and a powerful resource for JEE and NEET candidates. Through these solutions, students will be able to improve their fundamentals, gain accuracy, and gain confidence in the exam.
NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei: Download PDF
The Class 12 Physics Chapter 13 - Nuclei Solutions presented the answers to all the questions with step-by-step explanations. These solutions will make the students grasp tricky topics without difficulty and can be used both in the CBSE board exams as well as JEE and NEET exams. The PDF version can also be downloaded so that you can have an easy offline reference and a quick revision anytime.
NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei: Exercise Questions
Class 12 Physics Chapter 12 - Nuclei question answers (Exercise questions) offer step-by-step answers to all textbook questions, covering topics like nuclear structure, binding energy, and radioactivity. These solutions help students strengthen concepts, practice effectively, and prepare for board exams as well as competitive exams like JEE and NEET.
13.1 Obtain the binding energy( in MeV ) of a nitrogen nucleus
Answer:
Atomic mass of Nitrogen
Mass defect
Now 1u is equivalent to 931.5 MeV
Therefore binding energy of a Nitrogen nucleus is 101.01186 MeV
13.2 (i) Obtain the binding energy of the nuclei
Answer:
Atomic mass of
Mass defect
Now 1 u is equivalent to 931.5 MeV
Therefore binding energy of a
Average binding energy
13.2 (ii) Obtain the binding energy of the nuclei
Answer:
Atomic mass of
Mass defect
Now 1u is equivalent to 931.5 MeV
Therefore binding energy of a
Average binding energy
Answer:
Mass of the coin is
Total number of Cu atoms in the coin is n
Atomic mass of
Mass defect
Now 1u is equivalent to 931.5 MeV
Therefore binding energy of a
The nuclear energy that would be required to separate all the neutrons and protons from each other is
13.4 Obtain approximately the ratio of the nuclear radii of the gold isotope
Answer:
The nuclear radii are directly proportional to the cube root of the mass number.
The ratio of the radii of the given isotopes is
Answer:
The above negative value of mass defect implies there will be a negative Q value, and therefore the reaction is endothermic
Answer:
The above positive value of mass defect implies the Q value would be positive and therefore the reaction is exothermic.
Answer:
The reaction will be
The mass defect of the reaction will be
Since the mass defect is negative, the Q value will also be negative, and therefore, the fission is not energetically possible.
Answer:
Number of atoms present in
Energy per fission
Total Energy released if all the atoms in
Answer:
The energy liberated on the fusion of two atoms of deuterium is 3.27 MeV
Number of fusion reactions in 2 kg of deuterium = N A
The energy liberated by the fusion of 2.0 kg of deuterium atoms E
Power of lamp (P)= 100 W
The time for which the lamp would glow using E amount of energy is
=4.99
Answer:
For a head-on collision of two deuterons, the closest distances between their centres will be
charge on each deuteron
The maximum electrostatic potential energy of the system during the head-on collision will be E
The above basically means that bringing two deuterons from infinity to each other would require 360 keV of work to be done or would require 360 keV of energy to be spent.
Answer:
The mass of an element with mass number A will be about A u. The density of its nucleus, therefore, would be
As we can see, the above density comes out to be independent of mass number
NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei: Additional Questions
Nuclei class 12 question answers (Additional Questions) are designed to enhance conceptual clarity and problem-solving skills beyond the NCERT textbook. These questions cover nuclear forces, energy, and decay processes, making them highly useful for board exams and entrance tests like JEE/NEET.
Answer:
Mass of the two stable isotopes and their respective abundances are
m=6.940934 u
Answer:
The atomic mass of boron is 10.811 u
Mass of the two stable isotopes are
Let the two isotopes have abundances
Therefore the abundance of
Answer:
The atomic masses of the three isotopes are
Their respective abundances are
The average atomic mass of neon is 20.1771 u .
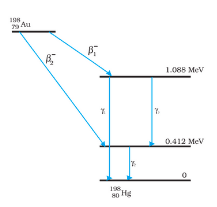
3. (i) Write nuclear reaction equations for
Answer:
The nuclear reaction equations for the given alpha decay
3. (ii) Write nuclear reaction equations for
Answer:
The nuclear reaction equations for the given alpha decay is
3. (iii) Write nuclear reaction equations for
Answer:
The nuclear reaction equations for the given beta minus decay is
3.(iv) Write nuclear reaction equations for
Answer:
The nuclear reaction equation for the given beta minus decay is
3.(v) Write nuclear reaction equations for
Answer:
The nuclear reaction for the given beta plus decay will be
Electron capture of
Answer:
The nuclear reaction for electron capture of
Answer:
(a) The activity is proportional to the number of radioactive isotopes present
The number of half years in which the number of radioactive isotopes reduces to x% of its original value is n.
In this case
It will take 5T years to reach 3.125% of the original activity.
(b) In this case
It will take 6.64T years to reach 1% of the original activity.
Answer:
Since we know that activity is proportional to the number of radioactive isotopes present in the sample.
Also
but
Therefore
The age of the Indus-Valley civilisation, calculated using the given specimen, is approximately 4217 years.
Answer:
Required activity
Mass of those many atoms of Cu will be
7. The half-life of
Answer:
The number of atoms in 15 mg of
The disintegration rate will be
The disintegration rate is therefore
8.(a) Find the Q-value and the kinetic energy of the emitted
Answer:
Mass defect is
By using Linear Momentum Conservation and Energy Conservation
Kinetic energy of alpha particle =
8.(b) Find the Q-value and the kinetic energy of the emitted
Answer:
Mass defect is
By using Linear Momentum Conservation and Energy Conservation
Kinetic energy of alpha particle =
9. The radionuclide
The maximum energy of the emitted positron is
calculate Q and compare it with the maximum energy of the positron emitted.
Answer:
If we use atomic masses
Q-value= 0.001033
Answer:
The
(we did not subtract the mass of the electron, as it is cancelled because of the presence of one more electron in the sodium atom)
Q=0.004696
Q=4.3743 eV
The emitted nucleus is way heavier than the
Answer:
The amount of energy liberated on fission of 1
The amount of energy liberated on fission of 1g
Total Energy produced in the reactor in 5 years
Mass of
=1537.8 kg
The amount present initially in the reactor = 2m
=2
=3075.6 kg
Answer:
For the electron capture, the reaction would be
The mass defect and q value of the above reaction would be
where m N
For positron emission, the reaction would be
The mass defect and q value for the above reaction would be
From the above values, we can see that if Q2 is positive, Q1 will also be positive but Q1 being positive does not imply that Q2 will also be positive.
Answer:
Let the abundances of
x+y+78.99=100
y=21.01-x
The average atomic mass of Mg is 24.312 u
The abundances of
Answer:
The reaction showing the neutron separation is
But 1u=931.5 MeV/
Therefore E=(0.008978)
E=8.363007 MeV
Therefore to remove a neutron from the
Answer:
The reaction showing the neutron separation is
But 1u=931.5 MeV/
Therefore E=(0.014019)
E=13.059 MeV
Therefore to remove a neutron from the
Answer:
Let initially there be
Let after time
Dividing equation (ii) by (i) and taking the natural
where
t comes out to be 208.5 days
Calculate the Q-values for these decays and determine that both are energetically allowed.
Answer:
1 u = 931.5 MeV/
Q=0.03419
=31.848 MeV
As the Q value is positive, the reaction is energetically allowed
1 u = 931.5 MeV/
Q=0.00642
=5.98 MeV
As the Q value is positive, the reaction is energetically allowed
Answer:
The fission reaction given in the question can be written as
The mass defect for the above reaction would be
In the above equation, mN represents nuclear masses
but 1u =931.5 MeV/
Q=0.247995
Q=231.007 MeV
Q value of the fission process is 231.007 MeV
18. (a)Consider the D–T reaction (deuterium-tritium fusion)
Answer:
The mass defect of the reaction is
1u = 931.5 MeV/
Q=0.018883
18. (b) Consider the D–T reaction (deuterium-tritium fusion)
Answer:
To initiate the reaction, both nuclei would have to come into contact with each other.
Just before the reaction, the distance between their centres would be 4.0 fm.
The electrostatic potential energy of the system at that point would be
The same amount of Kinetic Energy, K, would be required to overcome the electrostatic forces of repulsion to initiate the reaction
It is given that
Therefore, the temperature required to initiate the reaction is
Answer:
Frequency of
Planck's constant, h=6.62
Similarly, we can calculate the frequencies of
The energy of the highest level would be equal to the energy released after the decay
Mass defect is
We know 1u = 931.5 MeV/
Q value= 0.001473
The maximum Kinetic energy of
The maximum Kinetic energy of
Answer:
(a)
The above fusion reaction releases the energy of 26 MeV
The number of Hydrogen atoms in 1.0 kg of Hydrogen is
Therefore,
The energy released in the whole process is
(b) The energy released in the fission of one
The number of
The energy released on the fission of N atoms is
Answer:
Let the amount of energy produced using nuclear power per year in 2020 be E
(Only 10% of the required electrical energy is to be produced by Nuclear power, and only 25% of therm-nuclear is successfully converted into electrical energy)
The amount of Uranium required to produce this much energy is M
=3.076
NCERT Solutions for Class 12 Physics Chapter 13 - Nuclei: Higher Order Thinking Skills (HOTS) Questions
Class 12 Physics Chapter 13 - Nuclei: Higher Order Thinking Skills (HOTS) Questions encourage students to apply concepts like nuclear binding energy, radioactivity, and nuclear reactions in complex scenarios. These advanced problems help in developing analytical skills and are very useful for competitive exam preparation.
Q1:
The energy released in the fusion of
(Consider the fusion reaction as
Answer:
In each fusion reaction,
Energy released per Nuclei of
So,
Q2:
The disintegration energy
Given atomic masses of
Value of
Answer:
Disintegration energy
Q3:
The half-life of a radioactive isotope is 5.5 h. If there are initially
Answer:
Use,
Q4:
The
Answer:
From Moseley's law for
Q5:
The count rate from
Answer:
Initial count rate (C R) for
After 3 half -lives, langle
If
Nuclei NCERT Solutions: Topics
Class 12 Physics Chapter 13 - Nuclei deals with the study of the atomic nucleus, its properties, and the forces that hold it together. The topics cover atomic masses, binding energy, nuclear force, radioactivity, and nuclear energy, which are crucial for understanding both natural processes and modern technology.
- Atomic Masses And Composition Of Nucleus
- Size Of The Nucleus
- Mass-Energy And Nuclear Binding Energy
- Nuclear Force
- Radioactivity
- Nuclear Energy
Class 12 Physics Chapter 13 - Nuclei: Important Formulas
Class 12 Physics Chapter 13 - Nuclei formulas include key equations related to nuclear radius, binding energy, decay laws, half-life, and activity. These formulas are essential for solving numerical problems in board exams as well as JEE and NEET.
1. Atomic Masses and Composition of Nucleus
- Nucleus
- Atomic number (Z), Mass number (A), Neutrons = A - Z
- 1 atomic mass unit
- Isotopes: Same Z, different A
2. Size of the Nucleus
- Radius:
- Nuclear density is constant and very high (
3. Mass-Energy and Binding Energy
- Mass defect: Actual mass < sum of nucleons
- Binding energy = energy needed to break the nucleus
- Higher binding energy = more stable nucleus
4. Nuclear Force
- Strong, short-range, attractive force
- Acts between all nucleons
- Charge-independent and repulsive at very short distances
5. Radioactivity
- Spontaneous decay of unstable nuclei
- Types: Alpha (He nucleus), Beta (electron/positron), Gamma (photons)
- Law:
- Half-life:
6. Nuclear Energy
- Fission: Heavy nucleus splits (used in reactors)
- Fusion: Light nuclei combine (in stars)
- Both release huge energy due to binding energy changes
Approach to Solve Questions of Class 12 Physics Chapter 13 - Nuclei
In answering the questions of this chapter, attention should be drawn to developing the clarity of the stated problem, determining the correct physical principles, and systematically using formulas. Students are advised to be keen on derivations, units, and methods of approximation commonly examined in examinations. An organised approach to solving problems is not only time saving, but also minimises mistakes made when taking board as well as competitive exams, such as JEE as well as NEET.
-
Understand fundamental nuclear terms:
-
Atomic number (Z), Mass number (A), Neutrons (N = A – Z)
-
Nucleus structure: Protons and neutrons (nucleons)
- Understand the nuclear size formula:
Radius of nucleus: - Apply mass-energy equivalence:
E=mc2, where mass difference is utilised to generate energy - Calculate binding energy:
- Binding Energy (B.E) =Δm×931MeV
- Δm= mass defect = (total mass of nucleons – nucleus mass)
- Understand the stability of nuclei:
- More B.E per nucleon ⇒ stable nucleus
- See the graph for binding energy per nucleon versus mass number
- Learn about types of radioactive decay:
- Alpha decay: Mass number goes down by 4, atomic number goes down by 2
- Beta decay: Neutron → Proton (or vice versa), atomic number ±1
- Gamma decay: No change of mass or atomic number, only release of energy.
- Apply the radioactive decay law:
- Half-life:
- Activity:
- Use appropriate units and convert them:
Energy in MeV or joules, mass in u, time in seconds - Learn nuclear reactions:
Balance the equations so that they retain mass number and atomic number. - Practice numerical + conceptual questions:
Emphasise half-life, decay, reaction equations, and energy calculations
What Extra Should Students Study Beyond NCERT for JEE/NEET?
Beyond the NCERT, students preparing for JEE/NEET should focus on advanced concepts of the Nuclei chapter, such as mass defect and binding energy curve analysis, nuclear reactions (fission & fusion), Q-value calculations, and detailed radioactive decay series. These topics strengthen problem-solving ability and are frequently tested in competitive exams.
NCERT Solutions for Class 12 Physics Chapter-wise
The NCERT Solutions for Class 12 Physics provide step-by-step, detailed answers to all chapters, helping students strengthen their conceptual understanding and problem-solving skills. These chapter-wise solutions are designed as per the latest CBSE curriculum and are equally useful for JEE, NEET, and other competitive exams. With structured explanations and solved examples, these solutions make learning easier and revision more effective.
Also Check:
- NCERT Books Class 12 Physics
- NCERT Syllabus Class 12 Physics
- NCERT Books Class 12
- NCERT Syllabus Class 12
NCERT solutions subject-wise
- NCERT solutions for class 12 mathematics
- NCERT solutions for class 12 chemistry
- NCERT solutions for class 12 physics
- NCERT solutions for class 12 biology
Also, check NCERT Exemplar Class 12 Solutions
Frequently Asked Questions (FAQs)
Yes the NCERT chapter Nuclei are important for both the exams. Both in NEET and JEE main syllabus the chapter Nuclei is present and 1 or 2 questions from the chapter can be expected for the exams. The questions discussed in the NCERT Solutions for the chapter Nuclei will give a better idea on how to use the formulas and give a better understanding of the concepts discussed.
NCERT solutions are important for the Board exam as they provide clear explanations, help in solving questions, cover all important topics, provide a structured approach to solving problems, and are designed with the exam pattern in mind, helping in exam-oriented preparation.
The nucleus is made up of protons, which are positively charged particles, and neutrons, which are neutral particles.
Isotopes are atoms of the same element that have the same number of protons but different numbers of neutrons in their nuclei.
For CBSE board exam from NCERT class, 12 chapters 13 around 4 to 6 marks questions can be expected. All topics of the NCERT syllabus for the chapter Nuclei should be covered for the CBSE board exam.
Questions related to CBSE Class 12th
On Question asked by student community
Hello,
The date of 12 exam is depends on which board you belongs to . You should check the exact date of your exam by visiting the official website of your respective board.
Hope this information is useful to you.
Hello,
Class 12 biology questions papers 2023-2025 are available on cbseacademic.nic.in , and other educational website. You can download PDFs of questions papers with solution for practice. For state boards, visit the official board site or trusted education portal.
Hope this information is useful to you.
Hello Pruthvi,
Taking a drop year to reappear for the Karnataka Common Entrance Test (KCET) is a well-defined process. As a repeater, you are fully eligible to take the exam again to improve your score and secure a better rank for admissions.
The main procedure involves submitting a new application for the KCET through the official Karnataka Examinations Authority (KEA) website when registrations open for the next academic session. You must pay the required application fee and complete all formalities just like any other candidate. A significant advantage for you is that you do not need to retake your 12th board exams. Your previously secured board marks in the qualifying subjects will be used again. Your new KCET rank will be calculated by combining these existing board marks with your new score from the KCET exam. Therefore, your entire focus during this year should be on preparing thoroughly for the KCET to achieve a higher score.
For more details about the KCET Exam preparation,
CLICK HERE.
I hope this answer helps you. If you have more queries, feel free to share your questions with us, and we will be happy to assist you.
Thank you, and I wish you all the best in your bright future.
Yes, you can switch from Science in Karnataka State Board to Commerce in CBSE for 12th. You will need a Transfer Certificate from your current school and meet the CBSE school’s admission requirements. Since you haven’t studied Commerce subjects like Accountancy, Economics, and Business Studies, you may need to catch up before or during 12th. Not all CBSE schools accept direct admission to 12th from another board, so some may ask you to join Class 11 first. Make sure to check the school’s rules and plan your subject preparation.
Hello
For the 12th CBSE Hindi Medium board exam, important questions usually come from core chapters like “Madhushala”, “Jhansi ki Rani”, and “Bharat ki Khoj”.
Questions often include essay writing, letter writing, and comprehension passages. Grammar topics like Tenses, Voice Change, and Direct-Indirect Speech are frequently asked.
Students should practice poetry questions on themes and meanings. Important questions also cover summary writing and translation from Hindi to English or vice versa.
Previous years’ question papers help identify commonly asked questions.
Focus on writing practice to improve handwriting and presentation. Time management during exams is key to answering all questions effectively.
Popular CBSE Class 12th Questions
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters