NCERT Books and the NCERT Syllabus for Class 12 form the foundation of board exam preparation and competitive exam readiness. Easy access to these links helps students follow the latest curriculum, understand topic-wise weightage, and prepare systematically for CBSE board examinations.
NCERT Solutions for Class 12 Physics Chapter 10 - Wave Optics
Have you ever observed the coloured designs on the bubbles of soap, the diffusing of light about sharp corners, or the light and shade edges in experiments such as the Double Slit by Young? The wave nature of light, which is a topic of Class 12 Physics Chapter 10 - Wave Optics, explains these interesting phenomena. The key topics that have been addressed in this chapter include interference, diffraction and polarisation, which assist students in learning the behaviour of light as a wave.
LiveJEE Mains 2026 Result LIVE: NTA final answer key soon at jeemain.nta.nic.in; score normalisation methodFeb 11, 2026 | 1:24 AM ISTRead More
As per the NTA JEE Main 2026 schedule, session 1 results for both paper 1 and 2 will be declared by February 12 along with the final answer key.
This Story also Contains
- NCERT Solutions for Class 12 Physics Chapter 10: Download PDF
- Class 12 Physics Chapter 10 Wave Optics: Exercise Questions
- Wave Optics Class 12 NCERT Solutions: Additional Questions: Additional questions
- Class 12 Physics NCERT Chapter 10: Higher Order Thinking Skills (HOTS) Questions
- NCERT Solutions for Class 12 Physics Chapter 10 Wave Optics: Topics
- NCERT Solutions for Class 12 Physics Chapter 10 Wave Optics: Important Formulas
- How Can NCERT Solutions for Class 12 Physics Chapter 10 Wave Optics Help in Exam Preparation?
- Approach to Solve Questions of Wave Optics Class 12
- Importance of NCERT Solutions for Class 12 Physics Chapter 10: Wave Optics
- What Extra Should Students Study Beyond NCERT for JEE/NEET?
- NCERT Solutions for Class 12 Physics: Chapter-Wise
- NCERT Books and NCERT Syllabus
- NCERT Solutions Subject-wise
- NCERT Exemplar Class 12 Solutions
NCERT Solutions for Class 12 Physics Chapter 10 - Wave Optics are prepared by experienced subject experts according to the latest CBSE Class 12 Physics syllabus. These NCERT Solutions for Class 12 Physics Chapter 10 Wave Optics will give step-by-step answers to every question in the NCERT textbooks and can be used to help students to develop good conceptual knowledge and acquire better number problem-solving skills. These well-organised NCERT solutions prove very handy in the preparation of the CBSE Class 12 board exam and also competitive exams such as JEE and NEET, where Wave optics is also a significant and frequently asked chapter.
NCERT Solutions for Class 12 Physics Chapter 10: Download PDF
The NCERT Solutions to Class 12 Physics Chapter 10 Wave Optics are a great way to make the learning process much simpler, as the chapter provides explanations of concepts such as interference, diffraction, polarisation, and the principle of Huygens. The NCERT Solutions for Class 12 Physics Chapter 10 PDF can be downloaded and used to revise quickly and prepare the solved exercise questions before the exams and competitive tests like JEE and NEET.
Class 12 Physics Chapter 10 Wave Optics: Exercise Questions
Wave Optics Class 12 NCERT exercise questions cover important topics like interference, diffraction, and polarisation. These concepts help explain how light behaves as a wave. Below are the detailed NCERT solutions of Class 12 Physics NCERT Chapter 10 Wave Optics to help you understand and solve these questions easily.
Answer:
Given a monochromatic light has a wavelength of $589 nm\ or\ 589\times 10^{-9}m$.
And as we know, the speed of light in air is $c = 3\times 10^{8} m/s$.
Refractive index of water, $\mu = 1.33$
Therefore, we have now,
The ray is incident on the medium, and then it gets reflected back to the same medium; therefore, the wavelength, speed, and frequency will be the same as those of the incident ray.
We have the frequency of light given by the relation,
$v = \frac{c}{\lambda}$
$= \frac{3\times 10^8 m/s}{589\times 10^{-9}}$
$= 5.09\times 10^{14}Hertz$ $
Therefore, the speed, frequency, and wavelength of the reflected light are $3\times 10^{8} m/s$, $5.09\times 10^{14}Hertz$, and $589 nm$ respectively.
Answer:
Given,
The wavelength of the monochromatic light $\lambda =589nm=589*10^{-9}m$
Refractive index of the water $\mu_{water}=1.33$
b)In the case of refraction, speed and wavelength will change as the medium is changing; however, the frequency will remain the same because it doesn't change when the medium is changed,
so, frequency $f=5.09 * 10^{14}Hz$
Speed of rays: speed of rays in water with refractive index 1.33 is
$v=\frac{c}{\mu _{water}}=\frac{3*10^8}{1.33}=2.26*10^8m/s$
Now, the Wavelength of light
$\lambda =\frac{v}{f}=\frac{2.26*10^8}{5.09*10^{14}}=444.01*10^{-9}m$
Hence, the wavelength of light is 444.01nm, frequency is $5.09 * 10^{14}Hz$, and speed is $2.26*10^8m/s$.
Answer:
The shape of the wavefront when light is diverging from a point source is Spherical since light travels in all directions.
Answer:
The shape of light emerging out of a convex lens when a point source is placed at its focus is Parallel .when light rays come from infinity parallelly, they intersect at focus of convex lens and hence when light is emerging from the focus, the rays will get parallel to each other after coming out of the convex lens, because path of light rays are reversible.
Answer:
The portion of the wavefront of the light from the distant star which is seen from Earth is a plane since a small area of a large sphere will nearly look like a plane.
Answer:
Given,
Refractive index of the glass $\mu _{glass}$ = 1.5
Speed of light in vacuum $c=3*10^8m/s$
Now,
As we know,
Refractive index of a medium
$\mu _{medium }=\frac{c}{v_{medium}}$ where ${v_{medium}}$ is the speed of light in that medium.
So from here,
${v_{glass}}=\frac{c}{\mu _{glass}}=\frac{3*10^8}{1.5}=2*10^8m/s$
Hence, the speed of light in water is $2*10^8m/s$
Answer:
No, the speed of light in glass is not independent of the colour. The colour of the light does influence the refractive index and speed of light in the medium. The refractive index of the violet light is greater than the refractive index of red light, and hence the red component of the white light travels faster in the glass than the red component of the light.
as
$v=\frac{c}{\mu }$
The more the refractive index, the slower the speed.
Answer:
Given,
Distance between screen and slit $D=1.4m$
Distance between slits $d=0.28mm=0.28*10^{-3}m$
Distance between central and fourth bright fringe $u=1.2cm=1.2*10^{-2}m$
Now,
As we know, the distance between two fringes in constructive interference is given by
$u=n\lambda \frac{D}{d}$
where $n=$ order of fringe (which is 4 here) and $\lambda$ is the wavelength of light we are using.
So from here,
$\lambda = \frac{ud}{nD}=\frac{1.2*10^{-2}*0.28*10^{-3}}{4*1.4}=6*10^{-7}m$
Hence wavelength os the light is 600nm
Q10.5 In Young’s double-slit experiment using monochromatic light of wavelength $\lambda$, the intensity of light at a point on the screen where the path difference is $\lambda$ is K units. What is the intensity of light at a point where the path difference is $\frac{\lambda}{3}$?
Answer:
Given in Young's double-slit experiment.
the wavelength of monochromatic light = $\lambda$
The intensity of light when the path difference is $\lambda$ = K
Now,
As we know,
The phase difference $\phi$ is given by
$\phi =\frac{2\pi }{\lambda }(PathDifference)$
also
Total Intensity
$I=I_1+I_2+2\sqrt{I_1I_2}cos\phi$
Let $I_1=I_2=I_0$
Now, when the path difference is $\lambda$
the phase difference angle
$\phi=\frac{2\pi }{\lambda}*\lambda=2\pi$
so,
$I_0+I_0+2\sqrt{I_0I_0}cos2\pi=K$
$K=4I_0$
Now, when path difference is $\frac{\lambda }{3}$
$\phi=\frac{2\pi }{\lambda}*\frac{\lambda}{3}=\frac{2\pi}{3}$
Intensity of light
$K'=I_0+I_0+2\sqrt{I_0I_0}cos\frac{2\pi}{3}$
$K'=I_0$
Now, comparing the intensity in both cases
$\frac{K'}{K}=\frac{I_0}{4I_0}=\frac{1}{4}$
$K'=\frac{K}{4}$
Hence intensity will reduce to one-fourth of the initial when the path difference changes from $\lambda$ to $\frac{\lambda}{3}$.
Q10.6(a) A beam of light consisting of two wavelengths, 650 nm and 520 nm, is used to obtain interference fringes in a Young’s double-slit experiment. Find the distance of the third bright fringe on the screen from the central maximum for a wavelength of 650 nm.
Answer:
Given,
The wavelength of one light beam :
$\lambda_1=650nm=650*10^{-9}m$
The wavelength of another Light beam
$\lambda_2=520nm=520*10^{-9}m$
Let the distance between the two-slit be $d$, and the distance between the slit and the screen be $D$
Now,
As we know, the distance $x$ of the nth bright fringe from the central maxima is given by
$x=n\lambda \frac{D}{d}$
so for 3rd fringe,
$n=3$
$x=n\lambda_1 \frac{D}{d}=3*650*10^{-9}*\frac{D}{d}=1950\frac{D}{d}nm$
Hence distance of the 3rd fringe from the central maxima is $1950\frac{D}{d}nm$. Here, values D and d are not given in the question.
Answer:
Let the nth bright fringe due to wavelength $\lambda_2$ and the (n − 1) th bright fringe due to wavelength $\lambda_1$ coincide on the screen. We can equate the conditions for bright fringes as:
$n\lambda_2=(n-1)\lambda _1$
$520n=650n-650$
$650=130n$
$n=5$
Hence, the least distance from the central maximum can be obtained by the relation:
$X=n\frac{\lambda_2D}{d}=5*\frac{520*10^{-9}*D}{d}$
Hence, we can find out X if D and d are given.
Wave Optics Class 12 NCERT Solutions: Additional Questions: Additional questions
Wave Optics Class 12 NCERT Solutions: Additional Questions include extra practice problems to boost your understanding. These questions are helpful for board exam revision and competitive exams like JEE and NEET.
Answer:
Given
The angular width of the fringe when the medium is air
$\theta _{air}=0.2^0$
The distance of the screen from the slit is $D = 1m$
The wavelength of light we are using $\lambda=600nm=600*10^{-9}m$
Refractive index of water $\mu_{water}=4/3$
let the angular width of the fringe when the medium is water $\theta _{water}$
Now, as we know, the angular width is given by
$\theta =\frac{\lambda }{d}$
so,
$d=\frac{\lambda _{air}}{\theta _{air}}=\frac{\lambda _{water}}{\theta _{water}}$
$d=\frac{\lambda _{air}}{\lambda _{water}}=\frac{\theta _{air}}{\theta _{water}}=\mu$
From here
$\theta _{water}=\frac{\theta _{air}}{\mu _{water}}=\frac{3}{4}0.2^o=0.15^o$
Hence angular width of the fringe in the water is $0.15^o$.
Q2: What is the Brewster angle for air to glass transition? (Refractive index of glass = 1.5.)
Answer:
Given,
Refractive index of glass $\mu_{glass}=1.5$
Now, as we know,
$tan\theta =\mu$
where $\theta$ is the polarising angle, also called the Brewster angle, and $\mu$ is the refractive index.
So from here
$\theta =tan^{-1}(\mu _{glass})=tan^{-1}1.5=56.31^o$
Hence, Brewster angle is $56.31^o$.
Answer:
Given,
Wavelength of light $\lambda =5000 Å =5000*10^{-10}m$
Speed of light $c=3*10^8$
Now,
Wavelength and frequency will be the same when the ray is reflected.
Frequency of reflected light
$f=\frac{c}{\lambda }=\frac{3*10^{8}}{5000*10^{-10}}=6*10^{14}Hz$
Hence, wavelength and frequency of light are $5000*10^ {-10}m$ and $6*10^{14}Hz$ respectively.
Now,
As per the law of reflection, the angle of incidence $i$ is always equal to the angle of reflection $r$.
$i=r$
Now, when the reflected ray is perpendicular to the incident ray,
$i+r=90$
$i+i=90$
$2i=90$
$i=45$
Hence, the angle of incidence is 45 for this condition.
Answer:
Given
Aperture $a=4mm=4*10^{-3}m$
Wavelength of light $\lambda =400nm=400*10^{-9}m$
Now,
The distance for which ray optics is a good approximation, also called Fresnel's distance:
$Z_f=\frac{a^2}{\lambda }=\frac{(4*10^{-3})^2}{400*10^{-9}}=40m$
Hence distance for which ray optics is a good approximation is 40m.
Answer:
Given,
wavelength Hα line emitted by hydrogen:
$\lambda=6563*10^{-10}m$
The star is redshifted by
$\lambda'-\lambda=15*10^{-10}m$
Let the velocity of the star be $v$
Now,
As we know,
$\lambda'-\lambda=\frac{v}{c}*\lambda$
from here
$\lambda'-\lambda\frac{1}{{c}*\lambda }={v}$
$v=\frac{3*10^8*15*10^{-10}}{6563*10^{-10}}=6.87*10^5m/s$
Hence speed at which the star is receding away is $6.87*10^5m/s$
Answer:
According to corpuscular theory, when a corpuscle of light goes from a rare medium to a denser medium, the component of its velocity along the surface of the interface remains the same.
So we can write
$v_1sini=v_2sinr$
$\frac{v_2}{v_1}=\frac{sini}{sinr}=\mu$
As $\mu>1$ ,
$v_2>v_1$
That is, light should be faster in the dense medium than in the rare medium. This is the opposite of what we see experimentally.
Huygens' wave theory predicts that light is faster in a rare medium, which matches our experimental observation.
Answer:
Let an object M be placed in front of a plane mirror AB at a distance r.
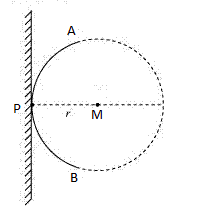
A circle is drawn from the centre, such that it just touches the plane mirror at point P. According to the Huygens’ principle, AB is the wavefront of the incident light.
If the mirror is absent, then a similar wavefront A'B' would form behind M at a distance r.
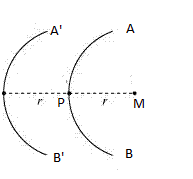
A'B' can be considered as a virtual reflected ray for the plane mirror. Hence, a point object placed in front of the plane mirror produces an image at the same distance as the object.
Answer:
The speed of light in a vacuum is constant and independent of anything, according to Einstein's theory of relativity.
Answer:
The speed of light in any medium depends upon the wavelength of the light and does not depend on the nature of the source, direction of propagation, the motion of the source and/or observer, or intensity of the wave.
Answer:
The sound wave requires a medium for propagation. So, even though both given situations may relate to the same relative motion, they are not identical physically since the motion of the observer, relative to the medium, is different in the two situations. Hence, we cannot expect the Doppler formula to be identical in both given cases.
When light waves are in a vacuum, there is clearly nothing to distinguish between the two cases.
For light propagation in a medium, two situations are not identical for the same reason as in the case of sound waves.
Answer:
Given,
Wavelength of light $\lambda$ = 600nm
Angular fringe width
$B_{\theta}=\frac{\lambda}{d}$
$d=\frac{\lambda}{B_{\theta}}=\frac{600*10^{-9}*180}{0.1*\pi }=3.44*10^{-4}m$
Hence spacing required between the two slits is $3.44*10^{-4}m$.
Answer:
As we know,
The width of the central diffraction band is given by
$2D\frac{\lambda}{d}$
where d is the width of the slit.
So when we double the width of the slit, the size of the central diffraction band reduces to half of its value. But the light amplitude becomes double, which increases the intensity 4 times.
Answer:
When we have a width in the order of $\lambda$, the intensity of interference fringes in Young's double-slit experiment is modified by the diffraction pattern of each slit.
Answer:
A bright spot is seen at the centre of the shadow of the obstacle because waves diffracted from the edge of a circular obstacle interfere constructively at the centre of the shadow, producing the bright spot.
Answer:
The size of the obstacle should be comparable to the wavelength for diffraction of waves by obstacles, on a large scale.
This comes from
$\sin\theta =\frac{\lambda}{a}$
$\sin\theta =\frac{10^{-7}}{10}=10^{-8}$
This implies $\theta\rightarrow 0$
It means the light goes almost unbent, and hence, students are unable to see each other.
Answer:
The typical size of the obstacle is much larger than the wavelength of light. Hence, the diffraction effect is negligibly small. Thus, the assumption that light travels in a straight line can be safely used in day-to-day life.
Answer:
Given,
Distance between two towers = 40km
size of aperture = $a=50m$
Now,
As we know
Fresnel's distance is equal to half of the distance between towers
$Z_f=\frac{40}{2}=20km$
Also from the formula:
$Z_f=\frac{a^2}{\lambda}=20m$
$\lambda=\frac{a^2}{Z_f}=\frac{50^2}{20*10^3}=12.5cm$
Hence, this is the required longest wavelength of the radio wave, which can be sent between the towers without a considerable diffraction effect.
Q13: A parallel beam of light of wavelength 500 nm falls on a narrow slit and the resulting diffraction pattern is observed on a screen 1 m away. It is observed that the first minimum is at a distance of 2.5 mm from the centre of the screen. Find the width of the slit.
Answer:
Given
The distance of the screen from the slit, $D=1m$
The distance of the first minimum $X_1=2.5mm=10^{-3}=2.5*10^{-3}mm$
The wavelength of the light $\lambda=500nm=500*10^{-9}m$
Now,
As we know,
$X_n=n\frac{\lambda D}{d}$
$d=n\frac{\lambda D}{X_n}=1*\frac{500*10^{-9}*1}{2.5*10^{-3}}=2*10^{-4}m=0.2mm$
Hence, the width of the slit is 0.2 mm.
Answer:
When a low-flying aircraft passes overhead, we notice slight shaking in the pictures on the TV. This is because aircraft interfere with signals and reflect them. So the shaking we see is the interference of the direct signal and the reflected signal.
Answer:
The superposition principle comes from the linear character of the differential equation of wave motion. That is, if $x_1$ and $x_2$ are the solutions of any wave equation, then a linear combination of $x_1$ and $x_2$ is also the solution of the wave equation.
Answer:
Let the width of the slit $b$ be divided into n equal parts so that
$b'=\frac{b}{n}$
$b=b'n$
Now,
$\theta=\frac{n\lambda}{b}=\frac{n\lambda}{b'n}=\frac{\lambda}{b'}$
At this angle, each slit will make the first diffraction minimum. Therefore, the resultant intensity for all the slits will be zero at the angle of $\frac{n\lambda}{b}$.
Class 12 Physics NCERT Chapter 10: Higher Order Thinking Skills (HOTS) Questions
Class 12 Physics Chapter 10 Wave Optics HOTS Questions help students develop analytical and reasoning skills by applying concepts like interference, diffraction, and polarisation to solve complex problems. These questions are ideal for exam preparation and competitive exams like JEE and NEET, strengthening conceptual understanding.
Q1: In Young's Double Slit Experiment, the distance between the slits and the screen is 1.2m, and the distance between the two slits is 2.4 m. If a thin transparent mica sheet of thickness 1 µm and R.I. 1.5 is introduced between one of the interfering beams, the shift in the position of the central bright fringe is
Answer:
$
(\mu-1) t=\frac{x d}{D} \text { or } \mu=1+\frac{x d}{D t}
$
Or $x=\frac{(\mu-1) t D}{d}=\frac{(1.5-1) \times 1 \times 10^{-6} \times 1.2}{2.4 \times 10^{-3}}=0.25 \mathrm{~mm}$
Q2: Three polaroid sheets, P1, P2 and P3, are kept parallel to each other such that the angle between the pass axes of P1 and P2 is 45° and that between P2 and P3 is 45°. If an unpolarised beam of light of intensity 128 Wm-2 is incident on P1. What is the intensity of light coming out of P3?
Answer:
The ray of light passing through polaroid $P_1$ will have its intensity reduced by half.
$
I_1=\frac{I_0}{2}
$
Now, the polaroid $\mathrm{P}_2$ is oriented at an angle $45^{\circ}$ with respect to $\mathrm{P}_1$.
Therefore the intensity is $I_2=I_1 \cos ^2 45^{\circ}=\frac{I_0}{2} \times \frac{1}{2}=\frac{I_0}{4}$
Now, the polaroid $P_3$ is oriented at an angle $45^{\circ}$.
Therefore, the intensity is $I_3=I_2 \cos ^2 45^{\circ}=\frac{I_0}{4} \cos ^2 45^{\circ}=\frac{I_0}{4} \times \frac{1}{2}=\frac{I_0}{8}$
And given, $I_o=128 \mathrm{Wm}^{-2}$
So, $I_3=\frac{I_o}{8}=\frac{128}{8}=16 \mathrm{Wm}^{-2}$
Q3: Two waves represented by
$
y_1=3 \sin (\omega t) \text { and } y_2=4 \sin (\omega t)
$
interfere at a point, then the amplitude of the resultant wave is
Answer:
Resultant amplitude of two waves -
$
A=\sqrt{A_1^2+A_2^2+2 A_1 A_2 \cos \theta}
$
wherein
$A_1=$ amplitude of wave 1
$A_2=$ amplitude of wave 2
$\phi=$ phase difference
here $\phi=0$
$A=\sqrt{9+16+2 \times 3 \times 4 \times 1}$
$A=\sqrt{49}=7$
Q4: Maximum intensity in YDSE is $I_1$. The intensity at a point on the screen where the phase difference between the two interfering beams is $\frac{\pi}{3}$:
Answer:
$
\begin{aligned}
& I=I_{\max } \cos ^2\left(\frac{\theta}{2}\right) \\
& I=I_1 \cos ^2\left(\frac{\pi}{6}\right) \text { for } \frac{\pi}{3} \\
& I=I_1 \times \frac{3}{4} \\
& 0.75 I_1
\end{aligned}
$
Q5: Figure 10.2 shows a standard two-slit arrangement with slits S1 and S2. P1 and P2 are the two minima points on either side of P (Fig. 10.2). At P2 on the screen, there is a hole and behind P2 is a second 2-slit arrangement with slits S3, S4 and a second screen behind them, then
1. There would be no interference pattern on the second screen, but it would be lit.
2. The second screen would be totally dark.
3. There would be a single bright point on the second screen.
4. There would be a regular two-slit pattern on the second screen.
Answer: In the given figure, there is a hole at the minima point $P_2$, the hole will act as a source of fresh light for the slits $\mathrm{S}_3$ and $\mathrm{S}_4$. Hence, there will be a regular two-slit pattern on the second screen.
CBSE Class 12th Syllabus: Subjects & Chapters
Select your preferred subject to view the chapters
NCERT Solutions for Class 12 Physics Chapter 10 Wave Optics: Topics
Class 12 Physics Chapter 10 Wave Optics explains the wave nature of light and its behaviour in different scenarios. The topics cover concepts like Huygens’ principle, interference, diffraction, and polarisation, helping students understand how light waves interact and propagate in various media.
- Huygens’ principle
- Refraction and reflection of plane waves using Huygens’ principle
- Coherent and incoherent addition of waves
- Interference of light waves and Young’s experiment
- Diffraction
- Polarisation
JEE Main Highest Scoring Chapters & Topics
Just Study 40% Syllabus and Score upto 100%
Download EBookNCERT Solutions for Class 12 Physics Chapter 10 Wave Optics: Important Formulas
All the important formulas of wave optics are presented here, saving the students the trouble of referring to a large number of books to revise. It provides formulas that are needed to solve interference, diffraction, polarisation, and fringe calculations, needed to solve NCERT problems, as well as competitive exams such as JEE and NEET.
1. Interference of Light
- Path difference for constructive interference:
$
\Delta L=n \lambda \quad(n=0,1,2, \ldots)
$
- Path difference for destructive interference:
$
\Delta L=\left(n+\frac{1}{2}\right) \lambda \quad(n=0,1,2, \ldots)
$
- Fringe width in Young's Double Slit Experiment:
$
\beta=\frac{\lambda D}{d}
$
where $\lambda=$ wavelength of light, $D=$ distance between slits and screen, $d=$ distance between slits.
2. Diffraction of Light
- Width of central maximum for single slit:
$
w=\frac{2 \lambda L}{a}
$
where $a=$ slit width, $L=$ distance to screen.
- Condition for minima in single slit diffraction:
$
a \sin \theta=m \lambda \quad(m=1,2,3, \ldots)
$
3. Polarisation of Light
- Malus' Law:
$
I=I_0 \cos ^2 \theta
$
where $I_0=$ initial intensity, $\theta=$ angle between light's polarisation and polariser axis.
4. Thin Films (Interference)
- Condition for constructive interference (reflection):
$
2 n t=m \lambda \quad(\text { or }(2 m+1) \lambda / 2 \text { depending on phase change })
$
where $n=$ refractive index of film, $t=$ thickness of film, $m=0,1,2 \ldots$.
- Condition for destructive interference (reflection):
$
2 n t=\left(m+\frac{1}{2}\right) \lambda
$
How Can NCERT Solutions for Class 12 Physics Chapter 10 Wave Optics Help in Exam Preparation?
NCERT Wave Optics Class 12 Solutions help students clearly understand the wave nature of light and its important phenomena such as interference, diffraction, and polarisation. These Class 12 Physics chapter 10 Wave Optics question answers simplify complex concepts and experiments in simple step-by-step explanations, making them easier to study and review to get better marks. Frequent application of these Class 12 Physics chapter 10 Wave Optics question answers enhances the ability to solve problems as well as minimise the errors regularly experienced in terms of formula and sign convention.
Moreover, Wave Optics class 12 question answers are extremely helpful for competitive exams like JEE and NEET, where conceptual clarity and application-based questions are important. Frequent practice with these Wave Optics Class 12 NCERT Solutions will create a firm background in related topics like the Dual Nature of Radiation and Matter, which allows improved performance and retention of wave optics ideas.
Approach to Solve Questions of Wave Optics Class 12
Students should follow a step-wise approach to solve Wave Optics questions in Class 12 Physics. Identify the phenomenon involved first: is it the interference, diffraction or polarisation? In the case of interference (such as in the case of Young's slit interference experiment), focus on the path difference and then apply the condition of constructive or destructive interference with the use of the fringe width formula. In diffraction problems, find a connection between slit width, wavelength and angle using the minimum condition and find the width of the central maximum. In the case of polarisation, use Malus' law and note the place of the polariser and analyser. Every time you write the values, use the correct formula and replace values with appropriate units. Finally, make neat drawings when possible, as they would help to make a visual image of the wave behaviour and reduce errors in the calculation.
Importance of NCERT Solutions for Class 12 Physics Chapter 10: Wave Optics
Class 12 Physics chapter 10 Wave Optics solutions are important for understanding the wave nature of light and phenomena that cannot be explained using ray optics alone. This Class 12 Physics NCERT Chapter 10 Wave Optics is very instrumental in the formulation of conceptual understanding and analytical ability.
- Helps students have a clear understanding of the basic concepts like interference, diffraction and polarisation of light.
- Discusses significant experiments such as the double slit experiment of Young clearly and in an understandable way.
- Establishes a firm foundation on advanced topics in relation to optics and modern physics.
- Highly important for CBSE board exams, where theory-based questions and numericals from wave optics are frequently asked.
- Useful for competitive exams like JEE and NEET, especially questions based on interference and diffraction patterns.
- Enhances problem-solving expertise by the use of step-by-step numerical answers as well as derivations.
- Helps students through clear explanations and diagrams to visualise concepts, thereby minimising common conceptual errors.
- Facilitates proper usage of equations in various physical scenarios that are related to the wave behaviour of light.
What Extra Should Students Study Beyond NCERT for JEE/NEET?
Beyond NCERT, students preparing for JEE/NEET Wave Optics should focus on advanced problem-solving and tricky applications of concepts like interference, diffraction, and polarisation. Extra study should include topics such as intensity distribution in interference and diffraction patterns, Brewster’s law in detail, resolving power of optical instruments, and numerical problems combining ray optics with wave optics. Practising previous years’ JEE/NEET questions will also strengthen conceptual clarity and speed.
NCERT Solutions for Class 12 Physics: Chapter-Wise
NCERT Class 12 Physics Solutions provide chapter-wise, detailed answers to all textbook exercises, making it easier for students to grasp tough concepts. These step-by-step solutions are designed as per the latest CBSE syllabus and are highly useful for both board exams and competitive exams like JEE & NEET. With chapter-wise links, students can directly access the solutions and download the PDFs for quick and effective revision.
NCERT Books and NCERT Syllabus
NCERT Solutions Subject-wise
NCERT Solutions subject-wise links provide easy access to chapter-wise answers for all major subjects in one place. These solutions help students understand concepts clearly, practise textbook questions effectively, and prepare confidently for exams
NCERT Exemplar Class 12 Solutions
NCERT Exemplar Solutions for Class 12 provide advanced and application-based questions to strengthen conceptual understanding. These subject-wise links help students practise higher-level problems and prepare effectively for board exams as well as competitive exams like JEE and NEET.
Frequently Asked Questions (FAQs)
Q: Are NCERT books for Class 12 Physics enough to prepare for CBSE Board exams?
A:
Yes, NCERT books are enough to prepare for the board exams, but you can refer to other reference books and sample papers as well. Try to cover all the concepts based on the NCERT syllabus. To get a good score in the CBSE board exam understand all the topics in the NCERT book and solve all the questions of NCERT exercise. Additionally students can refer NCERT exemplar problems and CBSE previous year question papers.
Q: Is the Wave Optics Class 12 NCERT PDF enough for board exam preparation?
A:
Yes, the Wave Optics Class 12 NCERT PDF covers all important concepts and questions. It’s a must for board exam prep and builds your foundation for competitive exams like JEE and NEET.
Q: Are there any Wave Optics questions with answers PDF for practice?
A:
You can download Wave Optics questions with answers PDF for extra practice. These help you solve different types of questions from exams and strengthen your understanding.
Q: How can I quickly solve the wave optics-based problems in Chapter 10 of wave optics class 12 ncert?
A:
To quickly solve ncert wave optics problems in ch 10 physics class 12, make sure you have a clear understanding of the fundamental concepts and practice solving problems. Read the problem statement carefully and use diagrams to visualize the problem. Review your work and seek help if needed.
Q: What do Class 12 Physics Chapter 11 Exercise Solutions include?
A:
Class 12 Physics Chapter 11 Exercise Solutions include detailed answers to all NCERT textbook questions, along with step-by-step explanations for better understanding.
Q: In Wave Optics, what are the most common mistakes that students commit?
A:
Students have the tendency to mix up maxima and minima conditions and commit errors in calculating the formulae involving fringe width and diffraction patterns.
Q: What is the difference between interference and diffraction?
A:
Interference occurs due to the superposition of light waves from two coherent sources, while diffraction occurs due to the bending of light around an obstacle or aperture.
Q: How is the resolving power of optical instruments described in the NCERT Solutions for Wave Optics?
A:
The resolving power is explained as the ability of an optical system, such as a microscope or telescope, to distinctly separate two close objects. NCERT Solutions discuss key factors affecting resolving power, including wavelength and aperture size, and explain the physical and mathematical interpretation as per CBSE guidelines.
Q: How do NCERT Solutions integrate updates from the CBSE 2025–26 deleted syllabus for Wave Optics?
A:
NCERT Solutions for Chapter 10 in the 2025–26 session include only the syllabus topics currently prescribed by CBSE. Questions or solutions based on removed topics, such as the Doppler Effect or extended derivations, are excluded, ensuring all solutions are strictly syllabus-aligned and exam-focused.
Articles
Related Stories
|
Upcoming School Exams
Certifications By Top Providers
Explore Top Universities Across Globe
Questions related to CBSE Class 12th
On Question asked by student community
Have a question related to CBSE Class 12th ?
Hello
You will be able to download the CBSE Previous Year Board Question Papers from our official website, careers360, by using the link given below.
https://school.careers360.com/boards/cbse/cbse-previous-year-question-papers
I hope this information helps you.
Thank you.
Hello
You will be able to download the CBSE Pre-Board Class 12 Question Paper 2025-26 from our official website by using the link which is given below.
https://school.careers360.com/boards/cbse/cbse-pre-board-class-12-question-paper-2025-26
I hope this information helps you.
Thank you.
Hello,
Yes, it's completely fine to skip this year's 12th board exams and give them next year as a reporter or private candidate, allowing you to prepare better; the process involves contacting your current school or board to register as a private candidate or for improvement exams during the specified
HELLO,
Yes i am giving you the link below through which you will be able to download the Class 12th Maths Book PDF
Here is the link :- https://school.careers360.com/ncert/ncert-book-for-class-12-maths
Hope this will help you!
Hello,
Here is your Final Date Sheet Class 12 CBSE Board 2026 . I am providing you the link. Kindly open and check it out.
https://school.careers360.com/boards/cbse/cbse-class-12-date-sheet-2026
I hope it will help you. For any further query please let me know.
Thank you.
Popular CBSE Class 12th Questions
A block of mass 0.50 kg is moving with a speed of 2.00 ms-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
A particle is projected at 600 to the horizontal with a kinetic energy . The kinetic energy at the highest point
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
In the reaction,
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
How many moles of magnesium phosphate, will contain 0.25 mole of oxygen atoms?
| Option 1)
0.02 |
Option 2)
3.125 × 10-2 |
| Option 3)
1.25 × 10-2 |
Option 4)
2.5 × 10-2 |
Colleges After 12th
Applications for Admissions are open.
As per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters
CBSE Class 12th Notifications
Never miss CBSE Class 12th update
Get timely CBSE Class 12th updates directly to your inbox. Stay informed!