• School
  • Engineering and Architecture
  • Management and Business Administration
  • Medicine and Allied Sciences
  • Law
  • Animation and Design
  • Media, Mass Communication and Journalism
  • Finance & Accounts
  • Computer Application and IT
  • Pharmacy
  • Hospitality and Tourism
  • Competition
  • Study Abroad
  • Arts, Commerce & Sciences
  • Learn
  • Online Courses and Certifications
NCERT Exemplar Class 11 Physics Solutions Chapter 8 Gravitation

NCERT Exemplar Class 11 Physics Solutions Chapter 8 Gravitation

Edited By Safeer PP | Updated on Aug 08, 2022 12:03 PM IST

The chapter consists of Newton’s most astonishing discovery about the apple falling on his head. It was very profoundly named ‘Gravitation.’ NCERT Exemplar Class 11 Physics solutions chapter 8, deals with the chapter where the law of attraction comes into the picture. A group of professionals that the students would enjoy their learning has prepared these solutions. NCERT Exemplar Class 11 Physics solutions chapter 8 has got all the topics covered, where the students will not feel burdened about the high amount of learning. It will easily help students grasp. The chapter not only covers gravitation but other topics like Kepler’s law. Here in the chapter, the effect on the earth’s centre regarding the attraction flow. NCERT Exemplar Class 11 Physics solutions chapter 8, has experts developed it, and thus the students will enjoy the learning.

JEE Main Scholarship Test Kit (Class 11): Narayana | Physics Wallah | Aakash | Unacademy

NEET Scholarship Test Kit (Class 11): Narayana | Physics Wallah | Aakash | ALLEN

Scholarship Test: Vidyamandir Intellect Quest (VIQ) | ALLEN TALLENTEX

This Story also Contains
  1. NCERT Exemplar Class 11 Physics Solutions Chapter 8: MCQI
  2. NCERT Exemplar Class 11 Physics Solutions Chapter 8: MCQII
  3. NCERT Exemplar Class 11 Physics Solutions Chapter 8: Very Short Answer
  4. NCERT Exemplar Class 11 Physics Solutions Chapter 8: Short Answer
  5. NCERT Exemplar Class 11 Physics Solutions Chapter 8: Long Answer
  6. Main Subtopics of NCERT Exemplar Class 11 Physics Solutions Chapter 8
  7. NCERT Exemplar Class 11 Physics Solutions Chapter-Wise
  8. NCERT Exemplar Class 11 Solutions
  9. Important Topics To Cover For Exams From NCERT Exemplar Class 11 Physics Solutions Chapter 8

Also, check NCERT Solutions for Class 11 other subjects

NCERT Exemplar Class 11 Physics Solutions Chapter 8: MCQI

Question:8.1

The earth is an approximate sphere. If the interior contained matter which is not of the same density everywhere, then on the surface of the earth, the acceleration due to gravity
a) will be directed towards the centre but not the same everywhere
b) will have the same value everywhere but not directed towards the centre
c) will be same everywhere in magnitude directed towards the centre
d) cannot be zero at any point

Answer:

The answer is the option (d) According to the formula of gravity
g=\frac{4\pi \rho GR}{3}
G can never be equal to 0 because G is constant and R and \rho can never be 0.

Question:8.2

As observed from earth, the sun appears to move in an approximately circular orbit. For the motion of another planet like mercury as observed from earth, this would
a) be similarly true
b) not be true because the force between earth and mercury is not inverse square law
c) not be true because the major gravitational force on mercury is due to sun
d) not be true because mercury is influenced by forces other than gravitational forces

Answer:

The answer is the option (c) The universal law of force of attraction is inversely dependent on the distance between them. For mercury, this force due to the sun is exceptionally large than that due to earth. Due to relative motion of sun and earth with mercury, this would not be true.

Question:8.3

Different points in the earth are at slightly different distances from the sun and hence experience different forces due to gravitation. For a rigid body, we know that if various forces act at various points in it, the resultant motion is as if a net force acts on the cm causing translation and a net torque at the cm causing rotation around an axis through the cm. For the earth-sun system
a) the torque is zero
b) the torque causes the earth to spin
c) the rigid body result is not applicable since the earth is not even approximately a rigid body
d) the torque causes the earth to move around the sun

Answer:

The answer is the option (a)The direction of gravitational force F and the line joining the center of mass of the earth (point of application of force) both lie in the same direction. So, the angle between them is 0.
In the formula :\overrightarrow{\tau }=\overrightarrow{r}\times \overrightarrow{F}=rF\; \sin\; \theta
\sin\; 0=0
Hence, torque is zero.

Question:8.4

Satellites orbiting the earth have a finite life and sometimes debris of satellites fall to the earth. This is because
a) the solar cells and batteries in satellites run out
b) the laws of gravitation predict a trajectory spiralling inwards
c) of viscous forces causing the speed of the satellite and hence height to gradually decrease
d) of collisions with other satellites

Answer:

The answer is the option (c)
P.E is given by \frac{-GM}{2r}
Negative sign shows the force of attraction between satellite and earth.
P.E gets gradually reduced due to atmospheric friction, and the radius/ height of satellite keeps decreasing until it comes back to earth with increasing speed and burns in the atmosphere.

Question:8.5

Both earth and moon are subject to the gravitational force of the sun. as observed from the sun, the orbit of the moon
a) will be elliptical
b) will not be strictly elliptical because the total gravitational force on it is not central
c) is not elliptical but will necessarily be a closed curve
d) deviates considerably from being elliptical due to the influence of planets other than earth

Answer:

The answer is the option (b) Moon is not always on the line joining the sun and earth. Therefore, the gravitational force of attraction due to earth and sun have different lines of action or let us say the forces are not central. So, the orbit of the moon will not be strictly elliptical.

Question:8.6

In our solar system, the inter-planetary region has chunks of matter called asteroids. They
a) will not move around the sun since they have very small masses compared to the sun
b) will move in an irregular way because of their small masses and will drift away outer space
c) will move around the sun in closed orbits but not obey Kepler’s laws
d) will move in orbits like planets and obey Kepler’s laws

Answer:

The answer is the option (d) Central gravitational forces act upon asteroids. Hence, they will move in orbits and obey Kepler's law.

Question:8.7

Choose the wrong option.
a) inertial mass is a measure of the difficulty of accelerating a body by an external force whereas the gravitational mass is relevant in determining the gravitational force on it by an external mass
b) that the gravitational mass and inertial mass are equal is an experimental result
c) that the acceleration due to gravity on earth is the same for all bodies is due to the equality of gravitational mass and inertial mass
d) gravitational mass of a particle-like proton can depend on the presence of neighbouring heavy objects, but the inertial mass cannot

Answer:

The answer is the option (d) The gravitational mass of a proton is equivalent to its inertial mass & is independent of the presence of neighbouring heavy objects.

Question:8.8

Particles of masses 2M, m and M are respectively at points A, B, and C with AB=\frac{1}{2}(BC).. M is much-much smaller than M and at time t=0, they are all at rest. At subsequent times before any collision takes place.
a) m will remain at rest
b) m will move towards M
c) m will move towards 2M
d) m will have oscillatory motion
capture-88

Answer:

The answer is the option (c) m will move towards 2M.
Let F_{BC} be the force experienced by m at point B due to M at point C and F_{BA} be force experienced by m at point due to 2 M at point A
F_{Bc}=\frac{GmM}{Bc^{2}}
F_{AB}=\frac{Gm2M}{AB^{2}}
If AB=x then BC=2x
BC^{2}>AB^{2}
F_{BA}>F_{BC}
Thus, m will move towards point A.

NCERT Exemplar Class 11 Physics Solutions Chapter 8: MCQII

Question:8.9

Which of the following options is correct?
a) acceleration due to gravity decreases with increasing altitude
b) acceleration due to gravity increases with increasing depth
c) acceleration due to gravity increases with increasing latitude
d) acceleration due to gravity is independent of the mass of the earth

Answer:

The answer is the option (a) and (c)
g at an altitude g(1-\frac{2h}{R})
Acceleration due to gravity decreases with increasing altitude. Hence a is correct
g^{\lambda }= g-\omega ^{2}R(cos\lambda )^{2}
As cos is decreasing function, the value of gravity increases with increase in latitude, i.e. from the equator to pole. Hence,c is correct
g=\frac{GM}{R^{2}} Where M is the mass of earth. Hence, d is incorrect

Question:8.10

If the law of gravitation, instead of being inverse-square law, becomes an inverse-cube-law
a) planets will not have elliptic orbits
b) circular orbits of planets is not possible
c) projectile motion of a stone thrown by hand on the surface of the earth will be approximately parabolic
d) there will be no gravitational force inside a spherical shell of uniform density

Answer:

The correct answer is the option (a), (b), and (c).
We know that Force of gravitation = Centrifugal force for a body in a circular motion
If the law of gravitation becomes inverse cube law then
\frac{GmM}{r^{3}}=\frac{v^{2}m}{r}
where m = mass of the planet, M = mass of the sun and r = radius of the orbit
Thus, v is inversely proportionate to r
The time period of a planet around the sun =\frac{2\pi r}{v}
T^2\propto r^4
So the path is not elliptical. (By Keplers law T^2\propto r^3)
So, a is correct
When \frac{GmM}{r^{3}}=mg'
g'=\frac{GM}{r^{2}}
Hence, b is incorrect
Since, T\alpha r^{2} ; the path of the projectile will be parabolic. Thus, (c) is correct
Gravitational force is a universal force as it acts everywhere. Hence, (d) is incorrect

Question:8.11

If the mass of sun were ten times smaller and gravitational constant G were ten times larger in magnitudes
a) walking on ground would become more difficult
b) the acceleration due to gravity on earth will not change
c) raindrops will fall much faster
d) aeroplanes will have to travel much faster

Answer:

The correct answer is the option (a), (c), and (d).
If G'=10G
And M'_{s}=\frac{M_{s}}{10}
Then g'=\frac{G'M_{E}}{r^{2}}=\frac{10GM}{r^{2}}=10g
Weight of a person =mg'=10 mg
Hence a is correct, and b is incorrect
F on man due to sun =\frac{GM'_{s}m}{r^{2}}=\frac{GM_{s}m}{10r^{2}}
The terminal velocity v_{T}\alpha g'\; \; \; or\; \; \; \; v_{T}\; \alpha 10g
Hence raindrops fall 10 times faster. Option c is correct
In order to maintain the speed after the increase in gravitational force, the aeroplane will have to travel faster.

Question:8.12

If the sun and the planets carried huge amounts of opposite charges,
a) all three of Kepler’s laws would still be valid
b) only the third law will be valid
c) the second law will not change
d) the first law will still be valid

Answer:

The correct answer is the option (a), (c), and (d). Due to opposite charges, large electrostatic forces of attraction will be produced in addition to the gravitational forces. Both forces, when added, will be radial in nature. Since they are central forces and obey inverse square law, all 3 Kepler's laws will be valid.

Question:8.13

There have been suggestions that the value of the gravitational constant G becomes smaller when considered over an exceptionally large time period in the future. If that happens for our earth,
a) nothing will change
b) we will become hotter after billions of years
c) we will be going around but not strictly in closed orbits
d) after a sufficiently long time we will leave the solar system

Answer:

The correct answer is the option (c) and (d)
Force of gravitation = Centrifugal force for a body in a circular motion
F=\frac{GmM}{r^{2}}
If G becomes smaller, the centripetal force also decreases
We know that v_{o}\alpha \sqrt{G}, Thus, the centrifugal force becomes larger than the centripetal force (F\; \alpha \; G)
Distance from sun increases and earth will move in larger orbit in time.
C, D are correct.

Question:8.14

Supposing Newton’s law of gravitation for gravitation forces F1 and F2 between two masses m1 and m2 at positions r1 and r2 read
\mathbf{F_{1}}=\mathbf{-F_{2}}=\frac{\mathbf{r_{12}}}{r_{12}^{3}}GM_{0}^{2}\left ( \frac{m_{1}m_{2}}{M_{1}^{2}} \right )^{n} where Mo is a constant of the dimension of mass, \mathbf{r_{12}} = \mathbf{r_{1}} - \mathbf{r_{2}} and n is a number. In such a case,
a) the acceleration due to gravity on earth will be different for different object
b) none of the three laws of Kepler will be valid
c) only the third law will become invalid
d) for n negative, an object lighter than water will sink in water

Answer:

The correct answer is the option (a) and (c)
\overrightarrow{F_{1}}=\overrightarrow{F_{2}}=\frac{\overrightarrow{r_{12}}}{r_{12}^{3}}GM_{0}^{2}\left [ \frac{m_{1}m_{2}}{M_{0}^{2}} \right ]^{n}
given, \overrightarrow{r_{12}}=\overrightarrow{r_{1}}-\overrightarrow{r_{2}}
Acceleration due to gravity (g) =
\frac{\left | F \right |}{mass (m)}
g=\left | \frac{GM_{0}^{2}(m_{1}m_{2})^{n}}{r_{12}^{2}M_{0}^{2n}}\times\frac{\hat{r}_{12}}{m} \right |
\hat{r}_{12} tells the direction of g from 1 to 2
g= \frac{GM_{0}^{2}(m_{1}m_{2})^{n}}{r_{12}^{2}M_{0}^{2n}}\; \frac{1}{n}
Since g is dependent on position vector

\overrightarrow{r_{12}}, mass of body m. So, g on eath will be different for different bodies of different mass and their
Position. Hence a and c are correct
As the force is central in nature, Kepler's first and second law is valid rejecting option b.
g= \frac{GM_{0}^{2}(m_{1}m_{2})^{-n}}{r_{12}^{2}M_{0}^{-2n}}\; \frac{1}{n}=\frac{GM_{0}^{2}M_{0}^{2n}}{(m_{1}m_{2})^{n}r_{12}^{2}}\; \frac{1}{m}=\frac{GM_{0}^{2}}{r_{12}^{2}}\left [ \frac{M_{0}^{2}}{m_{1}m_{2}} \right ]\; \frac{1}{m}
g>0
So M_{0}>m_{1}\; or \; m_{2}
The lighter object can sink in water. Hence, d is correct

Question:8.15

Which of the following are true?
a) a polar satellite goes around the earth’s pole in a north-south direction
b) a geostationary satellite goes around the earth in an east-west direction
c) a geostationary satellite goes around the earth in a west-east direction
d) a polar satellite goes around the earth in an east-west direction

Answer:

The correct answer is the option (a) and (c) A geostationary satellite appears stationary relative to the earth and revolves around the earth from west to east with same angular velocity as earth's rotation about its axis from west to east. Polar satellite revolves around the earth from north to south independent of the earth's rotation.

Question:8.16

The centre of mass of an extended body on the surface of the earth and its centre of gravity
a) are always at the same point for any size of the body
b) are always at the same point only for spherical bodies
c) can never be at the same point
d) is close to each other for objects, say of sizes less than 100 m
e) both can change if the object is taken deep inside the earth

Answer:

The correct answer is the option (d). For smaller bodies, the COM (Center of mass) and COG (Center of gravity) usually coincide. For extended objects like mountains and lake, they are far away. COG is the point in a body where net torque due to gravity is 0. The COM is where the whole mass of a body is supposed to be concentrated.

NCERT Exemplar Class 11 Physics Solutions Chapter 8: Very Short Answer

Question:8.17

Molecules in the air in the atmosphere are attracted by the gravitational force of the earth. Explain why all of them do not fall into the earth just like an apple falling from a tree.

Answer:

Air molecules possess thermal energy. The resultant velocity due to all the forces acting on them is not directed downwards, so they do not fall. In case of an apple only gravitational force s acting downward, that is why they fall into the earth.

Question:8.18

Give one example each of central force and non-central force.

Answer:

Gravitational force, electrostatic force and spring force are examples of central force Magnetic forces between 2 current carrying loops is an example of non-central force

Question:8.19

Draw areal velocity versus time graph for mars.
capture-819

Answer:

According to Kepler's second law, a planet sweeps an equal area in equal times. So the areal velocity is constant with respect to time.

Question:8.20

What is the direction of areal velocity of the earth around the sun?

Answer:

Real velocity of the earth around the sun is given by \frac{dA}{dt}=\frac{L}{2m}
Where L is angular momentum and m is mass of earth
Since \overrightarrow{L}=\overrightarrow{r}\times \overrightarrow{p}=\overrightarrow{r}\times m\overrightarrow{v}
\frac{dA}{dt}=\frac{\vec{r}\times m\vec{v}}{2m}=\frac{(\vec{r}\times\vec{v})}{2}
Thus, the direction of areal velocity is perpendicular to the plane of \vec{r} and \vec{v} (Maxwell's right-hand rule)

Question:8.21

How is the gravitational force between two point masses affected when they are dipped in the water keeping the separation between them the same?

Answer:

The force of attraction varies inversely with the distance between two objects. Since the separation between two point masses is same as the force of attraction also remains the same.

Question:8.22

Is it possible for a body to have inertia but no weight?

Answer:

Everybody has some mass or inertia. Weight of a body is equal to mg. It is possible for a body to have no weight when g=0 and hence mg=0, which is possible in space where there is no gravity.

Question:8.23

We can shield a charge from electric fields by putting it side a hollow conductor. Can we shield a body from the gravitational influence of nearby matter by putting it inside a hollow sphere or by some other means?

Answer:

The electric force depends on the nature of the medium, whereas the gravitational force is independent of the medium. So, we cannot shield a body from the gravitational force.

Question:8.24

An astronaut inside a small spaceship orbiting around the earth cannot detect gravity. If the space station orbiting around the earth has a large size, can he hope to detect gravity?

Answer:

If the space station orbiting around the earth has a large size, then the astronaut inside can experience acceleration due to gravity at very close proximity to the moon.

Question:8.25

The gravitational force between a hollow spherical shell and a point mass is F. Show the nature of F vs r graph where r is the distance of the point from the centre of the hollow spherical shell of uniform density.
capture-825

Answer:

The gravitational force inside the hollow spherical shell is 0 and on the surface is F_{0}
F_{0}=\frac{GmM}{r^{2}} for r\ge R and F_{0}=0 for 0<r<R

Question:8.27

What is the angle between the equatorial plane and the orbital plane of
a) polar satellite?
b) geostationary satellite?

Answer:

(a) The orbital plane of polar satellite makes 90^{o} with equatorial plane.
(b) Geostationary satellite makes 0^{o} with the equatorial plane as it revolves from west to east.

Question:8.26

Out of aphelion and perihelion, where is the speed of the earth more and why?

Answer:

According to Kepler's second law
\frac{dA}{dt}=\frac{L}{2m}=\frac{r\; *\; p}{2m}=\frac{r\; *\: mv}{2m}=\frac{r\; *\; v}{2}
Since “r” at aphelion is more; the velocity decreases and “r” at perihelion are smaller; the velocity increases.

NCERT Exemplar Class 11 Physics Solutions Chapter 8: Short Answer

Question:8.28

Mean solar day is the time interval between two successive noon when the sun passes through zenith point. The sidereal day is the time interval between two successive transits of a distant star through the zenith point. By drawing the appropriate diagram showing earth’s spin and orbital motion, show that mean solar day is four minutes longer than the sidereal day. In other words, distant stars would rise 4 minutes early every successive day.

Answer:

When the earth revolves at its own axis by 360^{o} it changes its angle
PSQ=0^{o}
So, 361^{o} rotation by the earth is one solar day or 24 hrs
1=24*\frac{3600}{361}sec=3\; min\; 59\; sec\cong 4\; min
Thus, the distant start rises 4 min. early every day
capture-828

Question:8.29

Two identical heavy spheres are separated by a distance 10 times their radius. Will an object placed at the midpoint of the line joining their centres be in stable equilibrium or unstable equilibrium? Give a reason for your answer.

Answer:

m_{1}=m_{2}=M
r=10\; R
capture-829
mass Let m be placed at the midpoint P of the line joining centres of spheres A and B
\left | F_{2} \right |=\left | F_{1} \right |=\frac{GMm}{(5R)^{2}}=\frac{GMm}{25R^{2}}
Net force F_{1}+F_{2}=0
F_{1}=-F_{2}
If m is diplaced slightly by x from P to A
PA=(5R-x)\; and\; PB=(5R+x)
F_{1}=\frac{GMm}{(5R-x)^{2}}
F_{2}=\frac{GMm}{(5R+x)^{2}}
F_{2}<F_{1}
This results in an unstable equilibrium.

Question:8.30

Show the nature of the following graph for a satellite orbiting the earth.
a) KE vs orbital radius R
b) PE vs orbital radius R
c) TE vs orbital radius R

Answer:

capture-830
Mass of Earth = M_{E}
Radius of orbit of satellite=R
mass of satellite=m
v_{0}=\sqrt{\frac{GM}{R}}
E_{k}=\frac{1}{2}mv_{0}^{2}=\frac{1}{2}m\frac{GM}{R}=\frac{GMm}{2R}
E_{k}\; \alpha \; \frac{1}{R}
E_{p}=-\frac{GMm}{R}
E_{p}\; \alpha \; -\frac{1}{R}
TE=\frac{1}{2}\left ( \frac{GMm}{R} \right )-\frac{GMm}{R}=-\frac{1}{2}\left ( \frac{GMm}{R} \right )

Question:8.31

Shown are several curves. Explain with reason, which ones amongst them can be possible trajectories traced by a projectile

Answer:

Incomplete question. Provide image

Question:8.31

Shown are several curves. Explain with reason, which ones amongst them can be possible trajectories traced by a projectile
1622175479626

Answer:

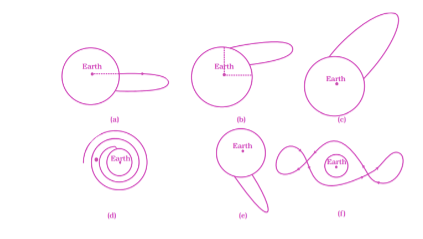
The trajectory of a projectile under gravitational force of earth should be a conic section or parabolic or elliptical with focus at the centre of the earth. Hence, c is correct.

Question:8.32

An object of mass m is raised from the surface of the earth to a height equal to the radius of the earth, that is, taken from a distance R to 2R from the centre of the earth. What is the gain in its potential energy?

Answer:

Let PE_{i} be the initial P.E when mass is at the surface of earth
Let PE_{f} be the final P.E when mass is lifted to height equal to R
PE_{i}=-\frac{GMm}{R}
PE_{f}=-\frac{GMm}{2R}
Gain in PE=PE_{f}-PE_{i}=-\frac{GMm}{2R}-\left (-\frac{GMm}{R} \right )=\frac{GMm}{2R}
GM=gR^{2}
Gain in PE=\frac{1}{2}mg\; R


Question:8.33

A mass m is placed at P a distance h along the normal through the centre O of a thin circular ring of mass M and radius r. If the mass is removed further away such that OP becomes 2h, by what factor the force of gravitation will decrease, if h = r?
capture-833

Answer:

Let us consider a small element of a ring of mass dM at point A and distance etween dM and m is x.
x^{2}=r^{2}+h^{2}
The gravitational force between dM and m, dF=\frac{G(dM)m}{x^{2}}
dF has 2 components: one along PO and another perpendicular to PO
\int \; \sin \theta =0 (due to symmetry of ring)
F=\int dF\; \cos \theta =\int \frac{G(dM)m}{x^{2}}\frac{h}{x}
=\frac{Gmh}{x^{3}}\int dM=\frac{GMmh}{x^{3}}=\frac{GMmh}{(r^{2}+h^{2})^{\frac{3}{2}}}
When the mass is displaced to a distance of 2 h F'=\frac{GMm(2h)}{(r^{2}+(2h)^{2})^{\frac{3}{2}}}=\frac{2GMmh}{(r^{2}+4h^{2})^{\frac{3}{2}}}
Where h =r F=\frac{GMmr}{(r^{2}+r^{2})^{\frac{3}{2}}}=\frac{GMm}{2\sqrt{2}r^{2}}
F'=\frac{GMmr}{(5r^{2})^{\frac{3}{2}}}=\frac{2GMm}{5\sqrt{5}r^{2}}
\frac{F^{'}}{F}=\frac{4\sqrt{2}}{5\sqrt{5}}
F'=\frac{4\sqrt{2}}{5\sqrt{5}}F

NCERT Exemplar Class 11 Physics Solutions Chapter 8: Long Answer

Question:8.34

A star like the sun has several bodies moving around it at different distances. Consider that all of them are moving in circular orbits. Let r be the distance of the body from the centre of the star and let its linear velocity be v, angular velocity \omega, kinetic energy K, gravitational potential energy U, total energy E, and angular momentum l. As the radius r of the orbit increases, determine which of the above quantities increase and which ones decrease.

Answer:

In equilibrium condition, the gravitational pull results in a centripetal force when a body moves around a star. Let us assume that a body of mass m is revolving around a star of mass M in a circle with radius r. Let us assume that a body of mass m is revolving around a star of mass M in a circle with radius r.
Orbital velocity
v_{0}=\sqrt{\frac{GM}{r}}\; or\; v_{0}\; \alpha\; \frac{1}{\sqrt{r}}
On the increasing radius of the circular path, orbital velocity decreases.
\omega =\frac{2\pi }{T}
T^{2}\; \alpha \; r^{3} (by Kepler's third law)
\omega =\frac{2\pi }{Kr^{\frac{3}{2}}}
\omega \; \alpha \; \frac{1}{\sqrt{r^{3}}}
On increasing the radius, angular velocity decreases.
E_{k}=\frac{1}{2}m\frac{GM}{r}
E_{k}\; \alpha \; \frac{1}{r}
On increasing the radius, the kinetic energy decreases.
Gravitation potential energy E_{p}=\frac{-GMm}{r}
E_{p}\; \alpha -\frac{1}{r}
On increasing the radius, P.E. increases.
Total Energy E=E_{k}+E_{p}=\frac{GMm}{2r}-\frac{GMm}{r}=-\frac{GMm}{2r}
Om increasing radius of the circular path the total energy also increases.
Angular momentum L=mvr=m\sqrt{\frac{GM}{r}}r
L=m\sqrt{GMr}
L\; \alpha \; \sqrt{r}
The increasing radius of the circular orbit increases angular momentum

Question:8.35

Six-point masses of mass m each are at the vertices of a regular hexagon of side l. Calculate the force on any of the masses.

Answer:

capture-835

Force on A due to B=f_{1}=\frac{Gmm}{l^{2}}=\frac{Gm^{2}}{l^{2}} along B to A

Force on A due to C=f_{2}=\frac{Gmm}{(\sqrt{3}l)^{2}}=\frac{Gm^{2}}{3l^{2}} along C to A

Force on A due to D =f_{3}=\frac{Gmm}{(2l)^{2}}=\frac{Gm^{2}}{4l^{2}} along D to A

Force on A due to E =f_{4}=\frac{Gmm}{(\sqrt{3}l)^{2}}=\frac{Gm^{2}}{3l^{2}} along E to A


F=F_{1}+F_{2}+F_{3}=\frac{Gm^{2}}{l^{2}}+\frac{Gm^{2}}{\sqrt{3}l^{2}}+\frac{Gm^{2}}{4l^{2}} along DA

Question:8.36

A satellite is to be placed in equatorial geostationary orbit around the earth for communication
a) calculate height of such a satellite
b) find out the minimum number of satellites that are needed to cover entire earth so that at least one satellites is visible from any point on the equator

Answer:

M=6\times10^{24}\; kg
R=6400\; km=6.4\times10^{6}m
Time \; Period \; (T)=24h=24\times3600s=24\times36\times10^{2}s
G=6.67\times10^{-11}Nm^{2}kg^{-2}
Orbital Radius = R+h
Orbital velocity is v_{0}=\sqrt{\frac{GM}{R+h}}\Rightarrow v_{0}^{2}=\frac{GM}{R+h}
T= \frac{2\pi (R+h)}{v_{0}}
T^{2}= \frac{4\pi^{2} (R+h)^{2}}{v_{0}^{2}}= \frac{4\pi^{2} (R+h)^{3}}{GM}
R+h=\left [ \frac{GT^{2}M}{4\pi^{2}} \right ]^{\frac{1}{3}}
h=\left [ \frac{GT^{2}M}{4\pi^{2}} \right ]^{\frac{1}{3}}-R
h=\left [ \frac{6.67\times10^{-11}\times(24\times36)^{2}\times(10^{2})^{2}\times6\times10^{24}}{4\times3.14\times3.14} \right ]^{\frac{1}{3}}-6.4\times10^{6}
h=35940\; km

Let a satellite S is at h m above the earth surface. Let the angle subtended by it at the centre of the earth be 2 \theta

\cos\; \theta =\frac{R}{R+h}=\frac{1}{[1+\frac{h}{R}]}

h=3.59\times10^{7}m (height of geostationary satellite)

\cos\; \theta =\frac{1}{[1+\frac{3.59\times10^{7}}{6.4\times10^{6}}]}=0.1515

\theta =81.28

2\; \theta =2\times 81.28

Number of satellites required to cover 360^{0}=\frac{360}{2\times 81.28}=2.21

Number of satellites required=3

Question:8.37

Earth’s orbit is an ellipse with eccentricity 0.0167. Thus, the earth’s distance from the sun and speed as it moves around the sun varies from day to day. This means that the length of the solar day is not constant throughout the year. Assume that earth’s spin axis is normal to its orbital plane and find out the length of the shortest and the longest day. A day should be taken from noon to noon. Does this explain the variation of the length of the day during the year?

Answer:

Perihelion distance for an ellipse of eccentricity e and semi-major axis a,r_{p}=a(1-e)
Aphelion distance r_{a}=a(1+e)
\frac{dA}{dt}=\frac{L}{2m}=const (Kepler's law)
capture-837
L_{a}=L_{p}
r_{a}\times p_{a}=r_{p}\times p_{p}
r_{a}\times mv_{a}=r_{p}\times mv_{p}
r_{a}\times m\omega _{a}r_{a}=r_{p}\times m\omega _{p}r_{p}
\omega _{a}r_{a}^{2}=\omega _{p}r_{p}^{2}
\frac{\omega _{p}}{\omega _{a}}=\left ( \frac{r_{a}}{r_{p}} \right )^{2}
\frac{\omega _{p}}{\omega _{a}}=\left ( \frac{a(1+e)}{a(1-e)} \right )^{2}
e=0.0167(given)
\frac{\omega _{p}}{\omega _{a}}=\frac{(1+0.0167)^{2}}{(1-0.0167)^{2}}=\left ( \frac{1.0167}{0.9833} \right )^{2}=1.0691
\frac{\omega _{p}}{\omega }\frac{\omega }{\omega _{a}}=1.0691 where \omega is the meanangular velocity of earth
\frac{\omega _{p}}{\omega }=\frac{\omega }{\omega _{a}}=\sqrt{1.0691}=1.034
If \omega is 1^{o} it corresponds to 1 day i.e., avg angular speed=1.034^{0} per day
and \omega _{a}0.9833^{0},\omega _{p}=1.0167^{0}
Since 361^{0} = 24 hrs mean solar day. We get 361.034^{0} which corresponds to 24 hrs 8.14'
This does not explain the actual variation of length of day during the year.

Question:8.38

A satellite is in an elliptic orbit around the earth with an aphelion of 6R and perihelion of 2R where R = 6400 km is the radius of the earth. Find eccentrically of the orbit. Find the velocity of the satellite at apogee and perigee. What should be done if this satellite has to be transferred to a circular orbit of radius 6R?

Answer:

r_{p}= 2R
r_{a}= 6R
r_{p}= a(1-e)=2R
r_{a}= a(1+e)=6R
\frac{1-e}{1+e}=\frac{2}{6}
3-3e=1+e
4e=2\Rightarrow e=\frac{1}{2}
L_{1}=L_{2}
m_{a}v_{a}r_{a}=m_{p}v_{p}r_{p}
m_{a}=m_{p}=m=mass\; of\; satellite
\frac{v_{a}}{v_{p}}=\frac{r_{p}}{r_{a}}=\frac{2R}{6R}=\frac{1}{3}
v_{p}=3v_{a}
Applying conservation of energy at apogee and perigee
\frac{1}{2}mv_{p}^{2}-\frac{GMm}{r_{p}}=\frac{1}{2}mv_{a}^{2}-\frac{GMm}{r_{a}}
Multiplying \frac{2}{m} to both side and putting r_{p}=2R and r_{a}=6R
v_{p}^{2}-\frac{2GM}{2R}=v_{a}^{2}-\frac{2GM}{6R}
v_{a}=\frac{v_{p}}{3}
v_{p}^{2}-v_{a}^{2}=\frac{GM}{R}-\frac{1}{3}\frac{GM}{R}
v_{p}^{2}-\left ( (\frac{v_{p}}{3})^{2} \right )=\frac{2GM}{3R}
v_{p}^{2}\left [ 1-\frac{1}{9} \right ]=\frac{2GM}{3R}
\frac{8}{9}v_{p}^{2}=\frac{2GM}{3R}
v_{p}^{2}=\frac{3GM}{4R}
v_{p}=\sqrt{\frac{3GM}{4R}}=\sqrt{\frac{3\times6.67\times10^{-11}\times6\times10^{24}}{4\times6.6\times10^{6}}}=\sqrt{46.89\times10^{6}}
v_{p}=6.85\; km/s
v_{a}=\frac{v_{p}}{3}=2.28\; km/s
v_{c}=\sqrt{\frac{GM}{r}}=\sqrt{\frac{GM}{6R}}=\sqrt{\frac{6.67\times10^{-11}\times6\times10^{24}}{6\times6.6\times10^{6}}}
v_{c}=3.23\; km/s
Thus, to transfer to a circular orbit at apogee, we have to boost the velocity by
v_{c}-v_{a}=(3.23-2.28)=0.95\; km/s

Class 11 Physics NCERT Exemplar solutions Chapter 8 includes the following topics:

Main Subtopics of NCERT Exemplar Class 11 Physics Solutions Chapter 8

  • 8.1 Introduction
  • 8.2 Kepler’s laws
  • 8.3 Universal law of gravitation
  • 8.4 The gravitational constant
  • 8.5 Acceleration due to gravity of the earth
  • 8.6 Acceleration due to gravity below and above the surface of the earth
  • 8.7 Gravitational potential energy
  • 8.8 Escape speed
  • 8.9 Earth satellites
  • 8.10 Energy of an orbiting satellite
  • 8.11 Geostationary and polar satellites
  • 8.12 Weightlessness

What will students learn from NCERT Exemplar Class 11 Physics solutions chapter 8? Here, the students will learn about the gravitational force and how it proves its existence on the inner and outer surface of the earth. Acceleration is the potential energy difference between the distances of two points on the earth’s surface from the centre. Acceleration increase or decrease is all-dependent upon the increase or decrease in altitude. NCERT Exemplar Class 11 Physics solutions chapter 8 focuses on many practical explanations when it comes to a clear understanding. The effect of gravity in the space limiting to the gravitational force of the earth is also discussed in the chapter. Astronauts, stars and the moon are all affected by the earth’s gravitational force.

NCERT Exemplar Class 11 Physics Solutions Chapter-Wise

NCERT Exemplar Class 11 Solutions


Read NCERT Class 11 Physics Solutions

Important Topics To Cover For Exams From NCERT Exemplar Class 11 Physics Solutions Chapter 8

Some of the important points are:

· Constant acceleration, about Galileo Galilei, explaining that the body being irrespective of their masses, is accelerated toward the earth’s surface with a constant acceleration.

Kepler’s law:

  • All planets move in elliptical orbits with the Sun at one of the focal points
  • The radius vector drawn from the sun to a planet sweeps out equal areas in equal time intervals. This follows from the fact that the force of gravitation on the planet is central and hence angular momentum is conserved.
  • (c) The square of the orbital period of a planet is proportional to the cube of the semi-major axis of the elliptical orbit of the planet.
JEE Main Highest Scoring Chapters & Topics
Just Study 40% Syllabus and Score upto 100%
Download EBook

Also, Read NCERT Solution subject wise -

Check NCERT Notes subject wise -

Check Class 11 Physics Chapter-wise Solutions

Also Check NCERT Books and NCERT Syllabus here

Frequently Asked Questions (FAQs)

1. Why should one prefer using Class 11 Physics NCERT Exemplar solutions Chapter 8 to solve their doubts?

NCERT Exemplar Class 11 Physics Solutions Chapter 8, do the experts solve a set of the solution; thus, being always reliable.

2. How helpful are these solutions?

The solutions are proven to be helpful for students to clear doubts and grasp knowledge easily. Based on the individual syllabus subject and our Subject Matter Expert and provide clear instructions throughout the learning process.

3. What are the topics covered in this chapter?

Here are the topics covered in this chapter

  • 8.1 Introduction
  • 8.2 Kepler’s laws
  • 8.3 Universal law of gravitation
  • 8.4 The gravitational constant
  • 8.5 Acceleration due to gravity of the earth
  • 8.6 Acceleration due to gravity below and above the surface of the earth
  • 8.7 Gravitational potential energy
  • 8.8 Escape speed
  • 8.9 Earth satellites
  • 8.10 Energy of an orbiting satellite
  • 8.11 Geostationary and polar satellites
  • 8.12 Weightlessness

Also Read

Articles

Upcoming School Exams

National Institute of Open Schooling 12th Examination

Admit Card Date:04 October,2024 - 29 November,2024

National Institute of Open Schooling 10th examination

Admit Card Date:04 October,2024 - 29 November,2024

Mizoram Board of School Education 12th Examination

Application Date:07 October,2024 - 22 November,2024

Mizoram Board 10th Examination

Application Date:07 October,2024 - 22 November,2024

Punjab Board of Secondary Education 10th Examination

Application Correction Date:08 October,2024 - 27 November,2024

View All School Exams
Get answers from students and experts

Popular Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1)

0.34\; J

 Option 2)

0.16\; J
Option 3)

1.00\; J

 Option 4)

0.67\; J
Read More

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times.  Assume that the potential energy lost each time he lowers the mass is dissipated.  How much fat will he use up considering the work done only when the weight is lifted up ?  Fat supplies 3.8×107 J of energy per kg which is converted to mechanical energy with a 20% efficiency rate.  Take g = 9.8 ms−2 :

Option 1)

2.45×10−3 kg

 Option 2)

 6.45×10−3 kg
Option 3)

 9.89×10−3 kg

 Option 4)

12.89×10−3 kg

 
Read More

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1)

2,000 \; J - 5,000\; J

 Option 2)

200 \, \, J - 500 \, \, J
Option 3)

2\times 10^{5}J-3\times 10^{5}J

 Option 4)

20,000 \, \, J - 50,000 \, \, J
Read More

A particle is projected at 600   to the horizontal with a kinetic energy K. The kinetic energy at the highest point

Option 1)

K/2\,

 Option 2)

\; K\;
Option 3)

zero\;

 Option 4)

K/4
Read More

In the reaction,

2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}

Option 1)

11.2\, L\, H_{2(g)}  at STP  is produced for every mole HCL_{(aq)}  consumed

 Option 2)

6L\, HCl_{(aq)}  is consumed for ever 3L\, H_{2(g)}      produced
Option 3)

33.6 L\, H_{2(g)} is produced regardless of temperature and pressure for every mole Al that reacts

 Option 4)

67.2\, L\, H_{2(g)} at STP is produced for every mole Al that reacts .
Read More

How many moles of magnesium phosphate, Mg_{3}(PO_{4})_{2} will contain 0.25 mole of oxygen atoms?

Option 1)

0.02

 Option 2)

3.125 × 10-2
Option 3)

1.25 × 10-2

 Option 4)

2.5 × 10-2
Read More

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1)

decrease twice

 Option 2)

increase two fold
Option 3)

remain unchanged

 Option 4)

be a function of the molecular mass of the substance.
Read More

With increase of temperature, which of these changes?

Option 1)

Molality

 Option 2)

Weight fraction of solute
Option 3)

Fraction of solute present in water

 Option 4)

Mole fraction.
Read More

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol-1) is

Option 1)

twice that in 60 g carbon

 Option 2)

6.023 × 1022
Option 3)

half that in 8 g He

 Option 4)

558.5 × 6.023 × 1023
Read More

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t2) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m2 , the number of rotations made by the pulley before its direction of motion if reversed, is

Option 1)

less than 3

 Option 2)

more than 3 but less than 6
Option 3)

more than 6 but less than 9

 Option 4)

more than 9
Read More

Colleges After 12th

Popular Course After 12th

Applications for Admissions are open.

VMC VIQ Scholarship Test
Apply

Register for Vidyamandir Intellect Quest. Get Scholarship and Cash Rewards.

JEE Main Important Physics formulas
Apply

As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters

JEE Main Important Chemistry formulas
Apply

As per latest 2024 syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters

TOEFL ® Registrations 2024
Apply

Accepted by more than 11,000 universities in over 150 countries worldwide

Pearson | PTE
Apply

Register now for PTE & Unlock 20% OFF : Use promo code: 'C360SPL20'. Valid till 30th NOV'24! Trusted by 3,500+ universities globally

JEE Main high scoring chapters and topics
Apply

As per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE

View All Application Forms
News and Notifications
MP: Private schools to pay back illegally collected tuition fees worth Rs 31 crores
Tamil Nadu teacher fatally stabbed in school, violence erupts; assailant detained
Bihar govt puts hold on new teachers transfer policy
Youth stabs teacher on school premises; now detained, says police
Air Pollution: Schools in Rajasthan's Khairthal-Tijara go online for classes 1 to 5
Govt informs SC about NCERT guidelines for development of e-content for children with disabilities
Government school principal in Andhra chops off girl students' hair for coming late
Back to top