NCERT Solutions for Class 11 Physics Chapter 6 System Of Particles And Rotational Motion
When a rubber ball is compressed, it alters its shape; when a metal rod is bent under pressure, it becomes deformed. In Physics, however, to make things simpler, we frequently consider such objects to be rigid bodies that neither change shape in response to forces exerted on them. With this assumption, we could simplify the study of their motion. Chapter 6, Class 11 Physics System of Particles and Rotational Motion presents students with some of the most significant concepts of mechanics, such as: torque, angular momentum, moment of inertia, and rotational dynamics. These principles are the foundation of the knowledge of motion and rotation of bodies in the real world.
This Story also Contains
- System of Particles and Rotational Motion Class 11 Question Answers: Download PDF
- System of Particles and Rotational Motion NCERT Solutions: Exercise Questions
- System of Particles and Rotational Motion NCERT Solutions: Higher Order Thinking Skills (HOTS) Questions
- Class 11 Physics Chapter 6 - System of Particles and Rotational Motion: Topics
- System of Particles and Rotational Motion NCERT Solutions: Key Formulas
- Approach to Solve Questions of Class 11 Physics Chapter 6 - System of Particles and Rotational Motion
- What Extra Should Students Study Beyond NCERT for JEE/NEET?
- Importance of NCERT Solutions for Class 11 Physics Chapter 6 - System of Particles and Rotational Motion
- NCERT Solutions for Class 11 Physics Chapter-Wise
The NCERT Solutions for Class 11 Physics Chapter 6 - System of Particles and Rotational Motion are well organised and prepared by subject experts so that it can give a step-by-step explanation of all concepts, derivations, formulas and numerical problems. These resources, which contain NCERT solutions to in-text and exercise questions in detail, simplify even complex concepts further and allow understanding and application. The chapter is very important not only to CBSE board exams but also to competitive exams such as JEE and NEET, where questions on rotational motion are often asked. Regular practice with NCERT Solutions for Class 11 Physics Chapter 6 - System of Particles and Rotational Motion assists the students to improve upon their basics, perform well in school examinations, and gain a strong foundation to continue their education in physics.
System of Particles and Rotational Motion Class 11 Question Answers: Download PDF
The Class 11 Physics Chapter 6 - System of Particles and Rotational Motion question answers provide solutions to all the questions in the book, and teach students what the centre of mass is, how torque and angular momentum work, and what determines the rotational dynamics. The PDF version can be downloaded and works well in helping to revise as well as prepare for both board exams and competitive exams like JEE and NEET.
System of Particles and Rotational Motion NCERT Solutions: Exercise Questions
System of Particles and Rotational Motion class 11 question answers enable the students to understand the important concepts such as centre of mass, torque, angular momentum and rotational dynamics. These are step-wise solutions that will help understand concepts, sharpen problem-solving and also equip students for school tests and competitive exams such as JEE and NEET.
Answer:
For uniform mass density, the location of the centre of mass is the same as that of the geometric centre.
No, it is not necessary that the centre of mass lies inside the body. For example, in the case of a ring, the centre of mass is outside the body.
Answer:
Let us assume that the centre of mass of the molecule is x cm away from the chlorine atom.
So, we can write
or
Here negative sign indicates that the centre of mass lies leftward of the chlorine atom.
Answer:
Since the child and trolley are considered in a system, the speed of the centre of mass changes only when an external force acts on the system. When the child starts to move on the trolley, the force produced is the internal force of the system, so this will produce no change in the velocity of the CM.
Q6.4 Show that the area of the triangle contained between the vectors
Answer:
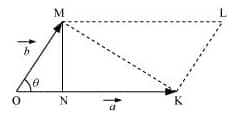
Let a and b be two vectors having an angle θ between them.
Consider
or
or
Therefore, the area of
Answer:
A parallelepiped is shown in the figure given below:-
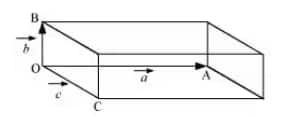
Volume is given by: abc
We can write :
Now,
This is equal to the volume of a parallelepiped.
Q6.6 Find the components along the
Answer:
Linear momentum of a particle is given by :
And the angular momentum is :
When a particle is confined to the x-y plane, then z = 0 and pz = 0.
When we put the value of z and p z in the equation of linear momentum, then we observe that only the z component is non-zero.
Answer:
Assume two points (say A, B) separated by distance d.
So the angular momentum of a point about point A is given by:
And about point B :
Now, assume a point between A and B as C, which is at a distance y from point B.
Now the angular momentum becomes :
Thus, it can be seen that angular momentum is independent of the point about which it is measured.
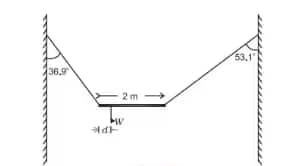
Answer:
The FBD of the given bar is shown below :
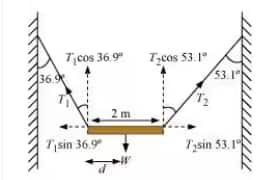
Since the bar is in equilibrium, we can write :
or
or
For the rotational equilibrium :
or
Thus, the centre of gravity is at 0.72 m from the left.
Answer:
The FBD of the car is shown below :
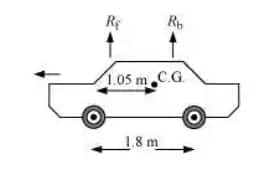
We will use the conditions of equilibrium here :
For rotational equilibrium :
or
From (i) and (ii), we get :
and
Thus force exerted by the front wheel is = 3675 N
and force exerted bythe back wheel = 5145 N.
Answer:
The moment of inertia of a hollow cylinder is given by
And the moment of inertia of a solid cylinder is given by:
We know that:
Let the torque for the hollow cylinder be
According to the question:
So we can write the ratio of the angular acceleration of both objects.
Now for angular velocity,
Clearly, the angular velocity of the solid sphere is more than the angular velocity of the hollow sphere. (As the angular acceleration of a solid sphere is greater).
Answer:
Firstly, we will calculate the moment of inertia of the solid cylinder:
So the kinetic energy is given by :
And the angular momentum is given by:
Answer:
We are given the initial angular speed and the relation between the moment of inertia of both cases.
Here we can use conservation of angular momentum as no external force is acting on the system.
So we can write :
Answer:
The final and initial velocities are given below :
Taking the ratio of both, we get,
or
or
Thus, the final energy is 2.5 times the initial energy.
The increase in energy is due to the internal energy of the boy.
Answer:
The moment of inertia is given by :
or
And the torque is given by :
Also,
So
And the linear acceleration is
Answer:
The relation between power and torque is given by :
or
Hence, the required power is 36 KW.
Answer:
Let the mass per unit area of the disc be
So total mass
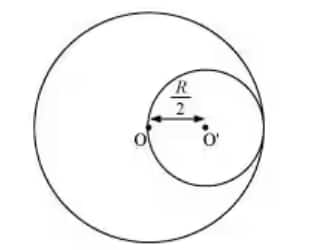
Mass of the smaller disc
Now, since the disc is removed, we can assume that part to have negative mass with respect to the initial condition.
So, the centre of mass of the disc is given by the formula :
Or
Or,
Hence, the centre of mass is shifted
Answer:
The centre of mass of the meter stick is at 50 cm.
Let the mass of the meter stick be m.
Now, according to the situation given in the question, we will use the rotational equilibrium condition at the centre point of the meter stick.
or
Thus, the mass of the meter stick is 66 g.
Answer:
We are given the moment of inertia and the velocity of the molecule.
Let the mass of an oxygen molecule be m.
So the mass of each oxygen atom is given by:
Moment of inertia is :
or
or
We are given that :
or
or
or
System of Particles and Rotational Motion NCERT Solutions: Higher Order Thinking Skills (HOTS) Questions
The Higher Order Thinking Skills (HOTS) questions of Class XII Physics Chapter 6: System of Particles and Rotational Motion are developed in such a way that they enhance theoretical knowledge, as well as assess the application of learning. These questions can be much more than simple problem-solving ones, and invite the students to analyse, evaluate and apply concepts of rotational motion and centre of mass to new and complicated scenarios, which are perfect to practice a competitive exam such as JEE.
Question 1) A solid sphere of mass
1)
2)
3)
4)
Answer:
For a rolling solid sphere, the total kinetic energy is the sum of translational and rotational kinetic energy:
For a solid sphere, the moment of inertia about its centre is:
Using the rolling without slipping condition,
Substituting
So, the total kinetic energy:
At maximum height, all kinetic energy converts into gravitational potential energy:
Equating energy:
Hence, the answer is the option (3).
Question 2) A rod starts rotating with angular velocity
1)
2)
3)
4)
Answer:
We know
Hence, the answer is the option (2).
Question 3) A wedge of mass M=2kg carries two blocks A and B, as shown in the figure. Block A lies on a smooth-incline surface of the wedge inclined at an angle
Answer:
Let the displacement of the wedge be
Displacement of A w.r.t wedge (on the incline) is
The COM of the entire system suffers no displacement in the horizontal direction.
Now, putting
Hence, the answer is 0.25.
Question 4)
A sphere is released on a smooth inclined plane from the top. When it moves down, its angular momentum is:
1) Conserved about every point
2) Conserved about the point of contact only
3) Conserved about the centre of the sphere only
4) Conserved about any point on a line parallel to the inclined plane and passing through the centre of the ball.
Answer:
As the inclined plane is smooth, the sphere can never roll; rather, it will just slip down. Hence, the angular momentum remains conserved at any point on a line parallel to the inclined plane and passing through the centre of the ball.
Hence, the answer is the option (4).
Question 5) A square plate of mass
Answer:
The torque by friction will be
Hence, angular acceleration will be
Now, as it is hinged at one corner, we can assume a plate of double-sided length
Now this is again the central axis, the value of angular acceleration will be half.
Hence, it will take
Hence, the answer is 2.
Class 11 Physics Chapter 6 - System of Particles and Rotational Motion: Topics
In Class 11 Physics Chapter 6 - System of Particles and Rotational Motion, the topics that are covered deal with centre of mass, motion of system of particles, torque, angular velocity, angular momentum and rotational dynamics. These topics constitute the basis of learning complex physical systems and are of great value in board exams and competitive exams such as JEE and NEET.
6.1 Introduction
6.1.1 What Kind Of Motion Can A Rigid Body Have?
6.2 Centre Of Mass
6.3 Motion Of the Centre Of Mass
6.4 Linear Momentum Of A System Of Particles
6.5 Vector Product Of Two Vectors
6.6 Angular Velocity And Its Relation With Linear Velocity
6.6.1 Angular Acceleration
6.7 Torque And Angular Momentum
6.7.1 Moment Of Force (Torque)
6.7.2 Angular Momentum Of A Particle
6.8 Equilibrium Of A Rigid Body
6.8.2 Centre Of Gravity
6.9 Moment Of Inertia
6.10 Kinematics Of Rotational Motion About A Fixed Axis
6.11 Dynamics Of Rotational Motion About A Fixed Axis
6.12 Angular Momentum In Case Of Rotation About A Fixed Axis
6.12.1 Conservation Of Angular Momentum
System of Particles and Rotational Motion NCERT Solutions: Key Formulas
System of Particles and Rotational Motion class 11 question answers: Key Formulas include important equations related to centre of mass, torque, moment of inertia, angular momentum, and rotational kinetic energy. These formulas act as quick revision tools and help students solve numerical problems efficiently in exams like CBSE, JEE, and NEET.
1. Centre of mass
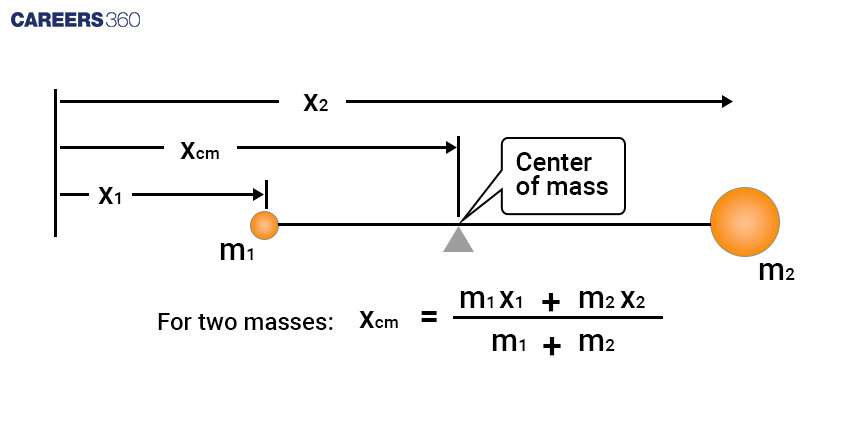
For a system of particles:
2. Motion of COM
3. Angular Velocity & Linear Velocity
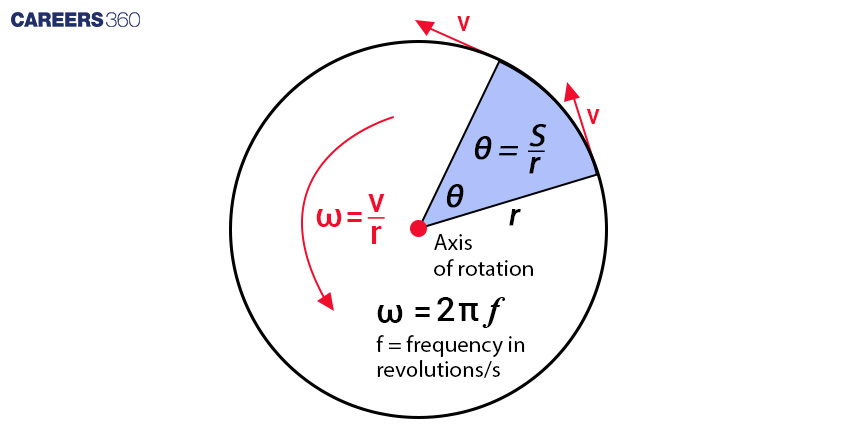
Average angular velocity =∆θ/∆t
Instantaneous angular velocity, ω=dθ/dt
4. Moment of Inertia (MI)
5. Moment of Inertia of a few useful configurations
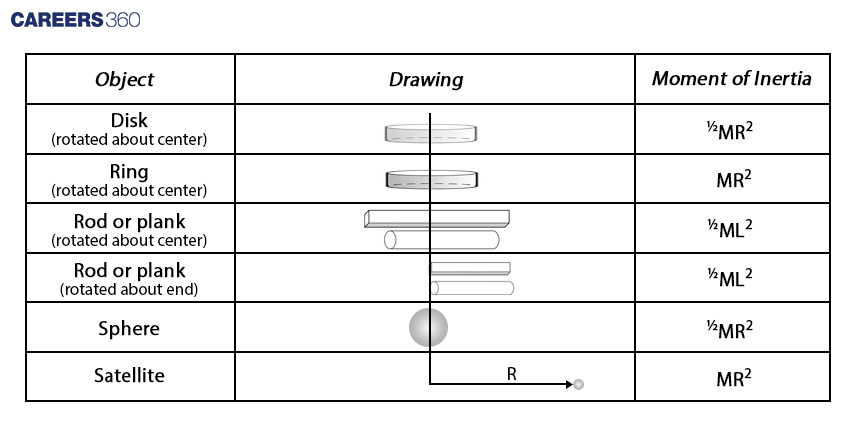
6. Torque (Moment of Force)
7. Angular Momentum
For a rotating body:
8. Torque-Angular Acceleration Relation
9. Rotational Kinetic Energy
10. Angular Equations of Motion (Uniform Angular Acceleration)
Approach to Solve Questions of Class 11 Physics Chapter 6 - System of Particles and Rotational Motion
In Physics, Chapter 11, System of Particles and Rotational Motion, Chapter 6 is concerned with the motion of rigid bodies, torque, angular momentum and moment of inertia. This chapter requires conceptual clarity and practice in problem-solving methods to address the questions effectively in this chapter. The systematic approach can be used to make sure that there are no inaccuracies in derivations, numericals, and applications.
- Knowledge of Rigid Body Assumption: It is always good to start by noting that the chapter assumes that the bodies are rigid (no deformation). This makes the problems of torque and rotational motion easier.
- Divide Problems into Translational and Rotational: When solving, separate linear motion (using Newton's laws) and rotational motion (using torque = Iα). They should only be combined when necessary.
- Use Vector Representation: Appropriate rules of vectors should be applied to answer questions on torque, angular momentum and cross products. Practice right-hand rule applications for direction.
- Learn and Use Theorems of Inertia: The theorems which are commonly applied in numerical questions are the parallel axis and the perpendicular axis theorems. Use them directly in practice in order to save time during exams.
- Study Motion About the Center of Mass (COM): In problem of particle systems find the COM first. Simplification can then be made on motion of the entire system by taking translation + rotation of a system about the COM.
- Focus on Derivations and Proofs: Derivations such as the correlation between torque and angular momentum, kinetic energy in rotation and rolling motion are common exam problems to be prepared.
- Connect with Real-Life Applications: Comparing rotational motion to real-life examples such as wheels, flywheels, ceiling fans or gyroscopes can be beneficial when it comes to writing a better response to an explanation.
What Extra Should Students Study Beyond NCERT for JEE/NEET?
To crack JEE, one must get out of NCERT and must practice higher level conceptual problems of torque, rotational motion of Chapter 6: System of Particles and Rotational Motion and rolling motion. Answering difficult questions and practising past year JEE questions will enable you to brush through the depth of knowledge needed to crack competitive exams.
Importance of NCERT Solutions for Class 11 Physics Chapter 6 - System of Particles and Rotational Motion
Rotational motion is a concept that students find difficult to understand because it entails the use of abstract concepts and mathematics. In an effort to eliminate these challenges, Class 11 Physics Chapter 6 - System of Particles and Rotational Motion question answers could be a good source of help that will provide a clear explanation and the correct answers. These solutions are useful not only in explaining how to solve the problems step by step but also in bringing out the key formulas and derivations that are very important in exams. They serve as the mediator between theory and practice making sure that students are both precise and quick in answering questions.
- Conceptual Clarity: The solutions provide a clear and detailed explanation of important concepts like the centre of mass, moment of inertia, and rotational motion, helping students develop a strong conceptual foundation.
- Step-by-Step Solutions: Each problem is solved step by step, making it easier for students to understand the methods and approaches used to tackle complex problems.
- Boosts Problem-Solving Skills: By practising the problems from the solutions, students improve their analytical and problem-solving skills, which are essential for both board exams and competitive tests.
- Reinforces Core Concepts: The solutions help reinforce key topics such as Newton's laws in rotational motion, torque, and angular momentum, which are essential for mastering the chapter.
- Effective Exam Preparation: These solutions are designed to help students prepare efficiently for exams by covering all important topics and providing a strong grasp of the subject.
- Quick Revision: The solutions are concise and well-organized, making them a great resource for quick revision before exams.
In summary, the NCERT solutions for Class 11 Physics help students effectively understand and solve problems in the chapter, strengthening their knowledge and enhancing their performance in exams.
Also, Check NCERT Books and NCERT Syllabus here
NCERT Solutions for Class 11 Physics Chapter-Wise
The NCERT Solutions to Class 11 Physics are available in chapter-wise format to have inexpensive, step-by-step solutions to each chapter. The links are useful in revising major concepts, doing numerical problems, and preparing a sound base on exams such as CBSE, JEE, and NEET. All solutions are designed by professionals according to the new syllabus.
NCERT solutions for class 11 Subject-wise
Frequently Asked Questions (FAQs)
It is about constraint on the motion of rigid bodies, rotation about a fixed axis and concepts such as torque, moment of inertia and angular momentum.
By assuming rigidity, it eliminates complications of deformation hence making the analysis easy.
Linear motion is the movement in a straight direction and rotational is the turning around an axis.
Yes it bears the same role in rotational motion as mass does in linear motion.
The most common example includes rotating wheels, ceiling fans, spinning tops, and rotating planets.
Popular Questions
Courses After 12th
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters


