Waves Class 11th Notes - Free NCERT Class 11 Physics Chapter 15 Notes - Download PDF

NCERT Notes for Class 11 Chapter 14: Download PDF

Access the PDF version of Class 11 Waves NCERT Notes by clicking on the download button. Using the PDF version, you can view the notes offline and revise the essential concepts at your own convenience.

Download PDF

NCERT Notes for Class 11 Chapter 14

Waves NCERT Notes by Careers360 is structured according to the latest CBSE syllabus and gives precise explanations of all the topics. Using these notes, students can prepare as well as revise for their exams.

Types of Waves

• The different types of waves can be broadly classified into three categories-

1. Mechanical waves: Mechanical waves can only be created or propagated in a material medium. Newton's laws of motion apply to these waves. For example waves on the water's surface, waves on strings, sound waves, and so on.

2. Electromagnetic Waves: These are waves that are generated and propagated without the usage of a material medium, such as vacuum or any other material medium. Visible light, ultraviolet light, radio waves, and microwaves are examples of electromagnetic waves.

3. Matter waves: These waves are related with moving matter particles such as electrons, protons, and neutrons, among others.

• Furthermore, based on the direction of vibration, waves can be divided into two types-

1. Transverse wave motion: In transverse waves, the particles of the medium vibrate at right angles to the wave's propagation direction. String waves, surface water waves, and electromagnetic waves are examples of transverse waves. The oscillation of electric and magnetic fields at right angles to the wave's travel direction causes the disturbance that travels in electromagnetic waves (which includes light waves).

2. Longitudinal wave motion: In these types of waves, particles in the medium vibrate back and forth about their mean location along the energy propagation direction. Pressure waves are another name for them. Sound waves are mechanical waves that travel along a longitudinal axis.

Characteristics of Waves

• Characteristics of waves are-

(i) The particles in the medium traversed by a wave vibrate very slightly around their mean positions, but they are not permanently displaced in the propagation direction of the wave.

(ii) Each subsequent particle of the medium performs a motion that is nearly identical to its predecessors when viewed perpendicular to the wave's path of travel.

(iii) During wave motion, only energy is transferred, but not a piece of the medium.

• Wavelength: The wavelength is the distance travelled by the disturbance in the time it takes a medium particle to vibrate once. In the case of a transverse wave, a wavelength can alternatively be defined as the distance between two subsequent crests or troughs. A longitudinal wave's wavelength is equal to the distance between the centres of two compressions (or refractions).

• Amplitude: The largest displacement of the medium's particles from their mean position is the amplitude of a wave.

• Frequency: Frequency is defined as the number of vibrations produced by a particle in one second.

Unit of frequency - Hertz(Hz)

• Time Period: Time period refers to how long it takes a particle to complete one vibration.

• Wave Velocity: The time rate at which wave motion propagates in a given medium is referred to as wave velocity. It's not the same as particle velocity. The type of the medium determines the wave velocity.

Wave velocity=frequency $\times$ wavelength

1. In a stretched string, the velocity of transverse waves is given by

$v=\sqrt{\frac{T}{\mu}}$

where T be the tension in the string and μ be the mass per unit length of the string,

2. In an elastic material, the longitudinal wave velocity is given by

$v=\sqrt{\frac{E}{\rho}}$

where E is the modulus of elasticity of the medium and ρ is the density of the medium

Newton’s Formula:

• According to Newton, when sound waves travel through air or a gaseous medium, they undergo isothermal change, and therefore it is found that

$v=\sqrt{\frac{B_{\text {isothermal }}}{\rho}}=\sqrt{\frac{P}{\rho}}$

• According to Newton's formula, the speed of sound in air at STP conditions is 280 ms^-1. The empirically determined values, on the other hand, are 332 m/s.
• According to Laplace, the change occurs under adiabatic conditions during sound wave transmission because gases are thermal insulators and compressions and refractions alternate at a high frequency.

Factors Influencing Velocity of Sound

• The velocity of sound in any gaseous medium is influenced by many factors such as density, pressure, temperature, humidity, wind velocity, and so on.
• In a gaseous state: The velocity of sound is inversely proportional to the square root of the gas's density.

(ii) If the temperature remains constant, the velocity of sound is unaffected by changes in gas pressure.

(iii) In a gas, sound velocity is proportional to the square root of the absolute temperature.

(iv) The sound velocity in moist air is larger than the sound velocity in dry air.

(v) If the wind is blowing at an angle θ to the sound propagation direction, the sound velocity is v+wcosθ, where w is the wind velocity.

General Equation of Progressive Waves

• A progressive wave travels in a given direction with constant amplitude.
• Because displacement is a function of both space and time in wave motion, the displacement relation is stated as a combination of position and time as:

$y(x, t)=A \sin (k x-\omega t+\phi)$

Relation Between Phase Difference And Path Difference

Phase difference= $(2 \pi / \lambda) \times$ path difference

The Principle of Superposition of Waves

The net displacement at a given time is the algebraic total of the displacements attributable to each wave at that moment when any number of waves meet concurrently at a point in a medium.

If y₁ and y₂ denote the movement of a particle caused by two separate waves. The resultant displacement at each point in the medium and at each instant of time is thus given by-

$y-y_1+y_2$

Standing waves or Stationary waves

A new set of waves is created when two sets of progressive wave trains of the same kind (both longitudinal or both transverse) with the same amplitude and time period/frequency/ wavelength traveling at the same speed in opposite directions superimpose. These are referred to as stationary or standing waves.

The equation of a standing wave can be represented as follows:

$y=2 A \sin k x \cos \omega t$

Progressive Waves

1. The disturbance continues to spread, passing from particle to particle. Each particle vibrates in the same way as the one before it, but at a different rate.

2. The waves have crests and troughs, which are sine/cosine functions that travel with a specified velocity.

3. Each particle has the same amplitude, which it achieves in its own time as the wave progresses.

4. Every particle's phase changes from 0 to 2π on a constant basis.

5. No particle can be said to be at rest indefinitely. The particles are momentarily at rest twice during each vibration. At different moments, different particles reach this point.

6. All particles have the same maximum velocity, which they achieve one by one as the wave progresses.

7. Energy flows in a predictable pattern across all planes in the wave's propagation path.

Stationary Waves

1. The disturbance is stationary, as the wave does not move forward or backward. Each particle has its own set of vibrational properties.

2. In each vibration, the waves resemble a sine/cosine function that shrinks to a straight line twice. It never moves forward.

3. Each particle has a specific amplitude. Some have no amplitude (nodes), whereas others constantly have maximum amplitude (anti nodes). This is consumed by each participant at the same time.

4. One-half of the waves' particles have a fixed phase, while the other half of the waves' particles have the same phase in the opposite direction at the same time.

5. There are particles that are always at rest (nodes), and all other particles have a maximum movement that they all reach at the same time. In each vibration, these particles are momentarily at rest twice, all at the same time.

6. At the same time, all of the particles achieve their individual assigned velocities based on their positions. Two particles (nodes) in a wave form have constant zero velocities.

7. There is no energy movement in any direction across any plane. Each particle is given its own amount of energy. They all reach their RE. values at the same moment, and all energy becomes KB. at a later time.

8. Closed pipe and open pipe: In a closed pipe, one end is closed while the other is open. In an open pipe, both the ends are open, having anti nodes.

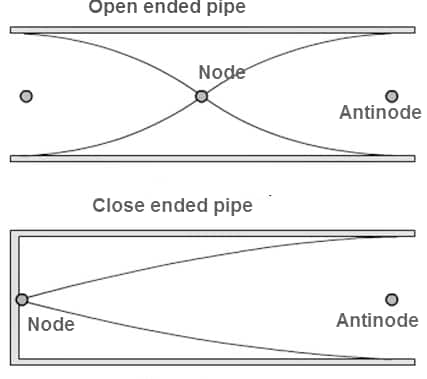
In a closed pipe, frequency of nth harmonic is fn=nv/4L where v is velocity, L is length

In a open pipe, frequency of nth harmonic is fn=nv/2L

Frequency of the Stretched String

• In general, if the string vibrates in P loops, its frequency is given by

$v=\frac{P}{2 L} \sqrt{\frac{T}{\mu}}$

• Three rules of transverse vibrations of stretched strings emerge from this relationship. They are the laws of length, tension, and mass.

1. Law of Length: $v \propto \frac{1}{L}$

2. Law of Tension: $v \propto \sqrt{T}$

3. Law of Mass: $v \propto \frac{1}{\sqrt{\mu}}$

Beats

• Beats are the regular increase and fall in sound intensity that occurs when two waves of almost equal frequencies move along the same line and in the same direction and superimpose each other.
• One beat is defined as a rise and fall in the intensity of sound, and the number of beats per second is known as beat frequency. It is written as follows:

$v_b=\left(v_1-v_2\right)$

Waves Previous year Question and Answer

Q1: Which of the following statements are true for a stationary wave?

a) every particle has a fixed amplitude which is different from the amplitude of its nearest particle

b) all the particles cross their mean position at the same time

c) all the particles are oscillating with the same amplitude

d) there is no net transfer of energy across any plane

e) there are some particles which are always at rest

Answer:

In a stationary wave, the particles between two nodes vibrate with different amplitude, which increases from node to antinode (0 to maximum) and decreases from antinode to node. The amplitude of particles varies with $\lambda$. The particles at a node are at rest, and hence there is no net transfer of energy. The particle between two nodes is in the same phase. The motion of particles between two nodes will be either upward or downward crossing the mean position at the same time. Hence, statements a,b,d and e are correct.

Q2: The speed of sound waves in a fluid depends upon

(a) directly on the density of the medium.
(b) square of the Bulk modulus of the medium.
(c) inversely on the square root of density.
(d) directly on the square root of the bulk modulus of the medium.

Answer:

The speed of sound wave in the fluid of bulk modulus K and density $\rho$ is given by

$
\begin{aligned}
& v=\sqrt{\frac{K}{\rho}} \\
& v \propto \sqrt{K} \text { (if } \rho \text { is constant) } \\
& v \propto \sqrt{\frac{1}{\rho}} \text { (if } \mathrm{K} \text { is constant) }
\end{aligned}
$

Hence, (c) and (d) options are correct.

Q3: A transverse harmonic wave on a string is described by $y(x, t)=3.0 \sin \left(36 t+0.018 x+\frac{\pi}{4}\right)$ where x and y are in cm and t is in s. The positive direction of x is from left to right.

(a) The wave is travelling from right to left.
(b) The speed of the wave is $20 \mathrm{~m} / \mathrm{s}$.
(c) The frequency of the wave is 5.7 Hz.
(d) The least distance between two successive crests in the wave is 2.5 cm.

Answer:

The standard form of a wave propagating in a positive direction

$
\begin{aligned}
& y=a \sin (\omega t-k x+\phi) \text { and } \\
& y=3.0 \sin \left(36 t+0.018 x+\frac{\pi}{4}\right)
\end{aligned}
$
A positive sign in the equation shows that the wave is travelling from right to left.

$\omega=36 ; 2 \pi \nu=36 \\$

$or \ v=\frac{36}{2 \pi}=\frac{18}{3.14}=5.7 \mathrm{~Hz} \\$
$ k=0.018=\frac{2 \pi}{\lambda} \\$
$ \lambda=\frac{2 \pi}{0.018} \\$
$ v=\nu \lambda=\frac{18}{\pi} \times \frac{2 \pi}{0.018}=\frac{2000 \mathrm{~cm}}{s}=\frac{20 \mathrm{~m}}{s}$
Distance between two successive crests $=\lambda=\frac{2 \pi}{0.018}=\frac{\pi}{0.009}=3.14 \times \frac{1000}{9}=\frac{3140}{9} \mathrm{~cm}=348.8 \mathrm{~cm}$

Hence, (a), (b) and (c) are the correct options.

NCERT Class 11 Notes Chapter-Wise

Subject-Wise NCERT Exemplar Solutions

• NCERT Exemplar Class 11 Solutions
• NCERT Exemplar Class 11 Maths
• NCERT Exemplar Class 11 Physics
• NCERT Exemplar Class 11 Chemistry
• NCERT Exemplar Class 11 Biology

Subject-Wise NCERT Solutions

• NCERT Solutions for Class 11 Mathematics
• NCERT Solutions for Class 11 Chemistry
• NCERT Solutions for Class 11 Physics
• NCERT Solutions for Class 11 Biology

NCERT Books and Syllabus