Kinetic Theory Class 11th Notes - Free NCERT Class 11 Physics Chapter 13 Notes - Download PDF

NCERT Notes for Class 11 Chapter 12: Download PDF

Use the PDF version of the NCERT Notes of Kinetic Theory for revision at any time and anywhere, without requiring internet. Download using the button given below and save it near your fingertips for later study.

Download PDF

NCERT Notes for Class 11 Chapter 12

The Kinetic Theory NCERT Notes are crucial for exam preparation and help students gain a better understanding. These notes contain the important derivations and concepts required for exams. Students can use the notes to prepare as well as to review their knowledge.

Properties of Gases

Some properties of gases

• Gases can be compressed easily.

• Gases have neither a definite volume nor a definite shape.

• When a substance is in the gaseous state, its density is very small in comparison to the density in its solid or liquid state.

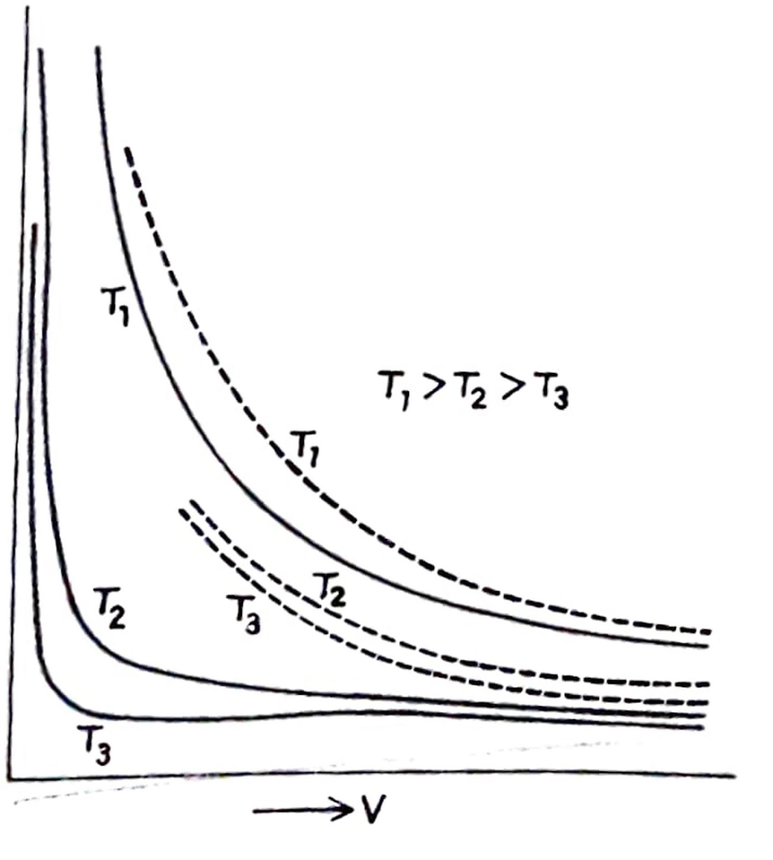
Boyle’s Law

• At constant temperature, the volume V of a given mass of a gas is inversely proportional to its pressure P, according to Boyle's law

$\begin{aligned} & V \propto \frac{1}{P} \\ & P V=\text { constant }\end{aligned}$

• If P₁ and V₁ are the initial pressure and volume, and P₂ and V₂ are the final pressure and volume for a given mass of a gas at constant temperature, then

$P_1 V_1=P_2 V_2$

• A graph is drawn between P and V for a gas at different constant temperatures T₁, T₂, and T₃ (T₁> T₂>T₃).
• The gases do not obey Boyle’s law strictly under all conditions of pressure and temperature, gas only obey Boyle’s law only at low pressures and high temperatures.

Charles’ Law

• At constant pressure, the volume of the given mass of a gas increases by $\frac{1}{273} t h$ part of its volume at 0℃ for 1℃ rise in temperature is called Charles's law.

$V_t=V_0\left(1+\frac{t}{273}\right)$

• Charles’ Law in terms of absolute temperature:- Let at constant pressure, the volume of a given mass of a gas at 0℃, t₁℃ and t₂℃ are V₀, V₁ and V₂ respectively. T₁ and T₂ are the absolute temperatures corresponding to t₁℃ and t₂ ℃. By Charles' Law

$\begin{aligned} & V_1=V_0\left(1+\frac{t_1}{273}\right)=V_0\left(\frac{273+t_1}{273}\right) \\ & V_2=V_0\left(1+\frac{t_2}{273}\right)=V_0\left(\frac{273+t_2}{273}\right) \\ & \therefore \frac{V_1}{V_2}=\frac{273+t_1}{273+t_2}=\frac{T_1}{T_2} \\ & \frac{V_1}{T_1}=\frac{V_2}{T_2} \\ & \frac{V}{T}=a \text { cons } \tan t \\ & V \propto T\end{aligned}$

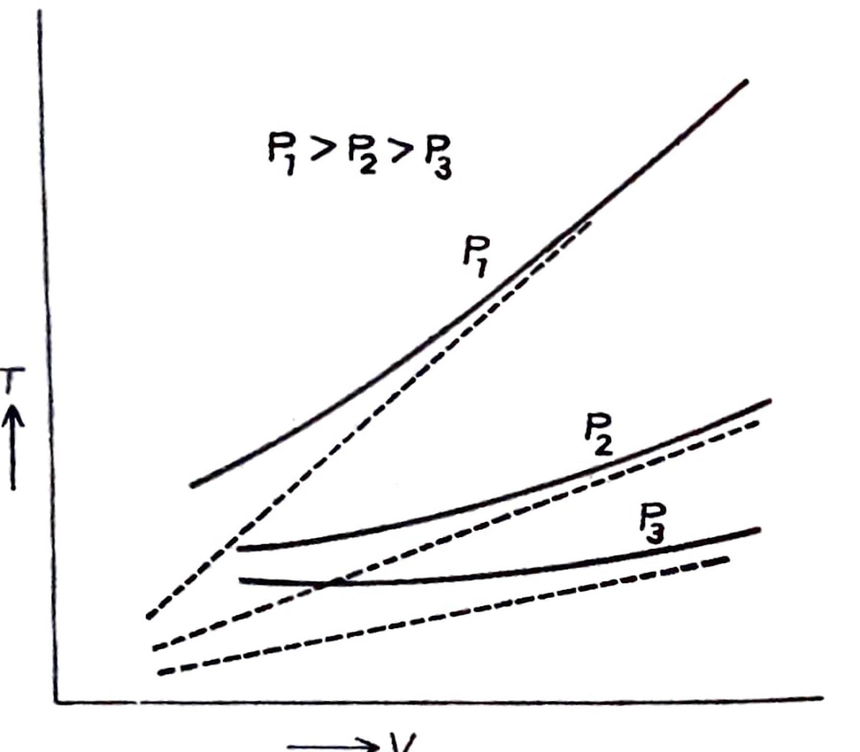
Therefore at constant pressure, the volume of a given mass of a gas is directly proportional to its absolute temperature. This is Charles’ Law in terms of absolute temperature.

• A graph is drawn between T and V for a gas at three different constant pressures P₁, P₂, and P₃ ( P₁> P₂>P₃).

Perfect Gas ( or ideal gas)

• A gas whose properties are similar to the properties of a real gas at infinitely low pressure such an imaginary gas is called perfect gas or ideal gas.
• Following are the properties of perfect gas:

1. It strictly obeys Boyle's Law, Charles’ Law and the law of pressure under all conditions of temperature and pressure.

2. Its pressure coefficient and volume coefficient are exactly equal to each other.

3. Its molecules are infinitesimally small.

4. There is no force attraction between its molecules.

Equation of State for an Ideal Gas

• Let initially pressure, volume, and temperature of a gas are P₁, V₁, and T₁ respectively and finally change to P₂ V₂, and T₂ respectively. Now,

i. Let the temperature of the gas be kept constant at T₁ and the pressure is changed from P₁ to P₂. If in this process the volume changes from V₁ to V₂ then by Boyle’s law,

$$
\begin{aligned}
& P_2 V_2=P_1 V_1 \\
& V_2=\frac{P_1 V_1}{P_2}
\end{aligned}
$$

ii. Let the pressure of the gas is kept constant at P₂ and its absolute temperature is changed from T₁ to T₂. If in this process the volume of the gas changes from V₁ to V₂ then by Charles’ law,

$$
\begin{aligned}
& \frac{V_1}{T_1}=\frac{V_2}{T_2} \\
& V_2=\frac{T_1 V_1}{T_2}
\end{aligned}
$$

From eqn (i) and (ii), we have

$\begin{aligned} & \frac{P_1 V_1}{P_2}=\frac{T_1 V_2}{T_2} \\ & \frac{P_1 V_1}{T_1}=\frac{P_2 V_2}{T_2}\end{aligned}$

Hence for a gas, the volume of PV/T remains constant. Let this constant be equal to K, then

$\begin{aligned} & \frac{P V}{T}=K \\ & P V=K T\end{aligned}$

We can write K = nk, k is the same for all gases. It is called Boltzmann constant.

$P V=n k T \quad \ldots \ldots(i i i)$

If P, V, and T are the same, then n is also the same for all gases.

If we take 1 gram-molecule (1 mole) of a gas-constant it will be the same for all gases. Then it is called the universal gas constant and it is represented by R. Then,

$P V=R T$

Where R = Nk, where N = Avogadro’s number and k = Boltzmann constant

It is called the ideal gas equation or perfect gas equation.

• The gas equation for μ moles of gas will be

$P V=\mu R T \quad \ldots \ldots(i v)$

• Unit of R:- Unit of R is Jmol⁻¹K⁻¹
• Dimension of R:- [ML²T⁻²K⁻¹].
• Numerical value of R:- The value of R is 8.31Jmol⁻¹K⁻¹.

Kinetic Theory of Gases

The following are the fundamental assumptions of the kinetic theory of gases:

• A gas is composed of a large number of tiny invisible, perfectly elastic particles, called molecules.
• The molecules are always in a state of continuous motion with varying velocities in all possible directions.
• The molecules traverse the straight path between any two collisions.
• The size of the molecules is infinitely small compared to the average distance traversed by a molecule between any two consecutive collisions. The distance between any two consecutive collisions is called the free path and the average distance between any two consecutive collisions is called the mean free path.
• The time of collisions is negligible as compared with the time taken to traverse the free path.
• The collisions between molecules and with the walls are perfectly elastic so that there is no loss of kinetic energy in the collisions.
• The molecules exert no force on each other except when they collide and the whole of the molecules energy is kinetic.
• The volume of the molecules is negligible as compared to the volume of a vessel containing gas.
• The inter molecular distances in a gas is much larger than that of a solid or liquid and the molecules of a gas are free to move in the entire space available to them.

Pressure Exerted by an Ideal Gas

• When a certain mass of a perfect gas is confined with a vessel the molecule frequently collides with the walls of the vessel and is reflected from the walls.During the collision with the walls each molecule suffers a change in momentum .

• According to Newton‘s second law of motion, the rate of momentum is equal to the force exerted. Thus, it exerts a pressure on the walls. This pressure may be calculated on the basis of postulates of the kinetic theory of gases.

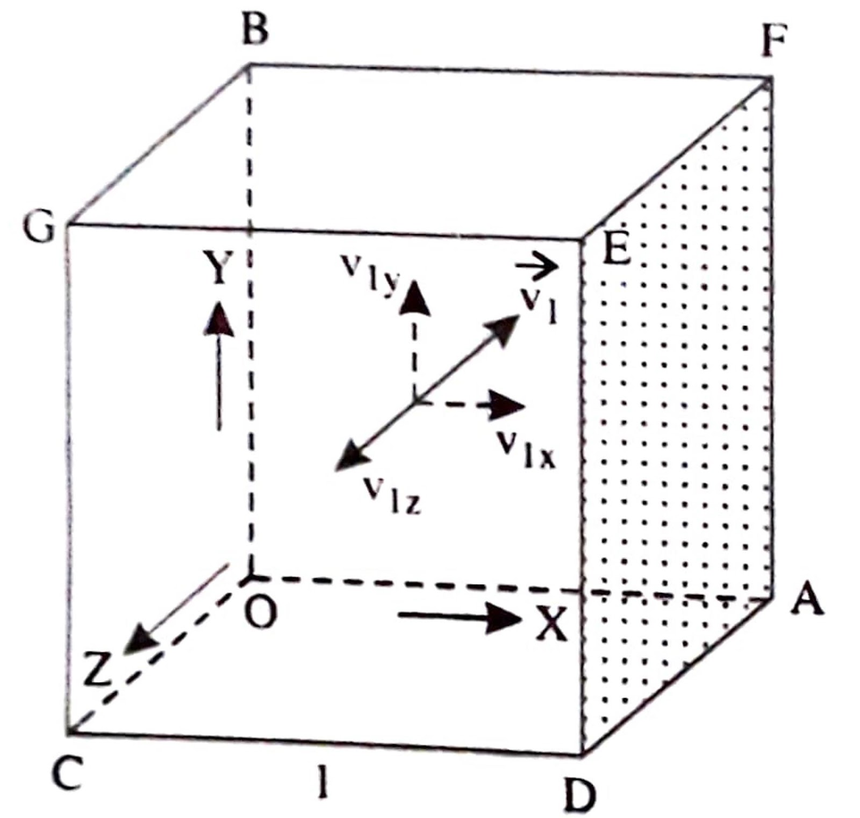
Let us consider a perfect gas contained in a cubical vessel of side (l) and perfectly elastic walls. Let n be the total total number of molecules of a gas in the vessel and m is the mass of the molecule. Let the sides of the cube represent mutually perpendicular X, Y, and Z-axes.

Consider a molecule molecule P moving in a random direction with velocity v₁. Let v_1x, v_1y, and v_1z be the components of the velocity v₁ along three mutually perpendicular X, Y, and Z axes, respectively.

Then

$v_1^2=v_{1 x}^2+v_{1 y}^2+v_{1 z}^2$

The component of velocity with which the molecule P will strike the face DEFA is v_1x and the momentum of the molecule X- direction before collisions

$=m v_{1 x}$

As the molecule and the wall are perfectly elastic, during collision speed of the molecule does not change and only the direction of velocity is changed.

Therefore, The momentum along X- axis after collisions

$=m\left(-v_{1 x}\right)=-m v_{1 x}$

The change in the momentum during one collision along X-axis

$=m v_{1 x}-\left(-m v_{1 x}\right)=2 m v_{1 x}$

The molecule suffering reflection at face DEFA traverses a distance l and strikes the face CGBO. It is equal to the force exerted on it. Hence, the force exerted by the molecule on the wall A will be mv_x²/l Since there are n molecules, the total force exerted on the wall A is given by

$\frac{m}{l}\left(v_{x_1}^2+v_{x_2}^2+\ldots \ldots v_{x_n}^2\right)$

Where v_xn is the x-component of velocity of the molecule 1, v_x2 of molecule 2,.... And so on. Since the area of the wall is l², the pressure on the wall A is given by

$\begin{aligned} & P=\frac{m}{l^3}\left(v_{x_1}^2+v_{x_2}^2+\ldots \ldots v_{x_n}^2\right) \\ & =\frac{m n}{l^3}\left(\frac{\left(v_{x_1}^2+v_{x_2}^2+\ldots \ldots v_{x_n}^2\right)}{n}\right) \\ & \text { But } l^3=\vee \\ & \therefore P=\frac{m n}{V}\left(\frac{\left(v_{x_1}^2+v_{x_2}^2+\ldots \ldots v_{x_n}^2\right)}{n}\right)\end{aligned}$

Now, $\left(\frac{\left(v_{x_1}^2+v_{x_2}^2+\ldots \ldots v_{x_n}^2\right)}{n}\right)$ is the average value of v_x² for all the n molecules. This can be written as

$\overline{v_x^2}$

Therefore,

$P=\frac{m n}{V} \bar{v}_x^2 \quad \ldots \ldots(i)$

We know that for any molecule, we have

$$
v^2=\overline{v_x^2}+\overline{v_y^2}+\overline{v_z^2} \quad \ldots \ldots
$$

Because this equation is true for every molecule, this will also be true for the mean value of all the molecules.

$\therefore v^{\overline{2}}=\bar{v}_x^2+\bar{v}_y^2+\bar{v}_z^2 \quad \ldots \ldots(i i i)$

The directions of the molecules are quite at random, that is, all directions are equally possible. Hence, the values, of $\overline{v_x^2}, \overline{v_y^2}, \overline{v_z^2}$ will be equal, ie. $\overline{v_x^2}=\overline{v_y^2}=\overline{v_z^2}$

Therefore from eqn (ii), we have

$$
\begin{aligned}
\overline{v^2} & =3 \overline{v_x^2} \\
\overline{v_x^2} & =\frac{1}{3} \overline{v^2}
\end{aligned}
$$

or

$$
P=\frac{1}{3} \frac{m n}{V} \overline{v^2} \ldots \ldots(i i i)
$$

This is the equation for the pressure of the gas. $\overline{v^2}$

is called the mean-square velocity of the molecules. Since, mn is the mass of the whole gas and V is the volume so, mn/V is the density ⍴ of the gas therefore, the above expression can be written as

$P=\frac{1}{3} \rho \bar{v}^2 \quad \ldots .(i v)$

Again,

$P=\frac{1}{3} \rho v^2=\frac{2}{3}\left(\frac{1}{2} \rho \overline{v^2}\right)=\frac{2}{3} E$

Where $E=\left(\frac{1}{2} \rho \overline{v^2}\right)$ is the transnational kinetic energy of the gas per unit volume. Thus, the pressure is equal to two-thirds of its transnational kinetic energy per unit volume.

Root mean-Square (rms) Speed of gas molecule

• The speeds of the individual molecules of a gas vary over a wide range. Let v₁, v₂, and v₃…...vₙ. Be the speed of molecules of a gas. Then, the root-mean-square speed of the molecules is defined as the square root of the mean of the squares of the speeds of the all gas molecules, and it is denoted by rms. Thus,

$v_{r m s}=\sqrt{\overline{v^2}} \sqrt{\frac{v_1^2+v_2^2+v_3^2}{n}}$

• We know that the pressure of a gas is given by

$\begin{aligned} & P=\frac{1}{3} \rho \overline{v^2} \\ & v_{r m s}=\sqrt{\overline{v^2}}=\sqrt{\frac{3 P}{\rho}}\end{aligned}$

Interpretation of temperature on the basis of Kinetic theory

Let us consider 1 gram molecule of a gas at absolute temperature, T, occupying a volume V. According to Kinetic theory, the pressure of the gas

$\begin{aligned} & P=\frac{1}{3} \cdot \frac{m N}{V} \overline{v^2} \ldots \ldots(i) \\ & P V=\frac{1}{3} m N \overline{v^2} \because \ldots(i i) \\ & \because P V=R T \ldots \ldots(i i i) \end{aligned} $

And mN = M

Comparing eqn (ii) and (iii)

$\begin{aligned} & \frac{1}{3} M \overline{v^2}=R T \\ & \overline{v^2}=\frac{3 R}{M} T \\ & \sqrt{\overline{v^2}}=\sqrt{\frac{3 R T}{M}} \\ & v_{r m s}=\sqrt{\frac{3 R T}{M}} \\ & v_{r m s} \propto \sqrt{T}\end{aligned}$

Thus, the root mean square speed of the molecules of a gas is directly proportional to the square root of the absolute temperature of the gas.

Relation between temperature and molecular kinetic energy:-

$$
\frac{1}{2} M \overline{v^2}=\frac{1}{2} M\left(\frac{3 R T}{M}\right)
$$

• Average kinetic energy of 1 mole of gas $=\frac{3}{2} R T$
• Average kinetic energy of one molecule =

$\frac{(3 / 2) R T}{N}=\frac{3}{2}\left(\frac{R}{N}\right) T$

R/N = k (Boltzmann constant)

$$
\text { Average kinetic energy of } 1 \text { molecule }=\frac{3}{2} K T
$$

It means different gases at the same temperature have the same average kinetic energy per molecule and this energy is directly proportional to the absolute temperature of the gas.

Law of Equipartition of Energy

• It states that the total kinetic energy of a dynamical system consisting of a large number of particles in thermal equilibrium is equally distributed among all degrees of freedom 0.5KT, and the energy associated with each degree of freedom is where k is the Boltzmann constant and T is the absolute temperature of the system.

Thus, the average kinetic energy of a molecule per degree of freedom is 0.5KT which is the law of equipartition of energy.

Explanation of Gas Laws from Kinetic Theory

1. Boyle’s Law

We know

$P V=\frac{1}{3} m N v^{\overline{2}} \quad \ldots .(i)$

According to kinetic theory, the average kinetic energy of translation of molecules of gas is directly proportional to its absolute temperature,

$\frac{1}{2} m \overline{v^2}=\alpha T$

i.e., at a given temperature, average kinetic energy of a gas is constant and N is also constant, so eqn (i)

$P V=\frac{2}{3} N \cdot \frac{1}{2} m \overline{v^2}=\frac{2}{3} N \alpha T$

= constant at a given temperature

This is Boyle's Law.

2. Charles’s Law

From eqn(i)

$P V=\frac{1}{3} m N \overline{v^2}$

Also the average K.E. of a molecule,

$\begin{aligned} & \frac{1}{2} m \overline{v^2}=\alpha T \\ & P V=\frac{2}{3} N \cdot \alpha T \\ & V \propto T\end{aligned}$

for constant value P.

Thus at a given pressure for a given mass of a gas, the volume is directly proportional to the absolute temperature. This is Charles' law.

Mean Free Path

• The average distance traversed by a molecule between two successive collisions is defined as the mean free path.

Thus, if λ₁, λ₂, λ₃, ….λₙ are the successive free paths traversed in the total time t, then,

$\lambda_1+\lambda_2+\ldots . \lambda_n=\bar{v} t$

• If N is the total no. of collisions suffered, then

$\begin{aligned} \lambda & =\frac{\lambda_1+\lambda_2+\lambda_3+\ldots . \lambda_n}{N} \\ & =\frac{\bar{v} t}{N}\end{aligned}$

• Expression for Mean Free Path

We know that,

$\begin{aligned} \lambda & =\frac{\text { total dis } \tan \text { ce covered in time } t}{\text { number of collision in time } t} \\ & =\frac{\bar{v} t}{n \times \pi d^2 \bar{v} t}=\frac{1}{\pi n d^2}\end{aligned}$

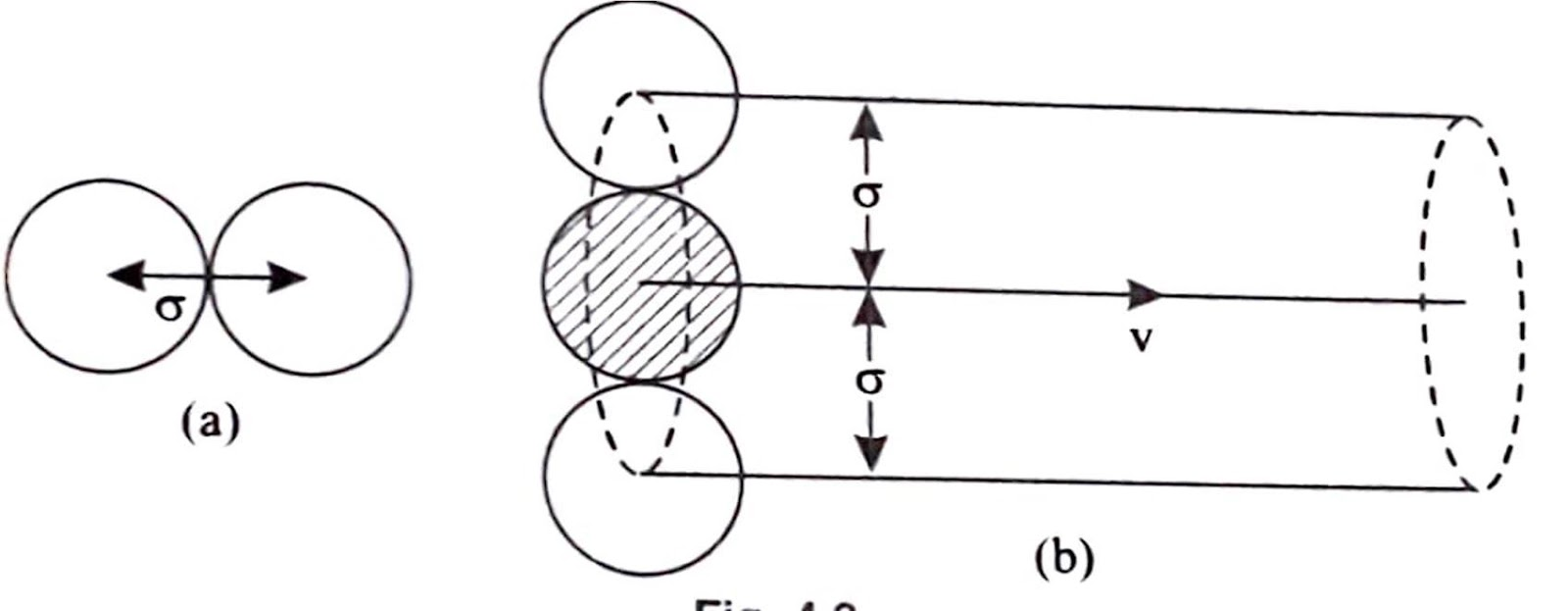
But by experiment, Maxwell found that

$\lambda=\frac{1}{\sqrt{2}\left(\pi \sigma^2 n\right)}$

The mean free path is inversely proportional to the number of molecules per unit volume (n) which is proportional to the density of the gas.

For N molecule of an ideal gas, we have

$\begin{aligned} & P V=N k T \\ & \therefore n=\frac{N}{V}=\frac{P}{k T} \\ & \lambda=\frac{k T}{\sqrt{2} \pi d^2 P}\end{aligned}$

This is the mean free path of the molecules of a gas is directly proportional to its absolute temperature and inversely proportional to the pressure of the gas.

Avagadro’s Number: The Avagadro’s number is the number of molecules in 1 mole of a substance. It is denoted by N and its value is 6.0221 X 10 ²³.

Kinetic Theory of Gases Previous year Question and Answer

Q1: Diatomic molecules like hydrogen have energies due to both translational as well as rotational motion. From the equation in kinetic theory PV = $\frac{2}{3}E$ , E is

(a) the total energy per unit volume

(b) only the translational part of energy because rotational energy is very small compared to the translational energy

(c) only the translational part of the energy because during collisions with the wall pressure relates to change in linear momentum

(d) the translational part of the energy because rotational energies of molecules can be of either sign and its average over all the molecules is zero

Answer:

As per the kinetic theory of gases, the perpendicular forces exerted by the molecules on the walls while in motion are only responsible for the pressure exerted due to the gas molecules. So, for molecules striking at angles other than 90 degrees, no pressure will be exerted. Hence, in this case, only translational motion change leads to pressure on the wall. So, PV = $\frac{2}{3}E$, which represents the translational motion part and hence (c) is the correct option.

Q2: When an ideal gas is compressed adiabatically, its temperature rises the molecules on average have more kinetic energy than before. The kinetic energy increases,

(a) because of collisions with moving parts of the wall only

(b) because of collisions with the entire wall

(c) because the molecules get accelerated in their motion inside the volume

(d) because of the redistribution of energy amongst the molecules

Answer:

The number of collisions per second between the molecules and walls increases and the mean free path becomes smaller as an ideal gas is compressed. This, in turn, increases the temperature of the gas, which increases the overall kinetic energy of the gas molecules as KE depends on the temperature. Hence, (a) is the correct option.

Q3: In a diatomic molecule, the rotational energy at a given temperature
(a) obeys Maxwell's distribution
(b) have the same value for all molecules
(c) equals the translational kinetic energy for each molecule
(d) is $\left(\frac{2}{3}\right) ^{rd}$ the translational kinetic energy for each molecule

Answer:

If we assume a diatomic molecule along the $z$-axis, its energy along that axis will be zero. The total energy of a diatomic molecule can be expressed as: $E=\frac{1}{2} m v x^2+\frac{1}{2} m v y^2+\frac{1}{2} m v z^2+\frac{1}{2} I x W x^2+\frac{1}{2} I x W y^2$.

The number of independent terms in the expression $=5$. The above expression obeys Maxwell's distribution as their velocities can be predicted with Maxwell's findings. In this case, for each molecule 3 translational and 2 rotational energies are associated. So, at any temperature, rotational energy $=\left(\frac{2}{3}\right) ^{rd}$ translational KE

Hence, (a) and (d) are the correct options.

NCERT Class 11 Notes Chapter-Wise

Subject Wise NCERT Exemplar Solutions

• NCERT Exemplar Class 11 Solutions
• NCERT Exemplar Class 11 Maths
• NCERT Exemplar Class 11 Physics
• NCERT Exemplar Class 11 Chemistry
• NCERT Exemplar Class 11 Biology

Subject Wise NCERT Solutions

• NCERT Solutions for Class 11 Mathematics
• NCERT Solutions for Class 11 Chemistry
• NCERT Solutions for Class 11 Physics
• NCERT Solutions for Class 11 Biology

NCERT Books and Syllabus