Oscillations Class 11th Notes - Free NCERT Class 11 Physics Chapter 14 Notes - Download PDF

NCERT Notes for Class 11 Physics Chapter 13: Download PDF

Study anytime and anywhere using the PDF version of Class 11 Physics Oscillations Notes. It is useful for revision and quick access to the complete notes. Download it now by clicking on the link below.

Download PDF

NCERT Notes for Class 11 Physics Chapter 13

The Class 11 Oscillation Notes give a complete overview of the contents of the chapter. They give the important formulas along with diagrams for easy understanding. Students can use the notes to revise effectively and prepare for their exams.

Periodic Motion

• A motion that repeats itself after a fixed interval of time is called periodic motion. e.g., orbital motion of the Earth around the Sun, motion of seconds’ arm of a clock, motion undergone by a simple pendulum, etc.

Oscillatory Motion

• A periodic motion which undergoes a to and fro else back and forth about a fixed point, is known as oscillatory motion, e.g., motion shown by a simple pendulum, motion of a spring etc.
• Note that all oscillatory motions are periodic motions but all periodic motion are not oscillatory motions. Thus, visa-versa is not true.

• Harmonic Oscillation: The oscillation that is expressed in terms of a single harmonic function, which are sine or cosine function, is harmonic oscillation.

• Simple Harmonic Motion: A Simple harmonic motion is such that it will move in accordance with to and fro along a straight line.Under restoring force magnitude is directly proportional to that of the displacement.

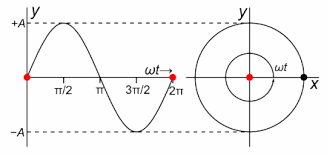
A SHM can be expressed in the following manner:

y = a sin ωt

or

y = a cos ωt

a = amplitude of oscillation.

• Non-harmonic Oscillation

A non-harmonic oscillation is the combination of at least two harmonic oscillations.

Non-harmonic Oscillation is expressed as:

y = a sin ωt + b sin 2ωt

Terms Related to SHM

The following are the important terms related to Simple harmonic Motion-

1. Time Period: Time taken by a body to complete one whole oscillation is known as time period. It is symbolized by T.

2. Frequency: The number of oscillations completed by the body in one second is frequency. It is symbolized by v.

SI unit = ‘Hertz’ or ‘second^-1

Frequency = 1 / Time period

3. Angular Frequency: The product of frequency and the factor 2π is called angular frequency. It is symbolized by ω.

Angular frequency (ω) = 2πv

SI unit = ‘Hertz’ or ‘second^-1.

4. Displacement: A physical quantity which changes uniformly with respect to time in a periodic motion is displacement. It is symbolized by y.

5. Amplitude: The maximum displacement in any direction with respect to mean position is called amplitude. It is symbolized by a.

6. Phase: A physical quantity which shows the position and direction of motion of an oscillating particle, is known as a phase. It is symbolized by φ.

SHM as Circular Motion

• SHM is defined as the projection of the uniform circular motion on a circle of any diameter as reference.

• Some Important Formulae of SHM

1. Displacement in SHM at any instant is shown as:

y = a sin ωt

or

y = a cos ωt , where a = amplitude and ω = angular frequency.

2. Velocity of a particle undergoing SHM at any instant is expressed as :

v = ω √(a² – y²)

When at mean position, y = 0 and v = maximum

v_max = aω

When at an extreme position, y = a and v=0.

3. Acceleration of a particle undergoing SHM at any instant is shown by

A or a = – ω² y

The acceleration is towards the mean position, and so the negative sign signifies the opposite to that of increase in displacement

At mean position y = 0 and acceleration = 0.

At extreme position y = a and acceleration = maximum

A_max = – aω²

4. Time period in SHM is shown by T = 2π √Displacement / Acceleration

Graphical Representation

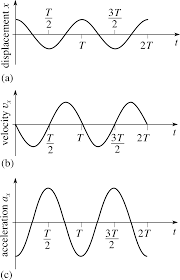
• The acceleration is maximum when the velocity is minimum and vice versa.
• Incase a particle undergoing SHM the phase difference between

(i) Instantaneous displacement and Instantaneous velocity = (π / 2)ͨ

(ii) Instantaneous velocity and Instantaneous acceleration= (π / 2)ͨ

(iii) Instantaneous acceleration and Instantaneous displacement= πͨ

The graph of velocity versus displacement for a particle executing SHM is elliptical.

Force in SHM

• Acceleration of body in SHM is α = -ω² x
• Applying the equation of motion F = ma,

F = – mω² x = -kx

Where, ω = √k / m and k = mω2 and ‘k’ constant and sometimes it is called the elastic constant.

• The force is proportional and opposite to the displacement ( in SHM).

Energy in SHM

• The Kinetic energy of a particle is K = 1 / 2 mω² (A² – x²)
• From above expression we can see that, the kinetic energy is maximum at centre (x = 0) and 0 at the extremes of the oscillation (that is x ± A).
• The Potential energy of the particle, U =mω² x²/2
• Above expression says, the potential energy has a minimum value at the centre (x = 0) and increases as the particle approaches either extremes of the oscillation (that is x ± A).
• Total energy is obtained by adding potential and kinetic energies. Thus,

E = K + U = [m² ω²(A² – x²) + mω²x²]/2= mω²A²/2

where A = amplitude

m = mass of particle executing SHM.

ω = angular frequency and

v = frequency

• Changes that occur in kinetic and potential energies during oscillations.

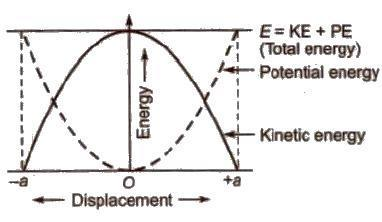
• The frequency of total energy of particles executing SHM equals zero as total energy in SHM remains constant at every position.
• When a particle of mass m undergoes SHM with a constant angular frequency (I), then the time period of oscillation (T)

Simple Pendulum

• A simple pendulum has a heavy point mass which is suspended through a rigid support with the help of an elastic inextensible string.
• The time period of a simple pendulum is expressed as :

T = 2π √(l / g)

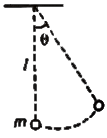
where l = effective length of pendulum and g = acceleration due to gravity.

• Vibrations of Loaded Spring

The restoring force acts on spring under the condition when spring is stretched or compressed through a small distance y.

Restoring force (F) = – ky; Here k = force constant of spring.

In equilibrium, mass m is suspended to a spring system.

mg = kl

This expression is also used for Hooke's law.

Time period of a loaded spring is expressed in terms as follows:

T = 2π √m / k

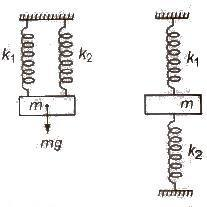
Let the two springs of force constants k₁ and k₂.

These two constants are connected in parallel to mass m.

1. Effective force constant will be therefore,

k = k₁ + k₂

(ii) Time period

T = 2π √m / (k₁ + k₂)

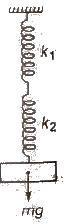
These two constants are connected in series to mass m.

(i) Effective force constant will be therefore,

1 / k = 1 / k₁ + 1 / k₂

(ii) Time period is expressed as follows: T = 2π √m(k₁ + k₂) / k₁k₂

Oscillations Previous year Question and Answer

Q1: The displacement of a particle is represented by the equation $y=\sin ^3 \omega t$. The motion is:

(a) non-periodic

(b) periodic but not simple harmonic

(c) simple harmonic with period $\frac{2 \pi}{\omega}$

(d) simple harmonic with period $\frac{\pi}{\omega}$

Answer:

Periodic Motion:
- A motion is periodic if it repeats itself after a fixed interval of time. The function $\sin ^3(\omega t)$ is derived from $\sin (\omega t)$, which is periodic with a period $T=\frac{2 \pi}{\omega}$. Since $\sin ^3(\omega t)$ repeats itself every $T$, the motion is periodic.

Simple Harmonic Motion (SHM):
- A motion is simple harmonic if it can be expressed as $y(t)=A \sin (\omega t+\phi)$, where:
- $A$ is the amplitude.
- $\omega$ is the angular frequency.
- $\phi$ is the phase constant.
- The function $\sin ^3(\omega t)$ is not a pure sine wave. Instead, it involves higher harmonics and can be expanded using trigonometric identities.

So the motion is periodic but not simple harmonic. Hence, the answer is the option (b).

Q2: A particle is acted simultaneously by mutually perpendicular simple harmonic motion $x=a \cos \omega t ,$ and $ y=a \sin \omega t$. The trajectory of motion of the particle will be:

(a) an ellipse

(b) a parabola

(c) a circle

(d) a straight line

Answer:

Resultant displacement $=x+y =a \cos \omega t+a \sin \omega t$

Thus,

$\begin{aligned}
y^{\prime}&=a(\cos \omega t+a \sin \omega t)\\
&= a \sqrt{2}\left[\frac{\cos \omega t}{\sqrt{2}}+\frac{\sin \omega t}{\sqrt{2}}\right] \\
& =a \sqrt{2}\left[\cos \omega t \cos 45^{\circ}+\sin \omega t \sin 45^{\circ}\right]
\end{aligned}$

(particle is acted simultaneously by mutually perpendicular direction)
$y^{\prime}=a \sqrt{2} \cos s\left(\omega t-45^{\circ}\right)$.

Thus, the displacement can neither be a straight line nor a parabola

Now, let us square and add $x$ \& $y$,

$x^2+y^2=a^2 \cos ^2 \omega t+a^2 \sin ^2 \omega t$

Thus, $x^2+y^2=a^2$

This represents a circle.

Q3: A particle executing S.H.M. has a maximum speed of $30 \frac{\mathrm{~cm}}{\mathrm{~s}}$ and a maximum acceleration of $60 \frac{\mathrm{~cm}}{\mathrm{~s}^2}$. The period of oscillation is

Answer:

Thus, $y=a \sin \omega t$

$\begin{aligned}
v&=\frac{d y}{d t} \\
&=a \omega \cos \omega t \quad\ldots(i)\\
a&=\frac{d v}{d t} \\
&=-a \omega^2 \sin \omega t \quad\ldots(ii)\\
V_{\text {max }}&=30 \frac{\mathrm{~cm}}{\mathrm{~s}}
\end{aligned}$

From (i),

$V_{\max }=a \omega$

Thus, $a \omega=30 \quad\ldots(iii)$

From (ii),
Now, $a_{\max }=a \omega^2=60 \quad\ldots(iv)$

Thus, $60=\omega \times 30$

Therefore, $\omega=2 \frac{\mathrm{rad}}{\mathrm{s}}$

Now, $\frac{2 \pi}{T}=2$
Thus, $T=\pi$.

NCERT Class 11 Notes Chapter-Wise

Subject Wise NCERT Exemplar Solutions

• NCERT Exemplar Class 11 Solutions
• NCERT Exemplar Class 11 Maths
• NCERT Exemplar Class 11 Physics
• NCERT Exemplar Class 11 Chemistry
• NCERT Exemplar Class 11 Biology

Subject Wise NCERT Solutions

• NCERT Solutions for Class 11 Mathematics
• NCERT Solutions for Class 11 Chemistry
• NCERT Solutions for Class 11 Physics

• NCERT Solutions for Class 11 Biology