• School
  • Engineering and Architecture
  • Management and Business Administration
  • Medicine and Allied Sciences
  • Law
  • Animation and Design
  • Media, Mass Communication and Journalism
  • Finance & Accounts
  • Computer Application and IT
  • Pharmacy
  • Hospitality and Tourism
  • Competition
  • Study Abroad
  • Arts, Commerce & Sciences
  • Learn
  • Online Courses and Certifications
NCERT solutions for Class 12 Physics Chapter 6 Electromagnetic Induction

NCERT solutions for Class 12 Physics Chapter 6 Electromagnetic Induction

Edited By Vishal kumar | Updated on Sep 09, 2023 12:41 PM IST | #CBSE Class 12th

NCERT Solutions for Class 12 Physics Chapter 6 –Access and Download Free PDF

NCERT Solutions for Class 12 Physics Chapter 6 Electromagnetic Induction- Welcome to the updated class 12 physics electromagnetic induction NCERT solutions. On this NCERT Solution page, you will find comprehensive and detailed solutions prepared by subject experts from Careers360. These solutions cover a total of seventeen questions, including exercise and additional exercise questions.

This Story also Contains
  1. NCERT Solutions for Class 12 Physics Chapter 6 –Access and Download Free PDF
  2. NCERT Solutions for Class 12 Physics Chapter 6 Electromagnetic Induction
  3. NCERT solutions for class 12 physics chapter 6 electromagnetic induction additional exercise
  4. Key Features of Electromagnetic Induction Class 12 Solutions
NCERT solutions for Class 12 Physics Chapter 6 Electromagnetic Induction
NCERT solutions for Class 12 Physics Chapter 6 Electromagnetic Induction

Physics class 12 chapter 6 ncert solutions are invaluable for 12th-grade students. This Chapter 6 PDF is available to make the subject more accessible and engaging. To gain a comprehensive understanding of the topic and enhance their Class 12 Physics Chapter 6 Electromagnetic Induction NCERT notes, students should make it a practice to regularly work through these solutions

Hence, students can rely upon the NCERT solutions for Class 12 Physics Chapter 6 Electromagnetic Induction and prepare for the exams. The NCERT exemplar Class 12 Physics Chapter 6 solutions focus on the question based on the concept that the changing magnetic field can produce an emf across an electrical conductor. Electromagnetic induction class 12 ncert solutions are an important tool to perform better in exams. The NCERT solutions for Class 12 also help in the preparations for competitive exams. Read further to know the NCERT solutions for Class 12 Physics Chapter 6 Electromagnetic Induction in detail.

VMC VIQ Scholarship Test

Register for Vidyamandir Intellect Quest. Get Scholarship and Cash Rewards.

Apply
Pearson | PTE

Register now for PTE & Unlock 20% OFF : Use promo code: 'C360SPL20'. Valid till 30th NOV'24! Trusted by 3,500+ universities globally

Apply

Free download electromagnetic induction class 12 solutions PDF for CBSE exam.

NCERT Solutions for Class 12 Physics Chapter 6 Electromagnetic Induction

Download PDF


NCERT solutions for class 12 physics chapter 6 electromagnetic induction: Exercise Question Answer

Q 6.1(a) Predict the direction of induced current in the situations described by the following

1643794334324

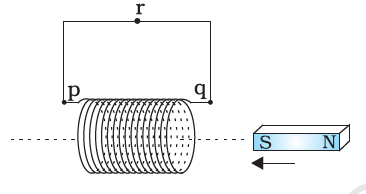
Answer:

To oppose the magnetic field current should flow in anti-clockwise, so the direction of the induced current is qrpq

Q 6.1 (b) Predict the direction of induced current in the situations described by the following Figs.

1594625768893

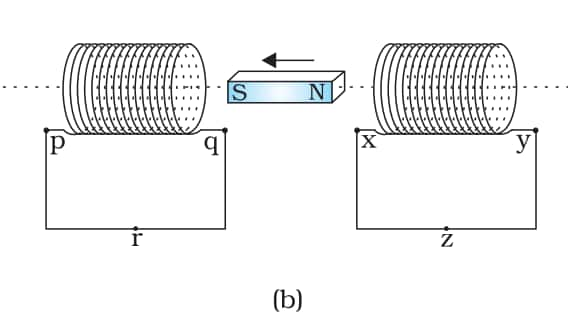
Answer:

Current in the wire in a way such that it opposes the change in flux through the loop. Here hence current will induce in the direction of p--->r--->q in the first coil and y--->z--->x in the second coil.

Q 6.1 (c) Predict the direction of induced current in the situations described by the following Figs.(c)

1594625855893

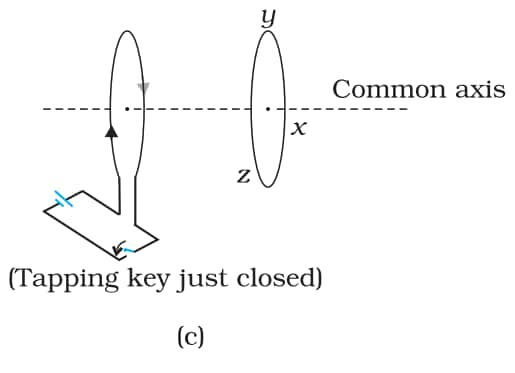
Answer:

When we close the key, the current will flow through the first loop and suddenly magnetic flux will flow through it such that magnetic rays will go from right to left of the first loop. Now, to oppose this change currently in the second loop will flow such that magnetic rays go from left to right which is the direction yzxy

Q 6.1 (d) Predict the direction of induced current in the situations described
by the following Fig. (d)

1594625913212

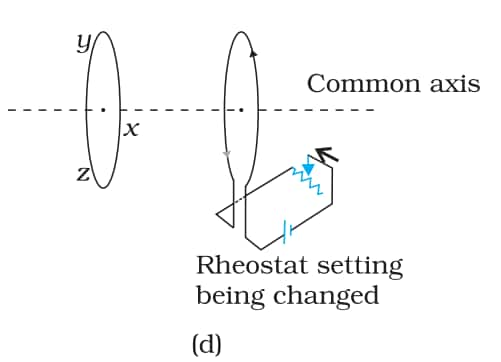
Answer:

hen we increase the resistance of the rheostat, the current will decrease which means flux will decrease so current will be induced to increase the flux through it. Flux will increase if current flows in xyzx.

On the other hand, if we decrease the resistance that will increase the current which means flux will be an increase, so current will induce to reduce the flux. Flux will be reduced if current goes in direction zyxz

Q 6.1 (e) Predict the direction of induced current in the situations described by the following Fig(e)

1594625999208

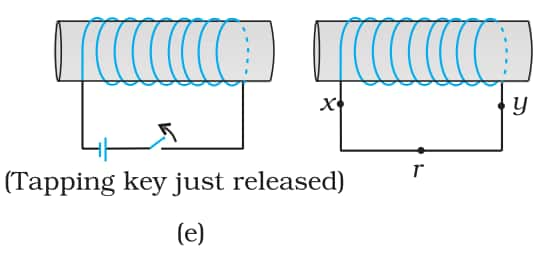
Answer:

As we release the tapping key current will induce to increase the flux. Flux will increase when current flows in direction xryx.

Q 6.1 (f) Predict the direction of induced current in the situations described by the following Fig (f)

1594626054253

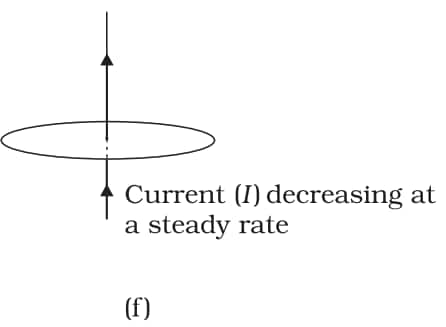
Answer:

The current will not induce as the magnetic field line are parallel to the plane. In other words, since flux through the loop is constant (zero in fact), there won't be any induction of the current.

Q6.2 (a) Use Lenz’s law to determine the direction of induced current in the situations described by Fig. 6.19: a

A wire of irregular shape turning into a circular shape;

1594626166541

Answer:

By turning the wire from irregular shape to circle, we are increasing the area of the loop so flux will increase so current will induce in such a way that reduces the flux through it. By right-hand thumb rule direction of current is adcba.

Q6.2 (b) Use Lenz’s law to determine the direction of induced current in the situations described by Fig. 6.19 b :

A circular loop being deformed into a narrow straight wire.

1594626217838

Answer:

Here, by changing shape, we are decreasing the area or decreasing the flux, so the current will induce in a manner such that it increases the flux. Since the magnetic field is coming out of the plane, the direction of the current will be adcba.

Q6.3 A long solenoid with 15 turns per cm has a small loop of area 2.0 cm^{2} placed inside the solenoid normal to its axis. If the current carried by the solenoid changes steadily from 2.0 A to 4.0 A in 0.1s , what is the induced emf in the loop while the current is changing?

Answer:

Given in a solenoid,

The number of turn per unit length :

n = 15turn/cm=1500turn/m

loop area :

A=2cm^2=2*10^{-4}m

Current in the solenoid :

initial current = I_{initial}=2

finalcurrent = I_{final}=4

change in current :

\Delta I = 4-2 = 2

change in time:

\Delta t=0.1s

Now, the induced emf :

e=\frac{d\phi }{dt}=\frac{d(BA)}{dt}=\frac{d(\mu _0nIA)}{dt} = \mu _0nA\frac{dI}{dt}=\mu _0nA\frac{\Delta I}{\Delta t}


e=4\pi*10^{-7}*1500*2*10^{-4}*\frac{2}{0.1}=7.54*10^{-6}

hence induced emf in the loop is 7.54*10^{-6} .

Q6.4 A rectangular wire loop of sides 8 cm and 2cm with a small cut is moving out of a region of uniform magnetic field of magnitude 0.3T directed normal to the loop. What is the emf developed across the cut if the velocity of the loop is 1 cm s^{-1} in a direction normal to the (a) longer side, (b) shorter side of the loop? For how long does the induced voltage last in each case?

Answer:

Given:

Length of rectangular loop :

l=8cm=0.08m

Width of the rectangular loop:

b=2cm=0.02m

Area of the rectangular loop:

A=l*b=(0.08)(0.02)m^2=16*10^{-4}m^2

Strength of the magnetic field

B=0.3T

The velocity of the loop :

v=1cm/s=0.01m/s

Now,

a) Induced emf in long side wire of rectangle:

e=Blv=0.3*0.08*0.01=2.4*10^{-4}V

this emf will be induced till the loop gets out of the magnetic field, so

time for which emf will induce :

t=\frac{distnce }{velocity}=\frac{b}{v}=\frac{2*10^{-2}}{0.01}=2s

Hence a 2.4*10^{-4}V emf will be induced for 2 seconds.

b) Induced emf when we move along the width of the rectangle:

e=Bbv=0.3*0.02*0.01=6*10^{-5}V

time for which emf will induce :

t=\frac{distnce }{velocity}=\frac{l}{v}=\frac{8*10^{-2}}{0.01}=8s

Hence a 6*10^{-5}V emf will induce for 8 seconds.

Q6.5 A 1.0m long metallic rod is rotated with an angular frequency of 400 rad\: s^{-1} about an axis normal to the rod passing through its one end. The other end of the rod is in contact with a circular metallic ring. A constant and uniform magnetic field of 0.5 T parallel to the axis exists everywhere. Calculate the emf developed between the centre and the ring.

Answer:

Given

length of metallic rod :

l=1m

Angular frequency of rotation :

\omega = 400s^{-1}

Magnetic field (which is uniform)

B= 0.5T

Velocity: here velocity at each point of the rod is different. one end of the rod is having zero velocity and another end is having velocity \omega r . and hence we take the average velocity of the rod so,

Average velocity =\frac{0+\omega l}{2}=\frac{\omega l }{2}

Now,

Induce emf

e=Blv=Bl\frac{wl}{2}=\frac{Bl^2\omega}{2}

e=\frac{0.5*1^2*400}{2}=100V

Hence emf developed is 100V.

Q6.6 A circular coil of radius 8.0 cm and 20 turns is rotated about its vertical diameter with an angular speed of 50 \: rads\: s^{-1} in a uniform horizontal magnetic field of magnitude 3.0\times 10^{-2} T. Obtain the maximum and average emf induced in the coil. If the coil forms a closed loop of resistance 10\Omega , calculate the maximum value of current in the coil. Calculate the average power loss due to Joule heating. Where does this power come from?

Answer:

Given

The radius of the circular loop r=8cm=0.08m

Number of turns N = 20

Flux through each turn

\phi = B.A=BAcos\theta =BAcos\omega t=B\pi r^2cos\omega t

Flux through N turn

\phi =NB\pi r^2cos\omega t

Induce emf:

e=\frac{d\phi }{dt}=\frac{d(NB\pi r^2cos\omega t)}{dt}=NBr^2\pi \omega sin\omega

Now,

maximum induced emf (when sin function will be maximum)

e_{max}=NB\pi r^2\omega=20*50*\pi *(0.08)^2*3*10^{-2}=0.603V


Average induced emf

e_{average}=0 as the average value of sin function is zero,


Maximum current when resistance R of the loop is 10\Omega .

I_{max}=\frac{e_{max}}{R}=\frac{0.603}{10}=0.0603A


Power loss :

P_{loss}= \frac{1}{2}E_0I_0=\frac{1}{2}(0.603)(0.0603)=0.018W

Here, power is getting lost as emf is induced and emf is inducing because we are MOVING the conductor in the magnetic field. Hence external force through which we are rotating is the source of this power.

Q6.7 (a) A horizontal straight wire 10m long extending from east to west isfalling with a speed of 5.0 m\: s^{-1} , at right angles to the horizontal component of the earth’s magnetic field, 0.30\times 10^{-4}\: wb\: m^{-2} .

What is the instantaneous value of the emf induced in the wire?

Answer:

Given

Length of the wire l=10m

Speed of the wire v=5m/s

The magnetic field of the earth B=0.3*10^{-4}Wbm^{-2}

Now,

The instantaneous value of induced emf :

e=Blv=0.3*10^{-4}*10*5=1.5*10^{-3}

Hence instantaneous emf induce is 1.5*10^{-3} .

Q6.7 (b) A horizontal straight wire 10m long extending from east to west is falling with a speed of 5.0 m\: s^{-1} , at right angles to the horizontal component of the earth’s magnetic field, 0.30\times 10^{-4}\: wb\: m^{-2} .

What is the direction of the emf?

Answer:

If we apply the Flemings right-hand rule, we see that the direction of induced emf is from west to east.

Q6.7(c) A horizontal straight wire 10m long extending from east to west is falling with a speed of 5.0 m\: s^{-1} , at right angles to the horizontal component of the earth’s magnetic field, 0.30\times 10^{-4}\: wb\: m^{-2} .

Which end of the wire is at the higher electrical potential?

Answer:

The eastern wire will be at the higher potential end.

Q6.8 Current in a circuit falls from 5.0A to 0.0A in 0.1s . If an average emf of 200V induced, give an estimate of the self-inductance of the circuit.

Answer:

Given

Initial current I_{initial}=5A

Final current I_{final}=0A

Change in time I_{final}=\Delta t=0.1s

Average emf e=200V

Now,

As we know, in an inductor

e=L\frac{di}{dt}=L\frac{\Delta I}{\Delta t}=L\frac{I_{final}-I_{initial}}{\Delta t}

L= \frac{e\Delta t}{I_{final}-I_{initial}}=\frac{200*0.1}{5-0}=4H

Hence self-inductance of the circuit is 4H.

Q6.9 A pair of adjacent coils has a mutual inductance of 1.5H . If the current in one coil changes from 0 to 20A in 0.5s , what is the change of flux linkage with the other coil?

Answer:

Given

Mutual inductance between two coils:

M = 1.5H

Currents in a coil:

I_{initial}=0

I_{final}=20

Change in current:

di=20-0=20

The time taken for the change

dt=0.5s

The relation between emf and mutual inductance:

e=M\frac{di}{dt}

e= \frac{d\phi }{dt}=M\frac{di}{dt}

d\phi =Mdi d\phi =Mdi=1.5*20=30Wb

Hence, the change in flux in the coil is 30Wb .

Q6.10 A jet plane is travelling towards west at a speed of 1800km/h . What is the voltage difference developed between the ends of the wing having a span of 25m , if the Earth’s magnetic field at the location has a magnitude of 5\times 10^{-4}T and the dip angle is 30^{0} .

Answer:

Given

Speed of the plane:

v=1800kmh^{-1}=\frac{1800*1000}{60*60}=500m/s

Earth's magnetic field at that location:

B = 5 *10^{-4}T

The angle of dip that is angle made with horizontal by earth magnetic field:

\delta = 30 ^0

Length of the wings

l=25m

Now, Since the only the vertical component of the magnetic field will cut the wings of plane perpendicularly, only those will help in inducing emf.

The vertical component of the earth's magnetic field :

B_{vertical}=Bsin\delta =5*10^{-4}sin30=5*10^{-4}*0.5=2.5*10^{-4}

So now, Induce emf :

e=B_{vertical}lv=2.5*10^{-4}*25*500=3.125V

Hence voltage difference developed between the ends of the wing is 3.125V.

NCERT solutions for class 12 physics chapter 6 electromagnetic induction additional exercise

Q6.11 Suppose the loop in Exercise 6.4 is stationary but the current feeding the electromagnet that produces the magnetic field is gradually reduced so that the field decreases from its initial value of 0.3T at the rate of 0.02\: T s^{-1} . If the cut is joined and the loop has a resistance of 1.6\Omega , how much power is dissipated by the loop as heat? What is the source of this power?

Answer:

Given,

Area of the rectangular loop which is held still:

A = l*b=(0.08)(0.02)m^2=16*10^{-4}

The resistance of the loop:

R=1.6\Omega

The initial value of the magnetic field :

B_{initial}= 0.3T

Rate of decreasing of this magnetic field:

\frac{dB}{dt}=0.02T/s

Induced emf in the loop :

e=\frac{d\phi }{dt}=\frac{d(BA)}{dt}=A\frac{dB}{dt}=16*10^{-4}*0.02=0.32*10^{-4}V

Induced Current :

I_{induced}=\frac{e}{R}=\frac{0.32*10^{-4}}{1.6}=2*10^{-5}A

The power dissipated in the loop:

P=I_{induced}^2R=(2*10^{-5})^2*1.6=6.4*10^{-10}W

The external force which is responsible for changing the magnetic field is the actual source of this power.

Q6.12 A square loop of side 12 cm with its sides parallel to X and Y axes is moved with a velocity of 8\: cm\: s^{-1} in the positive x-direction in an environment containing a magnetic field in the positive z-direction. The field is neither uniform in space nor constant in time. It has a gradient of 10^{-3}Tcm^{-1} along the negative x-direction (that is it increases by 10^{-3}Tcm^{-1} as one moves in the negative x-direction), and it is decreasing in time at the rate of 10^{-3}Ts^{-1} . Determine the direction and magnitude of the induced current in the loop if its resistance is 4.50m\Omega .

Answer:

Given,

Side of the square loop

l=12cm=0.12m

Area of the loop:

A=0.12*0.12m^2=144*10^{-4}m^2

The resistance of the loop:

R=4.5m\Omega = 4.5*10^{-3}\Omega

The velocity of the loop in the positive x-direction

v=8cm/s=0.08m/s

The gradient of the magnetic field in the negative x-direction

\frac{dB}{dx}=10^{-3}T/cm=10^{-1}T/m

Rate of decrease of magnetic field intensity

\frac{dB}{dt}=10^{-3}T/s

Now, Here emf is being induced by means of both changing magnetic field with time and changing with space. So let us find out emf induced by both changing of space and time, individually.

Induced emf due to field changing with time:

e_{withtime}=\frac{d\phi }{dt}=A\frac{dB}{dt}=144*10^{-4}*10^{-3}=1.44*10^{-5}Tm^2/s

Induced emf due to field changing with space:

e_{withspace}=\frac{d\phi }{dt}=\frac{d(BA)}{dt}=A\frac{dB}{dx}\frac{dx}{dt}=A\frac{dB}{dx}v

e_{withspace}=144*10^{4}*10^{-1}*0.08=11.52*10^{-5}Tm^2/s

Now, Total induced emf :

e_{total}=e_{withtime}+e_{withspace}=1.44*10^{-5}+11.52*10^{-5}=12.96*10^{-5}V

Total induced current :

I=\frac{e}{R}=\frac{12.96*10^{-5}}{4.5*10^{-3}}=2.88*10^{-2}A

Since the flux is decreasing, the induced current will try to increase the flux through the loop along the positive z-direction.

Q6.13 It is desired to measure the magnitude of field between the poles of a powerful loud speaker magnet. A small flat search coil of area 2cm^{2} with 25 closely wound turns, is positioned normal to the field direction, and then quickly snatched out of the field region. Equivalently, one can give it a quick 90^{0} turn to bring its plane parallel to the field direction). The total charge flown in the coil (measured by a ballistic galvanometer connected to coil) is 7.5mC . The combined resistance of the coil and the galvanometer is 0.50\Omega . Estimate the field strength of magnet.

Answer:

Given,

Area of search coil :

A=2cm^2=2*10^{-4}m^2

The resistance of coil and galvanometer

R=0.5\Omega

The number of turns in the coil:

N=25

Charge flowing in the coil

Q=7.5mC=7.5*10^{-3}C

Now.

Induced emf in the search coil

e=N\frac{d\phi }{dt}=N\frac{\phi _{final}-\phi _{initial}}{dt}=N\frac{BA-0}{dt}=\frac{NBA}{dt}

e=iR=\frac{dQ}{dt}R=\frac{NBA}{dt}

B=\frac{RdQ}{NA}=\frac{0.5*7.5*10^{-3}}{25*2*10^{-4}}=0.75T

Hence magnetic field strength for the magnet is 0.75T.

Q6.14 (a) Figure shows a metal rod PQ resting on the smooth rails AB and positioned between the poles of a permanent magnet. The rails, the rod, and the magnetic field are in three mutual perpendicular directions. A galvanometer G connects the rails through a switch K . Length of the rod=15cm , B=0.50T , resistance of the closed loop containing the rod=9.0m\Omega . Assume the field to be uniform. Suppose K is open and the rod is moved with a speed of 12cm\: s^{-1} in the direction shown. Give the polarity and magnitude of the induced emf.

1594626420269

Answer:

Given

Length of the rod

l=15cm=0.15m

Speed of the rod

v=12cm/s=0.12m/s

Strength of the magnetic field

B=0.5T

induced emf in the rod

e=Bvl=0.5*0.12*0.15=9*10^{-3}V

Hence 9mV emf is induced and it is induced in a way such that P is positive and Q is negative.

Q6.14 (b) Figure 6.20 shows a metal rod PQ resting on the smooth rails AB and positioned between the poles of a permanent magnet. The rails, the rod, and the magnetic field are in three mutual perpendicular directions. A galvanometer G connects the rails through a switch K . Length of the rod=15cm , B=0.50T , resistance of the closed loop containing the rod=9.0m\Omega . Assume the field to be uniform. Suppose K is open and the rod is moved with a speed of 12cm\: s^{-1} in the direction shown. Give the polarity and magnitude of the induced emf.

(b)Is there an excess charge built up at the ends of the rods when K is open? What if K is closed?

1594626446740

Answer:

Yes, there will be excess charge built up at the end of the rod when the key is open. This is because when we move the conductor in a magnetic field, the positive and negative charge particles will experience the force and move into the corners.

When we close the key these charged particles start moving in the closed loop and continuous current starts flowing.

Q6.14 (c) Figure 6.20 shows a metal rod PQ resting on the smooth rails AB and positioned between the poles of a permanent magnet. The rails, the rod, and the magnetic field are in three mutual perpendicular directions. A galvanometer G connects the rails through a switch K . Length of the rod=15cm , B=0.50T , resistance of the closed loop containing the rod=9.0m\Omega . Assume the field to be uniform. Suppose K is open and the rod is moved with a speed of 12cm\: s^{-1} in the direction shown. Give the polarity and magnitude of the induced emf.

(c) With K open and the rod moving uniformly, there is no net force on the electrons in the rod PQ even though they do experience magnetic force due to the motion of the rod. Explain.

1594626450814

Answer:

When the key K is open there is excess charge at both ends of the rod. this charged particle creates an electric field between both ends. This electric field exerts electrostatic force in the charged particles which cancel out the force due to magnetic force. That's why net force on a charged particle, in this case, is zero.

Q6.14 (d) Figure 6.20 shows a metal rod PQ resting on the smooth rails AB and positioned between the poles of a permanent magnet. The rails, the rod, and the magnetic field are in three mutual perpendicular directions. A galvanometer G connects the rails through a switch K . Length of the rod=15cm , B=0.50T , resistance of the closed loop containing the rod=9.0m\Omega . Assume the field to be uniform. Suppose K is open and the rod is moved with a speed of 12cm\: s^{-1} in the direction shown. Give the polarity and magnitude of the induced emf.

(d) what is the retarding force on the rod when K is closed?

1594626454785

Answer:

Induced emf = 9mV (calculated in a part of this question)

The resistance of loop with rod = 9 m\Omega

Induced Current

i = \frac{e}{R}=\frac{9mV}{9m\Omega }=1A

Now,'

Force on the rod

F= Bil= 0.5*1*0.15=7.5*10^{-2}

Hence retarding force when k is closed is 7.5*10^{-2} .

Q6.14 (e) Figure 6.20 shows a metal rod PQ resting on the smooth rails AB and positioned between the poles of a permanent magnet. The rails, the rod, and the magnetic field are in three mutual perpendicular directions. A galvanometer G connects the rails through a switch K . Length of the rod=15cm , B=0.50T , resistance of the closed loop containing the rod=9.0m\Omega . Assume the field to be uniform. Suppose K is open and the rod is moved with a speed of 12cm\: s^{-1} in the direction shown. Give the polarity and magnitude of the induced emf.

(e) How much power is required (by an external agent) to keep the rod moving at the same speed (=12cm\: s^{-1}) when K is closed? How much power is required when K is open?

1594626459136

Answer:

Force on the rod

F=7.5*10^{-2}

Speed of the rod

v=12cm/s=0.12m/s

Power required to keep moving the rod at the same speed

P=Fv=7.5*10^{-2}*0.12=9*10^{-3}=9mW

Hence required power is 9mW.

When the key is open, no power is required to keep moving rod at the same speed.

Q6.14 (f) Figure 6.20 shows a metal rod PQ resting on the smooth rails AB and positioned between the poles of a permanent magnet. The rails, the rod, and the magnetic field are in three mutual perpendicular directions. A galvanometer G connects the rails through a switch K . Length of the rod=15cm , B=0.50T , resistance of the closed loop containing the rod=9.0m\Omega . Assume the field to be uniform. Suppose K is open and the rod is moved with a speed of 12cm\: s^{-1} in the direction shown. Give the polarity and magnitude of the induced emf

(f) How much power is dissipated as heat in the closed circuit? What is the source of this power?

1594626463614

Answer:

Current in the circuit i = 1A

The resistance of the circuit R = 9m \Omega

The power which is dissipated as the heat

P_{heat}=i^2R=1^2*9*10^{-3}=9mW

Hence 9mW of heat is dissipated.

We are moving the rod which induces the current. The external agent through which we are moving our rod is the source of the power.

Q6.14 (g) Figure 6.20 shows a metal rod PQ resting on the smooth rails AB and positioned between the poles of a permanent magnet. The rails, the rod, and the magnetic field are in three mutual perpendicular directions. A galvanometer G connects the rails through a switch K . Length of the rod=15cm , B=0.50T , resistance of the closed loop containing the rod=9.0m\Omega . Assume the field to be uniform. Suppose K is open and the rod is moved with a speed of 12cm\: s^{-1} in the direction shown. Give the polarity and magnitude of the induced emf

(g) What is the induced emf in the moving rod if the magnetic field is parallel to the rails instead of being perpendicular?

1594626473478

Answer:

If the magnetic field is parallel to the rail then, the motion of the rod will not cut across the magnetic field lines and hence no emf will induce. Hence emf induced is zero in this case.

Q6.15 An air-cored solenoid with length 30cm , area of cross-section 25cm^{2} and number of turns 500 , carries a current of 2.5A . The current is suddenly switched off in a brief time of 10^{-3}s . How much is the average back emf induced across the ends of the open switch in the circuit? Ignore the variation in magnetic field near the ends of the solenoid.

Answer:

Given

Length od the solenoid l=30cm=0.3m

Area of the cross-section of the solenoid A=25cm^2=25*10^{-4}m^2

Number of turns in the solenoid N=500

Current flowing in the solenoid I=2.5A

The time interval for which current flows \Delta t=10^{-3}s

Now.

Initial flux:

\phi _{initial}=NBA=N\left ( \frac{\mu _0NI}{l} \right )A=\frac{\mu _0N^2IA}{l}

\phi _{initial}=\frac{4\pi*10^{-7}*500^2*2.5*25*10^{-4}}{0.3}=6.55*10^{-3}Wb

Final flux: since no current is flowing,

\phi _{final}=0

Now

Induced emf:

e=\frac{d\phi}{dt}=\frac{\Delta \phi}{\Delta t}=\frac{\phi_{final}-\phi_{initial}}{\Delta t}=\frac{6.55*10^{-3}-0}{10^{-3}}=6.55V

Hence 6.55V of average back emf is induced.

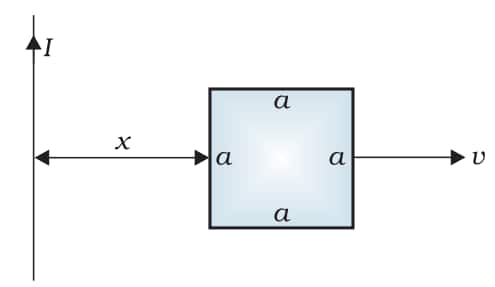
Q6.16 (a) Obtain an expression for the mutual inductance between a long straight wire and a square loop of side a as shown in Fig.

1594626584534

Answer:

Here let's take a small element dy in the loop at y distance from the wire

Area of this element dy :

dA=a*dy

The magnetic field at dy (which is y distance away from the wire)

B=\frac{\mu _0I}{2\pi y}

The magnetic field associated with this element dy

d\phi =BdA

d\phi=\frac{\mu _0I}{2\pi y}*ady=\frac{\mu _0Ia}{2\pi}\frac{dy}{y}

\phi=\int_{x}^{a+x}\frac{\mu _0Ia}{2\pi}\frac{dy}{y}=\frac{\mu _0Ia}{2\pi}[lnx]^{a+x}_a=\frac{\mu _0Ia}{2\pi}ln[\frac{a+x}{x}]


Now As we know

\phi=MI where M is the mutual inductance

so

\phi=MI=\frac{\mu _0Ia}{2\pi}ln[\frac{a+x}{x}]

M=\frac{\mu _0a}{2\pi}ln[\frac{a+x}{x}]

Hence mutual inductance between the wire and the loop is:

\frac{\mu _0a}{2\pi}ln[\frac{a+x}{x}]

Q6.16 (b) Now assume that the straight wire carries a current of 50A and the loop is moved to the right with a constant velocity, V=10m/s . Calculate the induced emf in the loop at the instant when X=0.2m . Take a=0.1m and assume that the loop has a large resistance.

Answer:

Given,

Current in the straight wire

I = 50A

Speed of the Loop which is moving in the right direction

V=10m/s

Length of the square loop

a=0.1m

distance from the wire to the left side of the square

X=0.2m

NOW,

Induced emf in the loop :

E=B_xav=\frac{\mu _0I}{2\pi x}av=\left ( \frac{4\pi*10^{-7}*50}{2\pi*0.2} \right )*0.1*10=5*10^{-5}V

Hence emf induced is 5*10^{-5}V .

6.17) A line charge \lambda per unit length is lodged uniformly onto the rim of a wheel of mass M and radius R . The wheel has light non-conducting spokes and is free to rotate without friction about its axis (Fig. 6.22). A uniform magnetic field extends over a circular region within the rim. It is given by, B=\: -B_{0}K\: \: \: \: (r\leq a;\: a< R) =0\: \: \: \: \: \: \: \: \: \: (otherwise)
What is the angular velocity of the wheel after the field is suddenly switched off?

15946266972741594626694082

Answer:

Given,

The radius of the wheel =R

The mass of the wheel = M

Line charge per unit length when the total charge is Q

\lambda=\frac{Q}{2\pi r}

Magnetic field :

B=\: -B_{0}K\: \: \: \: (r\leq a;\: a< R) =0

Magnetic force is balanced by centrifugal force when v is the speed of the wheel that is

BQv=\frac{mv^2}{r}

B2\pi r\lambda=\frac{Mv}{r}

v=\frac{B2\pi \lambda r^2}{M}

Angular velocity of the wheel

w=\frac{v}{r}=\frac{B2\pi \lambda r^2}{MR}

when (r\leq a;\: a< R)

w=-\frac{B2\pi \lambda a^2}{MR}

It is the angular velocity of the wheel when the field is suddenly shut off.

In the Class 10th NCERT book, a brief idea of Electromagnetic Induction is given. Physics chapter 6 Class 12 give a more detailed explanation of Electromagnetic Induction and the NCERT solutions for Class 12 Physics Chapter 6 list some questions and solutions on the concepts covered in ch 6 Physics Class 12. Questions from the following topics are covered in the Electromagnetic Induction Class 12 solutions.

  • The experiment of Faraday and Henry, magnetic flux and Faraday's laws related to Electromagnetic Induction- This part of chapter 6 Class 12 Physics discuss the relation between magnetic field, flux and emf through experiments and equations.

  • Lenz's law- This law gives the polarity of induced emf.

  • Motional emf and its equation

  • Lenz's law and laws of conservation of energy-This section of ch 6 Physics Class 12 give quantitative relation of, force, power and energy.

  • Eddy current methods to reduce the effect of eddy current

  • Concept of self and mutual induction and its quantitative relation

  • AC generator and it's working.

JEE Main Highest Scoring Chapters & Topics
Just Study 40% Syllabus and Score upto 100%
Download EBook

NCERT solutions for class 12 physics chapter wise

NCERT solutions for Class 12 Physics chapter 1 Electric Charges and Fields
NCERT solutions for Class 12 Physics chapter 2 Electrostatic Potential and Capacitance
NCERT solutions for Class 12 Physics chapter 3 Current Electricity
NCERT solutions for Class 12 Physics chapter 4 Moving Charges and Magnetism
NCERT solutions for Class 12 Physics chapter 5 Magnetism and Matter
NCERT solutions for Class 12 Physics chapter 6 Electromagnetic Induction
NCERT solutions for Class 12 Physics chapter 7 Alternating Current
NCERT solutions for Class 12 Physics chapter8 Electromagnetic Waves
NCERT solutions for Class 12 Physics chapter 9 Ray Optics and Optical Instruments
NCERT solutions for Class 12 Physics chapter 10 Wave Optics Solutions
NCERT solutions for Class 12 Physics chapter 11 Dual nature of radiation and matter
NCERT solutions for Class 12 Physics chapter 12 Atoms
NCERT solutions for Class 12 Physics chapter 13 Nuclei
NCERT solutions for Class 12 Physics chapter 14 Semiconductor Electronics Materials Devices and Simple Circuits

Also Check NCERT Books and NCERT Syllabus here:

Electromagnetic induction class 12 exercise solutions: Important Formulas and Diagrams

Following are the important formulas covered in the chapter 6 physics class 12 ncert solutions Electromagnetic Induction-

  • Relation between flux and magnetic field: \phi=B.A=BAcos\theta

  • Induced emf and magnetic field: E=-N\frac{d\phi}{dt}

  • Motional emf: E=-Blv (B is perpendicular to v)

  • Self-induced emf: E=-L\frac{di}{dt}

  • Self-inductance: L=\mu_r\mu_0 n^2Al

  • Motional emf(ac generator): E=NBA2\pi\nu sin(2\pi\nu t)

Importance of NCERT solutions for class 12 physics chapter 6 electromagnetic induction in exams:

  • The Class 12 Physics ch 6 NCERT solutions, is not only important for CBSE Class 12 board exams but also for many competitive exams like KVPY, NEET and JEE Main.

  • For exams like NEET and JEE Mains questions based on finding the direction of induced emf are expected. There are many such questions in the Electromagnetic Induction NCERT solutions.

  • On average 10% of questions are asked in the CBSE board exam from the chapters Electromagnetic Induction and alternating current.

Key Features of Electromagnetic Induction Class 12 Solutions

  1. Comprehensive Understanding: These solutions comprehensively cover all the topics and questions in the chapter, ensuring a deep understanding of electromagnetic induction.

  2. Step-by-Step Clarity: Each electromagnetic induction class 12 ncert solutions offers clear step-by-step explanations, making intricate concepts easily digestible for students.

  3. Accessible Language: The electromagnetic induction class 12 exercise solutions are presented in simple and understandable language, aiding students in grasping the content effortlessly.

  4. Effective Practice: Chapter 6 physics class 12 ncert solutions included in the solutions enable students to practice and assess their comprehension effectively.

  5. Exam Readiness: These class 12 physics chapter 6 ncert solutions are an essential tool for board exam preparation and are also beneficial for competitive exams.

  6. Foundation for Advanced Study: The chapter's concepts lay the foundation for more advanced physics and electrical engineering topics.

  7. No Cost Access: Students can access these class 12 electromagnetic induction ncert solutions for free, ensuring equal accessibility to all.

These features collectively make class 12 physics electromagnetic induction ncert solutions a valuable resource, facilitating exam success and future academic pursuits.

NCERT Exemplar Class 12 Solutions


NCERT solutions subject wise:

Frequently Asked Questions (FAQs)

1. Is the class 12 chapter Electromagnetic Induction helpful for higher studies?

Yes, the chapter is helpful for higher studies in the field of electronics and electrical engineering-related subjects and for scientific research.

2. How important is the chapter Electromagnetic Induction for CBSE board exam?

On an average 5 mark questions are asked for CBSE board exams. Follow the NCERT syllabus and NCERT book for a good score in the board exam. Along with the NCERT solutions exercises can also practice NCERT exemplar problems and CBSE board previous year papers.

Also Read

Articles

Upcoming School Exams

National Institute of Open Schooling 12th Examination

Admit Card Date:04 October,2024 - 29 November,2024

National Institute of Open Schooling 10th examination

Admit Card Date:04 October,2024 - 29 November,2024

Mizoram Board of School Education 12th Examination

Application Date:07 October,2024 - 22 November,2024

Mizoram Board 10th Examination

Application Date:07 October,2024 - 22 November,2024

Punjab Board of Secondary Education 10th Examination

Application Correction Date:08 October,2024 - 27 November,2024

View All School Exams

Certifications By Top Providers

Edx
 1113 courses
Coursera
 812 courses
Udemy
 394 courses
Futurelearn
 295 courses
Harvard University, Cambridge
 98 courses
IBM
 83 courses

Explore Top Universities Across Globe

University of Essex, Colchester
  Wivenhoe Park Colchester CO4 3SQ
University College London, London
  Gower Street, London, WC1E 6BT
The University of Edinburgh, Edinburgh
  Old College, South Bridge, Edinburgh, Post Code EH8 9YL
University of Bristol, Bristol
  Beacon House, Queens Road, Bristol, BS8 1QU
University of Delaware, Newark
  Newark, DE 19716
University of Nottingham, Nottingham
  University Park, Nottingham NG7 2RD

Related E-books & Sample Papers

Questions related to CBSE Class 12th

Have a question related to CBSE Class 12th ?
quartely question paper for mathematics cbse

Hello there! Thanks for reaching out to us at Careers360.

Ah, you're looking for CBSE quarterly question papers for mathematics, right? Those can be super helpful for exam prep.

Unfortunately, CBSE doesn't officially release quarterly papers - they mainly put out sample papers and previous years' board exam papers. But don't worry, there are still some good options to help you practice!

Have you checked out the CBSE sample papers on their official website? Those are usually pretty close to the actual exam format. You could also look into previous years' board exam papers - they're great for getting a feel for the types of questions that might come up.

If you're after more practice material, some textbook publishers release their own mock papers which can be useful too.

Let me know if you need any other tips for your math prep. Good luck with your studies!

Read Complete Answer
Jefferson 25 September,2024
i have taken admission in clg after my campartment exam in chemistry along with the neet 2025 preperation but unfortunately i again got campartment after doing all this preparation i got 15 marks only now I can't able to think what should I do next.

It's understandable to feel disheartened after facing a compartment exam, especially when you've invested significant effort. However, it's important to remember that setbacks are a part of life, and they can be opportunities for growth.

Possible steps:

  1. Re-evaluate Your Study Strategies:

    • Identify Weak Areas: Pinpoint the specific topics or concepts that caused difficulties.
    • Seek Clarification: Reach out to teachers, tutors, or online resources for additional explanations.
    • Practice Regularly: Consistent practice is key to mastering chemistry.

  2. Consider Professional Help:

    • Tutoring: A tutor can provide personalized guidance and support.
    • Counseling: If you're feeling overwhelmed or unsure about your path, counseling can help.

  3. Explore Alternative Options:

    • Retake the Exam: If you're confident in your ability to improve, consider retaking the chemistry compartment exam.
    • Change Course: If you're not interested in pursuing chemistry further, explore other academic options that align with your interests.

  4. Focus on NEET 2025 Preparation:

    • Stay Dedicated: Continue your NEET preparation with renewed determination.
    • Utilize Resources: Make use of study materials, online courses, and mock tests.

  5. Seek Support:

    • Talk to Friends and Family: Sharing your feelings can provide comfort and encouragement.
    • Join Study Groups: Collaborating with peers can create a supportive learning environment.

Remember: This is a temporary setback. With the right approach and perseverance, you can overcome this challenge and achieve your goals.

I hope this information helps you.







Read Complete Answer
Kanishka kaushikii 17 September,2024
i scored 84 percent in cbse 2 years ago.Am i eligible for medhavi scholarship if give 12th again after 2 drop years and will got 75+ in mpboard

Hi,

Qualifications:
Age: As of the last registration date, you must be between the ages of 16 and 40.
Qualification: You must have graduated from an accredited board or at least passed the tenth grade. Higher qualifications are also accepted, such as a diploma, postgraduate degree, graduation, or 11th or 12th grade.
How to Apply:
Get the Medhavi app by visiting the Google Play Store.
Register: In the app, create an account.
Examine Notification: Examine the comprehensive notification on the scholarship examination.
Sign up to Take the Test: Finish the app's registration process.
Examine: The Medhavi app allows you to take the exam from the comfort of your home.
Get Results: In just two days, the results are made public.
Verification of Documents: Provide the required paperwork and bank account information for validation.
Get Scholarship: Following a successful verification process, the scholarship will be given. You need to have at least passed the 10th grade/matriculation scholarship amount will be transferred directly to your bank account.

Scholarship Details:

Type A: For candidates scoring 60% or above in the exam.

Type B: For candidates scoring between 50% and 60%.

Type C: For candidates scoring between 40% and 50%.

Cash Scholarship:

Scholarships can range from Rs. 2,000 to Rs. 18,000 per month, depending on the marks obtained and the type of scholarship exam (SAKSHAM, SWABHIMAN, SAMADHAN, etc.).

Since you already have a 12th grade qualification with 84%, you meet the qualification criteria and are eligible to apply for the Medhavi Scholarship exam. Make sure to prepare well for the exam to maximize your chances of receiving a higher scholarship.

Hope you find this useful!

Read Complete Answer
Yuvan 30 August,2024
i upload 12th board result screenshot in ncweb form from the cbse site so it is valid

hello mahima,

If you have uploaded screenshot of your 12th board result taken from CBSE official website,there won,t be a problem with that.If the screenshot that you have uploaded is clear and legible. It should display your name, roll number, marks obtained, and any other relevant details in a readable forma.ALSO, the screenshot clearly show it is from the official CBSE results portal.

hope this helps.

Read Complete Answer
Ashvadha 12 July,2024
Kya mujhe class 12 ka important question milega jo exam mai per year puche jate hai

Hello Akash,

If you are looking for important questions of class 12th then I would like to suggest you to go with previous year questions of that particular board. You can go with last 5-10 years of PYQs so and after going through all the questions you will have a clear idea about the type and level of questions that are being asked and it will help you to boost your class 12th board preparation.

You can get the Previous Year Questions (PYQs) on the official website of the respective board.

I hope this answer helps you. If you have more queries then feel free to share your questions with us we will be happy to assist you.

Thank you and wishing you all the best for your bright future.

Read Complete Answer
kumardurgesh1802 10 July,2024
View All

Popular CBSE Class 12th Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1)

0.34\; J

 Option 2)

0.16\; J
Option 3)

1.00\; J

 Option 4)

0.67\; J
Read More

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times.  Assume that the potential energy lost each time he lowers the mass is dissipated.  How much fat will he use up considering the work done only when the weight is lifted up ?  Fat supplies 3.8×107 J of energy per kg which is converted to mechanical energy with a 20% efficiency rate.  Take g = 9.8 ms−2 :

Option 1)

2.45×10−3 kg

 Option 2)

 6.45×10−3 kg
Option 3)

 9.89×10−3 kg

 Option 4)

12.89×10−3 kg

 
Read More

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1)

2,000 \; J - 5,000\; J

 Option 2)

200 \, \, J - 500 \, \, J
Option 3)

2\times 10^{5}J-3\times 10^{5}J

 Option 4)

20,000 \, \, J - 50,000 \, \, J
Read More

A particle is projected at 600   to the horizontal with a kinetic energy K. The kinetic energy at the highest point

Option 1)

K/2\,

 Option 2)

\; K\;
Option 3)

zero\;

 Option 4)

K/4
Read More

In the reaction,

2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}

Option 1)

11.2\, L\, H_{2(g)}  at STP  is produced for every mole HCL_{(aq)}  consumed

 Option 2)

6L\, HCl_{(aq)}  is consumed for ever 3L\, H_{2(g)}      produced
Option 3)

33.6 L\, H_{2(g)} is produced regardless of temperature and pressure for every mole Al that reacts

 Option 4)

67.2\, L\, H_{2(g)} at STP is produced for every mole Al that reacts .
Read More

How many moles of magnesium phosphate, Mg_{3}(PO_{4})_{2} will contain 0.25 mole of oxygen atoms?

Option 1)

0.02

 Option 2)

3.125 × 10-2
Option 3)

1.25 × 10-2

 Option 4)

2.5 × 10-2
Read More

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1)

decrease twice

 Option 2)

increase two fold
Option 3)

remain unchanged

 Option 4)

be a function of the molecular mass of the substance.
Read More

With increase of temperature, which of these changes?

Option 1)

Molality

 Option 2)

Weight fraction of solute
Option 3)

Fraction of solute present in water

 Option 4)

Mole fraction.
Read More

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol-1) is

Option 1)

twice that in 60 g carbon

 Option 2)

6.023 × 1022
Option 3)

half that in 8 g He

 Option 4)

558.5 × 6.023 × 1023
Read More

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t2) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m2 , the number of rotations made by the pulley before its direction of motion if reversed, is

Option 1)

less than 3

 Option 2)

more than 3 but less than 6
Option 3)

more than 6 but less than 9

 Option 4)

more than 9
Read More

Colleges After 12th

Applications for Admissions are open.

VMC VIQ Scholarship Test
Apply

Register for Vidyamandir Intellect Quest. Get Scholarship and Cash Rewards.

JEE Main Important Physics formulas
Apply

As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters

JEE Main Important Chemistry formulas
Apply

As per latest 2024 syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters

TOEFL ® Registrations 2024
Apply

Accepted by more than 11,000 universities in over 150 countries worldwide

Pearson | PTE
Apply

Register now for PTE & Unlock 20% OFF : Use promo code: 'C360SPL20'. Valid till 30th NOV'24! Trusted by 3,500+ universities globally

JEE Main high scoring chapters and topics
Apply

As per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE

View All Application Forms
News and Notifications
Board Exam Time Table 2025 Live: UP Board exams from February 24; CBSE, BSEB date sheet soon
November 20, 2024 - 10:27 AM IST
CBSE Date Sheet 2025 Live: Class 10, 12 board exam dates soon; syllabus, sample papers
November 18, 2024 - 09:47 PM IST
Education News This Week: Fake news on CBSE exams; UPPSC protests, crackdown on coaching ads
November 17, 2024 - 01:51 PM IST
Extra time in CBSE exams for students with type 1 diabetes: Kerala SHRC seeks report on plea
November 16, 2024 - 09:45 PM IST
No changes in exam, IA system: CBSE rebuts claims of syllabus reduction, open book exams in 2025
November 14, 2024 - 07:31 PM IST
Fact-check: CBSE hasn't reduced syllabus by 15%, internal assessments will not be 40% of score in 2025
November 14, 2024 - 02:32 PM IST
CBSE date sheet 2025 for Classes 10, 12 likely in November last week: Reports
November 08, 2024 - 06:29 PM IST

Stay up-to date with CBSE Class 12th News

Back to top