NCERT Solutions for Class 12 Physics Chapter 7 Alternating current is a crucial and scoring chapter in the Class 12 syllabus. This NCERT solution page offers detailed step-by-step solutions prepared by physics experts from Careers360. It covers a total of twenty-six questions, including those from 7.1 to 7.11 in the exercise section and the remaining in the additional exercise section.

This Story also Contains

NCERT Solutions for Class 12 Physics Chapter 7 – Access and Download Free PDF
NCERT Solutions for Class 12 Physics Chapter 7 Alternating Current
Class 12 Physics Chapter 7 Exercise Solutions
NCERT Class 12 Physics Chapter 7 Exercise Solutions: Additional Exercise
NCERT Solutions for class 12 physics: Chapter-Wise
NCERT Class 12 Physics Chapter 7 Alternating Current: Important topics
Key Features of Class 12 Physics NCERT Solutions for Chapter 7 Alternating Current
Also Check NCERT Books and NCERT Syllabus here:

NCERT Solutions for Class 12 Physics Chapter 7 Alternating Current

The supply that is received in our home is alternating in nature. Do you know what is the value of the normal supply voltage that is coming into our home and what is the supply frequency? Alternating Current Class 12 chapter will help you to clear such doubts. NCERT questions explained in CBSE NCERT solutions for Class 12 physics chapter 7 are based on the single-phase alternating current.

Alternating Current Class 12 NCERT solutions are the basics for the topics needed to study for CBSE board exam. Students should go through the Alternating Current NCERT solutions to know the answer to the question of Alternating current. In this class 12 physics chapter 7 exercise solutions you will study the concept of phasor diagrams and related problems.

Free download ncert class 12 physics chapter 7 exercise solutions PDF for CBSE exam.

NCERT Solutions for Class 12 Physics Chapter 7 Alternating Current

Download PDF

Class 12 Physics Chapter 7 Exercise Solutions

Q7.1 (a) A $100\Omega$ resistor is connected to a $220\: V$ , $50\: Hz$ ac supply.

a)what is the RMS value of current?

Answer:

Given,

RMS voltage in the circuit $V_{rms}=220V$

Resistance in the circuit $R=100\Omega$

Now,

RMS current in the circuit:

$I_{rms}=\frac{V_{rms}}{R}=\frac{220}{100}=2.2A$

Hence, the RMS value of current is 2.2A.

Q7.1 (b) A $100W$ resistor is connected to a $220\: V$ , $50Hz$ ac supply.

What is the rms value of current in the circuit?

Answer:

Given,

RMS Voltage in the circuit $V_{rms}=200V$

Resistance in the circuit $R=100\Omega$

Now,

RMS Current in the circuit:

$I_{rms}=\frac{V_{rms}}{R}=\frac{220}{100}=2.2A$

Hence, the RMS value of current is 2.2A.

Q7.1 (c) A $100\Omega$ resistor is connected to a $220\: V$ , $50\: Hz$ ac supply.

What is the net power consumed over a full cycle?

Answer:

Given,

Supplied RMS Voltage $V_{rms}=220V$

Supplied RMS Current $I_{rms}=2.2A$

The net power consumed over a full cycle:

$P=V_{rms}I_{rms}=220*2.2=484W$

Hence net power consumed is 484W.

Q7.2 (a) The peak voltage of an ac supply is $300 V$ . What is the RMS voltage?

Answer:

Given

Peak Value of ac supply:

$V_{peak}=300V$

Now as we know in any sinusoidal function

$RMSvalue=\frac{peakvalue}{\sqrt{2}}$

Since our ac voltage supply is also sinusoidal

$V_{rms}=\frac{V_{peak}}{\sqrt{2}}=\frac{300}{\sqrt{2}}=212.13V$

Hence RMS value of voltage os 212.13V.

Q7.2 (b) The RMS value of current in an ac circuit is $10\: A$ . What is the peak current?

Answer:

Given,

RMS value of current $I_{rms}=10A$

Since Current is also sinusoidal (because only resistance is present in the circuit, not the capacitor and inductor)

$I_{rms}=\frac{I_{peak}}{\sqrt{2}}$

$I_{peak}=\sqrt{2}I_{rms}=\sqrt{2}*10=14.1A$

Hence the peak value of current is 14.1A.

Q7.3 A $44\: mH$ inductor is connected to $220\: V$ , $50\: Hz$ ac supply. Determine the RMS value of the current in the circuit.

Answer:

Given

Supply Voltage $V=220V$

Supply Frequency $f=50Hz$

The inductance of the inductor connected $L=44mH=44*10^{-3}H$

Now

Inductive Reactance

$X_L=\omega L=2\pi fL=2\pi *50*44*10^{-3}$

RMS Value of the current :

$I_{rms}=\frac{V_{rms}}{X_L}= \frac{220}{2\pi *50*44*10^{-3}}=15.92A$

Hence the RMS Value of current is 15.92A.

Q7.4 A $60\mu F$ capacitor is connected to a $100\: V$ , $60\; Hz$ ac supply. Determine the rms value of the current in the circuit.

Answer:

Given,

Supply Voltage $V = 110V$

Supply Frequency $f=60Hz$

The capacitance of the connected capacitor $C=60\mu F=60*10^{-6}F$

Now,

Capacitive Reactance

$X_C=\frac{1}{\omega C}=\frac{1}{2\pi fC}=\frac{1}{2\pi *60*60*10^{-6}}$

RMS Value of current

$I_{rms}=\frac{V_{rms}}{X_C}=V\omega C=V2\pi fC=110*2\pi *60*60*10^{-6}=2.49A$

Hence the RMS Value of current is 2.49A.

Q7.5 In Exercises 7.3 and 7.4, what is the net power absorbed by each circuit over a complete cycle. Explain your answer.

Answer:

As we know,

Power absorbed $P=VIcos\phi$

Where $\phi$ is the phase difference between voltage and current.

$\phi$ for the inductive circuit is -90 degree and $\phi$ for the capacitive circuit is +90 degree.

In both cases (inductive and capacitive), the power absorbed by the circuit is zero because in both cases the phase difference between current and voltage is 90 degree.

This can be seen as The elements(Inductor and Capacitor) are not absorbing the power, rather storing it. The capacitor is storing energy in electrostatic form and Inductor is storing the energy in magnetic form.

Q7.6 Obtain the resonant frequency $\omega _{r}$ of a series $LCR$ circuit with $L=2.0H$ , $C=32\mu F$ and $R=10\Omega$ . What is the Q -value of this circuit?

Answer:

Given, in a circuit,

Inductance, $L=2H$

Capacitance, $C=32\mu F=32*10^{-6}F$

Resistance, $R=10\Omega$

Now,

Resonance frequency (frequency of maximum current OR minimum impedance OR frequency at which inductive reactance cancels out capacitive reactance )

$\omega _r=\frac{1}{\sqrt{LC}}=\frac{1}{\sqrt{2*32*10^{-6}}}=\frac{1}{8*10^{-3}}=125s^{-1}$

Hence Resonance frequency is 125 per second.

Q-Value:

$Q=\frac{1}{R}\sqrt{\frac{L}{C}}=\frac{1}{10}\sqrt{\frac{2}{32*10^{-6}}}=25$

Hence Q - value of the circuit is 25.

Q7.7 A charged $30\mu F$ capacitor is connected to a $27mH$ inductor. What is the angular frequency of free oscillations of the circuit?

Answer:

Given

Capacitance $C=30\mu F=30*10^{-6}$

Inductance $L = 27mH = 27*10^{-3}H$

Now,

Angular Frequency

$\omega _r=\frac{1}{\sqrt{LC}}=\frac{1}{\sqrt{30*10^{-6}*27*10^{-3}}}=1.11*10^{3}rad/sec$

Hence Angular Frequency is $1.11*10^{3}rad/sec$

Q7.8 Suppose the initial charge on the capacitor in Exercise 7.7 is $6mC$ . What is the total energy stored in the circuit initially? What is the total energy at later time?

Answer:

Given

Capacitance $C=30\mu F=30*10^{-6}$

Inductance $L = 27mH = 27*10^{-3}H$

Charge on the capacitor $Q=6mC=6*10^{-3}C$

Now,

The total energy stored in Capacitor :

$E=\frac{Q^2}{2C}=\frac{(6*10^{-3})^2}{2*30*10^{-6}}=\frac{6}{10}=0.6J$

Total energy later will be same because energy is being shared with capacitor and inductor and none of them loses the energy, they just store it and transfer it.

Q7.9 A series LCR circuit with $R=20\Omega$ , $L=1.5H$ and $c=35\mu F$ is connected to a variable-frequency $200\; V$ ac supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power transferred to the circuit in one complete cycle?

Answer:

Given,

Resistance $R=20\Omega$

Inductance $L=1.5H$

Capacitance $C=35\mu F=35*10^{-6}F$

Voltage supply $V = 200V$

At resonance, supply frequency is equal to the natural frequency, and at the natural frequency, the total impedance of the circuit is equal to the resistance of the circuit

as inductive and capacitive reactance cancels each other. in other words,

$Z = \sqrt{\left ( \omega L-\frac{1}{\omega C} \right )^2+R^2}=\sqrt{0^2+R^2}=R=20\Omega$

$\omega L=\frac{1}{\omega C}$

Now,

Current in the circuit

$I=\frac{V}{Z}=\frac{200}{20}=10A$

Average Power transferred in the circuit :

$P=VI=200*10=2000W$

Hence average power transferred is 2000W.

Q7.10 A radio can tune over the frequency range of a portion of $MW$ broadcast band: $(800kHz\: to1200kHz)$ . If its $LC$ circuit has an effective inductance of $200\mu H$ , what must be the range of its variable capacitor?

Answer:

Given,

Range of the frequency in which radio can be tune = $(800kHz\: to1200kHz)$

The effective inductance of the Circuit = $200\mu H$

Now, As we know,

$w^2=1/\sqrt{LC}$

$C=1/w^2L$

where $w$ is tuning frequency.

For getting the range of the value of a capacitor, let's calculate the two values of the capacitor, one maximum, and one minimum.

first, let's calculate the minimum value of capacitance which is the case when tuning frequency = 800KHz.

$C_{minimum}=\frac{1}{w_{minimum}^2L}=\frac{1}{(2\pi(800*10^3))^2*200*10^{-6}}=1.981*10^{-10}F$

Hence the minimum value of capacitance is 198pF.

Now, Let's calculate the maximum value of the capacitor.

in this case, tuning frequency = 1200KHz

$C_{maximum}=\frac{1}{w_{maximum}^2L}=\frac{1}{(2\pi(1200*10^3))^2*200*10^{-6}}=88.04*10^{-12}F$

Hence the maximum value of the capacitor is 88.04pf

Hence the Range of the values of the capacitor is $88.04pF to 198.1pF$ .

Q7.11 (a) Figure shows a series LCR circuit connected to a variable frequency $230\: V$ source. $L=5.0H$ , $C=80\mu F$ , $R=40\Omega$ .

(a) Determine the source frequency which drives the circuit in resonance.

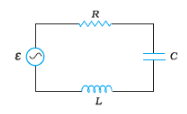
1594271673048

Answer:

Given,

Variable frequency supply voltage $V$ = 230V

Inductance $L=5.0H$

Capacitance $C=80\mu F=80*10^{-6}F$

Resistance $R=40\Omega$

a) Resonance angular frequency in this circuit is given by :

$w_{resonance}=\frac{1}{\sqrt{LC}}=\frac{1}{\sqrt{5*80*10^{-6}}}=\frac{1000}{20}=50rad/sec$

Hence this circuit will be in resonance when supply frequency is 50 rad/sec.

Q7.11 (b) Figure shows a series LCR circuit connected to a variable frequency $230\: V$ source.

$L=5.0H$ , $C=80\mu F$ , $R=40\Omega$ .

(b) Obtain the impedance of the circuit and the amplitude of current at the resonating frequency.

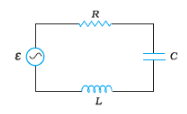
1594271689346

Answer:

Given,

Variable frequency supply voltage $V$ = 230V

Inductance $L=5.0H$

Capacitance $C=80\mu F=80*10^{-6}F$

Resistance $R=40\Omega$

Now,

The impedance of the circuit is

$Z=\sqrt{(wL-\frac{1}{wC})^2+R^2}$

at Resonance Condition

: $wL=\frac{1}{wC}$

$Z=R=40\Omega$

Hence, Impedance at resonance is 40 $\Omega$ .

Now, at resonance condition, impedance is minimum which means current is maximum which will happen when we have a peak voltage, so

Current in the Resonance circuit is Given by

$I_{resonance}=\frac{V_{peak}}{Z}=\frac{\sqrt{2}* 230}{40}=8.13A$

Hence amplitude of the current at resonance is 8.13A.

Q7.11 Figure shows a series LCR circuit connected to a variable frequency $230\: V$ source.

$L=5.0H$ , $C=80\mu F$ , $R=40\Omega$ .

Determine the rms potential drops across the three elements of the circuit. Show that the potential drop across the LC combination is zero at the resonating frequency.

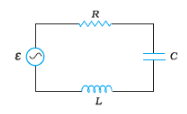
1594271702160

Answer:

Potential difference across any element = $I_{rms}*(impedance)$

$I_{rms}=\frac{I_{peak}}{\sqrt{2}}=\frac{8.13}{\sqrt{2}}=5.85A$

Now

The potential difference across the capacitor:

$V_{capacitor}=I_{rms}*\left (\frac{1}{w_{resonance}C} \right ) =5.85*\left ( \frac{1}{50*80*10^{-6}} \right )=1437.5V$

The potential difference across the inductor

$V_{inductor}=I_{rms}*(w_{resonance}L) =5.85* 50*5=1437.5V$

The potential difference across Resistor

=40 I_rms=230V

The potential difference across LC combination

$V_{LC}=I_{rms}*\left ( wL-\frac{1}{wC} \right )=5.85*0=0$

Hence at resonating, frequency potential difference across LC combination is zero.

NCERT Class 12 Physics Chapter 7 Exercise Solutions: Additional Exercise

Q7.12 (a) An $LC$ circuit contains a $20mH$ inductor and a $50\mu F$ capacitor with an initial charge of $10mC$ . The resistance of the circuit is negligible. Let the instant the circuit is closed be $t=0$ .

What is the total energy stored initially? Is it conserved during $LC$ oscillations?

Answer :

Given,

The inductance of the inductor:

$L=20mH=20*10^{-3}H$

The capacitance of the capacitor :

$C=50\mu F=50*10^{-6}F$

The initial charge on the capacitor:

$Q=10mC=10*10^{-3}C$

Total energy present at the initial moment:

$E_{initial}=\frac{Q^2}{2C}=\frac{(10*10^{-3})^2}{2*50*10^{-6}}=1J$

Hence initial energy in the circuit is 1J. Since we don't have any power-consuming element like resistance in the circuit, the energy will be conserved

Q7.12 (b) An $LC$ circuit contains a $20mH$ inductor and a $50\mu F$ capacitor with an initial charge of $10mC$ . The resistance of the circuit is negligible. Let the instant the circuit is closed be $t=0$ .

What is the natural frequency of the circuit?

Answer:

Given,

The inductance of the inductor:

$L=20mH=20*10^{-3}H$

The capacitance of the capacitor :

$C=50\mu F=50*10^{-6}F$

The initial charge on the capacitor:

$Q=10mC=10*10^{-3}C$

The natural angular frequency of the circuit:

$w_{natural}=\frac{1}{\sqrt{LC}}=\frac{1}{\sqrt{(20*10^{-3}*50*10^{-6})}}=10^3rad/sec$

Hence the natural angular frequency of the circuit is $10^3rad/sec$ .

The natural frequency of the circuit:

$f_{natural}=\frac{w_{natural}}{2\pi}=\frac{10^3}{2\pi}=159Hz$

Hence the natural frequency of the circuit is 159Hz.

Q7.12 (c-i) An $LC$ circuit contains a $20mH$ inductor and a $50\mu F$ capacitor with an initial charge of $10mC$ . The resistance of the circuit is negligible. Let the instant the circuit is closed be $t=0$ .

( c) At what time is the energy stored

(i) completely electrical (i.e., stored in the capacitor)?

Answer:

at any instant, the charge on the capacitor is:

$Q=Q_0cos(w_{natural}t)=Q_0cos(2\pi f_{natural}t)=Q_0cos\left ( \frac{2\pi t}{T} \right )$

Where time period :

$T=\frac{1}{f_{natural}}=\frac{1}{159}=6.28ms$

Now, when the total energy is purely electrical, we can say that

$Q=Q_0$

$Q_0=Q_0cos(\frac{2\pi}{T})$

$cos(\frac{2\pi t}{T})=1$

this is possible when

$t=0,\frac{T}{2},T,\frac{3T}{2}....$

Hence Total energy will be purely electrical(stored in a capacitor) at

$t=0,\frac{T}{2},T,\frac{3T}{2}....$ .

Q7.12 (c-ii) An $LC$ circuit contains a $20mH$ inductor and a $50\mu F$ capacitor with an initial charge of $10mC$ . The resistance of the circuit is negligible.
(C) Let the instant the circuit is closed be $t=0$ .

(ii) completely magnetic (i.e., stored in the inductor)?

Answer:

The stored energy will we purely magnetic when the pure electrical stored is zero. i.e. when the charge on the capacitor is zero, all energy will be stored in the inductor.

So, t for which charge on the capacitor is zero is

$t=\frac{T}{4},\frac{3T}{4},\frac{5T}{4}..$

Hence at these times, the total energy will be purely magnetic.

Q7.12 (d) An $LC$ circuit contains a $20mH$ inductor and a $50\mu F$ capacitor with an initial charge of $10mC$ . The resistance of the circuit is negligible. Let the instant the circuit is closed be $t=0$ .

At what times is the total energy shared equally between the inductor and the capacitor?

Answer:

The energy will be shared equally when the energy in the capacitor is half of the maximum energy it can store.i.e.

$\frac{Q^2}{2C}=\frac{1}{2}\frac{Q_0^2}{2C}$

From here, we got

$Q=\frac{Q_0}{\sqrt{2}}$

So now, we know the charge on the capacitor, we can calculate the time for which

$\frac{Q_0}{\sqrt{2}}=Q_0cos\left ( \frac{2\pi t}{T} \right )$

$\frac{1}{\sqrt{2}}=cos\left ( \frac{2\pi t}{T} \right )$

From here,

$t=\frac{T}{8},\frac{3T}{8},\frac{5T}{8}..$

Hence for these times, the total energy will be shared equally between capacitor and inductor.

Q7.12 (e) An $LC$ circuit contains a $20mH$ inductor and a $50\mu F$ capacitor with an initial charge of $10mC$ . The resistance of the circuit is negligible. Let the instant the circuit is closed be $t=0$ .

If a resistor is inserted in the circuit, how much energy is eventually dissipated as heat?

Answer:

If the resistance is added to the circuit, the whole energy will dissipate as heat eventually. energy will keep moving between the capacitor and inductor with reducing in magnitude in each cycle and eventually all energy will be dissipated.

Q 7.13 (a) A coil of inductance $0.50H$ and resistance $100\Omega$ is connected to a $240V$ , $50Hz$ ac supply. What is the maximum current in the coil?

Answer:

Given,

The inductance of the coil $L=0.50H$

the resistance of the coil $R=100\Omega$

Supply voltage $V=240V$

Supply voltage frequency $f=50Hz$

Now, as we know peak voltage = $\sqrt2$ (RMS Voltage)

Peak voltage

$V_{peak}=\sqrt2*240=339.4V$

The impedance of the circuit :

$Z=\sqrt{R^2+(wL)^2}=\sqrt{100^2+(2\pi (.5) *50)^2}$

Now peak current in the circuit :

$I_{peak}=\frac{V_{peak}}{Z}=\frac{339}{\sqrt{100^2+(2\pi (.5) *50)^2}}=1.82A$

Hence peak current is 1.82A in the circuit.

Q 7.13 (b) A coil of inductance $0.50H$ and resistance $100\Omega$ is connected to a $200V$ , $50Hz$ ac supply. What is the time lag between the voltage maximum and the current maximum?

Answer:

Let the voltage in the circuit be

$V = V_0coswt$ and

Current in the circuit be

$I = I_0cos(wt-\phi )$

Where $\phi$ is the phase difference between voltage and current.

V is maximum At

t = 0

$I$ is maximum At

$t=\frac{w}{\phi }$

Hence, the time lag between voltage maximum and the current maximum is

$\frac{w}{\phi }$ .

For phase difference $\phi$ we have

$tan\phi =\frac{wL}{R}=\frac{2\pi *50*0.5}{100}=1.57$

$\phi =57.5^0$

$t=\frac{\phi}{w}=\frac{57.5*\pi}{180*2\pi *50}=3.2ms$

Hence time lag between the maximum voltage and the maximum current is $3.2ms$

Q7.14 Obtain the answers (a) to (b) in Exercise 7.13 if the circuit is connected to a high-frequency supply $(240 V\: ,10 kHz)$ . Hence, explain the statement that at very high frequency, an inductor in a circuit nearly amounts to an open circuit. How does an inductor behave in a dc circuit after the steady state?

Answer:

Given,

The inductance of the coil $L=50H$

the resistance of the coil $R=100\Omega$

Supply voltage $V=240V$

Supply voltage frequency $f=10kHz$

Now, as we know peak voltage = $\sqrt2$ (RMS Voltage)

Peak voltage $V_{peak}=\sqrt2*240=339.4V$

Now,

The impedance of the circuit :

$Z=\sqrt{R^2+(wL)^2}=\sqrt{100^2+(2\pi 10*10^3 *50)^2}$

Now peak current in the circuit :

$I_{peak}=\frac{V_{peak}}{Z}=\frac{339}{\sqrt{100^2+(2\pi 10*10^3 *50)^2}}=1.1*10^{-2}A$

Hence peak current is $1.1*10^{-2}A$ in the circuit.

The current in the circuit is very small, which is one of the indications of inductor working as a nearly open circuit in the case of high frequency.

For phase difference $\phi$ we have

$tan\phi =\frac{wL}{R}=\frac{2\pi *10*10^3*0.5}{100}=100\pi$

$\phi =89.82^0$

Now

$t=\frac{\phi}{w}=\frac{89.82*\pi}{180*2\pi *10^3}=25\mu s$

Hence time lag between the maximum voltage and the maximum current is $25\mu s$ .

In the DC circuit, after attaining the steady state, inductor behaves line short circuit as $w$ is Zero.

Q7.15 (a) A $100\mu F$ capacitor in series with a $40\Omega$ resistance is connected to a $110\: V$ , $60\: Hz$ supply.
What is the maximum current in the circuit?

Answer:

Given,

The capacitance of the capacitor $C=100\mu F$

The resistance of the circuit $R=40\Omega$

Voltage supply $V = 100V$

Frequency of voltage supply $f=60Hz$

The maximum current in the circuit

$I_{max}=\frac{V_{max}}{Z}=\frac{\sqrt{2}V}{\sqrt{R^2+\left ( \frac{1}{wC} \right )^2}}=\frac{\sqrt{2}*110}{\sqrt{40^2+\left ( \frac{1}{2\pi *60*100*10^{-6}} \right )^2}}=3.24A$

Hence maximum current in the circuit is 3.24A.

Q 7.15 (b) A $100\mu F$ capacitor in series with a $40\Omega$ resistance is connected to a $110\; V$ , $60\: Hz$ supply. What is the time lag between the current maximum and the voltage maximum?

Answer:

In the case of a capacitor, we have

$tan\phi=\frac{\frac{1}{wC}}{R}=\frac{1}{wCR}$

So,

$tan\phi=\frac{1}{wCR}=\frac{1}{2\pi 60 *100*10^{-6}*40}=0.6635$

$\phi=33.56^0$

So the time lag between max voltage and the max current is :

$t=\frac{\phi }{w}=\frac{33.56\pi}{180*2\pi*60}=1.55ms$

Q7.16 Obtain the answers to (a) and (b) in Exercise 7.15 if the circuit is connected to a $110\: V$ , $10kHz$ supply? Hence, explain the statement that a capacitor is a conductor at very high frequencies. Compare this behaviour with that of a capacitor in a dc circuit after the steady state.

Answer:

Given,

The capacitance of the capacitor $C=100\mu F$

The resistance of the circuit $R=40\Omega$

Voltage supply $V = 100V$

Frequency of voltage supply $f=12kHz$

The maximum current in the circuit

$I_{max}=\frac{V_{max}}{Z}=\frac{\sqrt{2}V}{\sqrt{R^2+\left ( \frac{1}{wC} \right )^2}}=\frac{\sqrt{2}*110}{\sqrt{40^2+\left ( \frac{1}{2\pi *12*10^3*100*10^{-6}} \right )^2}}=3.9A$

Hence maximum current in the circuit is 3.9A.

In the case of capacitor, we have

$tan\phi=\frac{\frac{1}{wC}}{R}=\frac{1}{wCR}$

So,

$tan\phi=\frac{1}{wCR}=\frac{1}{2\pi 10*10^3 *100*10^{-6}*40}=\frac{1}{96\pi}$

$\phi=0.2^0$

So the time lag between max voltage and max current is :

$t=\frac{\phi }{w}=\frac{0.2\pi}{180*2\pi*60}=0.04\mu s$

At high frequencies, $\phi$ tends to zero. which indicates capacitor acts as a conductor at high frequencies.

In the DC circuit, after a steady state is achieved, Capacitor acts like an open circuit.

Q7.17 Keeping the source frequency equal to the resonating frequency of the series $LCR$ circuit, if the three elements, $L$ , $C$ and $R$ are arranged in parallel, show that the total current in the parallel $LCR$ circuit is minimum at this frequency. Obtain the current rms value in each branch of the circuit for the elements and source specified in Exercise 7.11 for this frequency.

Answer:

As we know, in the case of a parallel RLC circuit:

$\frac{1}{Z}=\sqrt{\frac{1}{R^2}+(wC-\frac{1}{wL})^2}$

$I=\frac{V}{Z}={V}{\sqrt{\frac{1}{R^2}+\left (wC- \frac{1}{wL} \right )^2}}$

The current will be minimum when

$wC=\frac{1}{wL}$

Which is also the condition of natural frequency. Hence the total current is minimum when source frequency is equal to the natural frequency.

RMS value of current in R

$I_{rms}=\frac{V_{rms}}{R}=\frac{230}{40}=5.75A$

RMS value in Inductor

$I_{inductor}=\frac{V_{rms}}{wL}=\frac{230}{5*50}=0.92A$

RMS value in capacitor

$I_{capacitor}=\frac{V_{rms}}{1/wL}={230*50*80*10^{-6}}=0.92A$

Capacitor current and inductor current will cancel out each other so the current flowing in the circuit is 5.75A.

Q7.18 (a) A circuit containing a $80mH$ inductor and a $60\mu F$ capacitor in series is connected to a $230\: V$ , $50\: Hz$ supply. The resistance of the circuit is negligible. Obtain the current amplitude and rms values.

Answer:

The inductance of the inductor $L=80mH=80*10^3H$

The capacitance of the capacitor $C=60\mu F$

Voltage supply $V = 230V$

Frequency of voltage supply $f=50Hz$ .

Here, we have

$V=V_{max}sinwt=V_{max}sin2\pi ft$

Impedance

$Z=\sqrt{R^2+\left ( wL-\frac{1}{wC} \right )^2}$

$Z=\sqrt{0^2+\left ( 2\pi*50*80*10^{-3}-\frac{1}{2\pi 50*60*10^{-6}} \right )^2}=8\pi-\frac{1000}{6\pi }$

Now,

Current in the circuit will be

$I=\frac{V}{Z}=\frac{V_{max}sinwt}{Z\angle \phi }=I_{max}sin(wt-\phi )$

where,

$I_{max}=\frac{V_{max}}{Z}=\frac{\sqrt{2}*230}{8\pi-\frac{1000}{6\pi}}=-11.63A$

The negative sign is just a matter of the direction of current.so,

$I=11.63sin(wt-\phi )$

here

$tan\phi=\frac{wL-\frac{1}{wC}}{R}$

But, since the value of R is zero(since our circuit have only L and C)

$\phi=90^0$

Hence

$I=11.63sin(wt-\frac{\pi}{2} )$

Now,

RMS value of this current:

$I_{rms}=\frac{I_{max}}{\sqrt{2}}=\frac{11.63}{\sqrt{2}}=8.22A$ .

Q7.18 (b) A circuit containing a $80mH$ inductor and a $60\mu F$ capacitor in series is connected to a $230\: V$ , $50\: Hz$ supply. The resistance of the circuit is negligible. Obtain the rms values of potential drops across each element.

Answer:

As we know,

RMS potential drop across an element with impedance Z:

$V_{element}=I_{rms}Z_{element}$

SO,

RMS potential difference across inductor:

$V_{inductor}=I_{rms}*wL=8.22*2\pi *60*80*10^{-3}=206.61V$

RMS potential drop across capacitor

$V_{capacitor}=I_{rms}*\frac{1}{wC}=8.22*\frac{1}{2\pi*60*60*10^{-6}}=436.3V$

Q7.18 (c) A circuit containing a $80mH$ inductor and a $60\mu F$ capacitor in series is connected to a $230\: V$ , $50\: Hz$ supply. The resistance of the circuit is negligible

Answer:

Since

$I=I_{max}sin(wt-\phi )$

Current flowing in the circuit is sinusoidal and hence average power will be zero as the average of sin function is zero.in other words, the inductor will store energy in the positive half cycle of the sin (0 degrees to 180 degrees) and will release that energy in the negative half cycle(180 degrees to 360 degrees), and hence average power is zero.

Q7.18 (d) A circuit containing a $80mH$ inductor and a $60\mu F$ capacitor in series is connected to a $230\: V$ , $50\: Hz$ supply. The resistance of the circuit is negligible . What is the average power transferred to the capacitor?

Answer:

As we know,

Average power $P=VIcos\theta$ where $\theta$ is the phase difference between voltage and current.

Since in the circuit, phase difference $\theta$ is $\pi/2$ , the average power is zero.

Q7.18 (e) A circuit containing a $80mH$ inductor and a $60\mu F$ capacitor in series is connected to a $230\: V$ , $50\: Hz$ supply. The resistance of the circuit is negligible . What is the total average power absorbed by the circuit? [‘Average’ implies ‘averaged over one cycle’.]

Answer:

Since the phase difference between voltage and current is 90 degree, even the total power absorbed by the circuit is zero. This is an ideal circuit, we can not have any circuit in practical that consumes no power, that is because practically resistance of any circuit is never zero. Here only inductor and capacitor are present and none of them consumes energy, they just store it and transfer it like they are doing it in this case.

Q7.19 Suppose the circuit in Exercise 7.18 has a resistance of $15\Omega$ . Obtain the average power transferred to each element of the circuit, and the total power absorbed.

Answer:

The inductance of the inductor $L=80mH=80*10^3H$

The capacitance of the capacitor $C=60\mu F$

The resistance of a $15\Omega$ resistor

Voltage supply $V = 230V$

Frequency of voltage supply $f=50Hz$

As we know,

Impedance

$Z=\sqrt{R^2+\left ( wL-\frac{1}{wC} \right )^2}$

$Z=\sqrt{15^2+\left ( 2\pi*50*80*10^{-3}-\frac{1}{2\pi 50*60*10^{-6}} \right )^2}=31.728$

Current flowing in the circuit :

$I=\frac{V}{Z}=\frac{230}{31.72}=7.25A$

Now,

Average power transferred to the resistor:

$P_{resistor}=I^2R=(7.25)^2*15=788.44W$

Average power transferred to the inductor = 0

Average power transferred to the capacitor = 0:

Total power absorbed by circuit :

$P_{resistor}+p_{inductor}+P_{capacitor}=788.44+0+0=788.44W$

Hence circuit absorbs 788.44W.

Q7.20 (a) A series LCR circuit with $L=0.12H$ , $C=480nF$ , $R=23\Omega$ is connected to a $230V$ variable frequency supply.

What is the source frequency for which current amplitude is maximum. Obtain this maximum value.

Answer:

The inductance of the inductor $L=0.12H$

The capacitance of the capacitor $C=480n F$

The resistance of the resistor $R=23\Omega$

Voltage supply $V = 230V$

Frequency of voltage supply $f=50Hz$

As we know,

the current amplitude is maximum at the natural frequency of oscillation, which is

$w_{natural}=\sqrt\frac{1}{LC}=\frac{1}{\sqrt{0.12*480*10^{-9}})}=4166.67rad/sec$

Also, at this frequency,

$Z=R=23$

SO,

The maximum current in the circuit :

$I_{max}=\frac{V_{max}}{Z}=\frac{V_{max}}{R}=\frac{\sqrt{2*}230}{23}=14.14A$

Hence maximum current is 14.14A.

Q7.20 (b) A series LCR circuit with $L=0.12H$ , $C=480nF$ , $R=23\Omega$ is connected to a $230V$ variable frequency supply.

What is the source frequency for which average power absorbed by the circuit is maximum. Obtain the value of this maximum power.

Answer:

Since the resistor is the only element in the circuit which consumes the power, the maximum absorbed power by circuit will be maximum when power absorbed by the resistor will be maximum. power absorbed by the resistor will be maximum at when current is maximum which is the natural frequency case,

Hence when source frequency will be equal to the natural frequency, the power absorbed will be maximum.

Hence frequency

$f=\frac{w_r}{2\pi}=\frac{4166.67}{2\pi}=663.48Hz$

Maximum Power Absorbed

$P=I^2R=(14.14)^2*23=2299.3W$ .

Q7.20 (c) A series LCR circuit with $L=0.12H$ , $C=480nF$ , $R=23\Omega$ is connected to a $230V$ variable frequency supply . What is the $Q$ factor of the given circuit?

Answer:

The value of maximum angular frequency is calculated in the first part of the question and whose magnitude is 4166.67

Q-factor of any circuit is given by

$Q=\frac{w_rL}{R}=\frac{4166.67*0.12}{23}=21.74$

Hence Q-factor for the circuit is 21.74.

Q7.20 (d) A series LCR circuit with $L=0.12H$ , $C=480nF$ , $R=23\Omega$ is connected to a $230V$ variable frequency supply. For which frequencies of the source is the power transferred to the circuit half the power at resonant frequency? What is the current amplitude at these frequencies?

Answer:

Power $P=I^2R$

Power $P$ will be half when the current $I$ is $1/\sqrt{2}$ times the maximum current.

As,

$I =I_{max}Sin(wt-\phi)$

At half powerpoint :

$\frac{i_{max}}{\sqrt{2}} =I_{max}Sin(wt-\phi)$

$\frac{1}{\sqrt{2}} =Sin(wt-\phi)$

$wt=\phi+\frac{\pi}{4}$

here,

$\phi=tan^{-1}(\frac{wL-\frac{1}{wC}}{R})$

On putting values, we get, two values of $w$ for which

$wt=\phi+\frac{\pi}{4}$

And they are:

$w_1=678.75Hz$

$w_2=648.22Hz$

Also,

The current amplitude at these frequencies

$I_{halfpowerpoint}=\frac{I_{max}}{\sqrt{2}}=\frac{14.14}{1.414}=10A$

Q7.21 Obtain the resonant frequency and $\varrho$ -factor of a series $LCR$ circuit with $L=0.3H$ , $C=27\mu F$ , and $R=7.4\Omega$ . It is desired to improve the sharpness of the resonance of the circuit by reducing its ‘full width at half maximum’ by a factor of 2. Suggest a suitable way.

Answer:

The inductance of the inductor $L=0.3H$

The capacitance of the capacitor $C=27\mu F$

The resistance of the resistor $R=7.4\Omega$

Now,

Resonant frequency

$w_r=\frac{1}{\sqrt{LC}}=\frac{1}{\sqrt{0.3*27*10^{-6}}}=111.11rad/sec$

Q-Factor of the circuit

$Q=\frac{w_rL}{R}=\frac{111.11*0.3}{7.4}=45.0446$

Now, to improve the sharpness of resonance by reducing its full width at half maximum, by a factor of 2 without changing $w_r$ ,

we have to change the resistance of the resistor to half of its value, that is

$R_{new}=\frac{R}{2}=\frac{7.4}{2}=3.7\Omega$

Q7.22 (a) In any ac circuit, is the applied instantaneous voltage equal to the algebraic sum of the instantaneous voltages across the series elements of the circuit? Is the same true for rms voltage?

Answer:

Yes, at any instant the applied voltage will be distributed among all element and the sum of the instantaneous voltage of all elements will be equal to the applied. But this is not the case in RMS because all elements are varying differently and they may not be in the phase.

7.22 (b) Answer the following questions: A capacitor is used in the primary circuit of an induction coil.

Answer:

Yes, we use capacitors in the primary circuit of an induction coil to avoid sparking. when the circuit breaks, a large emf is induced and the capacitor gets charged from this avoiding the case of sparking and short circuit.

Q 7.22 (c) Answer the following questions:

An applied voltage signal consists of a superposition of a $dc$ voltage and an ac voltage of high frequency. The circuit consists of an inductor and a capacitor in series. Show that the dc signal will appear across $C$ and the ac signal across $L$ .

Answer:

For a high frequency, the inductive reactance and capacitive reactance:

$X_L=wL=Large \:value\: And \:X_C = \frac{1}{wC}=Very\:small$

Hence the capacitor does not offer resistance to a higher frequency, so the ac voltage appears across L.

Similarly

For DC, the inductive reactance and capacitive reactance:

$X_L=wL=Very\:small\: And \:X_C = \frac{1}{wC}=Large \:value$

Hence DC signal appears across Capacitor only.

Q 7.22 (d) Answer the following questions:

A choke coil in series with a lamp is connected to a dc line. The lamp is seen to shine brightly. Insertion of an iron core in the choke causes no change in the lamp’s brightness. Predict the corresponding observations if the connection is to an ac line.

Answer:

For a steady state DC, the increasing inductance value by inserting iron core in the choke, have no effect in the brightness of the connected lamp, whereas, for ac when the iron core is inserted, the light of the lamp will shine less brightly because of increase in inductive impedance.

Q7.22 (e) Answer the following questions:

Why is choke coil needed in the use of fluorescent tubes with ac mains? Why can we not use an ordinary resistor instead of the choke coil?

Answer:

We need choke coil in the use of fluorescent tubes with ac mains to reduce the voltage across the tube without wasting much power. If we use simply resistor for this purpose, there will be more power loss, hence we do not prefer it.

Q7.23 A power transmission line feeds input power at $2300 V$ to a stepdown transformer with its primary windings having $40000$ turns. What should be the number of turns in the secondary in order to get output power at $230 V$ ?

Answer:

Given,

Input voltage:

$V_{input}=2300V$

Number of turns in the primary coil

$N_{primary}= 4000$

Output voltage:

$V_{output}=230V$

Now,

Let the number of turns in secondary be

$N=N_{secondary}$

Now as we know, in a transformer,

$\frac{V_{primary}}{V_{secondary}}=\frac{N_{primary}}{N_{secondary}}$

${N_{secondary}} =\frac{V_{secondary}}{V_{primary}}*N_{primary}=\frac{230}{2300}*4000=400$

Hence the number of turns in secondary winding id 400.

Q7.24 At a hydroelectric power plant, the water pressure head is at a height of $300m$ and the water flow available is $100m^{3}s^{-1}$ . If the turbine generator efficiency is $60^{0}/_{0}$ , estimate the electric power availablefrom the plant $(g=9.8ms^{-2})$ .

Answer:

Given,

Height of the water pressure head

$h=300m$

The volume of the water flow per second

$V=100m^3s^{-1}$

Turbine generator efficiency

$\eta =0.6$

Mass of water flowing per second

$M=100*10^3=10^5kg$

The potential energy stored in the fall for 1 second

$P=Mgh=10^5*9.8*300=294*10^6J$

Hence input power

$P_{input}=294*10^6J/s$

Now as we know,

$\eta =\frac{P_{output}}{P_{input}}$

$P_{output}=\eta *P_{input}=0.6*294*10^6=176.4*10^6W$

Hence output power is 176.4 MW.

Q7.25 (a) A small town with a demand of $800 kW$ of electric power at $220V$ is situated $15km$ away from an electric plant generating power at $440V$ . The resistance of the two wire line carrying power is $0.5\Omega$ per km. The town gets power from the line through a $4000-220V$ step-down transformer at a sub-station in the town. Estimate the line power loss in the form of heat.

Answer:

Power required

$P=800kW=800*10^3kW$

The total resistance of the two-wire line

$R=2*15*0.5=15\Omega$

Input Voltage

$V_{input}=4000V$

Output Voltage:

$V_{output}=220V$

RMS Current in the wireline

$I=\frac{P}{V_{input}}=\frac{800*10^3}{4000}=200A$

Now,

Power loss in the line

$P_{loss}=I^2R=200^2*15=600*10^3=600kW$

Hence, power loss in line is 600kW.

7.25 (b) A small town with a demand of $800 kW$ of electric power at $220V$ is situated $15km$ away from an electric plant generating power at $440V$ . The resistance of the two wire line carrying power is $0.5\Omega$ per km. The town gets power from the line through a $4000-220V$ step-down transformer at a sub-station in the town.

How much power must the plant supply, assuming there is negligible power loss due to leakage?

Answer:

Power required

$P=800kW=800*10^3kW$

The total resistance of the two-wire line

$R=2*15*0.5=15\Omega$

Input voltage

$V_{input}=4000V$

Output voltage:

$V_{output}=220V$

RMS current in the wireline

$I=\frac{P}{V_{input}}=\frac{800*10^3}{4000}=200A$

Now,

Total power delivered by plant = line power loss + required electric power = 800 + 600 = 1400kW.

Q7.25 (c) A small town with a demand of $800 kW$ of electric power at $220V$ is situated $15km$ away from an electric plant generating power at $440V$ . The resistance of the two wire line carrying power is $0.5\Omega$ per km. The town gets power from the line through a $4000-220V$ step-down transformer at a sub-station in the town. Characterise the step up transformer at the plant.

Answer:

Power required

$P=800kW=800*10^3kW$

The total resistance of the two-wire line

$R=2*15*0.5=15\Omega$

Input Voltage

$V_{input}=4000V$

Output Voltage:

$V_{output}=220V$

RMS Current in the wireline

$I=\frac{P}{V_{input}}=\frac{800*10^3}{4000}=200A$

Now,

Voltage drop in the power line = $IR=200*15=3000V$

Total voltage transmitted from the plant = 3000+4000=7000

as power is generated at 440V, The rating of the power plant is 440V-7000V.

Q7.26 Do the same exercise as above with the replacement of the earlier transformer by a $40,000-220V$ step-down transformer (Neglect, as before, leakage losses though this may not be a good assumption any longer because of the very high voltage transmission involved). Hence, explain why high voltage transmission is preferred?

Answer:

Power required

$P=800kW=800*10^3kW$

The total resistance of the two-wire line

$R=2*15*0.5=15\Omega$

Input Voltage

$V_{input}=40000V$

Output Voltage:

$V_{output}=220V$

RMS current in the wireline

$I=\frac{P}{V_{input}}=\frac{800*10^3}{40000}=20A$

Now,

a) power loss in the line

$P_{loss}=I^2R=20^2*15=6kW$

Power supplied by plant = 800 kW + 6 kW = 806kW.

Voltage drop in the power line = $IR=20*15=300V$

Total voltage transmitted from the plant = 300+40000=40300

as power is generated at 440V, The rating of the power plant is 440V-40300V.

We prefer high voltage transmission because power loss is a lot lesser than low voltage transmission.

NCERT Solutions for class 12 physics: Chapter-Wise

Here are the exercise-wise solutions of the NCERT Class 12 physics book:

NCERT solutions for class 12 physics chapter 1 Electric Charges and Fields

NCERT solutions for class 12 physics chapter 2 Electrostatic Potential and Capacitance

NCERT solutions for class 12 physics chapter 3 Current Electricity

NCERT solutions for class 12 physics chapter 4 Moving Charges and Magnetism

NCERT solutions for class 12 physics chapter 5 Magnetism and Matter

NCERT solutions for class 12 physics chapter 6 Electromagnetic Induction

NCERT solutions for class 12 physics chapter 7 Alternating Current

NCERT solutions for class 12 physics chapter8 Electromagnetic Waves

NCERT solutions for class 12 physics chapter 9 Ray Optics and Optical Instruments

NCERT solutions for class 12 physics chapter 10 Wave Optics Solutions

NCERT solutions for class 12 physics chapter 11 Dual nature of radiation and matter

NCERT solutions for class 12 physics chapter 12 Atoms

NCERT solutions for class 12 physics chapter 13 Nuclei

NCERT solutions for class 12 physics chapter 14 Semiconductor Electronics Materials Devices and Simple Circuits

Alternating current class 12 solutions: Important Formulas and Diagrams

Important Formulas and Diagrams serve as a crucial resource for exam preparation, be it for board exams or competitive ones like JEE and NEET. These formulas and diagrams condense complex concepts, aiding students in quick revision and problem-solving, ultimately boosting their confidence and performance in exams.

Alternating Current and Voltage

I=I_osinωt Alternating current

V=V_osinωt Alternating Voltage

Where: Io is the peak value of current and Vo is the peak value of voltage

Periodic Time:

$T=\frac{2 \pi}{\omega}$

Frequency(f):

$\mathrm{f}=1 / \mathrm{T}=\omega / 2 \Pi$

Mean Value of An Alternating Current:

$V_{r m s}=\frac{V_0}{\sqrt{2}}=0.707 V_0$

Impedance and Resistance

For L-R series circuit: $Z_{R L}=\sqrt{R^2+X L^2}$

For R-C series circuit: $Z_{R C}=\sqrt{R^2+X C^2}$

Series LRC Circuit

$\begin{aligned} & Z_{R L C}= \sqrt{R^2+\left(X_L-X_C\right)^2} \\ & \sqrt{R^2+\left(\omega L-\frac{1}{\omega C}\right)^2} \end{aligned}$

At resonant frequency,

$\begin{aligned} X_C & =X_L \\ \frac{1}{\omega C} & =\omega L \\ \omega_r & =\frac{1}{\sqrt{L C}} \Rightarrow f_r=\frac{1}{2 \pi \sqrt{L C}} \end{aligned}$

Voltage and power in a transformer

The voltage across secondary coil: $V_s=\left(\frac{N_s}{N_p}\right) V_p$

Input and Output power: $\begin{aligned} & P_{\text {input }}=P_{\text {output }} \\ & V_P I_P=V_S I_S \end{aligned}$

NCERT Class 12 Physics Chapter 7 Alternating Current: Important topics

Class 12 NCERT introduces the concept of different ac circuits including the elements, resistor, capacitor, inductor and ac voltage source. Single-phase ac circuits and their questions are discussed in the NCERT Solutions for Class 12 physics chapter 7. The following are the main headings covered in physics Class 12 chapter 7.

AC voltage applied to a resistor- In this section, the analysis of a resistor circuit applied with an ac voltage of Vmsinωt is done and the terms like RMS and peak voltage and current is introduced. Also, the power dissipated in the resistor circuit is discussed. Question 1 and 2 of chapter 7 Physics Class 12 NCERT solutions given are based on this topic. The main formulas used here are:

$RMSvalue=\frac{peakvalue}{\sqrt{2}}$

The power consumed in a resistor circuit connected with ac source, P= V_rms* I_rms
In the next part of Alternating Current class 12, phasor representation of voltage and current is introduced.
Ch 7 physics Class 12 gives a small analysis of circuits involving R, L and C. Alternating Current Class 12 NCERT pdf solutions discuss questions based on series RL, RC and RLC circuits. Also, chapter 7 Class 12 physics discuss circuit including capacitance and inductance only
The concepts of LC oscillation, resonance and transformer and their basic equations are discussed.

Key Features of Class 12 Physics NCERT Solutions for Chapter 7 Alternating Current

omprehensive Coverage: These ncert class 12 physics chapter 7 exercise solutions encompass all topics and questions presented in Chapter 7, ensuring a thorough understanding of alternating current.
Detailed Explanations: Each ac current class 12 solution offers in-depth explanations, helping students grasp complex concepts.
Clarity and Simplicity: The alternating current class 12 solutions are presented in clear and straightforward language, making it easier for students to comprehend.
Practice Questions: Exercise questions are included for students to practice and assess their understanding.
Exam Preparation: These physics chapter 7 class 12 solutions are instrumental in board exam preparation and provide valuable support for competitive exams like JEE and NEET.
Foundation for Advanced Study: The concepts covered in this chapter are foundational for advanced physics and electrical engineering studies.
Free Accessibility: These alternating current questions and answers pdf are available for free, ensuring accessibility to all students.

These features make Alternating Current Class 12 NCERT solutions a valuable tool for students, facilitating their success in exams and future studies.

Also Check NCERT Books and NCERT Syllabus here:

NCERT solutions subject wise

Significance of NCERT solutions for class 12 physics chapter 7 in board exam

From the unit electromagnetic induction and alternating current Class 12, around 10% of the questions are asked in the CBSE 12th board exam.
If all the Class 12 Physics Chapter 7 NCERT solutions are covered then it is easy to answer questions asked in board exams.
This chapter 7 of NCERT Class 12 physics solutions is also important for JEE Main, NEET, state board exams, and competitive exams.
Prepare the chapter well with the help of CBSE NCERT Class 12 Physics Chapter 7 solutions.

Also, check

Frequently Asked Question (FAQs)

1. Is the supply coming to our home alternating?

Yes, the supply received in our home is alternating. Usually, domestic supplies are single-phase and supply to industries, factories etc are three-phase.

2. What type of questions are asked in board exams from the chapter alternating current?

In the previous year question papers, questions related to identifying the circuit elements are repeatedly asked. Along with this simple numerical questions are also asked from Alternating Current. For practising numerical, solve NCERT exercise questions, NCERT exemplar questions and previous year papers.

3. What is the weightage of chapter for JEE Main?

One question for JEE main can be expected from the Class 12 chapter Alternating Current.

4. How important is the chapter for NEET?

One or two questions may be asked from NCERT chapter Alternating Current for NEET exam.

5. What is the difference between alternating current(AC) and direct current(DC)?

AC and DC are two types of electrical current that differ in the direction of electron flow. AC periodically changes direction while DC flows in only one direction. AC is commonly used for power transmission over long distances, while DC is used for electronic devices that require constant voltage or current. AC generators are simpler and cheaper to build than DC generators.

Also Read

NCERT Solutions for Exercise 4.1 Class 11 Maths Chapter 4 - Principle of Mathematical Induction

NCERT Solutions for Class 12 Biology - Check CBSE 12 Biology NCERT Chapterwise Solution

NCERT Solutions for Class 12 Maths Chapter 11 Three Dimensional Geometry

NCERT Solutions for Class 12

NCERT Solutions for Class 12 Maths (Updated 2023-24)

NCERT Solutions for Miscellaneous Exercise Chapter 7 Class 12 - Integrals

NCERT Syllabus for Class 6 to 12 (2023-24) - All Subjects PDF Download

NCERT Syllabus for Class 12 Maths 2023 - Download PDF

NCERT Syllabus for Class 12 Chemistry 2023 - Download PDF

NCERT Syllabus for Class 12 Biology 2023

NCERT Syllabus for Class 12 Physics 2023 - Download PDF

NCERT Syllabus for Class 12 2023 (All Subjects) - Download PDF Here

NCERT Books for Class 12 - Download All Subjects PDFs Here

NCERT Books for class 12 Chemistry 2023 - Download Pdf Here

NCERT Books for Class 12 Hindi 2023 - Download chapter wise PDFs here

NCERT Book for Class 12 Maths 2023 - Download PDF

NCERT Books for Class 12 Biology 2023 - Download PDF

NCERT Book for Class 12 Physics 2023 - Download PDF

Haloalkanes and haloarenes class 12th notes- free ncert class 12 Chemistry Chapter 10 Notes- Download PDF

Integrals 12th Notes - Free NCERT Class 12 Maths Chapter 7 Notes - Download PDF

Application of Derivatives Class 12th Notes - Free NCERT Class 12 Maths Chapter 6 Notes - Download PDF

NCERT Class 12th Maths Notes Chapter-wise - Download Free PDF

NCERT Class 12th Maths Chapter 5 Continuity and Differentiability Notes

NCERT Class 12th Biology Notes Chapter-wise - Download Free PDF

NCERT Exemplar Class 12 Physics Solutions Chapter 8 Electromagnetic Waves

Articles

Karnataka Board Time Table 2024- Check Karnataka 10th & 2nd PUC Date Sheet 2024

Dec 01, 2023

NCERT Solutions for Miscellaneous Exercise Chapter 5 Class 12 - Continuity and Differentiability

Dec 01, 2023

NCERT Solutions for Miscellaneous Exercise Chapter 4 Class 12 - Determinants

Dec 01, 2023

NCERT Solutions for Miscellaneous Exercise Chapter 3 Class 12 - Matrices

Dec 01, 2023

CBSE Class 12 Date Sheet 2024 - CBSE 12th Exam Date for Science, Commerce and Arts

Dec 01, 2023

12th Time Table 2024 - Check 12th Board Exam Date Here (State-Wise)

Dec 01, 2023

NCERT Solutions for Miscellaneous Exercise Chapter 2 Class 12 - Inverse Trigonometric Functions

Dec 01, 2023

NCERT Solutions for Miscellaneous Exercise Chapter 1 Class 12 - Relations and Functions

Dec 01, 2023

CBSE Class 12 Date Sheet 2024 - CBSE 12th Exam Date for Science, Commerce and Arts

Dec 01, 2023

12th Time Table 2024 - Check 12th Board Exam Date Here (State-Wise)

Dec 01, 2023

Karnataka Board Exam Date 2024 (Out)- Check SSLC and 2nd PUC Time Table PDF

Dec 01, 2023

NCERT Solutions for Exercise 13.4 Class 12 Maths Chapter 13 - Probability

Dec 01, 2023

NCERT Solutions for Exercise 13.5 Class 12 Maths Chapter 13 - Probability

Dec 01, 2023

NCERT Solutions for Miscellaneous Exercise Chapter 1 Class 12 - Relations and Functions

Dec 01, 2023

NCERT Solutions for Miscellaneous Exercise Chapter 3 Class 12 - Matrices

Dec 01, 2023

5 min ⋆ Dec 01, 2023

Study Help

What Is The Physics Behind Different Firecrackers?

5 min ⋆ Nov 30, 2023

Upcoming School Exams

All India Sainik Schools Entrance Examination

Application Date:06 November,2023 - 15 December,2023

Exam Pattern

Answer Key

Result

National Institute of Open Schooling 10th examination

Application Date:20 November,2023 - 19 December,2023

Application Process

Exam Pattern

Mock Test

National Institute of Open Schooling 12th Examination

Application Date:20 November,2023 - 19 December,2023

Application Process

Exam Pattern

Admit Card

National Means Cum-Merit Scholarship

Admit Card Date:22 November,2023 - 02 December,2023

Application Process

Exam Pattern

Mock Test

West Bengal Board 12th Examination

Exam Date:30 November,2023 - 14 December,2023

Result

Exam Pattern

Admit Card

View All School Exams

Certifications By Top Providers

Artificial Intelligence Projects

Via Great Learning

Full-Stack Web Development

Via Masai School

Master Data Management for Beginners

Via TCS iON Digital Learning Hub

Digital Banking Business Model

Via State Bank of India

Introduction to Web Development with HTML CSS JavaScript

Via IBM

Google Cloud IAM and Networking for AWS Professionals

Via Google

1110 courses

783 courses

328 courses

136 courses

Harvard University, Cambridge

98 courses

IBM

86 courses

IT & Software

Teaching and Academics

Programming and Development

Health, Fitness and Medicine

Business and Management

Engineering and Architecture

General Management

Financial Management

History

Environmental Studies

Cloud Computing

Law

Explore Top Universities Across Globe

University of Essex, Colchester

Wivenhoe Park Colchester CO4 3SQ

University College London, London

Gower Street, London, WC1E 6BT

The University of Edinburgh, Edinburgh

Old College, South Bridge, Edinburgh, Post Code EH8 9YL

University of Nottingham, Nottingham

University Park, Nottingham NG7 2RD

Lancaster University, Lancaster

Bailrigg, Lancaster LA1 4YW

Bristol Baptist College, Bristol

The Promenade, Clifton Down, Bristol BS8 3NJ

How to Apply for PhD After Bachelors?

7 min ⋆ Nov 06, 2023 19:11 PM IST

USMLE 2023 Step 1 - Dates, Application Form, Exam Pattern, Score

10 min ⋆ Nov 06, 2023 19:11 PM IST

Top MBA Colleges in Dubai (with Tuition fees) –List of Best Colleges

5 min ⋆ Nov 06, 2023 13:11 PM IST

IELTS Result 2023: Dates, How to Check Score, Download Scorecard

11 min ⋆ Oct 25, 2023 05:10 AM IST

Permanent residency in Australia after studies - Procedure,How to improve chances to apply

6 min ⋆ Oct 23, 2023 06:10 AM IST

Letter of Recommendation (LOR) for PhD Students (with Sample) - Need, Parts, Qualities

9 min ⋆ Oct 23, 2023 06:10 AM IST

Related E-books & Sample Papers

UP Board 11th English Syllabus 2024

26+ Downloads

CBSE Class 12th Psychology Question Paper 2023

202+ Downloads

CBSE Class 12th Entrepreneurship Question Paper 2023

29+ Downloads

CBSE Class 12th Sociology Question Paper 2023

43+ Downloads

CBSE Class 12th Geography Question Paper 2023

35+ Downloads

CBSE Class 12th Accountancy Question Paper 2023

81+ Downloads

Questions related to CBSE Class 12th

Have a question related to CBSE Class 12th ?

i couldn't clear 1st compartment (which held in August 2022) cbse class 12th so can I give improvement in all subjects which to be held on March 2023. tell me a solution i need more than 75% in class 12th

hello,

Yes you can appear for the compartment paper again since CBSE gives three chances to a candidate to clear his/her exams so you still have two more attempts. However, you can appear for your improvement paper for all subjects but you cannot appear for the ones in which you have failed.

I hope this was helpful!

Good Luck

Read Complete Answer

Upasana Barman 24 August,2022

i was not able to clear 1st compartment and now I have to give 2nd compartment so can I give improvement exam next year? plz tell me very much tensed(cbse class 12th)

Hello dear,

If you was not able to clear 1st compartment and now you giving second compartment so YES, you can go for your improvement exam next year but if a student receives an improvement, they are given the opportunity to retake the boards as a private candidate the following year, but there are some requirements. First, the student must pass all of their subjects; if they received a compartment in any subject, they must then pass the compartment exam before being eligible for the improvement.

As you can registered yourself as private candidate for giving your improvement exam of 12 standard CBSE(Central Board of Secondary Education).For that you have to wait for a whole year which is bit difficult for you.

Positive side of waiting for whole year is you have a whole year to preparing yourself for your examination. You have no distraction or something which may causes your failure in the exams. In whole year you have to stay focused on your 12 standard examination for doing well in it. By this you get a highest marks as a comparison of others.

Believe in Yourself! You can make anything happen

All the very best.

Read Complete Answer

Mayankjha.student2007 23 August,2022

if i am giving improvement in one subject this year, will i be able to give improvement in more subjects next year?

Hello Student,

I appreciate your Interest in education. See the improvement is not restricted to one subject or multiple subjects and we cannot say if improvement in one subject in one year leads to improvement in more subjects in coming year.

You just need to have a revision of all subjects what you have completed in the school. have a revision and practice of subjects and concepts helps you better.

All the best.

Read Complete Answer

Nuthalapati Vyshnavi 21 August,2022

I will have my boards in Feb 2023 (Class 12) and I still haven't completed 1 chapter in any subject (Physics, chemistry and maths) , My 11th concepts are not that great but its okish, I wanted to know that If I do start studying from 15th of August Can I get above 85?

If you'll do hard work then by hard work of 6 months you can achieve your goal but you have to start studying for it dont waste your time its a very important year so please dont waste it otherwise you'll regret.

Read Complete Answer

khushi.thakurbbk 07 September,2022

I want to take a admission in class 12 privately.. so can I???

Yes, you can take admission in class 12th privately there are many colleges in which you can give 12th privately.

Read Complete Answer

Parvati Solanki 02 June,2022

View All

Popular CBSE Class 12th Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms^-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times. Assume that the potential energy lost each time he lowers the mass is dissipated. How much fat will he use up considering the work done only when the weight is lifted up ? Fat supplies 3.8×10⁷J of energy per kg which is converted to mechanical energy with a 20% efficiency rate. Take g = 9.8 ms⁻² :

Option 1)

2.45×10⁻³ kg

Option 2)

6.45×10⁻³ kg

Option 3)

9.89×10⁻³ kg

Option 4)

12.89×10⁻³ kg

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

A particle is projected at 60⁰ to the horizontal with a kinetic energy $K$ . The kinetic energy at the highest point

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

In the reaction,

$2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

How many moles of magnesium phosphate, $Mg_{3}(PO_{4})_{2}$ will contain 0.25 mole of oxygen atoms?

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

With increase of temperature, which of these changes?

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol^-1) is

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t²) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m² , the number of rotations made by the pulley before its direction of motion if reversed, is

Option 1) less than 3	Option 2) more than 3 but less than 6
Option 3) more than 6 but less than 9	Option 4) more than 9

3 Jobs Available

Bank Probationary Officer (PO)

A career as Bank Probationary Officer (PO) is seen as a promising career opportunity and a white-collar career. Each year aspirants take the Bank PO exam. This career provides plenty of career development and opportunities for a successful banking future. If you have more questions about a career as Bank Probationary Officer (PO), what is probationary officer or how to become a Bank Probationary Officer (PO) then you can read the article and clear all your doubts.

3 Jobs Available

Operations Manager

3 Jobs Available

When it comes to an operation theatre, there are several tasks that are to be carried out before as well as after the operation or surgery has taken place. Such tasks are not possible without surgical tech and surgical tech tools. A single surgeon cannot do it all alone. It’s like for a footballer he needs his team’s support to score a goal the same goes for a surgeon. It is here, when a surgical technologist comes into the picture. It is the job of a surgical technologist to prepare the operation theatre with all the required equipment before the surgery. Not only that, once an operation is done it is the job of the surgical technologist to clean all the equipment. One has to fulfil the minimum requirements of surgical tech qualifications.

Radio Jockey

Radio Jockey is an exciting, promising career and a great challenge for music lovers. If you are really interested in a career as radio jockey, then it is very important for an RJ to have an automatic, fun, and friendly personality. If you want to get a job done in this field, a strong command of the language and a good voice are always good things. Apart from this, in order to be a good radio jockey, you will also listen to good radio jockeys so that you can understand their style and later make your own by practicing.

A career as radio jockey has a lot to offer to deserving candidates. If you want to know more about a career as radio jockey, and how to become a radio jockey then continue reading the article.

3 Jobs Available

Producer

An individual who is pursuing a career as a producer is responsible for managing the business aspects of production. They are involved in each aspect of production from its inception to deception. Famous movie producers review the script, recommend changes and visualise the story.

Are you searching for a Corporate Executive job description? A Corporate Executive role comes with administrative duties. He or she provides support to the leadership of the organisation. A Corporate Executive fulfils the business purpose and ensures its financial stability. In this article, we are going to discuss how to become corporate executive.

UX Architect

A UX Architect is someone who influences the design processes and its outcomes. He or she possesses a solid understanding of user research, information architecture, interaction design and content strategy.

2 Jobs Available