Integrals 12th Notes - Free NCERT Class 12 Maths Chapter 7 Notes - Download PDF

Have you ever thought about how we calculate the area under a curve? Total accumulation of any quantity over a period of time? Or the total distance travelled by a moving object with different speeds? Welcome to the world of Integrals, one of the most important concepts in calculus. From NCERT Class 12 Maths, the chapter Integrals contains the concepts of Integration as an Inverse Process of Differentiation, Indefinite Integrals, Methods of Integration, Definite Integrals, Fundamental Theorems of Calculus, etc. These concepts will help the students grasp more advanced calculus topics easily and will also enhance their problem-solving ability in real-world applications.

This article on NCERT notes Class 12 Maths Chapter 7 Integrals offers well-structured NCERT notes to help the students grasp the concepts of integration easily. Students who want to revise the key topics of Integrals quickly will find this article very useful. It will also boost the exam preparation of the students by many folds. These notes of NCERT Class 12 Maths Chapter 7 Integrals are made by the Subject Matter Experts according to the latest CBSE syllabus, ensuring that students can grasp the basic concepts effectively. NCERT solutions for class 12 maths and NCERT solutions for other subjects and classes can be downloaded from the NCERT Solutions.

Integrals Notes:

Adding or summing up the small parts to find the whole sum of the function is the basic meaning of integral calculus.

Indefinite integrals and definite integrals are the two types of integrals that are found in integral calculus.

Indefinite Integral

Thus, the formula that gives this anti-derivative is called the indefinite integral.

Integration as an Inverse Process of Differentiation

Integration is defined as the inverse of differentiation. Here we are given a function and asked to find its primitive (the original function). Such a process is called an anti-derivative or indefinite integral.

Let us see an example:-

We know that

$\frac{d}{dx}(\sin x+c)=\cos x$ or, $\int \cos xdx=\sin x+c$

Here, the actual function is “sinx + c”. Using the indefinite integral, we found the actual equation from the derived one.

Thus, we came to know that the integration of some functions is written as

$\int f(x)dx=F(x)+c$

Here, f(x) is “integrand”, f(x)dx is “element of integration”, $x$ is “variable of integration” and “c” is the integration constant.

Some of the formulas are listed below, which will help us to solve the problems.

$\begin{array}{rl}& \int x^{n}dx=\frac{x^{n+1}}{n+1}+c,(n\ne -1)\\ & \int dx=x+c\\ & \int \cos xdx=\sin x+c\\ & \int \sin xdx=-\cos x+c\\ & \int {\sec }^{2}xdx=\tan x+c\\ & \int {\mathrm{cosec}}^{2}xdx=-\cot x+c\\ & \int \sec x\tan x=\sec x+c\\ & \int \mathrm{cosec}x\cot x=-\mathrm{cosec}x+c\\ & \int \frac{dx}{\sqrt{1-x^2}}={\sin }^{-1}x+c\\ & \int \frac{dx}{\sqrt{1-x^2}}=-{\cos }^{-1}x+c\\ & \int \frac{dx}{1+x^2}={\tan }^{-1}x+c\\ & \int \frac{dx}{1+x^2}=-{\cot }^{-1}x+c\\ & \int \frac{dx}{x\sqrt{x^2-1}}={\sec }^{-1}x+c\\ & \int \frac{dx}{x\sqrt{x^2-1}}=-{\mathrm{cosec}}^{-1}+c\\ & \int e^{x}dx=e^x+c\\ & \int \frac{1}{x}dx=\log |x|+c\\ & \int a^{x}dx=\frac{a^x}{\log a}+c\end{array}$

Geometrical Interpretation of Indefinite Integral

Let us assume that y=f(x) be a curve such that $f^{\prime }(x)=2x\Rightarrow f(x)=x^2+c$

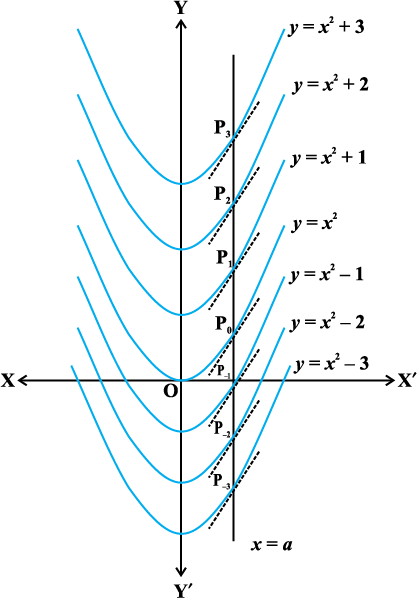
Now, if we give values like 4, 3, 2, 1, 0, -1, -2, -3 to the arbitrary constant c, then the equation will give

$y=x^2+4,x^2+3,x^2+2,x^2+1,x^2,x^2-1,x^2-2,x^2-3$ṣ

having loci as a parabola.

Thus, the equation $\int f(x)dx=F(x)+c=y$ represents a family of curves.

Some properties of the indefinite integral

Here we shall derive some properties of indefinite integrals.

(I) The process of integration and differentiation is the inverse of each other in the sense of the following results:

$\frac{d}{dx}\int f(x)dx=f(x)$ and $\int f^{\prime }(x)dx=f(x)+c$

where c is the arbitrary constant.

(II) Two indefinite integrals with the same derivative lead to the same family of curves; thus, they are equivalent.

$\frac{d}{dx}\int f(x)dx=\frac{d}{dx}\int g(x)dx$

(III) $\int [f(x)+g(x)]dx=\int f(x)dx+\int g(x)dx$
$(IV)$ For any real number $\mathrm{k},\int kf(x)dx=k\int f(x)dx$

(V) Using properties (III) and (IV) can be generalised to a finite number of functions f1, f2, f3, …, fn and the real numbers k1, k2, k3, …, kn give

$\begin{array}{rl}& \int [k_1{f}_1(x)+k_2{f}_2(x)+\dots k_n{f}_n(x)]dx\\ =& \int k_1{f}_1(x)dx+\int k_2{f}_2(x)dx+\dots \int k_n{f}_n(x)dx\end{array}$

Example

Find the anti-derivative (integral) of $\frac{1}{x},x\ne 0$
Solution:
We know that $\frac{d}{dx}(\log x)=\frac{1}{x},x>0$ and $\frac{d}{dx}(\log (-x))=\frac{1}{-x}(-1)=\frac{1}{x},x<0$ Thus combining the above $\frac{d}{dx}\log x=\frac{1}{x},x\ne 0$ Therefore $\frac{1}{x}dx=\log |x|+c$

Methods of Integration

We have many ways of solving integration. Mostly, we solve by using

1. Integration by substitution

2. Integration by Partial Fraction

3. Integration by Parts

Integration by Substitution

The given integral $\int f(x)dx$ can be transferred into another form by changing the independent variable x to t by substituting $\mathrm{x}=\mathrm{g}(\mathrm{t})$.

Let us assume that

$I=\int f(x)dx$

Substitute

x=g(t), so that dx/dt=g'(t)

Then we write dx=g’(t) dt

Thus $I=\int f(x)dx=\int f(g(t))g^{\prime }(t)dt$

Example: Integrate w.r.t x, sin mx

Solution:

We know that by differentiating mx, we get m. Thus, we can substitute mx = t so that mdx =dt

Thus $\int \sin (mx)dx=\int \frac{1}{m}\sin (t)dt=-\frac{1}{m}\cos t+c=-\frac{1}{m}\cos mx+c$

Integration by Trigonometric Identities

The identities are used to find the integral when integration involves some trigonometric functions.

Example: Find $\int {\cos }^{2}xdx$
Solution: We know that

${\cos }^{2}x=\frac{1+\cos 2x}{2}$

Using this, we get that

${\cos }^{2}xdx=\frac{1}{2}(1+\cos 2x)dx=\frac{1}{2}[dx+\cos 2xdx]=\frac{x}{2}+\frac{1}{4}\sin 2x+c$

Integrals of Some Particular Function

Some of the formulas are listed below

$\begin{array}{rl}& \int \frac{dx}{x^2-a^2}dx=\frac{1}{2a}\log \frac{|x-a|}{|x+a|}+c\\ & \int \frac{dx}{a^2-x^2}=\frac{1}{2a}\log \frac{|a+x|}{|a-x|}+c\\ & \int \frac{dx}{x^2+a^2}=\frac{1}{a}{\tan }^{-1}\frac{x}{a}+c\\ & \int \frac{dx}{\sqrt{x^2-a^2}}=\log |x+\sqrt{x^2-a^2}|+c\\ & \int \frac{dx}{\sqrt{x^2+a^2}}=\log |x+\sqrt{x^2+a^2}|+c\\ & \int \frac{dx}{\sqrt{a^2-x^2}}={\sin }^{-1}\frac{x}{a}+c\end{array}$

Let us see an example

$\begin{array}{rl}& \int \frac{dx}{(4+9x^2)}dx\\ & =\int \frac{dx}{9(\frac{4}{9}+x2)}\end{array}$

Now using a special formula

$\begin{array}{rl}& \int \frac{dx}{x^2+a^2}=\frac{1}{a}{\tan }^{-1}\frac{x}{a}+c\\ & =\frac{1}{9}\int \frac{dx}{x^2+{\left(\frac{2}{3}\right)}^2}\\ & =\frac{1}{9}\frac{1}{2/3}{\tan }^{-1}\frac{3x}{2}+c\\ & =\frac{1}{6}{\tan }^{-1}\frac{3x}{2}+c\end{array}$

Integration by Partial Fraction

The integration of a rational function can be solved by using partial fractions.

$\begin{array}{|lll|}\hline \text{ S.No. }& \text{ Form of a rational function }& \text{ Formation of a partial function }\\ 1& \frac{px+q}{(x-a)(x-b)},a\ne b& \frac{A}{x-a}+\frac{B}{x-b}\\ 2& \frac{px+q}{(x-a{)}^2}& \frac{A}{x-a}+\frac{B}{(x-a{)}^2}\\ 3& \frac{p{x}^2+qx+r}{(x-a)(x-b)(x-c)}& \frac{A}{x-a}+\frac{B}{x-b}+\frac{C}{x-c}\\ 4& \frac{p{x}^2+qx+r}{(x-a{)}^2(x-b)}& \frac{A}{x-a}+\frac{B}{(x-a{)}^2}+\frac{C}{x-b}\\ 5& \frac{p{x}^2+qx+r}{(x-a)(x^2+bx+c)}& \frac{A}{x-a}+\frac{Bx+c}{x^2+bx+c}\\ \hline\end{array}$
Let us see the following example
$\begin{array}{rl}& \int \frac{x^2}{x^2+x+6}dx\\ & =\int (1-\frac{x-6}{x^2+x+6}dx\\ & =\int dx-\int \frac{x-6}{x2+x+6}dx\\ & =x-\frac{9}{5}\int \frac{dx}{x+3}+\frac{4}{5}\int \frac{dx}{x-2}\\ & =x-\frac{9}{5}\ln |x+3|+\frac{4}{5}\ln |x-2|+c\end{array}$

Integration by Parts

ILATE rule needs to be followed while doing integration by parts. According to the ILATE rule, we can determine which function will be the 1st function and which will be the 2nd function.

ILATE

I → Inverse function

L → Logarithmic function

A → Algebraic function

T → Trigonometric function

E → Exponential function

From the above rule, as an example, we can say that there are two functions, like trigonometric functions and logarithmic functions. The logarithmic function will be treated as the first function, and the trigonometric function will be treated as the second function.

The general expression follows as

$\int f(x)g(x)dx=f(x)\int g(x)dx-\int [\{\int g(x)dx\}\frac{d}{dx}f(x)]dx$

“The integral of the product of two functions = (1st function) × (integral of the 2nd function) – Integral of [(differential coefficient of the 1st function) × (integral of the 2nd function)]”

Example: Solve $\int \ln xdx$
Solution:
$\begin{array}{rl}& \int \ln x\cdot 1dx\\ & \Rightarrow \ln x\int 1dx-\int [(\int 1dx)\frac{d}{dx}(\ln x)]dx\\ & \Rightarrow x\ln x-\int x\cdot \frac{1}{x}dx\\ & \Rightarrow x\ln x-x+c\end{array}$

Integration of special types of functions
The formulas are listed below

$\begin{array}{rl}& \int \sqrt{x^2+a^2}dx=\frac{1}{2}x\sqrt{x^2+a^2}+\frac{a^2}{2}\log |x+\sqrt{x^2+a^2}|+c\\ & \int \sqrt{x^2-a^2}dx=\frac{1}{2}x\sqrt{x^2-a^2}+\frac{a^2}{2}{\sin }^{-1}\frac{x}{a}+c\end{array}$

Definite Integral

In the indefinite, we saw that the result of the integration was not a unique value. But here indefinite integral we will find a unique value and it is well defined within a limited boundary. Generally, the definite integral is denoted by

${\int }_a^{b}f(x)dx$

Her “a” is the lower limit, and “b” is the upper limit of the integral. The value of the definite integral is computed by F(b) - F(a).

Basically, in Definite integral consists of two cases as discussed below:

Definite Integral as a Limit of the Sum

If the definite integral ${\int }_a^{b}f(x)dx$

is the area bounded by the curve y = f(x), the ordinates x = a, x = b and the x-axis. To evaluate this area, consider the region PRSQP between this curve, the x-axis, and the ordinates x = a and x =b

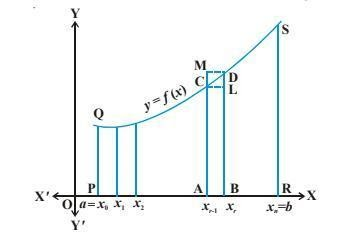
From the figure, we find the general equation of the definite integral as a limit of a sum

$\begin{array}{rl}& \begin{array}{rl}{\int }_a^{b}f(x)dx& =h[f(a)+f(a+h)+\dots +f(a+(n-1)h]\\ \Rightarrow {\int }_a^{b}f(x)dx& =\frac{(b-a)}{n}[f(a)+f(a+h)+\dots +f(a+(n-1)h]\end{array}\\ & \text{ Where }h=\frac{b-a}{n}\to 0\text{ as }n\to \mathrm{\infty }\end{array}$

Some Properties of the Definite Integral

$P_0:{\int }_a^{b}f(x)dx={\int }_a^{b}f(t)dt$
$P_1:{\int }_a^{b}f(x)dx=-{\int }_b^{a}f(x)dx;$ in particular, ${\int }_a^{a}f(x)dx=0$
$P_2:{\int }_a^{b}f(x)dx={\int }_a^{c}f(x)dx+{\int }_c^{b}f(x)dx$
$P_3:{\int }_a^{c}f(x)dx={\int }_a^{c}f(a+b-x)dx$
$P_4:{\int }_0^{b}f(x)dx={\int }_0^{b}f(b-x)dx$
$P_5:{\int }_0^{2b}f(x)dx={\int }_0^{b}f(x)dx+{\int }_0^{b}f(2b-x)dx$
$P_6:{\int }_0^{2b}f(x)dx=2{\int }_0^{b}f(x)dx$ if $f(2b-x)=f(x)$
and ${\int }_0^{2b}f(x)dx=0$ if $f(2b-x)=-f(x)$
$P_7:(i){\int }_{-a}^{a}f(x)dx=2{\int }_0^{a}f(x)dx$, if f is even function, i.e., if $\mathrm{f}(-\mathrm{x})=\mathrm{f}(\mathrm{x})$
(ii) ${\int }_{-a}^{a}f(x)dx=0$, if f is odd function, i.e., $\mathrm{f}(-\mathrm{x})=-\mathrm{f}(\mathrm{x})$

Let us see an example

$\begin{array}{rl}& {\int }_0^{\frac{\pi }{2}}\frac{\sqrt{\sin x}}{\sqrt{\sin x}+\sqrt{\cos x}}dx\\ & \text{ Let }{\int }_0^{\frac{\pi }{2}}\frac{\sqrt{\sin x}}{\sqrt{\sin x}+\sqrt{\cos x}}dx=I-\cdots \cdots (1)\\ & I={\int }_0^{\frac{\pi }{2}}\frac{\sqrt{\sin (\frac{\pi }{2}-x)}}{\sqrt{\sin (\frac{\pi }{2}-x)}+\sqrt{\cos (\frac{\pi }{2}-x)}}dx\\ & I={\int }_0^{\frac{\pi }{2}}\frac{\sqrt{\cos x}}{\sqrt{\sin x}+\sqrt{\cos x}}dx\cdots \cdots \cdots (2)\end{array}$
Now $(1)+(2)$
$\begin{array}{rl}& {\int }_0^{\frac{\pi }{2}}\frac{\sqrt{\sin x}}{\sqrt{\sin x}+\sqrt{\cos x}}dx+{\int }_0^{\frac{\pi }{2}}\frac{\sqrt{\cos x}}{\sqrt{\sin x}+\sqrt{\cos x}}dx=2I\\ & 2I={\int }_0^{\frac{\pi }{2}}dx\\ & I=\frac{\pi }{4}\end{array}$

This brings us to the end of the chapter.

NCERT Class 12 Notes Chapter Wise.

Subject-Wise NCERT Exemplar Solutions

After finishing the textbook exercises, students can use the following links to check the NCERT exemplar solutions for a better understanding of the concepts.

• NCERT Exemplar Class 12 Solutions
• NCERT Exemplar Class 12 Maths
• NCERT Exemplar Class 12 Physics
• NCERT Exemplar Class 12 Chemistry
• NCERT Exemplar Class 12 Biology

Subject-Wise NCERT Solutions

Students can also check these well-structured, subject-wise solutions.

• NCERT Solutions for Class 12 Mathematics
• NCERT Solutions for Class 12 Chemistry
• NCERT Solutions for Class 12 Physics
• NCERT Solutions for Class 12 Biology

NCERT Books and Syllabus

Students should always analyze the latest CBSE syllabus before making a study routine. The following links will help them check the syllabus. Also, here is access to more reference books.

• NCERT Book for Class 12
• NCERT Syllabus for Class 12

Important points to note:

• NCERT problems are very important in order to perform well in the exams. Students must try to solve all the NCERT problems, including miscellaneous exercises, and if needed, refer to the NCERT solutions for class 12 maths chapter 7, Integrals.
• Students are advised to go through the NCERT Class 12 Maths Chapter 3 Notes before solving the questions.
• To boost your exam preparation as well as for a quick revision, these NCERT notes are very useful.

Happy learning !!!