NCERT Solutions for Class 12 Physics Chapter 2 Electrostatic Potential and Capacitance

Access premium articles, webinars, resources to make the best decisions for career, course, exams, scholarships, study abroad and much more with

Plan, Prepare & Make the Best Career Choices

NCERT Solutions for Class 12 Physics Chapter 2 Electrostatic Potential and Capacitance

Edited By Vishal kumar | Updated on Sep 07, 2023 02:07 PM IST | #CBSE Class 12th

NCERT Solutions for Class 12 Physics Chapter 2 –Download Free PDF

NCERT Solutions for Class 12 Physics Chapter 2 Electrostatic Potential And Capacitance: If you're a Class 12 student seeking NCERT solutions, you've come to the right place. On this Careers360 page, you can access complete electrostatic potential and capacitance NCERT solutions covering questions from 2.1 to 2.36, including exercise questions from 2.1 to 2.11 and additional exercise questions from 2.12 to 2.36. These electric potential and capacitance NCERT solutions, prepared by subject matter experts, are presented in an easy-to-understand language with step-by-step explanations, making them invaluable for students to tackle chapter questions, homework, and assignments effectively.

Latest: JEE Main high scoring chapters | JEE Main 10 year's papers

New: Aakash iACST Scholarship Test. Up to 90% Scholarship. Register Now

This Story also Contains

NCERT Solutions for Class 12 Physics Chapter 2 –Download Free PDF
NCERT Solutions for Class 12 Physics Chapter 2 Electrostatic Potential and Capacitance
NCERT Solutions for Class 12 Physics Chapter 2 Electrostatic Potential and Capacitance: Exercises Solution
NCERT solutions for class 12 physics chapter 2 electrostatic potential and capacitance additional exercises:

NCERT Solutions for Class 12 Physics Chapter 2 Electrostatic Potential and Capacitance

NCERT 12 Physics Chapter 2 solutions talks about potential due to different systems of charges and about capacitance and dielectrics. The important derivations of the NCERT solutions for Class 12 Physics Chapter 2 are potential and potential energy due to a dipole. The chapter 2 physics class 12 ncert solutions along with the theories discussed in electric potential and capacitance help students to perform well in exams. Most of the engineering and medical entrance toppers advise considering NCERT as the base for preparation.

Apply to Aakash iACST Scholarship Test 2024

Applications for Admissions are open.

Apply Now

NCERT Solutions for Class 12 is important for CBSE board exam preparations. The NCERT solutions are helpful to understand the basic concepts. Read further to know the NCERT Class 12 Physics chapter 2 solutions and other important details.

Free download cbse class 12 physics chapter 2 exercise solutions PDF for CBSE exam.

NCERT Solutions for Class 12 Physics Chapter 2 Electrostatic Potential and Capacitance

Download PDF

NCERT Solutions for Class 12 Physics Chapter 2 Electrostatic Potential and Capacitance: Exercises Solution

2.1 Two charges $5 \times 10^{-8}C$ and $-3 \times 10^{-8}C$ are located 16 cm apart. At what point(s) on the line joining the two charges is the electric potential zero? Take the potential at infinity to be zero.

Answer:

Given, two charge particles

$q_1= 5*10^{-8}C$

$q_2= -3*10^{-8}C$

The separation between two charged particle $d=16cm=0.16m$

Now, let's assume the point P between two charged particles where the electric potential is zero is x meter away from $q_1$ and $( 9-x)$ meter away from $q_2$

So,

The potential at point P :

$V_p=\frac{kq_1}{x}+\frac{kq_2}{0.16-x}=0$

$V_p=\frac{k5*10^{-8}}{x}+\frac{k(-3*10^{-8})}{0.16-x}=0$

$\frac{k5*10^{-8}}{x}=-\frac{k(-3*10^{-8})}{0.16-x}$

$5(0.16-x)=3x$

$x=0.1m=10cm$

Hence the point between two charged particles where the electric potential is zero lies 10cm away from $q_1$ and 6 cm away from $q_2$

Now, Let's assume a point Q which is outside the line segment joining two charges and having zero electric potential .let the point Q lie r meter away from $q_2$ and (0.16+r) meter away from $q_1$

So electric potential at point Q = 0

$\frac{kq_1}{0.16+r}+\frac{kq_2}{r}=0$

$\frac{k5*10^{-8}}{0.16+r}+\frac{k(-3*10^{-8})}{r}=0$

$5r=3(0.16+r)$

$r=0.24m=24cm$

Hence the second point where the electric potential is zero is 24cm away from $q_2$ and 40cm away from $q_1$

2.2 A regular hexagon of side 10 cm has a charge $5\mu C$ at each of its vertices. Calculate the potential at the centre of the hexagon.

Answer:

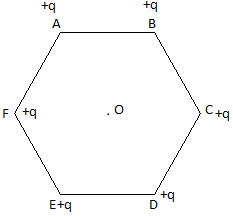
1643085531033

The electric potential at O due to one charge,

$V_1 = \frac{q}{4\pi\epsilon_0 r}$

q = 5 × 10 ^-6 C

r = distance between charge and O = 10 cm = 0.1 m

Using the superposition principle, each charge at corners contribute in the same direction to the total electric potential at point O.

$V = 6\times\frac{q}{4\pi\epsilon_0 r}$

$\implies V = 6\times\frac{9\times10^9 Nm^2C^{-2}\times5\times10^{-6}C}{0.1m}$

= $2.7 \times 10^6 V$

Therefore the required potential at the centre is $2.7 \times 10^6 V$

2.3 (a) Two charges $2\mu C$ and $-2\mu C$ are placed at points A and B 6 cm apart. (a) Identify an equipotential surface of the system.

Answer:

Given, 2 charges with charges $2\mu C$ and $-2\mu C$ .

An equipotential plane is a plane where the electric potential is the same at every point on the plane. Here if we see the plane which is perpendicular to line AB and passes through the midpoint of the line segment joining A and B, we see that at every point the electric potential is zero because the distance of all the points from two charged particles is same. Since the magnitude of charges is the same they cancel out the electric potential by them.

Hence required plane is plane perpendicular to line AB and passing through the midpoint of AB which is 3cm away from both charges.

2.3 (b) Two charges $2\mu C$ and $-2\mu C$ are placed at points A and B 6 cm apart. (b) What is the direction of the electric field at every point on this surface?

Answer:

The direction of the electric field in this surface is normal to the plane and in the direction of line joining A and B. Since both charges have the same magnitude and different sign, they cancel out the component of the electric field which is parallel to the surface.

2.4 (a) A spherical conductor of radius 12 cm has a charge of $1.6 \times 10^{-7}C$ distributed uniformly on its surface. What is the electric field (a) inside the sphere

Answer:

Since the charge is uniformly distributed and it always remains on the surface of the conductor, the electric field inside the sphere will be zero.

2.4 (b) A spherical conductor of radius 12 cm has a charge of $1.6 \times 10^{-7}C$ distributed uniformly on its surface. What is the electric field(b) just outside the sphere

Answer:

Given,

Charge on the conductor $q=1.6*10^{-7}C$

The radius of a spherical conductor $R=12cm=0.12m$

Now,

the electric field outside the spherical conductor is given by:

$E=\frac{kq}{r^2}=\frac{1}{4\pi \epsilon }\frac{q}{r^2}=\frac{9*10^9*1.6*10^{-7}}{0.12^2}=10^5NC^{-1}$

Hence electric field just outside is $10^5NC^{-1}$ .

2.4 (c) A spherical conductor of radius 12 cm has a charge of $1.6\times 10^{-7}C$ distributed uniformly on its surface. What is the electric field (c) at a point 18 cm from the centre of the sphere?

Answer:

Given,

charge on the conductor $q=1.6*10^{-7}C$

The radius of the spherical conductor $R=12cm=0.12m$

Now,

the electric field at point 18cm away from the centre of the spherical conductor is given by:

$E=\frac{kq}{r^2}=\frac{1}{4\pi \epsilon }\frac{q}{r^2}=\frac{9*10^9*1.6*10^{-7}}{0.18^2}=4.4*10^4NC^{-1}$

Hence electric field at the point 18cm away from the centre of the sphere is $4.4*10^4NC^{-1}$

2.5 A parallel plate capacitor with air between the plates has a capacitance of 8 pF $\left ( 1pF = 10^{-12}F \right )$ . What will be the capacitance if the distance between the plates is reduced by half, and the space between them is filled with a substance of dielectric constant 6?

Answer:

As we know,

$C=\frac{\epsilon_r\epsilon_0 A}{d}$

where A= area of the plate

$\epsilon_0$ = permittivity of the free space

d = distance between the plates.

Now, Given

The capacitance between plates initially

$C_{initial}=8pF=\frac{\epsilon A}{d}$

Now, capacitance when the distance is reduced half and filled with the substance of dielectric 6

$C_{final}=\frac{6\epsilon_0 A}{d/2}=12\frac{\epsilon _0A}{d}=12*8pF=96pF$

Hence new capacitance is 96pF.

2.6 (a) Three capacitors each of capacitance 9 pF are connected in series. (a) What is the total capacitance of the combination?

Answer:

Given, 3 capacitor of 9pF connected in series,

the equivalent capacitance when connected in series is given by

$\frac{1}{C_{equivalent}}=\frac{1}{C_1}+\frac{1}{C_2}+\frac{1}{C_3}$

$\frac{1}{C_{equivalent}}=\frac{1}{9}+\frac{1}{9}+\frac{1}{9}=\frac{3}{9}=\frac{1}{3}$

$C_{equivalent}=3pF$

Hence total capacitance of the combination is 3pF

2.6 (b) Three capacitors each of capacitance 9 pF are connected in series. (b) What is the potential difference across each capacitor if the combination is connected to a 120 V supply?

Answer:

Given,

supply Voltage V = 120 V

The potential difference across each capacitor will be one-third of the total voltage

$V_c=\frac{V}{3}=\frac{120}{3}=40V$

Hence potential difference across each capacitor is 40V.

2.7 (a) Three capacitors of capacitances 2 pF, 3 pF and 4 pF are connected in parallel. (a) What is the total capacitance of the combination?

Answer:

Given, 3 capacitors with $C_1=2pF,C_2=3pFandC_3=4pF$ are connected in series,

the equivalent capacitance when connected in parallel is given by

$C_{equivalant}=C_1+C_2+C_3$

$C_{equivalant}=2+3+4=9pF$

Hence, the equivalent capacitance is 9pF.

2.7 (b) Three capacitors of capacitances 2 pF, 3 pF and 4 pF are connected in parallel. (b) Determine the charge on each capacitor if the combination is connected to a 100 V supply.

Answer:

Given, 3 capacitors connected in parallel with

$C_1=2pF$

$C_2=3pF$

$C_3=4pF$

Supply voltage $V=100V$

Since they are connected in parallel, the voltage across each capacitor is 100V.

So, charge on 2pf capacitor :

$Q_1=C_1V=2*10^{-12}*100=2*10^{-10}C$

Charge on 3pF capacitor:

$Q_2=C_2V=3*10^{-12}*100=3*10^{-10}C$

Charge on 4pF capacitor:

$Q_3=C_3V=4*10^{-12}*100=4*10^{-10}C$

Hence charges on capacitors are 2pC,3pC and 4pC respectively

2.8 In a parallel plate capacitor with air between the plates, each plate has an area of $6 \times 10^{-3}m^{2}$ and the distance between the plates is 3 mm. Calculate the capacitance of the capacitor. If this capacitor is connected to a 100 V supply, what is the charge on each plate of the capacitor?

Answer:

Given,

Area of the capacitor plate $A$ = $6 \times 10^{-3}m^{2}$

Distance between the plates $d=3mm$

Now,

The capacitance of the parallel plate capacitor is

$C=\frac{\epsilon_0 A}{d}$

$\epsilon _0$ = permittivity of free space = $8.854*10^{-12}N^{-1}m^{-2}C^{_2}$

putting all know value we get,

$C=\frac{8.854*10^{-12}*6*10^{-3}}{3*10^{-3}}=17.71*10^{-12}F=17.71pF$

Hence capacitance of the capacitor is 17.71pF.

Now,

Charge on the plate of the capacitor :

$Q=CV=17.71*10^{-12}*100=1.771*10^{-9}C$

Hence charge on each plate of the capacitor is $1.771*10^{-9}C$ .

2.9 (a) Explain what would happen if in the capacitor given in exercise 2.8, a 3 mm thick mica sheet (of dielectric constant = 6) were inserted between the plates, (a) while the voltage supply remained connected.

Answer:

Given,

The dielectric constant of the inserted mica sheet = 6

The thickness of the sheet = 3mm

Supply voltage $V = 100V$

Initial capacitance = $C_{initial}=1.771 *10^{-11}F$

Final capacitance = $KC_{initial}=6*1.771 *10^{-11}F=106*10^{-12}F$

Final charge on the capacitor = $Q_{final}=C_{final}V=106*10^{-12}*100=106*10^{-10}C$

Hence on inserting the sheet charge on each plate changes to $106*10^{-10}C$ .

2.9 (b) Explain what would happen if in the capacitor given, a 3 mm thick mica sheet (of dielectric constant = 6) were inserted between the plates,(b) after the supply was disconnected.

Answer:

If a 3mm mica sheet is inserted between plates of the capacitor after disconnecting it from the power supply, the voltage across the capacitor be changed. Since the charge on the capacitor can not go anywhere, if we change the capacitance (which we are doing by inserting mica sheet here), the voltage across the capacitor has to be adjusted accordingly.

As obtained from question number 8 charge on each plate of the capacitor is $1.771*10^{-9}C$

$V_{final}=\frac{Q}{C_{final}}=\frac{1.771\times10^{-9}}{106\times 10^{-12}}=16.7V$

2.10 A 12pF capacitor is connected to a 50V battery. How much electrostatic energy is stored in the capacitor?

Answer:

As we know,

the electrostatic energy stored in the capacitor is

$E=\frac{1}{2}CV^2$

Here,

$C= 12pF$

$V=50V$

So,

$E=\frac{1}{2}CV^2=\frac{1}{2}12*10^{-12}*50^2=1.5*10^{-8}J$

Hence energy stored in the capacitor is $1.5*10^{-8}J$

2.11 A 600pF capacitor is charged by a 200V supply. It is then disconnected from the supply and is connected to another uncharged 600 pF capacitor. How much electrostatic energy is lost in the process?

Answer:

Given

$C=600pF$

$V=200V$

Energy stored :

$E=\frac{1}{2}CV^2=\frac{1}{2}*600*10^{-12}*200*200=1.2*10^{-5}J$

Now, when it is disconnected and connected from another capacitor of capacitance 600pF

New capacitance

$C'=\frac{600*600}{600+600}=300pF$

New electrostatic energy

$E'=\frac{1}{2}C'V^2=\frac{1}{2}*300*10^{-12}*200^2=0.6*10^{-5}J$

Hence loss in energy

$E-E'=1.2*10^{-5}-0.6*10^{-5}J=0.6*10^{-5}$

NCERT solutions for class 12 physics chapter 2 electrostatic potential and capacitance additional exercises:

2.12 A charge of 8 mC is located at the origin. Calculate the work done in taking a small charge of $-2\times 10^{-9}C$ from a point P (0, 0, 3 cm) to a point Q (0, 4 cm, 0), via a point R (0, 6 cm, 9 cm).

Answer:

Given,

The initial distance between two charges

$d_{initial}=3cm$

The final distance between two charges

$d_{final}=4cm$

Hence total work is done

$W=q_2\left ( \frac{kq_1}{d_{final}}-\frac{kq_1}{d_{initial}}\right )=\frac{kq_1q_2}{4\pi\epsilon _0}\left ( \frac{1}{d_{final}}-\frac{1}{d_{initial}} \right )$

$W=9*10^9*8*10^{-3}*(-2*10^{-9})\left ( \frac{1}{0.04}-\frac{1}{0.03} \right )=1.27J$

The path of the charge does not matter, only initial and final position matters.

2.13 A cube of side b has a charge q at each of its vertices. Determine the potential and electric field due to this charge array at the centre of the cube.

Answer:

As we know,

the distance between vertices and the centre of the cube

$d=\frac{\sqrt{3}b}{2}$

Where b is the side of the cube.

So potential at the centre of the cube:

$P=8*\frac{kq}{d}=8*\frac{kq}{b\sqrt{3}/2}=\frac{16kq}{b\sqrt{3}}$

Hence electric potential at the centre will be

$\frac{16kq}{b\sqrt{3}}=\frac{16q}{4\pi \epsilon_0 b\sqrt{3}}=\frac{4q}{\pi \epsilon_0 b\sqrt{3}}$

The electric field will be zero at the centre due to symmetry i.e. every charge lying in the opposite vertices will cancel each other's field.

2.14 (a) Two tiny spheres carrying charges $1.5 \mu C$ and $2.5 \mu C$ are located 30 cm apart. Find the potential and electric field:

(a) at the mid-point of the line joining the two charges

Answer:

As we know

outside the sphere, we can assume it like a point charge. so,

the electric potential at midpoint of the two-sphere

$V=\frac{kq_1}{d/2}+\frac{kq_2}{d/2}$

where q1 and q2 are charges and d is the distance between them

So,

$V=\frac{k1.5*10^{-6}}{0.15}+\frac{k2.5*10^{-6}}{0.15}=2.4*10^5V$

The electric field

$E=\frac{k1.5*10^{-6}}{0.15^2}-\frac{k2.5*10^{-6}}{0.15^2}=4*10^5V/m$

2.14 (b) Two tiny spheres carrying charges $1.5 \mu C$ and $2.5 \mu C$ are located 30 cm apart. Find the potential and electric field:

(b) at a point 10 cm from this midpoint in a plane normal to the line and passing through the mid-point.

Answer:

The distance of the point from both the charges :

$d=\sqrt{0.1^2+0.15^2}=0.18m$

Hence,

Electric potential:

$V=\frac{kq_1}{d}+\frac{kq_2}{d}=\frac{k}{0.18}(1.5+2.5)*10^{-6}=2*10^5V$

Electric field due to q1

$E_1=\frac{kq_1}{d^2}=\frac{k1.5\mu C}{0.18^2m^2}=0.416*10^6V/m$

Electric field due to q2

$E_2=\frac{kq_2}{d^2}=\frac{k2.5\mu C}{0.18^2m^2}=0.69*10^6V/m$

Now,

Resultant Electric field :

$E=\sqrt{E_1^2+E_2^2+2E_1E_2cos\theta}$

Where $\theta$ is the angle between both electric field directions

Here,

$cos\frac{\theta}{2}=\frac{0.10}{0.18}=\frac{5}{9}$

$\frac{\theta}{2}=56.25$

${\theta}=2*56.25=112.5$

Hence

$E=\sqrt{(0.416*10^6)^2+(0.69*10^6)^2+2(0.416*10^6)(0.69*10^6)cos112.5}$

$E=6.6*10^5V/m$

2.15 (a) A spherical conducting shell of inner radius r ₁ and outer radius r ₂ has a charge Q.

(a) A charge q is placed at the centre of the shell. What is the surface charge density on the inner and outer surfaces of the shell?

Answer:

The charge placed on the centre is q, so -q will be the charge induced in the inner shell and + q will be induced in the outer shell

So,

charge density on the inner shell

$\sigma_{inner}=\frac{-q}{4\pi r_1^2}$

charge Density on the outer shell

$\sigma_{outer}=\frac{Q+q}{4\pi r_2^2}$

2.15 (b) A spherical conducting shell of inner radius r ₁ and outer radius r ₂ has a charge Q.

(b)Is the electric field inside a cavity (with no charge) zero, even if the shell is not spherical, but has any irregular shape? Explain.

Answer:

Yes, the electric field inside the cavity is zero even when the shape is irregular and not the sphere. Suppose a Gaussian surface inside the cavity, now since there is no charge inside it, the electric flux through it will be zero according to the guess law. Also, all of the charges will reside on the surface of the conductor so, net charge inside is zero. hence electric field inside cavity is zero.

2.16 (a) Show that the normal component of the electrostatic field has a discontinuity from one side of a charged surface to another given by $(E_{1}-E_{2}).\widehat{n}= \frac{\sigma }{\epsilon _{0}}$

where nˆ is a unit vector normal to the surface at a point and σ is the surface charge density at that point. Hence, show that just outside a conductor, the electric field is $\sigma \frac{\widehat{n}}{\epsilon _{0}}$

Answer:

The electric field on one side of the surface with charge density $\sigma$

$E_1=-\frac{\sigma}{2\epsilon _0}\widehat{n}$

The electric field on another side of the surface with charge density $\sigma$

$E_2=-\frac{\sigma}{2\epsilon _0}\widehat{n}$

Now, resultant of both surfaces:

As E1 and E2 are opposite in direction. we have

$E_1-E_2=\frac{\sigma}{2\epsilon _0}-\left ( -\frac{\sigma}{2\epsilon _0} \right )\widehat{n}=\frac{\sigma}{\epsilon _0}$

There has to be a discontinuity at the sheet of the charge since both electric fields are in the opposite direction.

Now,

Since the electric field is zero inside the conductor,

the electric field just outside the conductor is

$E=\frac{\sigma}{\epsilon _0}\widehat{n}$

2.16 (b) Show that the tangential component of electrostatic field is continuous from one side of a charged surface to another. [Hint: For (a), use Gauss’s law. For, (b) use the fact that work done by electrostatic field on a closed loop is zero.]

Answer:

Let's assume a rectangular loop of length l and small width b.

Now,

Line integral along the loop :

$\oint E.dl=E_1l-E_2l=0$

This implies

$E_1cos\theta_1l-E_2cos\theta_2l=0$

From here,

$E_1cos\theta_1=E_2cos\theta_2$

Since $E_1cos\theta_1$ and $E_2cos\theta_2$ are the tangential component of the electric field, the tangential component of the electric field is continuous across the surface

2.17 A long charged cylinder of linear charged density $\lambda$ is surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders?

Answer:

The charge density of the cylinder with length l and radius r = $\lambda$

The radius of another hollow cylinder with the same length = R

Now, let our gaussian surface be a cylinder with the same length and different radius r

the electric flux through Gaussian surface

$\oint E.ds=\frac{q}{\epsilon _0}$

$E.2\pi rl=\frac{\lambda l}{\epsilon _0}$

$E.=\frac{\lambda }{2\pi \epsilon _0r}$

Hence electric field ar a distance r from the axis of the cylinder is

$E=\frac{\lambda }{2\pi \epsilon _0r}$

2.18 (a) In a hydrogen atom, the electron and proton are bound at a distance of about $0.53 \dot{A}$

(a) Estimate the potential energy of the system in eV, taking the zero of the potential energy at an infinite separation of the electron from proton

Answer:

As we know,

the distance between electron-proton of the hydrogen atom

$d=0.53*10^{-10}m$

The potential energy of the system = potential energy at infinity - potential energy at distance d

$PE=0-\frac{ke*e}{d}=-\frac{9*10^9(1.6*10^{-19})^2}{0.53*10^{10}}=-43.7*10^{-19}J$

As we know,

$1ev=1.6*10^{-19}J$

$PE=\frac{-43.7*10^{-19}}{1.6*10^{-19}}=-27.2eV$

Hence potential energy of the system is -27.2eV.

2.18 (b) In a hydrogen atom, the electron and proton are bound at a distance of about $0.53 \dot{A}$

(b) What is the minimum work required to free the electron, given that its kinetic energy in the orbit is half the magnitude of potential energy obtained in (a)?

Answer:

the potential energy of the system is -27.2eV. (from the previous question)

Kinetic energy is half of the potential energy in magnitude. so kinetic energy = 27/2 = 13.6eV

so,

total energy = 13.6 - 27.2 = -13.6eV

Hence the minimum work required to free the electron is 13.6eV

2.18 (c) In a hydrogen atom, the electron and proton are bound at a distance of about 0.53 $\dot{A}$ :

Answer:

When potential energy is zero at $d'$ 1.06 $\dot{A}$ away,

The potential energy of the system =potential energy at $d'$ -potential energy at d

$PE=\frac{ke*p}{d_1}-27.2= \frac{9*10^{9}*(1.6*10^{-19})^2}{1.06*10^{-10}}=-13.6eV$

Hence potential energy, in this case, would be -13.6eV

2.19 If one of the two electrons of a H ₂ molecule is removed, we get a hydrogen molecular ion $H^{+}_{2}$ . In the ground state of an $H^{+}_{2}$ , the two protons are separated by roughly 1.5 $\dot{A}$ , and the electron is roughly 1 $\dot{A}$ from each proton. Determine the potential energy of the system. Specify your choice of the zero of potential energy.

Answer:

Given,

Distance between proton 1 and 2

$d_{p_1-p_2}=1.5*10^{-10}m$

Distance between proton 1 and electron

$d_{p_1-e}=1*10^{-10}m$

Distance between proton 2 and electron

$d_{p_2-e}=1*10^{-10}m$

Now,

The potential energy of the system :

$V=\frac{kp_1e}{d_{p_1-e}}+\frac{kp_2e}{d_{p_2-e}}+\frac{kp_1p_2}{d_{p_1-p_2}}$

Substituting the values, we get

$V=\frac{9*10^{9}*10^{-19}*10^{-19}}{10^{-10}}\left [ -(16)^2+\frac{(1.6)^2}{1.5} -(1.6)^2\right ]=-19.2eV$

2.20 Two charged conducting spheres of radii a and b are connected to each other by a wire. What is the ratio of electric fields at the surfaces of the two spheres? Use the result obtained to explain why charge density on the sharp and pointed ends of a conductor is higher than on its flatter portions.

Answer:

Since both spheres are connected through the wire, their potential will be the same

Let electric field at A and B be $E_A and E_B$ .

Now,

$\frac{E_A}{E_B}=\frac{Q_A}{Q_B}*\frac{b^2}{a^2}$

also

$\frac{Q_A}{Q_B}=\frac{C_aV}{C_BV}$

Also

$\frac{C_A}{C_B}=\frac{a}{b}$

Therefore,

$\frac{E_A}{E_B}=\frac{ab^2}{ba^2}=\frac{b}{a}$

Therefore the ratio of the electric field is b/a.

2.21 (a) Two charges –q and +q are located at points (0, 0, –a) and (0, 0, a), respectively.

(a) What is the electrostatic potential at the points (0, 0, z) and (x, y, 0) ?

Answer:

1)electric potential at point (0,0,z)

distance from $q_1$

$d_1=\sqrt{0^2+0^2+(0-a-z)^2}=a+z$

distance from $q_2$

$d_2=\sqrt{0^2+0^2+(a-z)^2}=a-z$

Now,

Electric potential :

$V=\frac{kq_1}{a+z}+\frac{kq_2}{a-z}=\frac{2kqa}{z^2-a^2}$

2) Since the point,(x,y,0) lies in the normal to the axis of the dipole, the electric potential at this point is zero.

2.21 (b) Two charges –q and +q are located at points (0, 0, –a) and (0, 0, a), respectively.

(b) Obtain the dependence of potential on the distance r of a point from the origin when $\frac{r}{a}>>1$

Answer:

Here, since distance r is much greater than half the distance between charges, the potential V at a distance r is inversely proportional to the square of the distance

$V\propto \frac{1}{r^2}$

2.21 (c) Two charges –q and +q are located at points (0, 0, –a) and (0, 0, a), respectively

(c) How much work is done in moving a small test charge from the point (5,0,0) to (–7,0,0) along the x-axis? Does the answer change if the path of the test charge between the same points is not along the x-axis?

Answer:

Since point (5,0,0) is equidistance from both charges, they both will cancel out each other potential and hence potential at this point is zero.

Similarly, point (–7,0,0) is also equidistance from both charges. and hence potential at this point is zero.

Since potential at both the point is zero, the work done in moving charge from one point to other is zero. Work done is independent of the path.

2.22 Figure shows a charge array known as an electric quadrupole. For a point on the axis of the quadrupole, obtain the dependence of potential on r for $\frac{r}{a}>>1$ , and contrast your results with that due to an electric dipole, and an electric monopole (i.e., a single charge).

Answer:

Here, As we can see

The electrostatic potential caused by the system of three charges at point P is given by

$V = \frac{1}{4\pi \varepsilon _0}\left [ \frac{q}{r+a}-\frac{2q}{r}+\frac{q}{r-a} \right ]$

$V = \frac{1}{4\pi \varepsilon _0}\left [ \frac{r(r-a)-2(r+a)(r-a)+r(r+a)}{r(r+a)(r-a)}\right ]=\frac{q}{4\pi \epsilon _0}\left [ \frac{2a^2}{r(r^2-a^2)} \right ]$

$V =\frac{q}{4\pi \epsilon _0}\left [ \frac{2a^2}{r^3(1-\frac{a^2}{r^2})} \right ]$

Since

$\frac{r}{a}>>1$

$V=\frac{2qa^2}{4\pi \epsilon _0r^3}$

From here we conclude that

$V\propto \frac{1}{r^3}$

Whereas we know that for a dipole,

$V\propto \frac{1}{r^2}$

And for a monopole,

$V\propto \frac{1}{r}$

2.23 An electrical technician requires a capacitance of $2\mu F$ in a circuit across a potential difference of 1 kV. A large number of $1\mu F$ capacitors are available to him each of which can withstand a potential difference of not more than 400 V. Suggest a possible arrangement that requires the minimum number of capacitors

Answer:

Let's assume n capacitor connected in series and m number of such rows,

Now,

As given

The total voltage of the circuit = 1000V

and the total voltage a capacitor can withstand = 400

From here the total number of the capacitor in series

$n=\frac{1000}{400}=2.5$

Since the number of capacitors can never be a fraction, we take n = 3.

Now,

Total capacitance required = $2\mu F$

Number of rows we need

$m=2*n=2*3=6$

Hence capacitors should be connected in 6 parallel rows where each row contains 3 capacitors in series.

2.24 What is the area of the plates of a 2 F parallel plate capacitor, given that the separation between the plates is 0.5 cm? [You will realise from your answer why ordinary capacitors are in the range of $\mu F$ or less. However, electrolytic capacitors do have a much larger capacitance (0.1 F) because of very minute separation between the conductors.]

Answer:

Given,

The capacitance of the parallel plate capacitor $C=2F$

Separation between plated $d=0.5cm$

Now, As we know

$C=\frac{\epsilon _0A}{d}$

$A=\frac{Cd}{\epsilon _0}=\frac{2*5*10^{-3}}{8.85*10^{-12}}=1.13*10^9m^2$

$A=\1.13*10^3km^2=1130km^2$

Hence, to get capacitance in farads, the area of the plate should be of the order od kilometre which is not good practice, and so that is why ordinary capacitors are of range $\mu F$

2.25 Obtain the equivalent capacitance of the network in Figure. For a 300 V supply, determine the charge and voltage across each capacitor.

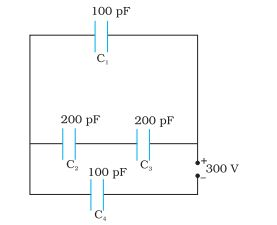
1643085694762 Answer:

Given.

$C_1=100pF$

$C_2=200pF$

$C_3=200pF$

$C_4=100pF$

Now,

Lets first calculate the equivalent capacitance of $C_2\: and \:C_3$

$C_{23}=\frac{C_2C_3}{C_2+C_3}=\frac{200*200}{200+200}=100pF$

Now let's calculate the equivalent of $C_1\:and\:C_{23}$

$C_{1-23}=C_1+C_{23}=100+100=200pF$

Now let's calculate the equivalent of $C_{1-23} \: and \:C_4$

$C_{equivalent}=\frac{C_{1-23}*C_4}{C_{1-23}+C_4}=\frac{100*200}{100+200}=\frac{200}{3}pF$

Now,

The total charge on $C_4$ capacitors:

$Q_4=C_{equivalent}V=\frac{200}{3}*10^{-12}*300=2*10^{-8}C$

So,

$V_4=\frac{Q_4}{C_4}=\frac{2*10^{-8}}{100*10^{-12}}=200V$

The voltage across $C_1$ is given by

$V_{1}=V-V_{4}=300-200=100V$

The charge on $C_1$ is given by

$Q_1=C_1V_1=100*10^{-12}*100=10^{-8}C$

The potential difference across $C_2\:and\:C_3$ is

$V_2=V_3=50V$

Hence Charge on $C_2$

$Q_2=C_2V_2=200*10^{-12}*50=10^{-8}C$

And Charge on $C_3$ :

$Q_3=C_3V_3=200*10^{-12}*50=10^{-8}C$

2.26 (a) The plates of a parallel plate capacitor have an area of 90 cm ² each and are separated by 2.5 mm. The capacitor is charged by connecting it to a 400 V supply.

(a) How much electrostatic energy is stored by the capacitor?

Answer:

Here

The capacitance of the parallel plate capacitor :

$C=\frac{\epsilon_0 A}{d}$

The electrostatic energy stored in the capacitor is given by :

$E=\frac{1}{2}CV^2=\frac{1}{2}\frac{\varepsilon _0A}{d}V^2 =\frac{1.885*10^{-12}90*10^{-4}*400^2}{2*2.5*10^{-3}}=2.55*10^{-6}J$

Hence, the electrostatic energy stored by the capacitor is $2.55*10^{-6}J$ .

2.26 (b) The plates of a parallel plate capacitor have an area of 90 cm2 each and are separated by 2.5 mm. The capacitor is charged by connecting it to a 400 V supply.

(b) View this energy as stored in the electrostatic field between the plates, and obtain the energy per unit volume u. Hence arrive at a relation between u and the magnitude of electric field E between the plates.

Answer:

The volume of the capacitor is:

$V=A*d=90*10^{-4}25*10^{-3}=2.25*10^{-4}m^3$

Now,

Energy stored in the capacitor per unit volume :

$u =\frac{E}{V}=\frac{2.55*10^{-6}}{2.55*10^{-4}}=0.113per \:m^3$

Now, the relation between u and E.

$u =\frac{E}{V}=\frac{\frac{1}{2}CV^2}{Ad}=\frac{\frac{1}{2}(\frac{\epsilon _0A}{d})V^2}{Ad}=\frac{1}{2}\epsilon _0E^2$

2.27 A 4 $\mu F$ capacitor is charged by a 200 V supply. It is then disconnected from the supply and is connected to another uncharged 2 $\mu F$ capacitors. How much electrostatic energy of the first capacitor is lost in the form of heat and electromagnetic radiation?

Answer:

Here,

The charge on the capacitance Initially

$Q=CV=4*10^{-6}*200=8*10^{-4}C$

Total electrostatic energy initially

$E_{initial}=\frac{1}{2}CV^2=\frac{1}{2}4*10^{-6}*(200)^2=8*10^{-2}J$

Now, when it is disconnected and connected to another capacitor

Total new capacitance = $C_{new}=4+2=6\mu F$

Now, by conserving the charge on the capacitor:

$V_{new}C_{new}=C_{initial}V_{initial}$

$V_{new}6\mu F=4\mu F *200$

$V_{new}=\frac{400}{3}V$

Now,

New electrostatic energy :

$E_{new}=\frac{1}{2}C_{new}V_{new}^2=\frac{1}{2}*6*10^{-6}*\left ( \frac{400}{3} \right )^2=5.33*10^{-2}J$

Therefore,

Lost in electrostatic energy

$E=E_{initial}-E_{new}=0.08-0.0533=0.0267J$

2.28 Show that the force on each plate of a parallel plate capacitor has a magnitude equal to $\left (\frac{1}{2} \right )$ QE, where Q is the charge on the capacitor, and E is the magnitude of electric field between the plates. Explain the origin of the factor $\left (\frac{1}{2} \right )$

Answer:

Let

The surface charge density of the capacitor = $\sigma$

Area of the plate = $A$

Now,

As we know,

$Q=\sigma A\:and \: E=\frac{\sigma}{\epsilon _0}$

When the separation is increased by $\Delta x$ ,

work done by external force= $F\Delta x$

Now,

Increase in potential energy :

$\Delta u=u*A\Delta x$

By work-energy theorem,

$F\Delta x=u*A\Delta x$

$F=u*A=\frac{1}{2}\epsilon _0E^2A$

putting the value of $\epsilon _0$

$F=\frac{1}{2}\frac{\sigma}{E}E^2A=\frac{1}{2}\sigma AE=\frac{1}{2}QE$

origin of 1/2 lies in the fact that field is zero inside the conductor and field just outside is E, hence it is the average value of E/2 that contributes to the force.

2.29 A spherical capacitor consists of two concentric spherical conductors, held in position by suitable insulating supports figure. Show that the capacitance of a spherical capacitor is given by $C = \frac{4\pi \epsilon_{0}r_{1}r_{2}}{r_{1}-r_{2}}$ where r ₁ and r ₂ are the radii of outer and inner spheres, respectively.

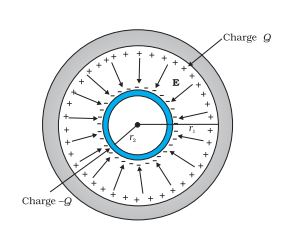
1643085726693

Answer:

Given

the radius of the outer shell = $r_1$

the radius of the inner shell = $r_2$

charge on Inner surface of outer shell = $Q$

Induced charge on the outer surface of inner shell = $-Q$

Now,

The potential difference between the two shells

$V=\frac{Q}{4\pi \epsilon _0r_2}-\frac{Q}{4\pi \epsilon _0r_1}$

Now Capacitance is given by

$C=\frac{Charge(Q)}{Potential\:difference(V)}$

$C=\frac{Q}{\frac{Q(r_1-r_2)}{4\pi \epsilon _0r_1r_2}}=\frac{4\pi \epsilon _0r_1r_2}{r_1-r_2}$

Hence proved.

2.30 (a) A spherical capacitor has an inner sphere of radius 12 cm and an outer sphere of radius 13 cm. The outer sphere is earthed and the inner sphere is given a charge of 2.5 $\mu C$ . The space between the concentric spheres is filled with a liquid of dielectric constant 32.

(a) Determine the capacitance of the capacitor.

Answer:

The capacitance of the capacitor is given by:

$C=\frac{4\pi \epsilon _0\epsilon _rr_1r_2}{r_1-r_2}$

Here,

$C=\frac{32*0.12*0.13}{9*10^9*(0.13-0.12)}=5.5*10^{-9}F$

Hence Capacitance of the capacitor is $5.5*10^{-9}F$ .

2.30 (b) A spherical capacitor has an inner sphere of radius 12 cm and an outer sphere of radius 13 cm. The outer sphere is earthed and the inner sphere is given a charge of 2.5 $\mu C$ . The space between the concentric spheres is filled with a liquid of dielectric constant 32.

(b) what is the potential of the inner sphere?

Answer:

Potential of the inner sphere is given by

$V=\frac{q}{C}=\frac{2.5*10^{-6}}{5.5*10^{-9}}=4.5*10^2$

Hence the potential of the inner sphere is $4.5*10^2 V$ .

2.30 (c) A spherical capacitor has an inner sphere of radius 12 cm and an outer sphere of radius 13 cm. The outer sphere is earthed and the inner sphere is given a charge of 2.5 $\mu$ C. The space between the concentric spheres is filled with a liquid of dielectric constant 32.

(c) Compare the capacitance of this capacitor with that of an isolated sphere of radius 12 cm. Explain why the latter is much smaller.

Answer:

The radius of the isolated sphere $r = 4.5*10^2$

Now, Capacitance of sphere:

$C_{new}=4\pi\epsilon _0r=4\pi 8.85*10^{-12}*12*10^{-12}=1.33*10^{-11}F$

On comparing it with the concentric sphere, it is evident that it has lesser capacitance.this is due to the fact that the concentric sphere is connected to the earth.

Hence the potential difference is less and capacitance is more than the isolated sphere.

Answer carefully:

2.31 (a)

Two large conducting spheres carrying charges Q ₁ and Q ₂ are brought close to each other. Is the magnitude of electrostatic force between them exactly given by $\frac{Q_{1}Q_{2}}{4\pi \epsilon_{0}r^{2}}$ , where r is the distance between their centres?

Answer:

The charge on the sphere is not exactly a point charge, we assume it when the distance between two bodies is large. when the two charged sphere is brought closer, the charge distribution on them will no longer remain uniform. Hence it is not true that electrostatic force between them exactly given by $\frac{Q_{1}Q_{2}}{4\pi \epsilon_{0}r^{2}}$ .

Answer carefully:

2.31 (b)

If Coulomb’s law involved $\frac{1}{r^{3}}$ dependence (instead of $\frac{1}{r^{2}}$ ), would Gauss’s law be still true?

Answer:

Since the solid angle is proportional to $\frac{1}{r^2}$ and not proportional to $\frac{1}{r^3}$ ,

The guess law which is equivalent of coulombs law will not hold true.

Answer carefully:

2.31 (c)

A small test charge is released at rest at a point in an electrostatic field configuration. Will it travel along the field line passing through that point?

Answer:

when a small test charge is released at rest at a point in an electrostatic field configuration it travels along the field line passing through that point only if the field lines are straight because electric field lines give the direction of acceleration, not the velocity

Answer carefully:

2.31 (d)

What is the work done by the field of a nucleus in a complete circular orbit of the electron? What if the orbit is elliptical?

Answer:

The initial and final position will be the same for any orbit whether it is circular or elliptical. Hence work done will always be zero.

Answer carefully:

2.31 (e)

We know that electric field is discontinuous across the surface of a charged conductor. Is electric potential also discontinuous there?

Answer:

Since the electric potential is not a vector quantity unlike the electric field, it can never be discontinuous.

Answer carefully:

2.31 (f) What meaning would you give to the capacitance of a single conductor?

Answer:

There is no meaning in the capacitor with a single plate factually. but we give it meaning by assuming the second plate at infinity. Hence capacitance of a single conductor is the amount of change required to raise the potential of the conductor by one unit amount.

Answer carefully:

2.31 (g)

Guess a possible reason why water has a much greater dielectric constant (= 80) than say, mica (= 6).

Answer:

Water has a much greater dielectric constant than mica because it posses a permanent dipole moment and has an unsymmetrical shape.

2.32 A cylindrical capacitor has two co-axial cylinders of length 15 cm and radii 1.5 cm and 1.4 cm. The outer cylinder is earthed and the inner cylinder is given a charge of 3.5 $\mu$ C. Determine the capacitance of the system and the potential of the inner cylinder. Neglect end effects (i.e., bending of field lines at the ends).

Answer:

Given

Length of cylinder $l=15cm$

inner radius $a=1.4cm$

outer radius $b=1.5cm$

Charge on the inner cylinder $q=3.5\mu C$

Now as we know,

The capacitance of this system is given by

$C=\frac{2\pi \epsilon _0l}{2.303log_{10}(b/a)}$

$C=\frac{2\pi *8.854*10^{-12}*15*10^{-2}}{2.303log_{10}(1.5*10^{-2}/1.4*10^{-2})}=1.21*10^{-10}F$

Now

Since the outer cylinder is earthed the potential at the inner cylinder is equal to the potential difference between two cylinders.

Potential of inner cylinder:

$V=\frac{q}{C}=\frac{3.5*10^{-6}}{1.21*10^{-10}}=2.89*10^4V$

2.33 A parallel plate capacitor is to be designed with a voltage rating 1 kV, using a material of dielectric constant 3 and dielectric strength about $10^{-7}Vm^{-1}$ . (Dielectric strength is the maximum electric field a material can tolerate without breakdown, i.e., without starting to conduct electricity through partial ionisation.) For safety, we should like the field never to exceed, say 10% of the dielectric strength. What minimum area of the plates is required to have a capacitance of 50 pF?

Answer:

Given

Voltage rating in designing capacitor $V=1kV=1000V$

The dielectric constant of the material $K=\epsilon _r=3$

Dielectric strength of material = $10^7V/m$

Safety Condition:

$E=\frac{10}{100}*10^7=10^6V/m$

The capacitance of the plate $C=50pF$

Now, As we know,

$E=\frac{V}{d}$

$d=\frac{V}{E}=\frac{10^3}{10^6}=10^{-3}m$

Now,

$C=\frac{\varepsilon _0\varepsilon_rA }{d}$

$A=\frac{Cd}{\epsilon_0 \epsilon_r }=\frac{50*10^{-12}*10^{-3}}{8.85*10^{-12}*3}=1.98*10^{-3}m^2$

Hence the minimum required area is $1.98*10^{-3}m^2$

Answer the following:

2.36 (a)

The top of the atmosphere is at about 400 kV with respect to the surface of the earth, corresponding to an electric field that decreases with altitude. Near the surface of the earth, the field is about 100 $Vm^{-1}$ . Why then do we not get an electric shock as we step out of our house into the open? (Assume the house to be a steel cage so there is no field inside!)

Answer:

The surface of the earth and our body, both are good conductors. So our body and the ground both have the same equipotential surface as we are connected from the ground. When we move outside the house, the equipotential surfaces in the air changes so that our body and ground is kept at the same potential. Therefore we do not get an electric shock.

Answer the following:

2.36 (b)

(b) A man fixes outside his house one evening a two metre high insulating slab carrying on its top a large aluminium sheet of area 1m ² . Will he get an electric shock if he touches the metal sheet next morning?

Answer:

Yes, the man will get an electric shock. the aluminium sheet is gradually charged up by discharging current of atmosphere.eventually the voltage will increase up to a certain point depending on the capacitance of the capacitor formed by aluminium sheet, insulating slab and the ground. When the man touches the that charged metal, he will get a shock.

Answer the following:

2.36 (c)

c) The discharging current in the atmosphere due to the small conductivity of air is known to be 1800 A on an average over the globe. Why then does the atmosphere not discharge itself completely in due course and become electrically neutral? In other words, what keeps the atmosphere charged?

Answer:

Thunderstorm and lightning across the globe keep the atmosphere charged by releasing the light energy, heat energy, and sound energy in the atmosphere. In a way or other, the atmosphere is discharged through regions of ordinary weather. on an average, the two opposing currents are in equilibrium. Hence the atmosphere perpetually remains charged.

Answer the following:

2.36 (d)

What are the forms of energy into which the electrical energy of the atmosphere is dissipated during a lightning? (Hint: The earth has an electric field of about 100 $Vm^{-1}$ at its surface in the downward direction, corresponding to a surface charge density = $-10^{-9}Cm^{-2}$ . Due to the slight conductivity of the atmosphere up to about 50 km (beyond which it is good conductor), about + 1800 C is pumped every second into the earth as a whole. The earth, however, does not get discharged since thunderstorms and lightning occurring continually all over the globe pump an equal amount of negative charge on the earth.)

Answer:

Electrical energy, of the atmosphere, is dissipated as light energy which comes from lightning, heat energy and sound energy which comes from the thunderstorm.

NCERT solutions for class 12 Physics Chapter-Wise

Electric Charges and Fields

Electrostatic Potential and Capacitance

Current Electricity

Moving Charges and Magnetism

Magnetism and Matter

Electromagnetic Induction

Alternating Current

Electromagnetic Waves

Ray Optics and Optical Instruments

Wave Optics Solutions

Dual Nature of Radiation and Matter

Atoms

Nuclei

Semiconductor Electronics Materials Devices and Simple Circuits

CBSE class 12 physics chapter 2 exercise solutions: Important Formulas and Diagrams

Potential due to the system of point charges

Aakash iACST Scholarship Test 2024

Get up to 90% scholarship on NEET, JEE & Foundation courses

Apply

NEET previous year papers with solutions

Solve NEET previous years question papers & check your preparedness

Apply

1694075255802

1694075253575

Electric potential due to a point charge:

1694075254341

Electric Potential Due to a Dipole

JEE Main Highest Scoring Chapters & Topics

Just Study 40% Syllabus and Score upto 100%

Download EBook

i) At an axial point

if r>>l

ii) At an equatorial point

$V_{equi}= 0$

iii) General point

Combination of capacitors

JEE Main Important Mathematics Formulas

As per latest 2024 syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters

Apply

JEE Main Important Physics formulas

As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters

Apply

For n capacitors connected in parallel, the net value of capacitance is

For n capacitors connected in series, the net value of capacitance is

Energy Stored in a Capacitor:

Electric potential and capacitance NCERT solutions: Main topics

The main headings of ch 2 Class 12 Physics are listed below-

Electric potential and potential energy

Chapter 2 Physics Class 12 discuss electric potential, potential due to an electric dipole at axial and equatorial points, the potential energy of a dipole, potential due to system of charges, the relationship between electric field and electric potential etc. Questions based on these concepts are discussed in the NCERT Solutions for Class 12 Physics Chapter 2 discussed above. Electrostatic Potential and Capacitance Class 12 solutions also discuss Electrostatics of conductor.

Dielectric and Capacitance

Another major discussion coming under the in chapter 2 Physics Class 12 NCERT solutions is about questions on dielectrics and capacitance. The concepts of the dielectric, capacitor, the effect of dielectric on capacitance, combinations of capacitance and energy stored in a capacitor are discussed

d in chapter 2 Physics Class 12. These topics and questions covered in Class 12 Physics Chapter 2 NCERT solutions are important from the exam point of view also.

Importance of NCERT solutions for Class 12 Physics chapter 2 Electrostatic Potential and Capacitance in board exams:

The CBSE NCERT solutions for Class 12 Physics Chapter 2 Electrostatic Potential and Capacitance will help in securing a good score in Physics Class 12 board exam.
On average 4 to 6 % of questions are asked from this chapter for the board exam. The same type of questions discussed in Electrostatic Potential and Capacitance Class 12 NCERT Solutions can also be expected for the exam.
For JEE Main on average, 6% of questions are asked from NCERT Solutions for Class 12 Physics Chapter 2 and for the NEET exam 4 to 6% of questions are asked from the Physics Class 12 chapter 2.
The unit Electrostatics contains the first two chapters of Class 12 NCERT Physics. Which are Electric Charges And Fields And Electric Potential And Capacitance.

Key features of ncert 12 physics chapter 2 solutions

Comprehensive Coverage: These ncert solutions of electric potential and capacitance cover all the important topics and questions presented in the chapter, ensuring a thorough understanding of electrostatic potential and capacitance.
Exercise and Additional Exercise Solutions: Detailed chapter 2 physics class 12 ncert solutions are provided for exercise questions (2.1 to 2.11) and additional exercise questions (2.12 to 2.36), allowing for comprehensive practice and self-assessment.
Clarity and Simplicity: The solutions are explained in clear and simple language, making complex concepts more accessible to students.
Problem-Solving Skills: By practising with these electrostatic potential and capacitance ncert solutions, students develop strong problem-solving skills, which are essential in physics and other subjects.
Exam Preparation: These ncert solutions of electric potential and capacitance are designed to help students prepare effectively for their exams, including board exams and competitive exams like JEE and NEET.

Also Check NCERT Books and NCERT Syllabus here:

NCERT Exemplar Class 12 Solutions

Subject-Wise Solutions:

Frequently Asked Question (FAQs)

1. What is the importance of the Class 12 Physics unit Electrostatics for CBSE board exam?

The unit Electrostatics have the first two chapters of Class 12 Physics. A total of 6 to 8 marks questions can be expected from the chapter for CBSE board exam. A good score can be obtained in the CBSE board exam by following NCERT syllabus and problems. For extra questions related to the chapter refer NCERT exemplar questions and the CBSE previous year board papers.

2. What is the weightage of the chapter Electrostatic Potential and Capacitance for NEET exams?

The Class 12 NCERT chapter Electrostatic Potential and Capacitance is an important chapter for NEET exams. Combining the chapter 1 and 2 of class 12 NCERT Physics a total of 8 to 10% questions can be expected for NEET.

3. How many questions are asked from the chapter Electrostatic Potential and Capacitance for JEE main?

A total of 2 or 3 questions can be expected from the unit Electrostatics. From the chapter Electrostatic Potential and Capacitance 1 or 2 questions can be expected or the questions may be using the combinations of concepts in the Class 12 Physics chapter 1 and 2

4. What are the main topics used to solve questions of of Electrostatic Potential And Capacitance Class 12 NCERT Physics Chapter?

The main topics covered in Electrostatic Potential And Capacitance Class 12 are-

Electrostatic Potential
Potential Due To A Point Charge
Potential Due To An Electric Dipole
Potential Due To A System Of Charges
Equipotential Surfaces
Relation Between Field And Potential
Potential Energy Of A System Of Charges
Potential Energy In An External Field
Potential Energy Of A Single Charge
Electrostatics Of Conductors
Dielectrics And Polarization
Capacitors And Capacitance
The Parallel Plate Capacitor
Effect Of Dielectric On Capacitance
Combination Of Capacitors
Energy Stored In A Capacitor
Van De Graaff Generator

5. What is the difference between Electrostatic Potential and Capacitance?

The electrostatic potential is a measure of the amount of work required to move a unit charge from a reference point to a specific location in an electric field. It is a scalar quantity and is often represented by the symbol V.

Capacitance is a measure of the ability of a device to store an electric charge. It is a property of a capacitor, which is a passive electrical component that consists of two conductive plates separated by an insulating material called a dielectric. The capacitance of a capacitor is determined by the size and shape of the plates, the distance between them, and the type of dielectric material used. It is a scalar quantity and is often represented by the symbol C

Also Read

NCERT Class 12 Physics Chapter 9 Notes Ray Optics and Optical Instruments - Download PDF

NCERT Class 12 Physics Chapter 8 Notes Electromagnetic Waves - Download PDF

NCERT Class 12 Physics Chapter 7 Notes Alternating Current - Download PDF

NCERT Class 12 Physics Chapter 6 Notes Electromagnetic Induction - Download PDF

NCERT Class 12 Physics Chapter 5 Notes Magnetism and Matter - Download PDF

NCERT Class 12 Physics Chapter 4 Notes Moving Charges and Magnetism - Download PDF

NCERT Books for Class 12 Hindi 2024 - Download chapter wise PDFs here

NCERT Books for Class 12 Biology 2024 - Download Free PDF

NCERT Books for Class 12 Chemistry 2024 - Download Free Pdf

NCERT Book for Class 12 Maths 2024 - Download Free PDF

NCERT Book for Class 12 Physics 2024 - Download Free PDF

NCERT Books for Class 12 - Download All Subjects PDFs Here

NCERT Syllabus for Class 12 English 2024 - Download Syllabus PDF

NCERT Syllabus for Class 12 2024 (All Subjects) - Download PDF Here

NCERT Syllabus for Class 6 to 12 (2023-24) - All Subjects PDF Download

NCERT Solutions for Miscellaneous Exercise Chapter 7 Class 12 - Integrals

NCERT Solutions for Exercise 4.1 Class 11 Maths Chapter 4 - Principle of Mathematical Induction

NCERT Solutions for Class 12 Biology - Check CBSE 12 Biology NCERT Chapterwise Solution

NCERT Solutions for Class 12 Maths Chapter 11 Three Dimensional Geometry

NCERT Solutions for Class 12

NCERT Solutions for Class 12 Maths (Updated 2023-24)

NCERT Exemplar Class 12 Physics Solutions Chapter 8 Electromagnetic Waves

Articles

UP Board Result 2024, Check UPMSP 10th, 12th Results 2024

Apr 19, 2024

UP Board Compartment Result 2024 for Class 10th & 12th @upmsp.edu.in

Apr 19, 2024

UPMSP UP Board Exam Date 2024 Class 10 and 12 (Out) - Download UP Board Time Table PDF

Apr 19, 2024

UP Board Scrutiny Result 2024 - Check UPMSP Class 10,12th Verification Results

Apr 19, 2024

UP Board Class 12 Model Paper 2023-24 PDF Download, Answer Key, Previous Year 12th UPMSP Model Papers here

Apr 19, 2024

CBSE Class 12 Physical Education Syllabus 2024-25 (New Released) : Download PDF

Apr 17, 2024

CBSE Result DigiLocker 2024 - Download 10th, 12th Result via DigiLocker Login, Passing & Migration Certificate

Apr 15, 2024

5 min ⋆ Sep 28, 2023

Study Help

Cancer Treatment: Why Chemotherapy Does Not Suit All Patients

Result

Mock Test

View All School Exams

Certifications By Top Providers

Artificial Intelligence Projects

Via Great Learning

Introduction to Web Development with HTML CSS JavaScript

Via IBM

Full-Stack Web Development

Via Masai School

Digital Banking Business Model

Via State Bank of India

Business Analytics Foundations

Via PW Skills

Python Fundamentals for Beginners

Via Great Learning

1110 courses

789 courses

328 courses

136 courses

Harvard University, Cambridge

98 courses

IBM

86 courses

IT & Software

Teaching and Academics

Programming and Development

Health, Fitness and Medicine

Business and Management

Engineering and Architecture

General Management

Financial Management

History

Environmental Studies

Cloud Computing

Law

Explore Top Universities Across Globe

University of Essex, Colchester

Wivenhoe Park Colchester CO4 3SQ

University College London, London

Gower Street, London, WC1E 6BT

The University of Edinburgh, Edinburgh

Old College, South Bridge, Edinburgh, Post Code EH8 9YL

University of Bristol, Bristol

Beacon House, Queens Road, Bristol, BS8 1QU

University of Nottingham, Nottingham

University Park, Nottingham NG7 2RD

Lancaster University, Lancaster

Bailrigg, Lancaster LA1 4YW

Top Benefits of Studying in New Zealand 2024 - Low Cost, Top Universities, Visa Free Travel, Job Opportunities

6 min ⋆ Mar 01, 2024 12:03 PM IST

Top MBBS Colleges in Asian Countries - Course Details, Tuition Fees, Eligibility

5 min ⋆ Mar 01, 2024 11:03 AM IST

Countries to Study Abroad for Indians other than Canada

6 min ⋆ Feb 28, 2024 07:02 AM IST

TOEFL Reading Section: Strategies for Effective Preparation

5 min ⋆ Mar 01, 2024 10:03 AM IST

TOEFL Preparation Time 2024: How To Prepare For TOEFL?

4 min ⋆ Mar 01, 2024 10:03 AM IST

IELTS General Training Preparation 2024 - Tips, Strategies & Practice

5 min ⋆ Mar 01, 2024 06:03 AM IST

Related E-books & Sample Papers

CBSE Class 11, 12 Physics Syllabus 2024-25

232+ Downloads

CBSE Class 11, 12 Economics Syllabus 2024-25

76+ Downloads

CBSE Class 11, 12 Sociology Syllabus 2024-25

108+ Downloads

CBSE Class 11, 12 Sanskrit (Elective) Syllabus 2024-25

31+ Downloads

CBSE Class 11, 12 Sanskrit (Core) Syllabus 2024-25

8+ Downloads

CBSE Class 11, 12 Psychology Syllabus 2024-25

33+ Downloads

Questions related to CBSE Class 12th

Have a question related to CBSE Class 12th ?

I am interested in graphic designing and coding. how do I make a career .which stream should I go.what engineering subject to take

Hello aspirant,

The purpose of graphic design extends beyond the brand's look. Nevertheless, by conveying what the brand stands for, it significantly aids in the development of a sense of understanding between a company and its audience. The future in the field of graphic designing is very promising.

There are various courses available for graphic designing. To know more information about these courses and much more details, you can visit our website by clicking on the link given below.

https://www.careers360.com/courses/graphic-designing-course

Thank you

Hope this information helps you.

Read Complete Answer

Sajal Trivedi 29 January,2024

i couldn't clear 1st compartment (which held in August 2022) cbse class 12th so can I give improvement in all subjects which to be held on March 2023. tell me a solution i need more than 75% in class 12th

hello,

Yes you can appear for the compartment paper again since CBSE gives three chances to a candidate to clear his/her exams so you still have two more attempts. However, you can appear for your improvement paper for all subjects but you cannot appear for the ones in which you have failed.

I hope this was helpful!

Good Luck

Read Complete Answer

Upasana Barman 24 August,2022

i was not able to clear 1st compartment and now I have to give 2nd compartment so can I give improvement exam next year? plz tell me very much tensed(cbse class 12th)

Hello dear,

If you was not able to clear 1st compartment and now you giving second compartment so YES, you can go for your improvement exam next year but if a student receives an improvement, they are given the opportunity to retake the boards as a private candidate the following year, but there are some requirements. First, the student must pass all of their subjects; if they received a compartment in any subject, they must then pass the compartment exam before being eligible for the improvement.

As you can registered yourself as private candidate for giving your improvement exam of 12 standard CBSE(Central Board of Secondary Education).For that you have to wait for a whole year which is bit difficult for you.

Positive side of waiting for whole year is you have a whole year to preparing yourself for your examination. You have no distraction or something which may causes your failure in the exams. In whole year you have to stay focused on your 12 standard examination for doing well in it. By this you get a highest marks as a comparison of others.

Believe in Yourself! You can make anything happen

All the very best.

Read Complete Answer

Mayankjha.student2007 23 August,2022

if i am giving improvement in one subject this year, will i be able to give improvement in more subjects next year?

Hello Student,

I appreciate your Interest in education. See the improvement is not restricted to one subject or multiple subjects and we cannot say if improvement in one subject in one year leads to improvement in more subjects in coming year.

You just need to have a revision of all subjects what you have completed in the school. have a revision and practice of subjects and concepts helps you better.

All the best.

Read Complete Answer

Nuthalapati Vyshnavi 21 August,2022

I got an RT for 2 subjects, so do I have to give exams for all the subjects in the coming year or just the 2. pls help me

Hi,

You just need to give the exams for the concerned two subjects in which you have got RT. There is no need to give exam for all of your subjects, you can just fill the form for the two subjects only.

Read Complete Answer

Muskan Dhalwal 17 August,2022

View All

Popular CBSE Class 12th Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms^-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times. Assume that the potential energy lost each time he lowers the mass is dissipated. How much fat will he use up considering the work done only when the weight is lifted up ? Fat supplies 3.8×10⁷J of energy per kg which is converted to mechanical energy with a 20% efficiency rate. Take g = 9.8 ms⁻² :

Option 1)

2.45×10⁻³ kg

Option 2)

6.45×10⁻³ kg

Option 3)

9.89×10⁻³ kg

Option 4)

12.89×10⁻³ kg

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

A particle is projected at 60⁰ to the horizontal with a kinetic energy $K$ . The kinetic energy at the highest point

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

In the reaction,

$2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

How many moles of magnesium phosphate, $Mg_{3}(PO_{4})_{2}$ will contain 0.25 mole of oxygen atoms?

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

With increase of temperature, which of these changes?

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol^-1) is

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t²) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m² , the number of rotations made by the pulley before its direction of motion if reversed, is

Option 1) less than 3	Option 2) more than 3 but less than 6
Option 3) more than 6 but less than 9	Option 4) more than 9

Data Administrator

Database professionals use software to store and organise data such as financial information, and customer shipping records. Individuals who opt for a career as data administrators ensure that data is available for users and secured from unauthorised sales. DB administrators may work in various types of industries. It may involve computer systems design, service firms, insurance companies, banks and hospitals.

4 Jobs Available

Bio Medical Engineer

The field of biomedical engineering opens up a universe of expert chances. An Individual in the biomedical engineering career path work in the field of engineering as well as medicine, in order to find out solutions to common problems of the two fields. The biomedical engineering job opportunities are to collaborate with doctors and researchers to develop medical systems, equipment, or devices that can solve clinical problems. Here we will be discussing jobs after biomedical engineering, how to get a job in biomedical engineering, biomedical engineering scope, and salary.

4 Jobs Available

Ethical Hacker

A career as ethical hacker involves various challenges and provides lucrative opportunities in the digital era where every giant business and startup owns its cyberspace on the world wide web. Individuals in the ethical hacker career path try to find the vulnerabilities in the cyber system to get its authority. If he or she succeeds in it then he or she gets its illegal authority. Individuals in the ethical hacker career path then steal information or delete the file that could affect the business, functioning, or services of the organization.

3 Jobs Available

GIS Expert

GIS officer work on various GIS software to conduct a study and gather spatial and non-spatial information. GIS experts update the GIS data and maintain it. The databases include aerial or satellite imagery, latitudinal and longitudinal coordinates, and manually digitized images of maps. In a career as GIS expert, one is responsible for creating online and mobile maps.

3 Jobs Available

Data Analyst

The invention of the database has given fresh breath to the people involved in the data analytics career path. Analysis refers to splitting up a whole into its individual components for individual analysis. Data analysis is a method through which raw data are processed and transformed into information that would be beneficial for user strategic thinking.

Data are collected and examined to respond to questions, evaluate hypotheses or contradict theories. It is a tool for analyzing, transforming, modeling, and arranging data with useful knowledge, to assist in decision-making and methods, encompassing various strategies, and is used in different fields of business, research, and social science.

3 Jobs Available

Geothermal Engineer

Individuals who opt for a career as geothermal engineers are the professionals involved in the processing of geothermal energy. The responsibilities of geothermal engineers may vary depending on the workplace location. Those who work in fields design facilities to process and distribute geothermal energy. They oversee the functioning of machinery used in the field.

3 Jobs Available

Database Architect

If you are intrigued by the programming world and are interested in developing communications networks then a career as database architect may be a good option for you. Data architect roles and responsibilities include building design models for data communication networks. Wide Area Networks (WANs), local area networks (LANs), and intranets are included in the database networks. It is expected that database architects will have in-depth knowledge of a company's business to develop a network to fulfil the requirements of the organisation. Stay tuned as we look at the larger picture and give you more information on what is db architecture, why you should pursue database architecture, what to expect from such a degree and what your job opportunities will be after graduation. Here, we will be discussing how to become a data architect. Students can visit NIT Trichy, IIT Kharagpur, JMI New Delhi.

3 Jobs Available

Remote Sensing Technician

Individuals who opt for a career as a remote sensing technician possess unique personalities. Remote sensing analysts seem to be rational human beings, they are strong, independent, persistent, sincere, realistic and resourceful. Some of them are analytical as well, which means they are intelligent, introspective and inquisitive.

Remote sensing scientists use remote sensing technology to support scientists in fields such as community planning, flight planning or the management of natural resources. Analysing data collected from aircraft, satellites or ground-based platforms using statistical analysis software, image analysis software or Geographic Information Systems (GIS) is a significant part of their work. Do you want to learn how to become remote sensing technician? There's no need to be concerned; we've devised a simple remote sensing technician career path for you. Scroll through the pages and read.

3 Jobs Available

Budget Analyst

Budget analysis, in a nutshell, entails thoroughly analyzing the details of a financial budget. The budget analysis aims to better understand and manage revenue. Budget analysts assist in the achievement of financial targets, the preservation of profitability, and the pursuit of long-term growth for a business. Budget analysts generally have a bachelor's degree in accounting, finance, economics, or a closely related field. Knowledge of Financial Management is of prime importance in this career.

4 Jobs Available

Data Analyst

3 Jobs Available

Underwriter

An underwriter is a person who assesses and evaluates the risk of insurance in his or her field like mortgage, loan, health policy, investment, and so on and so forth. The underwriter career path does involve risks as analysing the risks means finding out if there is a way for the insurance underwriter jobs to recover the money from its clients. If the risk turns out to be too much for the company then in the future it is an underwriter who will be held accountable for it. Therefore, one must carry out his or her job with a lot of attention and diligence.

3 Jobs Available

5 Jobs Available

The word “choreography" actually comes from Greek words that mean “dance writing." Individuals who opt for a career as a choreographer create and direct original dances, in addition to developing interpretations of existing dances. A Choreographer dances and utilises his or her creativity in other aspects of dance performance. For example, he or she may work with the music director to select music or collaborate with other famous choreographers to enhance such performance elements as lighting, costume and set design.

2 Jobs Available

A multimedia specialist is a media professional who creates, audio, videos, graphic image files, computer animations for multimedia applications. He or she is responsible for planning, producing, and maintaining websites and applications.

2 Jobs Available

Welding Engineer

5 Jobs Available

QA Manager

4 Jobs Available

Quality Controller

A quality controller plays a crucial role in an organisation. He or she is responsible for performing quality checks on manufactured products. He or she identifies the defects in a product and rejects the product.

A quality controller records detailed information about products with defects and sends it to the supervisor or plant manager to take necessary actions to improve the production process.

3 Jobs Available

An AWS Solution Architect is someone who specializes in developing and implementing cloud computing systems. He or she has a good understanding of the various aspects of cloud computing and can confidently deploy and manage their systems. He or she troubleshoots the issues and evaluates the risk from the third party.

4 Jobs Available

Azure Administrator

An Azure Administrator is a professional responsible for implementing, monitoring, and maintaining Azure Solutions. He or she manages cloud infrastructure service instances and various cloud servers as well as sets up public and private cloud systems.