NCERT Solutions for Class 12 Maths Chapter 2 Inverse Trigonometric Functions

Edited By Komal Miglani | Updated on Jun 17, 2025 08:11 AM IST | #CBSE Class 12th

Upcoming Event

CBSE Class 12th Exam Date : 15 Jul' 2025 - 15 Jul' 2025

In the universe of trigonometry, inverse functions are the keys to unlocking the angles when only the ratios are given. In the inverse trigonometric functions class 12 solutions, students will learn about functions that help to determine the angles of a right angle when only a specific ratio of the sides is given. NCERT solutions for class 12 Maths highlight that for every ratio, there exists a unique angle, just like every answer has a counter question, every sine, cosine, or tangent has an inverse.

New: CBSE 12 Class Free Mock Test

Latest: JEE Main high scoring chapters | JEE Main 10 year's papers

This Story also Contains

NCERT Solution for Class 12 Maths Chapter 2 Solutions: Download PDF
NCERT Solutions for Class 12 Maths Chapter 2: Exercise Questions
Class 12 Maths NCERT Chapter 2: Extra Question
Inverse Trigonometric Functions Class 12 Chapter 2: Topics
Inverse Trigonometric Functions Class 12 NCERT Solutions - Important Formulae
Approach to Solve Questions of Inverse Trigonometric Functions Class 12
What Extra Should Students Study Beyond the NCERT for JEE?
NCERT Solutions for Class 12 Maths: Chapter Wise

NCERT Solutions for Class 12 Maths Chapter 2 Inverse Trigonometric Functions

Inverse trigonometric functions class 12 NCERT solutions mainly focus on the restrictions on domains and ranges of trigonometric functions that ensure the existence of their inverses. This chapter's learning applies to many fields, including engineering, navigation, astronomy, architecture, and robotics. Experienced Careers360 experts prepared these solutions using the NCERT, following the latest CBSE guidelines.

NCERT Solution for Class 12 Maths Chapter 2 Solutions: Download PDF

Students who wish to access the Class 12 Maths Chapter 2 NCERT Solutions can click on the link below to download the complete solution in PDF.

Download PDF

NCERT Solutions for Class 12 Maths Chapter 2: Exercise Questions

NCERT Inverse Trigonometric Functions Class 12 Solutions: Exercise 2.1
Page number: 26-27
Total questions: 14

Aakash Repeater Courses

Take Aakash iACST and get instant scholarship on coaching programs.

Apply

Question 1: Find the principal values of the following: $\sin^{- 1} (\frac{- 1}{2})$

Answer:

Let $x = \sin^{- 1} (\frac{- 1}{2})$

$⟹ \sin x = \frac{- 1}{2} = - \sin (\frac{π}{6}) = \sin (- \frac{π}{6})$
We know, principle value range of $s i n^{- 1}$ is $[- \frac{π}{2}, \frac{π}{2}]$

$∴$ The principal value of $\sin^{- 1} (\frac{- 1}{2})$ is $- \frac{π}{6},$

Question 2: Find the principal values of the following: $\cos^{- 1} (\frac{\sqrt{3}}{2})$

Answer:

So, let us assume that $\cos^{- 1} (\frac{\sqrt{3}}{2}) = x$ then,

Taking the inverse of both sides, we get;

$c o s x = (\frac{\sqrt{3}}{2})$ , or $c o s (\frac{π}{6}) = (\frac{\sqrt{3}}{2})$

and as we know that the principal values of $c o s^{- 1}$ is from [0, $π$ ],

Hence $c o s x = (\frac{\sqrt{3}}{2})$ when x = $\frac{π}{6}$ .

Therefore, the principal value for $\cos^{- 1} (\frac{\sqrt{3}}{2})$ is $\frac{π}{6}$ .

Question 3: Find the principal values of the following: ${cosec}^{- 1} (2)$

Answer:

Let us assume that ${cosec}^{- 1} (2) = x$ , then we have;

$C o s e c x = 2$ , or

$C o s e c (\frac{π}{6}) = 2$ .

And we know the range of principal values is $[\frac{- π}{2}, \frac{π}{2}] - {0} .$

Therefore the principal value of ${cosec}^{- 1} (2)$ is $\frac{π}{6}$ .

Question 4: Find the principal values of the following: $\tan^{- 1} (- \sqrt{3})$

Answer:

Let us assume that $\tan^{- 1} (- \sqrt{3}) = x$ , then we have;

$\tan x = (- \sqrt{3})$ or

$- \tan (\frac{π}{3}) = \tan (\frac{- π}{3}) .$

and as we know that the principal value of $\tan^{- 1}$ is $(\frac{- π}{2}, \frac{π}{2})$ .

Hence the only principal value of $\tan^{- 1} (- \sqrt{3})$ when $x = \frac{- π}{3}$ .

Question 5: Find the principal values of the following: $\cos^{- 1} (- \frac{1}{2})$

Answer:

Let us assume that $\cos^{- 1} (- \frac{1}{2}) = y$ then,

Easily we have; $\cos y = (\frac{- 1}{2})$ or we can write it as:

$- \cos (\frac{π}{3}) = \cos (π - \frac{π}{3}) = \cos (\frac{2 π}{3}) .$

As we know that the range of the principal values of $\cos^{- 1}$ is $[0, π]$

Hence $\frac{2 π}{3}$ lies in the range; it is a principal solution.

Question 6: Find the principal values of the following $\tan^{- 1} (- 1)$

Answer:

Given $\tan^{- 1} (- 1)$ , we can assume it to be equal to 'z';

$\tan^{- 1} (- 1) = z$ ,

$\tan z = - 1$

$- \tan (\frac{π}{4}) = \tan (\frac{- π}{4}) = - 1$

And as we know the range of principal values of $\tan^{- 1}$ from $(\frac{- π}{2}, \frac{π}{2})$ .

As only one value z = $- \frac{π}{4}$ lies hence we have only one principal value that is $- \frac{π}{4}$ .

Question 7: Find the principal values of the following: $\sec^{- 1} (\frac{2}{\sqrt{3}})$

Answer:

Let us assume that $\sec^{- 1} (\frac{2}{\sqrt{3}}) = z$ then,

we can also write it as; $\sec z = (\frac{2}{\sqrt{3}})$ .

Or $\sec (\frac{π}{6}) = (\frac{2}{\sqrt{3}})$ and the principal values lies between $[0, π] - {\frac{π}{2}}$ .

Hence we get only one principal value of $\sec^{- 1} (\frac{2}{\sqrt{3}})$ i.e., $\frac{π}{6}$ .

Question 8: Find the principal values of the following: $\cot^{- 1} (\sqrt{3})$

Answer:

Let us assume that $\cot^{- 1} (\sqrt{3}) = x$ , then we can write in other way,

$\cot x = (\sqrt{3})$ or

$\cot (\frac{π}{6}) = (\sqrt{3})$ .

Hence when $x = \frac{π}{6}$ we have $\cot (\frac{π}{6}) = (\sqrt{3})$ .

and the range of principal values of $\cot^{- 1}$ lies in $(0, π)$ .

Then the principal value of $\cot^{- 1} (\sqrt{3})$ is $\frac{π}{6}$

Question 9: Find the principal values of the following: $\cos^{- 1} (- \frac{1}{\sqrt{2}})$

Answer:

Let us assume $\cos^{- 1} (- \frac{1}{\sqrt{2}}) = x$ ;

Then we have $\cos x = (\frac{- 1}{\sqrt{2}})$

$- \cos (\frac{π}{4}) = (\frac{- 1}{\sqrt{2}})$ ,

$\cos (π - \frac{π}{4}) = \cos (\frac{3 π}{4})$ .

And we know the range of principal values of $\cos^{- 1}$ is $[0, π]$ .

So, the only principal value which satisfies $\cos^{- 1} (- \frac{1}{\sqrt{2}}) = x$ is $\frac{3 π}{4}$ .

Question 10: Find the principal values of the following: ${cosec}^{- 1} (- \sqrt{2})$

Answer:

Let us assume the value of ${cosec}^{- 1} (- \sqrt{2}) = y$ , then

we have $c o s e c y = (- \sqrt{2})$
or

$- c o s e c (\frac{π}{4}) = (- \sqrt{2}) = c o s e c (\frac{- π}{4})$ .

and the range of the principal values of ${cosec}^{- 1}$ lies between $[\frac{- π}{2}, \frac{π}{2}] - {0}$ .

Hence the principal value of ${cosec}^{- 1} (- \sqrt{2})$ is $\frac{- π}{4}$ .

Question 11: Find the values of the following: $\tan^{- 1} (1) + \cos^{- 1} (- \frac{1}{2}) + \sin^{- 1} (- \frac{1}{2})$

Answer:

To find the values, first we declare each term to some constant ;

$t a n^{- 1} (1) = x$ , So we have $\tan x = 1$ ;

or $\tan (\frac{π}{4}) = 1$

Therefore, $x = \frac{π}{4}$

$c o s^{- 1} (\frac{- 1}{2}) = y$

So, we have

$\cos y = (\frac{- 1}{2}) = - \cos (\frac{π}{3}) = \cos (π - \frac{π}{3}) = \cos (\frac{2 π}{3})$ .

Therefore $y = \frac{2 π}{3}$ ,

$\sin^{- 1} (\frac{- 1}{2}) = z$ ,

So we have;

$\sin z = \frac{- 1}{2}$ or $- \sin (\frac{π}{6}) = \sin (\frac{- π}{6}) = \frac{- 1}{2}$

Therefore $z = - \frac{π}{6}$

Hence, we can calculate the sum:

$= \frac{π}{4} + \frac{2 π}{3} - \frac{π}{6}$

$= \frac{3 π + 8 π - 2 π}{12} = \frac{9 π}{12} = \frac{3 π}{4}$ .

Question 12: Find the values of the following: $\cos^{- 1} (\frac{1}{2}) + 2 \sin^{- 1} (\frac{1}{2})$

Answer:

Here we have $\cos^{- 1} (\frac{1}{2}) + 2 \sin^{- 1} (\frac{1}{2})$

Let us assume that the value of

$\cos^{- 1} (\frac{1}{2}) = x, a n d \sin^{- 1} (\frac{1}{2}) = y$ ;

Then we have to find out the value of +2y.

Calculation of x :

$\Rightarrow \cos^{- 1} (\frac{1}{2}) = x$

$\Rightarrow \cos x = \frac{1}{2}$

$\Rightarrow \cos \frac{π}{3} = \frac{1}{2}$ ,

Hence $x = \frac{π}{3}$ .

Calculation of y :

$\Rightarrow \sin^{- 1} (\frac{1}{2}) = y$

$\Rightarrow \sin y = \frac{1}{2}$

$\Rightarrow \sin \frac{π}{6} = \frac{1}{2}$ .

Hence $y = \frac{π}{6}$ .

The required sum will be = $\frac{π}{3} + 2 (\frac{π}{6}) = \frac{2 π}{3}$ .

Question 13: If $\sin^{- 1} x = y$ then

(A) $0 \leq y \leq π$

(B) $- \frac{π}{2} \leq y \leq \frac{π}{2}$

(D) $- \frac{π}{2} < y < \frac{π}{2}$

Answer:

Given if $\sin^{- 1} x = y$ then,

As we know that the $\sin^{- 1}$ can take values between $[\frac{- π}{2}, \frac{π}{2}] .$

Therefore, $- \frac{π}{2} \leq y \leq \frac{π}{2}$ .

Hence, answer choice (B) is correct.

Question 14: $\tan^{- 1} (\sqrt{3}) - \sec^{- 1} (- 2)$ is equal to

(A) $π$

(B) $- \frac{π}{3}$

(D) $\frac{2 π}{3}$

Answer:

Let us assume the values of $\tan^{- 1} (\sqrt{3})$ be 'x' and $\sec^{- 1} (- 2)$ be 'y'.

Then we have;

$\tan^{- 1} (\sqrt{3}) = x$ or $\tan x = \sqrt{3}$ or $\tan \frac{π}{3} = \sqrt{3}$ or

$x = \frac{π}{3}$ .

and $\sec^{- 1} (- 2) = y$
or $\sec y = - 2$

or $- \sec (\frac{π}{3}) = \sec (π - \frac{π}{3}) = \sec \frac{2 π}{3}$

$y = \frac{2 π}{3}$

also, the ranges of the principal values of $\tan^{- 1}$ and $\sec^{- 1}$ are $(\frac{- π}{2}, \frac{π}{2})$ . and

$[0, π] - {\frac{π}{2}}$ respectively.

$∴$ we have then;

$\tan^{- 1} (\sqrt{3}) - \sec^{- 1} (- 2)$

$= \frac{π}{3} - \frac{2 π}{3} = - \frac{π}{3}$

NCERT Inverse Trigonometric Functions Class 12 Solutions: Exercise 2.2
Page number: 29-30
Total questions: 15

Question 1: Prove the following: $3 \sin^{- 1} x = \sin^{- 1} (3 x - 4 x^{3}), x \in [- \frac{1}{2}, \frac{1}{2}]$

Answer:

Given to prove: $3 \sin^{- 1} x = \sin^{- 1} (3 x - 4 x^{3})$

where, $x ϵ [- \frac{1}{2}, \frac{1}{2}]$ .

Take $θ = \sin^{- 1} x$ or $x = \sin θ$

Take R.H.S value

$\sin^{- 1} (3 x - 4 x^{3})$

= $\sin^{- 1} (3 \sin θ - 4 \sin^{3} θ)$

= $\sin^{- 1} (\sin 3 θ)$

= $3 θ$

= $3 \sin^{- 1} x$ = L.H.S

Question 2: Prove the following: $3 \cos^{- 1} x = \cos^{- 1} (4 x^{3} - 3 x), x \in [\frac{1}{2}, 1]$

Answer:

Given to prove $3 \cos^{- 1} x = \cos^{- 1} (4 x^{3} - 3 x), x \in [\frac{1}{2}, 1]$ .

Take $\cos^{- 1} x = θ$ or $\cos θ = x$ ;

Then we have;

R.H.S.

$\cos^{- 1} (4 x^{3} - 3 x)$

= $\cos^{- 1} (4 \cos^{3} θ - 3 \cos θ)$ $[∵ 4 \cos^{3} θ - 3 \cos θ = \cos 3 θ]$

= $\cos^{- 1} (\cos 3 θ)$

= $3 θ$

= $3 \cos^{- 1} x$ = L.H.S

Hence, Proved.

Question 3: Write the following functions in the simplest form: $\tan^{- 1} \frac{\sqrt{1 + x^{2}} - 1}{x}, x \neq 0$

Answer:

We have $\tan^{- 1} \frac{\sqrt{1 + x^{2}} - 1}{x}$

Take

$∴$ $\tan^{- 1} \frac{\sqrt{1 + x^{2}} - 1}{x} = \tan^{- 1} \frac{\sqrt{1 + \tan^{2} Θ - 1}}{\tan Θ}$

$= \tan^{- 1} (\frac{s e c Θ - 1}{t a n Θ}) = \tan^{- 1} (\frac{1 - c o s Θ}{s i n Θ})$

$= \tan^{- 1} (\frac{2 s i n^{2} (\frac{Θ}{2})}{2 s i n \frac{Θ}{2} c o s \frac{Θ}{2}})$

$= \tan^{- 1} (\tan \frac{Θ}{2}) = \frac{Θ}{2} = \frac{1}{2} \tan^{- 1} x$

$= \frac{1}{2} \tan^{- 1} x$ is the simplified form.

Question 4: Write the following functions in the simplest form: $\tan^{- 1} (\sqrt{\frac{1 - \cos x}{1 + \cos x}}), 0 < x < π$

Answer:

Given that $\tan^{- 1} (\sqrt{\frac{1 - \cos x}{1 + \cos x}}), 0 < x < π$

We have in inside the root the term : $\frac{1 - \cos x}{1 + \cos x}$

Put $1 - \cos x = 2 \sin^{2} \frac{x}{2}$ and $1 + \cos x = 2 \cos^{2} \frac{x}{2}$ ,

Then we have,

$= \tan^{- 1} (\sqrt{\frac{2 \sin^{2} \frac{x}{2}}{2 \cos^{2} \frac{x}{2}}})$

$= \tan^{- 1} (\frac{\sin \frac{x}{2}}{\cos \frac{x}{2}})$

$= \tan^{- 1} (\tan \frac{x}{2}) = \frac{x}{2}$

Hence the simplest form is $\frac{x}{2}$

Question 5: Write the following functions in the simplest form: $\tan^{- 1} (\frac{\cos x - \sin x}{\cos x + \sin x}), \frac{- π}{4} < x < \frac{3 π}{4}$

Answer:

Given $\tan^{- 1} (\frac{\cos x - \sin x}{\cos x + \sin x})$ where $x ϵ (\frac{- π}{4} < x < \frac{3 π}{4})$

So,

$= \tan^{- 1} (\frac{\cos x - \sin x}{\cos x + \sin x})$

Taking $\cos x$ common from the numerator and the denominator.

We get:

$= \tan^{- 1} (\frac{1 - (\frac{\sin x}{\cos x})}{1 + (\frac{\sin x}{\cos x})})$

$= \tan^{- 1} (\frac{1 - \tan x}{1 + \tan x})$

= $\tan^{- 1} (1) - \tan^{- 1} (\tan x)$ as, $[∵ \tan^{- 1} x - \tan^{- 1} y = \frac{x - y}{1 + x y}]$

= $\frac{π}{4} - x$ is the simplest form.

Question 6: Write the following functions in the simplest form: $\tan^{- 1} \frac{x}{\sqrt{a^{2} - x^{2}}}, | x | < a$

Answer:

Given that $\tan^{- 1} \frac{x}{\sqrt{a^{2} - x^{2}}}, | x | < a$

Take $x = a \sin θ$ or

$θ = \sin^{- 1} (\frac{x}{a})$ and putting it in the equation above;

$\tan^{- 1} \frac{a \sin θ}{\sqrt{a^{2} - (a \sin θ)^{2}}}$

$= \tan^{- 1} \frac{a \sin θ}{a \sqrt{1 - \sin^{2} θ}}$

$= \tan^{- 1} (\frac{\sin θ}{\sqrt{\cos^{2} θ}}) = \tan^{- 1} (\frac{\sin θ}{\cos θ})$

$= \tan^{- 1} (\tan θ)$

$= θ = \sin^{- 1} (\frac{x}{a})$ is the simplest form.

Question 7: Write the following functions in the simplest form: $\tan^{- 1} (\frac{3 a^{2} x - x^{3}}{a^{3} - 3 a x^{2}}), a > 0; \frac{- a}{\sqrt{3}} < x < \frac{a}{\sqrt{3}}$

Answer:

Given $\tan^{- 1} (\frac{3 a^{2} x - x^{3}}{a^{3} - 3 a x^{2}})$

Here we can take $x = a \tan θ \Rightarrow \frac{x}{a} = \tan θ$

So, $θ = \tan^{- 1} (\frac{x}{a})$

$\tan^{- 1} (\frac{3 a^{2} x - x^{3}}{a^{3} - 3 a x^{2}})$ will become;

$= \tan^{- 1} (\frac{3 a^{2} a \tan θ - (a \tan θ)^{3}}{a^{3} - 3 a (a \tan θ)^{2}}) = \tan^{- 1} (\frac{3 a^{3} \tan θ - a^{3} \tan^{3} θ}{a^{3} - 3 a^{3} \tan^{2} θ})$

and as $[∵ (\frac{3 \tan θ - \tan^{3} θ}{1 - 3 \tan^{2} θ}) = \tan 3 θ]$ ;

$= 3 θ$

$= 3 \tan^{- 1} (\frac{x}{a})$

hence the simplest form is $3 \tan^{- 1} (\frac{x}{a})$ .

Question 8: Find the values of each of the following: $\tan^{- 1} [2 \cos (2 \sin^{- 1} \frac{1}{2})]$

Answer:

Given equation:

$\tan^{- 1} [2 \cos (2 \sin^{- 1} \frac{1}{2})]$

So, solving the inner bracket first, we take the value of $\sin x^{- 1} \frac{1}{2} = x .$

Then we have,

$\sin x = \frac{1}{2} = \sin (\frac{π}{6})$

Therefore, we can write $\sin^{- 1} \frac{1}{2} = \frac{π}{6}$ .

$\tan^{- 1} [2 \cos (2 \sin^{- 1} \frac{1}{2})] = \tan^{- 1} [2 \cos (2 \times \frac{π}{6})]$

$= \tan^{- 1} [2 \cos (\frac{π}{3})] = \tan^{- 1} [2 \times (\frac{1}{2})] = \tan^{- 1} 1 = \frac{π}{4}$ .

Question 9: Find the values of each of the following: $\tan \frac{1}{2} [\sin^{- 1} \frac{2 x}{1 + x^{2}} + c o s^{- 1} \frac{1 - y^{2}}{1 + y^{2}}], | x | < 1, y > 0$ and $x y < 1$

Answer:

Taking the value $x = \tan Θ$ or $\tan^{- 1} x = Θ$ and $y = \tan Θ$ or $\tan^{- 1} y = Θ$ then we have,

= $\tan \frac{1}{2} [\sin^{- 1} \frac{2 \tan Θ}{1 + (\tan Θ)^{2}} + c o s^{- 1} \frac{1 - \tan^{2} Θ}{1 + (\tan Θ)^{2}}]$ ,

= $\tan \frac{1}{2} [\sin^{- 1} (\sin 2 Θ) + c o s^{- 1} (\cos 2 Θ)]$

$∵ [\cos^{- 1} (\frac{1 - \tan^{2} Θ}{1 + \tan^{2} Θ}) = \cos^{- 1} (\cos 2 Θ),]$

$∵ [\sin^{- 1} (\frac{2 \tan Θ}{1 + \tan^{2} Θ}) = \sin^{- 1} (\sin 2 Θ)]$

Then,

$= \tan \frac{1}{2} [2 \tan^{- 1} x + 2 \tan^{- 1} y]$ $∵ [\tan^{- 1} x + \tan^{- 1} y = \tan^{- 1} \frac{x + y}{1 - x y}]$

$= \tan [\tan^{- 1} \frac{x + y}{1 - x y}]$

$= \frac{x + y}{1 - x y}$

Question 10: If $\tan^{- 1} \frac{x - 1}{x - 2} + \tan^{- 1} \frac{x + 1}{x + 2} = \frac{π}{4}$ , then find the value of $x$ .

Answer:

Using the identity $\tan^{- 1} x + \tan^{- 1} y = \tan^{- 1} \frac{x + y}{1 - x y}$ ,

We can find the value of x.

So, $\tan^{- 1} \frac{x - 1}{x - 2} + \tan^{- 1} \frac{x + 1}{x + 2} = \frac{π}{4}$

on applying,

= $\tan^{- 1} \frac{\frac{x - 1}{x - 2} + \frac{x + 1}{x + 2}}{1 - (\frac{x - 1}{x - 2}) (\frac{x + 1}{x + 2})}$

$= \tan^{- 1} \frac{\frac{(x - 1) (x + 2) + (x - 2) (x + 1)}{x^{2} - 4}}{1 - \frac{x^{2} - 1}{x^{2} - 4}} = \tan^{- 1} [\frac{2 x^{2} - 4}{- 3}] = \frac{π}{4}$

$= \frac{2 x^{2} - 4}{- 3} = \tan (\frac{π}{4}) = 1$

= $2 x^{2} = 1$ or $x = \pm \frac{1}{\sqrt{2}}$ ,

Hence, the possible values of x are $\pm \frac{1}{\sqrt{2}}$ .

Question 11: Find the values of each of the expressions $\sin^{- 1} (\sin \frac{2 π}{3})$

Answer:

Given $\sin^{- 1} (\sin \frac{2 π}{3})$ ;

We know that $\sin^{- 1} (\sin x) = x$

If the value of x belongs to $[\frac{- π}{2}, \frac{π}{2}]$ then we get the principal values of $\sin^{- 1} x$ .

Here, $\frac{2 π}{3} \notin [\frac{- π}{2}, \frac{π}{2}]$

We can write $\sin^{- 1} (\sin \frac{2 π}{3})$ is as:

= $\sin^{- 1} [\sin (π - \frac{2 π}{3})]$

= $\sin^{- 1} [\sin \frac{π}{3}]$ where $\frac{π}{3} ϵ [\frac{- π}{2}, \frac{π}{2}]$

$∴ \sin^{- 1} (\sin \frac{2 π}{3}) = \sin^{- 1} [\sin \frac{π}{3}] = \frac{π}{3}$

Question 12: Find the values of each of the expressions $\tan^{- 1} (\tan \frac{3 π}{4})$

Answer:

As we know $\tan^{- 1} (\tan x) = x$

If $x ϵ (- \frac{π}{2}, \frac{π}{2}) .$ which is the principal value range of $\tan^{- 1} x$ .

So, as in $\tan^{- 1} (\tan \frac{3 π}{4})$ ;

$\frac{3 π}{4} \notin (- \frac{π}{2}, \frac{π}{2})$

Hence we can write $\tan^{- 1} (\tan \frac{3 π}{4})$ as :

$\tan^{- 1} (\tan \frac{3 π}{4})$ = $\tan^{- 1} (\tan \frac{3 π}{4}) = \tan^{- 1} [\tan (π - \frac{π}{4})] = \tan^{- 1} [\tan (\frac{- π}{4})]$

Where $- \frac{π}{4} ϵ (- \frac{π}{2}, \frac{π}{2})$

and $∴ \tan^{- 1} (\tan \frac{3 π}{4}) = \tan^{- 1} [\tan (\frac{- π}{4})] = - \frac{π}{4}$

Question 13: Find the values of each of the expressions $\tan (\sin^{- 1} \frac{3}{5} + \cot^{- 1} \frac{3}{2})$

Answer:

Given that $\tan (\sin^{- 1} \frac{3}{5} + \cot^{- 1} \frac{3}{2})$

we can take $\sin^{- 1} \frac{3}{5} = x$ ,

then $\sin x = \frac{3}{5}$

or $\cos x = \sqrt{1 - \sin^{2} x} = \frac{4}{5}$

$\Rightarrow \tan x = \frac{\frac{3}{5}}{\frac{4}{5}} = \frac{3}{4}$

$\Rightarrow \tan^{- 1} \frac{3}{4} = x$

We have similarities

$\cot^{- 1} \frac{3}{2} = \tan^{- 1} \frac{2}{3}$

Therefore we can write $\tan (\sin^{- 1} \frac{3}{5} + \cot^{- 1} \frac{3}{2})$

$= \tan (\tan^{- 1} \frac{3}{4} + \tan^{- 1} \frac{2}{3})$

$= \tan [\tan^{- 1} (\frac{\frac{3}{4} + \frac{2}{3}}{1 - \frac{3}{4} . \frac{2}{3}})]$ from $A s, [\tan^{- 1} x + \tan^{- 1} y = \tan^{- 1} \frac{x + y}{1 - x y}]$

$= \tan (\tan^{- 1} \frac{9 + 8}{12 - 6}) = \tan (\tan^{- 1} \frac{17}{6}) = \frac{17}{6}$

Question 14: $\cos^{- 1} (\cos \frac{7 π}{6})$ is equal to

(A) $\frac{7 π}{6}$

(B) $\frac{5 π}{6}$

(D) $\frac{π}{6}$

Answer:

As we know that $\cos^{- 1} (c o s x) = x$ if $x ϵ [0, π]$ and is principal value range of $\cos^{- 1} x$ .

In this case $\cos^{- 1} (\cos \frac{7 π}{6})$ ,

$\frac{7 π}{6} \notin [0, π]$

hence we have then,

$\cos^{- 1} (\cos \frac{7 π}{6}) =$ $\cos^{- 1} (\cos \frac{- 7 π}{6}) = \cos^{- 1} [\cos (2 π - \frac{7 π}{6})]$

$[∵ \cos (2 π + x) = \cos x]$

$∴ w e h a v e \cos^{- 1} (\cos \frac{7 π}{6}) = \cos^{- 1} (\cos \frac{5 π}{6}) = \frac{5 π}{6}$

Hence the correct answer is $\frac{5 π}{6}$ (B).

Question 15: $\sin (\frac{π}{3} - \sin^{- 1} (- \frac{1}{2}))$ is equal to

(A) $\frac{1}{2}$

(B) $- \frac{π}{2}$

(D) $1$

Answer:

Solving the inner bracket of $\sin (\frac{π}{3} - \sin^{- 1} (- \frac{1}{2}))$ ;

$(\frac{π}{3} - \sin^{- 1} (- \frac{1}{2}))$ or

Take $\sin^{- 1} (- \frac{1}{2}) = x$ then,

$\sin x = - \frac{1}{2}$ and we know the range of principal value of $\sin^{- 1} x i s [- \frac{π}{2}, \frac{π}{2}] .$

Therefore we have $\sin^{- 1} (- \frac{1}{2}) = - \frac{π}{6}$ .

Hence, $\sin (\frac{π}{3} - \sin^{- 1} (- \frac{1}{2})) = \sin (\frac{π}{3} + \frac{π}{6}) = \sin (\frac{3 π}{6}) = \sin (\frac{π}{2}) = 1$

Hence, the correct answer is D.

Question 15: $\tan^{- 1} \sqrt{3} - \cot^{- 1} (- \sqrt{3})$ is equal to

(A) $π$

(B) $- \frac{π}{2}$

(D) $2 \sqrt{3}$

Answer:

We have $\tan^{- 1} \sqrt{3} - \cot^{- 1} (- \sqrt{3})$ ;

finding the value of $\cot^{- 1} (- \sqrt{3})$ :

Assume $\cot^{- 1} (- \sqrt{3}) = y$ then,

$\cot y = - \sqrt{3}$ and the range of the principal value of $\cot^{- 1}$ is $(0, π)$ .

Hence, principal value is $\frac{5 π}{6}$

Therefore $\cot^{- 1} (- \sqrt{3}) = \frac{5 π}{6}$

and $\tan^{- 1} \sqrt{3} = \frac{π}{3}$

So, we have now,

$\tan^{- 1} \sqrt{3} - \cot^{- 1} (- \sqrt{3}) = \frac{π}{3} - \frac{5 π}{6}$

$= \frac{2 π - 5 π}{6} = \frac{- 3 π}{6}$

or, $= \frac{- π}{2}$

Hence, the answer is option (B).

NCERT Inverse Trigonometric Functions Class 12 Solutions: Miscellaneous Exercise
Page number: 31-32
Total questions: 14

Question 1: Find the value of the following: $\cos^{- 1} (\cos (\frac{13 π}{6}))$

Answer:

If $x ϵ [0, π]$ then $\cos^{- 1} (\cos x) = x$ , which is principal value of $\cos^{- 1} x$ .

So, we have $\cos^{- 1} (\cos (\frac{13 π}{6}))$

$w h e r e \frac{13 π}{6} \notin [0, π] .$

$H e n c e w e c a n w r i t e \cos^{- 1} (\cos (\frac{13 π}{6})) a s$

$= \cos^{- 1} (\cos (2 π + \frac{π}{6}))$

$= \cos^{- 1} (\cos (\frac{π}{6}))$

$\frac{π}{6} ϵ [0, π]$

Therefore, we have,

$\cos^{- 1} (\cos (\frac{13 π}{6})) = \cos^{- 1} (\cos (\frac{π}{6})) = \frac{π}{6}$ .

Question 2: Find the value of the following: $\tan^{- 1} (\tan \frac{7 π}{6})$

Answer:

We have given $\tan^{- 1} (\tan \frac{7 π}{6})$ ;

so, as we know $\tan^{- 1} (\tan x) = x i f x ϵ (- \frac{π}{2}, \frac{π}{2})$

So, here we have $\frac{7 π}{6} \notin (- \frac{π}{2}, \frac{π}{2})$ .

Therefore we can write $\tan^{- 1} (\tan \frac{7 π}{6})$ as:

$= \tan^{- 1} (\tan (2 π - \frac{5 π}{6}))$ $[∵ \tan (2 π - x) = - \tan x]$

$= \tan^{- 1} [- \tan (\frac{5 π}{6})]$

$= \tan^{- 1} [\tan (π - \frac{5 π}{6})]$

$= \tan^{- 1} [\tan (\frac{π}{6})] w h e r e \frac{π}{6} ϵ (- \frac{π}{2}, \frac{π}{2})$

$∴ \tan^{- 1} (\tan \frac{7 π}{6}) = \tan^{- 1} (\tan \frac{π}{6}) = \frac{π}{6}$ .

Question 3: Prove that $2 \sin^{- 1} \frac{3}{5} = \tan^{- 1} \frac{24}{7}$

Answer:

To prove: $2 \sin^{- 1} \frac{3}{5} = \tan^{- 1} \frac{24}{7}$ ;

$L . H . S = 2 \sin^{- 1} \frac{3}{5}$

Assume that $\sin^{- 1} \frac{3}{5} = x$

then we have $\sin x = \frac{3}{5}$ .

or $\cos x = \sqrt{1 - {(\frac{3}{5})}^{2}} = \frac{4}{5}$

Therefore we have

$\tan x = \frac{3}{4} o r x = \sin^{- 1} \frac{3}{5} = \tan^{- 1} \frac{3}{4}$

Now,

We can write L.H.S as

$2 \sin^{- 1} \frac{3}{5} = 2 \tan^{- 1} \frac{3}{4}$

$= \tan^{- 1} [\frac{2 \times \frac{3}{4}}{1 - {(\frac{3}{4})}^{2}}]$ as we know $[2 \tan^{- 1} x = \tan^{- 1} \frac{2 x}{1 - x^{2}}]$

$= \tan^{- 1} [\frac{\frac{3}{2}}{(\frac{16 - 9}{16})}] = \tan^{- 1} (\frac{3}{2} \times \frac{16}{7})$

$= \tan^{- 1} \frac{24}{7} = R . H . S$

L.H.S = R.H.S

Question 4: Prove that $\sin^{- 1} \frac{8}{17} + \sin^{- 1} \frac{3}{5} = \tan^{- 1} \frac{77}{36}$

Answer:

Taking $\sin^{- 1} \frac{8}{17} = x$

then,

$\sin x = \frac{8}{17} \Rightarrow \cos x = \sqrt{1 - {(\frac{8}{17})}^{2}} = \sqrt{\frac{225}{289}} = \frac{15}{17} .$

Therefore, we have-

$\tan^{- 1} x = \frac{8}{15} \Rightarrow x = \tan^{- 1} \frac{8}{15}$

$∴ \sin^{- 1} \frac{8}{17} = \tan^{- 1} \frac{8}{15}$ .............(1).

$N o w, l e t \sin^{- 1} \frac{3}{5} = y$ ,

Then,

$\sin^{- 1} \frac{3}{5} = \tan^{- 1} \frac{3}{4}$ .............(2).

So, we have now,

L.H.S.

$\sin^{- 1} \frac{8}{17} + \sin^{- 1} \frac{3}{5}$

Using equations (1) and (2), we get,

$= \tan^{- 1} \frac{8}{15} + \tan^{- 1} \frac{3}{4}$

$= \tan^{- 1} \frac{\frac{8}{15} + \frac{3}{4}}{1 - \frac{8}{15} \times \frac{3}{4}}$
$[∵ \tan^{- 1} x + \tan^{- 1} y = \tan^{- 1} \frac{x + y}{1 - x y}]$

$= \tan^{- 1} (\frac{32 + 45}{60 - 24})$

$= \tan^{- 1} (\frac{77}{36})$

= R.H.S.

Question 5: Prove that $\cos^{- 1} \frac{4}{5} + \cos^{- 1} \frac{12}{13} = \cos^{- 1} \frac{33}{65}$

Answer:

Take $\cos^{- 1} \frac{4}{5} = x$ and $\cos^{- 1} \frac{12}{13} = y$ and $\cos^{- 1} \frac{33}{65} = z$

Then we have,

$\cos x = \frac{4}{5}$

$\sin x = \sqrt{1 - {(\frac{4}{5})}^{2}} = \frac{3}{5}$

Then we can write it as:

$\tan x = \frac{\frac{3}{5}}{\frac{4}{5}} = \frac{3}{4}$ or $x = \tan^{- 1} \frac{3}{4}$

$∴ \cos^{- 1} \frac{4}{5} = \tan^{- 1} \frac{3}{4}$ ...............(1)

Now, $\cos^{- 1} \frac{12}{13} = y$

$\cos y = \frac{12}{13} \Rightarrow$ $\sin y = \frac{5}{13}$

$∴ \tan y = \frac{5}{12} \Rightarrow y = \tan^{- 1} \frac{5}{12}$

So, $\cos^{- 1} \frac{12}{13} = \tan^{- 1} \frac{5}{12}$ ...................(2)

Also, we have similarities;

$\cos^{- 1} \frac{33}{65} = z$

Then,

$\cos^{- 1} \frac{33}{65} = \tan^{- 1} \frac{56}{33}$ ...........................(3)

Now, we have

L.H.S

$\cos^{- 1} \frac{4}{5} + \cos^{- 1} \frac{12}{13}$ so, using (1) and (2), we get,

$= \tan^{- 1} \frac{3}{4} + \tan^{- 1} \frac{5}{12}$

$= \tan^{- 1} (\frac{\frac{3}{4} + \frac{5}{12}}{1 - (\frac{3}{4} \times \frac{5}{12})})$
$∵ [\tan^{- 1} x + \tan^{- 1} y = \tan^{- 1} \frac{x + y}{1 - x y}]$

$= \tan^{- 1} (\frac{36 + 20}{48 - 15})$

$= \tan^{- 1} (\frac{56}{33})$ or we can write it as;

$= \cos^{- 1} \frac{33}{65}$

= R.H.S.

Hence proved.

Question 6: Prove that $\cos^{- 1} \frac{12}{13} + \sin^{- 1} \frac{3}{5} = \sin^{- 1} \frac{56}{65}$

Answer:

Converting all terms in tan form;

Let $\cos^{- 1} \frac{12}{13} = x$ , $\sin^{- 1} \frac{3}{5} = y$ and $\sin^{- 1} \frac{56}{65} = z$ .

Now, converting all the terms:

$\cos^{- 1} \frac{12}{13} = x$ or $\cos x = \frac{12}{13}$

We can write it in tan form as:

$\cos x = \frac{12}{13} \Rightarrow$ $\sin x = \frac{5}{13}$ .

$∴ \tan x = \frac{5}{12} \Rightarrow x = \tan^{- 1} \frac{5}{12}$

or $\cos^{- 1} \frac{12}{13} = \tan^{- 1} \frac{5}{12}$ ................(1)

$\sin^{- 1} \frac{3}{5} = y$ or $\sin y = \frac{3}{5}$

We can write it in tan form as:

$\sin y = \frac{3}{5} \Rightarrow$ $\cos y = \frac{4}{5}$

$∴ \tan y = \frac{3}{4} \Rightarrow y = \tan^{- 1} \frac{3}{4}$

or $\sin^{- 1} \frac{3}{5} = \tan^{- 1} \frac{3}{4}$ ......................(2)

Similarly, for $\sin^{- 1} \frac{56}{65} = z$ ;

we have $\sin^{- 1} \frac{56}{65} = \tan^{- 1} \frac{56}{33}$ .............(3)

Using (1) and (2), we have L.H.S

$\cos^{- 1} \frac{12}{13} + \sin^{- 1} \frac{3}{5}$

$= \tan^{- 1} \frac{5}{12} + \tan^{- 1} \frac{3}{4}$

On applying $\tan^{- 1} x + \tan^{- 1} y = \tan^{- 1} \frac{x + y}{1 - x y}$

We have,

$= \tan^{- 1} \frac{\frac{5}{12} + \frac{3}{4}}{1 - (\frac{5}{12} . \frac{3}{4})}$

$= \tan^{- 1} (\frac{20 + 36}{48 - 15})$

$= \tan^{- 1} (\frac{56}{33})$

$= \sin^{- 1} (\frac{56}{65})$ ...........[Using (3)]

=R.H.S.

Hence proved.

Question 7: Prove that $\tan^{- 1} \frac{63}{16} = \sin^{- 1} \frac{5}{13} + \cos^{- 1} \frac{3}{5}$

Answer:

Taking R.H.S;

We have $\sin^{- 1} \frac{5}{13} + \cos^{- 1} \frac{3}{5}$

Converting sin and cos terms to tan forms:

Let $\sin^{- 1} \frac{5}{13} = x$ and $\cos^{- 1} \frac{3}{5} = y$

now, we have $\sin^{- 1} \frac{5}{13} = x$ or $\sin x = \frac{5}{13}$

$\sin x = \frac{5}{13} o r \cos x = \frac{12}{13} o r \tan x = \frac{5}{12}$

$\tan x = \frac{5}{12} \Rightarrow x = \tan^{- 1} \frac{5}{12}$

$∴ \sin^{- 1} \frac{5}{13} = \tan^{- 1} \frac{5}{12}$ ............(1)

Now, $\cos^{- 1} \frac{3}{5} = y \Rightarrow \cos y = \frac{3}{5}$

$\cos y = \frac{3}{5} o r \sin y = \frac{4}{5} o r \tan y = \frac{4}{3}$

$\tan y = \frac{4}{3} \Rightarrow y = \tan^{- 1} \frac{4}{3}$

$∴ \cos^{- 1} \frac{3}{5} = \tan^{- 1} \frac{4}{5}$ ................(2)

Now, using (1) and (2), we get,

R.H.S.

$\sin^{- 1} \frac{5}{13} + \cos^{- 1} \frac{3}{5} = \tan^{- 1} \frac{5}{12} + \tan^{- 1} \frac{4}{3}$

$= \tan^{- 1} (\frac{\frac{5}{12} + \frac{4}{3}}{1 - \frac{5}{12} \times \frac{4}{3}})$ as we know $[\tan^{- 1} x + \tan^{- 1} y = \tan^{- 1} \frac{x + y}{1 - x y}]$

so,

$= \tan^{- 1} \frac{63}{16}$

equal to L.H.S

Hence proved.

Question 8: Prove that $\tan^{- 1} \sqrt{x} = \frac{1}{2} \cos^{- 1} \frac{1 - x}{1 + x}, x \in [0, 1]$

Answer:

By observing the square root, we will first put

$x = \tan^{2} θ$ .

Then,

we have $\tan^{- 1} \sqrt{\tan^{2} θ} = \frac{1}{2} \cos^{- 1} \frac{1 - \tan^{2} θ}{1 + \tan^{2} θ}$

or, R.H.S.

$\frac{1}{2} \cos^{- 1} \frac{1 - \tan^{2} θ}{1 + \tan^{2} θ} = \frac{1}{2} \cos^{- 1} (c o s 2 θ)$

$= \frac{1}{2} \times 2 θ = θ$ .

L.H.S. $\tan^{- 1} \sqrt{\tan^{2} θ} = \tan^{- 1} (\tan θ) = θ$

hence L.H.S. = R.H.S proved.

Question 9: Prove that $\cot^{- 1} (\frac{\sqrt{1 + \sin x} + \sqrt{1 - \sin x}}{\sqrt{1 + \sin x} - \sqrt{1 - \sin x}}) = \frac{x}{2}, x \in (0, \frac{π}{4})$

Answer:

Given that $\cot^{- 1} (\frac{\sqrt{1 + \sin x} + \sqrt{1 - \sin x}}{\sqrt{1 + \sin x} - \sqrt{1 - \sin x}})$

By observing, we can rationalise the fraction

$(\frac{\sqrt{1 + \sin x} + \sqrt{1 - \sin x}}{\sqrt{1 + \sin x} - \sqrt{1 - \sin x}})$

We get then,

$= (\frac{\sqrt{1 + \sin x} + \sqrt{1 - \sin x}}{\sqrt{1 + \sin x} - \sqrt{1 - \sin x}}) = (\frac{(\sqrt{1 + \sin x} + \sqrt{1 - \sin x})^{2}}{1 + \sin x - 1 + \sin x})$

$= (\frac{1 + \sin x + 1 - \sin x + 2 \sqrt{(1 + \sin x) (1 - \sin x)}}{1 + \sin x - 1 + \sin x})$

$= \frac{2 (1 + \sqrt{1 - \sin^{2} x})}{2 \sin x} = \frac{1 + \cos x}{\sin x} = \frac{2 \cos^{2} \frac{x}{2}}{2 \sin \frac{x}{2} \cos \frac{x}{2}}$

$= \cot \frac{x}{2}$

Therefore, we can write it as;

$\cot^{- 1} (\cot \frac{x}{2}) = \frac{x}{2}$

As L.H.S. = R.H.S.

Hence proved.

Question 10: Prove that $\tan^{- 1} (\frac{\sqrt{1 + x} - \sqrt{1 - x}}{\sqrt{1 + x} + \sqrt{1 - x}}) = \frac{π}{4} - \frac{1}{2} \cos^{- 1} x, - \frac{1}{\sqrt{2}} \leq x \leq 1$

[Hint: Put $x = \cos 2 θ$ ]

Answer:

By using the Hint, we will put $x = \cos 2 θ$ ;

We get then,

$= \tan^{- 1} (\frac{\sqrt{1 + \cos 2 θ} - \sqrt{1 - \cos 2 θ}}{\sqrt{1 + \cos 2 θ} + \sqrt{1 - \cos 2 θ}})$

$= \tan^{- 1} (\frac{\sqrt{2 \cos^{2} θ} - \sqrt{2 \sin^{2} θ}}{\sqrt{2 \cos^{2} θ} + \sqrt{2 \sin^{2} θ}})$

$= \tan^{- 1} (\frac{\sqrt{2} \cos θ - \sqrt{2} \sin θ}{\sqrt{2} \cos θ + \sqrt{2} \sin θ})$

$= \tan^{- 1} (\frac{\cos θ - \sin θ}{\cos θ + \sin θ})$ dividing numerator and denominator by $\cos θ$ ,

We get,

$= \tan^{- 1} (\frac{1 - \tan θ}{1 + \tan θ})$

$= \tan^{- 1} 1 - \tan^{- 1} (\tan θ)$ using the formula $[\tan^{- 1} x - \tan^{- 1} y = \tan^{- 1} \frac{x - y}{1 + x y}]$

$= \frac{π}{4} - θ = \frac{π}{4} - \frac{1}{2} \cos^{- 1} x$

As L.H.S = R.H.S

Hence proved

Question 11: Solve the following equations: $2 \tan^{- 1} (\cos x) = \tan^{- 1} (2 cosec x)$

Answer:

Given equation $2 \tan^{- 1} (\cos x) = \tan^{- 1} (2 cosec x)$ ;

Using the formula:

$[2 \tan^{- 1} z = \tan^{- 1} \frac{2 z}{1 - z^{2}}]$

We can write

$2 \tan^{- 1} (\cos x) = \tan^{- 1} [\frac{2 \cos x}{1 - (\cos x)^{2}}]$

$\tan^{- 1} [\frac{2 \cos x}{1 - (\cos x)^{2}}] = \tan^{- 1} [2 c o s e c x]$

So, we can equate;

$= [\frac{2 \cos x}{1 - (\cos x)^{2}}] = [2 c o s e c x]$

$= [\frac{2 \cos x}{\sin^{2} x}] = [\frac{2}{s i n x}]$

that implies that $\cos x = \sin x$ .

or $\tan x = 1$ or $x = \frac{π}{4}$

Hence we have solution $x = \frac{π}{4}$ .

Question 12: Solve the following equations: $\tan^{- 1} \frac{1 - x}{1 + x} = \frac{1}{2} \tan^{- 1} x, (x > 0)$

Answer:

Given equation is

$\tan^{- 1} \frac{1 - x}{1 + x} = \frac{1}{2} \tan^{- 1} x$ :

L.H.S can be written as;

$\tan^{- 1} \frac{1 - x}{1 + x} = \tan^{- 1} 1 - \tan^{- 1} x$

Using the formula $[\tan^{- 1} x - \tan^{- 1} y = \tan^{- 1} \frac{x - y}{1 + x y}]$

So, we have $\tan^{- 1} 1 - \tan^{- 1} x = \frac{1}{2} \tan^{- 1} x$

$\Rightarrow \tan^{- 1} 1 = \frac{3}{2} \tan^{- 1} x$

$\Rightarrow \frac{π}{4} = \frac{3}{2} \tan^{- 1} x$

$\Rightarrow \tan^{- 1} x = \frac{π}{6}$

$\Rightarrow x = \tan \frac{π}{6} = \frac{1}{\sqrt{3}}$

Hence the value of $x = \frac{1}{\sqrt{3}}$ .

Question 13: $\sin (\tan^{- 1} x), | x | < 1$ is equal to

(A) $\frac{x}{\sqrt{1 - x^{2}}}$

(B) $\frac{1}{\sqrt{1 - x^{2}}}$

(D) $\frac{x}{\sqrt{1 + x^{2}}}$

Answer:

Let $\tan^{- 1} x = y$ then we have;

$\tan y = x$ or

$y = \sin^{- 1} (\frac{x}{\sqrt{1 + x^{2}}}) \Rightarrow \tan^{- 1} x = \sin^{- 1} (\frac{x}{\sqrt{1 + x^{2}}})$

$\Rightarrow \sin (\tan^{- 1} x) = \sin (\sin^{- 1} (\frac{x}{\sqrt{1 + x^{2}}})) = \frac{x}{\sqrt{1 + x^{2}}}$

Hence, the correct answer is D.

Question 14: $\sin^{- 1} (1 - x) - 2 \sin^{- 1} x = \frac{π}{2}$ then $x$ is equal to

(A) $0, \frac{1}{2}$

(B) $1, \frac{1}{2}$

(D) $\frac{1}{2}$

Answer:

Given the equation: $\sin^{- 1} (1 - x) - 2 \sin^{- 1} x = \frac{π}{2}$

we can migrate the $\sin^{- 1} (1 - x)$ term to the R.H.S.

Then we have;

$- 2 \sin^{- 1} x = \frac{π}{2} - \sin^{- 1} (1 - x)$

or $- 2 \sin^{- 1} x = \cos^{- 1} (1 - x)$ ............................(1)

from $[∵ \cos^{- 1} (1 - x) + \sin^{- 1} (1 - x) = \frac{π}{2}]$

Take $\sin^{- 1} x = Θ$ $\Rightarrow \sin Θ = x$ or $\cos Θ = \sqrt{1 - x^{2}}$ .

So, we conclude that;

$\sin^{- 1} x = \cos^{- 1} (\sqrt{1 - x^{2}})$

Therefore we can put the value of $\sin^{- 1} x$ in equation (1) we get,

$- 2 \cos^{- 1} (\sqrt{1 - x^{2}}) = \cos^{- 1} (1 - x)$

Putting x = sin y in the above equation, we have then,

$\Rightarrow - 2 \cos^{- 1} (\sqrt{1 - (\sin y)^{2}}) = \cos^{- 1} (1 - \sin y)$

$\Rightarrow - 2 \cos^{- 1} (\sqrt{\cos^{2} y}) = \cos^{- 1} (1 - \sin y)$

$\Rightarrow - 2 \cos^{- 1} (\cos y) = \cos^{- 1} (1 - \sin y)$

$\Rightarrow \cos (- 2 y) = 1 - \sin y$

$\Rightarrow - 2 y = \cos^{- 1} (1 - \sin y)$

$\Rightarrow 1 - 2 \sin^{2} y = 1 - \sin y$

$\Rightarrow 2 \sin^{2} y - \sin y = 0$

$\Rightarrow \sin y (2 \sin y - 1) = 0$

So, we have the solution;

$\sin y = 0 o r \frac{1}{2}$ Therefore we have $x = 0 o r x = \frac{1}{2}$ .

When we have $x = \frac{1}{2}$ , we can see that :

$L . H . S . = \sin^{- 1} (1 - \frac{1}{2}) - 2 \sin^{- 1} \frac{1}{2} = - \sin^{- 1} \frac{1}{2} = - \frac{π}{6}$

So, it is not equal to the R.H.S. $- \frac{π}{6} \neq \frac{π}{2}$

Thus, we have only one solution, which is x = 0

Hence, the correct answer is (C).

Class 12 Maths NCERT Chapter 2: Extra Question

Question: If $3 \tan^{- 1} x + \cot^{- 1} x = π$ , then x equals to:

Solution:
Given That, $3 \tan^{- 1} x + \cot^{- 1} x = π$
$\begin{aligned} \Rightarrow 2 \tan^{- 1} x + \tan^{- 1} x + \cot^{- 1} x = π \\ \Rightarrow 2 \tan^{- 1} x = π - \frac{π}{2} [Since, \tan^{- 1} x + \cot^{- 1} x = \frac{π}{2}] \\ \Rightarrow \tan^{- 1} \frac{2 x}{1 - x^{2}} = \frac{π}{2} [Since, 2 \tan^{- 1} x = \tan^{- 1} \frac{2 x}{1 - x^{2}}] \end{aligned}$
$\Rightarrow \frac{2 x}{1 - x^{2}} = \tan \frac{π}{2}$
$\Rightarrow \frac{2 x}{1 - x^{2}} = \tan \frac{1}{0}$
Cross multiplying

$\begin{aligned} \Rightarrow 1 - x^{2} = 0 \\ \Rightarrow x^{2} = \pm 1 \end{aligned}$
Here, only $x = 1$ satisfies the given equation.
Note: By putting $x = - 1$ in the given equation, we get:

$\begin{aligned} 3 \tan^{- 1} (- 1) + \cot^{- 1} (- 1) = π \\ \Rightarrow 3 \tan^{- 1} [\tan (\frac{- π}{4})] + \cot^{- 1} [\cot (\frac{- π}{4})] = π \\ \Rightarrow 3 \tan^{- 1} [- \tan (\frac{π}{4})] + \cot^{- 1} [- \cot (\frac{π}{4})] = π \\ \Rightarrow 3 \tan^{- 1} [\tan (\frac{π}{4})] + π - \cot^{- 1} [\cot (\frac{π}{4})] = π \\ \Rightarrow - 3 \times \frac{π}{4} + π - \frac{π}{4} = π \\ \Rightarrow - π + π = π \\ \Rightarrow 0 \neq π \end{aligned}$
$∴$ x = -1 does not satisfy the given equation.

Hence, the correct answer is 1.

Inverse Trigonometric Functions Class 12 Chapter 2: Topics

Here is the list of important topics that are covered in Class 12 Chapter 2, Inverse Trigonometric Functions:

2.1 Introduction
2.2 Basic Concepts
2.3 Properties of Inverse Trigonometric Functions

JEE Main Highest Scoring Chapters & Topics

Just Study 40% Syllabus and Score upto 100%

Download EBook

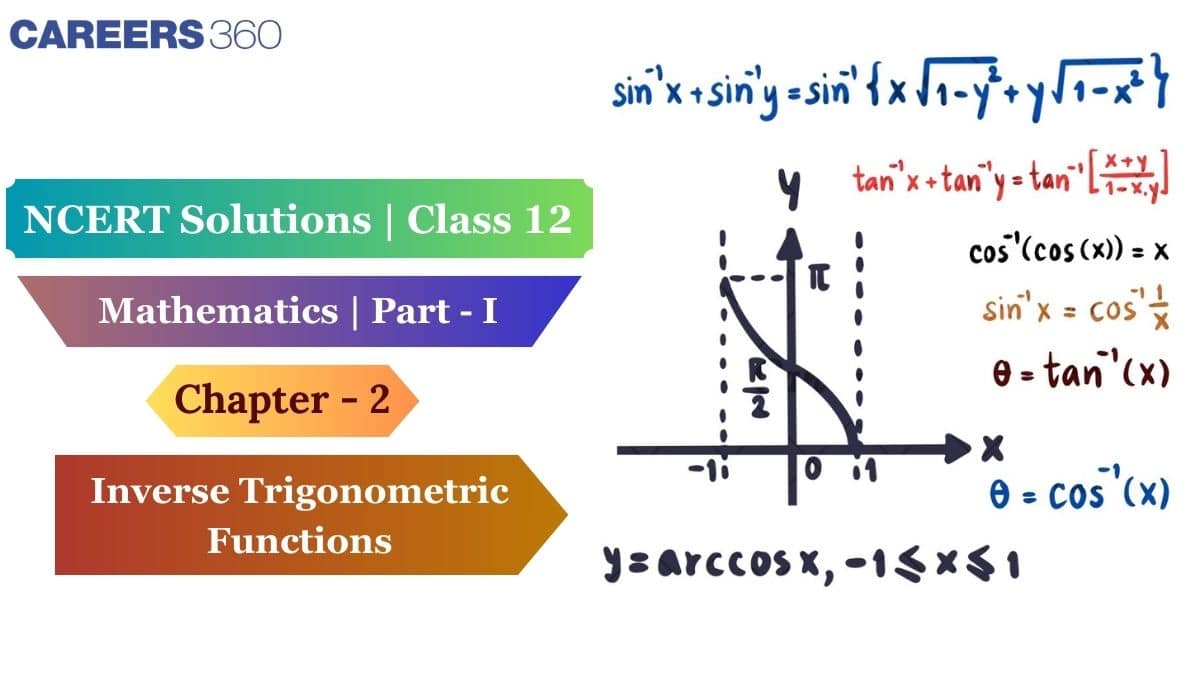
Inverse Trigonometric Functions Class 12 NCERT Solutions - Important Formulae

The inverse of the sine function: sin^-1(x) or arcsin(x) is defined on [-1, 1].

Properties of Inverse Trigonometric Functions:

Function	Domain	Range
y = sin^-1(x)	[-1, 1]	[-π/2, π/2]
y = cos^-1(x)	[-1, 1]	[0, π]
y = cosec^-1(x)	R - (-1, 1)	[-π/2, π/2] - {0}
y = sec^-1(x)	R - (-1, 1)	[0, π] - {π/2}
y = tan^-1(x)	R	(-π/2, π/2)
y = cot^-1(x)	R	(0, π)

Self-Adjusting Trigonometric Property:

sin(sin^-1(x)) = x

sin^-1(sin(x)) = x

cos(cos^-1(x)) = x

cos^-1(cos(x)) = x

tan(tan^-1(x)) = x

tan^-1(tan(x)) = x

sec(sec^-1(x)) = x

sec^-1(sec(x)) = x

cosec^-1(cosec(x)) = x

cosec(cosec^-1(x)) = x

cot^-1(cot(x)) = x

cot(cot^-1(x)) = x

Reciprocal Relations:

sin^-1(1/x) = cosec^-1(x), x ≥ 1 or x ≤ -1

cos^-1(1/x) = sec^-1(x), x ≥ 1 or x ≤ -1

tan^-1(1/x) = cot^-1(x), x > 0

Even and Odd Functions:

sin^-1(-x) = -sin^-1(x), x ∈ [-1, 1]

tan^-1(-x) = -tan^-1(x), x ∈ R

cosec^-1(-x) = -cosec^-1(x), |x| ≥ 1

cos^-1(-x) = π - cos^-1(x), x ∈ [-1, 1]

sec^-11(-x) = π - sec^-1(x), |x| ≥ 1

cot^-1(-x) = π - cot^-1(x), x ∈ R

Complementary Relations:

sin^-1(x) + cos^-1(x) = π/2

tan^-1(x) + cot^-1(x) = π/2

cosec^-1(x) + sec^-1(x) = π/2

Sum and Difference Formulas:

tan^-1(x) + tan^-1(y) = tan^-1((x+y)/(1-xy))

tan^-1(x) - tan^-1(y) = tan^-1((x-y)/(1+xy))

sin^-1(x) + sin^-1(y) = sin^-1[x√(1-y²)+y√(1-x²)]

sin^-1(x) - sin^-1(y) = sin^-1[x√(1-y²)-y√(1-x²)]

cos^-1(x) + cos^-1(y) = cos^-1[xy-√(1-x²)√(1-y²)]

cos^-1(x) - cos^-1(y) = cos^-1[xy+√(1-x²)√(1-y²)]

cot^-1(x) + cot^-1(y) = cot^-1((xy-1)/(x+y))

cot^-1(x) - cot^-1(y) = cot^-1((xy+1)/(y-x))

Double Angle Formula:

2 tan^-1(x) = sin^-1(2x/(1+x²))

2 tan^-1(x) = cos^-1((1-x²)/(1+x²))

2 tan^-1(x) = tan^-1(2x/(1-x²))

2 sin^-1(x) = sin^-1(2x√(1+x²))

2 cos^-1(x) = sin^-1(2x√(1-x²))

Conversion Properties:

sin^-1(x) = cos^-1(√(1-x²)) = tan^-1(x/√(1-x²)) = cot^-1(√(1-x²)/x)

cos^-1(x) = sin^-1(√(1-x²)) = tan^-1(√(1-x²)/x) = cot^-1(x/√(1-x²))

tan^-1(x) = sin^-1(x/√(1-x²)) = cos^-1(x/√(1+x²)) = sec^-1(√(1+x²)) = cosec^-1(√(1+x²)/x)

Approach to Solve Questions of Inverse Trigonometric Functions Class 12

Here are some approaches that students can follow to solve these problems smoothly.

Understand the domain and range: Learn the domain and range (Principal value branches) of inverse trigonometric functions efficiently to solve problems.
Graphical representation: Draw graphs of inverse trigonometric functions whenever you get stuck to visualise domains and ranges. It also helps to understand the behaviour of the function.
Focus on equations: Whenever you get an equation like $\sin^{- 1} (x) = θ$ , convert to $x = \sin (θ)$ to find exact values.
For identity based questions: Use basic identities such as $\sin^{- 1} x + \cos^{- 1} x = \frac{π}{2}, \tan^{- 1} x + \cot^{- 1} x = \frac{π}{2}$ , to solve composite expressions.
Avoid confusion about inverses: Do not confuse $\sin^{- 1} x$ with $(\sin x)^{- 1}$ . $(\sin x)^{- 1} = \frac{1}{\sin x}$ , which has a whole different meaning. Similarly, for other trigonometric functions, avoid the same confusion.
Shortcut tricks: For MCQ-based questions, put values like $x = \frac{1}{2}, 1, 0$ into options to check the answer and cancel out extreme options.

What Extra Should Students Study Beyond the NCERT for JEE?

Here is a comparison list of the concepts in Inverse Trigonometric Functions that are covered in JEE and NCERT, to help students understand what extra they need to study beyond the NCERT for JEE:

Concept Name	JEE	NCERT
Inverse Trigonometric Functions	✅	✅
Domain and Range of Trigonometric Functions	✅	✅
Graph of Inverse Trigonometric Function	✅	✅
Trigonometric Ratios of Complementary Angles	✅	❌
Sum and difference of angles in terms of arctan	✅	❌
Multiple Angles	✅	❌

NCERT Solutions for Class 12 Maths: Chapter Wise

Given below is the chapter-wise list of the NCERT Class 12 Maths solutions with their respective links:

NCERT Solutions for Class 12 Maths Chapter 1 Relations and Functions

NCERT solutions for class 12 maths chapter 2 Inverse Trigonometric Functions

NCERT solutions for class 12 maths chapter 3 Matrices

NCERT solutions for class 12 Maths Chapter 4 Determinants

NCERT solutions for class 12 maths chapter 5 Continuity and Differentiability

NCERT solutions for class 12 maths chapter 6 Application of Derivatives

NCERT solutions for class 12 maths chapter 7 Integrals

NCERT solutions for class 12 maths chapter 8 Application of Integrals

NCERT solutions for class 12 maths chapter 9 Differential Equations

NCERT solutions for class 12 maths chapter 10 Vector Algebra

NCERT solutions for class 12 maths chapter 11 Three Dimensional Geometry

NCERT solutions for class 12 Maths chapter 12 Linear Programming

NCERT solutions for class 12 maths chapter 13 Probability

NCERT Solutions class-wise

Given below are the links to class-wise NCERT solutions:

NCERT Books and NCERT Syllabus

Here are the links to NCERT Books and NCERT Syllabus:

Frequently Asked Questions (FAQs)

1. How to simplify inverse trigonometric expressions in Class 12 Maths?

Apply trigonometric identities to express inverse trigonometric functions in simpler forms. Also, use the principal values of the inverse trigonometric functions and convert them to algebraic form whenever necessary or use the substitution method to easily simplify inverse trigonometric expressions in Class 12 Maths.

2. What are the applications of inverse trigonometric functions in real life?

The main applications of inverse trigonometric functions in real life are:

Navigation: Inverse trigonometric functions are used in calculating angles of elevation and depression, as well as directions.
Engineering: Used in signal processing, wave analysis, and electrical engineering.
Physics: To calculate angles in problems involving vectors, motion, and forces.
Architecture: Helps in designing structures by calculating angles and slopes.

3. How to prove standard properties of inverse trigonometric functions?

To prove standard properties of inverse trigonometric functions, you can use definitions of Inverse Trigonometric Functions, Algebraic manipulations, Trigonometric Identities, Right Triangle approach, Graphs (for Principal Values), and the Substitution method.

4. What are the important topics covered in NCERT Solutions for Class 12 Maths Chapter 2?

The important topics covered in the NCERT Solutions for Class 12 Maths Chapter 2 are:

Domain and Range: The domain and range for each inverse trigonometric function are explained to help students understand the constraints on values
Graphical Representation: How to sketch the graphs of inverse trigonometric functions for better visual understanding.
Solving Equations: Techniques to solve equations involving inverse trigonometric functions.
Applications: Problems involving real-life applications, including trigonometric equations and angle calculations.

5. How many exercises are there in NCERT Class 12 Maths Chapter 2

There are 3 exercises in the NCERT class 12 maths chapter 2, they are:

Inverse Trigonometric Functions Class 12 Exercise 2.1
Inverse Trigonometric Functions Class 12 Exercise 2.2
Inverse Trigonometric Functions Class 12 Miscellaneous Exercise

Also Read

NCERT Solutions for Class 12 Maths Chapter 11 Three Dimensional Geometry

NCERT Solutions for Class 12 Physics Chapter 7 - Alternating Current

NCERT Solutions for Miscellaneous Exercise Chapter 7 Class 12 - Integrals

NCERT Solutions for Exercise 4.1 Class 11 Maths Chapter 4 - Principle of Mathematical Induction

Amines Class 12th Notes- Free NCERT Class 12 Chemistry Chapter 13 Notes- Download PDF

Aldehydes, Ketones and Carboxylic Acids Class 12th Notes- Free NCERT Class 12 Chemistry Chapter 12 Notes - Download PDF

Biomolecules class 12th notes- Free ncert class 12 Chemistry Chapter 14 Notes- Download PDF

Dual nature of radiation and matter Class 12th Notes - Free NCERT Class 12 Physics Chapter 11 Notes - Download PDF

Quadratic Equations Class 10th Notes - Free NCERT Class 10 Maths Chapter 4 Notes - Download PDF

NCERT Class 12th Maths Chapter 5 Continuity and Differentiability Notes

NCERT Book Class 12 Maths 2025-26 PDF Free Download

NCERT Book Class 12 Physics 2025-26 FREE PDF Download

NCERT Book Class 12 English 2025-26 Free PDF Download

NCERT Books for Class 12 Biology 2025-26: Download Free PDF

NCERT Books for Class 12 Hindi 2025-26; Download Chapter Wise PDF here

NCERT Books for Class 12 Chemistry 2025-26: Download Free PDF

NCERT Syllabus 2025-26 for Class 6-12 - Download All Subjects PDF Free

NCERT Exemplar Class 12 Physics Solutions Chapter 8 Electromagnetic Waves

Articles

NCERT solutions for Class 12 Physics Chapter 6 - Electromagnetic Induction

Jun 19, 2025

NCERT Solutions for Class 12 Physics Chapter 5 - Magnetism and Matter

Jun 19, 2025

NCERT Solutions for Class 12 Physics Chapter 4 - Moving Charges and Magnetism

Jun 19, 2025

NCERT Solutions for Class 12 Chemistry Chapter 8 Aldehydes, Ketones and Carboxylic Acids

Jun 19, 2025

TBSE 12th Question Papers 2023-24 - Download Tripura 12th Previous Year Question Paper PDF Here

Jun 19, 2025

NCERT solutions for Class 12 Chemistry Chapter 7 Alcohols, Phenols and Ethers

Jun 19, 2025

NCERT Solutions for Class 12 Maths Chapter 12 Linear Programming

Jun 19, 2025

NCERT Solutions for Class 12 Chemistry Chapter 6 Haloalkanes and Haloarenes

Jun 19, 2025

Upcoming School Exams

Bihar Open Board 10th Examination

Application Date:16 May,2025 - 30 June,2025

Result

Admit Card

Bihar Open Board 12th Examination

Application Date:16 May,2025 - 30 June,2025

Result

Admit Card

National Institute of Open Schooling 10th examination

Application Date:24 May,2025 - 23 June,2025

Result

Admit Card

Mock Test

National Institute of Open Schooling 12th Examination

Application Date:24 May,2025 - 23 June,2025

Result

Mock Test

Admit Card

Chattisgarh State Open School 12th Examination

Application Date:01 June,2025 - 30 June,2025

Result

Admit Card

View All School Exams

Certifications By Top Providers

Full-Stack Web Development

Via Masai School

Digital Banking Business Model

Via State Bank of India

Google Artificial Intelligence for JavaScript Developers with TensorFlow JavaScript

Via Google

Introduction to Psychology

Via San Jose State University, San Jose

Banking 101 A Guide for Beginners in the Banking Sector

Via EduBridge

Forensic Science DNA Analysis

Via University of Cambridge, Cambridge

1114 courses

818 courses

394 courses

294 courses

Harvard University, Cambridge

98 courses

University of Michigan, Ann Arbor

84 courses

IT & Software

Teaching and Academics

Programming and Development

Health, Fitness and Medicine

Business and Management

Engineering and Architecture

General Management

Financial Management

Environmental Studies

History

Psychology

Artificial Intelligence

Explore Top Universities Across Globe

University of Essex, Colchester

Wivenhoe Park Colchester CO4 3SQ

University College London, London

Gower Street, London, WC1E 6BT

The University of Edinburgh, Edinburgh

Old College, South Bridge, Edinburgh, Post Code EH8 9YL

University of Delaware, Newark

Newark, DE 19716

University of Nottingham, Nottingham

University Park, Nottingham NG7 2RD

Bristol Baptist College, Bristol

The Promenade, Clifton Down, Bristol BS8 3NJ

PTE Books for Exam Preparation 2025: Best Study Material and Resources

12 min ⋆ Jun 19, 2025 12:06 PM IST

Why Do Indian Students Go To Iran For Studies?

6 min ⋆ Jun 19, 2025 12:06 PM IST

PTE Test Day Tips 2025: Requirements, Tricks, Checklist

9 min ⋆ Jun 19, 2025 12:06 PM IST

SAT Exam Syllabus 2025 - Section Wise Pattern & Syllabus

9 min ⋆ Jun 19, 2025 05:06 AM IST

GMAT Cut Off for Top MBA Colleges Abroad 2025 - Check Top Universities Score

8 min ⋆ Jan 23, 2025 03:01 AM IST

Present Perfect Continuous Tense: Definition, Types, Structure, Rules & Examples

8 min ⋆ Jun 19, 2025 11:06 AM IST

Related E-books & Sample Papers

CBSE Class 12 English: Previous Year Question Papers

41+ Downloads

CBSE Class 12 Hindi: Previous Year Question Papers

23+ Downloads

CBSE Class 12 History: Previous Year Question Papers

4+ Downloads

CBSE Class 12 Economics: Previous Year Question Papers

21+ Downloads

CBSE Class 12 Geography: Previous Year Question Papers

2+ Downloads

CBSE Class 12 Biology: Previous Year Question Papers

22+ Downloads

Questions related to CBSE Class 12th

Have a question related to CBSE Class 12th ?

Can I change my board from CBSE to CHSE Odisha in Class 12th?

Changing from the CBSE board to the Odisha CHSE in Class 12 is generally difficult and often not ideal due to differences in syllabi and examination structures. Most boards, including Odisha CHSE , do not recommend switching in the final year of schooling. It is crucial to consult both CBSE and Odisha CHSE authorities for specific policies, but making such a change earlier is advisable to prevent academic complications.

Read Complete Answer

Manasvini Gupta 30 January,2025

quartely question paper for mathematics cbse

Hello there! Thanks for reaching out to us at Careers360.

Ah, you're looking for CBSE quarterly question papers for mathematics, right? Those can be super helpful for exam prep.

Unfortunately, CBSE doesn't officially release quarterly papers - they mainly put out sample papers and previous years' board exam papers. But don't worry, there are still some good options to help you practice!

Have you checked out the CBSE sample papers on their official website? Those are usually pretty close to the actual exam format. You could also look into previous years' board exam papers - they're great for getting a feel for the types of questions that might come up.

If you're after more practice material, some textbook publishers release their own mock papers which can be useful too.

Let me know if you need any other tips for your math prep. Good luck with your studies!

Read Complete Answer

Jefferson 25 September,2024

i have taken admission in clg after my campartment exam in chemistry along with the neet 2025 preperation but unfortunately i again got campartment after doing all this preparation i got 15 marks only now I can't able to think what should I do next.

It's understandable to feel disheartened after facing a compartment exam, especially when you've invested significant effort. However, it's important to remember that setbacks are a part of life, and they can be opportunities for growth.

Possible steps:

Re-evaluate Your Study Strategies:
- Identify Weak Areas: Pinpoint the specific topics or concepts that caused difficulties.
- Seek Clarification: Reach out to teachers, tutors, or online resources for additional explanations.
- Practice Regularly: Consistent practice is key to mastering chemistry.
Consider Professional Help:
- Tutoring: A tutor can provide personalized guidance and support.
- Counseling: If you're feeling overwhelmed or unsure about your path, counseling can help.
Explore Alternative Options:
- Retake the Exam: If you're confident in your ability to improve, consider retaking the chemistry compartment exam.
- Change Course: If you're not interested in pursuing chemistry further, explore other academic options that align with your interests.
Focus on NEET 2025 Preparation:
- Stay Dedicated: Continue your NEET preparation with renewed determination.
- Utilize Resources: Make use of study materials, online courses, and mock tests.
Seek Support:
- Talk to Friends and Family: Sharing your feelings can provide comfort and encouragement.
- Join Study Groups: Collaborating with peers can create a supportive learning environment.

Remember: This is a temporary setback. With the right approach and perseverance, you can overcome this challenge and achieve your goals.

I hope this information helps you.

Read Complete Answer

Kanishka kaushikii 17 September,2024

i scored 84 percent in cbse 2 years ago.Am i eligible for medhavi scholarship if give 12th again after 2 drop years and will got 75+ in mpboard

Hi,

Qualifications:
Age: As of the last registration date, you must be between the ages of 16 and 40.
Qualification: You must have graduated from an accredited board or at least passed the tenth grade. Higher qualifications are also accepted, such as a diploma, postgraduate degree, graduation, or 11th or 12th grade.
How to Apply:
Get the Medhavi app by visiting the Google Play Store.
Register: In the app, create an account.
Examine Notification: Examine the comprehensive notification on the scholarship examination.
Sign up to Take the Test: Finish the app's registration process.
Examine: The Medhavi app allows you to take the exam from the comfort of your home.
Get Results: In just two days, the results are made public.
Verification of Documents: Provide the required paperwork and bank account information for validation.
Get Scholarship: Following a successful verification process, the scholarship will be given. You need to have at least passed the 10th grade/matriculation scholarship amount will be transferred directly to your bank account.

Scholarship Details:

Type A: For candidates scoring 60% or above in the exam.

Type B: For candidates scoring between 50% and 60%.

Type C: For candidates scoring between 40% and 50%.

Cash Scholarship:

Scholarships can range from Rs. 2,000 to Rs. 18,000 per month, depending on the marks obtained and the type of scholarship exam (SAKSHAM, SWABHIMAN, SAMADHAN, etc.).

Since you already have a 12th grade qualification with 84%, you meet the qualification criteria and are eligible to apply for the Medhavi Scholarship exam. Make sure to prepare well for the exam to maximize your chances of receiving a higher scholarship.

Hope you find this useful!

Read Complete Answer

Yuvan 30 August,2024

i upload 12th board result screenshot in ncweb form from the cbse site so it is valid

hello mahima,

If you have uploaded screenshot of your 12th board result taken from CBSE official website,there won,t be a problem with that.If the screenshot that you have uploaded is clear and legible. It should display your name, roll number, marks obtained, and any other relevant details in a readable forma.ALSO, the screenshot clearly show it is from the official CBSE results portal.

hope this helps.

Read Complete Answer

Ashvadha 12 July,2024

View All

Stay up-to date with CBSE Class 12th News

Ask

Question

Student Community: Where Questions Find Answers

Ask and get expert answers on exams, counselling, admissions, careers, and study options.

Download Careers360 App

All this at the convenience of your phone

Regular Exam Updates
Best College Recommendations
College & Rank predictors
Detailed Books and Sample Papers
Question and Answers

Scan and download the app

Central Board of Secondary Education Class 12th Examination

JEE Test Series

Applications Open

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

Option 1) less than 3	Option 2) more than 3 but less than 6
Option 3) more than 6 but less than 9	Option 4) more than 9

Exams

Top Schools

Products & Resources

NCERT Study Material

Exams

Colleges

Predictors

Resources

Exams

Colleges & Courses

Predictors

Resources

Exams

Colleges

Predictors

Resources

Exams

Colleges

Predictors & E-Books

Resources

Exams

Predictors & Articles

Colleges

Resources

Exams

Colleges

Resources

Exams

Resources

Top Courses & Careers

Colleges

Exams

Colleges

Resources

Quick Links

Exams

Colleges

Resources

Exams

Colleges

Resources

Diploma Colleges

Exams

Resources

Upcoming Events

Other Exams

Exams

Colleges

Top Countries

Resources

Exams

Colleges

Upcoming Events

Resources

Engineering Preparation

Medical Preparation

Law Preparation

MBA Preparation

Top Streams

Specializations

Resources

Top Providers

NCERT Solutions for Class 12 Maths Chapter 2 Inverse Trigonometric Functions

NCERT Solution for Class 12 Maths Chapter 2 Solutions: Download PDF

NCERT Solutions for Class 12 Maths Chapter 2: Exercise Questions

Aakash Repeater Courses

Class 12 Maths NCERT Chapter 2: Extra Question

Inverse Trigonometric Functions Class 12 Chapter 2: Topics

Inverse Trigonometric Functions Class 12 NCERT Solutions - Important Formulae

Properties of Inverse Trigonometric Functions:

Self-Adjusting Trigonometric Property:

Reciprocal Relations:

Even and Odd Functions:

Complementary Relations:

Sum and Difference Formulas:

Double Angle Formula:

Conversion Properties:

Approach to Solve Questions of Inverse Trigonometric Functions Class 12

What Extra Should Students Study Beyond the NCERT for JEE?

NCERT Solutions for Class 12 Maths: Chapter Wise