NCERT Solutions for Class 12 Physics Chapter 1 - Electric Charges and Fields
CBSE Class 12th Exam Date:17 Feb' 26 - 17 Feb' 26
Have you ever noticed your hair standing up after rubbing a balloon on it? How about seeing dust cling to a television screen? These are some common examples demonstrating the behaviour of electric charges, interacting with invisible small particles that either attract or repel each other. NCERT Solutions Class 12 Ch 1 is a complete and handy source of understanding. In this chapter, the concepts of Coulomb law, electric fields and Gaussian electric flux and Gauss law are discussed.
This Story also Contains
- NCERT Solutions for Class 12 Physics Chapter 1 Electric Charges And Fields
- Electric Charges And Fields Class 12 NCERT Solutions: Exercise Questions
- NCERT Solution for Class 12 Chapter 1: Additional Questions
- Electric Charges And Fields Class 12 NCERT Solutions: Topics And Subtopics
- Electric Charges And Fields Class 12 NCERT Solutions: Important Formulas
- NCERT Solution for Class 12 Chapter 1: Importance in Board Exams
- What Extra Should Students Study Beyond NCERT for JEE/NEET?
- NCERT Solutions for Class 12 Physics: Chapter Wise

The NCERT solutions for class 12 physics are stepwise, such that the complexity of the topics is simplified to enable the students to have a clear understanding of the fundamentals of electrostatics. The solutions for excelling in the CBSE board exams are very useful not only in excelling in the same but also in preparing for competitive entrance examinations such as JEE and NEET. Through these explanations, the students will be able to reinforce their conceptual knowledge and develop a strong foundation in physics to study at advanced levels.
Also, Read -
NCERT Solutions for Class 12 Physics Chapter 1 Electric Charges And Fields
Download the Class 12 Physics Chapter 1 Exercise Solutions PDF for free and prepare for your CBSE exams. These NCERT solutions cover important topics like the electric field, Coulomb’s law, and Gauss’s law for easy revision.
Electric Charges And Fields Class 12 NCERT Solutions: Exercise Questions
Electric Charges and Fields Class 12 NCERT Solutions offer a solution to each question in the exercises of the chapter and allow students to gain a robust knowledge of electrostatics. Important concepts such as Coulomb law, electric field, electric flux, and Gauss theorem are explained with the right words in these solutions, hence preparing to undertake exams easily.
Answer:
Given,
$q_{1}$ = $2 \times 10^{-7}$ C
$q_{2}$ = $3 \times 10^{-7}C$
r = 30 cm = 0.3 m
We know,
The force between two charged particles, $q_{1}$ and $q_{2}$ separated by a distance r.
$F = \frac{1}{4\pi \epsilon _{0}} \frac{q_{1}q_{2}}{r^2}$
$ \Rightarrow F= \frac{1}{4\pi \epsilon _{0}} \frac{2\times10^{-7} \times 3 \times 10^{-7}}{(30\times10^{-2}\ m)^2\ }$
$\Rightarrow F= (9\times10^9\ N)\times \frac{6\times10^{-14+4}}{900\ m^2\ } = 6\times10^{-3} N$
Since the charges are of the same nature, the force is repulsive.
Answer:
Given,
$q_{1}$ = $0.4 \mu C$
$q_{2}$ = $-0.8 \mu C$
F = $-0.2N$ (Attractive)
We know,
The force between two charged particles, $q_{1}$ and $q_{2}$ separated by a distance r.
$F = \frac{1}{4\pi \epsilon _{0}} \frac{q_{1}q_{2}}{r^2}$
$\Rightarrow \\ -0.2 = 9\times10^9 \times \frac{(0.4\times10^{-6})(-0.8\times10^{-6})}{r^2} \\$
$\implies r^2 = 9\times10^9\times0.32\times10^{-12}/0.2 \\$
$\implies r^2 = 9\times0.16\times10^{-2} \\$
$\implies r = 1.2\times10^{-1} m = 0.12 m =12 cm$
Therefore, the distance between the two charged spheres is 12 cm.
Answer:
Using Newton's third law, the force exerted by the spheres on each other will be equal in magnitude.
Therefore, the force on the second sphere due to the first = 0.2 N
(This force will be attractive since charges are of opposite sign.)
Answer:
Electrostatic force
$F=\frac{KQ^2}{r^2}$
So the dimension of
$[Ke^2]=[Fr^2]$ ..................(1)
The gravitational force between two bodies of mass M and m is
$F=\frac{GMm}{r^2}$
So, the dimension of
$[Gm_em_p]=[Fr^2]$ .............(2)
Therefore, from (1) and (2)
$[\frac{ke^{2}}{Gm_{e}m_{p}} ]$ is dimensionless
or
Here,
K = $1/4\pi \epsilon _{0}$ , where $\epsilon _{0}$ is the permittivity of space.
$[1/ \epsilon _{0}] =[C/V.m] = [Nm^2 C^{-2}]$
e = Electric charge ([e] = [C])
G = Gravitational constant. ([G]= $[Nm^2kg^{-2}]$ )
$m_{e}$ and $m_{p}$ are mass of electron and proton ([ $m_{e}$ ] = [ $m_{p}$ ] = [Kg])
Substituting these units, we get
$[\frac{ke^{2}}{Gm_{e}m_{p}} ] = [\frac{C^2 \times Nm^2C^{-2}}{Nm^2kg^{-2}\times kg\times kg} ] = M^{0}L^{0}T^{0}$
Hence, this ratio is dimensionless.
Putting the value of the constants
$\frac{ke^{2}}{Gm_{e}m_{p}} = \frac{9\times10^9\times(1.6\times10^{-19})^{2}}{6.67\times10^{11}\times9.1\times10^{-31}\times1.67\times10^{-27}}=2.3 \times 10^{40}$
The given ratio is the ratio of the electric force $\frac{ke^{2}}{R^2}$ to the gravitational force between an electron and a proton $\frac{Gm_{e}m_{p}}{R^2}$, considering the distance between them is constant!
Q 1.4 (a) Explain the meaning of the statement ‘electric charge of a body is quantised’.
Answer:
The given statement "electric charge of a body is quantised" implies that the charge on a body can take only integral values. In other words, only an integral number of electrons can be transferred from one body to another and not in fractions.
Therefore, a charged body can only have an integral multiple of the electric charge of an electron.
Answer:
On a macroscopic level, the amount of charge transferred is very large compared to the charge of a single electron. Therefore, we tend to ignore the quantisation of electric charge in these cases and consider it to be continuous in nature.
Answer:
When a glass rod is rubbed with a silk cloth, opposite charges appear on both the rod and the cloth.
The phenomenon of charging bodies by rubbing them against each other is known as charging by friction. Here, electrons are transferred from one body to another, giving both bodies an equal but opposite charge. The number of electrons lost by one body (attains a positive charge due to loss of negatively charged electrons) is equal to the number of electrons gained by the other body (attains a negative charge). Therefore, the net charge of the system is zero. This is in accordance with the law of conservation of charge
Answer:
Charges at (A, C) and (B, D) are pairwise diametrically opposite and also equal.
Therefore, their force on a point charge at the centre of the square will be equal but opposite in direction.
Now, AC = BD = $\sqrt{2}\times(10\times10^{-2} m) = \sqrt{2}\times0.1 m$
$\therefore$ AO = BO = CO = DO = r = Half of diagonal = $\sqrt{2}\times0.05 m$
Force on point charge at centre due to charges at A and C = $F_{A} = -F_{C} = \frac{k(2\mu C)(1\mu C)}{(r)^2}$
Similarly, force on point charge at centre due to charges at B and D = $F_{B} = -F_{D} = \frac{k(-5\mu C)(1\mu C)}{(r)^2}$
$\therefore$ Net force on point charge = $\\F_{A} + F_{B} + F_{C} + F_{D} = -F_{B} +F_{B} - F_{D} + F_{D} = 0$ .
Hence, the charge at the centre experiences no force.
Answer:
A positive point charge experiences a force in an electrostatic field. Since the charge will experience a continuous force and cannot jump from one point to another, the electric field lines must be continuous.
Q 1.7 (b) Explain why two field lines never cross each other at any point.
Answer:
A tangent drawn at any point on a field line gives the direction of force experienced by a unit positive charge due to the electric field at that point. If two lines intersect at a point, then the tangent drawn there will give two directions of force, which is not possible. Hence, two field lines cannot cross each other at any point.
Answer:
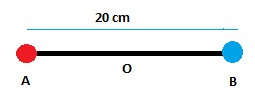
Given, AB = 20 cm
Since O is the midpoint of the line AB.
AO = OB = 10 cm = 0.1m
The electric field at a point caused by charge q is given as,
$E = \frac{kq}{r^2}$
Where q is the charge, r is the distance between the charges and the point O
k = 9x109 N m2 C -2
Now,
Due to the charge at A, the electric field at O will be $E_{A}$ and in the direction AO.
$E_{A} =\frac{ 9\times10^9 \times 3\times10^{-6}}{0.1^2}$
Similarly, the electric field at O due to the charge at B, also in the direction AO
$E_{B} =\frac{ 9\times10^9 \times (-3\times10^{-6})}{0.1^2}$
Since both the forces are acting in the same direction, we can add their magnitudes to get the net electric field at O:
E' = $E_{A}$ + $E_{B}$ = 2E (Since their magnitudes are same)
$E' =2\times \frac{ 9\times10^9 \times 3\times10^{-6}}{0.1^2} = 5.4\times 10^4 N/C$ along the direction AO.
Answer:
Let Q = $-1.5 \times10^{-9}$ C
The force experienced by Q when placed at O due to the charges at A and B will be:
$F = Q \times E$
where 'E' is the net electric field at point O.
$F=1.5 \times 10^{-9} C \times 5.4 \times 10^4 N/C = 8.1\times10^{-3 } N$
Q, being negatively charged, will be attracted by the positive charge at A and repelled by the negative charge at B. Hence, the direction of force experienced by it will be in the direction of OA.
Answer:
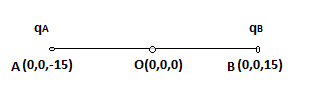
Given,
$q_{A}=2.5\times 10^{-7}C$ and $q_{B}=-2.5\times 10^{-7}$
The total charge of the system = $q_{A} + q_{B} = 0$
$\therefore$ The system is electrically neutral. (All dipole systems have net charge zero!)
Now, distance between the two charges, d = 15 + 15 = 30 cm = 0.3 m
We know, the electric dipole moment of the system, p = $q_{A}$ x d = $q_{B}$ x d (i.e, the magnitude of charge x distance between the two charges)
$\therefore p = 2.5\times10^{-7} C \times 0.3 m = 7.5\times10^{-8} Cm$
The direction of a dipole is towards the positive charge. Hence, in the positive z-direction.
Answer:
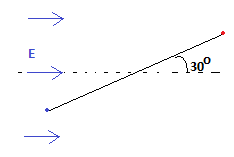
Given,
Electric dipole moment, p = $4\times 10^{-9} Cm$
$\theta = 30^{0} \ \therefore sin\theta = 0.5$
E = $5\times 10^{4}NC^{-1}$
We know, the torque acting on a dipole is given by:
$\tau = p \times E$
$\implies \tau = p Esin\theta = 4\times10^{-9} \times5\times10^{4}\times0.5 \ Nm$
$\implies \tau =10^{-4}Nm$
Therefore, the magnitude of torque acting on the dipole is $10^{-4}Nm$
Answer:
Clearly, polyethene being negatively charged implies that it has an excess of electrons(which are negatively charged!). Therefore, electrons were transferred from wool to polyethene.
Given, charge attained by polyethene = $-3\times 10^{-7}$ C
We know, Charge on 1 electron = $-1.6\times10^{-19} C$
Therefore, the number of electrons transferred to attain a charge of $-3\times 10^{-7} =\frac{-3\times 10^{-7}}{-1.6\times10^{-19} C} = 1.8\times10^{12}$ electrons.
Answer:
The charge attained by polyethene (and also wool!) is solely due to the transfer of free electrons.
We know, Mass of an electron = $9.1\times10^{-31}\ kg$
The total mass of electrons transferred = number of electrons transferred x mass of an electron
= $9.1\times10^{-31} \times 1.8\times10^{12}\ kg = 16.4\times10^{-19} \ kg$
Yes, there is a transfer of mass, but it is negligible.
Answer:
Since the radii of the spheres A and B are negligible compared to the distance of separation, we consider them as a point object.
Given,
charge on each of the spheres = $6.5\times 10^{-7}C$
and distance between them, r = 50 cm = 0.5 m
We know,
$F = k\frac{q_{1}q_{2}}{r^2}$
Therefore, the mutual force of electrostatic repulsion(since they have the same sign of charge)
$F = 9\times10^9Nm^{2}C^{-2}\times\frac{(6.5\times10^{-7}\ C)^2}{(0.5\ m )^2} = 1.5\times10^{-2} N$
Answer:
We know, the force between two charged particles separated by a distance r is:
$F = k\frac{q_{1}q_{2}}{r^2} = k\frac{q^2}{r^2}$ $(\because q_{1} = q_{2} = q)$
Now if $q\rightarrow 2q\ and\ r\rightarrow r/2$
The new value of force:
$F_{new} =k\frac{(2q)^2}{(r/2)^2} = 16k\frac{q^2}{r^2} = 16F$
Therefore, the force increases 16 times!
$F_{new} =16F = 16\times1.5\times10^{-2} N = 0.24\ N$
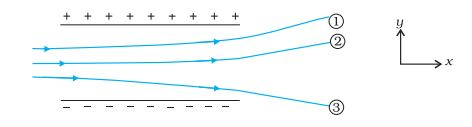
Answer:
Charges 1 and 2 are repelled by the negatively charged plate of the system
Hence, 1 and 2 are negatively charged.
Similarly, 3 being repelled by the positive plate is positively charged.
(charge to the mass ratio: charge per unit mass)
Since 3 is deflected the most, it has the highest charge-to-mass ratio.
Answer:
Given,
$E=3\times 10^{3}\ \widehat{i}\ \frac{N}{C}$
Area of the square = $0.01^2\ m^2$
Since the square is parallel to the yz plane, its normal is in the x-direction.(i.e $\widehat{i}$ direction )
Therefore, flux through this surface:
$\phi = E.A$
$\implies \phi = (3\times10^3\ \widehat{i}).(0.01\ \widehat{i}) Nm^2/C = \boldsymbol{30 Nm^2/C}$
Answer:
Now, since the normal of the square plane makes a $60^{\circ}$ angle with the x-axis
$cos\Theta = cos(60^{0}) = 0.5$
Therefore, flux through this surface:
$\phi = E.A = EAcos\theta$
$\implies \phi = (3\times10^3)(0.01)(0.5) Nm^2/C = \boldsymbol{15\ Nm^2/C}$
Answer:
The net flux of the uniform electric field through a cube oriented so that its faces are parallel to the coordinate planes is zero.
This is because the number of lines entering the cube is the same as the number of lines leaving the cube.
Alternatively,
Using Gauss’s law, we know that the flux of the electric field through any closed surface S is $1/\epsilon _{0}$ times the total charge enclosed by S.
i.e. $\phi = q/\epsilon _{0}$
where, q = net charge enclosed and $\epsilon _{0}$ = permittivity of free space (constant)
Since there is no charge enclosed in the cube, $\phi = 0$.
Answer:
Using Gauss’s law, we know that the flux of the electric field through any closed surface S is $1/\epsilon _{0}$ times the total charge enclosed by S.
i.e. $\phi = q/\epsilon _{0}$
where, q = net charge enclosed and $\epsilon _{0}$ = permittivity of free space (constant)
Given, $\phi = 8.0 \times10^3\ Nm^2/C$
$\therefore q = \phi \times\epsilon _{0} = (8.0\times10^3 \times8.85\times10^{-12})\ C$
$\implies q = 70 \times10^{-9} C = \boldsymbol{0.07 \mu C}$
This is the net charge inside the box.
Answer:
Using Gauss's law, we know that $\phi = q/\epsilon _{0}$
Since flux is zero, q = 0, but this q is the net charge enclosed by the surface.
Hence, we can conclusively say that the net charge is zero, but we cannot conclude that there are no charges inside the box.

Answer:
Let us assume that the charge is at the centre of the cube with an edge of 10 cm.
Using Gauss's law, we know that the flux of the electric field through any closed surface S is $1/\epsilon _{0}$ times the total charge enclosed by S.
i.e. $\phi = q/\epsilon _{0}$
where, q = net charge enclosed and $\epsilon _{0}$ = permittivity of free space (constant)
Therefore, flux through the cube: $\phi = (10\times10^{-6} C)/\epsilon _{0}$
Due to symmetry, we can conclude that the flux through each side of the cube, $\phi'$, will be equal.
$\therefore \phi' = \frac{\phi}{6} = \frac{10^{-5}}{6\times\epsilon _{0}} = \frac{10^{-5}}{6\times\ 8.85\times10^{-12}} = \boldsymbol{1.9 \times 10^5\ Nm^2C^{-1}}$
Answer:
Given,
q = net charge inside the cube = $2.0\mu C$
Using Gauss’s law, we know that the flux of the electric field through any closed surface S is $1/\epsilon _{0}$ times the total charge enclosed by S.
i.e. $\phi = q/\epsilon _{0}$
where, q = net charge enclosed and $\epsilon _{0}$ = permittivity of free space (constant)
$\therefore \phi = 2.0\times10^{-6}/8.85\times10^{-12}\ Nm^2C^{-1} = \boldsymbol{2.2\times10^5\ Nm^2C^{-1}}$
(Note: Using Gauss's formula, we see that the electric flux through the cube is independent of the position of the charge and the dimension of the cube! )
Answer:
Given,
$\phi = -1.0\times 10^{3}\frac{Nm^{2}}{C}$
Using Gauss’s law, we know that the flux of the electric field through any closed surface S is $1/\epsilon _{0}$ times the total charge enclosed by S.
i.e. $\phi = q/\epsilon _{0}$
where, q = net charge enclosed and $\epsilon _{0}$ = permittivity of free space (constant)
Therefore, flux does not depend on the radius of the sphere but only on the net charge enclosed. Hence, the flux remains the same although the radius is doubled.
$\phi' = -10^{3}\frac{Nm^{2}}{C}$
Answer:
Given,
$\phi = -1.0\times 10^{3}\frac{Nm^{2}}{C}$
Using Gauss’s law, we know that the flux of the electric field through any closed surface S is $1/\epsilon _{0}$ times the total charge enclosed by S.
i.e. $\phi = q/\epsilon _{0}$
where, q = net charge enclosed and $\epsilon _{0}$ = permittivity of free space (constant)
$\therefore q = -10^{3}Nm^2C^{-1} \times 8.85\times10^{-12}N^{-1}m^{-2}C^{2} = -8.8\times10^{-9}\ C \\ = \boldsymbol{-8.8\ nC}$
Answer:
We know that, for determining the electric field at r>R for a conducting sphere, the sphere can be considered as a point charge located at its centre.
Also, the electric field intensity at a point P, located at a distance r, due to the net charge q is given by,
$E = k\frac{q}{r^2}$
Given, r = 20 cm = 0.2 m (From the centre, not from the surface!)
$\\ \therefore 1.5\times10^3 = 9\times10^9\times \frac{q}{0.2^2} \\ \implies q = \frac{1.5\times0.04}{9}\times10^{-6} = 6.67\times10^{-9}\ C$
Therefore, charge on the conducting sphere is $- 6.67\ nC$ (since flux is inwards)
Answer:
Given,
Surface charge density = $80.0\mu Cm^{-2}$
Diameter of sphere = 2.4 m $\therefore$ radius of sphere, r = 1.2 m
The charge on the sphere, Q= surface charge density x surface area of the sphere
$= (80\times10^{-6})\times(4\pi r^2) = 320\times22/7\times(1.2)^2 = \boldsymbol{1.45\times10^{-3}\ C}$
Answer:
Using Gauss's law, we know that :
$\phi = q/ \epsilon_{0}$
$\implies \phi = 1.45\times10^{-3}/ 8.85\times10^{-12} = 1.6\times10^{8}\ Nm^2C^{-1}$
Answer:
Given,
$\lambda = 9\times 10^{4}\frac{N}{C}$
d = 2 cm = 0.02 m
We know, for an infinite line charge having linear charge density $\lambda$, the electric field at a distance d is:
$E = k\lambda / d$
$\therefore 9\times10^4 = 9\times10^{9}\lambda / 0.02$
The linear charge density is $10 \mu C/cm$.
Answer:
We know, electric field, E, due to an infinite plate (length>>thickness) having surface charge density $\sigma$ = $\sigma/2\epsilon_{0}$.
(To note: It's independent of distance from the plate!)
In the region outside the first plate,
since both plates have the same surface charge density(in magnitude only), their electric fields are the same in magnitude in this region but opposite in direction.
(E due to positive plate away from it and E due to negative plate towards it!)
Hence, the electric field in the outer region of the first plate is zero.
Answer:
We know, the electric field, E, due to an infinite plate (length>>thickness) having surface charge density $\sigma$ = $\sigma/2\epsilon_{0}$ .
(To note: It's independent of distance from the plate and the same everywhere!)
In the region outside the second plate,
Since both plates have the same surface charge density(in magnitude only), their electric fields are the same in magnitude in this region but opposite in direction.
(E due to positive plate away from it and E due to negative plate towards it!)
Hence, the electric field in the outer region of the second plate is zero.
Answer:
We know, the electric field, E, due to an infinite plate (length>>thickness) having surface charge density $\sigma$ = $\sigma/\epsilon_{0}$ .
(To note: It's independent of distance from the plate!)
Let A and B be the two plates such that:
$\sigma_{A}= 17\times10^{-22} Cm^{-2}$ = $\sigma$
$\sigma_{B}= -17\times10^{-22} Cm^{-2}$ = - $\sigma$
Therefore,
The electric field between the plates, E = $E_{A} + E_{B}$ = $\sigma_{A}/2\epsilon_{0} + (-\sigma_{B}/2\epsilon_{0})$
$\Rightarrow E = \sigma/2\epsilon_{0} = 17\times10^{-22}/8.85\times10^{-12} = 1.92\times10^{-10 } NC^{-1}$
NCERT Solution for Class 12 Chapter 1: Additional Questions
Answer:
When two spheres of the same size are touched, on attaining the equipotential state, the total charge of the system is equally distributed on both of them.
Therefore, (i) When uncharged third sphere C is touched with A, charge left on A = $0.5\times 6.5\times 10^{-7}C$
and charge attained by C = $0.5\times 6.5\times 10^{-7}C$
(ii) Now, charge on B + charge on C = $6.5\times 10^{-7}C$ + $0.5\times 6.5\times 10^{-7}C$ = $1.5\times 6.5\times 10^{-7}C$
When touched, charge left on B = $0.5\times1.5\times 6.5\times 10^{-7}C$
Therefore $q_{A}\rightarrow 0.5\times q_{A}\ and\ q_{B}\rightarrow 0.75\times q_{B}$
Therefore,
$F' = 0.5\times0.75 \times F = 0.375 \times 1.5\times10^{-2} N = \boldsymbol{5.7\times10^{-3}\ N}$
Answer:
The force due to the electric field is balancing the weight of the oil droplet.
weight of the oil drop = density x volume of the droplet x g = $\rho \times \frac{4}{3}\pi r^3 \times g$
Force due to the electric field = E x q
charge on the droplet, q = No. of excess electrons x charge of an electron = $12\times q_{e} = 12\times 1.6\times10^{-19} = 1.92\times10^{-18} C$
Balancing forces:
$\rho \times \frac{4}{3}\pi r^3 \times g = E\times q$
Putting known and calculated values:
$\\ r^3 =\frac{3}{4\pi} E\times q / \rho\times g $
$ \Rightarrow r^3 = \frac{3}{4}\times\frac{7}{22}\times(2.55\times10^4)\times 1.92\times10^{-18} / (1.26\times10^3 \times10 ) $
$ \Rightarrow r^3 = 0.927\times10^{-18} m^3$
$r = 0.975\times10^{-6} m = 9.75\times10^{-4} mm$
Q 3. Which among the curves shown in Figure cannot possibly represent electrostatic field lines?

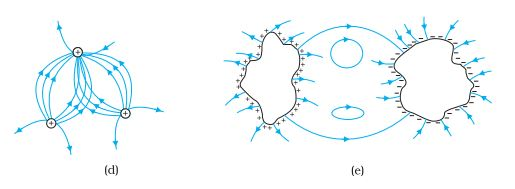
Answer:
(a) Wrong, because field lines must be normal to a conductor.
(b) Wrong, because field lines can only start from a positive charge. It cannot start from a negative charge,
(c) Right;
(d) Wrong, because field lines cannot intersect each other,
(e) Wrong, because electrostatic field lines cannot form closed loops.
Answer:
Force on a charge F=qE
But here E is varying along the Z direction.
Force can be written as,
$F=q\frac{dE}{dz}dz=P\frac{dE}{dz}=10^{-7}\times10^5=10^{-2}N$
Torque experienced =0 since both dipole and electric field are in the Z direction. The angle between dipole and the electric field is 180 degrees
$\tau = \mathbf{p}\times \mathbf{E}=pE \sin 180=0$
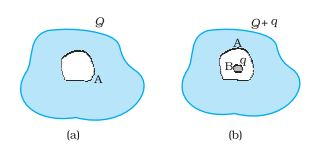
Answer:
We know that the electric field inside a conductor is zero.
Using Gauss' law, if we draw any imaginary closed surface inside the solid, the net charge must be zero.(Since E= 0 inside)
Hence, there cannot be any charge inside the conductor, and therefore, all charge must appear on the outer surface of the conductor.
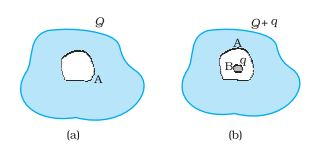
Answer:
We know that the electric field inside a conductor is zero.
Now, imagine a Gaussian surface just outside the cavity inside the conductor. Since E=0 (Using Gauss' law), the net charge must be zero inside the surface. Therefore, -q charge is induced on the inner side of the cavity(facing conductor B).
Now consider a Gaussian surface just outside the conductor A. The net electric field must be due to charge Q and q. Hence, q charge is induced on the outer surface of conductor A. Therefore, the net charge on the outer surface of A is Q + q.
Answer:
We know that the electric field inside a conductor is zero.
Therefore, a possible way to shield from the strong electrostatic fields in its environment is to enclose the instrument fully by a metallic surface.
Answer:
Let the surface area of the sphere be S.
And assume that the hole is filled. For a point B, just above the hole, considering a Gaussian surface passing through B, we have
$\oint E.dS = q/\epsilon_{0}$
Now, since the electric field is always perpendicular to the surface of the conductor.
$\\ \therefore \oint E.dS = E.S= q/\epsilon_{0} = (\sigma.S)/\epsilon_{0} \\ \implies E = \sigma/\epsilon_{0}$
Using Superposition principle, $E =E_{1} + E_{2}$ ,
where $E_{1}$ is due to the hole and $E_{2}$ is due to the rest of the conductor. (both pointing outwards, i.e away from the centre)
Again, for a point A, just below the hole, the Electric field will be zero because of electrostatic shielding.
Using the superposition principle, this will be due to $E_{1}$ pointing inwards(towards the centre) and due to $E_{2}$ (Pointing away from the centre)
$0 =E_{1}-E_{2}$ $\implies E_{1}=E_{2}$
Using this relation, we get:
$E =E_{1} + E_{2} = 2E_{1} \implies E_{1} = E/2 = \sigma/2\epsilon_{0}$
Since this is pointing outwards,
$\boldsymbol{E_{1}} = \sigma/2\epsilon_{0}\ \boldsymbol{\widehat{n}}$ is the electric field in the hole.
(Trick:
1. Assume the hole to be filled.
2. Consider 2 points just above and below the hole.
3. Electric fields at these points will be due to the hole and the rest of the conductor. Use the superposition principle.)
[Hint: Use Coulomb’s law directly and evaluate the necessary integral.]
Answer:
Let AB be a long, thin wire of uniform linear charge density λ.
Let us consider the electric field intensity due to AB at point P at a distance h from it, as shown in the figure.
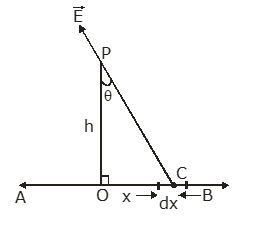
The charge on a small length dx on the line AB is q, which is given as q = λdx.
So, according to Coulomb’s law, the electric field at P due to this length dx is
$dE'=\frac{1}{4\pi \epsilon_o}\frac{\lambda dx}{(PC)^2}$
But $P C=\sqrt{h^2+x^2}$
$
\Rightarrow d E=\frac{1}{4 \pi \epsilon_o} \frac{\lambda d x}{\left(h^2+x^2\right)}
$
This electric field at P can be resolved into two components as dEcosθ and dEsinθ. When the entire length AB is considered, then the dEsinθ components add up to zero due to symmetry. Hence, there is only the dEcosθ component.
So, the net electric field at P due to dx is
dE' = dE cosθ
$
\Rightarrow d E^{\prime}=\frac{\lambda d x \cos \theta}{4 \pi \epsilon_o \left(h^2+x^2\right)}
$-----(1)
$\qquad$
$\ln \triangle \mathrm{POC}$
$
\tan \theta=\frac{x}{h}
$
$
\Rightarrow \mathrm{x}=\mathrm{h} \tan \theta
$
Differentiating both sides w.r.t. $\theta$,
$
\begin{aligned}
& \frac{d x}{d \theta}=h \sec ^2 \theta \\
& \Rightarrow \mathrm{dx}=\mathrm{h} \sec ^2 \theta \mathrm{~d} \theta .........(2)
\end{aligned}
$
Also, $h^2+x^2=h^2+h^2 \tan ^2 \theta$
$
\begin{aligned}
& \Rightarrow h^2+x^2=h^2\left(1+\tan ^2 \theta\right) \\
& \Rightarrow h^2+x^2=h^2 \sec ^2 \theta \ldots \ldots \ldots \ldots...........(3)
\end{aligned}
$
(Using the trigonometric identity, $1+\tan ^2 \theta=\sec ^2 \theta$ )
Using equations (2) and (3) in equation (1),
$
\begin{aligned}
& d E^{\prime}=\frac{\lambda \cos \theta \times h \sec ^2 \theta}{4 \pi \epsilon_o \times h^2 \sec ^2 \theta} d \theta \\
& d E^{\prime}=\frac{\lambda \cos \theta}{4 \pi \epsilon_o h} d \theta
\end{aligned}
$
The wire extends from $\theta=-\frac{\pi}{2}$ to $\theta=\frac{\pi}{2}$ since it is very long. Integrating both sides,
$
E^{\prime}=\int_{-\frac{\pi}{2}}^{\frac{\pi}{2}} \frac{\lambda \cos \theta}{4 \pi \epsilon_o h} d \theta
$
$\begin{aligned} & E^{\prime}=\frac{\lambda}{4 \pi \epsilon_o h} \int_{\frac{\pi}{-2}}^{\frac{\pi}{2}} \cos \theta d \theta \\ & E^{\prime}=\frac{\lambda}{4 \pi \epsilon_o h}\left(\sin \frac{\pi}{2}-\sin \left(-\frac{\pi}{2}\right)\right) \\ & E^{\prime}=\frac{\lambda}{4 \pi \epsilon_o h} \times 2 \\ & E^{\prime}=\frac{\lambda}{2 \pi \epsilon_o h}\end{aligned}$
This is the net electric field due to a long wire with linear charge density λ at a distance h from it.
In the question linear charge density =E
Therefore
$E'=\frac{E}{2\pi \epsilon_o h}$
Answer:
Given that a proton and a neutron consist of three quarks each.
And, ‘up’ quark is of charge + $\left ( \frac{2}{3} \right )$ e, and the ‘down’ quark of charge $\left (- \frac{1}{3} \right )$ e
Let the number of 'up' quarks be n. Therefore, the number of 'down' quarks is (3-n).
$\therefore$ The net charge = $\\ n\times\frac{2}{3}e + (3-n)\frac{-1}{3}e = (n-1)e$
Now, a proton has a charge +1e
$\therefore$ $(n-1)e = +1e \implies n =2$
Proton will have 2 u and 1 d, i.e, uud
Similarly, the neutron has a charge 0
$\therefore$ $(n-1)e = 0 \implies n =1$
Neutron will have 1 u and 2 d, i.e, udd
Answer:
For equilibrium to be stable, there must be a restoring force, and hence all the field lines should be directed inwards towards the null point. This implies that there is a net inward flux of the electric field. But this violates Gauss's law, which states that the flux of the electric field through a surface not enclosing any charge must be zero. Hence, the equilibrium of the test charge cannot be stable.
Therefore, the equilibrium is necessarily unstable.
Answer:
Two charges of the same magnitude and sign are placed at a certain distance apart. The mid-point of the line joining these charges will have E =0.
When a test charge is displaced along the line towards the charges, it experiences a restoring force(which is the condition for stable equilibrium). But if the test charge is displaced along the normal of the line, the net electrostatic force pushes it away from the starting point. Hence, the equilibrium is unstable.
Answer:
Let s be the vertical deflection, t be the time taken by the particle to travel between the plates
$\therefore$ $s = ut + \frac{1}{2}at^2$
Here, u =0, since initially there was no vertical component of velocity.
The particle in the electric field will experience a constant force (Since the electric field is constant).
F = ma = -qE (Using Newton's Second Law, F = ma)
$\therefore$ a = -qE/m (-ve sign implies here in downward direction)
Again, t = Distance covered/ Speed = $L/ v_{x}$
(In x-direction, since there is no force, the component of velocity in x-direction remains constant = $v_{x}$.
And, the distance covered in the x-direction = length of the plate = L)
Putting these values in our deflection equation,
$\\ s = ut + \frac{1}{2}at^2 = (0)(L/v_{x}) + \frac{1}{2}(-qE/m)(L/v_{x})^2 \\ \implies s =\frac{-qEL^2}{2mv_{x}^2}$
(S is -ve, which implies it deflects downwards.)
$\therefore$ The vertical deflection of the particle at the far edge of the plate is $\frac{qEL^{2}}{2mv_{x}^{2}}$.
This motion is similar to the motion of a projectile in a gravitational field, which is also a constant force. The force acting on the particle in the gravitational field is mg, while in this case, it is qE. The trajectory will be the same in both cases.
Answer:
$\therefore$ The vertical deflection of the particle at the far edge of the plate is $s=\frac{qEL^{2}}{2mv_{x}^{2}}$
given s= 0.5cm=0.005cm
Calculate for L from the above equation
$L=\sqrt{\frac{2smv_x^2}{qE}}=\sqrt{\frac{2\times 0.005\times 9.1\times 10^{-31}(2\times10^6)^2 }{1.6\times 10^{-19}\times9.1\times10^2}}$
L=1.6 cm
Electric Charges And Fields Class 12 NCERT Solutions: Topics And Subtopics
Chapter 1 – Electric Charges and Fields in Class 12 Physics introduces the fundamental concepts of electrostatics, focusing on how electric charges interact and create electric fields. This chapter lays the groundwork for understanding electric forces, Coulomb’s law, and field lines.
Topics and subtopics of class 12 Chapter 1, Electric Charge and Field, are given below:
1.1 Introduction
1.2 Electric Charge
1.3 Conductors and Insulators
1.4 Basic Properties of Electric Charge
1.4.1 Additivity of Charges
1.4.2 Charge Is Conserved
1.4.3 Quantisation of Charge
1.5 Coulomb’s Law
1.6 Forces Between Multiple Charges
1.7 Electric Field
1.7.1 Electric Field Due to a System of Charges
1.7.2 Physical Significance of Electric Field
1.8 Electric Field Lines
1.9 Electric Flux
1.10 Electric Dipole
1.10.1 The Field of an Electric Dipole
1.10.2 Physical Significance of Dipoles
1.11 Dipole in a Uniform External Field
1.12 Continuous Charge Distribution
1.13 Gauss’s Law
1.14 Applications of Gauss’s Law
1.14.1 Field Due to an Infinitely Long Straight Uniformly Charged Wire
1.14.2 Field Due to a Uniformly Charged Infinite Plane Sheet
1.14.3 Field Due to a Uniformly Charged Thin Spherical Shell
Electric Charges And Fields Class 12 NCERT Solutions: Important Formulas
Electric Charges and Fields Class 12 Important Formulas compile all the key equations and expressions from the chapter in one place, making revision quick and efficient. These formulas cover concepts like Coulomb’s law, electric field intensity, electric potential, and Gauss’s law, helping students solve problems with accuracy and speed.
Electric Charge (q)
1. Charge is a fundamental property of matter.
2. SI Unit: Coulomb (C)
3. Charge Quantization: $q=n e$ (where $n$ is an integer, $e=1.6 \times 10^{-19} \mathrm{C}$ )
Coulomb's Law
The force between two point charges at distance r is given by:

$
F=k \frac{\left|q_1 q_2\right|}{r^2}
$
where $k=\frac{1}{4 \pi f_0} \approx 9 \times 10^9 \mathrm{Nm}^2 / \mathrm{C}^2$
Electric Field (E)
1. The electric field at a point due to an electric charge $q$ :
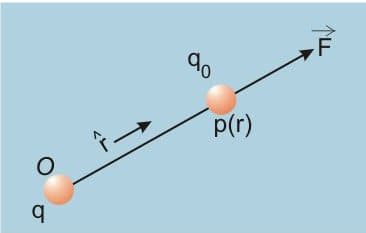
$
E=\frac{F}{q}=k \frac{q}{r^2}
$
2. For an electric Dipole at the Axial Position:
$
E=\frac{1}{4 \pi \varepsilon_0} \times \frac{2 p}{r^3}
$
3. For an electric Dipole at the Equatorial Position:
$
E=\frac{1}{4 \pi \varepsilon_0} \times \frac{p}{r^3}
$
Electric Dipole and Dipole Moment (p)
1. Dipole Moment: $p=q \times d$
2. Torque on a Dipole in a Uniform Electric Field:
$
\tau=p E \sin \theta
$
Electric Field Due to a Continuous Charge Distribution
1. Linear Charge Density $(\lambda): \lambda=\frac{q}{l}$
2. Surface Charge Density ( $\sigma$ ): $\sigma=\frac{q}{A}$
3. Volume Charge Density ( $\rho$ ): $\rho=\frac{q}{V}$
Gauss's Law
The total electric flux through a closed surface is:
$
\oint \mathbf{E} \cdot d \mathbf{A}=\frac{q}{\varepsilon_0}
$
Applications of Gauss's Law
1. Electric field due to an infinite line charge:
$
E=\frac{\lambda}{2 \pi \varepsilon_0 r}
$
2. Electric field due to a uniformly charged infinite plane:
$
E=\frac{\sigma}{2 \varepsilon_0}
$
3. Electric field due to a charged spherical shell:
Inside the shell: $E=0$
Outside the shell: $E=\frac{1}{4 \pi \varepsilon_0} \times \frac{q}{r^2}$
NCERT Solution for Class 12 Chapter 1: Importance in Board Exams
The CBSE Class 12 Physics exam is for 70 marks, and Chapter 1 is very important. In 2024-25, 5-mark questions were asked from the first chapter on electric charges fields and fields. Practising NCERT solutions helps in scoring well. Students can also download the PDF to study offline.
What Extra Should Students Study Beyond NCERT for JEE/NEET?
To score well in JEE and NEET, students must work beyond the NCERT syllabus with higher-level questions and be good at application-based concepts as well. The main differences in the additional preparation required to prepare for JEE and NEET are brought out in the table below.
NCERT Solutions for Class 12 Physics: Chapter Wise
NCERT Solutions for Class 12 Physics: Chapter-wise Links provide a structured pathway to access detailed solutions for every chapter in the syllabus. These solutions help students understand concepts clearly, improve problem-solving skills, and prepare effectively for board exams and competitive tests.
Also, check NCERT Books and NCERT Syllabus here:
- NCERT Books Class 12 Physics
- NCERT Syllabus Class 12 Physics
- NCERT Books Class 12
- NCERT Syllabus Class 12
NCERT solutions subject-wise
Frequently Asked Questions (FAQs)
Electric charges are present in many daily phenomena, like static electricity, lightning, photocopiers, and electronic devices.
Many conceptual and numerical questions on Coulomb’s Law, electric field, and the superposition principle are frequently asked in NEET and JEE exams.
Understanding electric charges and fields is fundamental to learning about electricity, electromagnetism, and how electric forces act at a distance—important for everything from electronics to physics research.
Yes, at certain points due to symmetry or superposition of fields, the net electric field can be zero.
The net charge inside a conductor is zero because charges redistribute on the surface. If the charge is zero then the electric field must be zero
Questions related to CBSE Class 12th
On Question asked by student community
Hello,
The date of 12 exam is depends on which board you belongs to . You should check the exact date of your exam by visiting the official website of your respective board.
Hope this information is useful to you.
Hello,
Class 12 biology questions papers 2023-2025 are available on cbseacademic.nic.in , and other educational website. You can download PDFs of questions papers with solution for practice. For state boards, visit the official board site or trusted education portal.
Hope this information is useful to you.
Hello Pruthvi,
Taking a drop year to reappear for the Karnataka Common Entrance Test (KCET) is a well-defined process. As a repeater, you are fully eligible to take the exam again to improve your score and secure a better rank for admissions.
The main procedure involves submitting a new application for the KCET through the official Karnataka Examinations Authority (KEA) website when registrations open for the next academic session. You must pay the required application fee and complete all formalities just like any other candidate. A significant advantage for you is that you do not need to retake your 12th board exams. Your previously secured board marks in the qualifying subjects will be used again. Your new KCET rank will be calculated by combining these existing board marks with your new score from the KCET exam. Therefore, your entire focus during this year should be on preparing thoroughly for the KCET to achieve a higher score.
For more details about the KCET Exam preparation,
CLICK HERE.
I hope this answer helps you. If you have more queries, feel free to share your questions with us, and we will be happy to assist you.
Thank you, and I wish you all the best in your bright future.
Yes, you can switch from Science in Karnataka State Board to Commerce in CBSE for 12th. You will need a Transfer Certificate from your current school and meet the CBSE school’s admission requirements. Since you haven’t studied Commerce subjects like Accountancy, Economics, and Business Studies, you may need to catch up before or during 12th. Not all CBSE schools accept direct admission to 12th from another board, so some may ask you to join Class 11 first. Make sure to check the school’s rules and plan your subject preparation.
Hello
For the 12th CBSE Hindi Medium board exam, important questions usually come from core chapters like “Madhushala”, “Jhansi ki Rani”, and “Bharat ki Khoj”.
Questions often include essay writing, letter writing, and comprehension passages. Grammar topics like Tenses, Voice Change, and Direct-Indirect Speech are frequently asked.
Students should practice poetry questions on themes and meanings. Important questions also cover summary writing and translation from Hindi to English or vice versa.
Previous years’ question papers help identify commonly asked questions.
Focus on writing practice to improve handwriting and presentation. Time management during exams is key to answering all questions effectively.
Popular CBSE Class 12th Questions
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters
