NCERT Class 12 Physics Chapter 1 Notes Electric Charges and Fields
Have you ever been shocked as you touched the handle of a car door on a dry winter day, or as you took off your woollen sweater? it is not magic--it is statical electricity at work. NCERT Class 12 Physics Chapter 1 Notes Electric charges and fields provides an introduction to the wonderful world of electrostatics and how charges accumulate, interact and create electric fields that affect various phenomena around us.
This Story also Contains
- NCERT Class 12 Physics Chapter 1 Notes: Download PDF
- NCERT Class 12 Physics Chapter 1 Notes
- Electric Field and Charges Previous year Question and Answer
- Importance of Chapter 1: Electric Charges and Fields Notes
- Electric charges and fields Class 12 Notes: Chapterwise
- NCERT Books and Syllabus
The students will study the science behind lightning in a thunderstorm, the shock of a doorknob, and numerous other examples of charge interaction in the real world in this chapter. It is an essential basis of Class 12 Physics and also plays a pivotal role in Class 12 board exams, JEE, NEET, or any other competitive examinations. The basic concepts are the Coulomb Law, law of superposition, lines of electric fields as well as the quantization of charge which are merely stepping stones towards solving problems of higher physics. Concepts related to Class 12 Physics Notes on Electric Charges and Fields are simplified by providing an organized summary, clearly labeled diagrams to visualize the field lines and force directions, vital formulas to revise quickly and sample questions to self-assess. These notes can help students to streamline the learning process, enhance their problem solving abilities and gain confidence in preparing both the board and competitive exams.
Also Read,
NCERT Class 12 Physics Chapter 1 Notes: Download PDF
NCERT Class 12 Physics Chapter 1 Notes - Electric Charges and Fields are a brief summary of all the important concepts, formulas, and diagrams to understand them quickly. These notes make learning electrostatics much easier, and harder subjects such as Coulumb Law and electric field lines are much easier to understand. The free PDF also allows students to revise and enhance their examination preparation at will.
NCERT Class 12 Physics Chapter 1 Notes
The NCERT Class 12 Physics Chapter 1 Notes- Electric Charges and Fields are a comprehensive and concise representation of the study material in form of concepts, formulas and illustrations. The notes can be used by students to revise the basics of electrostatics in quick time and are therefore, most suitable for CBSE, JEE and NEET exams.
Electric Charge:
- Charge of a material body or particle is the property (acquired or natural) due to which it produces and experiences electrical and magnetic effects. Some of naturally occurring charged particles are electrons, protons, α-particles etc.
- Charge is a derived physical quantity & is measured in Coulomb in S.I. unit. In practice we use mC(10−3C),μC(10−6C),nC(10−9C) etc.
- C.G.S. unit of charge = electrostatic unit= esu
- 1 coulomb =3×109esu of charge
- Dimensional formula of charge =[M∘L∘T1I1]
- Electric charge is a scalar quantity
- SI unit is Coulomb, and it is represented by the symbol "C."
- Dimension-[AT].
- Like charges repel each other and unlike charges attract each other
Point Charge:
- When the size of charged bodies is significantly smaller than the distance between them, their representation is simplified and they are treated as point charges.
Conductors and Insulators:
- Conductors are materials that allow electric charges to move through them and thus facilitate the flow of electricity.
- Insulators, on the other hand, are materials that obstruct the passage of electricity, making it difficult for electric charges to pass through them.
Methods of charging:
- A body can be charged by means of (a) friction, (b) conduction, (c) induction,
(a) Charging by Friction : When a neutral body is rubbed against other neutral body then some electrons are transferred from one body to other. The body which can hold electrons tightly, draws some electrons and the body which can not hold electrons tightly, loses some electrons. The body which draws electrons becomes negatively charged and the body which loses electrons becomes positively charged.

For example : Suppose a glass rod is rubbed with a silk cloth. As the silk can hold electrons more tightly and a glass rod can hold electrons less tightly (due to their chemical properties), some electrons will leave the glass rod and get transferred to the silk. So, in the glass rod their will be deficiency of electrons, therefore it will become positively charged. And in the silk, there will be some extra electrons, so it will become negatively charged
(b) Charging by induction: When a charged body comes into contact with an uncharged body, one side of the neutral body becomes oppositely charged while the other side remains unchanged.
For example, if a positively charged glass rod is brought close to a piece of paper, the paper is attracted. This occurs because the rod attracts electrons in the paper, causing the edge of the paper closest to the rod to become negatively charged, while the opposite end becomes positively charged due to an electron deficiency.
(c) Charging by conduction: When two conductors come into contact, the charges will be distributed evenly between them.
For example, When a negatively charged plastic rod comes into contact with a neutral pith ball, some electrons from the rod transfer to the pith ball, causing the pith ball to become negatively charged.
Properties of Charge:
- Additivity: The total charge (Q) in a system containing multiple charges(q1,q2,q3…………qn) is the sum of individual charges: q1+q2+.....qn.
- Conservation of Charge: It is not possible to create or destroy a charge; it can only be transferred. When rubbing a glass rod with silk, for example, the charge is transferred rather than created.
- Independence of Velocity: The velocity of a particle has no effect on its charge.
- Quantization: The charge on a body is quantized, meaning it is an integral multiple of the elementary charge (e), where e=1.6×10−19C is the charge of an electron. Therefore, the charge (q) on a body is q = ne, where n is an integer.
Coulomb's Law:
Coulomb's Law states that the force of attraction or repulsion between two charges is directly proportional to the product of the charges and inversely proportional to the square of the distance between them. Mathematically, it is expressed as:
F∝Q1Q2r2F=KQ1Q2r2
Where,
- F is the electrostatic force between the charges,
- K is Coulomb's constant
- Q1 and Q2 are the magnitudes of the charges
- r is the separation between the charges.
K=14πϵ0
Where
ϵ0=8.854×10−12C2N−m2, known as absolute permittivity of air or free space
The vector form of Coulomb's Law:
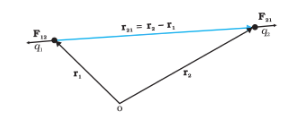
Consider two charges, q1 and q2, separated by r. Let the position vectors of q1 be r1 and that of q2 be r2. The force due to q1 on q2 is then directed along the unit vector r12, as shown in figure F12.
F12→=Kq1q2r2r^12 here r^12=r→2−r→1|r2−r1|=r→12rF12→=Kq1q2r3r→12
Force when dielectric inserted between the charges:
When a dielectric material with a dielectric constant (k) is completely filled between charges, the force
Fmed=q1q24πε0kr2=q1q24πε0ϵrr2
ϵr is the relative permittivity / dielectric constant of the medium.
- The dielectric constant is the ratio of the permittivity of a substance to the permittivity of free space. (dielectric will be explained later in detail in this chapter)
Principle of Superposition:
It states that the total force acting on a given charge as a result of a group of other charges is the vector sum of the individual forces acting on that charge as a result of each of the other charges.
Electrostatic Equilibrium:
The point where the resultant force on a charged particle becomes zero is called equilibrium position.
- Stable Equilibrium: A charge is initially in equilibrium position and is displaced by a small distance. If the charge tries to return back to the same equilibrium position then this equilibrium is called position of stable equilibrium.
- Unstable Equilibrium: If a charge is displaced by a small distance from its equilibrium position and the charge has no tendency to return to the same equilibrium position. Instead it goes away from the equilibrium position.
- Neutral Equilibrium: If charge is displaced by a small distance and it is still in equilibrium condition then it is called neutral equilibrium.
Electric Field:
The region surrounding a charge, where another charged particle would experience a force, is referred to as the electric field in that space.
Electric Field Intensity:
The electric field intensity at any point is defined as the force experienced by a unit positive charge placed at that point.
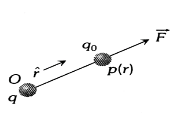
Electric field intensity,
E=Fq0
where,
F is the force experienced by q0,
- The SI unit of E is, Newton Coulomb = Volt meter = Joule Coulomb × Meter and the dimensional formula is [MLT−3A−1]
- The electric field is a vector quantity and due to the positive charge is away from the charge and for the negative charge, it is towards the charge.
Properties of electric field intensity E→ :
(i) It is a vector quantity. Its direction is the same as the force experienced by positive charge.
(ii) Direction of electric field due to positive charge is always away from it while due to negative charge, always towards it.
(iii) Its S.I. unit is Newton/Coulomb.
(iv) Its dimensional formula is [MLT−3 A−1]
(v) Electric force on a charge q placed in a region of electric field at a point where the electric field intensity is E→ is given by F→=qE→. Electric force on point charge is in the same direction of electric field on positive charge and in opposite direction on a negative charge.
(vi) It obeys the superposition principle, that is, the field intensity at a point due to a system of charges is vector sum of the field intensities due to individual point charges,
i.e. E→=E→1+E→2+E→3+….
(vii) It is produced by source charges. The electric field will be a fixed value at a point unless we change the distribution of source charges.
Electric Field Due To A Point Charge:
The electric field produced by a point charge q can be obtained in general terms from Coulomb's law. First note that the magnitude of the force exerted by the charge q on a test charge q0 is

Fe=14πεo⋅qqor2
then divide this value by qo to obtain the magnitude of the field.
E=14πεo⋅qr2
- If q is positive, E is directed away from q. On the other hand, if q is negative, then E is directed towards q.
- The electric field at a point is a vector quantity. Suppose E1 is the field at a point due to a charge q1 and E2 in the field at the same point due to a charge q2. The resultant field when both the charges are present is
E=E1+E2
- If the given charge distribution is continuous, we can use the technique of integration to find the resultant electric field at a point.
Electric field due to a system of charges:
The electric field obeys the superposition principle. That is the electric field due to a system of charge at a point is equal to the vector sum of all the electric fields.
Electric Lines of Force:
An electric field line is an abstract line or curve that is traced through a region of space so that its tangent at any point indicates the direction of the electric field at that point.
Properties of Electric Lines of Force (Electric Field Lines):
i) Electric forces diverge from positive charges and converge on negative charges.


ii) The number of field lines is proportional to the charge magnitude.
iii) Force lines never intersect because this would imply a contradiction in the direction of the electric field at the intersection point.
iv) Electric field lines do not form closed loops, as they cannot start and end on the same charge.
v) The direction of the force on a charged particle placed at any point on an electric field line is given by the tangent.
vi) Electric field lines do not represent a particle's actual path; they can only indicate the path if the electric field lines are straight.
vii) Electric field lines are normal to conductors if they originate or terminate on a conductor.
viii) Straight, parallel, and equidistant lines represent a uniform electric field.
ix) An electric field does not exist within a conductor.
Electric Flux ( ϕ ):
The Electric flux through an area is the number of electric field lines passing normally through that area.

- Flux through an area dA is given by
dϕ=E→⋅d→A=EdAcosθ
here θ is the angle between the area vector and the electric field.
-
Total flux through area A is
ϕ=∫E→⋅d→A
-
Flux is a scalar quantity and the SI unit of flux is volt-meter or weber (Wb).
Electric Dipole:
An electric dipole is made up of two equal and opposing charges separated by a small vector distance. The direction of the dipole moment is from the negative to the positive charge.

Electric Dipole Moment:
(p→)=q(2l→)
Here, 2l is the dipole length.
- The electric dipole moment(p) is a vector quantity from negative to positive charge and Its SI unit is Coulomb-meter (C·m).
Electric Field Due To An Electric Dipole At Axial and Equatorial Point:
i) At the axial point
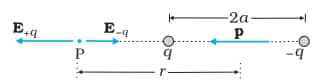
Earial =2kpr(r2−l2)2
if r>>>l
Eaxial=14πϵ02pr3
ii) At Equatorial Point
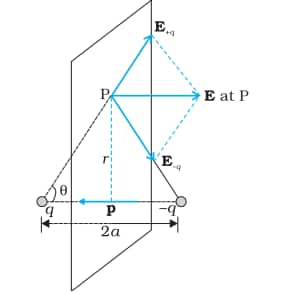
Eequatorial=kp(r2+l2)32 If r>>>lEequatorial=14πε0pr3
Torque Experienced by the Dipole:
τ→=p→×E→τ→=pEsinΘ
Potential Energy of Dipole
When an electric dipole is placed in an electric field E, a torque τ→=p×E acts on it. If we rotate the dipole through a small angle dθ, the work done by the torque is
dW=τdθdW=−pEsinθdθ
The work is negative as the rotation dθ is opposite to the torque. The change in electric potential energy of the dipole is therefore
dU=−dW=pEsinθdθ
Now, at angle θ=90∘, the electric potential energy of the dipole may be assumed to be zero as net work done by the electric forces in bringing the dipole from infinity to this position will be zero.

Integrating,
From 90∘ to θ, we have or
U(θ)−U(90∘)=pE[−cosθ]90∘θ
∴U(θ)=−pEcosθ=−p⋅E
If the dipole is rotated from an angle θ1 to θ2, then
Work done by external forces =U(θ2)−U(θ1)Wext forces =−pEcosθ2−(−pEcosθ1)Wext. foreses =pE(cosθ1−cosθ2)
or
and work done by electric forces,
Welectric force =−Wext. forsec =pE(cosθ2−cosθ1)
Continuous Charge Distribution:
A continuous charge distribution refers to the distribution of an amount of charge either uniformly or non-uniformly on a body. There are three main types of continuous charge distribution:
i) Linear charge distribution:
λ=qL=Cm=Cm−1
(λ)− charge per unit length.
Example: wire, circular ring
ii) Surface charge distribution:
σ=QA=Cm2=Cm−2
(σ)− charge per unit Area
Example: plane sheet
iii) Volume Charge distribution
ρ=QV=Cm3=Cm−3
(ρ)− charge per unit volume.
(charge on a dielectric sphere etc)
Gauss's Law:
The total electric flux through a charged closed surface is equal to 1ϵ0 times the total charge q enclosed by the surface.
ϕ=∮E→⋅d→A=1ϵ0q
Notes:
- Gauss's law can be applied to any closed surface. The surface's size and shape do not matter.
- Gauss' law considers the electric field (E) as the result of all charges, whether inside or outside the Gaussian surface.
- The electric field outside the Gaussian surface has no effect on the net flux through it.
- The Gaussian surface is the surface chosen for the application of Gauss' law. Any Gaussian surface can be chosen, but be careful not to choose one that passes through any discrete charge. This is due to the fact that the electric field generated by a system of discrete charges is undefined at the location of any charge.
- Continuous charge distributions are allowed to pass through the Gaussian surface.
- Gauss' law is especially useful for simplifying the calculation of the electrostatic field in systems with some symmetry.
Applications of Gauss's Law:
1. Electric field due to an infinitely long straight uniformly charged wire:
The electric field produced by an infinitely long straight uniformly charged wire at a distance x from the wire must be determined.
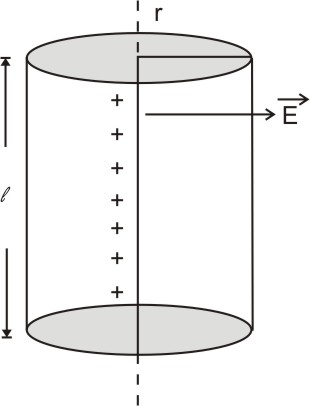
We have a cylindrical Gaussian surface of radius r=x and length l.
charge enclosed by the surface
E=λ2πxε0
2. Field due to a uniformly charged infinite plane sheet:
Let the plane sheet be positively charged
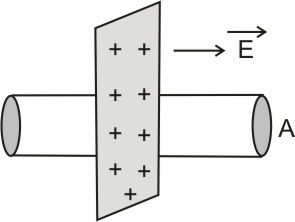
Let the Gaussian surface be a cylinder of cross-sectional area A
ϕ=E×2A
( the flux passes only through 2 circular cross-sections of the cylinder)
According to Gauss's law
ϕ=qεoE×2A=σAε0E=σ2ε0
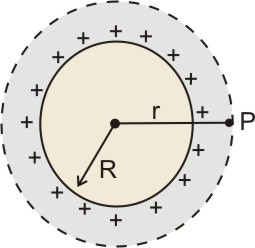
3. Field due to a uniformly charged thin spherical shell
The radius of the shell is R and the radius of the Gaussian surface (sphere) is r.
(i) Outside shell
E=q4πεor2
(ii) Inside the shell
Since inside a spherical shell charge =0, E=0
4. Electric field due to a point charge

The electric field due to a point charge is everywhere radial. We wish to find the electric field at a distance r from the charge q. We select Gaussian surface, a sphere at distance r from the charge. At every point of this sphere the electric field has the same magnitude E and it is perpendicular to the surface itself. Hence, we can apply the simplified form of Gauss's law,
ES=qin ε0
Here, S= area of sphere =4πr2 and
qin = net charge enclosing the Gaussian surface =qE(4πr2)=qε0
E=14πε0⋅qr2
It is nothing but Coulomb's law.
Electric Field and Charges Previous year Question and Answer
Q1: Consider three metal spherical shells A, B and C, each of radius R. Each shell has a concentric metal ball of radius R/10. The spherical shells A, B and C are given charges +6q,−4q, and +14q respectively. Their inner metal balls are also given charges −2q,+8q and −10q respectively. Compare the magnitude of the electric fields due to shells A, B and C at a distance 3 R from their centres.
Answer:
The electric field at a distance 3R from the center of a spherical shell depends only on the net charge enclosed and is given by Gauss's law:
E=14πϵ0Qnetr2
where Qnet is the total charge enclosed by each shell.
Net Charge on Each Shell -
For Shell A:
QA=6q+(−2q)=4q
For Shell B:
QB=−4q+8q=4q
For Shell C:
QC=14q+(−10q)=4q
Since the total charge enclosed for all three shells is the same (4q), the magnitude of the electric field at a distance 3R is identical for all:
EA=EB=EC=14πϵ04q(3R)2
Hence, the electric fields due to shells A,B, and C at a distance 3R are equal.
Q2: A charge Q in fixed in position. Another charge q is brought near charge Q and released from rest. Which of the following graphs is the correct representation of the acceleration of the charge q as a function of its distance r from charge Q?
A. 
B. 
C. 
D. 
Answer:
When a charge q is brought close to a stationary charge Q, the force on q due to Q is provided by Coulomb's Law:
F=k|Qq|r2
where k is Coulomb's constant and r is the distance between the charges. The acceleration a of charge q can be expressed using Newton's second law:
a=Fm=k|Qq|mr2
where m is the mass of charge q.
From this formula, we can observe that as distance r gets smaller, acceleration a gets much greater, tending towards infinity as r tends towards zero. Therefore, the graph that best illustrates this relationship is graph (1), which illustrates that acceleration gets smaller as distance gets greater, in a hyperbolic manner.
Hence, the answer is the option (A).
Q3: State Gauss's law on electrostatics and derive an expression for the electric field due to a long straight thin uniformly charged wire (linear charge density λ ) at a point lying at a distance r from the wire.
Answer:
Gauss' Law
The total electric flux over a closed surface is equal to 1ε0 times the net charge enclosed within the surface.
ϕE=∮E⋅ds=1ε0q
Electric field due to an infinitely long straight charged wire

Consider infinitely long straight charged wire of linear charge density λC/m
For calculating electric field consider an imaginary cylindrical Gaussian Surface of radius r and length l.
Here the field is radial everywhere, so flux through the two ends of the cylinder is Zero.
At the Gaussian cylindrical surface, the electric field E is normal to the surface at every point. The magnitude of E depends only on radius 'r', so it is constant.
Therefore flux through the Gaussian surface
=E×2πrl−−−(1)
According to Gauss's law, flux
ϕ= charge enclosed ε0
Here, total charge enclosed = linear charge density × length=λl
Therefore flux ϕ=λlε0−−−(2)
Using equation (1) and (2)
E×2πrl=λlε0
That is,
E=λ2πε0r
The vector notation is
E→=λ2πε0rn^
where n^ is the radial unit vector normal to the line charge
Importance of Chapter 1: Electric Charges and Fields Notes
- Electrostatics Foundation
-
This chapter presents the science of charges at rest, which is electrostatics.
-
The basic concepts in the construction of subsequent chapters, such as Electrostatic Potential, Capacitance, and Current Electricity, include charge, Coulomb's Law, electric field, electric field lines, and the superposition principle.
- Clarity of Core Concepts
- Complex definitions like quantisation of charge, continuous charge distribution, and electric dipole are made simple through notes.
- The charts and briefings will assist students in having visual representations of abstract concepts such as field lines and their characteristics.
- Quick Revision Tool
-
Notes contain important derivations, key formulas and definitions all in a single place.
-
Students would be able to reread the entire chapter within a limited time period before exams.
- Boost to Competitive Exams (JEE/NEET)
-
In JEE Mains/Advanced and NEET, electrostatics carries a high weightage.
-
Significant formulas (such as the force between charges, field due to point charges, the moment of a dipole, and so on) and common problem-solving strategies are highlighted with notes.
-
Diagrammatic representation
-
Charge distributions, field lines, and the direction of forces are commonly difficult to visualise.
-
Notes have well-labelled diagrams that enhance comprehension and aid in scoring marks on exams.
- Step-by-Step Problem Solving
-
Notes comprised of previous year question examples that train students to apply concepts.
-
They develop the analytical thinking skills that will be needed in numerical questions in CBSE and in the entrance exams.
Electric charges and fields Class 12 Notes: Chapterwise
NCERT Class 12 Physics Chapter 1 Notes Electric charges and fields give a clear and structured explanation of electrostatics, from the basics of charge to advanced applications like electric fields and Gauss’s law. These notes are organized chapterwise, making it simple for students to revise systematically and strengthen their preparation for board and competitive exams.
|
NCERT Class 12 Physics Chapter 1 Notes |
Subject Wise NCERT Exemplar Solutions
Subject Wise NCERT Solutions
NCERT Books and Syllabus
Frequently Asked Questions (FAQs)
Yes, Electric Charges and Fields Class 12 notes are important for JEE preparation, covering fundamental concepts frequently tested in both JEE Main and JEE Advanced exams.
No, this is a short note which can be used to revise the chapter. The necessary derivations are not mentioned here in the NCERT Class 12 Physics chapter 1 notes. Along with this, you can also go through the NCERT Solutions and also CBSE previous year papers and derivations.
The study of charge under rest is known as electrostatics
When iron is isolated, then the net charge of the iron is zero. There is no drift of charge to start the conduction.
The main topics covered here are coulombs law, the concept of the electric field and electric dipole, Gauss’s law and its applications.
Popular Questions
Courses After 12th
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters