Careers360 Logo
School
Search Colleges, Exams, Schools & more
NCERT Solutions for Class 12 Physics Chapter 9 Ray Optics and Optical Instruments

NCERT Solutions for Class 12 Physics Chapter 9 Ray Optics and Optical Instruments

Edited By Vishal kumar | Updated on Sep 11, 2023 09:47 AM IST | #CBSE Class 12th

NCERT Solutions for Class 12 Physics Chapter 9 –Download Free PDF

NCERT solutions for class 12 physics chapter 9 Ray Optics and Optical Instruments are a valuable resource for students studying this chapter. These ncert solutions class 12 physics chapter 9 cover a wide range of topics related to the behaviour of light, reflection, refraction, optical instruments, and more. They provide detailed explanations for each question, making it easier for students to understand and apply the concepts. These class 12 physics chapter ray optics ncert solutions are essential for board exams and can also be helpful for competitive exams. Students can access these solutions for free, making them an accessible and convenient study aid.

This Story also Contains
  1. NCERT Solutions for Class 12 Physics Chapter 9 –Download Free PDF
  2. NCERT Solutions for Class 12 Physics Chapter 9 Ray Optics and Optical Instruments
  3. NCERT solutions for class 12 physics chapter 9 ray optics and optical instruments exercise:
  4. Power of a lens
  5. Topics Covered in Class 12 Physics Chapter Ray Optics
  6. Importance of NCERT solutions for class 12 physics chapter 9 ray optics and optical instruments:
  7. Key features of Cbse Class 12 Ray Optics NCERT Solutions
  8. NCERT solutions subject wise
  9. NCERT Exemplar Class 12 Solutions
NCERT Solutions for Class 12 Physics Chapter 9 Ray Optics and Optical Instruments
NCERT Solutions for Class 12 Physics Chapter 9 Ray Optics and Optical Instruments

The chapter comes under the unit optics. The solutions of NCERT class 12 physics chapter 9 ray optics and the optical instrument is unavoidable as far as the CBSE board exam is considered. Sometimes in such exams, questions are directly asked from NCERT for which CBSE NCERT solutions for class 12 physics chapter 9 ray optics and the optical instrument can be helpful to score well. There are many problems related to refraction and reflection in the NCERT solutions for class 12 physics chapter 9 ray optics and optical instruments.

Each and every topic of chapter 9 ray optics and optical instruments are important for exams and there is no scope of skipping any topic. NCERT solutions are one of the keys to success in CBSE board exams. Knowledge of sign convention is mandatory to understand the solutions of NCERT Class 12 Physics Solutions chapter 9 ray optics and optical instruments. Some of the important formulas that will help in NCERT solutions for Class 12 Physics Chapter 9 PDF download are listed below:

VMC VIQ Scholarship Test

Register for Vidyamandir Intellect Quest. Get Scholarship and Cash Rewards.

Apply
Pearson | PTE

Register now for PTE & Unlock 20% OFF : Use promo code: 'C360SPL20'. Valid till 15th NOV'24! Trusted by 3,500+ universities globally

Apply

Free ray optics class 12 ncert solutions pdf download for CBSE exam.

NCERT Solutions for Class 12 Physics Chapter 9 Ray Optics and Optical Instruments

Download PDF


NCERT solutions for class 12 physics chapter 9 ray optics and optical instruments exercise:

Q 9.1 A small candle, 2.5 cm in size is placed at 27 cm in front of a concave mirror of radius of curvature 36 cm. At what distance from the mirror should a screen be placed in order to obtain a sharp image? Describe the nature and size of the image. If the candle is moved closer to the mirror, how would the screen have to be moved?

Answer:

Given, size of the candle, h = 2.5 cm

Object distance, u = 27 cm

The radius of curvature of the concave mirror, R = -36 cm

focal length of a concave mirror = R/2 = -18 cm

let image distance = v

now, as we know

\frac{1}{u}+\frac{1}{v}=\frac{1}{f}

\frac{1}{-27}+\frac{1}{v}=\frac{1}{-18}

\frac{1}{v}=\frac{1}{-18} + \frac{1}{27}

v= -54cm

now, let the height of image be h'

magnification of the image is given by

m=\frac{h'}{h}=-\frac{v}{u}

from here

{h'}=-\frac{-54}{-27}*2.5=-5cm

Hence the size of the image will be -5cm. negative sign implies that the image is inverted and real

if the candle is moved closer to the mirror, we have to move the screen away from the mirror in order to obtain the image on the screen. if the image distance is less than the focal length image cannot be obtained on the screen and image will be virtual.

Ray Optics and Optical Instruments Excercise:

Question:

Q 9.2 A 4.5 cm needle is placed 12 cm away from a convex mirror of focal length 15 cm. Give the location of the image and the magnification. Describe what happens as the needle is moved farther from the mirror.

Answer:

Given, the height of needle, h = 4.5 cm

distance of object = 12 cm

focal length of convex mirror = 15 cm.

Let the distance of the image be v

Now as we know

\frac{1}{u}+\frac{1}{v}=\frac{1}{f}

\frac{1}{v}=\frac{1}{f} - \frac{1}{u}

\frac{1}{v}=\frac{1}{15} - \frac{1}{-12}

\frac{1}{v}=\frac{1}{15} + \frac{1}{12}

v = 6.7 cm

Hence the distance of the image is 6.7 cm from the mirror and it is on the other side of the mirror.

Now, let the size of the image be h'

so.

m=-\frac{v}{u} = \frac{h'}{h}

h'= -\frac{v}{u}*h

h'= -\frac{6.7}{-12}*4.5

h'= 2.5 cm

Hence the size of the image is 2.5 cm. positive sign implies the image is erect, virtual and diminished.


magnification of the image = \frac{h'}{h} = \frac{2.5}{4.5} = 0.56

m = 0.56

The image will also move away from the mirror if we move the needle away from the mirror, and the size of the image will decrease gradually.

Q 9.3 A tank is filled with water to a height of 12.5 cm. The apparent depth of a needle lying at the bottom of the tank is measured by a microscope to be 9.4 cm. What is the refractive index of water? If water is replaced by a liquid of refractive index 1.63 up to the same height, by what distance would the microscope have to be moved to focus on the needle again?

Answer:

Given:

Actual height of the tank,h = 12.5 cm

Apparent height of tank,h' = 9.4 cm

let refrective index of the water be \mu

\mu = \frac{h}{h'} =\frac{12.5}{9.4} = 1.33 (approx)

so the refractive index of water is approximately 1.33.

Now, when water is replaced with a liquid having \mu = 1.63

\mu = \frac{h}{h'} =\frac{12.5}{h'_{new}} = 1.63

h'_{new}= \frac{12.5}{1.63}= 7.67 cm

Hence the new apparent height of the needle is 7.67 cm.

Total distance we have to move in a microscope = 9.4 - 7.67 = 1.73 cm.

Since new apparent height is lesser than the previous apparent height we have to move UP the microscope in order to focus the needle.

Q 9.4 Figures of (a) and (b) show refraction of a ray in air incident at 60^{\circ} with the normal to a glass-air and water-air interface, respectively. Predict the angle of refraction in glass when the angle of incidence in water is 45^{\circ} with the normal to a water-glass interface [Fig.(c)].

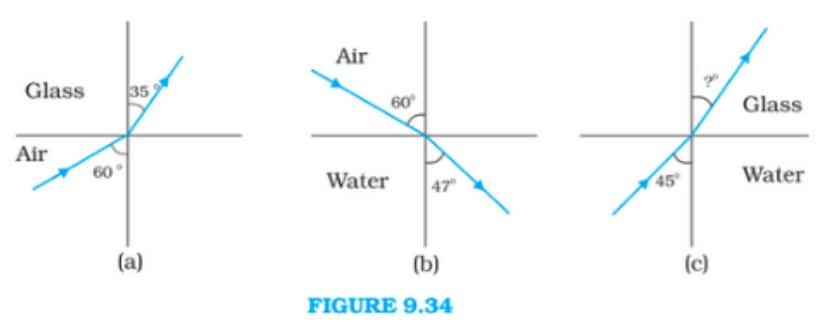
15946199732371594619969855

Answer:

As we know,by snell's law

\mu_1sin\theta _1=\mu _2sin\theta _2 where,

\mu_1 = refrective index of medium 1

\theta _1 = incident angle in medium 1

\mu _2 = refrective index of medium 2

\theta _2 = refraction angle in medium 2

NOW, APPLYING IT FOR fig (a)

1sin60=\mu _{glass}sin35

\mu _{glass}=\frac{sin60}{sin35}=\frac{0.866025}{0.573576 } = 1.509

Now applying for fig (b)

1sin60=\mu _{water}sin47

\mu _{water}=\frac{sin60}{sin47}=\frac{.8660}{.7313} = 1.184

Now in fig (c) Let refraction angle be \theta so,

\mu _{water}sin45=\mu _{glass}sin\theta

sin\theta =\frac{\mu _{water}*sin45}{\mu _{glass}}

sin\theta =\frac{1.184*0.707}{1.509} = 0.5546

\theta = sin^{-1}(0.5546) = 38.68

Therefore the angle of refraction when ray goes from water to glass in fig(c) is 38.68.

Q 9.5 A small bulb is placed at the bottom of a tank containing water to a depth of 80cm. What is the area of the surface of water through which light from the bulb can emerge out? Refractive index of water is 1.33. (Consider the bulb to be a point source.)

Answer:

Rays of light will emerge out in all direction and upto the angle when total internal reflection starts i.e. when the angle of refraction is 90 degree.

let the incident angle be i when refraction angle is 90 degree.

so, by snell's law

\mu _{water}sini=1sin90

from here, we get

sini=\frac{1}{1.33}

i=sin^{-1}(\frac{1}{1.33})=48.75^0

Now Let R be Radius of the circle of the area from which the rays are emerging out. and d be the depth of water which is = 80 cm.

From the figure:

tani=\frac{R}{d}

R = tani*d=tan48.75^0*80cm

R = 91 cm

So the area of water surface through which rays will be emerging out is

\Pi R^2 = 3.14*(91)^2cm^2

= 2.61m^2

therefore required area = 2.61m^2 .

Q 9.6 A prism is made of glass of unknown refractive index. A parallel beam of light is incident on a face of the prism. The angle of minimum deviation is measured to be 40^{\circ} . What is the refractive index of the material of the prism? The refracting angle of the prism is 60^{\circ} . If the prism is placed in water, predict the new angle of minimum deviation of a parallel beam of light.

Answer:

In Prism :

Prism angle ( A ) = First Refraction Angle ( r _1 ) + Second refraction angle ( r _2 )

also, Deviation angle ( \delta ) = incident angle( i ) + emerging angle( e ) - Prism angle ( A ) ..............(1)

the deviation angle is minimum when the incident angle( i ) and an emerging angle( e ) are the same. in other words

i=e ...........(2)

from (1) and (2)

\delta_{min} = 2i-A

i=\frac{\delta_{min} +A}{2} ..........................(3)

We also have

r _1 = r_2 = r = \frac{A}{2} .................(4)

Now applying snells law using equation (3) and (4)

\mu _1sini=\mu _2sinr

1sin(\frac{\delta _{min}+A}{2})=\mu _2sin\frac{A}{2}

\mu _2=\frac{sin(\frac{\delta _{min}+A}{2})}{sin\frac{A}{2}} ...................(5)

Given

\\\delta _{min}= 40 \\A = 60

putting those values in (5) we get

\mu _2=\frac{sin(\frac{40+60}{2})}{sin\frac{60}{2}}=\frac{sin50}{sin30}=1.532

Hence the refractive index of the prism is 1.532.

Now when the prism is in the water.

Applying Snell's law:

\mu _1sin(\frac{\delta _{min}+A}{2})=\mu _2sin\frac{A}{2}

1.33sin(\frac{\delta _{min}+60}{2})=1.532sin\frac{60}{2}

sin(\frac{\delta _{min}+60}{2})=\frac{1.532*0.5}{1.33}

\frac{\delta _{min}+60}{2}=sin^{-1}\frac{1.532*0.5}{1.33}

\delta _{min} =2sin^{-1}0.5759 - 60

\delta _{min} =2*35.16- 60 =10.32^0

Hence minimum angle of deviation inside water is 10.32 degree.

Q 9.7 Double-convex lenses are to be manufactured from a glass of refractive index 1.55, with both faces of the same radius of curvature. What is the radius of curvature required if the focal length is to be 20cm?

Answer:

As we know the lens makers formula

\frac{1}{f}=(\mu _{21}-1)(\frac{1}{R_1}-\frac{1}{R_2})

[ This is derived by considering the case when the object is at infinity and image is at the focus]

Where f = focal length of the lens

\mu _{21} = refractive index of the glass of lens with the medium(here air)

R_1 and R_2 are the Radius of curvature of faces of the lens.

Here,

Given, f = 20cm,

R_1 = R and R_2 = -R

\mu _{21} = 1.55

Putting these values in te equation,

\frac{1}{20}=(1.55-1)(\frac{1}{R}-\frac{1}{-R})

\frac{2}{R}=\frac{1}{20}*\frac{1}{0.55}

R = 40*0.55

R = 22cm

Hence Radius of curvature of the lens will be 22 cm.

Q 9.8 A beam of light converges at a point P. Now a lens is placed in the path of the convergent beam 12cm from P. At what point does the beam converge if the lens is (a) a convex lens of focal length 20cm, and (b) a concave lens of focal length 16cm?

Answer:

In any Lens

: \frac{1}{v} - \frac{1}{u}=\frac{1}{f}

\\v= the distance of the image from the optical centre

\\u= the distance of the object from the optical centre

\\f= the focal length of the lens

a)

Here, The beam converges from the convex lens to point P. This image P will now act as an object for the new lens which is placed 12 cm from it and focal length being 20 cm.

So

, \frac{1}{v} - \frac{1}{12}=\frac{1}{20}

\frac{1}{v}=\frac{1}{20} + \frac{1}{12}

\frac{1}{v}=\frac{8}{60}

v = 7.5 cm

Hence distance of image is 7.5 cm and it will form towards the right as the positive sign suggests.

b)

HERE, Focal length f = -16cm

so,

\frac{1}{v} - \frac{1}{12}=\frac{1}{-16}


\frac{1}{v} =\frac{1}{-16} + \frac{1}{12} = \frac{1}{48}

v = 48 cm

Hence image distance will be 48 cm in this case, and it will be in the right direction(as the positive sign suggests)

Q 9.9 An object of size 3.0cm is placed 14cm in front of a concave lens of focal length 21cm. Describe the image produced by the lens. What happens if the object is moved further away from the lens?

Answer:

In any Lens

: \frac{1}{v} - \frac{1}{u}=\frac{1}{f}

\\v= the distance of the image from the optical centre

\\u= the distance of the object from the optical centre

\\f= the focal length of the lens

Here Given,

\\u= -14 cm

\\f= -21 cm

\frac{1}{v} - \frac{1}{-14}=\frac{1}{-21}

\frac{1}{v} =\frac{1}{-21}- \frac{1}{14} = \frac{-5}{42}

v = -\frac{42}{5}= -8.4cm

Hence image distance is -8.4 cm. the negative sign indicates the image is erect and virtual.

Also as we know,]

m= -\frac{v}{u} = \frac{h'}{h}

From Here

h'= -\frac{v}{u}h

h'= -\frac{-8.4}{-12}*3= 1.8 cm

Hence the height of the image is 1.8 cm.

As we move object further away from the lens, the image will shift toward the focus of the lens but will never go beyond that.size of the object will decrease as we move away from the lens.

Q 9.10 What is the focal length of a convex lens of focal length 30cm in contact with a concave lens of focal length 20cm? Is the system a converging or a diverging lens? Ignore the thickness of the lenses.

Answer:

When two lenses are in contact the equivalent is given by

\frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2}

where f_1 and f_2 are the focal length of two individual lenses.

SO,Given,

f_1 = 30 cm and f_2 = -20cm (as focal length of the convex lens is positive and of the concave lens is negative by convention)

putting these values we get,

\frac{1}{f}=\frac{1}{30}+\frac{1}{-20}

\frac{1}{f}=-\frac{1}{60}

f = -60cm

Hence equivalent focal length will be -60 cm and since it is negative, equivalent is behaving as a concave lens which is also called diverging lens.

Q 9.11 A compound microscope consists of an objective lens of focal length 2.0 cm and an eyepiece of focal length 6.25 cm separated by a distance of 15cm. How far from the objective should an object be placed in order to obtain the final image at (a) the least distance of distinct vision (25cm), and (b) at infinity? What is the magnifying power of the microscope in each case?

Answer:

In a compound microscope, first, the image of an object is made by the objective lens and then this image acts as an object for eyepiece lens.

Given

the focal length of objective lens = f_{objective} = 2 cm

focal length of eyepiece lense = f_{eyepiece} = 6.25cm

Distance between the objective lens and eyepiece lens = 15 cm

a)

Now in Eyepiece lense

Image distance = v_{final} = -25 cm (least distance of vision with sign convention)

focal length = f_{eyepiece} = 6.25 cm

\frac{1}{f_{eyepiece}} = \frac{1}{v_{final}} - \frac{1}{u}

\frac{1}{u} = \frac{1}{v_{final}} -\frac{1}{f_{eyepiece}}

\frac{1}{u} = \frac{1}{-25} -\frac{1}{6.25} = -\frac{1}{5}

u = -5 cm.

Now, this object distance u is from the eyepiece lens since the distance between lenses is given we can calculate this distance from the objective lens.

the distance of u from objective lens = d+u= 15 - 5=10cm . This length will serve as image distance for the objective lens.

v = 10 cm

so in the objective lens

\frac{1}{f_{objective}} = \frac{1}{v} - \frac{1}{u_{initial}}

\frac{1}{u_{initial}} = \frac{1}{v} - \frac{1}{f_{objective}}

\frac{1}{u_{initial}} = \frac{1}{10} - \frac{1}{2} = -\frac{4}{10}=-\frac{2}{5}

u_{intial} = -2.5 cm

Hence the object distance required is -2.5 cm.

Now, the magnifying power of a microscope is given by

m=\frac{v}{|u_{initial}|}(1+\frac{d}{f_{eyepiece}}) where d is the least distance of vision

so putting these values

m=\frac{10}{2.5}(1+\frac{25}{6.25}) = 20

Hence the lens can magnify the object to 20 times.

b) When image is formed at infinity

in eyepiece lens,

\frac{1}{f_{eyepiece}} = \frac{1}{v_{final}} - \frac{1}{u}

\frac{1}{6.25} = \frac{1}{infinity} - \frac{1}{u}

from here u = - 6.25., this distance from objective lens = d+u = 15 - 6.25 = 8.75 = v

in the optical lens:

\frac{1}{f_{objective}} = \frac{1}{v} - \frac{1}{u_{initial}}

\frac{1}{2} = \frac{1}{-6.25} - \frac{1}{u_{initial}}

\frac{1}{u_{initial}} =- \frac{6.75}{17.5}

u_{initial}=-2.59cm

Now,

m=\frac{v}{|u_{initial}|}(1+\frac{d}{f_{eyepiece}}) where d is the least distance of vision

putting the values, we get,


m=\frac{8.75}{2.59}(1+\frac{25}{6.25})= 13.51

Hence magnifying power, in this case, is 13.51.

Q 9.12 A person with a normal near point (25 cm) using a compound microscope with objective of focal length 8.0 mm and an eyepiece of focal length 2.5cm can bring an object placed at 9.0mm from the objective in sharp focus. What is the separation between the two lenses? Calculate the magnifying power of the microscope

Answer:

Inside a microscope,

For the eyepiece lens,

\frac{1}{f_{eyepiece}}=\frac{1}{v_{eyepiece}} - \frac{1}{u_{eyepiece}}

we are given

v_{eyepiece}= -25cm

f_{eyepiece}= 2.5cm

\frac{1}{2.5}=\frac{1}{-25} - \frac{1}{u_{eyepiece}}

\frac{1}{u_{eyepiece}}=\frac{1}{-25} - \frac{1}{2.5}=-\frac{11}{25}

u_{eyepiece}=- \frac{25}{11}=-2.27cm

we can also find this value by finding image distance in the objective lens.

So, in objective lens

\frac{1}{f_{objective}}=\frac{1}{v_{objective}} - \frac{1}{u_{objective}}

we are given

f_{objective}= 0.8

u_{objective}= -0.9

\frac{1}{0.8}=\frac{1}{v_{objective}} - \frac{1}{-0.9}

\frac{1}{v_{objective}} = \frac{0.1}{0.72}

v_{objective} = 7.2cm

Distance between object lens and eyepiece = |u_{eyepiece}| + v_{objective} = 2.27 + 7.2 = 9.47 cm.

Now,

Magnifying power :

m = \frac{v_{obejective}}{|u_{objective}|}(1+\frac{d}{f_{eyepiece}})

m = \frac{7.2}{0.9}(1+\frac{25}{2.5})= 88

Hence magnifying power for this case will be 88.

Q 9.13 A small telescope has an objective lens of focal length 144cm and an eyepiece of focal length 6.0cm. What is the magnifying power of the telescope? What is the separation between the objective and the eyepiece?

Answer:

The magnifying power of the telescope is given by

m=\frac{f_{objective}}{f_{eyepiece}}

Here, given,

focal length of objective lens = f_{objective}= 144 cm

focal length of eyepiece lens = f_{eyepiece}= 6 cm

m=\frac{f_{objective}}{f_{eyepiece}}=\frac{144}{6}=24

Hence magnifying power of the telescope is 24.

in the telescope distance between the objective and eyepiece, the lens is given by

d= f_{objective}+ f_{eyepiece}

d = 144+6=150

Therefore, the distance between the two lenses is 250 cm.

Q 9.14 (a) A giant refracting telescope at an observatory has an objective lens of focal length 15m. If an eyepiece of focal length 1.0cm is used, what is the angular magnification of the telescope?

Answer:

Angular magnification in the telescope is given by :

angular magnification = \alpha = \frac{f_{objective} }{f_{eyepiece}}

Here given,

focal length of objective length = 15m = 1500cm

the focal length of the eyepiece = 1 cm

so, angular magnification, \alpha = \frac{1500}{1}

\alpha = 1500

Q 9.14 (b) If this telescope is used to view the moon, what is the diameter of the image of the moon formed by the objective lens? The diameter of the moon is 3.48 \times 106m , and the radius of the lunar orbit is 3.8 \times 108m .

Answer:

Given,

The radius of the lunar orbit,r = 3.8 \times 108m .

The diameter of the moon,d = 3.48 \times 106m

focal length f = 15m

let d_1 be the diameter of the image of the moon which is formed by the objective lens.

Now,

the angle subtended by diameter of the moon will be equal to the angle subtended by the image,

\frac{d}{r}=\frac{d_1}{f}

\frac{3.48*10^6}{3.8*10^8}=\frac{d_1}{15}

d_1=13.74 cm

Hence the required diameter is 13.74cm.

Q 9.15 (a) Use the mirror equation to deduce that:

an object placed between f and 2f of a concave mirror produces a real image beyond 2f.

Answer:

The equation we have for a mirror is:

\frac{1}{f}=\frac{1}{v}+\frac{1}{u}

\frac{1}{u}=\frac{1}{f}-\frac{1}{v}

Given condition f<u<2f and v>2f

\frac{1}{2f}<\frac{1}{u}<\frac{1}{f} and \frac{1}{v}<\frac{1}{2f}

-\frac{1}{2f}>-\frac{1}{u}>-\frac{1}{f}

\frac{1}{f}-\frac{1}{2f}>\frac{1}{f}-\frac{1}{u}>\frac{1}{f}-\frac{1}{f}

\frac{1}{2f}>\frac{1}{v}>0

{2f}<{v}<0

Here f has to be negative in order to satisfy the equation and hence we conclude that our mirror is a concave Mirror. It also satisfies that -v>-2f (image lies beyond 2f)

Q. 9.15 (b) Use the mirror equation to deduce that:

a convex mirror always produces a virtual image independent of the location of the object.

Answer:

In a convex mirror focal length is positive conventionally.

so we have mirror equation

\frac{1}{f}=\frac{1}{u}+\frac{1}{v}

\frac{1}{v}= \frac{1}{f}-\frac{1}{u}

Here, since object distance is always negative whenever we put our object in the left side of the convex mirror(which we always do, generally). So \frac{1}{v} is always the sum of two positive quantity(negative sign in the equation and negative sign of the u will always make positive) and hence we conclude that v is always greater than zero which means the image is always on the right side of the mirror which means it is a virtual image. Therefore, a convex lens will always produce a virtual image regardless of anything.

Q 9.15 (c) Use the mirror equation to deduce that:

the virtual image produced by a convex mirror is always diminished in size and is located between the focus and the pole.

Answer:

In a convex mirror focal length is positive conventionally.

so we have mirror equation

\frac{1}{f}=\frac{1}{u}+\frac{1}{v}

\frac{1}{v}= \frac{1}{f}-\frac{1}{u}

here since f is positive and u is negative (conventionally) so we have,

\frac{1}{v}>\frac{1}{f} that is '

v<f

which means the image will always lie between pole and focus.

Now,

\frac{1}{v}= \frac{1}{f}-\frac{1}{u}=\frac{u-f}{uf}

magnification (m)=-\frac{v}{u} = \frac{f}{f-u}

here since u is always negative conventionally, it can be seen that magnification of the image will be always less than 1 and hence we conclude that image will always be diminished.

Q 9.15 (d) Use the mirror equation to deduce that:

an object placed between the pole and focus of a concave mirror produces a virtual and enlarged image.

Answer:

The focal length f of concave mirror is always negative.

Also conventionally object distance u is always negative.

So we have mirror equation:

\frac{1}{f} = \frac{1}{v}+\frac{1}{u}

\frac{1}{v}=\frac{1}{f}-\frac{1}{u}

Now in this equation whenever u<f , \frac{1}{v} will always be positive which means v is always positive which means it lies on the right side of the mirror which means image is always virtual.

Now,

m=-\frac{v}{u}=-\frac{f}{u-f}

since the denominator is always less than the numerator, so the magnitude magnification will always be greater than 1

Hence we conclude that image is always gonna be enlarged.

Hence an object placed between the pole and focus of a concave mirror produces a virtual and enlarged image.

Q 9.16 A small pin fixed on a table top is viewed from above from a distance of 50cm. By what distance would the pin appear to be raised if it is viewed from the same point through a 15cm thick glass slab held parallel to the table? Refractive index of glass = 1.5. Does the answer depend on the location of the slab?

Answer:

As we know,

Refractive index =

\frac{actualdepth}{apparentdepth}

Here actual depth = 15cm

let apparent depth be d'

And refractive index of the glass = 1.5

now putting these values, we get,

1.5 = \frac{15}{d'}

d' =10

the change in the apparent depth = 15 - 10 = 5 cm.

as long as we are not taking slab away from the line of sight of the pin, the apparent depth does not depend on the location of the slab.

Q 9.17 (a) In the following f igure shows a cross-section of a ‘light pipe’ made of a glass fibre of refractive index 1.68. The outer covering of the pipe is made of a material of refractive index 1.44. What is the range of the angles of the incident rays with the axis of the pipe for which total reflections inside the pipe take place, as shown in the figure.

1648463021447

Answer:

We are given,

Refractive index of glass( \mu _{glass} ) and outer covering( \mu _{outerlayer} ) is 1.68 and 1.44 respectively.

Now applying snell's law on upper glass - outer layer,

\mu _{glass}sini'=\mu_{outerlayer}sin90

i' = the angle from where total Internal reflection starts

sini'=\frac{\mu_{outerlayer}}{\mu_{glass}} = \frac{1.44}{1.68}=0.8571

i' = 59^0

At this angle, in the air-glass interface

Refraction angle r = 90 - 59 = 31 degree

let Incident Angle be i .

Applying Snell's law

1sini=\mu_{glass}sinr

sini=1.68sin31=0.8652

i=60 (approx)

Hence total range of incident angle for which total internal reflection happen is 0<i<60

Q 9.17 (b) What is the answer if there is no outer covering of the pipe?

Answer:

In the case when there is no outer layer,

Snell's law at glass-air interface(when the ray is emerging out from the pipe)

\mu _{glass}sini'=1sin90

sini'=\frac{1}{\mu_{glass}} = \frac{1}{1.68}=0.595

i'= 36.5

refractive angle r corresponding to this = 90 - 36.5 = 53.5.

the angle r is greater than the critical angle

So for all of the incident angles, the rays will get total internally reflected.in other words, rays won't bend in air-glass interference, it would rather hit the glass-air interference and get reflected

Q 9.18 (a) Answer the following question:

You have learned that plane and convex mirrors produce virtual images of objects. Can they produce real images under some circumstances? Explain.

Answer:

If our object is virtual then Plane and convex mirrors can produce a real image. That is, when the light coming from infinity goes into the convex mirror, it creates a virtual object behind the convex mirror. the reflection of this virtual object in the convex mirror can be taken out on screen and hence convex mirror can make a real image.

Q 9.18 (b) Answer the following question:

A virtual image, we always say, cannot be caught on a screen. Yet when we ‘see’ a virtual image, we are obviously bringing it on to the ‘screen’ (i.e., the retina) of our eye. Is there a contradiction?

Answer:

No, there is no contradiction. A virtual image is formed whenever the light rays are diverging. We have a convex lens in our eye. This convex lens converges the diverging rays into our retina and forms a real image. In other words, the virtual image acts as an object to the convex lens of our eye to form a real image, which we see on the screen called retina.

Q 9.18 (c) Answer the following question:

A diver underwater looks obliquely at a fisherman standing on the bank of a lake. Would the fisherman look taller or shorter to the diver than what he actually is?

Answer:

The diver is in denser medium (water) and fisherman is in lighter medium (air). As the diver is looking at the fisherman, rays of light will go from fisherman to divers eye, that is, from lighter medium to denser medium. Since rays deflect toward normal when it goes form lighter to a denser medium, the fisherman will look taller than actual to the diver.

Q 9.18 (d) Answer the following question:

Does the apparent depth of a tank of water change if viewed obliquely? If so, does the apparent depth increase or decrease?

Answer:

Yes, appearing depth of water will decrease when we view obliquely, this happens because of the fact that light bends from its direction whenever it goes from one medium to another medium.

Q 9.18 (e) Answer the following question:

The refractive index of diamond is much greater than that of ordinary glass. Is this fact of some use to a diamond cutter?

Answer:

We use diamond as a cutter because it is very hard and sharp. The refractive index is high in diamond ensures that light goes through multiple total internal reflections so that light goes in all direction. This is the reason behind the shining of the diamond. Light entering is totally reflected from faces before it getting out, hence producing a sparkling effect

Q 9.19 The image of a small electric bulb fixed on the wall of a room is to be obtained on the opposite wall 3m away by means of a large convex lens. What is the maximum possible focal length of the lens required for the purpose?

Answer:

As we know for real image, the maximum focal length is given by

f_{max}= \frac{d}{4}

where d is the distance between the object and the lens.

So putting values we get,

f_{max}= \frac{3}{4}=0.75

Hence maximum focal length required is 0.75.

Q 9.20 A screen is placed 90cm from an object. The image of the object on the screen is formed by a convex lens at two different locations separated by 20cm. Determine the focal length of the lens.

Answer:

As we know that the relation between focal length f , the distance between screen D and distance between two locations of the object d is :

f=\frac{D^2-d^2}{4D}

Given: D = 90 cm., d = 20 cm ,

so

f=\frac{90^2-20^2}{4*90}

f=\frac{90^2-20^2}{4*90}=\frac{770}{36}=21.39cm

Hence the focal length of the convex lens is 21.39 cm.

Q 9.21 (a) Determine the ‘effective focal length’ of the combination of the two lenses in Exercise 9.10, if they are placed 8.0cm apart with their principal axes coincident. Does the answer depend on which side of the combination a beam of parallel light is incident? Is the notion of effective focal length of this system useful at all?

Answer:

Here there are two cases, first one is the one when we see it from convex side i.e. Light are coming form infinite and going into convex lens first and then goes to concave lens afterwords. The second case is a just reverse of the first case i.e. light rays are going in concave first.

1)When light is incident on convex lens first

\frac{1}{f}=\frac{1}{v}-\frac{1}{u}

\frac{1}{v}=\frac{1}{f}+\frac{1}{u}

\frac{1}{v}=\frac{1}{30}+\frac{1}{infinite}

v= 30cm

Now this will act as an object for the concave lens.

\frac{1}{f_{concave}}=\frac{1}{v_{fromconcave}}-\frac{1}{u_{fromconcave}}

u_{fromconcave}= 30 - 8 = 22 cm

\frac{1}{-20}=\frac{1}{v_{fromconcave}}-\frac{1}{22}

\frac{1}{v}=-\frac{1}{220}

v = -220cm

Hence parallel beam of rays will diverge from this point which is (220 - 4 = 216) cm away from the centre of the two lenses.

2) When rays fall on the concave lens first

\frac{1}{f}=\frac{1}{v}-\frac{1}{u}

\frac{1}{v}=\frac{1}{f}+\frac{1}{u}

\frac{1}{v}=\frac{1}{-20}+\frac{1}{infinite}

v=-20cm

Now this will act as an object for convex lens.

\frac{1}{f_{convex}}=\frac{1}{v_{fromconvex}}-\frac{1}{u_{fromconvex}}

u_{fromconvex}= -20-8=-28cm

\frac{1}{-30}=\frac{1}{v_{fromconvex}}-\frac{1}{-28}

\frac{1}{v_{fromconvex}}=\frac{1}{30}-\frac{1}{28}=-\frac{1}{420}

v_{fromconvex}= -420cm

Hence parallel beam will diverge from this point which is (420 - 4 = 146 cm ) away from the centre of two lenses.

As we have seen for both cases we have different answers so Yes, answer depend on the side of incidence when we talk about combining lenses. i .e. we can not use the effective focal length concept here.

Q 9.21 (b) An object 1.5 cm in size is placed on the side of the convex lens in the arrangement (a) above. The distance between the object and the convex lens is 40cm. Determine the magnification produced by the two-lens system, and the size of the image.

Answer:

Given

Object height = 1.5 cm

Object distance from convex lens = -40cm

According to lens formula

\frac{1}{v_{fromconvex}}=\frac{1}{f_{convex}}+\frac{1}{u_{fromconvex}}

\frac{1}{v_{fromconvex}}=\frac{1}{30}+\frac{1}{-40}=\frac{1}{120}

v_{fromconvex}=120

Magnificatio due to convex lens:

m_{convex}=-\frac{v}{u}=-\frac{120}{-40}=3

The image of convex lens will act as an object for concave lens,

so,

\frac{1}{v_{fromconcave}}=\frac{1}{f_{concave}}+\frac{1}{u_{fromconcave}}

u_{concave}= 120 - 8 = 112

\frac{1}{v_{fromconcave}}= \frac{1}{-20}+\frac{1}{112}

\frac{1}{v_{fromconcave}}= -\frac{92}{2240}

v_{fromconcave}= \frac{-2240}{92}

Magnification due to concave lens :

m_{concave}= \frac{2240}{92}*\frac{1}{112}=\frac{20}{92}

The combined magnification:

m_{combined}=m_{convex}*m_{concave}

m_{combined}=3*\frac{20}{92}=0.652

Hence height of the image = m_{combined}*h

= 0.652 * 1.5 = 0.98cm

Hence height of image is 0.98cm.

Q 9.22 At what angle should a ray of light be incident on the face of a prism of refracting angle 60^{\circ} so that it just suffers total internal reflection at the other face? The refractive index of the material of the prism is 1.524 .

Answer:

Let prism be ABC ,

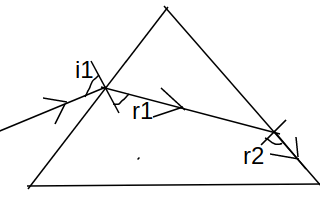
1648463067266

as emergent angle e=90^0 ,

\mu_{glass}sinr_2 = 1sin90

sinr_2=\frac{1}{1.524} = 0.6562

r_2= 41 ^0 (approx)

Now as we know in the prism

r_1+r_2=A

Hence, r_1+=A-r_2=60-41=19^0

Now applying snells law at surface AB

1sini=\mu _{glass}sinr_1

sini=1.524sin19

sini=0.496

i = 29.75^0

Hence the angle of incident is 29.75 degree.

Q 9.23 A card sheet divided into squares each of size 1 mm 2 is being viewed at a distance of 9 cm through a magnifying glass (a converging lens of focal length 10 cm) held close to the eye.

(a) What is the magnification produced by the lens? How much is the area of each square in the virtual image?

(b) What is the angular magnification (magnifying power) of the lens?

(c) Is the magnification in (a) equal to the magnifying power in (b)? Explain.

Answer:

Given,

Object distance u = -9cm

Focal length of convex lens = 10cm

According to the lens formula

\frac{1}{f}=\frac{1}{v}-\frac{1}{u}

\frac{1}{10}=\frac{1}{v}-\frac{1}{-9}

\\\frac{1}{v}=\frac{1}{10}-\frac{1}{9}\\\Rightarrow v=-90\ cm

a) Magnification

m=\frac{v}{u}=\frac{-90}{9}=10 \ cm

The area of each square in the virtual image

=10\times10\times1=100mm^2=1cm^2

b) Magnifying power

=\frac{d}{|u|}=\frac{25}{9}=2.8

c) No,

magnification = \frac{v}{u}

magnifying\ power = \frac{d}{|u|} .

Both the quantities will be equal only when image is located at the near point |v| = 25 cm

Q 9.24 (a) At what distance should the lens be held from the figure in Exercise 9.29 in order to view the squares distinctly with the maximum possible magnifying power?

(b) What is the magnification in this case?

(c) Is the magnification equal to the magnifying power in this case?

Answer:

a)

maximum magnifying is possible when our image distance will be equal to minimum vision point that is,

v=-25

f = 10cm (Given)

Now according to the lens formula

\frac{1}{f}=\frac{1}{v}-\frac{1}{u}

\frac{1}{u}=\frac{1}{v}-\frac{1}{f}

\frac{1}{u}=\frac{1}{-25}-\frac{1}{10}

\frac{1}{u}=-\frac{7}{50}

u=-\frac{50}{7}=-7.14cm

Hence required object distance for viewing squares distinctly is 7.14 cm away from the lens.

b)

Magnification of the lens:

m = \left | \frac{v}{u} \right |=\frac{25}{50}*7= 3.5

c)

Magnifying power

M = \frac{d}{u} =\frac{25}{50}*7= 3.5

Since the image is forming at near point ( d = 25 cm ), both magnifying power and magnification are same.

Q 9.25 What should be the distance between the object in figure 9.23 and the magnifying glass if the virtual image of each square in the figure is to have an area of 6.25 mm 2 . Would you be able to see the squares distinctly with your eyes very close to the magnifier?

Answer:

Given

Virtual image area = 6.25 mm 2

Actual ara = 1 mm 2

We can calculate linear magnification as

m=\sqrt{\frac{6.25}{1}}=2.5

we also know

m=\frac{v}{u}

v = mu

Now, according to the lens formula

\frac{1}{f}=\frac{1}{v}-\frac{1}{u}

\frac{1}{10}=\frac{1}{mu}-\frac{1}{u}

\frac{1}{u}(\frac{1}{2.5}-1)=\frac{1}{10}

u=-6cm and

v=mu=2.5*(-6)=-15cm

Since the image is forming at a distance which is less than 25 cm, it can not be seen by eye distinctively.

Answer the question

Q 9.26 (a) The angle subtended at the eye by an object is equal to the angle subtended at the eye by the virtual image produced by a magnifying glass. In what sense then does a magnifying glass provide angular magnification?

Answer:

Angular magnification is the ratio of tangents of the angle formed by object and image from the centre point of the lens. In this question angle formed by the object and a virtual image is same but it provides magnification in a way that, whenever we have object place before 25cm, the lens magnifies it and make it in the vision range. By using magnification we can put the object closer to the eye and still can see it which we couldn't have without magnification.

Answer the question

Q 9.26. (b) In viewing through a magnifying glass, one usually positions one’s eyes very close to the lens. Does angular magnification change if the eye is moved back?

Answer:

Yes, angular magnification will change if we move our eye away from the lens. this is because then angle subtended by lens would be different than the angle subtended by eye. When we move our eye form lens, angular magnification decreases. Also, one more important point here is that object distance does not have any effect on angular magnification.

Answer the question?

Q 9.26 (c) Magnifying power of a simple microscope is inversely proportional to the focal length of the lens. What then stops us from using a convex lens of smaller and smaller focal length and achieving greater and greater magnifying power?

Answer:

Firstly, grinding a lens with very small focal length is not easy and secondly and more importantly, when we reduce the focal length of a lens, spherical and chronic aberration becomes more noticeable. they both are defects of the image, resulting from the ways of rays of light.

Q 9.26 Answer the Question?

(d) Why must both the objective and the eyepiece of a compound microscope have short focal lengths?

Answer:

We need more magnifying power and angular magnifying power in a microscope in order to use it effectively. Keeping both objective focal length and eyepiece focal length small makes the magnifying power greater and more effective.

Answer the Question?

Q 9.26 (e) When viewing through a compound microscope, our eyes should be positioned not on the eyepiece but a short distance away from it for best viewing. Why? How much should be that short distance between the eye and eyepiece?

Answer:

When we view through a compound microscope, our eyes should be positioned a short distance away from the eyepiece lens for seeing a clearer image. The image of the objective lens in the eyepiece lens is the position for best viewing. It is also called "eye-ring" and all reflected rays from lens pass through it which makes it the ideal position for the eye for the best view.

When we put our eyes too close to the eyepiece lens, then we catch the lesser refracted rays from eyes, i.e. we reduce our field of view because of which the clarity of the image gets affected.

Q9.27 An angular magnification (magnifying power) of 30X is desired using an objective of focal length 1.25cm and an eyepiece of focal length 5cm. How will you set up the compound microscope?

Answer:

Given,

magnifying power = 30

objective lens focal length

f_{objeective} = 1.25cm

eyepiece lens focal length

f_{eyepiece} = 5 cm

Normally, image is formed at distance d = 25cm

Now, by the formula;

Angular magnification by eyepiece:

m_{eyepiece}=1+\frac{d}{f_{eyepiece}}=1+\frac{25}{5}=6

From here, magnification by the objective lens :

m_{objective}=\frac{30}{6}=5 since ( m_{objective}*m_{eyepiece}=m_{total} )

m_{objective}=-\frac{v}{u}=5

v=-5u

According to the lens formula:

\frac{1}{f}=\frac{1}{v}-\frac{1}{u}

\frac{1}{1.25}=\frac{1}{-5u}-\frac{1}{u}

from here,

u = -1.5cm

hence object must be 1.5 cm away from the objective lens.

v= -mu=(-1.5)(5)=7.5

Now for the eyepiece lens:

\frac{1}{f}=\frac{1}{v}-\frac{1}{u}

\frac{1}{5}=\frac{1}{-25}-\frac{1}{u}

\frac{1}{u}=-\frac{6}{25}

u = -4.17 cm

Hence the object is 4.17 cm away from the eyepiece lens.

The separation between objective and eyepiece lens

u_{eyepiece} +v_{objectivve}=4.17 + 5.7 = 11.67 cm

Q 9.28 (a) A small telescope has an objective lens of focal length 140cm and an eyepiece of focal length 5.0cm. What is the magnifying power of the telescope for viewing distant objects when
(a) the telescope is in normal adjustment (i.e., when the final image is at infinity)?

Answer:

Given,

the focal length of the objective lens f_{objective}=140cm

the focal length of the eyepiece lens f_{eyepiece}=5cm

normally, least distance of vision = 25cm

Now,

As we know magnifying power:

m = \frac{f_{objective}}{f_{eyepiece}}=\frac{140}{5}=28

Hence magnifying power is 28.

Q 9.28 (b) A small telescope has an objective lens of focal length 140cm and an eyepiece of focal length 5.0cm. What is the magnifying power of the telescope for viewing distant objects when

(b) the final image is formed at the least distance of distinct vision (25cm)?

Answer:

Given,

the focal length of the objective lens f_{objective}=140cm

the focal length of the eyepiece lens f_{eyepiece}=5cm

normally, least distance of vision = 25cm

Now,

as we know magnifying power when the image is at d = 25 cm is

m=\frac{f_{objective}}{f_{eyepiece}}(1+\frac{f_{eyepiece}}{d})=\frac{140}{5}(1+\frac{5}{25}) = 33.6

Hence magnification, in this case, is 33.6.

Q 9.29 (a) For the telescope described in Exercise 9.28 (a), what is the separation between the objective lens and the eyepiece?

Answer:

a) Given,

focal length of the objective lens = f_{objective} = 140cm

focal length of the eyepiece lens = f_{eyepiece} = 5 cm

The separation between the objective lens and eyepiece lens is given by:

f_{eyepiece}+f_{objective}=140+5=145cm

Hence, under normal adjustment separation between two lenses of the telescope is 145 cm.

Q 9.29 (b) If this telescope is used to view a 100 m tall tower 3 km away, what is the height of the image of the tower formed by the objective lens?

Answer:

Given,

focal length of the objectove lens = f_{objective} = 140cm

focal length of the eyepiece lens = f_{eyepiece} = 5 cm

Height of tower h_{tower} = 100m

Distance of object which is acting like a object u = 3km = 3000m.

The angle subtended by the tower at the telescope

tan\theta=\frac{h_{tower}}{u}=\frac{100}{3000}=\frac{1}{30}

Now, let the height of the image of the tower by the objective lens is h_{image} .

angle made by the image by the objective lens :

tan\theta'=\frac{h_{image}}{f_{objective}}=\frac{h_{image}}{140}

Since both, the angles are the same we have,

tan\theta=tan\theta'

\frac{1}{30}=\frac{h_{image}}{140}

h_{image}= \frac{140}{30}=4.7cm

Hence the height of the image of the tower formed by the objective lens is 4.7 cm.

Q 9.29 (c) What is the height of the final image of the tower if it is formed at 25cm

Answer:

Given, image is formed at a distance d = 25cm

As we know, magnification of eyepiece lens is given by :

m=1+\frac{d}{f_{eyepiece}}

m=1+\frac{25}{5}=6

Now,

Height of the final image is given by :

h_{image}= mh_{object}= 6*4.7 = 28.2cm

Therefore, the height of the final image will be 28.2 cm

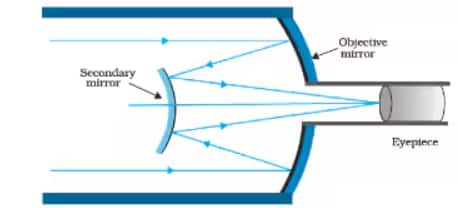
Q9.30 A Cassegrain telescope uses two mirrors as shown in Fig. 9.30. Such a telescope is built with the mirrors 20mm apart. If the radius of curvature of the large mirror is 220mm and the small mirror is 140mm, where will the final image of an object at infinity be?

15946203088301594620302945

Answer:

Given,

Distance between the objective mirror and secondary mirror d= 20mm

The radius of curvature of the Objective Mirror

R_{objective}=220mm

So the focal length of the objective mirror

f_{objective}=\frac{220}{2}=110mm

The radius of curvature of the secondary mirror

R_{secondary}=140mm

so, the focal length of the secondary mirror

f_{secondary}=\frac{140}{2}=70mm

The image of an object which is placed at infinity, in the objective mirror, will behave like a virtual object for the secondary mirror.

So, virtual object distance for the secondary mirror

u_{secondary}=f_{objective}-d=110-20=90mm

Now, applying the mirror formula in the secondary mirror:

\frac{1}{f}=\frac{1}{v}+\frac{1}{u}

\frac{1}{v}=\frac{1}{f}-\frac{1}{u}

\frac{1}{v}=\frac{1}{70}-\frac{1}{90}

v=315mm

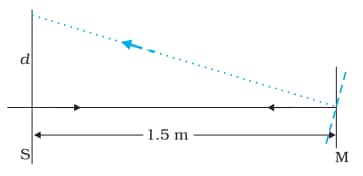
Q 9.31 Light incident normally on a plane mirror attached to a galvanometer coil retraces backwards as shown in Fig. 9.33. A current in the coil produces a deflection of 3.5 o of the mirror. What is the displacement of the reflected spot of light on a screen placed 1.5 m away?

15946204708881594620467004

Answer:

Given

Angle of deflection \delta = 3.5^0

The distance of the screen from the mirror D=1.5m

The reflected rays will bet deflected by twice angle of deviation that is

2\delta =3.5*2=7^0

Now from the figure, it can be seen that

tan2\delta =\frac{d}{1.5}

d=1.5*\tan2\delta = 2*\tan7=0.184m=18.4cm

Hence displacement of the reflected spot of the light is 18.4cm .

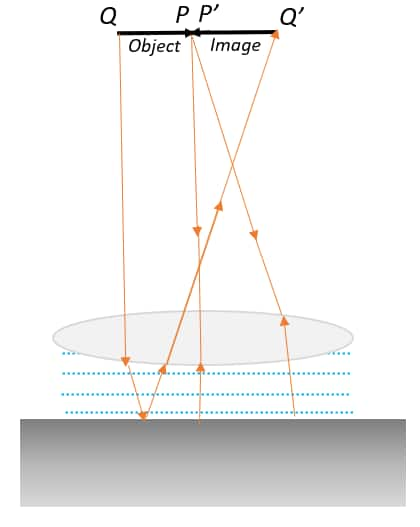
Q 9.32 Figure 9.34 shows an equiconvex lens (of refractive index 1.50) in contact with a liquid layer on top of a plane mirror. A small needle with its tip on the principal axis is moved along the axis until its inverted image is found at the position of the needle. The distance of the needle from the lens is measured to be 45.0cm. The liquid is removed and the experiment is repeated. The new distance is measured to be 30.0cm. What is the refractive index of the liquid?


15946205854861594620581502

Answer:

Given

The focal length of the convex lens f_{convex}=30cm

here liquid is acting like the mirror so,

the focal length of the liquid =f_{liquid}

the focal length of the system(convex + liquid) f_{system}=45cm

Equivalent focal length when two optical systems are in contact

\frac{1}{f_{system}}=\frac{1}{f_{convex}}+\frac{1}{f_{liquid}}

\frac{1}{f_{liquid}}=\frac{1}{f_{system}}-\frac{1}{f_{convex}}

\frac{1}{f_{liquid}}=\frac{1}{45}-\frac{1}{30}=-\frac{1}{90}

f_{liquid}=-90cm


Now, let us assume refractive index of the lens be \mu _{lens}

The radius of curvature are R and -R .

As we know,

\frac{1}{f_{convex}}=(\mu _{lens}-1)\left ( \frac{1}{R}-\frac{1}{-R} \right )

\frac{1}{f_{convex}}=(\mu _{lens}-1)\frac{2}{R}

R=2(\mu _{lens-1})f_{convex}=2(1.5-1)30=30cm

Now, let refractive index of liquid be \mu _{liquid}

The radius of curvature of liquid in plane mirror side = infinite

Radius of curvature of liquid in lens side R = -30cm

As we know,

\frac{1}{f_{liquid}}=(\mu_{liquid-1})\left ( \frac{1}{R}-\frac{1}{infinite} \right )

-\frac{1}{90}=(\mu_{liquid}-1)(\frac{1}{30})

\mu_{liquid}= 1+\frac{1}{3}

\mu_{liquid}= 1.33

Therefore the refractive index of the liquid is 1.33.

NCERT solutions for class 12 physics chapter 9 ray optics and optical instruments- Important formulas:

Formula

Description

Mirror equation:

1/v+1/u=1/f

v is object distance from the pole of the mirror.

u is the image distance from the pole of the mirror.

F is the focal length of the mirror which is approximately equal to half the radius of curvature

Magnification of a mirror

m= \frac{h_{i}}{h_{o}}= \frac{-v}{u}

h_{i}= height of the image from the principal axis

h_{o}= height of the object from the principal axis

If a prism of refractive index n2is placed in a medium of refractive index n1then

\boldsymbol{\frac{n_2}{n_1} = \frac{\sin \left ( \frac{D _{m}+A}{2} \right )}{\sin \left ( A/2 \right )}}

A is the angle of the prism

Dm is the angle of minimum deviation

Thin lens formula

\frac{1}{v}-\frac{1}{u}= \frac{1}{f}

Lens makers formula

\boldsymbol{\frac{1}{f}= \left ( \frac{\mu _{2}}{\mu _{1}}-1 \right )\left ( \frac{1}{R_{1}}- \frac{1}{R_{2}}\right )}

v, u are image and object distance from the optical centre of the lens and f is the focal length

\mu _{1}= the refractive index of the medium of the object

\mu _{2}= the refractive index of the lens

R_{1}\, and \, R_{2} are the radius of curvature of two surface

Power of a lens

\boldsymbol{P=\frac{1}{f}}

Where P is the power and f is the focal length in meters

The magnifying power of a simple microscope m=1+ \frac{D}{f}

For compound microscope magnification m=m_e \times m_0

D = 25 cm is the least distance of distinct vision and f is the focal length of the convex lens

m_e is magnification due to the eyepiece.

m_o is magnification produced by objective.

For a telescope magnifying power is given by

m=\frac{\beta}{\alpha}=\frac{f_o}{f_e}

Beta is the angle subtended by eyepiece at the image

Alpha is the angle subtended at the eye by the object

JEE Main Highest Scoring Chapters & Topics
Just Study 40% Syllabus and Score upto 100%
Download EBook

The Class 12 ch 9 is an important chapter for the CBSE board exam as 10 to 14% of questions in Physics can be expected from the unit optics. 32 questions are covered in the Ray Optics Class 12 NCERT solutions. Ray Optics Class 12 NCERT solutions covers the major formulas discussed in Class 12 NCERT Physics chapter 9. The solutions for the following NCERT Class Physics chapters are also provided by careers360. Click on the link to read the solutions. The Ray Optics solutions can also be downloaded in PDF format.

NCERT solutions for class 12 physics chapter wise

NCERT solutions for class 12 physics chapter 1 Electric Charges and Fields
NCERT solutions for class 12 physics chapter 2 Electrostatic Potential and Capacitance
NCERT solutions for class 12 physics chapter 3 Current Electricity
NCERT solutions for class 12 physics chapter 4 Moving Charges and Magnetism
NCERT solutions for class 12 physics chapter 5 Magnetism and Matter
NCERT solutions for class 12 physics chapter 6 Electromagnetic Induction
NCERT solutions for class 12 physics chapter 7 Alternating Current
NCERT solutions for class 12 physics chapter8 Electromagnetic Waves
NCERT solutions for class 12 physics chapter 9 Ray Optics and Optical Instruments
NCERT solutions for class 12 physics chapter 10 Wave Optics Solutions
NCERT solutions for class 12 physics chapter 11 Dual nature of radiation and matter
NCERT solutions for class 12 physics chapter 12 Atoms
NCERT solutions for class 12 physics chapter 13 Nuclei
NCERT solutions for class 12 physics chapter 14 Semiconductor Electronics Materials Devices and Simple Circuits

Also Check NCERT Books and NCERT Syllabus here:

NCERT Solutions Class 12 Physics Ray Optics: Important Formulas and Diagrams

  • Mirror Formula

1/v+1/u=1/f

Where:-

u is the distance of the object measured from its pole

v is the distance of the image measured from the pole of the mirror

f is the distance of the principal focus measured from the pole

  • Magnification of a mirror

m= \frac{h_{i}}{h_{o}}= \frac{-v}{u}

Where:

hi= height of the image from the principal axis

ho= height of the object from the principal axis

** If a prism of refractive index n2 is placed in a medium of refractive index n1then

\boldsymbol{\frac{n_2}{n_1} = \frac{\sin \left ( \frac{D _{m}+A}{2} \right )}{\sin \left ( A/2 \right )}}

Where: A is the angle of the prism and Dm is the angle of minimum deviation

  • Thin lens formula

1694405359233

Lens makers formula

1694405357632

Where: v, u are image and object distance from the optical centre of the lens and f is the focal length

μ1= the refractive index of the medium of the object

μ2= the refractive index of the lens

R1 andR2 are the radius of curvature of two surface

  • Power of a lens

\boldsymbol{P=\frac{1}{f}}

The magnifying power of a simple microscope

m=1+ \frac{D}{f}

For compound microscope magnification m=m_e \times m_0

Where: D = 25 cm is the least distance of distinct vision and f is the focal length of the convex lens, me is magnification due to the eyepiece and mo is magnification produced by objective.

For a telescope magnifying power is given by

m=\frac{\beta}{\alpha}=\frac{f_o}{f_e}

Where: Beta is the angle subtended by the eyepiece at the image and Alpha is the angle subtended at the eye by the object

Topics Covered in Class 12 Physics Chapter Ray Optics

The mirror formula, lens formula, lens makers formula and their applications to solve numerical problems are discussed in the NCERT Class 12 Physics book chapter 9. Ray Optics and Optical Instruments also Covers the topics of optical instruments like microscope and telescope. The questions related to the concepts of refraction through prism are also covered the NCERT solutions for Class 12 Physics chapter 9 Ray Optics and Optical Instruments. The chapter Ray Optics also covers the concepts of totlal internal reflection and some applications of reflection and refraction that can be seen in the nature such as rainbow and marriage.

Importance of NCERT solutions for class 12 physics chapter 9 ray optics and optical instruments:

The NCERT solutions for class 12 physics are important in the CBSE 12th exams since on average 12% of questions are asked in the board exam from NCERT Physics class 12 chapter 9. For exams like NEET and JEE Mains, from the unit optics, 2 to 3 questions are expected. Learning the NCERT class 12 Physics chapter 9 solutions can be helpful to solve questions from other reference books also.

Key features of Cbse Class 12 Ray Optics NCERT Solutions

  1. Comprehensive Coverage: These ncert solutions class 12 physics chapter 9 encompass all the topics and questions presented in Chapter 9, ensuring a thorough understanding of ray optics and optical instruments.

  2. Detailed Explanations: Each class 12 physics chapter ray optics ncert solutions offers detailed step-by-step explanations, simplifying complex concepts for students.

  3. Clarity and Simplicity: The ray optics class 12 ncert solutions pdf download are presented in clear and simple language, ensuring ease of understanding.

  4. Practice Questions: Exercise questions are included for practice and self-assessment.

  5. Exam Preparation: These ncert solutions class 12 physics ray optics are essential for board exam preparation and provide valuable support for competitive exams.

NCERT solutions subject wise

NCERT Exemplar Class 12 Solutions

Frequently Asked Questions (FAQs)

1. What is the weightage of the ray optics for CBSE board exam

From the NCERT chapter ray optics, 6 to 9 marks questions are asked for CBSE board exam

2. What type of questions are asked from optics for board exam

The questions may be a numerical, theory or derivation. You may get all these three types of questions from ray optics in a single paper

3. Is the chapter ray optics and optical instrument important for JEE Main and NEET

Yes this chapter is important for JEE Main and NEET exam. One question for JEE main and 2 questions for NEET can be expected

4. According to ray optics class 12 ncert solutions Physics, what is refraction?

Refraction is the bending of light as it passes through a medium with a different refractive index. This occurs because light travels at different speeds in different materials, causing the light to change direction as it enters or exits a medium.

5. Where can I find the class 12 physics chapter 9 ncert solutions exercise-by-exercise answers?

The right study materials should be chosen by Class 12 students in order to encourage efficient textbook problem-solving. Finding the best reference book from the many available on the market demands for a lot of patience. In Careers360 the answers to the chapter- and exercise-specific issues are given in PDF format. Students can use it to quickly dispel their doubts while working through challenges.

Also Read

Articles

Upcoming School Exams

Himachal Pradesh Board of Secondary Education 12th Examination

Application Date:09 September,2024 - 14 November,2024

Himachal Pradesh Board of Secondary Education 10th Examination

Application Date:09 September,2024 - 14 November,2024

Maharashtra Higher Secondary School Certificate Examination

Application Date:01 October,2024 - 30 October,2024

Maharashtra School Leaving Certificate Examination

Application Date:01 October,2024 - 30 October,2024

National Institute of Open Schooling 10th examination

Admit Card Date:04 October,2024 - 29 November,2024

View All School Exams

Certifications By Top Providers

Edx
 1113 courses
Coursera
 812 courses
Udemy
 394 courses
Futurelearn
 295 courses
Harvard University, Cambridge
 98 courses
IBM
 83 courses

Explore Top Universities Across Globe

University of Essex, Colchester
  Wivenhoe Park Colchester CO4 3SQ
University College London, London
  Gower Street, London, WC1E 6BT
The University of Edinburgh, Edinburgh
  Old College, South Bridge, Edinburgh, Post Code EH8 9YL
University of Bristol, Bristol
  Beacon House, Queens Road, Bristol, BS8 1QU
University of Delaware, Newark
  Newark, DE 19716
University of Nottingham, Nottingham
  University Park, Nottingham NG7 2RD

Related E-books & Sample Papers

Questions related to CBSE Class 12th

Have a question related to CBSE Class 12th ?
quartely question paper for mathematics cbse

Hello there! Thanks for reaching out to us at Careers360.

Ah, you're looking for CBSE quarterly question papers for mathematics, right? Those can be super helpful for exam prep.

Unfortunately, CBSE doesn't officially release quarterly papers - they mainly put out sample papers and previous years' board exam papers. But don't worry, there are still some good options to help you practice!

Have you checked out the CBSE sample papers on their official website? Those are usually pretty close to the actual exam format. You could also look into previous years' board exam papers - they're great for getting a feel for the types of questions that might come up.

If you're after more practice material, some textbook publishers release their own mock papers which can be useful too.

Let me know if you need any other tips for your math prep. Good luck with your studies!

Read Complete Answer
Jefferson 25 September,2024
i have taken admission in clg after my campartment exam in chemistry along with the neet 2025 preperation but unfortunately i again got campartment after doing all this preparation i got 15 marks only now I can't able to think what should I do next.

It's understandable to feel disheartened after facing a compartment exam, especially when you've invested significant effort. However, it's important to remember that setbacks are a part of life, and they can be opportunities for growth.

Possible steps:

  1. Re-evaluate Your Study Strategies:

    • Identify Weak Areas: Pinpoint the specific topics or concepts that caused difficulties.
    • Seek Clarification: Reach out to teachers, tutors, or online resources for additional explanations.
    • Practice Regularly: Consistent practice is key to mastering chemistry.

  2. Consider Professional Help:

    • Tutoring: A tutor can provide personalized guidance and support.
    • Counseling: If you're feeling overwhelmed or unsure about your path, counseling can help.

  3. Explore Alternative Options:

    • Retake the Exam: If you're confident in your ability to improve, consider retaking the chemistry compartment exam.
    • Change Course: If you're not interested in pursuing chemistry further, explore other academic options that align with your interests.

  4. Focus on NEET 2025 Preparation:

    • Stay Dedicated: Continue your NEET preparation with renewed determination.
    • Utilize Resources: Make use of study materials, online courses, and mock tests.

  5. Seek Support:

    • Talk to Friends and Family: Sharing your feelings can provide comfort and encouragement.
    • Join Study Groups: Collaborating with peers can create a supportive learning environment.

Remember: This is a temporary setback. With the right approach and perseverance, you can overcome this challenge and achieve your goals.

I hope this information helps you.







Read Complete Answer
Kanishka kaushikii 17 September,2024
i scored 84 percent in cbse 2 years ago.Am i eligible for medhavi scholarship if give 12th again after 2 drop years and will got 75+ in mpboard

Hi,

Qualifications:
Age: As of the last registration date, you must be between the ages of 16 and 40.
Qualification: You must have graduated from an accredited board or at least passed the tenth grade. Higher qualifications are also accepted, such as a diploma, postgraduate degree, graduation, or 11th or 12th grade.
How to Apply:
Get the Medhavi app by visiting the Google Play Store.
Register: In the app, create an account.
Examine Notification: Examine the comprehensive notification on the scholarship examination.
Sign up to Take the Test: Finish the app's registration process.
Examine: The Medhavi app allows you to take the exam from the comfort of your home.
Get Results: In just two days, the results are made public.
Verification of Documents: Provide the required paperwork and bank account information for validation.
Get Scholarship: Following a successful verification process, the scholarship will be given. You need to have at least passed the 10th grade/matriculation scholarship amount will be transferred directly to your bank account.

Scholarship Details:

Type A: For candidates scoring 60% or above in the exam.

Type B: For candidates scoring between 50% and 60%.

Type C: For candidates scoring between 40% and 50%.

Cash Scholarship:

Scholarships can range from Rs. 2,000 to Rs. 18,000 per month, depending on the marks obtained and the type of scholarship exam (SAKSHAM, SWABHIMAN, SAMADHAN, etc.).

Since you already have a 12th grade qualification with 84%, you meet the qualification criteria and are eligible to apply for the Medhavi Scholarship exam. Make sure to prepare well for the exam to maximize your chances of receiving a higher scholarship.

Hope you find this useful!

Read Complete Answer
Yuvan 30 August,2024
i upload 12th board result screenshot in ncweb form from the cbse site so it is valid

hello mahima,

If you have uploaded screenshot of your 12th board result taken from CBSE official website,there won,t be a problem with that.If the screenshot that you have uploaded is clear and legible. It should display your name, roll number, marks obtained, and any other relevant details in a readable forma.ALSO, the screenshot clearly show it is from the official CBSE results portal.

hope this helps.

Read Complete Answer
Ashvadha 12 July,2024
Kya mujhe class 12 ka important question milega jo exam mai per year puche jate hai

Hello Akash,

If you are looking for important questions of class 12th then I would like to suggest you to go with previous year questions of that particular board. You can go with last 5-10 years of PYQs so and after going through all the questions you will have a clear idea about the type and level of questions that are being asked and it will help you to boost your class 12th board preparation.

You can get the Previous Year Questions (PYQs) on the official website of the respective board.

I hope this answer helps you. If you have more queries then feel free to share your questions with us we will be happy to assist you.

Thank you and wishing you all the best for your bright future.

Read Complete Answer
kumardurgesh1802 10 July,2024

Popular CBSE Class 12th Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1)

0.34\; J

 Option 2)

0.16\; J
Option 3)

1.00\; J

 Option 4)

0.67\; J
Read More

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times.  Assume that the potential energy lost each time he lowers the mass is dissipated.  How much fat will he use up considering the work done only when the weight is lifted up ?  Fat supplies 3.8×107 J of energy per kg which is converted to mechanical energy with a 20% efficiency rate.  Take g = 9.8 ms−2 :

Option 1)

2.45×10−3 kg

 Option 2)

 6.45×10−3 kg
Option 3)

 9.89×10−3 kg

 Option 4)

12.89×10−3 kg

 
Read More

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1)

2,000 \; J - 5,000\; J

 Option 2)

200 \, \, J - 500 \, \, J
Option 3)

2\times 10^{5}J-3\times 10^{5}J

 Option 4)

20,000 \, \, J - 50,000 \, \, J
Read More

A particle is projected at 600   to the horizontal with a kinetic energy K. The kinetic energy at the highest point

Option 1)

K/2\,

 Option 2)

\; K\;
Option 3)

zero\;

 Option 4)

K/4
Read More

In the reaction,

2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}

Option 1)

11.2\, L\, H_{2(g)}  at STP  is produced for every mole HCL_{(aq)}  consumed

 Option 2)

6L\, HCl_{(aq)}  is consumed for ever 3L\, H_{2(g)}      produced
Option 3)

33.6 L\, H_{2(g)} is produced regardless of temperature and pressure for every mole Al that reacts

 Option 4)

67.2\, L\, H_{2(g)} at STP is produced for every mole Al that reacts .
Read More

How many moles of magnesium phosphate, Mg_{3}(PO_{4})_{2} will contain 0.25 mole of oxygen atoms?

Option 1)

0.02

 Option 2)

3.125 × 10-2
Option 3)

1.25 × 10-2

 Option 4)

2.5 × 10-2
Read More

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1)

decrease twice

 Option 2)

increase two fold
Option 3)

remain unchanged

 Option 4)

be a function of the molecular mass of the substance.
Read More

With increase of temperature, which of these changes?

Option 1)

Molality

 Option 2)

Weight fraction of solute
Option 3)

Fraction of solute present in water

 Option 4)

Mole fraction.
Read More

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol-1) is

Option 1)

twice that in 60 g carbon

 Option 2)

6.023 × 1022
Option 3)

half that in 8 g He

 Option 4)

558.5 × 6.023 × 1023
Read More

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t2) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m2 , the number of rotations made by the pulley before its direction of motion if reversed, is

Option 1)

less than 3

 Option 2)

more than 3 but less than 6
Option 3)

more than 6 but less than 9

 Option 4)

more than 9
Read More

Colleges After 12th

Applications for Admissions are open.

VMC VIQ Scholarship Test
VMC VIQ Scholarship Test
Apply

Register for Vidyamandir Intellect Quest. Get Scholarship and Cash Rewards.

JEE Main Important Physics formulas
JEE Main Important Physics formulas
Apply

As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters

JEE Main Important Chemistry formulas
JEE Main Important Chemistry formulas
Apply

As per latest 2024 syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters

TOEFL ® Registrations 2024
TOEFL ® Registrations 2024
Apply

Accepted by more than 11,000 universities in over 150 countries worldwide

Pearson | PTE
Pearson | PTE
Apply

Register now for PTE & Unlock 20% OFF : Use promo code: 'C360SPL20'. Valid till 15th NOV'24! Trusted by 3,500+ universities globally

JEE Main high scoring chapters and topics
JEE Main high scoring chapters and topics
Apply

As per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE

View All Application Forms
News and Notifications
CBSE Board Exams 2025: 75% attendance must for students to be eligible, reiterates board
October 11, 2024 - 08:42 AM IST
CBSE Class 10, 12 practical exams 2025 for winter-bound schools from November 5
October 11, 2024 - 07:26 AM IST
CBSE Date Sheet 2025: When to expect Class 10, 12 board exam time table?
October 07, 2024 - 01:58 PM IST
CBSE introduces CCTV policy in board exams 2025; schools fixed as centres must have facility
September 27, 2024 - 04:37 PM IST
CBSE gives parents, schools till October 16 to register students in Classes 9, 11
September 21, 2024 - 05:15 PM IST
CBSE Board Exam 2025: Class 9, 11 registration begins today; fee details, guidelines
September 18, 2024 - 09:10 AM IST
Submit list of candidates for CBSE board exams 2025 correctly, on time: Schools reminded
September 12, 2024 - 01:16 PM IST

Stay up-to date with CBSE Class 12th News

Back to top