NCERT Solutions for Class 11 Physics Chapter 10 Thermal Properties of Matter
The NCERT textbook solutions of Class 11 Physics Chapter - Thermal Properties of Matter have been created by our own subject matter experts in such a way that these solutions give a clear and concise answer of all the questions of all the exercises. Not only do these solutions assist the students in gaining a solid base, but also enhance their problem-solving abilities as the NCERT solutions are clearly explained through steps. These questions help the student gain knowledge in important issues such as heat, temperature, thermal expansion, and heat transfer by practicing them on a regular basis. These NCERT solution for class 11 physics are particularly helpful in revising in school exams, competitive exams such as JEE and NEET and in evaluating classrooms.
This Story also Contains
- NCERT Solutions for Class 11 Physics Chapter 10: Download Solution PDF
- NCERT Solutions for Class 11 Physics Thermal Properties of Matter - Exercise Solution
- Class 11 physics NCERT Chapter 10: Higher Order Thinking Skills (HOTS) Questions
- NCERT Chapter 10 Thermal Properties of Matter Topics
- NCERT Solutions for Class 11 Chapter 10: Important Formulae
- What Extra Should Students Study Beyond NCERT for JEE/NEET?
- NCERT Solutions for Class 11 Physics Chapter-Wise
- NCERT solutions for class 11 subject-wise
.jpg)
Did you ever think about why a metal spoon is colder than a wooden spoon even at the same temperature? Or why blacksmiths heat iron rings before placing them on wooden wheels? Thermal Properties of Matter Class 11 NCERT Solutions for Physics will provide you with answers to these questions. In this chapter, we learn about how heat moves, why the temperature sometimes does not change despite the addition of heat, and the physics involved in everyday processes such as sea breezes and boiling water. With step-by-step solutions, learning is a breeze and successful.
Also Read
- NCERT Exemplar Class 11 Physics Solutions Chapter Thermal Properties Of Matter
- NCERT Class 11 Physics Chapter 10 Notes Thermal Properties of Matter - Download PDF
NCERT Solutions for Class 11 Physics Chapter 10: Download Solution PDF
The NCERT Solutions to Chapter 10 give step by step answers to all the questions in the textbook to enable students to understand these topics within their grasp. Students get the benefit of downloading the PDF document with the solution and they can carry it offline and re-read it whenever they want.
NCERT Solutions for Class 11 Physics Thermal Properties of Matter - Exercise Solution
Answer:
The relation between Kelvin and Celcius scale is T K = T C + 273.15
Triple Point of Neon in Kelvin T K = 24.57 K
Triple Point of Neon in Celcius T C = T K -273.15 = 24.57 -273.15 =-248.58 o C
Triple Point of carbon dioxide in Kelvin T K = 216.55 K
Triple Point of carbon dioxide in Celcius T C = T K -273.15 = 216.55 - 273.15 = -56.60 o C
The relation between Celcius and Fahrenheit scale is $T_{F}=\frac{9}{5}T_{C} + 32$
Triple Point of Neon in Fahrenheit is
$T_{F}=\frac{9}{5}\times (-248.58) + 32$
T F = -415.44 o C
Triple Point of carbon dioxide in Fahrenheit is
$T_{F}=\frac{9}{5}\times (-56.60) + 32$
T F = -69.88 o C
Answer:
200 A = 273 K
$T_{K}=\frac{273}{200}T_{A}$
350 B = 273 K
$T_{K}=\frac{273}{350}T_{B}$
Equating T K From the above two equations we have
$\frac{273}{200}T_{A}=\frac{273}{350}T_{B}$
$T_{A}=\frac{4}{7}T_{B}$
Q. 10.3 The electrical resistance in ohms of a certain thermometer varies with temperature according to the approximate law:$R=R_{0}\left [ 1+\alpha (T-T_{0}) \right ]$ The resistance is $101.6\Omega$ at the triple-point of water $273.16\; K,$ and $165.5\; \Omega$ at the normal melting point of lead $(600.5\; K).$ What is the temperature when the resistance is $123.4\; \Omega$ ?
Answer:
$R=R_{0}\left [ 1+\alpha (T-T_{0}) \right ]$
R 0 = $101.6\Omega$
T 0 = $273.16 K$
R = $165.5\; \Omega$
T = $600.5 K$
Putting the above values in the given equation we have
$\\\alpha =\frac{165.5-101.6}{600.5-27316}\\ $
$\alpha =1.92\times 10^{-2}\ K^{-1}$
For R = $123.4\; \Omega$
$\\T=T_{0}+\frac{1}{\alpha }\left ( \frac{R}{R_{0}}-1 \right )\\ $
$T=273.16+\frac{1}{1.92\times 10^{-2}}\left ( \frac{123.4}{101.6}-1 \right )\\ $
$T=384.75K$
Q. 10.4 (a) Answer the following:
The triple-point of water is a standard fixed point in modern thermometry. Why ? What is wrong in taking the melting point of ice and the boiling point of water as standard fixed points (as was originally done in the Celsius scale)?
Answer:
Unlike the melting point of ice and boiling point of water, the triple point of water has a fixed value of 273.16 K. The melting point of ice and boiling point of water vary with pressure.
Q. 10.4 (b) Answer the following :
Answer:
The other fixed point on the Kelvin scale is 0 K. 0K is the absolute zero
Q. 10.4 (c) Answer the following :
The absolute temperature (Kelvin scale) $T$ is related to the temperature $t_{c}$ on the Celsius scale by $t_{c}=T-273.15$. Why do we have $273.15$ in this relation, and not $273.16?$
Answer:
This is because 0oC on the Celcius scale corresponding to the melting point at standard pressure is equal to 273.15 K whereas 273.16 K is the triple point of water. The triple point of water is 0.01oC , not 0oC
Q. 10.4 (d) Answer the following :
Answer:
Let at a certain temperature the reading on Fahrenheit and Kelvin Scale be T F and T K respectively
$T_{F}-32=\frac{9}{5}(T_{K}-273)$ $(i)$
Let at another temperature the reading on Fahrenheit and Kelvin Scale be T' F and T' K respectively
$T'_{F}-32=\frac{9}{5}(T'_{K}-273)$ $(ii)$
Subtracting equation (ii) from (i)
$T_{F}-T'_{F}=\frac{9}{5}(T_{K}-T'_{K})$
For T K - T' K = 1 K,
T F - T' F = 9/5
Therefore corresponding to 273.16 K the absolute scale whose unit interval size is equal to that of the Fahrenheit scale
$\\T_{F}=\frac{9}{5}\times 273.16\\ $
$T_{F}=491.688$
|
Temperature
|
Pressure
Thermometer A
|
Pressure
Thermometer B
|
|
Triple-point of water
| ||
|
Normal melting point of sulphur
|
What is the absolute temperature of normal melting point of sulphur as read by thermometers A and B?
Answer:
$\\PV=nRT\\$
$\frac{P}{T}=\frac{nR}{V}$
As the moles of oxygen and hydrogen inside the thermometers and the volume occupied by the gases remain constant P/T would remain constant.
The triple point of water(T 1 ) = 273.16 K
Pressure in thermometer A at a temperature equal to the triple point of water (P 1 ) = $1.250\times 10^{5}Pa$
Pressure in thermometer A at a temperature equal to Normal melting point of sulphur (P 2 ) = $1.797\times 10^{5}Pa$
The normal melting point of sulphur as read by thermometer A, T 2 would be given as
$T_{2}=\frac{P_{2}T_{1}}{P_{1}}$
$\\T_{2}=\frac{1.797\times 10^{5}\times 273.16}{1.250\times 10^{5}}\\ $
$T_{2}=392.69\ K$
Pressure in thermometer B at a temperature equal to the triple point of water (P1' ) = $0.200\times 10^{5}Pa$
Pressure in thermometer B at a temperature equal to Normal melting point of sulphur (P2' ) = $0.287\times 10^{5}Pa$
The normal melting point of sulphur as read by thermometer B, T2' would be given as
$\\T'_{2}=\frac{P'_{2}T_{1}}{P'_{1}}\\ $
$T'_{2}=\frac{0.287\times 10^{5}\times 273.16}{0.200\times 10^{5}}\\$
$ T'_{2}=391.98\ K$
|
Temperature
|
Pressure
Thermometer A
|
Pressure
Thermometer B
|
|
Triple-point of water
| ||
|
Normal melting point of sulphur
|
What do you think is the reason behind the slight difference in answers of thermometers A and B? (The thermometers are not faulty). What further procedure is needed in the experiment to reduce the discrepancy between the two readings?
Answer:
The slight difference in answers of thermometers A and B occur because the gases used in the thermometers are not ideal gases. To reduce this discrepancy the experiments should be carried out at low pressures where the behaviour of real gases tend close to that of ideal gases.
Answer:
At 27 o C the 63 cm ($l1$) mark on the steel tape would be measuring exactly 63 cm as the tape is calibrated at 27 o C
Coefficient of linear expansion of steel $=1.20\times 10^{-5}K^{-1}.$
Actual length when the scale is giving a reading of 63 cm on at 45 o C is $l2$
$\\l_{2}=l_{1}(1+\alpha \Delta T)\\ =63\times (1+1.20\times 10^{-5}\times (45-27))\\ =63.013608cm$
The actual length of the steel rod on a day when the temperature is 45oC is 63.013608 cm.
Length of the same steel rod on a day when the temperature is 63 cm.
Answer:
Diameter of the steel shaft at 27 o C (T 1 ) d 1 = 8.70 cm
The diameter of the central hole in the wheel d 2 = 8.69 cm
Coefficient of linear expansion of the steel $\alpha _{steel}=1.20\times 10^{-5}K^{-1}$ .
The wheel will slip on the shaft when the diameter of the steel shaft becomes equal to the diameter of the central hole in the wheel.
Let this happen at temperature T
$\\d_{2}=d_{1}(1+\alpha (T-T_{1}))\\ 8.69=8.7(1+1.2\times 10^{5}(T-27))\\ $
$T=-68.79\ ^{o}C$
Answer:
Coefficient of linear expansion of copper $\alpha$ $=1.70\times 10^{-5}K^{-1}.$
Coefficient of superficial expansion of copper is $\beta$
$\beta =2\alpha \\ $
$\beta =2\times 1.7\times 10^{-5}\\ $
$\beta =3.4\times 10^{-5}K^{-1}$
Diameter of the hole at 27 o C (d 1 ) = 4.24 cm
Area of the hole at 227 o C is
$A_{2}=A_{1}(1+\beta \Delta T)\\ $
$A_{2}=\pi \left ( \frac{4.24}{2} \right )^{2}(1+3.4\times 10^{-5}(227-27))$
$A_{2}=14.215\ cm^{2}$
Let the diameter at 227 o C be d 2
$\\\pi \left ( \frac{d_{2}}{4} \right )^{2}=14.215\\$
$ d_{2}=4.254cm$
Change in diameter is d 2 -d 1 = 4.24 -4.254 = 0.014 cm.
Answer:
Youngs Modulus of Brass, $Y=0.91\times 10^{11}$
Co-efficient of linear expansion of Brass, $\alpha =2.0\times 10^{-5}K^{-1}$
The diameter of the given brass wire, d = 2.0 mm
Length of the given brass wire, $l$ = 1.8 m
Initial Temperature T 1 = 27 o C
Final Temperature T 2 = -39 o C
$\\Y=\frac{Stress}{Strain}\\ $
$Y=\frac{\frac{F}{A}}{\frac{\Delta l}{l}}\\ $
$F=\frac{\Delta lAY}{l}\\ $
$F=\frac{(l\alpha \Delta T)AY}{l}\\ $
$F=\alpha \Delta TAY\\ $
$F=\alpha \Delta TY\pi \frac{d^{2}}{4}\\ $
$F=\frac{2.0\times 10^{-5}\times (-39-27)\times 0.91\times 10^{11}\times \pi \times (2\times 10^{-3})^{2}}{4}$
$F=-378N$
The tension developed in the wire is 378 N. The negative sign signifies this tension is inward.
Answer:
Length of the rods $l$ = 50 cm
Co-efficient of linear expansion of brass, $\\\alpha _{b}=2\times 10^{-5}K^{-1}$
Co-efficient of linear expansion of steel, $\\\alpha _{s}=1.2\times 10^{-5}K^{-1}$
Initial Temperature T 1 = 40.0 o C
Final Temperature T 2 = 250 o C
Change in length of brass rod is
$\\\Delta l_{t}=l_{t}\alpha_{b} \Delta T\\ $
$\Delta l_{t}=50\times 2.0\times 10^{-5}\times (250-40)\\ $
$\Delta l_{t}=0.21cm$
Change in length of the steel rod is
$\\\Delta l_{s}=l_{s}\alpha_{s} \Delta T\\ $
$\Delta l_{s}=50\times 1.2\times 10^{-5}\times (250-40)\\ $
$\Delta l_{s}=0.126cm$
Change in length of the combined rod is
$\\\Delta l=\Delta l_{s}+\Delta l_{b}\\ $
$\Delta l=0.126+0.21\\ $
$\Delta l=0.336cm$
Answer:
Coefficient of volume expansion of glycerine is $\gamma =49\times 10^{-5}K^{-1}$
Let initial volume and mass of a certain amount of glycerine be V and m respectively.
Initial density is
$\rho =\frac{m}{V}$
Change in volume for a 30 o C rise in temperature will be
$\\\Delta V=V(\gamma \Delta T)\\ $
$\Delta V=V(49\times 10^{-5}\times 30)\\ $
$\Delta V=0.0147V$
Final Density is
$\\\rho' =\frac{m}{V+\Delta V}\\ $
$\rho'=\frac{m}{1.0147V}\\ $
$\rho'=\frac{0.986m}{V}$
Fractional Change in density is
$\\\frac{\rho'-\rho}{\rho}\\ =\frac{\frac{0.986m}{v}-\frac{m}{v}}{\frac{m}{v}}\\ =-0.014$
The negative sign signifies with an increase in temperature density will decrease.
Answer:
Power of the drilling machine, P = 10 kW
Time. t = 2.5 min
Total energy dissipated E is
$\\E=Pt\\$
$ E=10\times 10^{3}\times 2.5\times 60\\ $
$E=1.5\times 10^{6}J$
Thermal energy absorbed by aluminium block is
$\\\Delta Q=\frac{E}{2}\\$
$ \Delta Q=\frac{1.5\times 10^{6}}{2}\\ $
$\Delta Q=7.5\times 10^{5}J$
Mass of the aluminium block, m = 8.0 kg
Specific heat of aluminium, c = 0.91 J g -1 K -1
Let rise in temperature be $\Delta T$
$\\mc\Delta T=\Delta Q\\ $
$\Delta T=\frac{\Delta Q}{mc}\\ $
$\Delta T=\frac{7.5\times 10^{5}}{0.91\times 8\times 10^{3}}\\ $
$\Delta T=103.02\ ^{o}C$
Answer:
Mass of copper block m = 2.5 kg
Initial Temperature of the copper block, T 1 = 500 o C
Final Temperature of Copper block, T 2 = 0 o C
Specific heat of copper, c = 0.39 J g -1 K -1
Thermal Energy released by the copper block is $\Delta Q$
$\\\Delta Q=mc\Delta T\\ $
$\Delta Q=2.5\times 10^{3}\times 0.39\times 500\\ $
$\Delta Q=487500\ J$
Latent heat of fusion of water, L = 335 j g -1
Amount of ice that can melt is
$\\w=\frac{\Delta Q}{L}\\ $
$w=\frac{487500}{335}\\$
$ w=1455.22g$
1.455 kg of ice can melt using the heat released by the copper block.
Answer:
Let the specific heat of the metal be c.
Mass of metal block m = 200 g
Initial Temperature of metal block = 150 o C
Final Temperature of metal block = 40 o C
The heat released by the block is
$\\\Delta Q=mc\Delta T\\ $
$\Delta Q=200\times c\times (150-40)\\ $
$\Delta Q=22000c$
Initial Temperature of the calorimeter and water = 27 o C
Final Temperature of the calorimeter and water = 40 o C
Amount of water = 150 cm
Mass of water = 150 g
Water equivalent of calorimeter = 25 g
Specific heat of water = 4.186 J g -1 K -1
Heat absorbed by the Calorimeter and water is $\\\Delta Q'$
$\\\Delta Q'=(150+25)\times 4.186\times (40-27)\\ $
$\Delta Q'=9523.15J$
The heat absorbed by the Calorimeter and water is equal to the heat released by the block
$\\\Delta Q=\Delta Q'\\ 22000c=9523.15\\ c=0.433\ J\ g^{-1}\ K^{-1}$
The above value would be lesser than the actual value since some heat must have been lost to the surroundings as well which we haven't accounted for.
Q. 10.15 Given below are observations on molar specific heats at room temperature of some common gases.
Gas Molar specific heat (Cv)
$(cal\; mol^{-1}K^{-1})$
Hydrogen 4.87
Nitrogen 4.97
Oxygen 5.02
Nitric oxide 4.99
Carbon monoxide 5.01
Chlorine 6.17
The measured molar specific heats of these gases are markedly different from those for monatomic gases. Typically, molar specific heat of a monatomic gas is $2.92\; cal/mol\; K.$ Explain this difference. What can you infer from the somewhat larger (than the rest) value for chlorine?
Answer:
Monoatomic gases have only translational degree of freedom but diatomic gases have rotational degrees of freedom as well. The temperature increases with increase in the spontaneity of motion in all degrees. Therefore, to increase the temperature of diatomic gases more energy is required than that required to increase the temperature of monoatomic gases by the same value owing to higher degrees of freedom in diatomic gases.
If we only consider rotational modes of freedom the molar specific heat of the diatomic gases would be given as
$\\c=\frac{fR}{2}$
$ c=\frac{5}{2}\times 1.92$
$ c=4.95\ cal\ mol^{-1}\ K^{-1}$
The number of degrees of freedom = 5 (3 translational and 2 rotational)
The values given in the table are more or less in accordance with the above calculated one. The larger deviation from the calculated value in the case of chlorine is because of the presence of vibrational motion as well.
Answer:
Initial Temperature of the boy = 101 o F
Final Temperature of the boy = 98 o F
Change in Temperature is
$\\\Delta T=3\ ^{o}F\\ $
$\Delta T=3\times \frac{5}{9}\\ $
$\Delta T=1.67\ ^{o}C$
Mass of the child is m = 30 kg
Specific heat of human body = 1000 cal kg -1 o C -1
Heat released is $\\\Delta Q$
$\\\Delta Q=mc\Delta T\\$
$\Delta Q=30\times 1000\times 1.67\\ $
$\Delta Q=50000\ cal$
Latent heat of evaporation of water = 580 cal g -1
The amount of heat lost by the body of the boy has been absorbed by water.
Let the mass of water which has evaporated be m'
$\\\Delta Q=m'L\\ $
$ m'=\frac{Q}{L}\\ $
$m'=\frac{50000}{580}\\$
$ m'=86.2\ g$
Time in which the water has evaporated, t = 20 min.
The rate of evaporation is m'/t
$\\\frac{m'}{t}=\frac{86.2}{20}\\ $
$\frac{m'}{t}=4.31\ g\ min^{-1}$
Answer:
Side of the box s = 30 cm
Area available for conduction A
A = 6s 2
A=6(30) 2
A=5400 cm 2 = 0.54 m 2
Temperature difference = 45 o C
Co-efficient of thermal conductivity of thermacole is k = 0.01 J s -1 m -1 K -1
Width of the box is d = 5 cm
Heat absorbed by the box in 6 hours is $\Delta Q$
$\\\Delta Q=\frac{kA\Delta T}{l}\\ $
$\Delta Q=\frac{0.01\times 0.54\times 45\times 6\times 60\times 60}{0.05}\\ $
$\Delta Q=104976\ J$
The heat of fusion of water is $L=335\times 10^{3}\ J\ kg^{-1}$
The amount of ice which has melted is m'
$\\m'=\frac{\Delta Q}{L}\\ $
$m'=\frac{104976}{335\times 10^{3}}\\ $
$m'=0.313\ kg$
Amount of ice left after 6 hours = 4 - 0.313 = 3.687 kg
Answer:
The rate at which water boils, R = 6.0 kg min -1
The heat of vaporisation of water, $L=2256\times 10^{3}\ J\ kg^{-1}$
The rate at which heat enters the boiler
$\\\frac{dQ}{dT}=RL\\ $
$\frac{dQ}{dT}=\frac{6\times 2256\times 10^{3}}{60}\\ $
$\frac{dQ}{dT}=2.256\times 10^{5}Js^{-1}$
The base area of the boiler, A = 0.15 m 2
Thickness, $l$ = 1.0 cm
Thermal conductivity of brass $=109\; J\; s^{-1}m^{-1}K^{-1};$
The temperature inside the boiler = Boiling point of water = 100 o C
Let the temperature of the flame in contact with the boiler be T
The amount of heat flowing into the boiler is
$\begin{aligned}
& \frac{d Q}{d t}=\frac{K A \Delta T}{l} \\
& 2.256 \times 10^5=\frac{109 \times 0.15 \times(T-100)}{1 \times 10^{-2}} \\
& T-100=137.98 \\
& T=237.98^{\circ} \mathrm{C}
\end{aligned}$
The temperature of the flame in contact with the boiler is 237.98 o C
Q. 10.19 (a) Explain whya body with large reflectivity is a poor emitter
Answer:
A body with a large reflectivity is a poor absorber. As we know a body which is a poor absorber will as well be a poor emitter. Therefore, a body with large reflectivity is a poor emitter.
Q .10.19 (b) Explain whya brass tumbler feels much colder than a wooden tray on a chilly day
Answer:
Brass is a good conductor of heat. Therefore once someone touches brass heat from their body flows into it and it feels cold, in case of a wooden tray, no such conduction of heat from the body takes place as wood is a very poor conductor of heat.
Q. 10.19 (c) Explain whyan optical pyrometer (for measuring high temperatures) calibrated for an ideal black body radiation gives too low a value for the temperature of a red hot iron piece in the open but gives a correct value for the temperature when the same piece is in the furnace
Answer:
An optical pyrometer relates the brightness of a glowing body with its temperature. In the open because of other sources of light the sensor in the optical pyrometer does not detect the true brightness of a red hot piece of iron and thus does not predict its temperature correctly whereas in the furnace the piece of iron is the only source of light and the sensor detects its brightness correctly thus giving the correct value of the temperature.
Q. 10.19 (d) Explain whythe earth without its atmosphere would be inhospitably cold
Answer:
The sun rays contain infrared radiations. These are reflected back by the lower part of the atmosphere after being reflected by the surface of the earth and are trapped inside the atmosphere thus maintaining the Earth's temperature at a hospitable level. Without these rays being trapped the temperature of the earth will go down severely and thus the Earth without its atmosphere would be inhospitably cold.
Q. 10.19 (e) Explain whyheating systems based on circulation of steam are more efficient in warming a building than those based on circulation of hot water
Answer:
Heating systems based on the circulation of steam are more efficient in warming a building than those based on the circulation of hot water because the same amount of steam at 100 o C contains more energy available for heat dissipation than the same amount of water at 100 o C in the form of latent heat of vaporization.
Answer:
Let a body initially be at temperature T 1
Let its final Temperature be T 2
Let the surrounding temperature be T 0
Let the temperature change in time t.
According to Newton's Law of cooling
$\\-\frac{dT}{dt}=K(T-T_{0})\\ $
$\frac{dT}{T-T_{0}}=-Kdt\\$
$ \int_{T_{1}}^{T_{2}}\frac{dT}{T-T_{0}}=-K\int_{0}^{t}dt\\$
$ \left [ ln(T-T_{0}) \right ]_{T_{1}}^{T_{2}}=-K[t]_{0}^{t}\\ $
$ln\left ( \frac{T_{2}-T_{0}}{T_{1}-T_{0}} \right )=-Kt$
where K is a constant.
We have been given that the body cools from 80 o C to 50 o C in 5 minutes when the surrounding temperature is 20 o C.
T 2 = 50 o C
T 1 = 80 o C
T 0 = 20 o C
t = 5 min = 300 s.
$\\ln\left ( \frac{50-20}{80-20} \right )=-K\times 300\\ K=\frac{ln(2)}{300}\\$
For T 1 = 60 o C and T 2 = 30 o C we have
$\\ln\left ( \frac{30-20}{60-20} \right )=-\frac{ln2}{300}t\\ $
$t=\frac{ln(4)\times 300}{ln(2)} \\$
$t=\frac{2ln(2)\times 300}{ln(2)}\\ $
$t=600\ s\\ $
$t= 10\ min$
The body will take 10 minutes to cool from 60 o C to 30 o C at the surrounding temperature of 20 o C.
Class 11 physics NCERT Chapter 10: Higher Order Thinking Skills (HOTS) Questions
Q1:
When 100 g of liquid A at 100oC is added to 50 g of liquid B at a temperature of 75oC, the temperature of the mixture becomes 90oC. The temperature of the mixture (in oC), if 100 g of liquid A at 100oC is added to 50 g of liquid B at 50oC, will be :
Answer:
Specific Heat -
$
\begin{aligned}
& S=\frac{Q}{m \cdot \Delta \theta} \\
& \text { - wherein } \\
& \mathrm{S}=\text { specific heat } \\
& \Delta \theta=\text { Change in temperature } \\
& \mathrm{m}=\text { Amount of mass } \\
& \text { For (I) } \\
& 100 \times S_A \times(100-90)=50 \times S_B \times(90-75) \\
& S_A=\frac{1.5}{2} S_B=\frac{3}{4} S_B \cdots(1) \\
& \text { now for }(\mathrm{II}) \\
& 100 \times S_A \times(100-T)=50 \times S_B \times(T-50) \\
& \Rightarrow 2 S_A(100-T)=S_B(T-50) \cdots(2)
\end{aligned}
$
From (1) & (2)
$
\begin{aligned}
& 2 \times \frac{3}{4} \times(100-T)=(T-50) \\
& 3(100-T)=2(T-50) \\
& 300-3 T=2 T-100 \\
& 400=5 T \\
& T=80^{\circ} \mathrm{C}
\end{aligned}
$
Q2:
If the length of cylinder on heating increases by 2% then the area of base of cylinder will increase by
Answer:
Assuming the initial length of the cylinder is $\ell_1$, the linear expansion coefficient is $\alpha$, and the temperature rise is $\Delta \mathrm{t}^{\circ} \mathrm{C}$,
$
\begin{aligned}
& \Delta \ell=\ell \alpha \Delta \mathrm{t} \Rightarrow \frac{\Delta \ell}{\ell} \times 100=\alpha \Delta \mathrm{t} \times 100 \\
& \therefore 2=\alpha \Delta \mathrm{t} \times 100
\end{aligned}
$
Now, for area
$
\begin{aligned}
& \Delta A=A \beta \Delta t \Rightarrow \frac{\Delta A}{A}=\beta \Delta t \\
& \Rightarrow \frac{\Delta A}{A} \times 100=\beta \Delta t \times 100 \\
& \% \text { change in area }=\beta \Delta t \times 100 \\
& =\beta \Delta t \times 100
\end{aligned}
$
We know that $2 \times \alpha \times \Delta \mathrm{t}=\beta \times \Delta \mathrm{t}$
$
\begin{aligned}
& =2 \alpha \Delta \mathrm{t} \times 100 \\
& =2(\alpha \Delta \mathrm{t} \times 100) \\
& =2 \times 2 \\
& =4 \%
\end{aligned}
$
Q3: A cylindrical metal rod of length L0 is shaped into a ring with a small gap as shown in Figure. On heating the system,
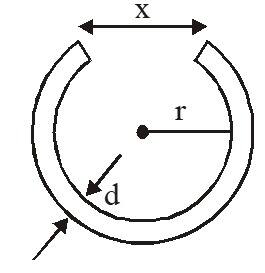
A. x will decrease, r will increase
B. Both x and r will decrease
C. Both x and r will increase
D. r will increase, x will remain same
Answer:
Linear Expansion - When a solid is heated and its length increases, then the Expansion is called linear Expansion.
On the heating, the system x and r both will increase since the expansion of isotropic solids is similar to true photographic enlargement.
Q4:
The coefficient of thermal expansion of a rod varies with temperature as $\alpha=\alpha_0+\alpha_0 \frac{T}{T_0}$ where T is the temperature of the body and all others are positive constants. If the temperature of the rod of length $\ell_0$ is changed from $T_0 / 2$ to $T_0$ uniformly with time, then what is the ratio of rate of fractional change of length initially and finally?
Answer:
We know coefficient of thermal expansion is given as
$
\begin{gathered}
\quad \frac{\mathrm{d} L}{L \mathrm{~d} T}=\alpha \\
\Rightarrow \frac{\mathrm{d} L}{L \mathrm{dt}}=\alpha \frac{\mathrm{d} T}{\mathrm{~d} t} \\
\frac{\left(\frac{d L}{L d t}\right)_1}{\left(\frac{d L}{L d t}\right)_2}=\frac{\alpha_1 \frac{d T}{d t}}{\alpha_2 \frac{d T}{d t}} \\
\Rightarrow \frac{\left(\frac{d L}{L d}\right)_1}{\left(\frac{d L}{L d t}\right)_2}=\frac{\alpha_1}{\alpha_2} \\
\Rightarrow
\frac{\alpha_0+\alpha_0 \frac{T_0 / 2}{T_0}}{\alpha_0+\alpha_0 \frac{T_0}{T_0}}=\frac{3 / 2}{2}=\frac{3}{4}
\end{gathered}
$
Q5:
A red hot iron (mass 25 g) has a temperature of 100ºC. It is immersed in a mixture of ice and water at thermal equilibrium. The volume of the mixture is found to be reduced by $0.15 \mathrm{~cm}^3$with the temperature of the mixture remains constant. Find the specific heat (in cal gm ${ }^0 \mathrm{C}$) of the metal.
Given specific gravity of ice = 0.92, specific gravity of water at $0^{\circ} \mathrm{C}=1.0$, latent heat of fusion of ice $=80 \mathrm{calg}^{-1}$.
Answer:
As the ice melts its density changes which causes change in volume. Let m g of ice melt.
$\begin{aligned} & \frac{m}{\rho_{i c e}}-\frac{m}{\rho_{\mathrm{H}_2 \mathrm{O}}}=0.15 \\ & m=\frac{0.15 \times 0.92}{0.08} \\ & \therefore \quad H_{\text {lost }}=H_{\text {gaired }} \\ & \Rightarrow \quad 25 \times S \times 100=\frac{0.15 \times 0.92}{0.08} \times 80 \\ & S=0.06 \mathrm{cal} \mathrm{gm}^{\circ} \mathrm{C}\end{aligned}$
NCERT Chapter 10 Thermal Properties of Matter Topics
Chapter 10 Thermal Properties of Matter in Class 11 Physics introduces the property of matter on how it reacts to heat. It discusses important concepts, which include heat capacity, thermal expansion, change of state, and ways of heat transfer through conduction, convection, and radiation.
10.1 Introduction
10.2 Temperature And Heat
10.3 Measurement Of Temperature
10.4 Ideal-Gas Equation And Absolute Temperature
10.5 Thermal Expansion
10.6 Specific Heat Capacity
10.7 Calorimetry
10.8 Change Of State
10.8.1 Latent Heat
10.9 Heat Transfer
10.9.1 Conduction
10.9.2 Convection
10.9.3 Radiation
10.9.4 Blackbody Radiation
10.10 Newton’S Law Of Cooling
NCERT Solutions for Class 11 Chapter 10: Important Formulae
The significant formulae of Chapter 10: Thermal Properties of Matter in Class 11 Physics enable students to know and work out problems concerning heat, temperature, expansion and the transfer of heat.
1. Heat and Temperature Relation
$
Q=m c \Delta T
$
Where:
$
\begin{aligned}
& Q=\text { heat added or removed } \\
& m=\text { mass } \\
& c=\text { specific heat capacity } \\
& \Delta T=\text { change in temperature }
\end{aligned}
$
2. Specific Heat Capacity
$c=\frac{Q}{m \Delta T}$
3. Heat Capacity
$\begin{aligned} & C=m c \\ & C=\frac{Q}{\Delta T}\end{aligned}$
4. Latent Heat
$
Q=m L
$
Where:
$L=$ latent heat of fusion or vaporization
(Use for phase changes with no temperature change)
5. Linear, Areal and Volume Expansion
$
\Delta L=L \alpha \Delta T
$
$
\Delta A=A \beta \Delta T
$
Where $\beta=2 \alpha$
$
\Delta V=V \gamma \Delta T
$
Where $\gamma=3 \alpha$
8. Calorimetry Principle
Heat lost=Heat gained
(Applies in thermal equilibrium problems)
9. Thermal Conduction
$
Q=\frac{k A\left(T_1-T_2\right) t}{d}
$
Where:
$k=$ thermal conductivity
$A=$ area
$d=$ thickness
$t=$ time
$T_1-T_2=$ temperature difference
10. Newton’s Law of Cooling
$\frac{d T}{d t} \propto\left(T-T_{\text {env }}\right)$
Approach to Solve Questions of Thermal Properties of Matter
- Have Clear Conceptual Understanding. Revise the simple terms like heat, temperature, specific heat, thermal expansion, and types of the heat transfer.
- Categorize the Kind of Problem Find out whether the question involves temperature change, thermal expansion, heat capacity, phase change or heat transfer.
- These should be Calculated Using the Appropriate Formula Choose the right formula according to the topic
- Apply conduction, convection, radiation formulae where necessary.
- Convert Units, if required. Put everything in SI (e.g. mass as kg, temperature in Kelvin, time in second).
- Use the principle of Calorimetry: Heat lost=Heat gained
- Include Latent Heat in the case of Phase Change. Remember to add or delete latent heat in melting, boiling or condensation questions.
What Extra Should Students Study Beyond NCERT for JEE/NEET?
Comparison of NCERT with JEE/ NEET requirements has been done in the table below so that the students know what they need to know more than the highlights in NCERTs to enhance their preparation in such competitive exams.
NCERT Solutions for Class 11 Physics Chapter-Wise
NCERT solutions for class 11 subject-wise
Also Check NCERT Books and NCERT Syllabus here
- NCERT Books Class 11 Physics
- NCERT Syllabus Class 11 Physics
- NCERT Books Class 11
- NCERT Syllabus Class 11
Subject wise NCERT Exemplar solutions
Frequently Asked Questions (FAQs)
Thermal properties of matter describe the behaviour of substances when heat is added or removed, such as temperature changes, expansion, heat transfer and phase changes.
Metals are good conductors of heat and hence they tend to conduct heat away at a very fast rate from our body and thus they feel colder, whereas wood is a poor conductor and does not conduct heat at a high rate.
Heat is a type of energy that is exchanged between bodies with respect to a difference in temperature whereas temperature is a measure of how hot or cold a body is.
Specific heat capacity is used to establish the amount of heat energy needed to increase the temperature of one unit mass of a substance by 1 °C (or 1 K), commonly encountered in calculations-based questions.
Yes, a lot of conceptual and numerical questions, depending on the heat transfer, thermal expansion, and calorimetry, are regularly asked in JEE, NEET, and other entrance tests.
Popular Questions
Courses After 12th
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters
