NCERT Solutions for Class 12 Physics Chapter 4 - Moving Charges and Magnetism
Have you ever questioned how an electric fan spins when you pass a current through the motor or how a magnetic compass needle bounces off a current carrying wire? These common things in our daily life are practical demonstrations of the concepts of Chapter 4 of Physics Class 12 - Moving Charges and Magnetism.
This Story also Contains
- Moving Charges and Magnetism Class 12 Question Answers: Download PDF
- Moving Charges and Magnetism NCERT Solutions: Exercise Questions
- Moving charges and magnetism NCERT Solutions: Additional Questions
- Class 12 Physics Chapter 4 - Moving charges and magnetism: Higher Order Thinking Skills (HOTS) Questions
- Moving Charges and Magnetism Class 12 Question Answers: Topics
- Class 12 Physics Chapter 4 - Moving charges and magnetism: Important Formulas
- Approach to Solve Questions of Class 12 Physics Chapter 4 - Moving charges and magnetism
- What Extra Should Students Study Beyond the NCERT for JEE/NEET?
- Importance in Exams:
- NCERT Solutions for Class 12 Physics: Chapter-Wise
The NCERT Solutions for Class 12 Physics Chapter 4 - Moving charges and magnetism are aimed at providing step wise explanation of all the exercise problems so that the students can readily comprehend the process of application of the laws of magnetism. Such solutions have a great benefit in establishing solid foundations that can be needed to pass the CBSE Class 12 board exams, JEE, NEET, and other competitive exams. Theese NCERT solutions contain elaborated answers to exercise questions, extra questions, and HOTS (Higher Order Thinking Skills) in order to enhance the problem-solving capability. The students also have a good explanation of some of the significant formulas and derivation including BiotSavart Law, Ampere Circuital Law, Magnetic Field due a Current Loop and Force on a Current carrying Conductor. The main concepts that were discussed in this chapter are the Lorentz Force and Motion of a Charged Particle in a Magnetic Field, Applications of Biot-Savart Law, Force between Two Parallel Current-Carrying Wires, Ampere Law and Applications as well as the Moving Coil Galvanometer. By using these organized NCERT Solutions for Class 12 Physics Chapter 4 - Moving charges and magnetism, the student is able to not only revise, but also have confidence in their ability to apply physics concepts to real life and exam level problems.
Moving Charges and Magnetism Class 12 Question Answers: Download PDF
The Class 12 Physics Chapter 4 - Moving charges and magnetism question answers are available in the structure of a well formatted PDF file to the students. The downloadable solutions are available with step-by-step instructions of all the exercises, new questions, and HOTS, and the preparation and revision of the exams becomes simpler and more efficient. The PDF becomes a valuable tool in order to reinforce the conceptual clarity and train problem-solving skills at any place and any time.
Moving Charges and Magnetism NCERT Solutions: Exercise Questions
The Class 12 Physics Chapter 4 - Moving charges and magnetism Exercise Questions are answered in a very clear, stepwise fashion so as to enable the student to enhance his/her understanding of all the concepts. All these solutions offer comprehensive explanations to every problem, and so it becomes simpler to practice numericals, solve equations, and prepare well to take exams such as CBSE, JEE, and NEET.
Answer :
The magnitude of the magnetic field at the centre of a circular coil of radius r carrying current I is given by,
|B|=μ0I2r
For 100 turns, the magnitude of the magnetic field will be,
|B|=100×μ0I2r
(Given, current=0.4A, radius = 0.08m, permeability of free space = 4 π× 10 -7 TmA-1)
|B|=100×4π×10−7×0.42×0.08=3.14×10−4T
Answer:
The magnitude of the magnetic field at a distance r from a long straight wire carrying current I is given by,
|B|=μ0I2πr
In this case
(Given, current=0.4A, radius = 0.08m, permeability of free space = 4 π× 10 -7 TmA-1)
|B|=4π×10−7×352π×0.2=3.5×10−5T
Answer:
The magnitude of the magnetic field at a distance r from a long straight wire carrying current I is given by,
|B|=μ0I2πr
In this case
(Given, current=0.4A, radius = 0.08m, permeability of free space = 4 π× 10 -7 TmA-1)
|B|=4π×10−7×502π×2.5=4×10−6T
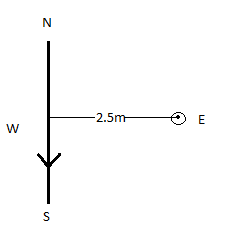
The current is going from the North to South direction in the horizontal plane and the point lies to the East of the wire. Applying Maxwell's right-hand thumb rule we can see that the direction of the magnetic field will be vertically upwards.
Answer:
The magnitude of the magnetic field at a distance r from a long straight wire carrying current I is given by,
|B|=μ0I2πr
In this case
(Given, current=0.4A, radius = 0.08m, permeability of free space = 4 π× 10 -7 TmA-1)
|B|=4π×10−7×902π×1.5=1.2×10−5T
The current in the overhead power line is going from the East to West direction and the point lies below the power line. Applying Maxwell's right-hand thumb rule we can see that the direction of the magnetic field will be towards the South.
Answer:
The magnetic force on an infinitesimal current-carrying conductor in a magnetic field is given by dF→=Idl→×B→ where the direction of vector dl is in the direction of flow of current.
For a straight wire of length l in a uniform magnetic field, the Force equals to
F→=∫0lIdl→×B→|F→|=BIlsinθ
In the given case the magnitude of force per unit length is equal to
(Given, I=8A, B=0.15 T, θ =30o)
|F| = 0.15 × 8 × sin30o =0.6 Nm-1
Answer:
For a straight wire of length l in a uniform magnetic field, the Force equals to
F→=∫0lIdl→×B→
⇒|F→|=BIlsinθ
In the given case the magnitude of the force is equal to
(Given, I=10A, B=0.27 T, θ =90o)
|F| = 0.27 × 10 × 0.03 × sin90o =0.081 N
The direction of this force depends on the orientation of the coil and the current-carrying wire and can be known using the Flemings Left-hand rule.
Answer:
The magnitude of magnetic field at a distance r from a long straight wire carrying current I is given by,
|B|=μ0I2πr
In this case the magnetic field at a distance of 4.0 cm from wire B will be
(Given, I=5 A, r=4.0 cm)
|B|=4π×10−7×52π×0.04=2.5×10−5T
The force on a straight wire of length l carrying current I in a uniform magnetic field B is given by
F=BIlsinθ , where θ is the angle between the direction of flow of current and the magnetic field.
The force on a 10 cm section of wire A will be
(Given, B=2.5 T, I=8 A, l = 10 cm, θ =90o)
F=2.5×10−5×8×0.1×sin90∘
F=2×10−5N
Answer:
The magnitude of the magnetic field at the centre of a solenoid of length ℓ, total turns N and carrying current I is given by
B=μoNIl , where μo is the permeability of free space.
In the given question N= number of layers of winding × number of turns per each winding
N=5 × 400=2000
I=8.0 A
ℓ=80cm
B=4π×10−7×2000×80.8
B=2.51×10−2T
Answer:
The magnitude of torque experienced by a current-carrying coil in a magnetic field is given by
τ=nBIAsinθ
where n = number of turns, I is the current in the coil, A is the area of the coil and θ is the angle between the magnetic field and the vector normal to the plane of the coil.
In the given question n = 20, B=0.8 T, A=0.1 × 0.1=0.01 m2 , I=12 A, θ =30o
τ=20×0.8×12×0.01×sin30o=0.96Nm
The coil, therefore, experiences a torque of magnitude 0.96 Nm.
4.10 (a) Two moving coil meters, M1 and M2 have the following particulars:
R1=10Ω,N1=30,A1=3.6×10−3m2,B1=0.25T R2=14Ω,N2=42,A2=1.8×10−3m2,B2=0.50T
(The spring constants are identical for the two meters).
Determine the ratio of current sensitivity of M2 and M1
Answer:
The torque experienced by the moving coil M1 for a current I passing through it will be equal to τ=B1A1N1I
The coil will experience a restoring torque proportional to the twist ϕ
ϕk=B1A1N1I
The current sensitivity is therefore B1A1N1k
Similarly, for the coil M2, current sensitivity is B2A2N2k
Their ratio of current sensitivity of coil M2 to that of coil M1 is, therefore,
B2A2N2B1A1N1=0.5×1.8×10−3×420.25×3.6×10−3×30=1.4
4.10(b) Two moving coil meters, M1 and M2 have the following particulars:
Determine the ratio of voltage sensitivity of M2 and M1
Answer:
The torque experienced by the moving coil M1 for a current I passing through it will be equal to τ=B1A1N1I
The coil will experience a restoring torque proportional to the twist ϕ
ϕk=B1A1N1I
we know V=IR
Therefore, ϕk=B1A1N1VR1
Voltage sensitivity of coil M1 = B1A1N1kR1
Similarly for coil M2 Voltage sensitivity = B2A2N2kR2
Their ratio of voltage sensitivity of coil M2 to that of coil M1 is:
B2A2N2R1B1A1N1R2=1.4×1014=1
Answer:
The magnetic force on a moving charged particle in a magnetic field is given by FB→=qv→×B→
Since the velocity of the shot electron is perpendicular to the magnetic field, there is no component of velocity along the magnetic field and therefore the only force on the electron will be due to the magnetic field and will be acting as a centripetal force causing the electron to move in a circular path. (if the initial velocity of the electron had a component along the direction of the magnetic field it would have moved in a helical path)
Magnetic field(B)= 6.5G(1G=10−4T)
Speed of electron(v)= 4.8×106ms−1
Charge of electron= −1.6×10−19C
Mass of electron= 9.1×10−31kg
The angle between the direction of velocity and the magnetic field = 90o
Since the force due to the magnetic field is the only force acting on the particle,
mv2r=qv→×B→
mv2r=|qvBsinθ|
r=|mvqBsinθ|
r=9.1×10−31×4.8×1061.6×10−19×6.5×10−4=4.2cm
Answer:
In exercise 4.11 we saw r=eBmv
Time taken in covering the circular path once(time period (T))= 2πrv=2πmeB
Frequency, ν=1T=eB2πm
From the above equation, we can see that this frequency is independent of the speed of the electron.
ν=1.6×10−19×4.8×1062π×9.1×10−31=18.2MHz
Answer:
Number of turns in the coil(n)=30
The radius of the circular coil(r)=8.0 cm
Current flowing through the coil=6.0 A
Strength of magnetic field=1.0 T
The angle between the field lines and the normal of the coil=60o
The magnitude of the counter-torque that must be applied to prevent the coil from turning would be equal to the magnitude of the torque acting on the coil due to the magnetic field.
τ=nBIAsinθ
τ=30×1×6×π×(0.08)2×sin60o=3.13Nm
A torque of magnitude 3.13 Nm must be applied to prevent the coil from turning.
Answer:
From the relation τ=nBIAsinθ we can see that the torque acting on the coil depends only on the area and not its shape, therefore, the answer won't change if the circular coil in (a) were replaced by a planar coil of some irregular shape that encloses the same area.
Moving charges and magnetism NCERT Solutions: Additional Questions
In the Additional Questions of Class 12 Physics Chapter 4 - Moving charges and magnetism, the solutions are done step-by-step in a way that can develop the problem-solving skills and the understanding of the concept. These Moving charges and magnetism class 12 question answers are not just limited to textbook activities, and assist students to master higher-level numericals and to be in good readiness to compete in examinations, like JEE, NEET, and board tests.
Answer:
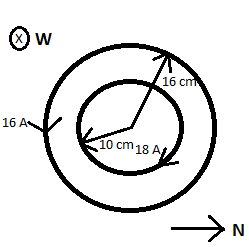
Using the right-hand thumb rule we can see that the direction of the magnetic field due to coil X will be towards the east direction and that due to coil Y will be in the West direction.
We know the magnetic field at the centre of a circular loop of radius r carrying current I is given by
B=μoI2r
Bx=nxμoIx2rx
Bx=20×4π×10−7×162×0.16
Bx=4π×10−4T (towards East)
By=nyμoIy2ry
By=25×4π×10−7×182×0.1
By=9π×10−4T (towards west)
The net magnetic field at the centre of the coils,
Bnet =By - Bx =1.57 × 10-3 T
The direction of the magnetic field at the centre of the coils is towards the west direction.
Answer:
Strength of the magnetic field required is 100G(1G=10−4)T
B=μonI
nI=Bμo
nI=100×10−44π×10−7=7957.74≈8000
Therefore keeping the number of turns per unit length and the value of current within the prescribed limits such that their product is approximately 8000 we can produce the required magnetic field.
e.g. n=800 and I=10 A.
Show that this reduces to the familiar result for the field at the centre of the coil.
Answer:
For a circular coil of radius R and N turns carrying current I, the magnitude of the magnetic field at a point on its axis at a distance x from its centre is given by,
B=μ0IR2N2(x2+R2)3/2
For finding the field at the centre of coil we put x=0 and get the familiar result
B=μ0IN2R
Answer:
Let a point P be at a distance of l from the midpoint of the centres of the coils.
The distance of this point from the centre of one coil would be R/2+l and that from the other would be R/2−l.
The magnetic field at P due to one of the coils would be
B1=μ0IR2N2((R/2+l)2+R2)3/2
The magnetic field at P due to the other coil would be
B2=μ0IR2N2((R/2−l)2+R2)3/2
Since the direction of current in both the coils is same the magnetic fields B1 and B2 due to them at point P would be in the same direction
Bnet =B2+B1Bnet=μ0IR2N2((R/2−l)2+R2)3/2+μ0IR2N2((R/2+l)2+R2)3/2Bnet=μ0IR2N2[((R/2−l)2+R2)−3/2+((R/2+l)2+R2)−3/2]Bnet=μ0IR2N2[(R24−Rl+l2+R2)−3/2+(R24+Rl+l2+R2)−3/2]Bnet=μ0IR2N2[(5R24−Rl+l2)−3/2+(5R24+Rl+l2)−3/2]Bnet=μ0IR2N2×(5R24)−3/2[(1−4l5R+4l25R2)−3/2+(1+4l5R+4l25R2)−3/2]
Since l<<R we can ignore term $l2 /R2
Bnet=μ0IN2R×(54)−3/2[(1−4l5R)−3/2+(1+4l5R)−3/2]
Bnet=μ0IN2R×(45)3/2[1+6l5R+1−6l5R]
Bnet=μ0IN2R×(45)3/2×2
Bnet=0.715μ0INR≈0.72μ0INR
Since the above value is independent of l for small values it is proved that about the midpoint the Magnetic field is uniform.
Answer:
Outside the toroid, the magnetic field will be zero.
Answer:
The magnetic field inside the core of a toroid is given by
B=μoNIl
Total number of turns(N)=3500
Current flowing in toroid =11 A
Length of the toroid,
l=2π(r1+r22)l=π(r1+r2)(r1= inner radius =25 cm,r2= outer radius =26 cm)l=π(0.25+0.26)l=0.51π
B=4π×10−7×3500×110.51π=0.031T
Answer:
The magnetic field in the empty space surrounded by the toroid is zero.
Answer:
The charged particle is not deflected by the magnetic field even while having a non zero velocity, therefore, its initial velocity must be either parallel or anti-parallel to the magnetic field i.e. It's velocity is either towards the east or the west direction.
Answer:
Yes, its final speed will be equal to the initial speed if it has not undergone any collision as the work done by the magnetic field on a charged particle is always zero because it acts perpendicular to the velocity of the particle.
Answer:
The electron would experience an electrostatic force towards the north direction, therefore, to nullify its force due to the magnetic field must be acting on the electron towards the south direction. By using Fleming's left-hand rule we can see that the force will be in the north direction if the magnetic field is in the vertically downward direction.
Explanation:
The electron is moving towards the east and has a negative charge therefore v→ is towards the west direction, Force will be towards south direction if the magnetic field is in the vertically downward direction as F→=qv→×B→
Answer:
The electron has been accelerated through a potential difference of 2.0 kV .
Therefore K.E of electron =1.6×10−19×2000=3.2×10−16 J
12mv2=3.2×10−16v=2×3.2×10−169.1×10−31v=2.67×107 ms−1
Since the electron initially has velocity perpendicular to the magnetic field it will move in a circular path.
The magnetic field acts as a centripetal force. Therefore,
mv2r=evBr=mveBr=9.1×10−31×2.67×1071.6×10−19×0.15=1.01 mm
Answer:
The electron has been accelerated through a potential difference of 2.0 kV .
Therefore K.E of electron =1.6×10−19×2000=3.2×10−16 J
12mv2=3.2×10−16v=2×3.2×10−169.1×10−31v=2.67×107 ms−1
The component of velocity perpendicular to the magnetic field is
vp=vsin30∘vp=1.33×107 ms−1
The electron will move in a helical path of radius r given by the relation,
mvp2r=evpBr=mvpeBr=9.1×10−31×1.33×1071.6×10−19×0.15r=5 mr=5×10−4 mr=0.5 mm
The component of velocity along the magnetic field is
vt=vcos30∘vt=2.31×107 ms−1
The electron will move in a helical path of pitch p given by the relation,
p=2πrvp×vtp=2π×5×10−41.33×107×2.31×107p=5.45×10−3 mp=5.45 mm
The electron will, therefore, move in a helical path of radius 5 mm and pitch 5.45 mm.
Answer:
qE=qvBE=vB…(i)
Let the beam consist of particles having charge q and mass m.
After being accelerated through a potential difference V its velocity can be found out by using the following relation,
12mv2=qVv=2qVm…(ii)
Using the value of v from equation (ii) in (i) we have
E=B2qVmqm=E22VB2qm=(9×10−5)22×15×103×(0.75)2=4.8×10−13
Answer:
In order for the tension in the wires to be zero the force due to the magnetic field must be equal to the gravitational force on the rod.
mg=BIl
mass of rod=0.06 g
length of rod=0.45m
the current flowing through the rod=5 A
B=mgIl
B=0.06×9.85×0.45
B=0.261 T
A magnetic field of strength 0.261 T should be set up normal to the conductor in order that the tension in the wires is zero
Answer:
If the direction of the current is reversed the magnetic force would act in the same direction as that of gravity.
Total tension in wires(T)=Gravitational force on rod + Magnetic force on rod
T=mg+BIlT=0.06×9.8+0.261×5×0.45T=1.176 N
The total tension in the wires will be 1.176 N.
Answer:
Since the distance between the wires is much smaller than the length of the wires we can calculate the Force per unit length on the wires using the following relation.
F=μoI1I22πd
Current in both wires=300 A
Distance between the wires=1.5 cm
Permeability of free space=4 π× 10-7 TmA-1
F=4π×10−7×300×3002π×0.015
F=1.2 Nm-1
Answer:
The length of wire inside the magnetic field is equal to the diameter of the cylindrical region=20.0 cm=0.2 m.
Magnetic field strenth=1.5 T.
Current flowing through the wire=7.0 A
The angle between the direction of the current and magnetic field=90o
Force on a wire in a magnetic field is calculated by relation,
F=BIlsinθ
F=1.5×7×0.2
F=2.1 N
This force due to the magnetic field inside the cylindrical region acts on the wire in the vertically downward direction.
Answer:
Magnetic field strenth=1.5 T.
Current flowing through the wire=7.0 A
The angle between the direction of the current and magnetic field=45o
The radius of the cylindrical region=10.0 cm
The length of wire inside the magnetic field, l=2rsinθ
Force on a wire in a magnetic field is calculated by relation,
F=BIlsinθ
F=1.5×7×2×0.1sin45o×sin45o
F=2.1 N
This force due to the magnetic field inside the cylindrical region acts on the wire in the vertically downward direction.
This force will be independent of the angle between the wire and the magnetic field as we can see in the above case.
Note: There is one case in which the force will be zero and that will happen when the wire is kept along the axis of the cylindrical region.
Answer:
The wire is lowered by a distance d=6cm.
In this case, the length of the wire inside the cylindrical region decreases.
Let this length be l.
(l2)2+d2=r2(l2)2=0.12−0.062(l2)2=0.01−0.0036(l2)2=0.0064l2=0.08l=0.16 mF=BIlsinθF=1.5×7×0.16 F=1.68 N
This force acts in the vertically downward direction.
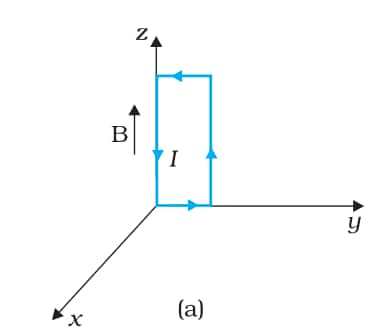
Answer:
The magnetic field is
B→=3000 Gk^=0.3 Tk^
Current in the loop=12 A
Area of the loop = length × breadth
A=0.1 × 0.05
A=0.005 m2
A→=0.005 m2i^
Torque is given by,
τ→=IA→×B→τ→=12×0.005i^×0.3k^τ→=−0.018j^
The torque on the loop has a magnitude of 0.018 Nm and acts along the negative-y direction. The force on the loop is zero.
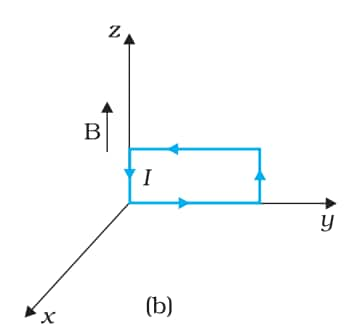
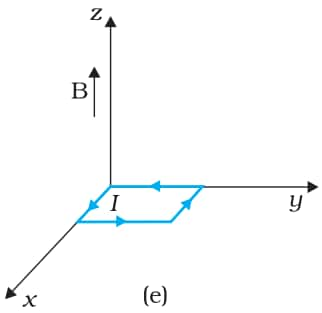
Answer:
The magnetic field is
B→=3000 Gk^=0.3 Tk^
Current in the loop=12 A
Area of the loop = length × breadth
A=0.1 × 0.05
A=0.005 m2
A→=0.005 m2i^ (same as that in the last case)
τ→=IA→×B→τ→=12×0.005i^×0.3k^τ→=−0.018j^
The torque on the loop has a magnitude of 0.018 Nm and acts along the negative-y direction. The force on the loop is zero.
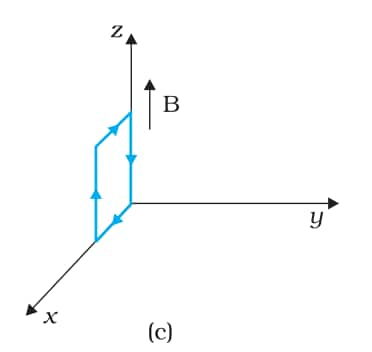
Answer:
The magnetic field is
B→=3000 Gk^=0.3 Tk^
Current in the loop=12 A
Area of the loop = length × breadth
A=0.1 × 0.05
A=0.005 m2
A→=−0.005 m2j^
Torque is given by,
τ→=IA→×B→τ→=12×−0.005j^×0.3k^τ→=−0.018i^
The torque on the loop has a magnitude of 0.018 Nm and acts along the negative-x-direction. The force on the loop is zero.
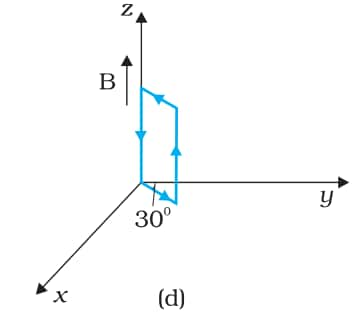
Answer:
The magnetic field is
B→=3000 Gk^=0.3 Tk^
Current in the loop=12 A
Area of the loop = length × breadth
A=0.1 × 0.05
A=0.005 m2
A→=0.005 m2(−i^2+32j^)
Torque is given by,
τ→=IA→×B→τ→=12×0.005(−i^2+32j^)×0.3k^τ→=−0.018(i^2+32j^)
The torque on the loop has a magnitude of 0.018 Nm and at an angle of 240o from the positive x-direction. The force on the loop is zero.
Answer:
The magnetic field is
B→=3000 Gk^=0.3 Tk^
Current in the loop=12 A
Area of the loop = length × breadth
A=0.1 × 0.05
A=0.005 m2
A→=0.005 m2k^
Since the area vector is along the direction of the magnetic field the torque on the loop is zero. The force on the loop is zero.
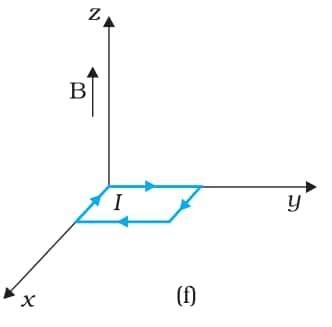
Answer:
The magnetic field is
B→=3000 Gk^=0.3 Tk^
Current in the loop=12 A
Area of the loop = length × breadth
A=0.1 × 0.05
A=0.005 m2
A→=−0.005 m2k^
Since the area vector is in the opposite direction of the magnetic field the torque on the loop is zero. The force on the loop is zero.
The force on the loop in all the above cases is zero as the magnetic field is uniform
Answer:
As we know the torque on a current-carrying loop in a magnetic field is given by the following relation
τ→=IA→×B→
It is clear that the torque, in this case, will be 0 as the area vector is along the magnetic field only.
Answer:
The total force on the coil will be zero as the magnetic field is uniform.
Answer:
The average force on each electron in the coil due to the magnetic field will be eV d B where V d is the drift velocity of the electrons.
The current is given by
I=neAvd
where n is the free electron density and A is the cross-sectional area.
vd=IneA
vd=51029×1.6×10−19×10−5
vd=3.125×10−5ms−1
The average force on each electron is
F=evdB
F=1.6×10−19×3.125×10−5×00.1
F=5×10−25N
Answer:
The magnetic field inside the solenoid is given by
B=μ0nI
n is number of turns per unit length
n=3×3000.6
n=1500 m-1
Current in the wire Iw = 6 A
Mass of the wire m = 2.5 g
Length of the wire l = 2 cm
The windings of the solenoid would support the weight of the wire when the force due to the magnetic field inside the solenoid balances weight of the wire
BIwl=mgB=mgIwlμ0nI=mgIwlI=mgIwlμ0nI=2.5×10−3×9.86×0.02×4π×10−7×1500I=108.37 A
Therefore a current of 108.37 A in the solenoid would support the wire.
Answer:
The galvanometer can be converted into a voltmeter by connecting an appropriate resistor of resistance R in series with it.
At the full-scale deflection current(I) of 3 mA the voltmeter must measure a Voltage of 18 V.
The resistance of the galvanometer coil G = 12Ω
I × (R+G)=18V
R=183×10−3−12
R=6000−12=5988Ω
The galvanometer can be converted into a voltmeter by connecting a resistor of resistance 5988Ω in series with it.
Answer:
The galvanometer can be converted into an ammeter by connecting an appropriate resistor of resistance R in series with it.
At the full-scale deflection current(I) of 4 mA, the ammeter must measure a current of 6 A.
The resistance of the galvanometer coil is G = 15Ω
Since the resistor and galvanometer coil are connected in parallel the potential difference is the same across them.
IG=(6−I)RR=IG6−IR=4×10−3×156−4×10−3R≈0.01Ω
The galvanometer can be converted into an ammeter by connecting a resistor of resistance 0.01Ω in parallel with it.
Class 12 Physics Chapter 4 - Moving charges and magnetism: Higher Order Thinking Skills (HOTS) Questions
The HOTS Question of Class 12 Physics Chapter 4 - Moving charges and magnetism is all about critical thinking and problem solving as opposed to using the standard exercise questions. The solutions take students through tricky situations that enable them to come to terms with the real life application of magnetic fields, force on charges in motion and other laws involved, and gives them confidence in passing tests, even during exams and other competitive tests.
Question 1: A charged particle is thrown into space with a uniform magnetic field and uniform electric field.
Choose the correct possibility of the path of the charged particle.
1) The path of a charged particle may be a straight line, cycloidal or helical.
2) The path of the charged particle may be elliptical.
3) If electric field is removed path of the charge particle must be circular
4) None of the above
Answer:
In a pure uniform electric field, any charged particle will either move in a straight line or a parabolic path.
In a pure uniform magnetic field, any charged particle will either move on a circular path or a helical path.
In the presence of an electric field and magnetic field, the charged particle may move on a straight line if magnetic force balances the electric force
This situation comes in the case of a velocity selector.
If the electric field and magnetic field are parallel then the charge particle will follow a helical path.
If the electric field and magnetic field are perpendicular to each other then the charged particle may follow the cycloidal path.
Hence, the answer is the option (1).
Question 2: A particle of charge ' q ' and mass ' m ' is thrown from origin with velocity v→=vi^+vj^ in the magnetic field B→=Bi^. Find out the correct statement
1) The coordinates of the charge particle at t=2πmqB are x=2πmqB;y=0;z=0
2) The coordinates of the charge particle at t=πmqB are x=πmvqB;y=0;z=−2mvqB
3) The coordinates of the charge particle at t=πm6qB are x=πmv6qB;y=mv2qB;z=−32mvqB
4) All are incorrect
Answer:
The particle will follow the helical path, which has a circular projection in the Y – Z plane and a straight-line motion along the x-axis.
In the Y-Z plane, it will perform circular motion with
R=mvqB and T=2πmqB
and centre of circle is at (Z=−R,Y=0)
At general time its Y and Z coordinate will be
Y=Rsinθ,Z=−R(1−cosθ) and
Its X coordinate increases linearly with speed v.
(a) at t=2π mqB⇒θ=2π⇒x=2πmvqB;y=0;z=0
(b) at t=πmqB⇒θ=π⇒x=πmvqB;y=0;z=(−2mvqB)
(c) at t=πm6qB⇒θ=π/6⇒x=πmv6qB;y=mv2qB;z=−mvqB(1−32)
Hence, the answer is the option(1) and option(2).
Question 3: Choose the correct statement about magnetic field.
1) Any charged particle in the magnetic field must experience a force due to it.
2) Any moving charge particle in the magnetic field must experience force due to it.
3) The work done by magnetic force on a moving charge particle is always zero.
4) The work done by magnetic force on a current carrying wire maybe non-zero.
Answer:
The magnetic field can apply force on a moving charge particle if it's velocity is not parallel to magnetic field.
Result can be easily viewed on basis of Lorentz force.
It says the force is obtained by cross product of velocity of charge particle and magnetic field.
Magnetic force never does any work, neither on charge nor on current carrying wire.
Hence, the answer is the option (3).
Question 4: Two proton beams are moving parallel to each other with same velocity v along their beam direction at some separation. Find out the correct option(s):
1) Ratio of electrostatic repulsive and magnetic attraction force is c2v2
2) Ratio of electrostatic repulsive and magnetic attraction force is v2c2
3) Electrostatic force per unit length of any beam is 2Kn2e2r
Magnetic force per unit length of any beam is μ0n2e2v22πr
4) Electrostatic force per unit length of any beam is μ0n2e2v22πr
Magnetic force per unit length of any beam is 2Kn2e2r
Answer:
Let there be n protons in unit length
So charge / length, λ= ne
Effective current in proton beam = nev
Now electrostatic force per unit length of any beam
Fe=2 Kλ1λ2r=2 K(ne)(ne)r
Magnetic force per unit length of any beam
Fm=μ0i1i22πr=μ0(nev)(nev)2πr So, Fe Fm=1μ0∈0v2=c2v2
Hence, the correct answer is the option(1) and (3).
Question 5: A proton, a deuterium nucleus, and an alpha particle are accelerated through the same potential difference. Now, these particles are sent in a uniform magnetic field perpendicularly. Choose the correct ratio(s):
1) Their radii of circles =1:2:2
2) Their angular momentum =1:2:4
3) Their frequencies of periodic motion =2:1:1
4) All the above are correct
Answer:
If charge ' q ' is accelerated through potential difference ΔV, its kinetic energy will be :
K.E. =qΔ V⇒12mv2=qΔ V
In a magnetic field,
r=mvqB;L=m2v2qB;f=qB2πm
Proton, deuterium, and a particle have charge and mass as (e,m)(e,2m)&(2e,4m) respectively.
(a) So, their ratio of radii :
rp:rd:rα=2meΔVeB:4meΔVeB:16meΔV2eB⇒rp:rd:rα=1:
2:2
(b) Their ratio of angular momentum :
Lp:Ld:Lα=2meΔVeB:4meΔVeB:16meΔV2eB⇒Lp:Ld:Lα=1:
(c) Their ratio of frequencies:
fp:fd:fα=eB2πm:eB4πm:2eB8πm⇒fp:fd:fα=2:1:1
Hence, the answer is the option (4).
CBSE Class 12th Syllabus: Subjects & Chapters
Select your preferred subject to view the chapters
Moving Charges and Magnetism Class 12 Question Answers: Topics
Class 12 Physics Chapter 4 - Moving charges and magnetism addresses the nature of magnetic fields generated by moving electric charges, the properties of such fields, how they interact with other moving charges, and with currents. It prepares the ground to study the magnetic effects of current, which play an essential role both in electromagnetism and in modern technology.
4.1 Introduction
4.2 Magnetic Force
4.2.1 Sources And Fields
4.2.2 Magnetic Field, Lorentz Force
4.2.3 Magnetic Force On A Current-Carrying Conductor
4.3 Motion In A Magnetic Field
4.4 Magnetic Field Due To A Current Element, Biot-Savart Law
4.5 Magnetic Field On The Axis Of A Circular Current Loop
4.6 Ampere’S Circuital Law
4.7 The Solenoid
4.8 Force Between Two Parallel Currents, The Ampere
4.9 Torque On Current Loop, Magnetic Dipole
4.9.1 Torque On A Rectangular Current Loop In A Uniform Magnetic Field
4.9.2 Circular Current Loop As A Magnetic Dipole
4.10 The Moving Coil Galvanometer
Class 12 Physics Chapter 4 - Moving charges and magnetism: Important Formulas
The Important Formulas section of Class 12 Physics Chapter 4 - Moving charges and magnetism has all the important equations in a single section, and therefore, the student can easily revise and solve numerical problems like a machine. Such equations deal with concepts such as magnetic force on a moving charge, Biot-Savart Law, Ampere Circuital Law, force between current carrying wires, and acceleration of charged particles in magnetic fields.
1. Magnetic Force on a Moving Charge (Lorentz Force):
F=q(v→×B→)
Where:
F= Force on the charge
q= Charge of the particle
v→= Velocity of the particle
B→= Magnetic field
The force is perpendicular to both the velocity and magnetic field.
2. Magnetic Force on a Current-Carrying Conductor:
F=ILBsinθ
Where:
I= Current
L= Length of the conductor
B= Magnetic field
θ= Angle between the magnetic field and the conductor
3. Magnetic Field due to a Current-Carrying Conductor (Biot-Savart Law):
dB=μ04πIdlsinθr2
Where:
dB= Small magnetic field element
I= Current
dl= Small length of the conductor
r= Distance from the point to the wire
θ= Angle between dl and the line joining the point and element
4. Magnetic Field due to a Long Straight Current-Carrying Wire:
B=μ0I2πr
Where:
I= Current
r= Distance from the wire
B= Magnetic field at a distance r from the wire
5. Ampere's Law:
∮B→⋅dl→=μ0Ienc
Where:
∮B→⋅dl→= Line integral of the magnetic field
μ0= Permeability of free space
Ienc= Total enclosed current
6. Magnetic Field due to a Circular Loop:
B=μ0I2R at the center of the loop
Where:
I= Current in the loop
R= Radius of the loop
7. Force between Two Parallel Current-Carrying Conductors:
F=μ0I1I2L2πr
Where:
I1,I2= Currents in the two wires
L= Length of the wire
r= Distance between the wires
Approach to Solve Questions of Class 12 Physics Chapter 4 - Moving charges and magnetism
The answers to questions in Class 12 Physics Chapter 4 - Moving Charges and Magnetism need to be approached in a systematical manner. By concentrating on relevant physical quantities, appropriate choice of laws, and visualization of directions of forces and fields, one can effectively address numerical and conceptual problems with a high degree of accuracy and excellent understanding of underlying concepts.
Learn about the Concept First
- Revise simple concepts such as magnetic force on a moving charge, magnetic field resulting from a current element (Biot-Savart Law), and Ampere Circuital Law.
- Obtain the directions of the vectors through the use of the right-hand rule.
JEE Main Highest Scoring Chapters & Topics
Just Study 40% Syllabus and Score upto 100%
Download EBookMake Neat Diagram
- When loop or wires are carrying current draw the correct diagrams indicating the current flow and magnetic field lines.
Apply Right-Hand Rules
- The direction of magnetic field caused by current may by found by right-hand thumb rule.
- Use the rule of Lorentz force (F = q(v x B)) on moving charge.
Determine the required Formula
- Identify the concept underlying the question:
- Moving charge and magnetic force
- Biot-Savart Law
- The forces acting on a current-carrying wire
- Movement of charged particles in magnetic fields
- Applications of Ampere law
What Extra Should Students Study Beyond the NCERT for JEE/NEET?
In order to succeed in competitive exams such as JEE and NEET, students must look beyond NCERT and learn more about the practice of concepts in Class 12 Physics Chapter 4 - Moving charges and magnetism. These involve solving complicated numerical problems, learning relations in the magnetic fields and practising derivations and real world application to reinforce problem solving capabilities and conceptual clarity.
Importance in Exams:
As the CBSE board exam is concerned, the solutions of NCERT Class 12 Physics chapter 4 Moving Charges and Magnetism is important. In CBSE board exams, 12 % of questions are asked from chapters 4 and 5. Same questions discussed in the chapter 4 Physics Class 12 NCERT solutions can be expected in the board exams.
NCERT Solutions for Class 12 Physics: Chapter-Wise
The NCERT Solutions for Class 12 Physics are compiled in a chapter-wise format to make learning and revision simple. Each chapter link provides step-by-step solutions to textbook exercises, additional questions, HOTS, and key formulas. With these well-structured resources, students can prepare effectively for CBSE board exams as well as competitive exams like JEE and NEET.
Also, check NCERT Books and NCERT Syllabus here:
- NCERT Books Class 12 Physics
- NCERT Syllabus Class 12 Physics
- NCERT Books Class 12
- NCERT Syllabus Class 12
NCERT solutions subject-wise
- NCERT solutions for class 12 mathematics
- NCERT solutions for class 12 chemistry
- NCERT solutions for class 12 physics
- NCERT solutions for class 12 biology
Also, check NCERT Exemplar Class 12 Solutions
Frequently Asked Questions (FAQs)
Q: What is the Biot Savart law and why is it important?
A:
The BiotSavart Law is useful to determine the magnetic field of a small element of current at a point in space. It is critical to the study of magnetic action of currents in wires and loops.
Q: What is the contribution of Ampere's Circuital Law in solving problems?
A:
The Ampere's Circuital Law is used to calculate the magnetic field in the field around a closed loop in relation to the total current flowing through the loop simplifying the computations of symmetrical distributions of current such as solenoids and toroids.
Q: Are these concepts applicable in real life devices?
A:
Indeed, this chapter has concepts that are used in electric motors, solenoids, generators, loudspeakers, and MRI machines.
Q: What are the methods of solving numerical problems in this chapter?
A:
Apply a mix of magnetic field, magnetic force formulae, Ampere law and right hand/left hand rule. Demonstrate through draw diagrams to get a clear picture of the directions of fields and forces.
Q: Are the NCERT solutions enough to prepare for exams?
A:
Yes, the NCERT solutions cover all textbook questions with step-by-step explanations, making them sufficient for CBSE exams.
Articles
Related Stories
|
Upcoming School Exams
Certifications By Top Providers
Explore Top Universities Across Globe
Questions related to CBSE Class 12th
On Question asked by student community
Have a question related to CBSE Class 12th ?
Yes, you can switch from Science in Karnataka State Board to Commerce in CBSE for 12th. You will need a Transfer Certificate from your current school and meet the CBSE school’s admission requirements. Since you haven’t studied Commerce subjects like Accountancy, Economics, and Business Studies, you may need to catch up before or during 12th. Not all CBSE schools accept direct admission to 12th from another board, so some may ask you to join Class 11 first. Make sure to check the school’s rules and plan your subject preparation.
Hello
For the 12th CBSE Hindi Medium board exam, important questions usually come from core chapters like “Madhushala”, “Jhansi ki Rani”, and “Bharat ki Khoj”.
Questions often include essay writing, letter writing, and comprehension passages. Grammar topics like Tenses, Voice Change, and Direct-Indirect Speech are frequently asked.
Students should practice poetry questions on themes and meanings. Important questions also cover summary writing and translation from Hindi to English or vice versa.
Previous years’ question papers help identify commonly asked questions.
Focus on writing practice to improve handwriting and presentation. Time management during exams is key to answering all questions effectively.
Hello,
If you want to improve the Class 12 PCM results, you can appear in the improvement exam. This exam will help you to retake one or more subjects to achieve a better score. You should check the official website for details and the deadline of this exam.
I hope it will clear your query!!
For the 2025-2026 academic session, the CBSE plans to conduct board exams from 17 February 2026 to 20 May 2026.
You can download it in pdf form from below link
all the best for your exam!!
Hii neeraj!
You can check CBSE class 12th registration number in:
- Your class 12th board exam admit card. Please do check admit card for registration number, it must be there.
- You can also check the registration number in your class 12th marksheet in case you have got it.
- Alternatively you can also visit your school and ask for the same in the administration office they may tell you the registration number.
Hope it helps!
Popular CBSE Class 12th Questions
A block of mass 0.50 kg is moving with a speed of 2.00 ms-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
A particle is projected at 600 to the horizontal with a kinetic energy . The kinetic energy at the highest point
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
In the reaction,
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
How many moles of magnesium phosphate, will contain 0.25 mole of oxygen atoms?
| Option 1)
0.02 |
Option 2)
3.125 × 10-2 |
| Option 3)
1.25 × 10-2 |
Option 4)
2.5 × 10-2 |
Colleges After 12th
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters
CBSE Class 12th Notifications
Never miss an CBSE Class 12th update
Get timely CBSE Class 12th updates directly to your inbox. Stay informed!