Motion Class 9th Notes - Free NCERT Class 9 Science Chapter 8 Notes - Download PDF

Have you ever been in a train journey when you saw that a train gains pace slowly and rides at a steady pace and then again decelerates as it comes close to a station? This daily observation reflects an ideal real life scenario of the concepts of motion including speed, velocity and acceleration which are topics of focus in Chapter 7: Motion in Class 9 Science. The CBSE Class 9 Science Chapter 7 Motion Notes are an effective study aid not only at the Class 9 level but also as a conceptual stepping stones to the knowledge covered in greater detail in Classes 11 and 12- particularly in mechanics. These notes are provided by subject matter experts at Careers360 and simplify complex concepts into easy-to-understand explanations so that students can learn about how objects move and the reasons behind the movements.

The chapter is particularly significant to students taking competitive exams such as JEE, NEET and Olympiads, since the chapter will introduce them to the fundamentals of kinematics, which is the backbone of physics. Important topics covered in Class 9 Science Notes Chapter 7 include displacement and distance, acceleration, speed and velocity, uniform and non uniform motion, representation of motion graphically, motion equations and applications. These notes give an organized brief review, and include clear definitions of terms and quantities, key formulas and derivations, properly labeled diagrams and graphs, the important concepts with examples and exam practice questions. Apart from assisting in the preparation of CBSE exams, these revision-oriented notes will also provide a strong basis in studying more about physics in advanced classes as well as in competitive examinations.

Also, students can refer,

• NCERT notes Class 9 Science
• NCERT Solutions for Class 9 Science Chapter 7 Motion
• NCERT Exemplar Class 9 Science Chapter 7 Solutions Motion

NCERT Class 9 Science Chapter 7 Notes

Some objects are stationary while others are moving throughout our daily lives. Birds can fly, fish can swim, blood can flow via veins and arteries, and automobiles can move. Atoms, molecules, planets, stars, and galaxies are all moving. When an object's position varies over time, we frequently interpret it as moving. The majority of motions are complicated. Some objects move in a straight line, while others move in a circle. Some of them may rotate, while others may vibrate. There may be times when you need to use a combination of these.

Motion Along a Straight Line

• A straight-line motion is the most basic sort of motion. Distance and Displacement are two separate quantities used to represent an object's overall motion and to locate its end position in relation to its initial position at a given moment.
• In physics, distance refers to the length of an object's path (the line or curve) through space.
• Distance is not dependent on direction. Scalars are physical quantities that can be described completely without the need for direction.
• The shortest distance between a body's initial and final positions, as well as its direction, is called displacement as it moves from one location to another.
• Because displacement requires both direction and magnitude to be fully described, such physical quantities are referred to as vectors.
• A moving body's distance travelled cannot be zero, yet its eventual displacement can be zero.

Uniform Motion and Non-Uniform Motion

• A body is said to be in uniform motion if it travels equal distances at equal intervals of time. Non-uniform motion occurs when a body traverses unequal distances in equal intervals or equal distances in unequal intervals.

Speed

• The total distance travelled by the object during the time interval in which the motion occurs is defined as its speed. The metric unit of speed is the meter per second. So, Speed=Distance travelled/time taken=s/t

Here’s is the distance travelled by the object and t is the time taken by the object to travel the distance s. The speed of a body tells us how slowly or quickly it is moving. The average speed of a body is determined by the ratio of the total distance travelled to the total time spent by the body. A body's instantaneous speed is its speed at a given instant. If a body travels the same distance in equal intervals of time, it is said to have constant or uniform speed.

Velocity

• The rate at which a body's displacement changes with time is referred to as its velocity.
• In SI units, an object's velocity is measured in meters per second.
• Velocity is simply the speed of an object moving in a specific direction. An object's velocity can be either uniform or variable. It can be altered by varying the speed, direction, or both of the object's motion. So velocity is a vector quantity, whereas speed is a scalar quantity with only magnitude and no specific direction.
• When a body travels the same distance in the same period of time in the same direction, it is said to be moving at uniform velocity. If a body covers unequal distances in equal intervals of time and vice versa in a specified direction, or if it changes direction, it is said to be moving with non-uniform velocity.
• A body's velocity can be changed in two ways: first, by changing its speed, and second, by changing its direction of motion while keeping its speed constant. In order to change the velocity of the body, both the speed and direction of the body can be changed.
• When the velocity of an object changes at a constant rate, the arithmetic mean of the initial and final velocity for a given period of time is used to calculate average velocity, which is, average velocity=(u+v)/2

where u is the initial velocity of the body and v is the final velocity of the body.

Acceleration

• The change in velocity of an object per unit time is called acceleration. It is given as,

Acceleration=Change in velocity/time taken

• If the velocity of a body varies from an initial value u to a final value v in time t. acceleration ‘a’ is given by,

a = (v-u)/t

• If a body moves in a straight path and its velocity increases by the same amount every time interval, it is said to have uniform acceleration. Examples include freely falling bodies, balls rolling down an inclined plane, and so on.
• If a body's velocity changes by an unequal amount at equal periods of time, it is said to have non uniform acceleration.
• Positive acceleration occurs when acceleration occurs in the direction of velocity; negative acceleration occurs when acceleration occurs in the opposite direction of velocity; negative acceleration is known as retardation.

Equations of Motion

1. First Equation of motion

• v=u+at, is the first equation of motion, where v is the final velocity and u is the body's initial velocity. The first equation of motion determines the body's velocity at any time t.

We know, Acceleration= Change in velocity time taken

So, a = (v-u)/t

∴ at=v-u

Now, rearranging the above equation we get,

v=u+at

In this manner, we get the first equation of motion.

2. Second Equation of motion:

• s=ut+at²/2 is the second equation of motion. ‘u’ is the initial velocity here,‘s’ is distance travelled by the body in time ‘t’ and ‘a’ is uniform acceleration.

Now consider the average velocity formula, when the velocity of an object changes at a constant rate. That is,

average velocity=(u+v)/2

Distance travelled by an object is,

s= (u+v)t/2

The first equation of motion is, v=u+at

Substituting the first equation of motion in the above equation, we get

s=(u+u+at)t/2

=(2u+at)t/2

=(2ut+at²)/2

Rearranging the above equation we get,

s=ut+at²/2

3. Third Equation of motion:

• v²=u²+2as is the third equation of motion. ‘u’ is the initial velocity here, ‘v’ is the final velocity. ‘s’ is distance travelled by body in time ‘t’ and ‘a’ is uniform acceleration.

This equation gives the body's velocity as it travels a distance of s.

v=u+at And s=ut+at²/2 are the first and second equations of motions respectively.

Rearranging the first equation we get,

t=(v-u)/a

Substituting this in the second equation of motion we get,

s=u((v-u)/a + a((v-u)/a)²/2

s=(2uv-2u²+v²+u²-2uv)/2a

Rearranging the above equation we get,

v²=u²+2as

• The following three crucial points should be remembered while addressing uniformly accelerated motion problems using these three equations of motion.

1. u=0 is the initial velocity of a body starting from rest.
2. When a body comes to a complete stop, its final velocity is equal to zero.
3. The acceleration of a body moving at a constant velocity is zero.

Graphical representation of motion

• A graph depicts the relationship between two sets of data, one of which contains dependent variables and the other of which contains independent variables.
• Line graphs can be used to depict the motion of an object. Line graphs in this case show the relationship between one physical quantity, such as distance or velocity, and another physical quantity, such as time.

1. Distance Time Graphs

• On a distance-time graph, the change in an object's position over time can be represented.
• The x-axis in this graph represents time, while the y-axis represents distance.
• Because they precisely depict velocity, distance time graphs of a moving body can be utilized to compute the speed of the body.
• As illustrated in Figure a, the distance time graph for a body moving at a constant speed is always a straight line since the distance travelled by the body is directly proportional to time.
• The distance time graph for a body travelling at a non-uniform speed is depicted in figure b below as a curve.

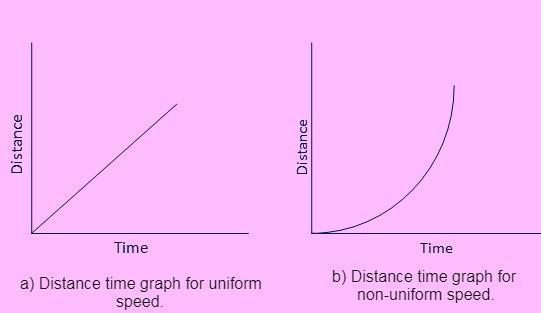
• When the object is at rest, the distance time graph is parallel to the time axis as shown in below figure a),

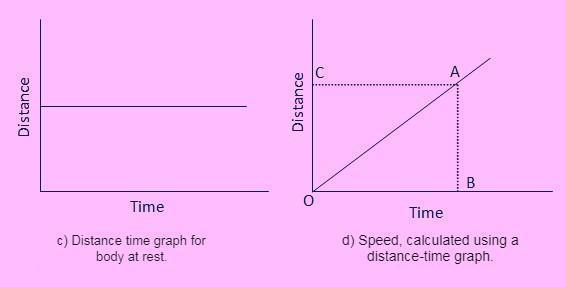
To determine the body's speed from a distance time graph [figure d)], say at point A, first draw a perpendicular AB on the time axis and a perpendicular AC on the distance axis, so that AB represents the body's distance travelled in time interval OB and we know,

Speed=Distance travelled/time taken=s/t=AB/OB

2. Velocity time graphs

• A velocity-time graph depicts the variation in velocity with time for an object travelling in a straight line. Time is shown on the x-axis, while velocity is displayed on the y-axis in this graph. The displacement of an object travelling with uniform velocity is given by the product of velocity and time. The magnitude of the displacement will be equal to the area contained by the velocity-time graph and the time axis.
• If a body moves at a steady velocity, its velocity time graph will be a straight line parallel to the time axis, as seen in Figure (e).

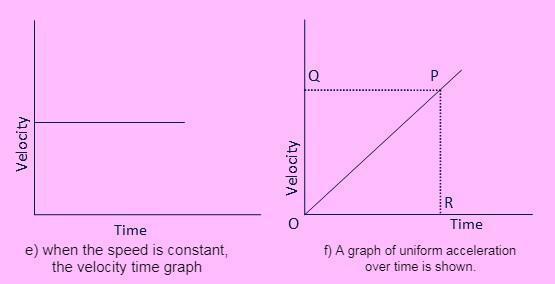
Figure f) depicts a straight-line velocity time graph with uniformly changing velocity. The velocity-time graph can be used to determine the value of acceleration.

Draw a perpendicular RP from point R to calculate acceleration at the time corresponding to point R, as shown in figure f).

Acceleration=Change in velocity/time taken

The time taken is equal to OR, and the change in velocity is indicated by PR.

Acceleration=PR/OR

This is the same as the velocity time graph's slope. As a result, we can deduce that the slope of a moving body's velocity-time graph determines its acceleration.

As shown in Figure f), the distance travelled by a moving body in a given time is equal to the area of the triangle OPR.

Distance travelled=Area of triangle OPR

=0.5Area of rectangle ORPQ

Thus, Distance travelled=(OQ)(OR)/2

When a body's velocity varies in an irregular pattern, the body's velocity-time graph is a curved line.

Equations of motion by graphical method

When an object moves in a straight path with uniform acceleration, we already understand equations of motion. We already know how to calculate them, but a graphical way can also be used.

1. Equation for velocity time relation:

Consider the velocity-time graph of an object moving with uniform acceleration, as seen in Figure a).

The initial velocity of the object is u (at point A) and rises to v (at point B) in time t, as shown by this graph. The velocity is changing at a constant rate of a.

Again, the time‘t’ is represented by OC, the initial velocity u by OA, and the final velocity of the body after time t by BC in the figure.

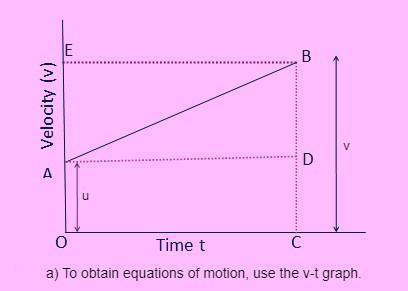
The graph in figure a) clearly shows that BC=BD+DC=BD+OA. As a result,

We have v = BD+u ……………………………… (1)

We should now determine the value of BD. The object's acceleration from the velocity-time graph is given by,

Acceleration=Change in velocity time taken=BDAD=BDOC=BDt

We get, BD=at

Substituting the value of BD in eqn (1) we get,

v=u+at

Which is the first equation of motion and velocity time relation.

2. Equation for position time relation:

Assume the body has travelled s distance in time t with uniform acceleration a. The area encompassed within OABC under the velocity-time graph AB in Fig. (a) is used to calculate the distance travelled by the body.

Thus the object travels a distance, which is given by,

s = Area of OABC (trapezium).

s = area of the rectangle OADC + area of the triangle ABD.

s=OA x OC+(AD)(BD/2

Putting OA= u, OC = t and BD = at, we have

s=(u x t)+(t x at)/2

Then we get,

s=ut+at²/2

This is an equation of position-time relation.

3. Equation for position velocity relation:

Consider the graph in Figure (a). We know that the area under line AB, which is the area of trapezium OABC, gives the distance s travelled by a body in time t.

Thus, we have.

distance travelled by object= s=Area of trapezium OABC

s=(sum of parallel sides x height)/2=(OA+CB) x OC/2

we have, OA+CB=u+ v and OC=t, so we have

s=(u+v)t/2

By velocity time relation, t=(v-u)/a

Substituting this ‘t’ in equation for ‘s’ we have,

s=(u+v)(v-u)t/2a

Therefore we have,

v²=u²+2as

This is an equation for position velocity relation.

Uniform circular motion

• The motion of an object is called uniform circular motion when it moves along a circular route at a constant speed.
• Many examples of circular motion may be found in our daily lives, such as vehicles driving around a circular track and many others.
• In addition, the earth and other planets orbit the sun in roughly circular orbits.
• Even if the speed of a particle moving in a circular motion remains constant, the particle accelerates due to the continually changing direction of the velocity. A uniform circular motion occurs when an object moves in a circular route at a constant speed.
• In circular motion, we use angular velocity instead of velocity as we did in linear motion. The force required to move a body along a circular route is referred to as centripetal force.
• We know that 2πr equals the circumference of a circle of radius r. If a body takes t seconds to move once around a circular path of radius r, the velocity v is given by v =2πrt.
• Although uniform linear motion is not accelerated, uniform circular motion is.

Motion Previous year Question and Answer

Q1: A particle is moving with constant acceleration from A to B in a straight line AB. If u and v are the velocities at A and B, respectively, then its velocity at the midpoint C will be:

Answer:

If the length of $AB=s$, then mispoint of $AB$ is at $s/2$
The velocity of the particle changes from $u$ to $v$ when it moves through the distance $s$

$a=\frac{v^2-u^2}{2s}$

Velocity at midpoint is

$v_c^2=u^2+\frac{2as}{2}\dots$

Substituting (1) in (2) we get

$\begin{array}{rl}& v_c^2=\frac{v^2+u^2}{2}\\ & v_c=\sqrt{\frac{v^2+u^2}{2}}\end{array}$

Q2: The velocity-time plot for a particle moving on a straight line is shown in figure.

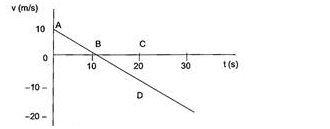
(a) The particle has a constant acceleration

(b) The particle has never turned around.

(c) The particle has zero displacement.

(d) The average speed in the interval 0 to 10s is the same as the average speed in the interval 10s to 20s.

Which of the above statements are correct?

Answer:

Since the velocity-time graph is a straight line, the slope of this straight line is constant, and hence the particle has a constant acceleration, and the areas OAB and BCD are the same, so the average speeds in the intervals 0 to 10 s and 10 s to 20 s are the same.

Hence, the correct options are (a) and (d)

Q3: The graph below shows the distance travelled and the time taken by four cars.

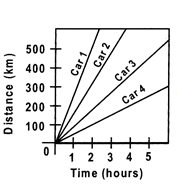
Which car travelled the slowest?

Answer:

The slope of the distance-time graph represents the velocity. The slope for car 4 is minimum, so its speed is also minimum.

Hence, car 4 travels the slowest.

NCERT Solutions of Class 9 Subject-Wise

• NCERT solutions for class 9 Maths
• NCERT solutions for class 9 Science

NCERT Class 9 Exemplar Solutions for Other Subjects:

• NCERT Exemplar Class 9 Maths solutions
• NCERT Exemplar Class 9 Science solutions

Class 9 Chapter Wise Notes