NCERT Solutions for Exercise 9.3 Class 9 Maths Chapter 9 - Areas Of Parallelograms And Triangles

Edited By Safeer PP | Updated on Jul 29, 2022 06:20 PM IST

The NCERT Solutions for Class 9 Maths exercise 9.3 deal with triangles rather than parallelograms. In Class 9 Maths chapter 9 exercise 9.3 we deal with triangles that have a common base and are in the same parallels.

The important concepts related to NCERT solutions for Class 9 Maths chapter 9 exercise 9.3 in order to solve this exercise are:

Triangles that have the same base or the equal base and are between the same parallel lines have equivalent areas. This is on the grounds that we realize that the space of the triangle is half of the product of base and tallness since the height (distance between the parallels) remains the same for the two triangles and they have the same bases thus their areas are equal.
Scholarship Test: Vidyamandir Intellect Quest (VIQ)
Don't Miss: JEE Main 2027: Narayana Scholarship Test Preparation Kit for Class 9
This Story also Contains

The figure shown below is a set of triangles with the same base and between the same parallel

ex9

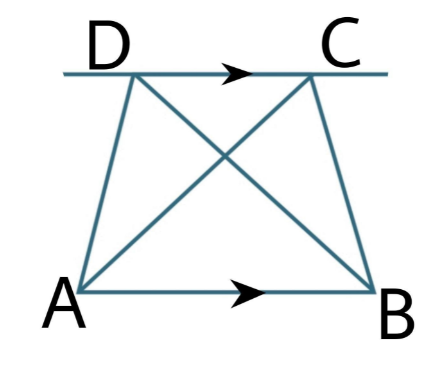
The triangles ABC and BCD have the same area.

Along with NCERT book exercise 9.3 Class 9 Maths, the following exercises are also present.

Areas Of Parallelograms And Triangles Class 9 Chapter 9 Exercise: 9.3

Q1 In Fig. $\small 9.23$ , E is any point on median AD of a $\small \Delta ABC$ . Show that. $\small ar(ABE)=ar(ACE)$

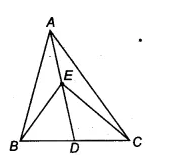
1595869014446

Answer:
We have $\Delta$ ABC such that AD is a median. And we know that median divides the triangle into two triangles of equal areas.
Therefore, ar( $\Delta$ ABD) = ar( $\Delta$ ACD)............(i)

Similarly, In triangle $\Delta$ BEC,
ar( $\Delta$ BED) = ar ( $\Delta$ DEC)................(ii)

On subtracting eq(ii) from eq(i), we get
ar( $\Delta$ ABD) - ar( $\Delta$ BED) =
$\small ar(ABE)=ar(ACE)$

Hence proved.

Q2 In a triangle ABC, E is the mid-point of median AD. Show that $\small ar(BED)=\frac{1}{4}ar(ABC)$ .

Answer:

We have a triangle ABC and AD is a median. Join B and E.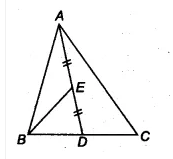
1595869100838
Since the median divides the triangle into two triangles of equal area.
$\therefore$ ar( $\Delta$ ABD) = ar ( $\Delta$ ACD) = 1/2 ar( $\Delta$ ABC)..............(i)
Now, in triangle $\Delta$ ABD,
BE is the median [since E is the midpoint of AD]
$\therefore$ ar ( $\Delta$ BED) = 1/2 ar( $\Delta$ ABD)........(ii)

From eq (i) and eq (ii), we get

ar ( $\Delta$ BED) = 1/2 . (1/2 ar(ar ( $\Delta$ ABC))
ar ( $\Delta$ BED) = 1/4 .ar( $\Delta$ ABC)

Hence proved.

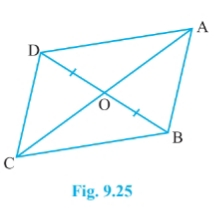
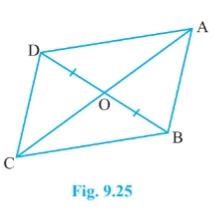
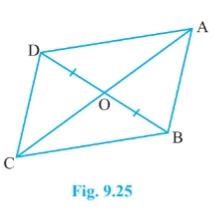
Q3 Show that the diagonals of a parallelogram divide it into four triangles of equal area.

Answer:

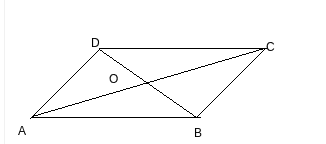
1595869168733
Let ABCD is a parallelogram. So, AB || CD and AD || BC and we know that Diagonals bisects each other. Therefore, AO = OC and BO = OD

Since OD = BO
Therefore, ar ( $\Delta$ BOC) = ar ( $\Delta$ DOC)...........(a) ( since OC is the median of triangle CBD)

Similarly, ar( $\Delta$ AOD) = ar( $\Delta$ DOC) ............(b) ( since OD is the median of triangle ACD)

and, ar ( $\Delta$ AOB) = ar( $\Delta$ BOC)..............(c) ( since OB is the median of triangle ABC)

From eq (a), (b) and eq (c), we get

ar ( $\Delta$ BOC) = ar ( $\Delta$ DOC)= ar( $\Delta$ AOD) = ( $\Delta$ AOB)

Thus, the diagonals of ||gm divide it into four equal triangles of equal area.

Q4 In Fig. $\small 9.24$ , ABC and ABD are two triangles on the same base AB. If line- segment CD is bisected by AB at O, show that $\small ar(ABC)=ar(ABD)$ .

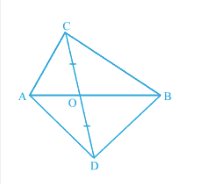
1595869232559
Answer:

We have, $\Delta$ ABC and $\Delta$ ABD on the same base AB. CD is bisected by AB at point O.
$\therefore$ OC = OD
Now, in $\Delta$ ACD, AO is median
$\therefore$ ar ( $\Delta$ AOC) = ar ( $\Delta$ AOD)..........(i)

Similarly, in $\Delta$ BCD, BO is the median
$\therefore$ ar ( $\Delta$ BOC) = ar ( $\Delta$ BOD)............(ii)

Adding equation (i) and eq (ii), we get

ar ( $\Delta$ AOC) + ar ( $\Delta$ BOC) = ar ( $\Delta$ AOD) + ar ( $\Delta$ BOD)

$\small ar(ABC)=ar(ABD)$

Hence proved.

Q5 (i) D, E and F are respectively the mid-points of the sides BC, CA and AB of a $\small \Delta ABC$ . Show that BDEF is a parallelogram.

Answer:

We have a triangle $\Delta$ ABC such that D, E and F are the midpoints of the sides BC, CA and AB respectively.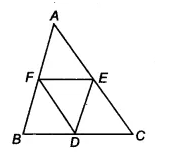
1595869300862
Now, in $\Delta$ ABC,
F and E are the midpoints of the side AB and AC.
Therefore according to mid-point theorem, the line segment connecting the midpoints of two sides of a triangle is parallel to the third side and half of the third side.
$\therefore$ EF || BC or EF || BD

also, EF = 1/2 (BC)
$\Rightarrow EF = BD$ [ D is the midpoint of BC]
Similarly, ED || BF and ED = FB
Hence BDEF is a parallelogram.

Q5 (ii) D, E and F are respectively the mid-points of the sides BC, CA and AB of a $\small \Delta ABC$ . Show that

$\small ar(DEF)=\frac{1}{4}ar(ABC)$

Answer:

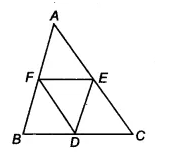
1595869319420
We already proved that BDEF is a ||gm.
Similarly, DCEF and DEAF are also parallelograms.
Now, ||gm BDEF and ||gm DCEF is on the same base EF and between same parallels BC and EF
$\therefore$ Ar (BDEF) = Ar (DCEF)
$\Rightarrow$ Ar( $\Delta$ BDF) = Ar ( $\Delta$ DEF) .............(i)

It is known that diagonals of ||gm divides it into two triangles of equal area.
$\therefore$ Ar(DCE) = Ar (DEF).......(ii)

and, Ar( $\Delta$ AEF) = Ar ( $\Delta$ DEF)...........(iii)

From equation(i), (ii) and (iii), we get

Ar( $\Delta$ BDF) = Ar(DCE) = Ar( $\Delta$ AEF) = Ar ( $\Delta$ DEF)

Thus, Ar ( $\Delta$ ABC) = Ar( $\Delta$ BDF) + Ar(DCE) + Ar( $\Delta$ AEF) + Ar ( $\Delta$ DEF)
Ar ( $\Delta$ ABC) = 4 . Ar( $\Delta$ DEF)
$\Rightarrow$ $ar(\Delta DEF) = \frac{1}{4}ar(\Delta ABC)$

Hence proved.

Q5 (iii) D, E and F are respectively the mid-points of the sides BC, CA and AB of a $\small \Delta ABC$ . Show that

$\small ar(BDEF)=\frac{1}{2}ar(ABC)$

Answer:

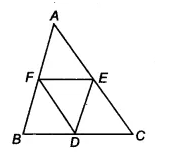
1595869344427
Since we already proved that,
ar( $\Delta$ DEF) = ar ( $\Delta$ BDF).........(i)

So, ar(||gm BDEF) = ar( $\Delta$ BDF) + ar ( $\Delta$ DEF)
= 2 . ar( $\Delta$ DEF) [from equation (i)]
$\\= 2[\frac{1}{4}ar(\Delta ABC)]\\ =\frac{1}{2} ar(\Delta ABC)$

Hence proved.

Q6 (i) In Fig. $\small 9.25$ , diagonals AC and BD of quadrilateral ABCD intersect at O such that. If $\small AB=CD$ , then show that:

$\small ar(DOC)=ar(AOB)$

[ Hint: From D and B, draw perpendiculars to AC.]

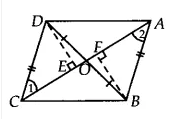
1640236346940 Answer:
We have ABCD is quadrilateral whose diagonals AC and BD intersect at O. And OB = OD, AB = CD
Draw DE $\perp$ AC and FB $\perp$ AC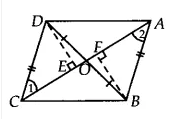
1595869408243
In $\Delta$ DEO and $\Delta$ BFO
$\angle$ DOE = $\angle$ BOF [vertically opposite angle]
$\angle$ OED = $\angle$ BFO [each $90^0$ ]
OB = OD [given]

Therefore, by AAS congruency
$\Delta$ DEO $\cong$ $\Delta$ BFO
$\Rightarrow$ DE = FB [by CPCT]

and ar( $\Delta$ DEO) = ar( $\Delta$ BFO) ............(i)

Now, In $\Delta$ DEC and $\Delta$ ABF
$\angle$ DEC = $\angle$ BFA [ each $90^0$ ]
DE = FB
DC = BA [given]
So, by RHS congruency
$\Delta$ DEC $\cong$ $\Delta$ BFA
$\angle$ 1 = $\angle$ 2 [by CPCT]
and, ar( $\Delta$ DEC) = ar( $\Delta$ BFA).....(ii)

By adding equation(i) and (ii), we get
$\small ar(DOC)=ar(AOB)$
Hence proved.

Q6 (ii) In Fig. $\small 9.25$ , diagonals AC and BD of quadrilateral ABCD intersect at O such that $\small OB=OD$ . If $\small AB=CD$ , then show that: $\small ar(DCB)=ar(ACB)$

[ Hint: From D and B, draw perpendiculars to AC.]

1640236384556

Answer:

1595869418016
We already proved that,
$ar(\Delta DOC)=ar(\Delta AOB)$
Now, add $ar(\Delta BOC)$ on both sides we get

$\\ar(\Delta DOC)+ar(\Delta BOC)=ar(\Delta AOB)+ar(\Delta BOC)\\ ar(\Delta DCB) = ar (\Delta ACB)$
Hence proved.

Q6 (iii) In Fig. $\small 9.25$ , diagonals AC and BD of quadrilateral ABCD intersect at O such that $\small OB=OD$ . If $\small AB=CD$ , then show that:

$\small DA\parallel CB$ or ABCD is a parallelogram.

[ Hint : From D and B, draw perpendiculars to AC.]

1640236400803

Answer:

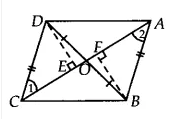
1595869431927
Since $\Delta$ DCB and $\Delta$ ACB both lie on the same base BC and having equal areas.
Therefore, They lie between the same parallels BC and AD
$\Rightarrow$ CB || AD
also $\angle$ 1 = $\angle$ 2 [ already proved]
So, AB || CD
Hence ABCD is a || gm

Q7 D and E are points on sides AB and AC respectively of $\small \Delta ABC$ such that $\small ar(DBC)=ar(EBC)$ . Prove that $\small DE\parallel BC$ .

Answer:

We have $\Delta$ ABC and points D and E are on the sides AB and AC such that ar( $\Delta$ DBC ) = ar ( $\Delta$ EBC)

1595869522642
Since $\Delta$ DBC and $\Delta$ EBC are on the same base BC and having the same area.
$\therefore$ They must lie between the same parallels DE and BC
Hence DE || BC.

Q8 XY is a line parallel to side BC of a triangle ABC. If $\small BE\parallel AC$ and $\small CF\parallel AB$ meet XY at E and F respectively, show that $\small ar(ABE)=ar(ACF)$

Answer:

We have a $\Delta$ ABC such that BE || AC and CF || AB
Since XY || BC and BE || CY
Therefore, BCYE is a ||gm
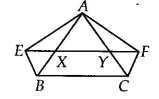1640236430685 Now, The ||gm BCEY and $\Delta$ ABE are on the same base BE and between the same parallels AC and BE.
$\therefore$ ar( $\Delta$ AEB) = 1/2 .ar(||gm BEYC)..........(i)
Similarly, ar( $\Delta$ ACF) = 1/2 . ar(||gm BCFX)..................(ii)

Also, ||gm BEYC and ||gmBCFX are on the same base BC and between the same parallels BC and EF.
$\therefore$ ar (BEYC) = ar (BCFX).........(iii)

From eq (i), (ii) and (iii), we get

ar( $\Delta$ ABE) = ar( $\Delta$ ACF)
Hence proved.

Q9 The side AB of a parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed (see Fig. $\small 9.26$ ). Show that $\small ar(ABCD)=ar(PBQR)$ . [ Hint: Join AC and PQ. Now compare $\small ar(ACQ)$ and $\small ar(APQ)$ .]

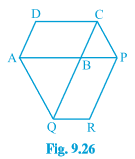1640236457256 Answer:

Join the AC and PQ.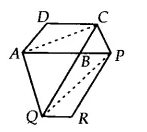
1595869753492
It is given that ABCD is a ||gm and AC is a diagonal of ||gm
Therefore, ar( $\Delta$ ABC) = ar( $\Delta$ ADC) = 1/2 ar(||gm ABCD).............(i)

Also, ar( $\Delta$ PQR) = ar( $\Delta$ BPQ) = 1/2 ar(||gm PBQR).............(ii)

Since $\Delta$ AQC and $\Delta$ APQ are on the same base AQ and between same parallels AQ and CP.
$\therefore$ ar( $\Delta$ AQC) = ar ( $\Delta$ APQ)

Now, subtracting $\Delta$ ABQ from both sides we get,

ar( $\Delta$ AQC) - ar ( $\Delta$ ABQ) = ar ( $\Delta$ APQ) - ar ( $\Delta$ ABQ)
ar( $\Delta$ ABC) = ar ( $\Delta$ BPQ)............(iii)

From eq(i), (ii) and (iii) we get

$\small ar(ABCD)=ar(PBQR)$

Hence proved.

Q10 Diagonals AC and BD of a trapezium ABCD with $\small AB\parallel DC$ intersect each other at O. Prove that $\small ar(AOD)=ar(BOC)$ .

Answer:

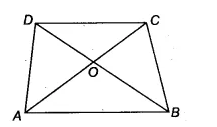
1595869859506

We have a trapezium ABCD such that AB || CD and it's diagonals AC and BD intersect each other at O
$\Delta$ ABD and $\Delta$ ABC are on the same base AB and between same parallels AB and CD
$\therefore$ ar( $\Delta$ ABD) = ar ( $\Delta$ ABC)

Now, subtracting $\Delta$ AOB from both sides we get

ar ( $\Delta$ AOD) = ar ( $\Delta$ BOC)

Hence proved.

Q11 In Fig. $\small 9.27$ , ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that

(i) $\small ar(ACB)=ar(ACF)$
(ii) $\small ar(AEDF)=ar(ABCDE)$

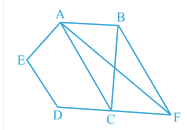
1595869929784

Answer:
We have a pentagon ABCDE in which BF || AC and CD is produced to F.

(i) Since $\Delta$ ACB and $\Delta$ ACF are on the same base AC and between same parallels AC and FB.
$\therefore$ ar( $\Delta$ ACB) = ar ( $\Delta$ ACF)..................(i)

(ii) Adding the ar (AEDC) on both sides in equation (i), we get

ar( $\Delta$ ACB) + ar(AEDC) = ar ( $\Delta$ ACF) + ar(AEDC)
$\therefore$ $\small ar(AEDF)=ar(ABCDE)$

Hence proved.

Q12 A villager Itwaari has a plot of land of the shape of a quadrilateral. The Gram Panchayat of the village decided to take over some portion of his plot from one of the corners to construct a Health Centre. Itwaari agrees to the above proposal with the condition that he should be given an equal amount of land in lieu of his land adjoining his plot so as to form a triangular plot. Explain how this proposal will be implemented.

Answer:

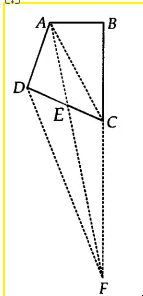
1595870011551
We have a quadrilateral shaped plot ABCD. Draw DF || AC and AF || CF.
Now, $\Delta$ DAF and $\Delta$ DCF are on the same base DF and between same parallels AC and DF.
$\therefore$ ar ( $\Delta$ DAF) = ar( $\Delta$ DCF)
On subtracting $\Delta$ DEF from both sides, we get

ar( $\Delta$ ADE) = ar( $\Delta$ CEF)...............(i)
The portion of $\Delta$ ADE can be taken by the gram panchayat and on adding the land $\Delta$ CEF to his (Itwaari) land so as to form a triangular plot.( $\Delta$ ABF)

We need to prove that ar( $\Delta$ ABF) = ar (quad. ABCD)

Now, adding ar(quad. ABCE) on both sides in eq (i), we get

ar ( $\Delta$ ADE) + ar(quad. ABCE) = ar( $\Delta$ CEF) + ar(quad. ABCE)
ar (ABCD) = ar( $\Delta$ ABF)

Q13 ABCD is a trapezium with $\small AB\parallel DC$ . A line parallel to AC intersects AB at X and BC at Y. Prove that $\small ar(ADX)=ar(ACY)$ . [ Hint: Join CX.]

Answer:

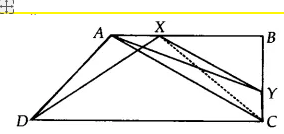
1595870079586
We have a trapezium ABCD, AB || CD
XY ||AC meets AB at X and BC at Y. Join XC

Since $\Delta$ ADX and $\Delta$ ACX lie on the same base CD and between same parallels AX and CD
Therefore, ar( $\Delta$ ADX) = ar( $\Delta$ ACX)..........(i)
Similarly ar( $\Delta$ ACX) = ar( $\Delta$ ACY).............(ii) [common base AC and AC || XY]
From eq (i) and eq (ii), we get

ar( $\Delta$ ADX) = ar ( $\Delta$ ACY)

Hence proved.

Q14 In Fig. $\small 9.28$ , $\small AP\parallel BQ\parallel CR$ . Prove that $\small ar(AQC)=ar(PBR)$ .

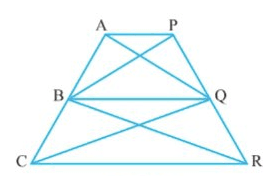
1595870192732
Answer:

We have, AP || BQ || CR
$\Delta$ BCQ and $\Delta$ BQR lie on the same base (BQ) and between same parallels (BQ and CR)
Therefore, ar ( $\Delta$ BCQ) = ar ( $\Delta$ BQR)........(i)

Similarly, ar ( $\Delta$ ABQ) = ar ( $\Delta$ PBQ) [common base BQ and BQ || AP]............(ii)

Add the eq(i) and (ii), we get

ar ( $\Delta$ AQC) = ar ( $\Delta$ PBR)

Hence proved.

Q15 Diagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that $\small ar(AOD)=ar(BOC)$ . Prove that ABCD is a trapezium.

Answer:

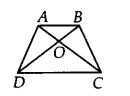
1595870246292
We have,
ABCD is a quadrilateral and diagonals AC and BD intersect at O such that ar( $\Delta$ AOD) = ar ( $\Delta$ BOC) ...........(i)

Now, add ar ( $\Delta$ BOA) on both sides, we get

ar( $\Delta$ AOD) + ar ( $\Delta$ BOA) = ar ( $\Delta$ BOA) + ar ( $\Delta$ BOC)
ar ( $\Delta$ ABD) = ar ( $\Delta$ ABC)
Since the $\Delta$ ABC and $\Delta$ ABD lie on the same base AB and have an equal area.
Therefore, AB || CD

Hence ABCD is a trapezium.

Q16 In Fig. $\small 9.29$ , $\small ar(DRC)=ar(DPC)$ and $\small ar(BDP)=ar(ARC)$ . Show that both the quadrilaterals ABCD and DCPR are trapeziums.

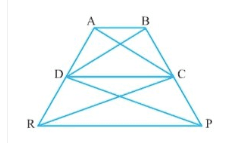
1595870351963
Answer:

Given,
ar( $\Delta$ DPC) = ar( $\Delta$ DRC) ..........(i)
and ar( $\Delta$ BDP) = ar(ARC)............(ii)

from equation (i),
Since $\Delta$ DRC and $\Delta$ DPC lie on the same base DC and between same parallels.
$\therefore$ CD || RP (opposites sides are parallel)

Hence quadrilateral DCPR is a trapezium

Now, by subtracting eq(ii) - eq(i) we get

ar( $\Delta$ BDP) - ar( $\Delta$ DPC) = ar( $\Delta$ ARC) - ar( $\Delta$ DRC)
ar( $\Delta$ BDC) = ar( $\Delta$ ADC) (Since theya are on the same base DC)
$\Rightarrow$ AB || DC

Hence ABCD is a trapezium.

Benefits of NCERT Solutions for Class 9 Maths Exercise 9.3

Exercise 9.3 Class 9 Maths, is based on the AREAS OF PARALLELOGRAMS AND TRIANGLES by providing us with a general (much simpler) approach to calculate the area of triangles and parallelograms and concepts of figures in the same base and same parallel lines.
NCERT syllabus Class 9 Maths chapter 9 exercise 9.3 introduces us to a new concept of triangles on the same base and between the same parallels and its properties, like calculating the area and height.
Understanding the concepts from Class 9 Maths chapter 9 exercise 9.3 will help us to compare the areas in multiple-choice questions easily and thus save time for a particular question.

Also, See