NCERT Solutions for Class 11 Physics Chapter 7 Gravitation
Ever wondered how apples drop onto the ground and the Moon does not collide with the Earth? Or how the planets remain in their orbit. The reason is —Gravitation! In our daily lives, we often observe the effects of gravity—every object is pulled toward the Earth, and anything thrown upward eventually falls back down due to this force. Similarly, walking uphill requires more effort than walking downhill, highlighting the influence of gravity.
This Story also Contains
- NCERT Solution for Class 11 Physics Chapter 7 Gravitation: Download PDF
- NCERT Solutions for Class 11 Physics Chapter 7: Exercise Questions
- NCERT Solutions for Class 11 Physics Chapter 7 Gravitation: Additional Questions
- Class 11 Physics NCERT Chapter 7: Higher Order Thinking Skills (HOTS) Questions
- NCERT Chapter 7 Gravitation Topics
- NCERT Solutions for Class 11 Chapter 7: Important Formulae
- Approach to Solve Questions of Gravitation Class 11
- What Extra Should Students Study Beyond the NCERT for JEE/NEET?
- NCERT Solutions for Class 11 Physics Chapter-Wise

Gravitation is an important topic in the Class 11 NCERT syllabus, as it explains the fundamental force responsible for the attraction between objects and the Earth. If you find it challenging to understand the concepts or solve the questions in the NCERT textbook, Careers360 offers comprehensive and well-explained NCERT solutions to support your learning and make the topic easier to grasp. The NCERT solutions for Class 11 Chapter 7 – Gravitation cover all major concepts, including Kepler’s Laws, which are especially important for competitive exams like NEET and JEE Main.
NCERT Solution for Class 11 Physics Chapter 7 Gravitation: Download PDF
Gravitation, the key force that provides the structure of the universe, the motion of the planets and the tides, is discussed in the Chapter 7 of Class 11 of the subject Physics. The NCERT solutions also elaborate major concepts such as the Newton Law of Gravitation, the acceleration because of the gravity and motion of the satellite. The PDF can also be downloaded to give step-by-step solutions to improve their knowledge and examination results.
NCERT Solutions for Class 11 Physics Chapter 7: Exercise Questions
Chapter 7 of Class 11 Physics has Exercise Questions that will assess a student on conceptual understanding and problem-solving. NCERT solutions make all exercises of the textbook clear and step-by-step, allowing students to learn about such concepts as gravitational force, satellite motion, and escape velocity in a convenient way. These are useful both in preparing for board exams and competitive exams such as JEE.
Q: 7.1 (a) Answer the following questions:
Answer:
No, because gravitational force doesn't depend upon the material medium. It is independent of the presence of other materials.
Q: 7.1 (b) Answer the following :
Answer:
Yes if the size of the space station is large then he can detect gravity.
Q: 7.1 (c) Answer the following:
Answer:
Apart from the gravitational pull, the tidal effect also depends upon the cube of the distance between the two. Since the distance between earth and sun is much larger than the distance between the sun and moon so it not also balances but is more than the effect of gravitational force. Thus the tidal effect of the moon’s pull is greater than the tidal effect of the sun.
Q: 7.2 Choose the correct alternative :
(a) Acceleration due to gravity increases/decreases with increasing altitude.
(c) Acceleration due to gravity is independent of mass of the earth/mass of the body.
Answer:
(a) Acceleration due to gravity decreases with increasing altitude.
The relation between the two is given by :
$g_h\ =\ \left ( 1\ -\ \frac{2h}{R_e} \right )g$
(b) Acceleration due to gravity decreases with increasing depth.
The relation is given below:
$g_d\ =\ \left ( 1\ -\ \frac{d}{R_e} \right )g$
(c) Acceleration due to gravity is independent of the mass of the body.
$g\ =\ \frac{GM}{R^2}$ Here M is the mass of the earth.
(d) The formula $-G M m\left(1 / r_2-1 / r_1\right)$ is more accurate than the formula $m g\left(r_2-r_1\right)$ for the difference of potential energy between two points $r_2$ and $r_1$ distance away from the centre of the earth.
Answer:
Time taken by planet to complete a revolution around sun = $\frac{1}{2}T_e$
Using Kepler's law of planetary motion we can write :
$\left ( \frac{R_p}{R_e} \right )^3\ =\ \left ( \frac{T_p}{T_e} \right )^2$
or $\frac{R_p}{R_e}\ =\ \left ( \frac{\frac{1}{2}}{1} \right )^\frac{2}{3}$
or $\frac{R_p}{R_e}\ =\ 0.63$
Thus the planet is 0.63 times smaller than earth.
Answer:
The orbital period in days is $=\ 1.769\times 24 \times 60\times 60\ s$
Mass is given by :
$M\ =\ \frac{4 \pi ^2 R^3}{GT^2}$
Thus the ratio of the mass of Jupiter and mass of the sun is :
$\frac{M_s}{M_j} =\ \frac{\frac{4 \pi ^2 R_e^3}{GT_e^2}}{\frac{4 \pi ^2 R_{io}^3}{GT_{io}^2}}$
or $\frac{M_s}{M_j}=\ \left ( \frac{1.769 \times 24\times 60\times 60}{365.25\times 24\times 60\times 60} \right )^2\times \left ( \frac{1.496\times 10^{11}}{4.22\times 10^8} \right )^3$
or $\frac{M_s}{M_j}\approx 1045$
Thus the mass of Jupiter is nearly one-thousandth that of the sun.
Answer:
We know that one light year is $9.45\times 10^{15}\ m$ .
The time period of rotation is given by :
$T\ =\ \left ( \frac{4 \pi r^3}{GM} \right )^\frac{1}{2}$
Putting all the value (in SI units) in the above equation we get :
$T=\ \left ( \frac{4 \times \left ( 3.14 \right )^2\times (4.73)^3\times 10^{60}}{6.67\times 10^{-11} \times 5\times 10^{41}} \right )^\frac{1}{2}$
or $T=\ 1.12\ \times 10^{16}\ s$
In years :
$T=\ \frac{1.12\ \times 10^{16}}{365 \times 24 \times 60 \times 60}\ =\ 3.55\times 10^8\ years$
Q: 7.6 Choose the correct alternative:
Answer:
(a) The total energy will be negative of its kinetic energy . Since at infinity potential energy is zero and total energy is negative.
(b) The energy required will be less as the stationary object on earth has no energy initially whereas the satellite has gained energy due to rotational motion.
Answer:
The escape velocity from the earth is given by :
$v_{esc}\ =\ \sqrt{2gR}$
Since the escape velocity depends upon the reference (potential energy), so it only depends upon the height of location.
(a) No
(b) No
(c) No
(d) Yes
Answer:
Among all only angular momentum and total energy of the comet will be constant other all factors given will vary from point to point.
Answer:
(a) In space we have state of weightlessness, so the swollen feet does not affect astronaut as there is no gravitational pull (so cannot stand).
(b) The swollen face will be affected as the sense organs such as eyes, ears etc. will be affected.
(c) It will affect the astronaut as it may cause mental strain.
(d) It will affect the astronaut as space has different orientations.
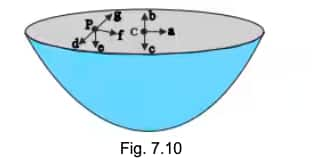
Answer:
We know that the gravitational potential (V) in a sphere is constant throughout. Also, the gravitational intensity will act in the downward direction as its upper half is cut (gravitational force will also act in a downward direction). Hence the required direction of gravitational intensity is shown by arrow c.
.png)
Answer:
As stated in the previous question, the gravitational potential in a spherical shell is constant throughout. So the gravitational intensity will act in downward direction (as upper half is cut). So the required direction is shown by arrow e .
Answer:
Let the distance where the gravitational force acting on satellite P becomes zero be x from the earth.
Thus we can write :
$\frac{GmM_s}{(r-x)^2}\ =\ \frac{GmM_e}{r^2}$
or $\left ( \frac{r-x}{x} \right )\ =\ \left ( \frac{2\times 10^{30}}{60\times 10^{24}} \right )^\frac{1}{2}$
or $\left ( \frac{r-x}{x} \right )=\ 577.35$
Hence :
$x\ =\ \frac{1.5\times 10^{11}}{578.35}\ =\ 2.59\times 10^8\ m$ (Since r = $1.5\times 10^{11}$ )
Answer:
Mass of sun can be calculated by using the following formula:-
$M\ =\ \frac{4 \times \pi^2 \times r^3 }{GT^2}$
Putting the known values in the above formula, we obtain :
$M=\ \frac{4 \times (3.14)^2 \times (1.5\times 10^{11})^3 }{6.67\times 10^{-11}\times (365.25\times 24\times 60 \times 60)^2}$
or $M=\ \frac{133.24\times 10}{6.64\times 10^4}\ =\ 2\times 10^{30}\ Kg$
Thus the mass of the sun is nearly $2\times 10^{30}\ Kg$ .
Answer:
Kepler's third law give us the following relation :
$T\ =\ \left ( \frac{ \pi^2 r^3}{GM} \right )^ \frac{1}{2}$
Thus we can write :
$\frac{r_s^3}{r_e^3}\ =\ \frac{T_s^2}{T_e^2}$
or $r_s\ =\ r_e\left ( \frac{T_s}{T_e} \right )^ \frac{2}{3}$
or $r_s=\ 1.5 \times 10^{11}\times \left ( \frac{29.5 T_e}{T_e} \right )^ \frac{2}{3}$
or $r_s=\ 14.32 \times 10^{11}\ m$
Thus the distance between the sun and Saturn is $14.32 \times 10^{11}\ m$ .
Answer:
Acceleration due to gravity at height h from the surface of eath is :
$g'\ =\ \frac {g}{\left ( 1\ +\ \frac{h}{R} \right )^2}$
For $h\ =\ \frac{R}{2}$ we have :
$g'\ =\ \frac {g}{\left ( 1\ +\ \frac{\frac{R}{2}}{R} \right )^2}$
or $g'=\ \frac{4}{9}g$
Thus the weight of the body will be :
$W\ =\ mg'$
or $W=\ m\times \frac{4}{9}g\ =\ \frac{4}{9}mg$
or $W=\ 28\ N$
Answer:
Position of the body is (depth) :
$=\ \frac{1}{2}R_e$
Acceleration due to gravity at depth d is given by :
$g'\ =\ \left ( 1\ -\ \frac{d}{R_e} \right )g$
or $g'=\ \left ( 1\ -\ \frac{\frac{R_e}{2}}{R_e} \right )g$
or $g'=\ \frac{1}{2}g$
Thus the weight of the body is:-
$W\ =\ mg'$
or $W=\ m\times \frac{1}{2}g\ =\ \frac{mg}{2}$
or $W=\ 125\ N$
Thus the weight of the body is 125 N.
Answer:
The total energy is given by :
Total energy = Potential energy + Kinetic energy
$=\ \left ( \frac{ -GmM_e}{R_e} \right )\ +\ \frac{1}{2}mv^2$
At the highest point velocity will be zero.
Thus the total energy of the rocket is :
$=\ \left ( \frac{ -GmM_e}{R_e\ +\ h} \right )\ +\ 0$
Now we will use the conservation of energy :
Total energy initially (at earth's surface) = Total energy at height h
$\left ( \frac{ -GmM_e}{R_e} \right )\ +\ \frac{1}{2}mv^2\ =\ \left ( \frac{ -GmM_e}{R_e\ +\ h} \right )$
or $\frac{1}{2}v^2\ =\ \frac{ gR_eh}{R_e\ +\ h}$
or $h\ =\ \frac{R_e v^2}{2gR_e\ -\ v^2}$
or $h=\ \frac{6.4\times 10^{6}\times (5\times 10^3)^2}{2g\times 6.4\times 10^6\ -\ (5\times 10^3)^2}$
or $h=\ 1.6\times 10^6\ m$
Hence the height achieved by the rocket from earth's centre = R + h
$R+h=\ 6.4\times 10^6\ +\ 1.6 \times 10^6$
or $R+h=\ 8\times 10^6\ m$
Answer:
Let us assume the speed of the body far away from the earth is $v_f$ .
Total energy on earth is :
$=\ \frac{1}{2}mv_p^2\ -\ \frac{1}{2}mv_{esc}^2$
And the total energy when the body is far from the earth is :
$=\ \frac{1}{2}mv_f^2$
(Since the potential energy at far from the earth is zero.)
We will use conservation of energy : -
$\frac{1}{2}mv_p^2\ -\ \frac{1}{2}mv_{esc}^2\ =\ \frac{1}{2}mv_f^2$
or $v_f\ =\ \sqrt{\left ( v_p^2\ -\ v_{esc}^2 \right )}$
or $v_f=\ \sqrt{\left ( \left ( 3v_{esc} \right )^2\ -\ v_{esc}^2 \right )}$
or $v_f=\ \sqrt{8}v_{esc}$
or $v_f=\ 31.68\ km/s$
Answer:
The total energy of the satellite at height h is given by :
$=\ \frac{1}{2}mv^2\ +\ \left ( \frac{-GM_em}{R_e\ +\ h} \right )$
We know that the orbital speed of the satellite is :
$v\ =\ \sqrt{\left ( \frac{GM_e}{R_e\ +\ h} \right )}$
Thus the total energy becomes :
$E=\ \frac{1}{2}m\times \left ( \frac{GM_e}{R_e\ +\ h} \right )\ +\ \left ( \frac{-GM_em}{R_e\ +\ h} \right )$
or $E=\ - \frac{1}{2} \left ( \frac{GM_em}{R_e\ +\ h} \right )$
Thus the required energy is negative of the total energy :
nbsp; $E_{req}\ =\ \frac{1}{2} \left ( \frac{GM_em}{R_e\ +\ h} \right )$
or $E_{req}=\ \frac{1}{2} \left ( \frac{6.67\times 10^{-11}\times 6 \times 10^{24}\times 200}{6.4\times 10^6\ +\ 0.4\times 10^6} \right )$
or $E_{req}=\ 5.9\times 10^9\ J$
Answer:
The total energy of stars is given by :
$E\ =\ \frac{-GMM}{r}\ +\ \frac{1}{2}Mv^2$
or $E=\ \frac{-GMM}{r}\ +\ 0$
or $E=\ \frac{-GMM}{r}$
Now when starts are just to collide the distance between them is 2R.
The total kinetic energy of both the stars is :
$KE=\ \frac{1}{2}Mv^2\ +\ \frac{1}{2}Mv^2\ =\ Mv^2$
And the total energy of both the stars is :
$E'=\ Mv^2\ +\ \frac{-GMM}{2r}$
Using conservation of energy we get :
$Mv^2\ +\ \frac{-GMM}{2r}\ =\ \frac{-GMM}{r}$
or $v^2\ =\ GM \left ( \frac{-1}{r}\ +\ \frac{1}{2R} \right )$
or $v^2=\ 6.67\times 10^{-11}\times 2\times 10^{30} \left ( \frac{-1}{10^{12}}\ +\ \frac{1}{2\times 10^7} \right )$
or $v^2=\ 6.67\times 10^{12}$
Thus the velocity is : $\sqrt{6.67\times 10^{12}}\ =\ 2.58\times 10^6\ m/s$
Answer:
The gravitational force at the midpoint will be zero. This is because both spheres are identical and their forces will be equal but opposite in direction.
The gravitational potential is given by :
$=\ \frac {-GM}{\frac{r}{2}}\ -\ \frac{-GM}{\frac{r}{2}}\ =\ \frac{-4GM}{r}$
$=\ \frac{-4\times 6.67\times 10^{-11}\times 100}{1}$
$=\ -2.67\times 10^{-8}\ J/Kg$
At the midpoint, we have equal forces in the opposite direction so it is in equilibrium but if we move the body slightly then the particle will move in one direction (as one force will be greater). So it is an unstable equilibrium.
NCERT Solutions for Class 11 Physics Chapter 7 Gravitation: Additional Questions
The Additional Questions of Chapter 7 Gravitation in Class 11 Physics have been framed in such a way that it's supposed to cover more than what NCERT textbook covers and enhance the knowledge of major principles. These questions revolve around learning application-based and numerical problem-solving and critical thinking. They are very practical when it comes to practicing by the students who want to get a better score in their school exams and other competitive tests such as JEE.
Answer:
Height of satellite from earth's surface : $3.6\times 10^7\ m$
Gravitational potential is given by :
$=\ \frac{-GM}{R\ +\ h}$
$=\ \frac{-6.67\times 10^{-11}\times 6\times 10^{24}}{3.6\times 10^7\ +\ 0.64\times 10^7 }$
$=\ -\ 9.4\times 10^6\ J/Kg$
Thus potential due to earth gravity is $-\ 9.4\times 10^6\ J/Kg$ .
Answer:
A body will get stuck at the star's surface if the centrifugal force of star is less than the gravitational force.
The gravitational force is given by :
$F_g\ =\ \frac{GMm}{r^2}$
or $F_g=\ \frac{6.67\times 10^{-11}\times 5\times 10^{30}\times m}{(1.2\times 10^4)^2}\ =\ 2.31\times 10^{12}\ m\ N$
The centrifugal force is given by :
$F_c\ =\ mr\omega ^2$
or $F_c=\ mr(2\pi v) ^2$ $=\ m\times 1.2\times 10^4\times (2\pi \times 1.2) ^2$
or $F_c=\ 6.8\times 10^5\ m\ N$
As we can see that the gravitational force is greater than the centrifugal force thus the body will remain at the star.
Answer:
Firstly, the potential energy of spaceship due to the sun is given by :
$=\ \frac{-GMm_s}{r}$
and the potential energy of spaceship due to mars is given by :
$=\ \frac{-GMm_m}{R}$
It is given that the spaceship is stationary so its kinetic energy is zero.
Thus the total energy of spaceship is :
$=\ \frac{-GMm_s}{r}\ +\ \frac{-GMm_m}{R}$
Thus the energy needed to launch the spaceship is :
$=\ \frac{GMm_s}{r}\ +\ \frac{GMm_m}{R}$
$=\ 6.67\times 10^{-11}\times 10^3 \left ( \frac{2\times 10^{30}}{2.28\times 10^{11}}\ +\ \frac{6.4\times 10^{23}}{3.395\times 10^6} \right )$
$=\ 596.97\times 10^9\ J$
$=\ 6\times 10^{11}\ J$
Answer:
The kinetic energy of the rocket is (initial):-
$=\ \frac{1}{2}mv^2$
And the initial potential energy is :
$=\ \frac{-GMm}{R}$
Thus total initial energy is given by :
$=\ \frac{1}{2}mv^2\ +\ \frac{-GMm}{R}$
Further, it is given that 20 per cent of kinetic energy is lost.
So the net initial energy is :
$=\ 0.4mv^2\ -\ \frac{GMm}{R}$
The final energy is given by :
$=\ \frac{GMm}{R\ +\ h}$
Using the law of energy conservation we get :
$0.4mv^2\ -\ \frac{GMm}{R}\ =\ \frac{GMm}{R\ +\ h}$
Solving the above equation, we get :
$h\ =\ \frac{R}{\frac{GM}{0.4v^2R}\ -\ 1}$
or $h=\ \frac{0.4R^2v^2}{GM\ -\ 0.4v^2R}$
or $h=\ \frac{18.442\times 10^{18}}{42.688\times 10^{12}\ -\ 5.432\times 10^{12}}$
or $h=\ 495\times 10^3\ m$
or $h=\ 495\ Km$
Thus, the required distance is 495 Km.
Class 11 Physics NCERT Chapter 7: Higher Order Thinking Skills (HOTS) Questions
Chapter 7- Gravitation Higher Order Thinking Skills (HOTS) questions in Class 11 Physics challenges the students to use concepts in unfamiliar and complex situations. These questions are not mere direct formulas and challenge more in-depth reasoning, understanding and analytical capability. They are particularly good in enhancing competitive exam skills such as JEE.
Question 1: Earth has a mass of 8 inches and a radius of 2 times that of a planet. If the escape velocity from the earth is $11.2 \mathrm{~km} / \mathrm{s}$, the escape velocity in $\mathrm{km} / \mathrm{s}$ from the planet will be:
1) 11.2
2) 5.6
3) 2.8
4) 8.4
Solution:
$
\begin{aligned}
& \mathrm{v}_{\text {escape }}=\sqrt{\frac{2 \mathrm{GM}}{\mathrm{R}}} \\
& \frac{\left(\mathrm{~v}_{\text {escape }}\right)_{\text {Planet }}}{\left(\mathrm{v}_{\text {escape }}\right)_{\text {Earth }}}=\sqrt{\left(\frac{\mathrm{M}_{\mathrm{P}}}{\mathrm{M}_{\mathrm{E}}}\right) \times\left(\frac{\mathrm{R}_{\mathrm{E}}}{\mathrm{R}_{\mathrm{P}}}\right)}=\frac{1}{2} \\
& \left(\mathrm{~v}_{\text {escape }}\right)_{\text {Planet }}=\frac{1}{2}\left(\mathrm{~v}_{\text {escape }}\right)_{\text {Earth }}=5.6 \mathrm{~km} / \mathrm{s}
\end{aligned}
$
Hence, the answer is the option (2).
Question 2: A satellite of mass $\frac{M}{2}$ is revolving around Earth in a circular orbit at a height of $\frac{R}{3}$ from the Earth's surface. The angular momentum of the satellite is $M \sqrt{\frac{\text { GMR }}{x}}$. The value of $x$ is _____ where $M$ and $R$ are the mass and dive of earth, respectively. ( $G$ is the gravitational constant.)
Solution:
If earth is assumed do be stationary
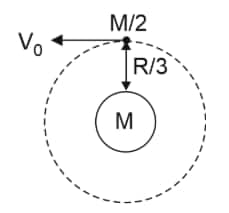
orbital velocity $\mathrm{v}_0=\sqrt{\frac{\mathrm{GM}}{4 \mathrm{R} / 3}}=\sqrt{\frac{3 \mathrm{GM}}{4 \mathrm{R}}}$
Angular momentum of satellite $=\frac{M}{2} v_0 \frac{4 R}{3}$
$\begin{aligned} & =\frac{M}{2} \cdot \sqrt{\frac{3 \mathrm{GM}}{4 \mathrm{R}}} \cdot \frac{4 \mathrm{R}}{3} \\ & =M \sqrt{\frac{\mathrm{GMR}}{3}} \\ & x=3\end{aligned}$
Question 3: A satellite is launched into a circular orbit of radius ' $R$ ' around the earth. A second satellite is launched into an orbit of radius $1.03 R$. The time period of revolution of the second satellite is larger than the first one approximately by:-
1) $3 \%$
2) $ 4.5 \% $
3) $ 9 \% $
4) $ 2.5 \% $
Solution:
$
\begin{aligned}
&\begin{aligned}
& \mathrm{T}^2=\mathrm{KR}^3 \\
& \frac{2 \Delta \mathrm{~T}}{\mathrm{~T}}=\frac{3 \Delta \mathrm{R}}{\mathrm{R}} \\
& \frac{2 \Delta \mathrm{~T}}{\mathrm{~T}}=\frac{3 \times 0.03 \mathrm{R}}{\mathrm{R}} \\
& \frac{\Delta \mathrm{~T}}{\mathrm{~T}}=\frac{3 \times 0.03}{2} \times 100=4.5 \%
\end{aligned}\\
&\text { Hence, the answer is the option (2). }
\end{aligned}
$
Question 4: If a satellite orbiting the Earth is 9 times closer to the Earth than the Moon, what is the time period of rotation of the satellite? Given rotational time period of Moon $=27$ days and gravitational attraction between the satellite and the moon is neglected.
1) 1 day
2) 81 days
3) 27 days
4) 3 days
Solution:
$
\begin{aligned}
&\begin{aligned}
& \mathrm{T}^2 \propto \mathrm{R}^3 \\
& \left(\frac{\mathrm{~T}_{\mathrm{m}}}{\mathrm{~T}_{\mathrm{s}}}\right)^2=\left(\frac{\mathrm{R}}{\mathrm{R} / 9}\right)^3 \\
& \frac{\mathrm{~T}_{\mathrm{m}}}{\mathrm{~T}_{\mathrm{s}}}=(3)^3 \\
& \Rightarrow \mathrm{~T}_{\mathrm{s}}=\left(\frac{27}{27}\right)=1 \text { day }
\end{aligned}\\
&\text { Hence, the answer is the option (1). }
\end{aligned}
$
Question 5: An object of mass ' $m$ ' is projected from origin in a vertical xy plane at an angle $45^{\circ}$ with the $x$-axis with an initial velocity $v_0$. The magnitude and direction of the angular momentum of the object with respect to origin, when it reaches at the maximum height, will be [ g is acceleration due to gravity
1) $\frac{\mathrm{mv}_0^3}{2 \sqrt{2} \mathrm{~g}}$ along negative z -axis
2) $\frac{m v_0^3}{2 \sqrt{2} g}$ along negative $z$-axis
3) $\frac{m v_0^3}{2 \sqrt{2} g}$ along positive $z$-axis
4) $\frac{m v_0^3}{4 \sqrt{2} g}$ along negative $z$-axis
Solution:
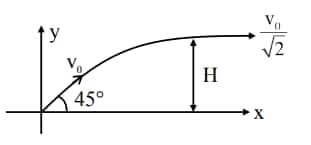
$\mathrm{H}=\frac{\left(\frac{\mathrm{v}_0}{\sqrt{2}}\right)^2}{2 \mathrm{~g}}=\frac{\mathrm{v}_0^2}{4 \mathrm{~g}} \\$
$ \mathrm{~L}=\mathrm{mvh} \\$
$ \mathrm{~L}=\mathrm{m} \frac{\mathrm{v}_0}{\sqrt{2}} \frac{\mathrm{v}_0^2}{4 \mathrm{~g}}$
Hence, the answer is the option (4).
NCERT Chapter 7 Gravitation Topics
Chapter 7 of Class 11 Physics, Gravitation covers the first basic force which is known to hold the motion of a planet as well as celestial mechanics. It makes children realize why objects tend to attract one another by virtue of their mass and the universal force it influences both earthly and space events. This chapter provides the foundation of more in-depth physics and astronomy.
7.1 Introduction
7.2 Kepler’S Laws
7.3 Universal Law Of Gravitation
7.4 The Gravitational Constant
7.5 Acceleration Due To Gravity Of The Earth
7.6 Acceleration Due To Gravity Below And Above The Surface Of Earth
7.7 Gravitational Potential Energy
7.8 Escape Speed
7.9 Earth Satellites
7.10 Energy Of An Orbiting Satellite
NCERT Solutions for Class 11 Chapter 7: Important Formulae
It is important to know and use the significant formulae in this chapter in order to work out numerical problems and have an insight about the celestial body motion. The formulas are important both in class 11 and in competitive exams such as JEE and NEET.
1. Gravitational Force
$ F=\frac{G m_1 m_2}{r^2} $
2. Acceleration Due to Gravity (g)
$ g=\frac{G M}{R^2} $
3. Gravitational Potential
$ V=-\int \vec{I} \cdot \overrightarrow{d r} \text { or } V=-\frac{W}{m}=-\int \frac{\vec{F} \cdot \overrightarrow{d r}}{m} $
4. Work Done Against Gravity
$ W=\Delta U=G M m\left[\frac{1}{r_1}-\frac{1}{r_2}\right] $
5. Escape Velocity
$ V_c=\sqrt{\frac{2 G M}{R}} $
6. Kepler's Laws
$ \begin{aligned} & \frac{d A}{d t}=\frac{L}{2 m} \\ & T^2 \alpha a^3 \end{aligned} $
7. Orbital speed
$
v=\sqrt{\frac{G M}{r}}
$
8. Time period of revolution:
$
T=2 \pi \sqrt{\frac{r^3}{G M}}
$
9. Energy of Satellite-
$ E=-\frac{G M m}{2 r} $
Approach to Solve Questions of Gravitation Class 11
How to Apply Conservation Laws
- When dealing with complex problems, it becomes useful to apply principles of energy conservation and momentum conservation:
- Energy remains preserved if all processes lack non-conservative forces.
- The method aids in solving problems linked to orbital alterations, along with escape motions and falling situations.
Effective Problem-Solving Tips
- Use clear diagrams to represent forces along with their distances.
- Competitive exams require using SI units, and therefore, you must verify unit consistency.
Identify Whether the Problem Involves:
- Point masses
- Spherically symmetric objects
- Satellite or orbital dynamics
- When dealing with several forces or fields, it is necessary to perform vector addition.
What Extra Should Students Study Beyond the NCERT for JEE/NEET?
Competitive examinations such as JEE or NEET require students to move past the NCERT textbook in Chapter 7 Gravitation and brush up their conceptual understanding and solving speed. This involves studying of higher grade types of numerical problems, the nature of real life applications, more detailed theory behind gravitational potential energy, the motion of satellites and escape velocity.
NCERT Solutions for Class 11 Physics Chapter-Wise
NCERT Solutions of Class 11 Physics are systematically arranged to equip the learner with a thorough understanding of concepts and improve problem-solving skills. These solutions are in line with the new CBSE syllabus and solutions are both theoretical and numerical and are done in steps. By using chapter wise links students will find it easier to study one topic at a time and revise methodically.
NCERT Solutions for Class 11 Subject-wise
Also, Check NCERT Books and NCERT Syllabus here
- NCERT Books Class 11 Physics
- NCERT Syllabus Class 11 Physics
- NCERT Books Class 11
- NCERT Syllabus Class 11
Subject-Wise NCERT Exemplar Solutions
Frequently Asked Questions (FAQs)
Yes, it contains higher order thinking skills (HOTS) based questions and answers of challenging practice.
The concept of gravitation is the basis of studying planetary motion, orbits, satellites and gravitation is also important in competitive exams since there is a conceptual and numerical weight to
Yes, the solutions include not only conceptual questions but also numerical ones, step-by-step explanations are also included.
Yes, revised NCERT solutions are according to 2025-26 CBSE curriculum and modification done by NCERT.
Yes, when there is no air resistance all objects will fall at the same rate irrespective of mass since they are naturally pulled by gravity that has uniform acceleration.
Popular Questions
Courses After 12th
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters
