NCERT Solutions for Class 11 Physics Chapter 2 Motion In a Straight Line
When you imagine a car traveling along a straight road, you are actually looking at one of the most basic and most critical concepts in Physics, the straight line motion. This is the most basic chapter that is presented to us in class 11 Physics Chapter 2, and with the assistance of the NCERT Solutions of Motion in a Straight Line, learning will become an ordered and simple process. These solutions address all the main concepts, such as displacement, distance, speed, velocity, acceleration, equations of motion, and the motion graph, and they dictate everything in steps, thus making it easier to understand.
This Story also Contains
- Motion in a straight line class 11 Question Answers: Download PDF
- Motion in a Straight Line NCERT Solutions: Exercise Questions
- NCERT Solutions Class 11 Physics Chapter 2: Additional Questions
- Class 11 Physics Chapter 2 - Motion in a straight line: Higher Order Thinking Skills (HOTS) Questions
- Class 11 Physics Chapter 2 - Motion in a Straight Line Question Answers: Topics
- What Extra Should Students Study Beyond NCERT for JEE?
- NCERT Solutions for Class 11 Physics Chapter-Wise

The NCERT Solutions for Class 11 Physics Chapter 2 - Motion in a straight line are based on the recent CBSE syllabus and provide elaborate answers to questions in the textbook, problems in exercises, and additional numerical work. Higher Order Thinking Skills (HOTS) questions, additional solved problems, and conceptual descriptions are also there in these NCERT solutions, which enhance our problem-solving ability. These are extremely convenient in board exams and class tests, homework, and assignments, and form the foundation of competitive exams such as JEE, NEET, and Physics Olympiads. Also, understanding this chapter will be a foundation for more complicated chapters, such as plane motion and laws of motion by Newton, so this chapter is a pillar in further Physics studies. These NCERT Solutions for Class 11 Physics Chapter 2 - Motion in a straight line will help us to clear our concepts, score more marks, as well as develop good analytical skills that can be used in our future.
Motion in a straight line class 11 Question Answers: Download PDF
Class 11 Physics Chapter 2 - Motion in a straight line question answers provide clear and step-by-step answers to all textbook questions, helping students understand concepts like displacement, speed, velocity, and motion graphs. These solutions are available in a free downloadable PDF format, making it easy for students to revise anytime and prepare effectively for exams like CBSE, JEE, and NEET.
Download Solutions PDF
Motion in a Straight Line NCERT Solutions: Exercise Questions
Exercise Solutions of Class 11 Physics Chapter 2 - Motion in a straight line provide a step-by-step explanation to each of the back exercise questions, and can help one understand straight line motion more easily. The solutions sharpen the problem-solving technique and are also very practical in exam practice, along with competitive exams such as the JEE and NEET.
Answer:
Since the length of the railway carriage is quite small compared to the distance between two stations, it could be considered as a point object.
A monkey is sitting on top of a man cycling smoothly on a circular track.
Answer:
The monkey can be considered a point object as its size is quite small compared to the circumference of the circular track.
A spinning cricket ball that turns sharply on hitting the ground.
Answer:
The ball cannot be considered as a point object because the distance covered around the instant when it hits the ground is comparable to its size.
a tumbling beaker that has slipped off the edge of a table
Answer:
Since the size of the beaker is comparable to the distance it travels after slipping off the edge of the table, it can not be considered to be a point object.
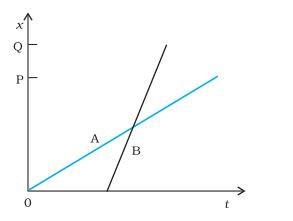
(A/B) lives closer to the school than (B/A)
Answer:
It is clear from the graph that A Lives closer to the school than B.
(A/B) starts from the school earlier than (B/A)
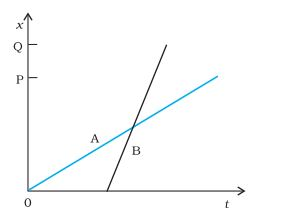
Answer:
From the graph, we can see that the position of A starts changing at time t=0, whereas in the case of B starts changing at some finite time and therefore A starts from the school earlier than B.
(A/B) walks faster than (B/A)
Answer:
The velocity of a particle is equal to the slope of its position-time (x-t) graph. Since the graph of B is steeper, B walks faster than A
A and B reach home at the (same/different) time
Answer:
The time(x-coordinate) is different for both A and B when their position(y-coordinate) is equal to that of their home. Therefore, A and B reach home at a different time.
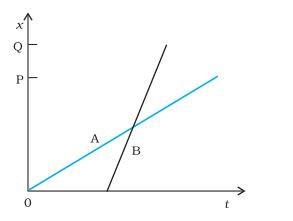
Q2.2(e) The position-time (x-t) graphs for two children A and B returning from their school O to their homes P and Q respectively are shown in Figure. Choose the correct entries in the brackets below
(A/B) overtakes (B/A) on the road (once/twice)
Answer:
B starts after A at a higher speed and overtakes A on the road once.
Answer:
Distance between the office and the home = 2.5 Km
The speed of the women=5 kmh -1
The time taken by the women to reach the office is
At 9:30 am, the woman is at the office till 5 pm
The speed of auto = 25 kmh -1
The time taken by the women to reach back to home is
At 5:06 pm, women reach home.
The position-time graph will be

Answer:
| time | 0 | 5 | 8 | 13 | 16 | 21 | 24 | 29 | 32 | 37 |
| distance covered | 0 | 5 | 2 | 7 | 4 | 9 | 6 | 11 | 8 | 13 |
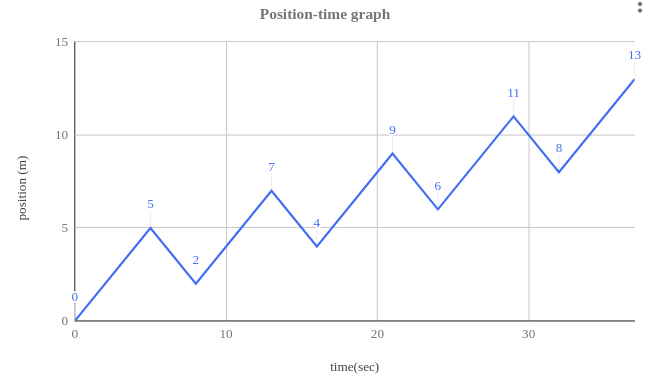
At time t= 37 sec, the man falls into a pit at 13 meters from the start
Answer:
Initial velocity(u)=126 km h-1 =35 m s-1
Final velocity(v)=0
Distance travelled before coming to rest(s)=200m
Using the third equation of motion
Using the first equation of motion
The retardation of the car is 3.0625 m s-2, and it takes 11.428 seconds for the car to stop.
Q 2.6(a) A player throws a ball upwards with an initial speed of 29.4 ms-1.What is the direction of acceleration during the upward motion of the ball?
Answer:
The acceleration of the ball will always be in the downward direction, irrespective of its position and direction of motion, since the gravitational force always acts in the downward direction.
Q 2.6(b) A player throws a ball upwards with an initial speed of 29.4 ms-1. What are the velocity and acceleration of the ball at the highest point of its motion?
Answer:
The velocity is 0 ms-1 and the acceleration is 9.8 m s-2 in the downward direction at the highest point of the motion of the ball.
Q 2.6(c) A player throws a ball upwards with an initial speed of 29.4 ms -1. Choose the x = 0 m and t = 0 s to be the location and time of the ball at its highest point, vertically downward direction to be the positive direction of x-axis, and give the signs of position, velocity and acceleration of the ball during its upward and downward motion.
Answer:
The sign of velocity is positive during the motion in the downward direction and negative during the motion in the upward direction. The signs of position and acceleration are positive during motion in both directions.
Q 2.6(d) A player throws a ball upwards with an initial speed of 29.4 ms -1. To what height does the ball rise and after how long does the ball return to the player’s hands? (Take g = 9.8 m s -2 and neglect air resistance).
Answer:
Consider the motion from the instant the ball starts travelling in the upward direction to the instant it reaches the highest point. Let the upwards direction be positive.
At the highest point, its velocity v is 0 m s-1.
Initially, the velocity u is 29.4 m s-1.
Acceleration is -g = -9.8 m s-2
Using the third equation of motion, we have
Consider the motion from the instant the ball starts travelling in the upward direction to the instant it reaches back to the player's hand.
The displacement during this period is s = 0 m.
Initial velocity is u=29.4 m s -1
Acceleration is a=-g=-9.8 m s -2
Using the second equation of motion, we have
The ball, therefore, reaches back to the player's hand in 6 seconds.
Note: The second solution of the above quadratic equation, t = 0, signifies that at the instant the ball starts travelling, its displacement is 0 m.
A particle in one-dimensional motion with zero speed at an instant may have non-zero acceleration at that instant
Answer:
True since non-zero velocity is not at all a necessary condition for non-zero acceleration. A ball thrown upwards at its highest point in motion has zero velocity but non-zero acceleration due to gravity.
A particle in one-dimensional motion with zero speed may have a non-zero velocity
Answer:
False, as speed is the magnitude of velocity; therefore, a non-zero velocity would imply a non-zero speed.
Q 2.7 (c) Read each statement below carefully and state with reasons and examples, if it is true or false;
A particle in one-dimensional motion with constant speed must have zero acceleration
Answer:
True. A particle moving in a straight line with constant speed will have constant velocity since its direction of motion is constant and as acceleration is defined as the rate of change of velocity, its acceleration will be zero.
Q 2.7 (d) Read each statement below carefully and state with reasons and examples, if it is true or false;
A particle in one-dimensional motion with a positive value of acceleration must be speeding up
Answer:
False. The answer to this question depends on the choice of a positive direction. If a ball is thrown upwards and the downwards direction is chosen to be positive, then its acceleration will be positive, but its speed will still be decreasing.
Answer:
At time t = 0 velocity is 0
Initial velocity, u = 0
Acceleration = 10 ms-2
Height, s = 90 m
The above is the speed with which the ball will collide with the ground. After colliding, the upward velocity becomes
Acceleration
While the ball will again reach the ground, its velocity will have the same magnitude
Let the time between the successive collisions be
After the first collision, its speed will become 0 in
Total time
After this much time, it will again bounce back with a velocity v given by
Q 2.9(a) Explain clearly, with examples, the distinction between: the magnitude of displacement (sometimes called distance) over an interval of time, and the total length of path covered by a particle over the same interval
Answer:
The magnitude of displacement is defined as the shortest distance between the initial and final position of a particle, whereas the total length of the path covered by a particle is the actual distance it has travelled. e.g a ball thrown upwards which goes to a height h and comes down to its starting position has its magnitude of displacement to be zero, but the total length of the path covered by the ball is 2h. In the case of 1-D motion, the two will be equal if the particle moves only in one direction.
Q 2.9(b) Explain clearly, with examples, the distinction between magnitude of average velocity over an interval of time, and the average speed over the same interval. [Average speed of a particle over an interval of time is defined as the total path length divided by the time interval]. Show in both (a) and (b) that the second quantity is either greater than or equal to the first. When is the equality sign true? [For simplicity, consider one-dimensional motion only]
Answer:
The magnitude of average velocity over an interval of time is defined as the total displacement upon the time taken, whereas the average speed over the same interval is defined as the actual distance travelled by the particle divided by the total time taken. e.g a ball thrown upwards which goes to a height h in time t and comes down to its starting position in the same time has the magnitude of average velocity zero, whereas the average speed of the ball is h/t. In the case of 1-D motion, the two will be equal if the particle moves only in one direction.
Answer:
(a).(i) Time taken by the man to reach the market is t1
Displacement in 30 minutes is d1 =2.5 km
Magnitude of average velocity=d1 /t1 = 5 km h-1
(b).(i) Distance travelled in 30 minutes is s1 =2.5 km
Average speed=s1 /t1 = 5 km h-1
In the first 30 minutes, the man travels from his home to the market.
During the next 10 minutes, he travels with a speed of 7.5 km h-1 towards his home, covering a distance of
t3 =40 min
Magnitude of average velocity =(2.5-1.25)/t3 =1.875 km h -1
Average speed=(2.5+1.25)/t 3 =5.625 km h-1
Q 2.11 The instantaneous speed is always equal to the magnitude of instantaneous velocity. Why?
Answer:
Instantaneous speed is defined as the first derivative of distance travelled with respect to time, and the magnitude of instantaneous velocity is the first derivative of the magnitude of displacement with respect to time. The time interval considered is so small that it is safe to assume that the particle won't change its direction of motion during it and therefore the magnitude of displacement during this interval will be the same as the distance travelled and therefore instantaneous speed is always equal to the magnitude of instantaneous velocity.
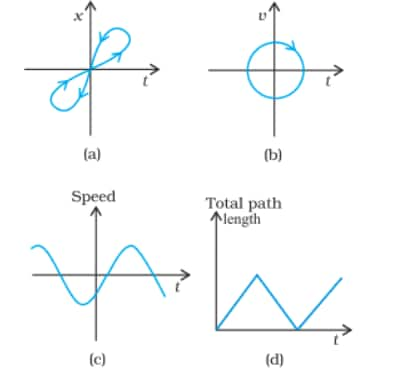
Answer:
(a) The given x-t graph cannot represent the one-dimensional motion of a particle as the particle cannot be at two positions at the same instant.
(b) The given v-t graph cannot represent the one-dimensional motion of a particle as the particle cannot be travelling at two velocities at the same instant.
(c) The given speed-time graph cannot represent the one-dimensional motion of a particle as the particle cannot have negative speed.
(d) The given path length-time graph cannot represent the one-dimensional motion of a particle as the total path length cannot decrease.
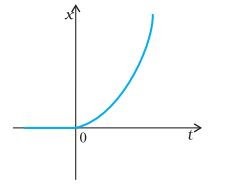
Answer:
It cannot be said from the above-given graph whether the particle is moving along a straight line or along a parabolic path, as the x-t graph does not tell us about the trajectory taken by the particle. From the given graph, we can only say that the position of the particle along the x-axis does not change till time t=0, and after that, it starts increasing in a non-linear manner.
Answer:
Muzzle speed of the bullet is V B =150 m s -1 =540 km h -1
Speed of the Police van is VV =30 km h -1
Resultant Speed of the bullet is V=540+30=570 km h -1
Speed of the thief's car= VT =192 km h -1
The speed with which the bullet hits the thief's car=V-VT =570-192=378 km h -1 =105 m s -1
Q 2.15 Suggest a suitable physical situation for each of the following graphs.
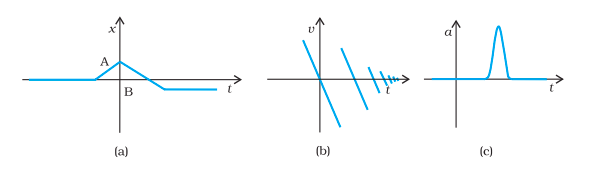
Answer:
(a)The particle is initially at rest. Then it starts moving with a constant velocity for some time and then its velocity changes instantaneously and it starts moving in the opposite direction, crosses the point where it was at rest initially and then comes to a halt.
A similar physical situation arises when a bowler throws a ball towards the batsman, the ball travels towards the batsman with some constant speed and after the batsman hits it, the ball goes past the bowler and gets caught by a fielder and its velocity comes down to zero within an instant.
(b)The velocity of a particle starts coming down to zero from some velocity with some constant acceleration, goes to zero and changes its direction. Then suddenly the direction of velocity changes andthe magnitude decreases.
This is the case when a ball is thrown in the air. It starts moving with some velocity and gets retarded by g, its velocity becomes zero at the highest point and its velocity then changes direction and as it hits the ground, it loses some of its speed and gets rebound and this keeps on happening till it comes to rest.
(c) The acceleration of a body is zero. It increases to some value for a very small period of time and again comes to zero.
This is the case when a football travelling horizontally hits a wall or is kicked by a player.
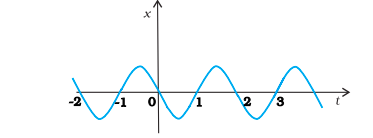
Answer:
At t=0.3 s signs of position, velocity and acceleration are negative, negative and positive.
At t=1.2 s signs of position, velocity and acceleration are positive, positive and negative.
At t=-1.2 s signs of position, velocity and acceleration are negative, positive and positive.
Note: The displacement of the particle as a function of time can be thought of as
where A is some positive real number equal to the amplitude of oscillation.
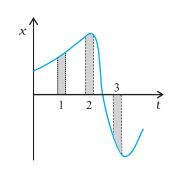
Answer:
The average speed is greatest in interval 3 and least in interval 2, as the average of the magnitude of the slope is maximum in 3 and minimum in 2.
Average velocity is positive in interval 1 and interval 2, as the slope is positive over these intervals, and average velocity is negative in interval 3, as the slope is negative over this interval.
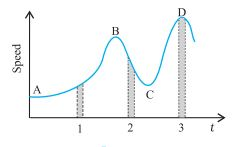
Answer:
The average acceleration is greatest in magnitude in interval 2, as in this interval the magnitude of change in speed is the greatest.
The average speed is greatest in interval 3.
| v | a | |
| Interval 1 | Positive | Positive |
| Interval 2 | Positive | Negative |
| Interval 3 | Positive | Zero |
In interval 1, speed increases, in interval 2 it decreases, and in interval 3 it remains constant.
At points A, B, C and D, acceleration is zero as at these points the curve is parallel to the time axis.
NCERT Solutions Class 11 Physics Chapter 2: Additional Questions
NCERT Solutions for Class 11 Physics Chapter 2: Motion in a Straight Line – Additional Questions provide extra practice beyond the textbook to build a deeper understanding of concepts like displacement, velocity, and motion graphs. These questions enhance analytical thinking and prepare students for competitive exams and higher-level problem-solving.
Answer:
V airplane =500 km h -1
V prod/airplane =-1500 km h -1 (the negative sign signifies the Velocity of the plane and the velocity of the ejected combustion products relative to the plane is in opposite directions)
V prod/airplane =V prod - V airplane
V prod =V prod/airplane +V airplane
V prod =-1500+500=-1000 km h -1
The speed of the ejected combustion products with respect to an observer on the ground is 1000 km h -1
Answer:
Since both objects are in motion, it will be easier for us to solve this problem in the relative frame. We take the train A as an observer.
Let the distance between the guard of B and the driver of A be s.
Since both trains are travelling with the same velocity initially, the relative initial velocity of B with respect to A(u) is 0.
Since A is not accelerating, the relative acceleration would be the same as the acceleration wrt the ground frame, a= 1ms -2
The time taken to cover s distance by B wrt A = 50 s
Using the second equation of motion
The distance between the guard of A and the driver of B initially is 1250 meters
Answer:
Velocity of car A=36 km h-1 = 10 m s-1
Velocity of car B and car C = 54 km h-1 = 15 m s-1
A and B are travelling in the same direction.
A and C are travelling in opposite directions.
The velocity of A w.r.t C is VAC = 25 m s-1.
The time in which A would reach C is t
The velocity of B with respect to A is VBA = 5 m s-1
Distance between A and B is s = 1000 m
Maximum time in which B has to overtake A=40s
The required acceleration can therefore be calculated using the second equation of motion
B has to have a minimum acceleration of 1 ms-2 to avoid an accident.
Answer:
Let the velocity of the bus be V km h -1
Let the period of the bus be T minutes.
The speed of the bus travelling in the same direction as the cyclist relative to the cyclist is = (V-20) km h -1
The speed of the bus travelling in the opposite direction as the cyclist relative to the cyclist is = (V+20) km h -1
The distance between two consecutive buses travelling in the same direction is s
This distance is, in turn, equal to the distance between the cyclist and the next bus at an instant when one bus goes past him.
Solving the above equations (i) and (ii) we get V=40 km h -1 and T=9 min
The buses travel at a speed of 40 km h-1 and the period of the bus service is 9 minutes.
Answer:
Initial velocity u = 0
Acceleration, a = 1 ms-2
t = n seconds
Let the total distance travelled in n seconds be Sn
Similarly, total distance travelled in
Distance travelled in
As we can see the dependency of x n on n is linear we conclude the plot of the distance covered by the vehicle during the nth second versus n would be a straight line.
Answer:
Let us consider the upward direction to be positive
Initial velocity of the ball (u) = 49 m s -1
The speed of the ball will be the same when it reaches the boy's hand's but will be moving in a downward direction. Therefore final velocity (v) = -49 m s -1
Acceleration (a) = -9.8 m s -2
Using the first equation of motion, we have
In the second case, as the ball has been thrown after the lift has started moving upwards with a constant velocity, the relative velocity of the ball with respect to the boy remains the same and therefore the ball will again take 10 seconds to reach the boy's hands.
Q 7 (a) On a long horizontally moving belt (Fig.), a child runs to and fro with a speed 9 kmh-1 (with respect to the belt) between his father and mother located 50 m apart on the moving belt. The belt moves with a speed of 4 kmh-1 . For an observer on a stationary platform outside, what is the speed of the child running in the direction of motion of the belt?
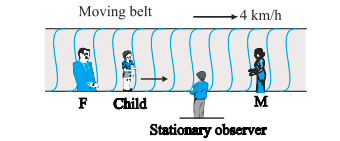
Answer:
(a) Speed of the child when the boy is running in the direction of the motion of the belt = 9 + 4 = 13 km h -1
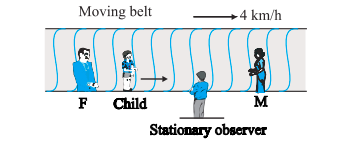
Answer:
(b) Speed of the child with respect to the stationary observer when the boy is running in the direction opposite to the motion of the belt = 9 - 4 = 5 km h -1
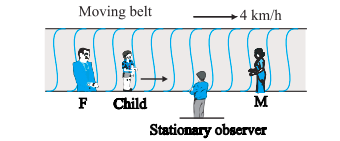
Answer:
The distance between the parents is s = 50 m
The relative velocity of the child with respect to both his parents remains the same, as the parents are also standing on the moving belt.
v = 9 km h -1 = 2.5 m s -1
Time taken by the child in (a) and (b) is t = s/v = 20 s.
As both parents are also standing on the belt as well the speed of the child would appear to be 9 km h -1 to both parents, irrespective of the direction in which the child is moving. Therefore, answers (a) and (b) would change.
The time taken by an object to travel from one point to another is independent of the observer and therefore the answer to question (c) would not change.
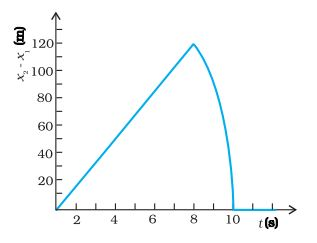
Answer:
As both the stones are being accelerated due to gravity, their effect will come on the relative motion only when one of them reaches the ground. Till that point of time, the relative velocity of the second stone would remain the same with respect to the first stone.
Let us consider the upward direction to be positive.
Initial velocity of the first stone(u) = 15 m s -1
Displacement from the point it has been thrown to the final point in its motion(s) = 200 m
Acceleration(a) = -g = -10 m s -2
Using the second equation of motion, we have
As time cannot be negative the correct value of
For this much time the relative distance changes with the relative velocity.
Maximum relative distance is
The graph is, therefore, correct till 8 seconds.
After that, the second stone will be moving towards the first stone with acceleration
The velocity of the second stone 8 seconds after it has been thrown can be calculated as follows using the first equation of motion
The time taken by it to travel 120 m in the downward direction can be calculated as follows using the second equation of motion
As t has to be positive, the correct answer is 2 seconds.
The relative distance will become zero after a total time of 10 seconds which is the case as shown in the graph and therefore the graph shown in Figure correctly represents the time variation of the relative position of the second stone with respect to the first.
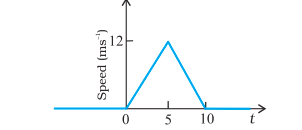
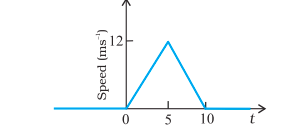
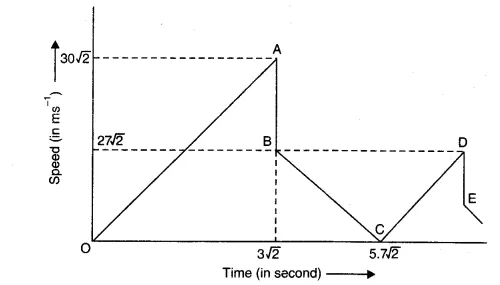
What is the average speed of the particle over the intervals in (a) and (b)?
Answer:
The distance traversed by the particle equals the area under the speed-time graph
The area under the curve is
The particle has travelled a distance of 60 m from t=0 s to t=10 s.
The average speed over this interval is
Q 9 The speed-time graph of a particle moving along a fixed direction is shown in Figure. Obtain the distance traversed by the particle between t = 2 s to 6 s.
What is the average speed of the particle over the intervals in (a) and (b)?
Answer:
As the speed is increasing in the time interval t = 0 s to t = 5 s, the acceleration is positive and can be given by
Speed at
Speed at
Distance travelled in interval
Acceleration is negative after
Speed at
Distance travelled in this interval can be calculated as follows
Total distance travelled from
The average speed over this interval is
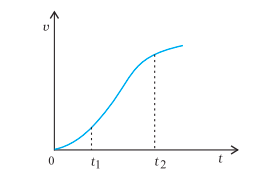
Q 10 The velocity-time graph of a particle in one-dimensional motion is shown in the figureWhich of the following formulae are correct for describing the motion of the particle over the time-interval t 1 to t 2 :
(f)
Answer:
Only the formulae given in (c), (d) and (f) are correct for describing the motion of the particle over the time interval t 1 to t 2.
The formulae given in (a), (b) and (e) are incorrect, as from the slope of the graph we can see that the particle is not moving with constant acceleration.
Also Read,
Class 11 Physics Chapter 2 - Motion in a straight line: Higher Order Thinking Skills (HOTS) Questions
Class 11 Physics Chapter 2 - Motion in a straight line Higher Order Thinking Skills (HOTS) Questions are aimed at testing the conceptual understanding and logical mind. The advanced problems also enable students to use the concepts of physics, such as speed, velocity, and equations of motion, and solve interesting cases, so that students are ready to take an exam and also enhance their problem-solving.
Q1: Particles A and B are moving with constant velocity in two perpendicular directions as shown in the figure. Find their velocity of separation

Answer:

Q2: A stone is dropped from a height h, simultaneously another stone is thrown up from the ground, which reaches the maximum height 3h, the two stones cross each other after time
Answer:
Let the initial velocities of stone 1 and stone 2 be u1 and u2, respectively, and their final velocities be v1 and v2, respectively.
At a maximum height of 3h, Velocity v2= O
Using
Let h1 be the distance covered by the stone which is dropped from a height h
Let h2 be the distance covered by the stone which is thrown up from the ground.
Let at time t, both stones crossed each other.
Adding h1 and h2 gives the height h
Using the value of
Q3: An escalator at a metro station moves at a speed of 2 ms–1 and is 200m long. If a man steps on it and walks at 3 ms–1 relative to the escalator, then which of the following statement(s) is/are correct? (t is the time required by him to reach the opposite side)
A. If he walks in the same direction as the escalator, t = 40 s
B. If he walks in the opposite direction of the escalator, t = 200 s
C. Both options A and B are correct.
D. Both options A and B are wrong.
Answer:
(a)
So
(b)
So
Hence, the answer is option (C).
Q4: A particle starts to move along the x-axis from x = -18 with an initial velocity of 3m/s such that its acceleration as a function of time is given as a = 4 - 2t. Find out the time when the velocity is 6m/s towards the right.
Answer:
Now as
Hence,
Now,
Therefore, by the above equation, the particle will be at the origin at
Hence, for the second time, when it reaches origin,
Q5: A rescue chopper is rising vertically up with a speed of
Answer:
Let the speed of the bomb w.r.t. the ground be V
In 3 s , the chopper rises by
It means the bomb is at a height of 15 m from its initial point of projection after 3 s. Using the second equation of motion, we have
Class 11 Physics Chapter 2 - Motion in a Straight Line Question Answers: Topics
NCERT Solutions Class 11 Physics Chapter 2: Motion in a Straight Line - This chapter concentrates on fundamental topics in motion, such as displacement, distance, average speed, instantaneous velocity, equations of motion and motion graphs. These are some of the aspects that form an effective background to learn about advanced topics in mechanics and competitive exam-oriented learning.
2.1 Introduction
2.2 Instantaneous velocity and speed
2.3 Acceleration
2.4 Kinematic equations for uniformly accelerated motion
What Extra Should Students Study Beyond NCERT for JEE?
In JEE preparation, NCERT gives a good foundation of Motion in a Straight Line, which can not be sufficient to solve some complicated questions. Students should study more than the basics and learn on higher-level problem-solving, graphical analysis, relative motion and calculus-based applications to improve their concepts and increase accuracy in exams.
Also Check NCERT Books and NCERT Syllabus here
NCERT Solutions for Class 11 Physics Chapter-Wise
NCERT Solutions for Class 11 Physics are provided chapter-wise to make learning structured and systematic. These solutions cover every exercise, important question, and additional practice material, helping students strengthen concepts and prepare effectively for both CBSE exams and competitive tests like JEE/NEET
NCERT Solutions for Class 11 Subject Wise
Frequently Asked Questions (FAQs)
Yes, the solutions PDF for Motion in a Straight Line in Class 11 is available and includes both theory-based and numerical questions with easy-to-follow answers.
The PDF includes solved numerical problems based on displacement, speed, velocity, acceleration, and motion graphs, all designed to strengthen your understanding and improve problem-solving skills.
You will find textbook exercises, conceptual questions, and additional problems to test your understanding and prepare well for exams.
Yes, when an object is moving with constant velocity (no change in speed or direction), its acceleration is zero.
A horizontal line in a velocity-time graph means the object is moving with uniform velocity (zero acceleration).
Popular Questions
Courses After 12th
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters