NCERT Solutions for Class 10 Maths Chapter 6 Exercise 6.3 - Triangles
CBSE Class 10th Exam Date:17 Feb' 26 - 17 Feb' 26
Geometry consists of an important portion dedicated to triangles, which gains clarity through studying congruence rules for triangle properties. The concepts of triangular inequalities receive attention in this exercise. The lesson demonstrates the connections between sides and angles and shows how we can derive important findings through such comparisons. Basic triangle properties enable us to foretell various side-angle relationships without performing physical measurements.
This Story also Contains
- NCERT Solutions Class 10 Maths Chapter 6: Exercise 6.3
- Access Solution of Triangles Class 10 Chapter 6 Exercise: 6.3
- Topics covered in Chapter 10 Triangles: Exercise 6.3
- NCERT Solutions of Class 10 Subject Wise
- NCERT Exemplar Solutions of Class 10 Subject Wise

The content of Exercise 6.3 within the NCERT Solutions allows students to study questions concerning triangle inequalities and side–angle relationships. Such a study enables students to build their logical thinking combined with proof-writing competency by analysing relationships between "the side opposite to a larger angle is longer" and "the angle opposite to a longer side is greater." Through problem-solving activities in the NCERT Books, students gain confidence, which enables them to handle triangle inequality properties both in problems of daily life and competitive testing.
Access Solution of Triangles Class 10 Chapter 6 Exercise: 6.3
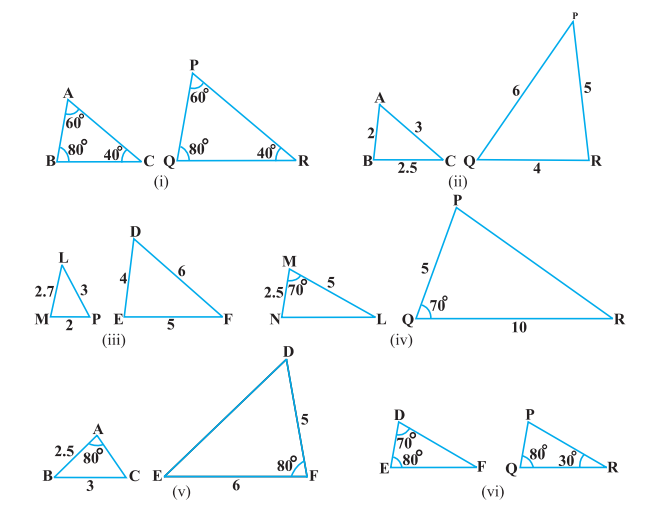
Answer:
(i) $\angle A=\angle P=60 ^\circ$
$\angle B=\angle Q=80 ^\circ$
$\angle C=\angle R=40 ^\circ$
$\therefore \triangle ABC \sim \triangle PQR$ (By AAA)
So , $\frac{AB}{QR}=\frac{BC}{RP}=\frac{CA}{PQ}$
(ii) As corresponding sides of both triangles are proportional.
$\therefore \triangle ABC \sim \triangle PQR$ (By SSS)
(iii) Given triangles are not similar because corresponding sides are not proportional.
(iv) $\triangle MNL \sim \triangle PQR$ by SAS similarity criteria.
(v) Given triangles are not similar because the corresponding angle is not contained by two corresponding sides
(vi) In $\triangle DEF$ , we know that
$\angle D+\angle E+\angle F=180 ^\circ$
$\Rightarrow 70 ^\circ+80 ^\circ+\angle F=180 ^\circ$
$\Rightarrow 150 ^\circ+\angle F=180 ^\circ$
$\Rightarrow \angle F=180 ^\circ-150 ^\circ=30 ^\circ$
In $\triangle PQR$ , we know that
$\angle P+\angle Q+\angle R=180 ^\circ$
$\Rightarrow 30 ^\circ+80 ^\circ+\angle R=180 ^\circ$
$\Rightarrow 110 ^\circ+\angle R=180 ^\circ$
$\Rightarrow \angle R=180 ^\circ-110 ^\circ=70 ^\circ$
$\angle Q=\angle P=70 ^\circ$
$\angle E=\angle Q=80 ^\circ$
$\angle F=\angle R=30 ^\circ$
$\therefore \triangle DEF\sim \triangle PQR$ ( By AAA)
Q2 In Fig. 6.35, $\Delta ODC \sim \Delta OBA$ , $\angle BOC = 125 ^\circ$ and $\angle CDO = 70 ^\circ$ . Find $\angle DOC , \angle DCO , \angle OAB$
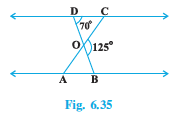
Answer:
Given : $\Delta ODC \sim \Delta OBA$ , $\angle BOC = 125 ^\circ$ and $\angle CDO = 70 ^\circ$
$\angle DOC+\angle BOC=180 ^\circ$ (DOB is a straight line)
$\Rightarrow \angle DOC+125 ^\circ=180 ^\circ$
$\Rightarrow \angle DOC=180 ^\circ-125 ^\circ$
$\Rightarrow \angle DOC=55 ^\circ$
In $\Delta ODC ,$
$\angle DOC+\angle ODC+\angle DCO=180 ^\circ$
$\Rightarrow 55 ^\circ+ 70 ^\circ+\angle DCO=180 ^\circ$
$\Rightarrow \angle DCO+125 ^\circ=180 ^\circ$
$\Rightarrow \angle DCO=180 ^\circ-125 ^\circ$
$\Rightarrow \angle DCO=55 ^\circ$
Since , $\Delta ODC \sim \Delta OBA$ , so
$\Rightarrow\angle OAB= \angle DCO=55 ^\circ$ ( Corresponding angles are equal in similar triangles).
Answer:
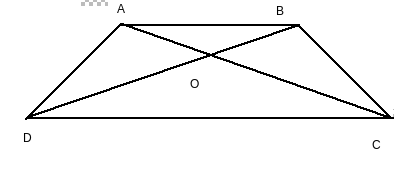
In $\triangle DOC\, and\, \triangle BOA$ , we have
$\angle CDO=\angle ABO$ ( Alternate interior angles as $AB||CD$ )
$\angle DCO=\angle BAO$ ( Alternate interior angles as $AB||CD$ )
$\angle DOC=\angle BOA$ ( Vertically opposite angles are equal)
$\therefore \triangle DOC\, \sim \, \triangle BOA$ ( By AAA)
$\therefore \frac{DO}{BO}=\frac{OC}{OA}$ ( corresponding sides are equal)
$\Rightarrow \frac{OA }{OC} = \frac{OB }{OD }$
Hence proved.
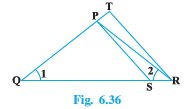
Answer:
Given : $\frac{QR }{QS } = \frac{QT}{PR}$ and $\angle 1 = \angle 2$
To prove : $\Delta PQS \sim \Delta TQR$
In $\triangle PQR$ , $\angle PQR=\angle PRQ$
$\therefore PQ=PR$
$\frac{QR }{QS } = \frac{QT}{PR}$ (Given)
$\Rightarrow \frac{QR }{QS } = \frac{QT}{PQ}$
In $\Delta PQS\, and\, \Delta TQR$ ,
$\Rightarrow \frac{QR }{QS } = \frac{QT}{PQ}$
$\angle Q=\angle Q$ (Common)
$\Delta PQS \sim \Delta TQR$ ( By SAS)
Answer:
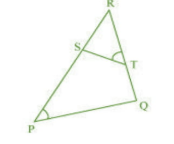
Given : $\angle$ P = $\angle$ RTS
To prove RPQ ~ $\Delta$ RTS.
In $\Delta$ RPQ and $\Delta$ RTS,
$\angle$ P = $\angle$ RTS (Given )
$\angle$ R = $\angle$ R (common)
$\Delta$ RPQ ~ $\Delta$ RTS. ( By AA)
Q6 In Fig. 6.37, if $\Delta$ ABE $\equiv$ $\Delta$ ACD, show that $\Delta$ ADE ~ $\Delta$ ABC.
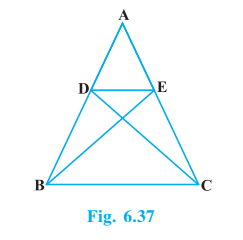
Answer:
Given : $\triangle ABE \cong \triangle ACD$
To prove ADE ~ $\Delta$ ABC.
Since $\triangle ABE \cong \triangle ACD$
$AB=AC$ ( By CPCT)
$AD=AE$ (By CPCT)
In $\Delta$ ADE and $\Delta$ ABC,
$\angle A=\angle A$ ( Common)
and
$\frac{AD}{AB}=\frac{AE}{AC}$ ( $AB=AC$ and $AD=AE$ )
Therefore, $\Delta$ ADE ~ $\Delta$ ABC. ( By SAS criteria)
Q7 (1) In Fig. 6.38, altitudes AD and CE of $\Delta ABC$ intersect each other at the point P. Show that: $\Delta AEP \sim \Delta CDP$
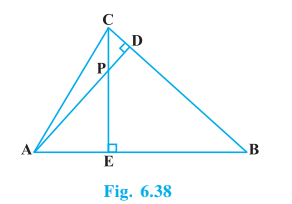
Answer:
To prove : $\Delta AEP \sim \Delta CDP$
In $\Delta AEP \, \, and\, \, \Delta CDP$ ,
$\angle AEP=\angle CDP$ ( Both angles are right angle)
$\angle APE=\angle CPD$ (Vertically opposite angles )
$\Delta AEP \sim \Delta CDP$ ( By AA criterion)
Q7 (2) In Fig. 6.38, altitudes AD and CE of $\Delta ABC$ intersect each other at the point P. Show that: $\Delta ABD \sim \Delta CBE$
Answer:
To prove : $\Delta ABD \sim \Delta CBE$
In $\Delta ABD \, \, and\, \, \Delta CBE$ ,
$\angle ADB=\angle CEB$ ( Both angles are right angle)
$\angle ABD=\angle CBE$ (Common )
$\Delta ABD \sim \Delta CBE$ ( By AA criterion)
Q7 (3) In Fig. 6.38, altitudes AD and CE of $\Delta ABC$ intersect each other at the point P. Show that: $\Delta AEP \sim \Delta ADB$
Answer:
To prove : $\Delta AEP \sim \Delta ADB$
In $\Delta AEP \, \, \, and\, \, \Delta ADB$ ,
$\angle AEP=\angle ADB$ ( Both angles are right angle)
$\angle A=\angle A$ (Common )
$\Delta AEP \sim \Delta ADB$ ( By AA criterion)
Q7 (4) In Fig. 6.38, altitudes AD and CE of $\Delta ABC$ intersect each other at the point P. Show that: $\Delta PDC \sim \Delta BEC$
Answer:
To prove : $\Delta PDC \sim \Delta BEC$
In $\Delta PDC \, \, and\, \, \, \Delta BEC$ ,
$\angle CDP=\angle CEB$ ( Both angles are right angle)
$\angle C=\angle C$ (Common )
$\Delta PDC \sim \Delta BEC$ ( By AA criterion)
Answer:
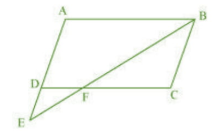
To prove : $\Delta ABE \sim \Delta CFB$
In $\Delta ABE \, \, \, and\, \, \Delta CFB$ ,
$\angle A=\angle C$ ( Opposite angles of a parallelogram are equal)
$\angle AEB=\angle CBF$ ( Alternate angles of AE||BC)
$\Delta ABE \sim \Delta CFB$ ( By AA criterion )
Q9 (1) In Fig. 6.39, ABC and AMP are two right triangles, right angled at B and M respectively. Prove that: $\Delta ABC \sim \Delta AMP$
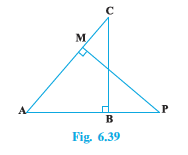
Answer:
To prove : $\Delta ABC \sim \Delta AMP$
In $\Delta ABC \, \, and\, \, \Delta AMP$ ,
$\angle ABC=\angle AMP$ ( Each $90 ^\circ$ )
$\angle A=\angle A$ ( common)
$\Delta ABC \sim \Delta AMP$ ( By AA criterion )
Answer:
To prove :
$\frac{CA }{PA } = \frac{BC }{MP}$
In $\Delta ABC \, \, and\, \, \Delta AMP$ ,
$\angle ABC=\angle AMP$ ( Each $90 ^\circ$ )
$\angle A=\angle A$ ( common)
$\Delta ABC \sim \Delta AMP$ ( By AA criterion )
$\frac{CA }{PA } = \frac{BC }{MP}$ ( corresponding parts of similar triangles )
Hence proved.
Q10 (1) CD and GH are respectively the bisectors of $\angle ACB$ and $\angle EGF$ such that D and H lie on sides AB and FE of $\Delta ABC\: \: and\: \: \Delta EGF$ respectively. If $\Delta ABC \sim \Delta EGF$ , show that: $\frac{CD}{GH} = \frac{AC}{FG}$
Answer:
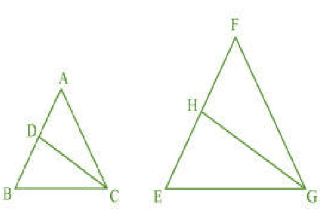
To prove :
$\frac{CD}{GH} = \frac{AC}{FG}$
Given : $\Delta ABC \sim \Delta EGF$
$\angle A=\angle F,\angle B=\angle E\, \, and \, \, \angle ACB=\angle FGE,\angle ACB=\angle FGE$
$\therefore \angle ACD=\angle FGH$ ( CD and GH are bisectors of equal angles)
$\therefore \angle DCB=\angle HGE$ ( CD and GH are bisectors of equal angles)
In $\Delta ACD \, \, and\, \, \Delta FGH$
$\therefore \angle ACD=\angle FGH$ ( proved above)
$\angle A=\angle F$ ( proved above)
$\Delta ACD \sim \Delta FGH$ ( By AA criterion)
$\Rightarrow \frac{CD}{GH} = \frac{AC}{FG}$
Hence proved.
Q 10 (2) CD and GH are respectively the bisectors of $\angle ABC \: \:and \: \: \angle EGF$ such that D and H lie on sides AB and FE of $\Delta ABC \: \:and \: \: \Delta EGF$ respectively. If $\Delta ABC \sim \Delta EGF$ , show that: $\Delta DCB \sim \Delta HGE$
Answer:
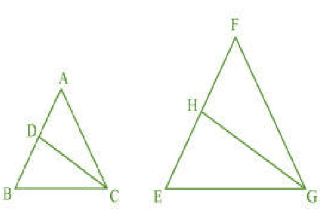
To prove : $\Delta DCB \sim \Delta HGE$
Given : $\Delta ABC \sim \Delta EGF$
In $\Delta DCB \,\, \, and\, \, \Delta HGE$ ,
$\therefore \angle DCB=\angle HGE$ ( CD and GH are bisectors of equal angles)
$\angle B=\angle E$ ( $\Delta ABC \sim \Delta EGF$ )
$\Delta DCB \sim \Delta HGE$ ( By AA criterion )
Q10 (3) CD and GH are respectively the bisectors of $\angle ABC \: \:and \: \: \angle EGF$ such that D and H lie on sides AB and FE of $\Delta ABC \: \:and \: \: \Delta EGF$ respectively. If $\Delta ABC\sim \Delta EGF$ , show that: $\Delta DCA \sim \Delta HGF$
Answer:
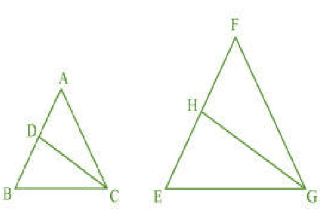
To prove : $\Delta DCA \sim \Delta HGF$
Given : $\Delta ABC \sim \Delta EGF$
In $\Delta DCA \, \, \, and\, \, \Delta HGF$ ,
$\therefore \angle ACD=\angle FGH$ ( CD and GH are bisectors of equal angles)
$\angle A=\angle F$ ( $\Delta ABC \sim \Delta EGF$ )
$\Delta DCA \sim \Delta HGF$ ( By AA criterion )
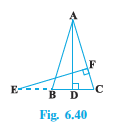
Answer:
To prove : $\Delta ABD \sim \Delta ECF$
Given: ABC is an isosceles triangle.
$AB=AC \, \, and\, \, \angle B=\angle C$
In $\Delta ABD \, \, and\, \, \Delta ECF$ ,
$\angle ABD=\angle ECF$ ( $\angle ABD=\angle B=\angle C=\angle ECF$ )
$\angle ADB=\angle EFC$ ( Each $90 ^\circ$ )
$\Delta ABD \sim \Delta ECF$ ( By AA criterion)
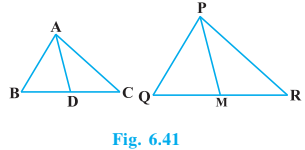
Answer:
AD and PM are medians of triangles. So,
$BD=\frac{BC}{2}\, and\, QM=\frac{QR}{2}$
Given :
$\frac{AB}{PQ}=\frac{BC}{QR}=\frac{AD}{PM}$
$\Rightarrow \frac{AB}{PQ}=\frac{\frac{1}{2}BC}{\frac{1}{2}QR}=\frac{AD}{PM}$
$\Rightarrow \frac{AB}{PQ}=\frac{BD}{QM}=\frac{AD}{PM}$
In $\triangle ABD\, and\, \triangle PQM,$
$\frac{AB}{PQ}=\frac{BD}{QM}=\frac{AD}{PM}$
$\therefore \triangle ABD\sim \triangle PQM,$ (SSS similarity)
$\Rightarrow \angle ABD=\angle PQM$ ( Corresponding angles of similar triangles )
In $\triangle ABC\, and\, \triangle PQR,$
$\Rightarrow \angle ABD=\angle PQM$ (proved above)
$\frac{AB}{PQ}=\frac{BC}{QR}$
Therefore, $\Delta ABC \sim \Delta PQR$ . ( SAS similarity)
Answer:
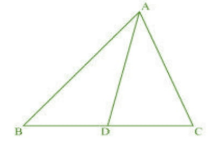
In, $\triangle ADC \, \, and\, \, \triangle BAC,$
$\angle ADC = \angle BAC$ ( given )
$\angle ACD = \angle BCA$ (common )
$\triangle ADC \, \, \sim \, \, \triangle BAC,$ ( By AA rule)
$\frac{CA}{CB}=\frac{CD}{CA}$ ( corresponding sides of similar triangles )
$\Rightarrow CA^2=CB\times CD$
Answer:
$\frac{AB}{PQ}=\frac{AC}{PR}=\frac{AD}{PM}$ (given)
Produce AD and PM to E and L such that AD=DE and PM=DE. Now,
join B to E,C to E,Q to L and R to L.
AD and PM are medians of a triangle, therefore
QM=MR and BD=DC
AD = DE (By construction)
PM=ML (By construction)
So, diagonals of ABEC bisecting each other at D,so ABEC is a parallelogram.
Similarly, PQLR is also a parallelogram.
Therefore, AC=BE ,AB=EC and PR=QL,PQ=LR
$\frac{AB}{PQ}=\frac{AC}{PR}=\frac{AD}{PM}$ (Given )
$\Rightarrow \frac{AB}{PQ}=\frac{BE}{QL}=\frac{2.AD}{2.PM}$
$\Rightarrow \frac{AB}{PQ}=\frac{BE}{QL}=\frac{AE}{PL}$
$\Delta ABE \sim \Delta PQL$ (SSS similarity)
$\angle BAE=\angle QPL$ ...................1 (Corresponding angles of similar triangles)
Similarity, $\triangle AEC=\triangle PLR$
$\angle CAE=\angle RPL$ ........................2
Adding equation 1 and 2,
$\angle BAE+\angle CAE=\angle QPL+\angle RPL$
$\angle CAB=\angle RPQ$ ............................3
In $\triangle ABC\, and\, \, \triangle PQR,$
$\frac{AB}{PQ}=\frac{AC}{PR}$ ( Given )
$\angle CAB=\angle RPQ$ ( From above equation 3)
$\triangle ABC\sim \triangle PQR$ ( SAS similarity)
Answer:
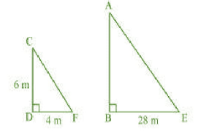
CD = pole
AB = tower
Shadow of pole = DF
Shadow of tower = BE
In $\triangle ABE\, \, and\, \triangle CDF,$
$\angle CDF=\angle ABE$ ( Each $90 ^\circ$ )
$\angle DCF=\angle BAE$ (Angle of sun at same place )
$\triangle ABE\, \, \sim \, \triangle CDF,$ (AA similarity)
$\frac{AB}{CD}=\frac{BE}{QL}$
$\Rightarrow \frac{AB}{6}=\frac{28}{4}$
$\Rightarrow AB=42$ cm
Hence, the height of the tower is 42 cm.
Answer:
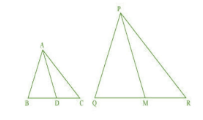
$\Delta AB C \sim \Delta PQR$ ( Given )
$\frac{AB}{PQ}=\frac{AC}{PR}=\frac{BC}{QR}$ ............... ....1( corresponding sides of similar triangles )
$\angle A=\angle P,\angle B=\angle Q,\angle C=\angle R$ ....................................2
AD and PM are medians of triangle.So,
$BD=\frac{BC}{2}\, and\, QM=\frac{QR}{2}$ ..........................................3
From equation 1 and 3, we have
$\frac{AB}{PQ}=\frac{BD}{QM}$ ...................................................................4
In $\triangle ABD\, and\, \triangle PQM,$
$\angle B=\angle Q$ (From equation 2)
$\frac{AB}{PQ}=\frac{BD}{QM}$ (From equation 4)
$\triangle ABD\, \sim \, \triangle PQM,$ (SAS similarity)
$\frac{AB}{PQ}=\frac{BD}{QM}=\frac{AD}{PM}$
Topics covered in Chapter 10 Triangles: Exercise 6.3
1. Understanding the relationship between sides and angles of a triangle: All triangles follow the pattern where large angles pair with extended sides, but small angles match short sides, thus creating a direct relationship between angle measures and side lengths.
2. Applying the triangle inequality property to compare sides: Understand how the rule functions, that the two connecting triangle sides should exceed the third side measure for triangle formation.
3. Determining which angle is larger based on side lengths: Strategies must be developed to understand side length ratios so that one can predict angles' relative size measurements without performing actual measurements.
4. Determining which side is longer based on angle measures: Interpretation of given angle measures allows students to determine opposite side relations, leading to improved triangle analysis abilities.
5. Logical reasoning with inequalities in triangles: Logical reasoning with inequalities in triangles: Students should apply triangle inequality properties to critical problem-solving through proofs and comparisons, and effective problem-solving methods.
Also Read,
Also, See:
- NCERT Solutions for Class 10 Maths Chapter 6 – Triangles
- NCERT Exemplar Solutions for Class 10 Maths Chapter 6- Triangles
NCERT Solutions of Class 10 Subject Wise
Students must check the NCERT solutions for class 10 of Mathematics and Science Subjects.
NCERT Exemplar Solutions of Class 10 Subject Wise
Students must check the NCERT Exemplar solutions for class 10 of Mathematics and Science Subjects.
Frequently Asked Questions (FAQs)
Yes, because a triangle has three angles and their sum remains 180 degrees. So, if two angels are equal then the third also becomes equal.
We can find the length of the corresponding side of the other triangle using the side ratio and the length of any side. Practice 10th class maths exercise 6.3 answers to command the concepts.
This exercise is about the criteria or theorems for congruence of triangles. Practice this class 10 ex 6.3 to command the concepts.
In this class 10 maths ex 6.3 following theorems are discussed in detail.
Angle-angle-angle
Angle-side-angle
Side-side-side
Angle-angle
Angle-angle-side
Side-angle-side
Go through this ex 6.3 class 10 to get deeper understanding of related concepts.
The sum of a triangle's angels is 180 degrees.
No, similarity simply refers to the shape of the figures; it does not imply equality.
Yes, if all the three sides of two triangles are in proportion, then does this means the triangles are similar. By side-side-side criteria.
If the ratio becomes equal to 1 then the triangles are congruent to each other.
Questions related to CBSE Class 10th
On Question asked by student community
Hello,
The CBSE exam fee for Class 10 students is as follows:
-
For up to 5 subjects: Rs. 1,600 per student
-
For each additional subject: Rs. 320
-
Late fee (after the due date): Rs. 2,000
These fees are applicable for students studying in India as per the latest CBSE notification.
The school fee depends upon the particular school.
Hope it helps !
Hello aspirant,
The Sample Question Paper (SQP) and marking guidelines have been made available by the Central Board of Secondary Education (CBSE). Although the board does not formally provide distinct half-yearly sample papers, many of the final CBSE sample papers' questions address subjects that are covered in the exams.
To get the sample papers, you can visit our site through following link:
https://school.careers360.com/boards/cbse/cbse-class-12-half-yearly-sample-papers-2025-26
Thank you
You can get CBSE half yearly english question papers on the Careers360 website by searching for your class and subject. You can view or download the papers in PDF format and use them to prepare well for your exams.
Hello! If you are looking for the CM Shri School admission result, here is the link provided by Careers360. You can check your result directly and stay updated about the admission status. I’ll be attaching it for your reference.
https://school.careers360.com/articles/cm-shri-school-admission-test-2025
https://school.careers360.com/articles/cm-shri-school-admission-test-result-2025
Hello Rajesh!!!
I have question paper of science of 9th standard . Here is your question paper link where you have click to open and wrap it up.
https://school.careers360.com/boards/dge-tamil-nadu/tn-class-9-quarterly-exam-question-paper-2025-26
Popular CBSE Class 10th Questions
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters
