Heron's Formula Class 9th Notes - Free NCERT Class 9 Maths Chapter 12 Notes - Download PDF

A triangle is a geometrical figure that has three sides and three corners, and three angles. It is a closed shape figure with the least number of sides. There are many types of triangles, and based on the properties, there are some formulas to calculate the area or perimeter of the triangle. The word triangle comes from the Latin word triangulus, and it means three-cornered or having three angles. Heron was a mathematician and engineer in Alexandria in Egypt, who derived the formula for calculating the area of triangles by using only the sides of the triangle. Triangles are used in many areas, like in construction, building design, sailing and navigation, art and design, pyramids, calculus, calculating distance, locating objects, etc.

New: Get up to 90% Scholarship on NEET/JEE Coaching from top Coaching Institutes

This Story also Contains

1. NCERT notes Class 9 Maths Chapter 12 Heron's Formula
2. Area of a Triangle by Heron's Formula
3. Class 9 Chapter Wise Notes
4. NCERT Solutions for Class 9
5. NCERT Exemplar Solutions for Class 9
6. NCERT Books and Syllabus

These notes cover the basic definition of triangles, types of triangles, and formulae for calculating the area and perimeter of the triangle. CBSE Class 9 chapter includes examples for the given topics as required. Students must practice all the questions and examples to ace the topic. Subject Matter Experts designed the NCERT class 9th maths notes as per the latest syllabus. Students can download NCERT notes according to their standard and subjects.

NCERT notes Class 9 Maths Chapter 12 Heron's Formula

Triangle: A geometrical closed figure that has three sides, three angles and three corners. The three corners are also called the vertices of the triangle.

Types of Triangles

Triangles can be divided into two categories:
1. Based on sides
2. Based on angels

Based on Sides

1. Equilateral Triangle
2. Isosceles Triangle
3. Scalene Triangle

Equilateral Triangle:

A triangle in which all sides are equal is called an equilateral triangle.

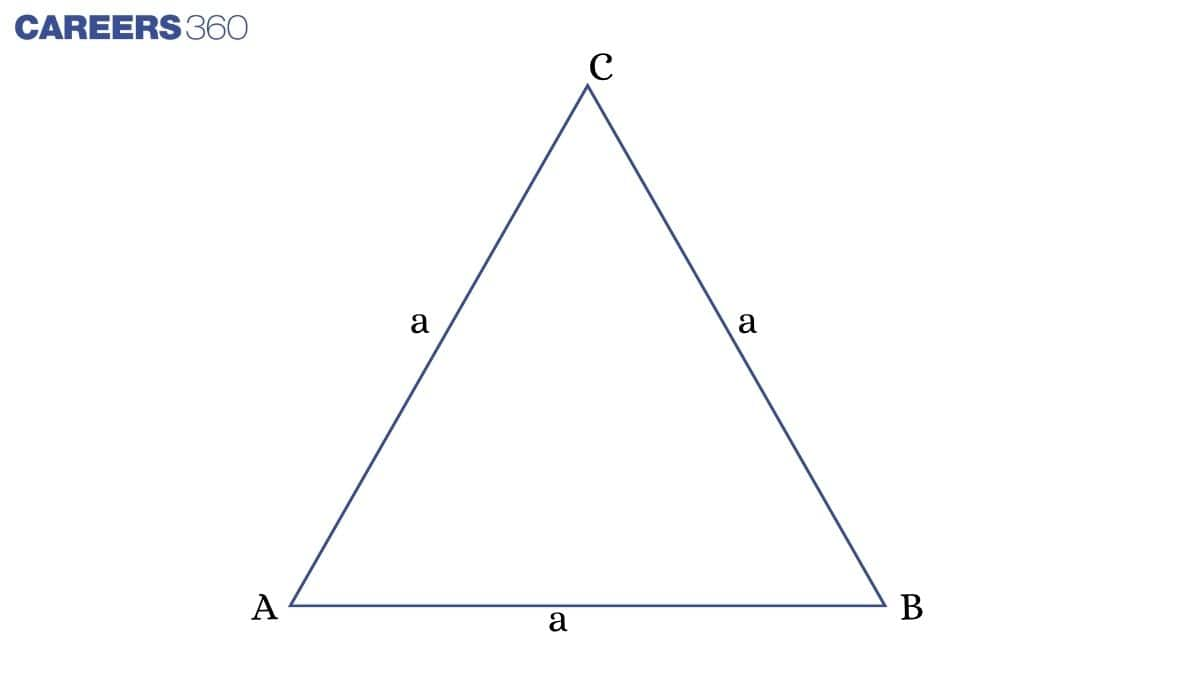
Area of equilateral triangle = $\frac{\sqrt3}{4}a^2$

Perimeter of equilateral triangle = $3a$

Where,

a is the side of the triangle.

Example: Find the area and perimeter of the equilateral triangle, in which the length of the side is 10cm.

Area of equilateral triangle = $\frac{\sqrt3}{4}10^2$

= $25\sqrt3$$cm^2$

Perimeter of equilateral triangle = $3 × 10$

= $30$

Isosceles Triangle:

A triangle in which any two sides are equal is called an isosceles triangle.

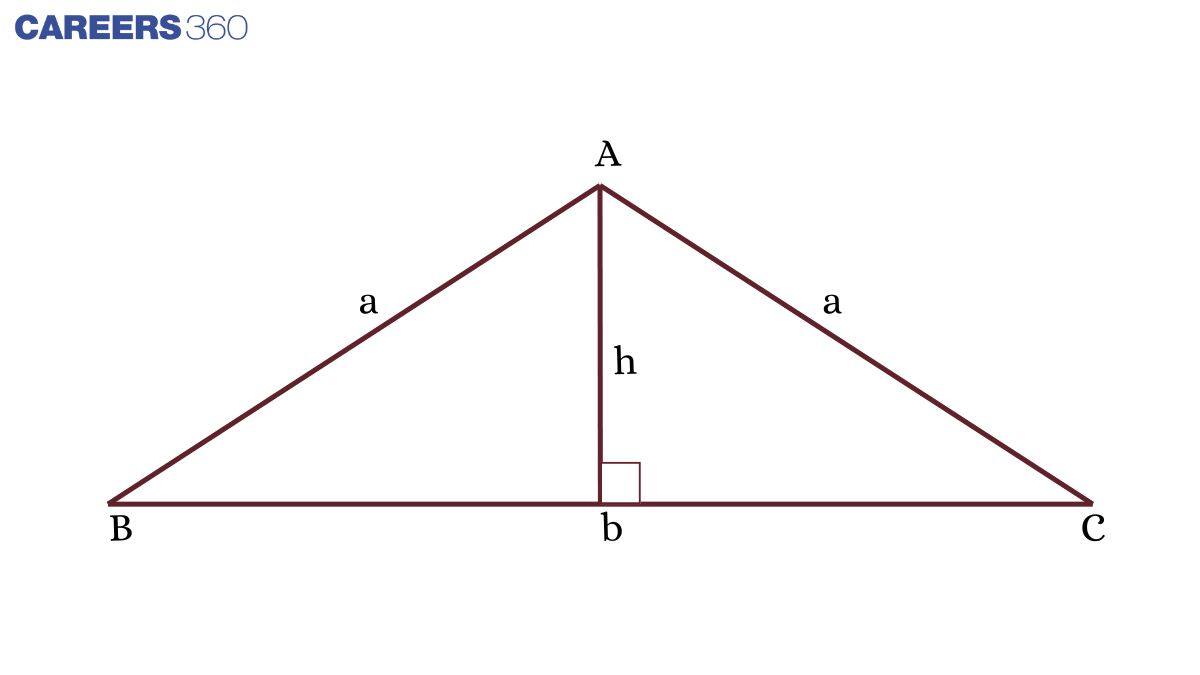
Area of isosclales triangle = $\frac{1}{2}bh$

Perimeter of an isosceles triangle = $2a + b$

Example: Find the area and perimeter of the triangle whose base is 12 cm long and each equal side is 16 cm long.

Area of isosclales triangle = $\frac{1}{2}12 × 16$

Area of isosclales triangle = $96$

Perimeter of an isosceles triangle = $2 × 16 + 12$

Perimeter of an isosceles triangle = $44$

Scalene Triangle

A triangle where the length of each side is different is known as a scalene triangle.

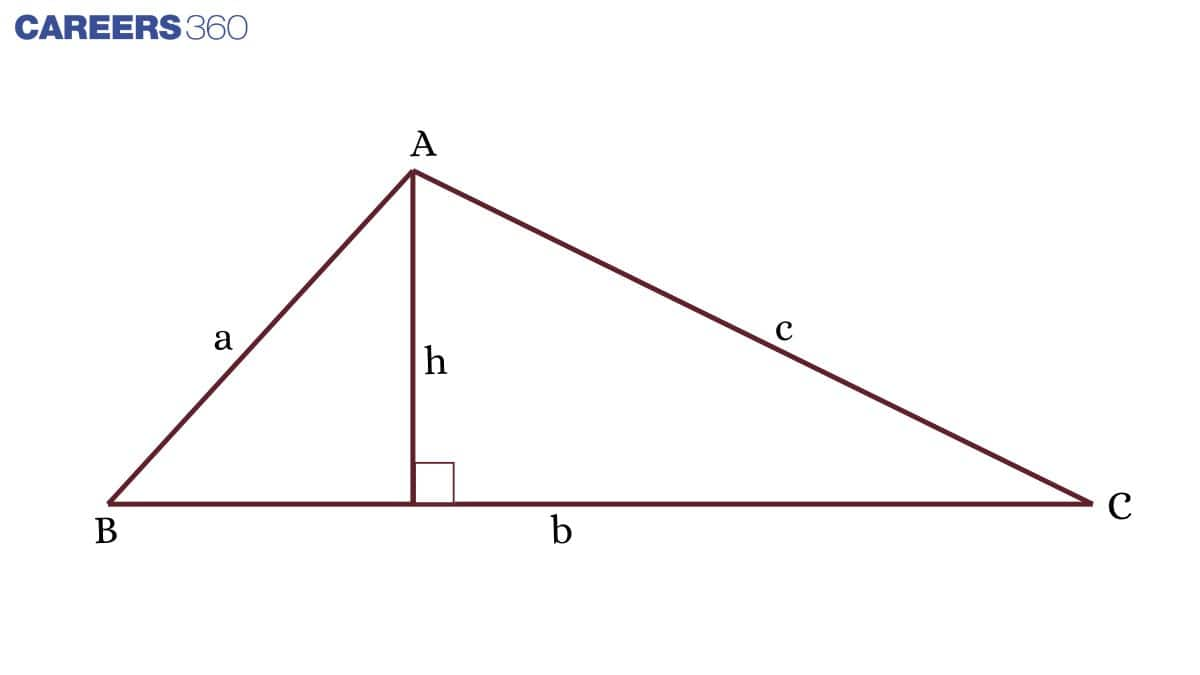
Area of scalene triangle = $\frac{1}{2}bh$

Perimeter of an scalene triangle = $a + b + c$

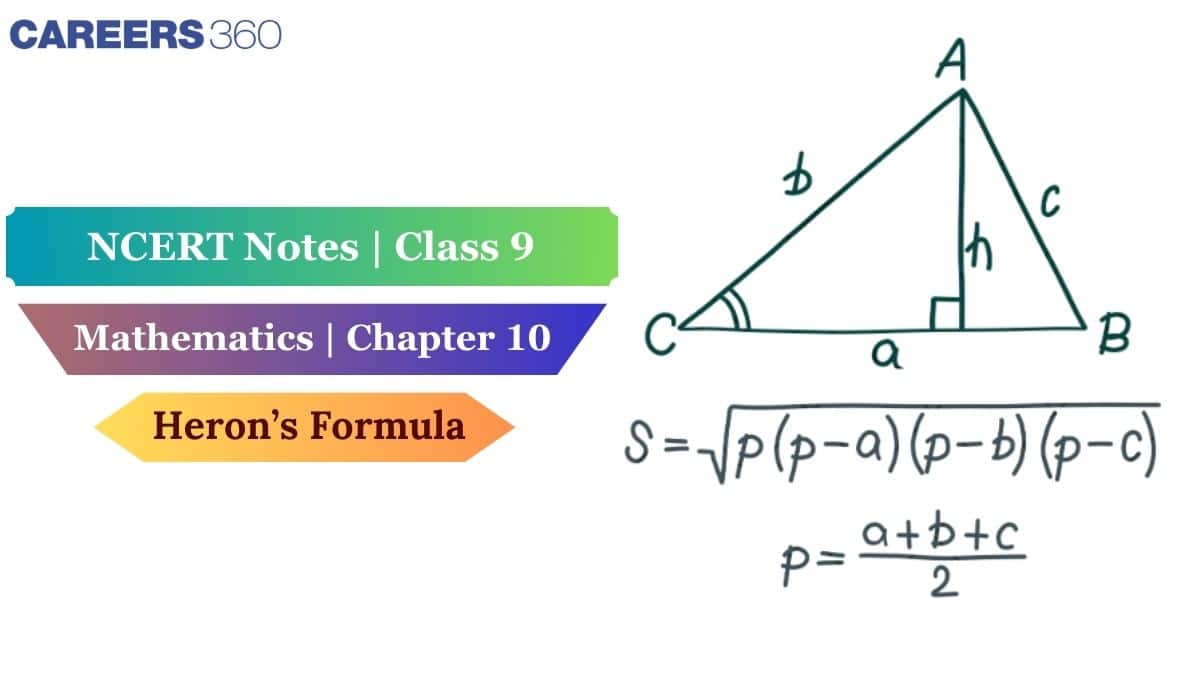
Area of a Triangle by Heron's Formula

Heron is a mathematician and an engineer in Alexandria in Egypt, he defined a formula to determine the area of a triangle when only all three sides are known. The Heron's formula for determining the area of a triangle is as follows:

Semiperimeter (S) = $\frac{(a + b + c)}{2}$

Where a, b, and c are the sides of the triangle.

Area of triangle = $\sqrt {s(s - a)(s - b)(s - c)}$

Example: Find the area of a triangle whose sides are 4 cm, 5 cm and 7cm respectively.

Semiperimeter (S) = $\frac{(a + b + c)}{2}$

Semiperimeter (S) = $\frac{(4 + 5 + 7)}{2}$

Semiperimeter (S) = $8$

Area of triangle = $\sqrt {8(8 - 4)(8 - 5)(8 - 7)}$

Area of triangle = $\sqrt {96}$$cm^2$

Class 9 Chapter Wise Notes

Students must download the notes below for each chapter to ace the topics.

NCERT Solutions for Class 9

Students must check the NCERT solutions for Class 10 Maths and Science given below:

• NCERT Solutions for Class 9 Maths
• NCERT Solutions for Class 9 Science

NCERT Exemplar Solutions for Class 9

Students must check the NCERT exemplar solutions for Class 10 Maths and Science given below:

• NCERT Exemplar Class 9 Maths Solutions
• NCERT Exemplar Class 9 Science Solutions

NCERT Books and Syllabus

To learn about the NCERT books and syllabus, read the following articles and get a direct link to download them.

• NCERT Syllabus for Class 9

• NCERT Books for Class 9