NCERT Solutions for Class 9 Maths Chapter 5 Introduction to Euclids Geometry

Class 9 maths chapter 5 question answer Excercise: 5.1

Q1 Which of the following statements are true and which are false? Give reasons for your answers.

(i) Only one line can pass through a single point.

(ii) There are an infinite number of lines which pass through two distinct points.

(iii) A terminated line can be produced indefinitely on both the sides.

(iv) If two circles are equal, then their radii are equal.

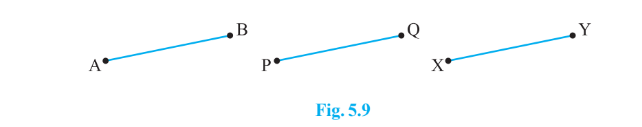
(v) In Fig. 5.9, if AB = PQ and PQ = XY, then AB = XY.

Answer:

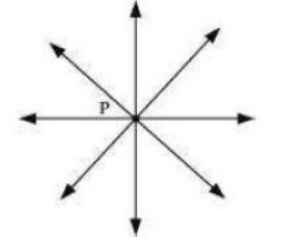
i) FALSE
Because there is the infinite number of lines that can be passed through a single point. As shown in the diagram below
ii) FALSE
Because only one line can pass through two distinct points. As shown in the diagram below
iii) TRUE
Because a terminated line can be produced indefinitely on both sides. As shown in the diagram below
iv) TRUE
Because if two circles are equal, then their centre and circumference will coincide and hence, the radii will also be equal.

v) TRUE
By Euclid’s first axiom things which are equal to the same thing, are equal to one another

Q2 (i) Give a definition for each of the following terms. Are there other terms that need to be defined first? What are they, and how might you define them?
(i) parallel lines

Answer:

Yes, there are other terms that are needed to be defined first which are:

Plane: A plane is a flat surface on which geometric figures are drawn.

Point: A point is a dimensionless dot which is drawn on a plane surface.

Line: A line is the collection of n number of points which can extend in both the directions and has only one dimension.
i) Parallel line:-
If the perpendicular distance between two lines is always constant and they never intersect with each other in a plane. Then, two lines are called parallel lines.

Q3 Consider two ‘postulates’ given below:

(i) Given any two distinct points A and B, there exists a third point C which is in between A and B.

(ii) There exist at least three points that are not on the same line.

Do these postulates contain any undefined terms? Are these postulates consistent?

Do they follow from Euclid’s postulates? Explain.

Answer:

There are various undefined terms in the given postulates.:

1) There is no information given about the plane whether the points are in the same plane or not.

2) There is the infinite number of points lie in a plane. But here the position of the point C has not specified whether it lies on the line segment joining AB or not.

Yes, these postulates are consistent when we deal with these two situations:

(i) Point C is lying in between and on the line segment joining A and B.

(ii) Point C does not lie on the line segment joining A and B.

No, they don’t follow from Euclid’s postulates. They follow the axioms.

Q4 If a point C lies between two points A and B such that AC = BC, then prove that AC = 1/2 AB. Explain by drawing the figure.

Answer:

It is given that
AC = BC
Now,
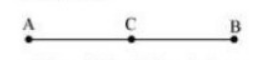
In the figure given above, AB coincides with AC + BC.
Also, Euclid’s Axiom (4) says that things which coincide with one another are equal to one another. So, it can be deduced that AC + BC = AB
Now,
2AC = AB
Therefore,

Hence proved.

Q5 In Question 4, point C is called a mid-point of line segment AB. Prove that every line segment has one and only one mid-point.

Answer:

Let's assume that there are two midpoints C and D
Now,
If C is the midpoint then, AC = BC
And
In the figure given above, AB coincides with AC + BC.
Also, Euclid’s Axiom (4) says that things which coincide with one another are equal to one another. So, it can be deduced that AC + BC = AB
From this, we can say that
2AC = AB -(i)

Similarly,
If D is the midpoint then, AD = BD
And
In the figure given above, AB coincides with AD + BD.
Also, Euclid’s Axiom (4) says that things which coincide with one another are equal to one another. So, it can be deduced that AD + BD = AB
From this, we can say that
2AD = AB -(ii)
Now,
From equation (i) and (ii) we will get
AD = AC
and this is only possible when C and D are the same points
Hence, our assumption is wrong and there is only one midpoint of line segment AB.

Q6 In Fig. 5.10, if AC = BD, then prove that AB = CD.

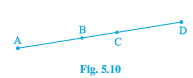
Answer:

From the figure given in the problem,
We can say that
AC = AB + BC and BD = BC + CD
Now,
It is given that AC = BD
Therefore,
AB + BC = BC + CD
Now, According to Euclid's axiom, when equals are subtracted from equals, the remainders are also equal. Subtracting BC from both sides.
We will get
AB + BC - BC = BC + CD - BC
AB = CD
Hence proved

Q7 Why is Axiom 5, in the list of Euclid’s axioms, considered a ‘universal truth’? (Note that the question is not about the fifth postulate.)

Answer:

Axiom 5 states that the whole is greater than the part.
Lets take A = x + y + z
where A , x , y , z all are positive numbers
Now, we can clearly see that A > x , A > y , A > z
Hence, by this we can say that the whole (A) is greater than the parts. (x , y , z)

Class 9 maths chapter 5 NCERT solutions Excercise: 5.2

Q1 How would you rewrite Euclid’s fifth postulate so that it would be easier to understand?

Answer:

Euclid's postulate 5: If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced indefinitely, meet on that side on which the sum of angles is less than two right angles.

Now, in an easy way
Let the line PQ in falls on lines AB and CD such that the sum of the interior angles 1 and 2 is less than 180° on the left side of PQ. Therefore, the lines AB and CD will eventually intersect on the left side of PQ.

Q2 Does Euclid’s fifth postulate imply the existence of parallel lines? Explain.

Answer: According to Euclid's 5 postulates, the line PQ falls on lines AB and CD such that the sum of the interior angles 1 and 2 is less than 180° on the left side of PQ. Therefore, the lines AB and CD will eventually intersect on the left side of the PQ
Now,
If then, the line never intersects with each other.
Therefore, we can say that lines AB and CD are parallel to each other