NCERT Solutions for Class 9 Maths Chapter 1 help students develop a clear understanding of number systems, including rational and irrational numbers. These step-by-step explanations make operations on real numbers and laws of exponents easy to apply. Regular practice with these solutions improves accuracy and conceptual clarity. Overall, they build a strong foundation for algebra and higher mathematics.
NCERT Solutions for Class 9 Maths Chapter 1 Number Systems
Imagine you return from the grocery store and see that you forgot to buy 3 out of 10 things your mother asked you to buy. Or, during the calculation of your savings at the end of the month, you wonder if the spending is greater than your pocket money. Or checking the current temperature on your phone. These are all examples of various forms of numbers like fractions, negative, positive, etc., and in Class 9 Maths NCERT chapter 1, students will get to know more about the number systems. In this chapter, we will study rational and irrational numbers, real numbers and the operations and laws of exponents of real numbers. These NCERT Solutions for Class 9 Maths provide a clear understanding of real numbers, helping in concept clarity and exam preparation.
This Story also Contains
- Number Systems Class 9 Questions And Answers PDF Free Download
- NCERT Solutions for Class 9 Maths Chapter 1 Number Systems: Exercise Questions
- Number Systems Class 9 NCERT Solutions: Exercise-wise
- Class 9 Maths NCERT Chapter 1 Solutions: Extra Questions
- Number Systems Class 9 Chapter 1: Topics
- Number Systems NCERT Solutions: Important Formulae
- Approach to Solve Questions of Number Systems Class 9
- Why are Class 9 Maths Chapter 1 Number Systems question answers important?
- NCERT Solutions for Class 9 Mathematics: Chapter-wise
- NCERT Books And NCERT Syllabus
Numbers are everywhere, and without them, our daily lives are incomplete. These NCERT Solutions for Class 9 are trustworthy and reliable, as they are created by subject matter experts at Careers360, making them an essential resource for exam preparation. Additionally, after analysing these solutions, students can practice various methods for converting decimals and applying exponents effectively. Many toppers rely on NCERT Solutions because they are designed in accordance with the latest syllabus. Find everything in one place – NCERT Books, Solutions, Syllabus, and Exemplar Problems with Solutions – in this NCERT article.
Also, read,
Number Systems Class 9 Questions And Answers PDF Free Download
These NCERT Solutions for Class 9 Maths Chapter 1 Number Systems have been prepared by Careers360 experts to make learning simpler and to help you score better in exams. A downloadable PDF has been provided — click on the link below to access it.
NCERT Solutions for Class 9 Maths Chapter 1 Number Systems: Exercise Questions
NCERT Class 9 Maths Chapter 1 Number Systems question answers with detailed explanations are provided below.
|
Number Systems Class 9 Question Answers
Exercise: 1.1 Total Questions: 4
Page number: 5
|
Question 1: Is zero a rational number? Can you write it in the form $\frac{p}{q}$, where p and q are integers and q ≠ 0?
Answer:
Any number that can represent in the form of $\frac{p}{q}$
$(where \ q \neq 0)$ is a rational number
Now, we can write 0 in the form of $\frac{p}{q}$
for eg. $\frac{0}{1},\frac{0}{2},\frac{0}{-1}$ etc.
Therefore, 0 is a rational number.
Question 2: Find six rational numbers between 3 and 4.
Answer:
There is an infinite number of rational numbers between 3 and 4. One way to take them is$\Rightarrow 3 = \frac{21}{7} $ and $ 4 = \frac{28}{7}$
Therefore, six rational numbers between 3 and 4 are $\frac{22}{7}, \frac{23}{7},\frac{24}{7},\frac{25}{7},\frac{26}{7},\frac{27}{7}.$
Question 3: Find five rational numbers between $\frac{3}{5}$ and $\frac{4}{5}$ .
Answer:
We can write
$\Rightarrow \frac{3}{5}= \frac{3\times 6}{5\times 6} = \frac{18}{30}$
And
$\Rightarrow \frac{4}{5}= \frac{4\times 6}{5\times 6} = \frac{24}{30}$
Therefore, five rational numbers between $\frac{3}{5}$ and $\frac{4}{5}$ are $\frac{19}{30},\frac{20}{30},\frac{21}{30},\frac{22}{30},\frac{23}{30}.$
Question 4: (i) State whether the following statements are true or false. Give reasons for your answers.
(i)Every natural number is a whole number.
Answer:
(i) TRUE
The numbers that start from 1, i.e 1, 2, 3, 4, 5, 6, .................. are natural numbers.
The numbers that start from 0, i.e, 0, 1, 2, 3, 4, 5,..., are whole numbers.
Therefore, we can clearly see that the collection of whole numbers contains all natural numbers.
Question 4: (i) State whether the following statements are true or false. Give reasons for your answers.
(ii) Every integer is a whole number.
Answer:
(ii) FALSE
Integers may be negative or positive, but whole numbers are always positive.
For example, -1 is an integer but not a whole number.
Question 4: (i) State whether the following statements are true or false. Give reasons for your answers.
(iii) Every rational number is a whole number.
Answer:
(iii) FALSE
Numbers that can be represented in the form of $\frac{p}{q}$
(where $q \neq 0)$ are a rational number.
And numbers that start from 0, i.e., 0,1,2,3,4,......... are whole numbers.
Therefore, we can clearly see that every rational number is not a whole number.
for eg. $\frac{3}{4}$ is a rational number but not a whole number
|
Number Systems Class 9 Question Answers
Exercise: 1.2 Total Questions: 3
Page number: 8
|
Question 1: (i) State whether the following statements are true or false. Justify your answers.
(i) Every irrational number is a real number.
Answer:
(i) TRUE
Since the real numbers are the collection of all rational and irrational numbers.
Answer:
(ii) FALSE
Because negative numbers are also present on the number line, and no negative number can be the square root of any natural number
Question 1: (i) State whether the following statements are true or false. Justify your answers.
(iii) Every real number is an irrational number.
Answer:
(iii) FALSE
Real numbers include both rational and irrational numbers. Therefore, every real number is not an irrational number.
For example, 4 is a real number but not an irrational number.
Answer:
NO, the Square root of all positive integers is not irrational. For example, the square of 4 is 2, which is a rational number.
Question 3: Show how $\sqrt{5}$ can be represented on the number line.
Answer:
We know that
$\sqrt{5} = \sqrt{(2)^2+(1)^2}$
Now,
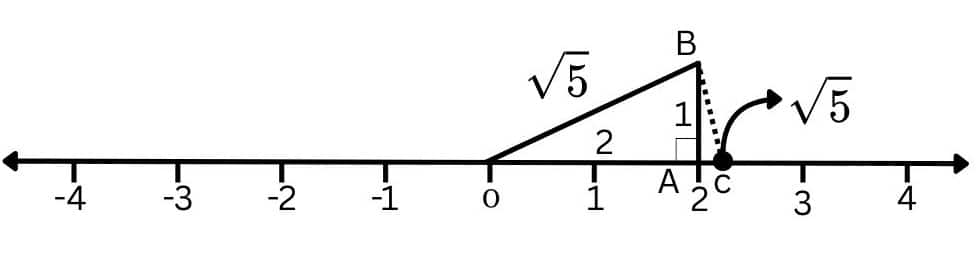
Let OA be a line of length 2 units on the number line.
Now, construct AB of unit length perpendicular to OA, and join OB.
Now, in right-angle triangle OAB, by Pythagoras' theorem
$OB = \sqrt{(2)^2+(1)^2}=\sqrt{5}$
Now, take O as the centre and OB as the radius, and draw an arc intersecting the number line at C.
Point C represents $\sqrt{5}$ on a number line.
|
Number Systems Class 9 Question Answers
Exercise: 1.3 Total Questions: 9
Page number: 14
|
Question 1: (i) Write the following in decimal form and say what kind of decimal expansion each has : (i) $\frac{36}{100}$
Answer:
We can write $\frac{36}{100}$ as
$\Rightarrow \frac{36}{100}= 0.36$
Since the decimal expansion ends after a finite number of steps. Hence, it is terminating
Question 1: (ii) Write the following in decimal form and say what kind of decimal expansion each has : (ii) $\frac{1}{11}$
Answer:
We can rewrite $\frac{1}{11}$ as
$\Rightarrow \frac{1}{11}= 0.09090909..... = 0.\overline{09}$
Since decimal expansion repeats itself,
So, it is a non-terminating recurring decimal expansion.
Question 1: (iii) Write the following in decimal form and say what kind of decimal expansion each has : (iii) $4\frac{1}{8}$
Answer:
We can rewrite $4\frac{1}{8}$ as
$\Rightarrow 4\frac{1}{8} = \frac{33}{8}= 4.125$
Since the decimal expansion ends after a finite number.
Therefore, it is terminating.
Question 1: (iv) Write the following in decimal form and say what kind of decimal expansion each has: (iv) $\frac{3}{13}$
Answer:
We can rewrite $\frac{3}{13}$ as
$\Rightarrow \frac{3}{13} = 0.230769230769 = 0.\overline{230769}$
Since decimal expansion repeats itself,
So, it is a non-terminating recurring decimal expansion.
Question 1: (v) Write the following in decimal form and say what kind of decimal expansion each has: (v) $\frac{2}{11}$
Answer:
We can rewrite $\frac{2}{11}$ as
$\Rightarrow \frac{2}{11} = 0.181818......= 0.\overline{18}$
Since decimal expansion repeats itself, it is a non-terminating recurring decimal expansion.
Question 1: (vi) Write the following in decimal form and say what kind of decimal expansion each has : (vi) $\frac{329}{400}$
Answer:
We can rewrite $\frac{329}{400}$ as
$\Rightarrow \frac{329}{400}= 0.8225$
Since decimal expansion ends after a finite no. of figures.
Hence, it is terminating.
Answer:
It is given that $\frac{1}{7}=0.\overline{142857}$
Therefore,
$\Rightarrow \frac{2}{7} = 2\times \frac{1}{7} = 2 \times 0.\overline{142857}= 0.\overline{285714}$
Similarly,
$\Rightarrow \frac{3}{7} = 3\times \frac{1}{7} = 3 \times 0.\overline{142857}= 0.\overline{428571}$
$\Rightarrow \frac{4}{7} = 4\times \frac{1}{7} = 4 \times 0.\overline{142857}= 0.\overline{571428}$
$\Rightarrow \frac{5}{7} = 5\times \frac{1}{7} = 5 \times 0.\overline{142857}= 0.\overline{714285}$
$\Rightarrow \frac{6}{7} = 6\times \frac{1}{7} = 6 \times 0.\overline{142857}= 0.\overline{857142}$
Question 3: (i) Express the following in the form $\frac{p}{q}$, where p and q are integers and q ≠ 0. (i) $0.\bar{6}$
Answer:
Let $x = 0.\overline6= 0.6666....$ -(i)
Now, multiply by 10 on both sides,
$10x= 6.6666...$
$\Rightarrow 10x = 6 + x$ (using (i))
$\Rightarrow 9x = 6$
$\Rightarrow x = \frac{6}{9} = \frac{2}{3}$
Therefore, $\frac{p}{q}$ form of $0.\bar{6}$ is $\frac{2}{3}$.
Question 3: (ii) Express the following in the form $\frac{p}{q}$, where p and q are integers and q ≠ 0. (ii) $0.4\bar{7}$
Answer:
We can write $0.4\overline7$ as
$\Rightarrow 0.4\overline7 = \frac{4}{10}+ \frac{0.777..}{10}$ -(i)
Now,
Let $x = 0.\overline7= 0.7777....$ -(ii)
Now, multiply by 10 on both sides,
$10x= 7.7777...$
$\Rightarrow 10x = 7 + x$ (using (ii))
$\Rightarrow 9x = 7$
$\Rightarrow x = \frac{7}{9}$
Now, putting the value of x in equation (i), we will get,
$\Rightarrow 0.4\overline7 = \frac{4}{10}+ \frac{7}{10\times 9}= \frac{4}{10}+ \frac{7}{90} = \frac{36+7}{90} = \frac{43}{90}$
Therefore, $\frac{p}{q}$ form of $0.4\overline7$ is $\frac{43}{90}$
Question 3: (iii) Express the following in the form $\frac{p}{q}$, where p and q are integers and q ≠ 0. (iii) $0.\overline{001}$
Answer:
Let $x = 0.\overline{001}= 0.001001....$ -(i)
Now, multiply by 1000 on both sides.
$1000x= 1.001001...$
$\Rightarrow 1000x = 1 + x $ (using (i))
$\Rightarrow 999x = 1$
$\Rightarrow x = \frac{1}{999}$
Therefore, $\frac{p}{q}$ form of $0.\overline{001}$ is $\frac{1}{999}$
Question 4: Express 0.99999 .... in the form $\frac{p}{q}$. Are you surprised by your answer?
Answer:
Let $x = 0.\overline{9}= 0.9999....$ -(i)
Now, multiply by 10 on both sides.
$10x= 9.999....$
$\Rightarrow 10x = 9 + x$ (using (i))
$\Rightarrow 9x = 9$
$\Rightarrow x = \frac{9}{9} = 1$
Therefore, $\frac{p}{q}$ form of $0.999....$ is 1
The difference between 1 and 0.999999 is 0.000001, which is almost negligible.
Therefore, 0.999 is much closer to 1. Hence, we can write 0.999999.... as 1
Answer:
We can rewrite $\frac{1}{17}$ as
$\Rightarrow \frac{1}{17} = 0.05882352941176470588235294117647= 0.\overline{0588235294117647}$
Therefore, there are a total 16 number of digits in the repeating block of digits in the decimal expansion of $\frac{1}{17}$
Answer:
We can observe that when q is 2, 4, 5, 8, 10…
Then the decimal expansion is terminating. For example:
$\frac{3}{2}= 1.5$ , denominator $q = 2^1$
$\frac{8}{5}= 1.6$ , denominator $q = 5^1$
$\frac{15}{10} = 1.5$ , denominator $q =10=2\times 5= 2^1 , 5^1$
Therefore,
It can be observed that the terminating decimal can be obtained in a condition where prime factorisation of the denominator of the given fractions has the power of 2 only, 5 only, or both.
Question 7: Write three numbers whose decimal expansions are non-terminating and non-recurring.
Answer:
Three numbers whose decimal expansions are non-terminating non-recurring are
1) 0.02002000200002......
2) 0.15115111511115.......
3) 0.27227222722227.......
Question 8: Find three different irrational numbers between the rational numbers $\frac{5}{7}$ and $\frac{9}{11}$.
Answer:
We can write $\frac{5}{7}$ as
$\Rightarrow \frac{5}{7} = 0.714285714285.... = 0.\overline{714285}$
And $\frac{9}{11}$ as
$\Rightarrow \frac{9}{11} = 0.818181.... = 0.\overline{81}$
Therefore, three different irrational numbers between the rational numbers $\frac{5}{7}$ and $\frac{9}{11}$ are
1) 0.72737475....
2) 0.750760770780...
3) 0.790780770760....
Question 9: (i) Classify the following numbers as rational or irrational: $\sqrt{23}$
Answer:
We can rewrite $\sqrt{23}$ in decimal form as
$\Rightarrow \sqrt{23} = 4.7958152....$
Now, as the decimal expansion of this number is non-terminating and non-recurring.
Therefore, it is an irrational number.
Question 9: (ii) Classify the following numbers as rational or irrational: $\sqrt{225}$
Answer:
We can rewrite $\sqrt{225}$ as
$\Rightarrow \sqrt{225} = 15$
We can clearly see that it is a rational number because we can represent it in $\frac{p}{q}$ form
Question 9: (iii) Classify the following numbers as rational or irrational: 0.3796
Answer:
We can rewrite 0.3796 as
$\Rightarrow 0.3796 = \frac{3796}{10000}$
Now, we can clearly see that it is a rational number as the decimal expansion of this number is terminating, and we can also write it in $\frac{p}{q}$ form.
Question 9: (iv) Classify the following numbers as rational or irrational: 7.478478....
Answer:
We can rewrite 7.478478.... as
$\Rightarrow 7.478478.... = 7.\overline{478}$
Now, as the decimal expansion of this number is non-terminating and recurring. Therefore, it is a rational number.
Question 9: (v) Classify the following numbers as rational or irrational: 1.101001000100001...
Answer:
In the case of number 1.101001000100001...
As the decimal expansion of this number is non-terminating and non-repeating.
Therefore, it is an irrational number.
|
Number Systems Class 9 Question Answers
Exercise: 1.4 Total Questions: 5
Page number: 20-21
|
Question 1: (i) Classify the following numbers as rational or irrational: $2-\sqrt{5}$
Answer:
Value of $\sqrt{5}$ is 2.23606798....
Now,
$\Rightarrow 2 - \sqrt{5} = 2 - 2.23606798... = -0.23606798...$
Now,
Since the number is non-terminating and non-recurring.
Therefore, it is an irrational number.
Question 1: (ii) Classify the following numbers as rational or irrational: $\left ( 3+\sqrt{23} \right )-\sqrt{23}$
Answer:
Given number is $\left ( 3+\sqrt{23} \right )-\sqrt{23}$
$\Rightarrow \left ( 3+\sqrt{23} \right )-\sqrt{23} = 3+\sqrt{23}-\sqrt{23} = 3$
Now, it is clearly a rational number because we can represent it in the form of $\frac{p}{q}$
Question 1: (iii) Classify the following numbers as rational or irrational: $\frac{2\sqrt{7}}{7\sqrt{7}}$
Answer:
Given number is $\frac{2\sqrt{7}}{7\sqrt{7}}$
$\Rightarrow \frac{2\sqrt{7}}{7\sqrt{7}} = \frac{2}{7}$
As we can clearly see, it can be represented in $\frac{p}{q}$ form. Therefore, it is a rational number.
Question 1: (iv) Classify the following numbers as rational or irrational: $\frac{1}{\sqrt{2}}$
Answer:
Given number is $\frac{1}{\sqrt{2}}$
$\Rightarrow \frac{1}{\sqrt{2}}= \frac{\sqrt{2}}{2}=\frac{1.41421356..}{2} =0.7071068...$
Now,
The decimal expansion of this expression is non-terminating and non-recurring.
Therefore, it is an irrational number.
Question 1: (v) Classify the following numbers as rational or irrational: $2\pi$
Answer:
Given number is $2\pi$
We know that the value of $\pi = 3.14159265...$
Now,
$\Rightarrow 2\pi = 2\times 3.14159265... = 6.2831853...$
Now,
The decimal expansion of this expression is non-terminating and non-recurring.
Therefore, it is an irrational number.
Question 2: (i) Simplify each of the following expressions: $(3+\sqrt{3})(2+\sqrt{2})$
Answer:
Given number is $(3+\sqrt{3})(2+\sqrt{2})$
Now, we will reduce it to
$\Rightarrow (3+\sqrt{3})(2+\sqrt{2})$
$= 3.2+3.\sqrt{2}+\sqrt{3}.2+\sqrt{3}.\sqrt{2}$
$= 6+3\sqrt{2}+2\sqrt{3}+\sqrt{6}$
Therefore, answer is $6+3\sqrt{2}+2\sqrt{3}+\sqrt{6}$
Question 2: (ii) Simplify each of the following expressions: $\left (3+\sqrt{3} \right )\left ( 3-\sqrt{3} \right )$
Answer:
The given number is
$\left (3+\sqrt{3} \right )\left ( 3-\sqrt{3} \right )$
Now, we will reduce it to
$\Rightarrow (3+\sqrt{3})(3-\sqrt{3})$
$= \left ( (3)^2-(\sqrt{3})^2 \right )$ Using $\left ( \ (a+b)(a-b)=a^2-b^2 \right )$
$=9 - 3 = 6$
Therefore, the answer is 6.
Question 2: (iii) Simplify each of the following expressions: $\left (\sqrt{5}+\sqrt{2} \right )^{2}$
Answer:
Given number is $\left (\sqrt{5}+\sqrt{2} \right )^{2}$
Now, we will reduce it into$\Rightarrow \left (\sqrt{5}+\sqrt{2} \right )^{2}$
$= \left ( (\sqrt{5})^2+(\sqrt{2})^2+2.\sqrt{5}.\sqrt{2} \right )$ Uisng $\left (\ (a+b)^2=a^2+b^2 +2ab\right )$
$=5+2+2\sqrt{10}$
$=7+2\sqrt{10}$
Therefore, the answer is $7+2\sqrt{10}$
Question 2: (iv) Simplify each of the following expressions: $\left (\sqrt{5}-\sqrt{2} \right )\left ( \sqrt{5}+\sqrt{2} \right )$
Answer:
Given number is $\left (\sqrt{5}-\sqrt{2} \right )\left ( \sqrt{5}+\sqrt{2} \right )$
Now, we will reduce it to
$= \left (\sqrt{5}-\sqrt{2} \right )\left ( \sqrt{5}+\sqrt{2} \right )$
$= \left ( (\sqrt{5})^2-(\sqrt{2})^2 \right )$ Using $\left ( \ (a+b)(a-b)=a^2-b^2\right )$
$=5-2$
$=3$
Therefore, the answer is 3.
Answer:
There is no contradiction.
When we measure a length with a scale or any other instrument, we only obtain an approximate rational value.
We never obtain an exact value.
For this reason, we cannot say that either c or d is irrational.
Therefore, the fraction $\frac{c}{d}$ is irrational.
Hence, the value of $\pi$ is approximately equal to $\frac{22}{7} = 3.142857....$
Therefore, $\pi$ is irrational.
Question 4: Represent $\sqrt{9.3}$ on the number line.
Answer:
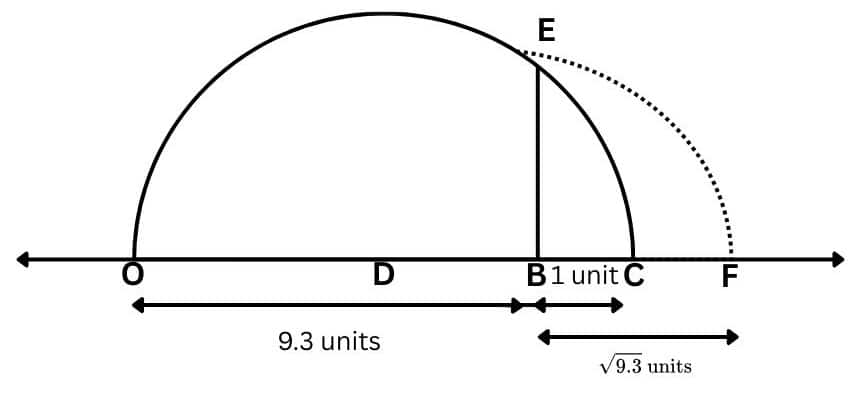
Draw a line segment OB of 9.3 units.
Then, extend it to C so that BC = 1 unit.
Find the mid-point D of OC and draw a semicircle on OC while taking D as its centre and OD as the radius.
Now, draw a perpendicular to line OC passing through point B and intersecting the semi-circle at E.
Now, take B as the centre and BE as the radius,
draw an arc intersecting the number line at F.
The length BF is $9\sqrt{3}$ units.
Question 5: (i) Rationalise the denominators of the following: $\frac{1}{\sqrt{7}}$
Answer:
Given number is $\frac{1}{\sqrt{7}}$
Now, on rationalisation, we will get,
$\Rightarrow \frac{1}{\sqrt 7} = \frac{1}{\sqrt 7}\times \frac{\sqrt 7}{\sqrt 7 } = \frac{\sqrt7}{7}$
Therefore, the answer is $\frac{\sqrt7}{7}$.
Question 5: (ii) Rationalise the denominators of the following: $\frac{1}{\sqrt{7}-\sqrt{6}}$
Answer:
Given number is $\frac{1}{\sqrt{7}-\sqrt{6}}$
Now, on rationalisation, we will get
$= \frac{1}{\sqrt 7-\sqrt6}$
$ = \frac{1}{\sqrt 7-\sqrt 6}\times \frac{\sqrt 7+\sqrt 6 }{\sqrt 7+\sqrt 6 }$
$ = \frac{\sqrt7+\sqrt 6}{(\sqrt7)^2-(\sqrt6)^2} $
$= \frac{\sqrt7+\sqrt6}{7-6}$
$= \sqrt7+\sqrt6$
Therefore, the answer is $\sqrt7+\sqrt6$.
Question 5: (iii) Rationalise the denominators of the following: $\frac{1}{\sqrt{5}+\sqrt{2}}$
Answer:
Given number is $\frac{1}{\sqrt{5}+\sqrt{2}}$
Now, on rationalisation, we will get
$= \frac{1}{\sqrt 5+\sqrt2}$
$= \frac{1}{\sqrt 5+\sqrt 2}\times \frac{\sqrt 5-\sqrt 2 }{\sqrt 5-\sqrt 2 }$
$ = \frac{\sqrt5-\sqrt 2}{(\sqrt5)^2-(\sqrt2)^2} $
$= \frac{\sqrt5-\sqrt2}{5-2}$
$= \frac{\sqrt5-\sqrt2}{3}$
Therefore, the answer is $\frac{\sqrt5-\sqrt2}{3}$
Question 5: (iv) Rationalise the denominators of the following: $\frac{1}{\sqrt{7}-2}$
Answer:
Given number is $\frac{1}{\sqrt{7}-2}$
Now, on rationalisation, we will get
$= \frac{1}{\sqrt 7-2}$
$= \frac{1}{\sqrt 7- 2}\times \frac{\sqrt 7+ 2 }{\sqrt 7+ 2 } $
$= \frac{\sqrt7+ 2}{(\sqrt7)^2-(2)^2} $
$= \frac{\sqrt7+2}{7-4}$
$= \frac{\sqrt7+2}{3}$
Therefore, answer is $\frac{\sqrt7+2}{3}$
|
Number Systems Class 9 Question Answers
Exercise: 1.5 Total Questions: 3
Page number: 23
|
Question 1: (i) Find : $64^{\frac{1}{2}}$
Answer:
Given number is $64^{\frac{1}{2}}$
Now, on simplifying it, we will get,
$\Rightarrow 64^{\frac{1}{2}} = (8^2)^\frac{1}{2} = 8$
Therefore, the answer is 8.
Question 1: (ii) Find : $32^{\frac{1}{5}}$
Answer:
Given number is $32^{\frac{1}{5}}$
Now, on simplifying it, we will get,
$\Rightarrow 32^{\frac{1}{5}} = (2^5)^\frac{1}{5} = 2$
Therefore, the answer is 2.
Question 1: (iii) Find : $125^{\frac{1}{3}}$
Answer:
Given number is $125^{\frac{1}{3}}$
Now, on simplifying it, we will get,
$\Rightarrow 125^{\frac{1}{3}} = (5^3)^\frac{1}{3} = 5$
Therefore, the answer is 5.
Question 2: (i) Find : $9^{\frac{3}{2}}$
Answer:
Given number is $9^{\frac{3}{2}}$
Now, on simplifying it, we will get,
$\Rightarrow 9^{\frac{3}{2}} = (3^2)^\frac{3}{2} = 3^3 = 27$
Therefore, the answer is 27.
Question 2: (ii) Find : $32^{\frac{2}{5}}$
Answer:
Given number is $32^{\frac{2}{5}}$
Now, on simplifying it, we will get,
$\Rightarrow 32^{\frac{2}{5}} = (2^5)^\frac{2}{5} = 2^2 = 4$
Therefore, the answer is 4.
Question 2: (iii) Find : $16^{\frac{3}{4}}$
Answer:
Given number is $16^{\frac{3}{4}}$
Now, on simplifying it, we will get,
$\Rightarrow 16^{\frac{3}{4}} = (2^4)^\frac{3}{4} = 2^3 = 8$
Therefore, the answer is 8.
Question 2: (iv) Find : $125^{\frac{-1}{3}}$
Answer:
Given number is $125^{\frac{-1}{3}}$
Now, on simplifying it, we will get
$\Rightarrow 125^{\frac{-1}{3}} = (5^3)^\frac{-1}{3} = 5^{-1} = \frac{1}{5}$
Therefore, the answer is $\frac{1}{5}$.
Question 3: (i) Simplify : $2^{\frac{2}{3}}.2^{\frac{1}{5}}$
Answer:
Given number is $2^{\frac{2}{3}}.2^{\frac{1}{5}}$
Now, on simplifying it, we will get,
$\Rightarrow 2^{\frac{2}{3}}.2^{\frac{1}{5}} = 2^{\frac{2}{3}+\frac{1}{5}} = 2^{\frac{10+3}{15}} = 2^\frac{13}{15}$
$\left ( \because a^n.a^m = a^{n+m} \right )$
Therefore, the answer is $2^{\frac{13}{15}}$.
Question 3: (ii) Simplify : $\left (\frac{1}{3^{3}} \right )^{7}$
Answer:
Given number is $\left (\frac{1}{3^{3}} \right )^{7}$
Now, on simplifying it, we will get,
$\Rightarrow \left ( \frac{1}{3^3} \right )^7= \frac{1^7}{3^{3\times7}} = \frac{1}{3^{21}} = 3^{-21}$
$\left ( \because (a^n)^m = a^{n.m} \ and \ \frac{1}{a^m}= a^{-m}\right )$
Therefore, the answer is $3^{-21}$.
Question 3: (iii) Simplify : $\frac{11^{\frac{1}{2}}}{11^{\frac{1}{4}}}$
Answer:
Given number is $\frac{11^{\frac{1}{2}}}{11^{\frac{1}{4}}}$
Now, on simplifying it, we will get,
$\Rightarrow \frac{11^{\frac{1}{2}}}{11^{\frac{1}{4}}} = 11^{\frac{1}{2}-\frac{1}{4}}= 11^{\frac{2-1}{4}} = 11^\frac{1}{4}$
$\left ( \because \frac{a^n}{a^m}= a^{n-m}\right )$
Therefore, the answer is $11^{\frac{1}{4}}$.
Question 3: (iv) Simplify : $7^{\frac{1}{2}}.8^{\frac{1}{2}}$
Answer:
Given number is $7^{\frac{1}{2}}.8^{\frac{1}{2}}$
Now, on simplifying it, we will get,
$\Rightarrow 7^{\frac{1}{2}}.8^{\frac{1}{2}}= (7\times8)^{\frac{1}{2}} = 56^{\frac{1}{2}}$
$\left ( \because a^n.b^n=(a.b)^n\right )$
Therefore, the answer is $56^{\frac{1}{2}}$.
Number Systems Class 9 NCERT Solutions: Exercise-wise
Exercise-wise NCERT Solutions of Number Systems Class 9 Maths Chapter 1 are provided in the links below.
Class 9 Maths NCERT Chapter 1 Solutions: Extra Questions
Question 1: Simplify: $ \left(\frac{5}{2}\right)^3 \times \left(\frac{2}{5}\right)^2 $
Answer:
$\left(\frac{5}{2}\right)^3 = \frac{5^3}{2^3} = \frac{125}{8}, \quad \left(\frac{2}{5}\right)^2 = \frac{2^2}{5^2} = \frac{4}{25}$
$\frac{125}{8} \times \frac{4}{25} = \frac{125 \times 4}{8 \times 25} = \frac{500}{200} = \frac{5}{2}$
Question 2:
What is the value of x?
If x = 0.05 × 0.36 ÷ 0.4 + 0.055 + 1.50 ÷ 0.03
Answer:
x = 0.05 × 0.36 ÷ 0.4 + 0.055 + 1.50 ÷ 0.03
⇒ x = 0.05 × 0.9 + 0.055 + 50
⇒ x = 0.045 + 0.055 + 50
⇒ x = 50.1
Hence, the correct answer is 50.1.
Question 3:
What is the value of $\frac{\frac{5}{3} \text { of } \frac{1}{4} \times \frac{24}{5}-\frac{1}{5} \text { of } \frac{25}{8} \times \frac{24}{5}}{\frac{5}{12} \text { of } \frac{3}{4} \times \frac{4}{3}+\frac{3}{4} \text { of } \frac{12}{5} \times \frac{5}{6}}$?
Answer:
$\frac{\frac{5}{3} \text { of } \frac{1}{4} \times \frac{24}{5}-\frac{1}{5} \text { of } \frac{25}{8} \times \frac{24}{5}}{\frac{5}{12} \text { of } \frac{3}{4} \times \frac{4}{3}+\frac{3}{4} \text { of } \frac{12}{5} \times \frac{5}{6}}$
$=\frac{\frac{5}{12}\times\frac{24}{5}-\frac{25}{40}\times\frac{24}{5}}{\frac{15}{48}\times\frac{4}{3}+\frac{36}{20}\times \frac{5}{6}}$
$=\frac{2-3}{\frac{5}{12}+\frac{3}{2}}$
$=\frac{-1}{\frac{5+18}{12}}$
$=\frac{-12}{23}$
Hence, the correct answer is $\frac{-12}{23}$.
Question 4:
The greatest number among the following
$\frac{4}{9},\sqrt{\frac{9}{49}},0.\overline{47},(0.7)^{2}$ is:
Answer:
$\frac{4}{9} = 0.44$
$\sqrt{\frac{9}{49}} = \frac{3}{7} = 0.42$
$0.\overline{47} = 0.4747........$
$(0.7)^{2} = 0.49$
So, the largest number among these is $(0.7)^{2}$.
Hence, the correct answer is $(0.7)^{2}$.
Question 5:
The simplified value of $(0.2)^{3}\times 200\div 2000$ of $(0.2)^{2}$ is:
Answer:
$(0.2)^{3}\times 200\div 2000$ of $(0.2)^{2}$
$=0.008\times200\div 2000\times0.04$
$=0.008\times200\div 80$
$=0.008\times200\times \frac{1}{80}$
$=2\times \frac{1}{100}$
$=\frac{1}{50}$
Hence, the correct answer is $\frac{1}{50}$.
Number Systems Class 9 Chapter 1: Topics
The topics discussed in the NCERT Solutions for class 9 Maths chapter 1 Number Systems are:
- Introduction
- Irrational Numbers
- Real Numbers and Their Decimal Expansions
- Operations on Real Numbers
- Laws of Exponents for Real Numbers
Number Systems NCERT Solutions: Important Formulae
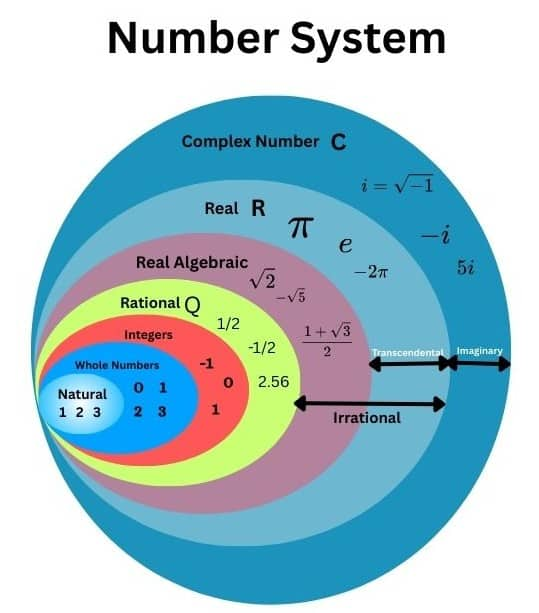
Any unique real number can be represented on a number line.
If r = rational number and s = irrational number
Then (r + s), (r – s), (r × s), and ($\frac rs$) are all irrational.
Rules for positive real numbers:
- (i) $\sqrt{a b}=\sqrt{a} \sqrt{b}$
- (ii) $\sqrt{\frac{a}{b}}=\frac{\sqrt{a}}{\sqrt{b}}$
- (iii) $(\sqrt{a}+\sqrt{b})(\sqrt{a}-\sqrt{b})=a-b$
- (iv) $(a+\sqrt{b})(a-\sqrt{b})=a^2-b$
- (v) $(\sqrt{a}+\sqrt{b})^2=a+2 \sqrt{a b}+b$
To rationalise the denominator of $\frac{1}{\sqrt{a}+b}$, we multiply this by $\frac{\sqrt{a}-b}{\sqrt{a}-b}$, where $a$ and $b$ are integers.
Let $a>0$ be a real number and $p$ and $q$ be rational numbers. Then,
(i) $a^p \cdot a^q=a^{p+q}$
(ii) $\left(a^p\right)^q=a^{p q}$
(iii) $\frac{a^p}{a^q}=a^{p-q}$
(iv) $a^p b^p=(a b)^p$
Approach to Solve Questions of Number Systems Class 9
Given below are useful strategies to access and use the Number Systems Class 9 NCERT Solutions effectively:
- Understand types of numbers: The study should begin with natural numbers and whole numbers, followed by integers, rational numbers, and irrational numbers, to construct knowledge for advanced learning.
- Learn how to represent numbers on the number line: Using number lines for visually showing rational numbers together with irrational numbers teaches students how real numbers function with density and continuous distribution.
- Work on rational and irrational number properties: Students should familiarise themselves with the methods to identify numbers as rational or irrational entities while understanding operational behaviours.
- Use laws of exponents for real numbers: Efficient number simplification occurs through applying exponent laws when working with rational and irrational numbers during expression solutions.
- Practice converting decimals to fractions: Students should demonstrate total mastery in converting recurring and terminating decimals into rational form because this skill appears in both school tests and competitive examinations.
- Apply real number operations: Practice numerous examples, including real number addition, subtraction, multiplication and division, until fully mastering number operational skills.
Why are Class 9 Maths Chapter 1 Number Systems question answers important?
Numbers are the base of all maths we learn. This chapter helps us understand how different types of numbers work together to form the number systems we use every day. These Class 9 Maths Chapter 1 Number Systems question answers help students build this basic understanding in a clear way. Here are some more points about why these question answers are important:
- These solutions help you understand real and irrational numbers, which are used in many important maths topics.
- These question answers make it easy to learn how to do operations like addition, subtraction, and exponents with real numbers.
- These Class 9 Maths Chapter 1 Number Systems question answers prepare you for higher classes where you will study algebra, roots, and complex numbers.
NCERT Solutions for Class 9 Mathematics: Chapter-wise
We at Careers360 compiled all the NCERT class 9 Maths solutions in one place for easy student reference. The following links will allow you to access them.
NCERT Books And NCERT Syllabus
The following links will help students find some additional reference books and the latest NCERT syllabus.
- NCERT Books Class 9 Maths
- NCERT Syllabus Class 9 Maths
- NCERT Books Class 9
- NCERT Books for Class 9 Science
- NCERT Syllabus Class 9
Frequently Asked Questions (FAQs)
Q: How can NCERT Class 9 Maths Chapter 1 Number Systems solutions help in competitive exams?
A:
Number systems are an important part of mathematics, and this chapter forms the foundation of higher mathematics. Practising these NCERT Solutions helps in building concepts that are useful for exams like NTSE, Olympiads, and even JEE foundation.
Q: How many exercises are there in Chapter 1 Number System?
A:
There are 5 exercises given in the NCERT textbook for this chapter. Students can also refer to the NCERT exemplar questions after completing the NCERT textbook for further practice purposes.
Q: Does CBSE provides the solutions of NCERT textbook for Class 9 Maths?
A:
No, CBSE doesn’t provide NCERT solutions for any class or subject. But many trusted educational websites, such as Careers360, provide the solutions to the NCERT textbook for Class 9 Maths.
Q: Where can I find the complete solutions of NCERT textbook for Class 9 Maths ?
A:
Here, students can get detailed NCERT solutions for Class 9 Maths on the links given in this article, or they can check the official website of Careers360. Practising these Number Systems Class 9 NCERT solutions thoroughly is important as these provide confidence to students, which ultimately leads to a high score.
Q: How does the NCERT solutions are helpful ?
A:
NCERT solutions are helpful for students if they get stuck while solving NCERT problems. Also, these solutions are provided in a very detailed manner, which will give them conceptual clarity.
Q: What are irrational numbers?
A:
Irrational numbers cannot be written as a fraction of two integers. Their decimal form is non-terminating and non-repeating.
Examples: √2, π, √3.
Q: Can the square root of any integer be irrational?
A:
Not always. The square root of a perfect square, like √9 = 3, is rational, but the square root of a non-perfect square, like √2, is irrational.
Q: How can we tell if a number is rational or irrational by its decimal expansion?
A:
Rational numbers have terminating or repeating decimals.
Irrational numbers have non-terminating, non-repeating decimals.
Articles
Upcoming School Exams
Popular Questions
A block of mass 0.50 kg is moving with a speed of 2.00 ms-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
A particle is projected at 600 to the horizontal with a kinetic energy . The kinetic energy at the highest point
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
In the reaction,
| Option 1)
|
Option 2)
|
| Option 3)
|
Option 4)
|
How many moles of magnesium phosphate, will contain 0.25 mole of oxygen atoms?
| Option 1)
0.02 |
Option 2)
3.125 × 10-2 |
| Option 3)
1.25 × 10-2 |
Option 4)
2.5 × 10-2 |
Colleges After 12th
Courses After 12th
Applications for Admissions are open.
As per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters