Aakash Repeater Courses
ApplyTake Aakash iACST and get instant scholarship on coaching programs.
A triangle is a geometrical shape having three sides and three angles. We can see different shapes around us that resemble a triangle, such as a slice of pizza, a clothes hanger, etc. 'Triangles' is one of the important topics in the Class 9 NCERT syllabus. In this chapter, students learn about the special types of triangles, such as equilateral triangles, isosceles triangles, and right-angled triangles etc. It introduces the concept of congruency of triangles, wherein triangles are said to be congruent if they have identical form and length. The chapter explains important congruence rules like SSS, SAS, AAS, and RHS. It also covers properties of triangles, inequalities in a triangle, and basic theorems such as the angle sum property. These NCERT Solutions are developed by subject matter experts at careersers360 considering the latest CBSE syllabus.
Don't Miss: Best Courses after 10th | Competitive Exams after 10th
The NCERT Solutions for Class 9 can be a good resource whenever you find difficulty in solving any of the problems. By using the NCERT class 9 maths chapter 7 solutions, you can prepare well for your exams at the school level as well as create a strong base for the competitive examinations. NCERT Solutions for Class 9 Maths and NCERT solutions for other subjects and classes can be downloaded from NCERT Solutions.
| Class 9 Maths Chapter 7 Question Answer: Exercise: 7.1 Total Questions: 8 Page number: 92-94 |
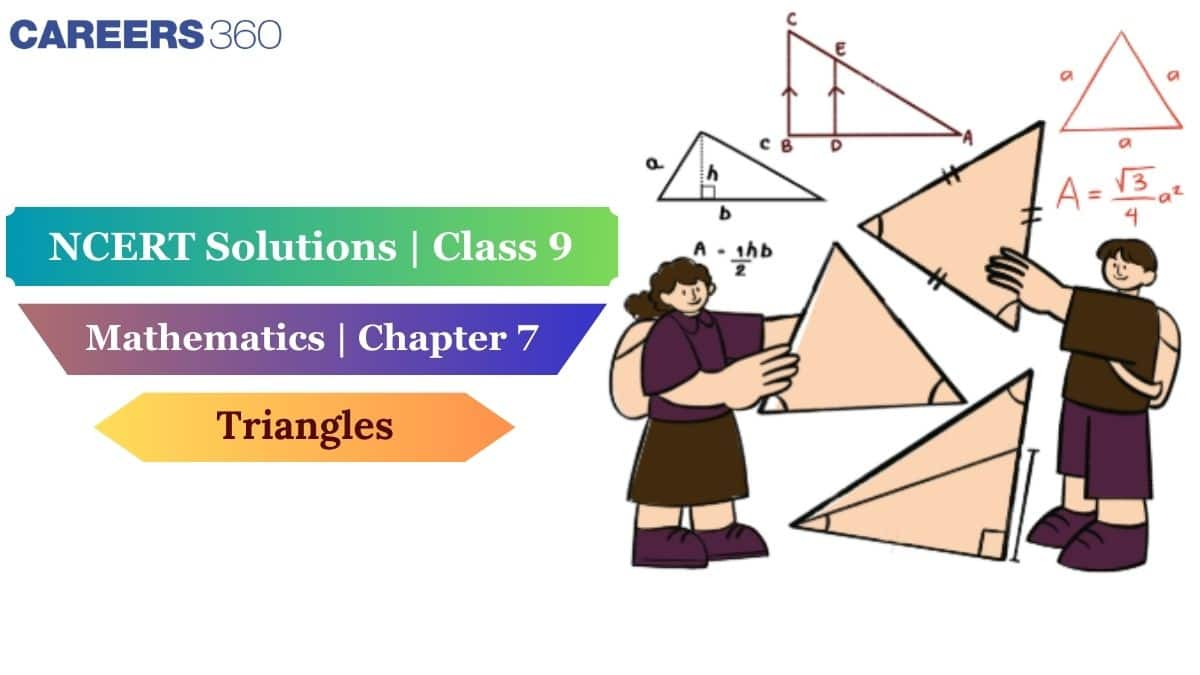
Question 1: In quadrilateral
Answer:
In the given triangles we are given that:-
(i)
(ii) Further, it is given that AB bisects angle A. Thus
(iii) Side AB is common in both the triangles.
Hence by SAS congruence, we can say that:
By c.p.c.t. (corresponding parts of congruent triangles are equal) we can say that
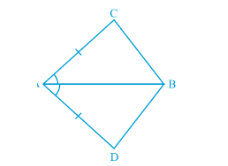
Question 2: (i)
Answer:
It is given that:-
(i) AD = BC
(ii)
(iii) Side AB is common in both the triangles.
So, by SAS congruence, we can write :
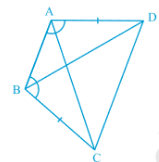
Question 2: (ii)
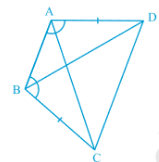
Answer:
In the previous part, we have proved that
Thus by c.p.c.t. , we can write :
Question 2: (iii)
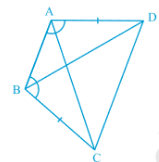
Answer:
In the first part, we have proved that
Thus by c.p.c.t. , we can conclude :
Question 3:
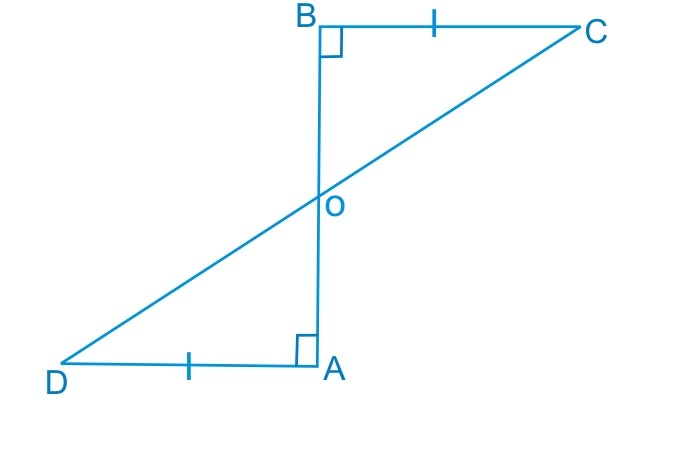
Answer:
In the given figure consider
(i) AD = BC (given)
(ii)
(iii)
Thus by AAS Postulate, we have
Hence by c.p.c.t. we can write :
And thus CD bisects AB.
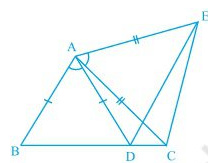
Question 4:
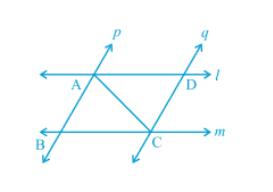
Answer:
In the given figure, consider
(i)
(ii)
(iii) Side AC is common in both the triangles.
Thus by ASA congruence, we have :
Question 5: (i) Line
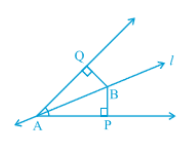
Answer:
In the given figure consider
(i)
(ii)
(iii) Side AB is common in both triangles.
Thus, AAS congruence, we can write :
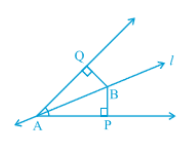
Answer:
In the previous part, we have proved that $\small \Delta APB\cong \Delta AQB.
Thus, by c.p.c.t.. we can write :
Thus B, is equidistant from the arms of angle A.
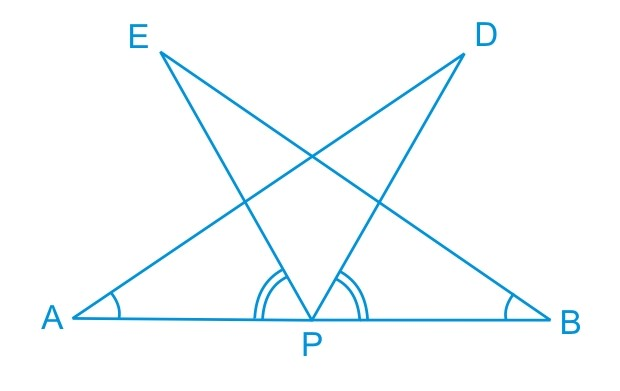
Question 6: In Fig,
Answer:
From the given figure following result can be drawn:-
Adding
Now consider
(i)
(ii)
(iii)
Thus by SAS congruence, we can say that :
Hence by c.p.c.t., we can say that :
Question 7: (i)
Answer:
From the figure, it is clear that :
Adding
or
Now, consider
(i)
(ii)
(iii)
Hence by ASA congruence, we can say that :
Question 7: (ii) AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that
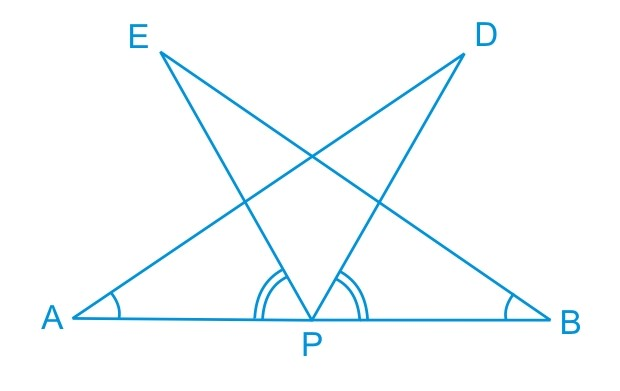
Answer:
In the previous part, we have proved that $\small \Delta DAP\cong \Delta EBP.
Thus by c.p.c.t., we can say that :
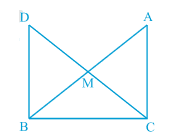
Answer:
Consider
(i)
(ii)
(iii)
Thus by SAS congruency, we can conclude that :
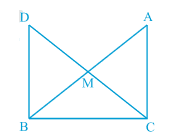
Answer:
In the previous part, we have proved that
By c.p.c.t. we can say that :
This implies side AC is parallel to BD.
Thus we can write :
and,
or
Hence,
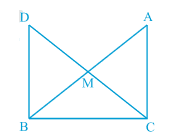
Answer:
Consider
(i)
(ii)
(iii)
Thus, SAS congruence, we can conclude that :
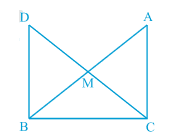
Answer:
In the previous part, we have proved that
Thus by c.p.c.t., we can write :
or
or
Hence proved.
|
Class 9 Maths Chapter 7 Question Answer: Exercise: 7.2
Total Questions: 8
Page number: 97-98
|
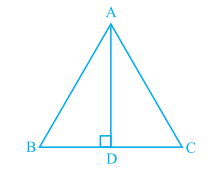
Question 1: (i) In an isosceles triangle ABC, with
Answer:
In the triangle ABC,
Since AB = AC, thus
or
or
Thus
Question 1: (ii) In an isosceles triangle ABC, with
Answer:
Consider
(i)
(ii)
(iii)
Thus by the SSS congruence rule, we can conclude that :
Now, by c.p.c.t.,
Hence AO bisects
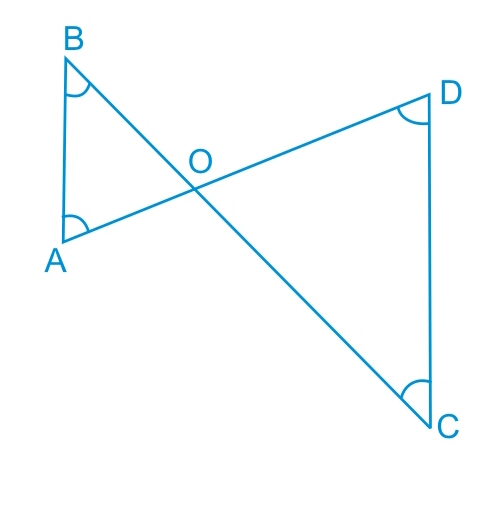
Question 2: In
Answer:
Consider
(i)
(ii)
(iii)
Thus, by SASthe congruence axiom, we can state :
Hence, by c.p.c.t., we can say that :
Thus
Answer:
Consider
(i)
(ii)
(iii)
Thus, by the AAS congruence axiom, we can conclude that :
Now, by c.p.c.t. we can say :
Hence, these altitudes are equal.
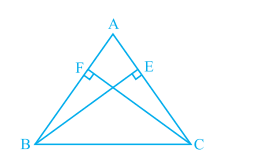
Question 4: (i) ABC is a triangle in which altitudes BE and CF to sides AC and AB are equal (see Fig). Show that
Answer:
Consider
(i)
(ii)
(iii)
Thus by AAS congruence, we can say that :
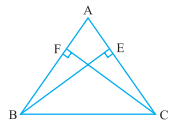
Question 4: (ii) ABC is a triangle in which altitudes BE and CF to sides AC and AB are equal (see Fig.). Show that
Answer:
From the previous part of the question we found out that:
Now, by c.p.c.t. we can say that :
Hence,
Question 5: ABC and DBC are two isosceles triangles on the same base BC (see Fig.). Show that
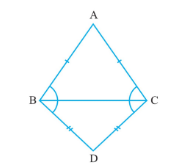
Answer:
Consider
(i)
(ii)
(iii)
Thus, by SSS congruency, we can conclude that :
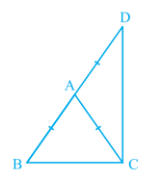
Answer:
Consider
It is given that AB = AC
So,
Similarly in
We have AD = AB
and
So,
or
And in
Adding (i) and (ii), we get :
or
and
Question 7: ABC is a right-angled triangle in which
Answer:
In the triangle ABC, sides AB and AC are equal.
We know that angles opposite to equal sides are also equal.
Thus,
Also, the sum of the interior angles of a triangle is
So, we have :
or
or
Hence,
Question 8: Show that the angles of an equilateral triangle are
Answer:
Consider a triangle ABC that has all sides equal.
We know that angles opposite to equal sides are equal.
Thus we can write :
Also, the sum of the interior angles of a triangle is
Hence,
or
or
So, all the angles of the equilateral triangle are equal (
|
Class 9 Maths Chapter 7 Question Answer: Exercise: 7.3
Total Questions: 5
Page number: 102
|
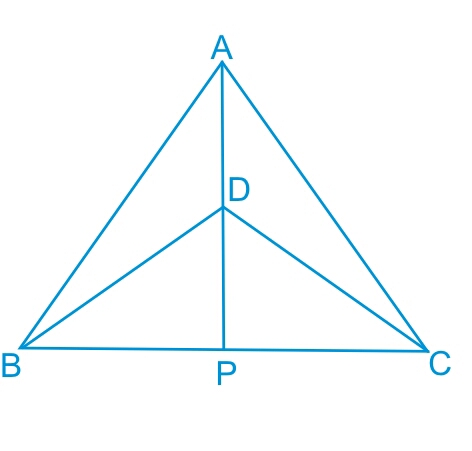
Answer:
Consider
(i)
(ii)
(iii)
Thus by SS,S congruency, we can conclude that :
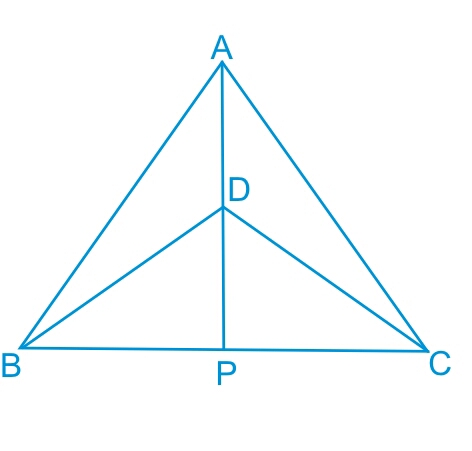
Answer:
Consider
(i)
(ii)
(iii)
Thus, by the SAS axiom, we can conclude that :
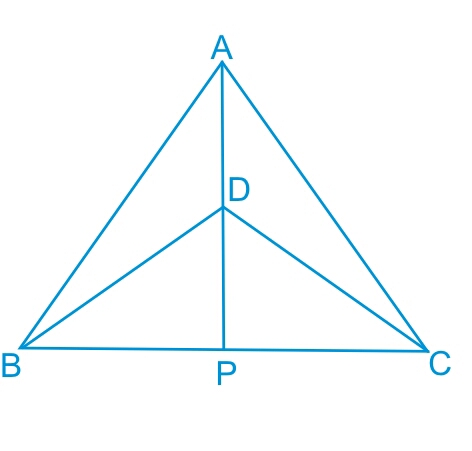
Answer:
In the first part, we have proved that
So, by c.p.c.t.
Hence AP bisects
Now consider
(i)
(ii)
(iii)
Thus, by SSS congruency, we have :
Hence by c.p.c.t. we have :
or AP bisects
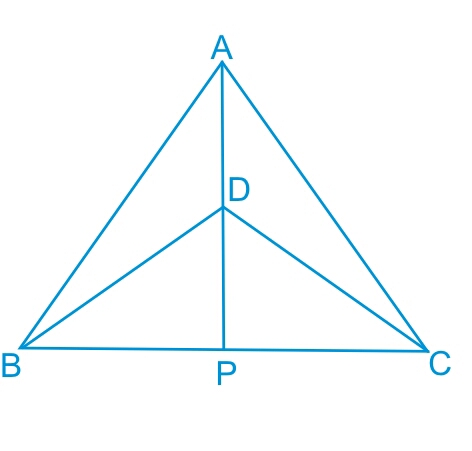
Answer:
In the previous part, we have proved that $\Delta BPD\ \cong \ \Delta CPD.
Thus by c.p.c.t. we can say that :
Also,
Since BC is a straight line, thus :
or
or
Hence, it is clear that AP is a perpendicular bisector of line BC.
Question 2: (i) AD is an altitude of an isosceles triangle ABC in which
Answer:
Consider
(i)
(ii)
(iii)
Thus, by the RHS axiom, we can conclude that :
Hence, by c.p.c.t. we can say that:
Question 2: (ii) AD is an altitude of an isosceles triangle ABC in which
Answer:
In the previous part of the question, we have proved that
Thus by c.p.c.t., we can write :
Hence,
(i)
(ii)
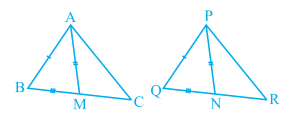
Answer:
(i) From the figure we can say that :
or
or
Now, consider
(a)
(b)
(c)
Thus, by the SSS congruence rule, we can conclude that :
(ii) Consider
(a)
(b)
(c)
Thus, by the SAS congruence rule,
Answer:
Using the given conditions, consider
(i)
(ii)
(iii)
Thus by RHS axiom, we can say that:
Hence by c.p.c.t.,
And thus
Question 5: ABC is an isosceles triangle with
Answer:
Consider
(i)
(ii)
(iii)
Thus, by the RHS axiom, we can conclude that :
Now, by c.p.c.t. we can say that :
Also, practice class 9 maths chapter 7 question answer using the exercise given below.
Question: In triangle ABC, AB = AC and
Answer:
Given AB = AC, so it is an isosceles triangle
Therefore,
Now, using the angle sum property:
The topics discussed in the NCERT Solutions for class 9, chapter 7, Triangles, are:
Congruence:
Congruent refers to figures that are identical in all aspects, including their shapes and sizes. For example, two circles with the same radius or two squares with the same side lengths are considered congruent.
Congruent Triangles:
Two triangles are considered congruent if and only if one of them can be superimposed (placed or overlaid) over the other in such a way that they entirely cover each other.
Congruence Rules for Triangles:
Side-Angle-Side (SAS) Congruence:
Angle-Side-Angle (ASA) Congruence:
Angle-Angle-Side (AAS) Congruence:.
Side-Side-Side (SSS) Congruence:
Right-Angle Hypotenuse Side (RHS) Congruence:
Given below are some ways in which students can approach the problems of the Triangles in class 9 mathematics:
We at Careers360 compiled all the NCERT class 9 Maths solutions in one place for easy student reference. The following links will allow you to access them.
Keep Working Hard and Happy Learning!
Congruence of triangles, Criteria for congruence of triangles, Properties of triangles, and inequalities of triangles are the important topics covered in this chapter. Students can practice NCERT solutions for class 9 maths to get a command of these concepts that ultimately help during the exams.
NCERT Solutions for maths chapter 7 class 9 explain that "congruence of triangles" refers to the condition where two triangles are identical copies of each other and overlap perfectly when superimposed. In simpler terms, two triangles are considered congruent when the angles and sides of one triangle are equivalent to the corresponding angles and sides of the other triangle.
You can get the detailed NCERT solutions for class 9 maths from the link given below. you can practice these solutions to have a better understanding of the concepts.
Link: school.careers360.com/ncert/ncert-solutions-class-9-maths.
NCERT Solutions for Class 9 Maths Chapter 7 can assist students in achieving a high score and excelling in the subject in their CBSE exams. These solutions are designed based on the latest CBSE syllabus and cover all the essential topics in the respective subject. By practicing these solutions, students can gain confidence and be better prepared to face the board exams. The topics covered in these solutions are fundamental and contribute significantly to obtaining top scores. Moreover, solving problems of varying difficulty levels helps students get accustomed to answering questions of all types. Thus, these solutions are highly recommended for students as a reference and for practice in preparation for their CBSE exams.
There are 15 chapters starting from the number system to probability in the CBSE class 9 maths.

Take Aakash iACST and get instant scholarship on coaching programs.

This ebook serves as a valuable study guide for NEET 2025 exam.

This e-book offers NEET PYQ and serves as an indispensable NEET study material.

As per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters

As per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
As per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE