Careers360 Logo
School
Search Colleges, Exams, Schools & more
NCERT Solutions for Exercise 7.3 Class 9 Maths Chapter 7 - Triangles

NCERT Solutions for Exercise 7.3 Class 9 Maths Chapter 7 - Triangles

Edited By Vishal kumar | Updated on Oct 07, 2023 09:21 AM IST

NCERT Solutions for Class 9 Maths Chapter 7 Triangles Exercise 7.3- Download Free PDF

NCERT Solutions for Class 9 Maths Chapter 7 Triangles Exercise 7.3- In this chapter of NCERT Solutions, we explore triangles and their properties, and Exercise 7.3 focuses on practical problem-solving using these concepts. Our free PDF 9th class maths exercise 7.3 answers break down complex concepts, making it easier for students to understand and practice. These resources are designed to help your learning and are accessible at your convenience. Whether you need to revise, prepare for exams, or strengthen your grasp of triangle properties, these NCERT class 9 maths chapter 7 exercise 7.3 solutions are here to assist you on your academic journey. Along with NCERT book Class 9 Maths, chapter 7 exercise 7.3 the following exercises are also present.

This Story also Contains
  1. NCERT Solutions for Class 9 Maths Chapter 7 Triangles Exercise 7.3- Download Free PDF
  2. NCERT Solutions for Class 9 Maths Chapter 7 – Triangles Exercise 7.3
  3. Access Triangles Class 9 Chapter 7 Exercise: 7.3
  4. More About NCERT Solutions for Class 9 Maths Exercise 7.3
  5. Benefits of NCERT Solutions for Class 9 Maths Exercise 7.3
  6. Key Features of Exercise 7.3 Class 9 Maths
  7. NCERT Solutions of Class 10 Subject Wise
  8. Subject Wise NCERT Exemplar Solutions
NCERT Solutions for Exercise 7.3 Class 9 Maths Chapter 7 - Triangles
NCERT Solutions for Exercise 7.3 Class 9 Maths Chapter 7 - Triangles

NCERT Solutions for Class 9 Maths Chapter 7 – Triangles Exercise 7.3

Download PDF


Access Triangles Class 9 Chapter 7 Exercise: 7.3

Q1 (i) \small \Delta ABC and \small \Delta DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig.). If AD is extended to intersect BC at P, show that \small \Delta ABD\cong \Delta ACD

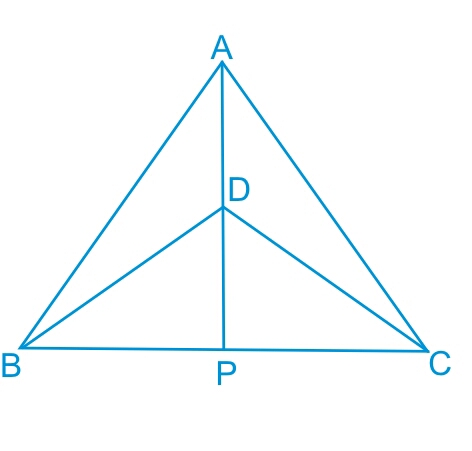
1640157485851

Answer:

Consider \Delta ABD and \Delta ACD ,

(i) AD\ =\ AD (Common)

(ii) AB\ =\ AC (Isosceles triangle)

(iii) BD\ =\ CD (Isosceles triangle)

Thus by SSS congruency we can conclude that :

\small \Delta ABD\cong \Delta ACD

Q1 (ii) Triangles ABC and Triangle DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig). If AD is extended to intersect BC at P, show that \small \Delta ABP \cong \Delta ACP

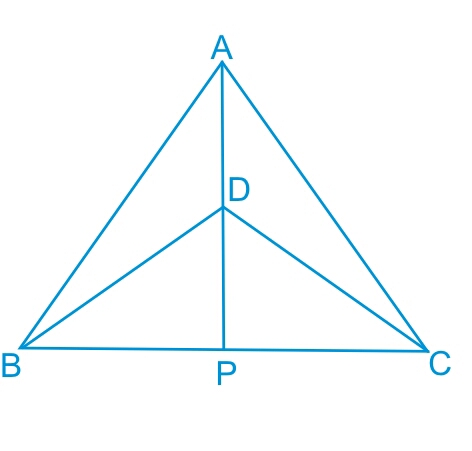
1640157528618

Answer:

Consider \Delta ABP and \Delta ACP ,

(i) AP is common side in both the triangles.

(ii) \angle PAB\ =\ \angle PAC (This is obtained from the c.p.c.t. as proved in the previous part.)

(iii) AB\ =\ AC (Isosceles triangles)

Thus by SAS axiom, we can conclude that :

\small \Delta ABP \cong \Delta ACP

Q1 (iii) \small \Delta ABC and \small \Delta DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig.). If AD is extended to intersect BC at P, show that AP bisects \small \angle A as well as \small \angle D .

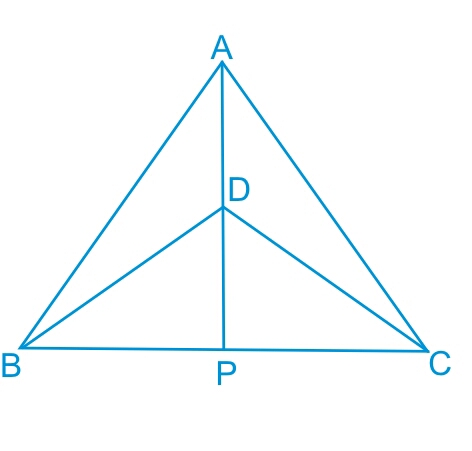
1640157546671

Answer:

In the first part, we have proved that \small \Delta ABD\cong \Delta ACD .

So, by c.p.c.t. \angle PAB\ =\ \angle PAC .

Hence AP bisects \angle A .

Now consider \Delta BPD and \Delta CPD ,

(i) PD\ =\ PD (Common)

(ii) BD\ =\ CD (Isosceles triangle)

(iii) BP\ =\ CP (by c.p.c.t. from the part (b))

Thus by SSS congruency we have :

\Delta BPD\ \cong \ \Delta CPD

Hence by c.p.c.t. we have : \angle BDP\ =\ \angle CDP

or AP bisects \angle D .

Q1 (iv) \small \Delta ABC and \small \Delta DBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see Fig.). If AD is extended to intersect BC at P, show that AP is the perpendicular bisector of BC.

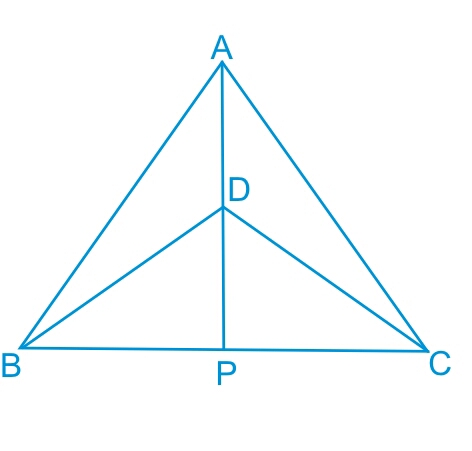
1640157712234

Answer:

In the previous part we have proved that \Delta BPD\ \cong \ \Delta CPD .

Thus by c.p.c.t. we can say that : \angle BPD\ =\ \angle CPD

Also, BP\ =\ CP

SInce BC is a straight line, thus : \angle BPD\ +\ \angle CPD\ =\ 180^{\circ}

or 2\angle BPD\ =\ 180^{\circ}

or \angle BPD\ =\ 90^{\circ}

Hence it is clear that AP is a perpendicular bisector of line BC.

Q2 (i) AD is an altitude of an isosceles triangle ABC in which \small AB=AC . Show that AD bisects BC

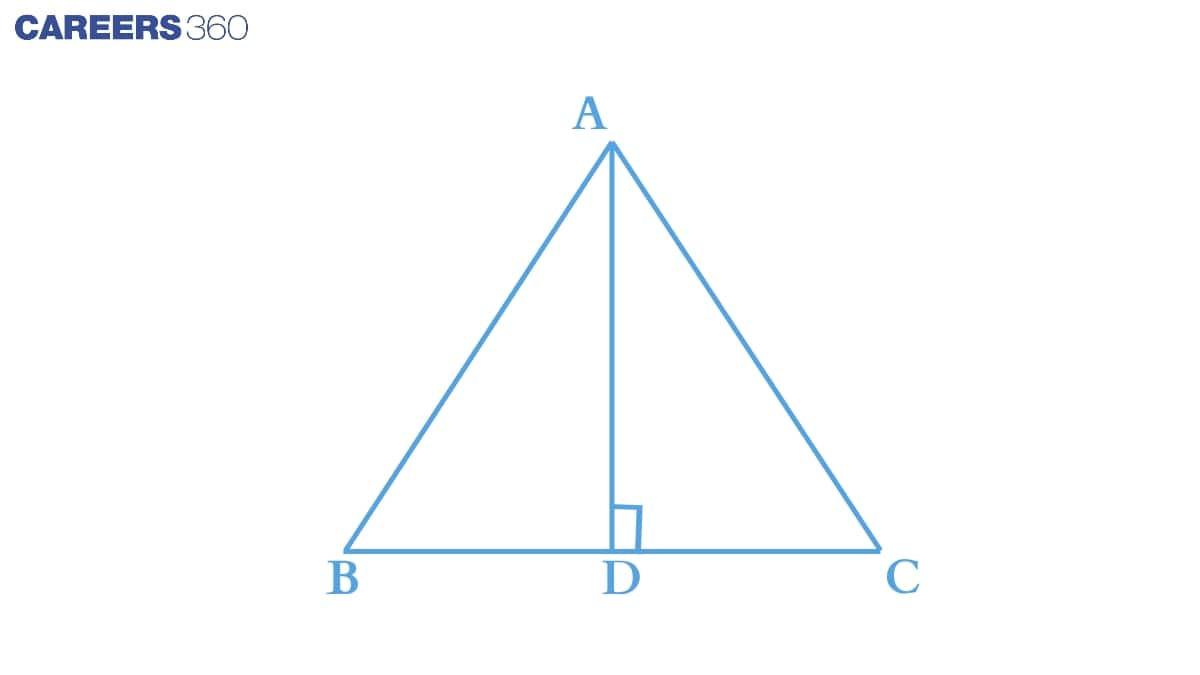
Answer:

Consider \Delta ABD and \Delta ACD ,

(i) AB\ =\ AC (Given)

(ii) AD\ =\ AD (Common in both triangles)

(iii) \angle ADB\ =\ \angle ADC\ =\ 90^{\circ}

Thus by RHS axiom we can conclude that :

\Delta ABD\ \cong \ \Delta ACD

Hence by c.p.c.t. we can say that : BD\ =\ CD or AD bisects BC.

Q2 (ii) AD is an altitude of an isosceles triangle ABC in which \small AB=AC . Show that AD bisects \small \angle A .

Answer:

In the previous part of the question we have proved that \Delta ABD\ \cong \ \Delta ACD

Thus by c.p.c.t., we can write :

\angle BAD\ =\ \angle CAD

Hence AD bisects \angle A .

Q3 Two sides AB and BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of \small \Delta PQR (see Fig). Show that:

(i) \small \Delta ABM \cong \Delta PQN

(ii) \small \Delta ABC \cong \Delta PQR

1640157733376

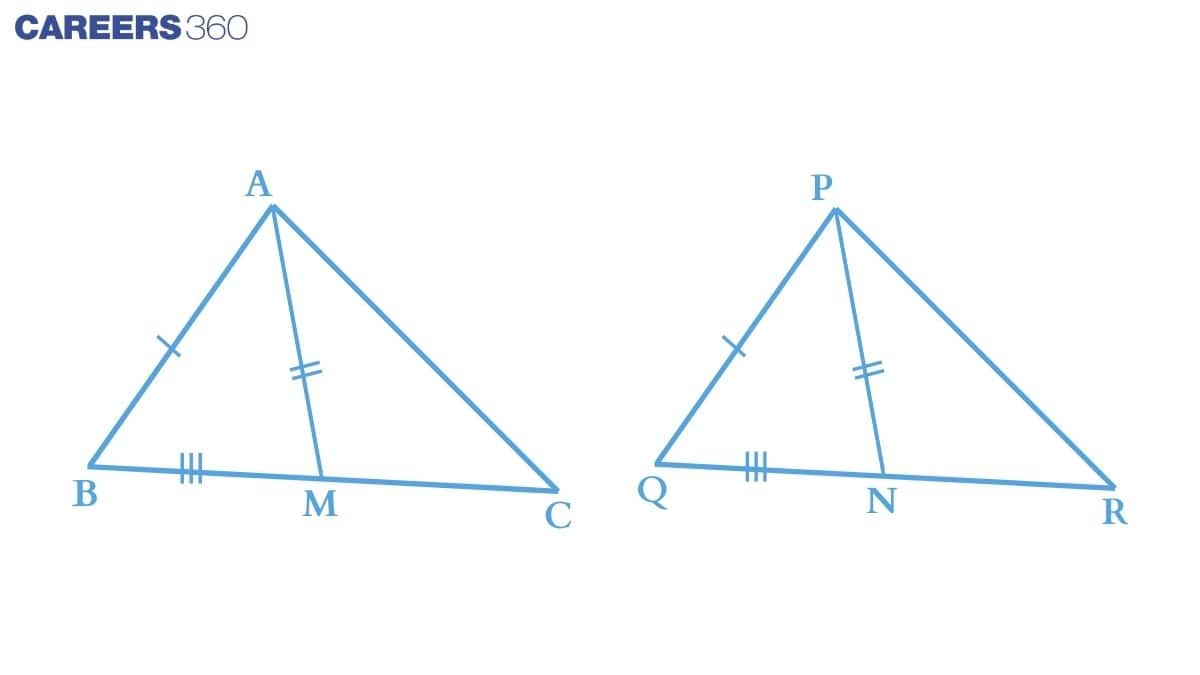
Answer:

(i) From the figure we can say that :

BC\ =\ QR

or \frac{1}{2}BC\ =\ \frac{1}{2}QR

or BM\ =\ QN

Now, consider \Delta ABM and \Delta PQN ,

(a) AM\ =\ PN (Given)

(b) AB\ =\ PQ (Given)

(c) BM\ =\ QN (Prove above)

Thus by SSS congruence rule, we can conclude that :

\small \Delta ABM \cong \Delta PQN

(ii) Consider \Delta ABC and \Delta PQR :

(a) AB\ =\ PQ (Given)

(b) \angle ABC\ =\ \angle PQR (by c.p.c.t. from the above proof)

(c) BC\ =\ QR (Given)

Thus by SAS congruence rule,

\small \Delta ABC \cong \Delta PQR

Q4 BE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.

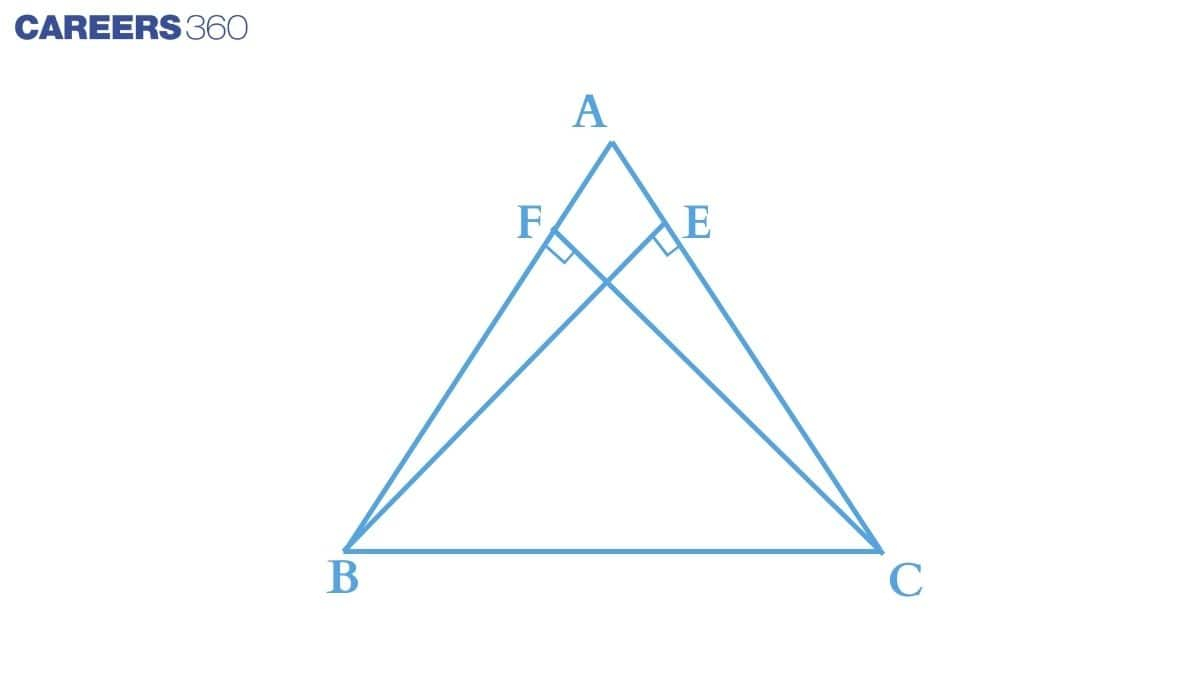
Answer:

Using the given conditions, consider \Delta BEC and \Delta CFB ,

(i) \angle BEC\ =\ \angle CFB (Right angle)

(ii) BC\ =\ BC (Common in both the triangles)

(iii) BE\ =\ CF (Given that altitudes are of the same length. )

Thus by RHS axiom, we can say that : \Delta BEC\ \cong \Delta CFB

Hence by c.p.c.t., \angle B\ =\ \angle C

And thus AB\ =\ AC (sides opposite to equal angles are also equal). Thus ABC is an isosceles triangle.

Q5 ABC is an isosceles triangle with \small AB=AC . Draw \small AP\perp BC to show that \small \angle B=\angle C .

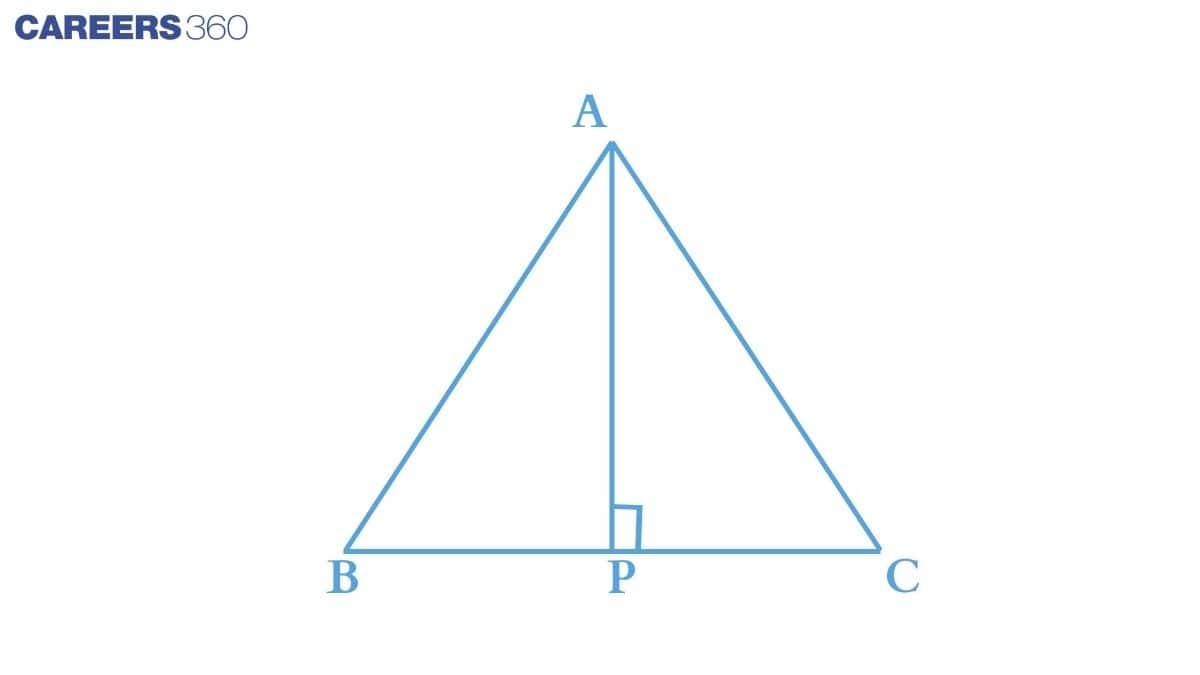
Answer:

Consider \Delta ABP and \Delta ACP ,

(i) \angle APB\ =\ \angle APC\ =\ 90^{\circ} (Since it is given that AP is altitude.)

(ii) AB\ =\ AC (Isosceles triangle)

(iii) AP\ =\ AP (Common in both triangles)

Thus by RHS axiom we can conclude that :

\Delta ABP\ \cong \Delta ACP

Now, by c.p.c.t.we can say that :

\angle B\ =\ \angle C

NCERT Solutions for Class 9 Maths Exercise 7.3 -The SSS congruence principle expresses that if three sides of one triangle are equivalent to those of another triangle, the two triangles are congruent to one other.

The RHS Congruence Rule explained in exercise 7.3 Class 9 Maths expresses that two right triangles are congruent if the hypotenuse and one side of one triangle are equivalent to the hypotenuse and one side of the other triangle.

A point that is equidistant from two given points is found on the perpendicular bisector of the line segment that connects them, and vice versa. On the bisectors of the angles formed by the two lines, a point equidistant from two intersecting lines lies.

More About NCERT Solutions for Class 9 Maths Exercise 7.3

The NCERT solutions for Class 9 Maths chapter 7 exercise 7.3 majorly deals with RHS (Right angle Hypotenuse Side) criteria of congruence and the SSS (Side Side Side) criteria of congruence apart from this. We get some questions related to the above criteria.

The NCERT solutions for Class 9 Maths exercise 7.3 majorly wraps all the six criteria of congruence related to triangles.

In this exercise, we are also introduced to the properties of an angle bisector. Like an instance on the bisectors of the angles formed by the intersection of the two lines, a point equidistant from two intersecting lines in the angle bisector.

Also Read| Triangles Class 9 Notes

Benefits of NCERT Solutions for Class 9 Maths Exercise 7.3

  • Exercise 7.3 Class 9 Maths, is based on RHS and SSS criteria of congruent triangles.

  • Understanding the concepts from Class 9 Maths chapter 7 exercise 7.3 will allow us to understand the new concepts which are going to be introduced in exercise 7.4

Key Features of Exercise 7.3 Class 9 Maths

  1. Step-by-Step Solutions: The 9th class maths exercise 7.3 answers offers step-by-step solutions to guide students through solving problems, promoting a clear understanding of the concepts.

  2. Varied Difficulty Levels: Problems in this class 9 maths ex 7.3 come with varying levels of difficulty, allowing students to gradually build their skills and confidence.

  3. Preparation for Exams: Ex 7.3 class 9 helps students prepare for exams by offering a range of problems that may be encountered in assessments.

  4. Consolidation of Knowledge: It serves as a tool to consolidate knowledge acquired in previous chapters related to triangles and their properties.

Also, See

NCERT Solutions of Class 10 Subject Wise

Subject Wise NCERT Exemplar Solutions

Frequently Asked Question (FAQs)

1. What is the main concept of NCERT solutions for Class 9 Maths exercise 7.3?

This exercise includes new methods of congruency of triangles like right-angle hypotenuse side, side-side- side theorem and some properties.

2. Name the new criteria for congruence of two triangles in this exercise?

We are introduced to two new criteria of congruence:

The RHS (Right angle Hypotenuse Side) and the SSS (Side Side Side) congruence.

3. What do you understand by congruent triangles?

Two triangles are said to be congruent if all three corresponding sides of the two triangles are equal and all the three corresponding angles of the triangles are equal in measurement.

4. What do you understand by RHS criteria for congruence?

It expresses that if the hypotenuse and side of one right-calculated triangle are equivalent to the hypotenuse and the relating side of another right-angled triangle, then the two triangles are congruent. 

5. What do you comprehend by SSS criteria for congruence?

It expresses that two triangles are congruent if three sides of a triangle are equivalent to the relating sides of the other triangle.

6. We have two triangles with angles in the ratio of 2 : 3 : 5 can they be congruent?

No, these triangles are similar, not congruent. 

7. Name the different types of triangles?

The different types of triangles are:

Scalene Triangle.

Isosceles Triangle.

Equilateral Triangle.

8. Can an equilateral triangle be a right-angled triangle?

No, an equilateral triangle can never be a right-angled triangle as each angle of an equilateral triangle is 60 degrees.

Also Read

Articles

Upcoming School Exams

National Institute of Open Schooling 12th Examination

Admit Card Date:28 March,2024 - 22 May,2024

National Institute of Open Schooling 10th examination

Admit Card Date:28 March,2024 - 22 May,2024

Bihar Board 12th Examination

Admit Card Date:19 April,2024 - 11 May,2024

Karnataka Pre-University Certificate Examination

Exam Date:04 May,2024 - 04 May,2024

Bihar Board 10th Examination

Exam Date:04 May,2024 - 11 May,2024

View All School Exams
Get answers from students and experts

Popular Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1)

0.34\; J

 Option 2)

0.16\; J
Option 3)

1.00\; J

 Option 4)

0.67\; J
Read More

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times.  Assume that the potential energy lost each time he lowers the mass is dissipated.  How much fat will he use up considering the work done only when the weight is lifted up ?  Fat supplies 3.8×107 J of energy per kg which is converted to mechanical energy with a 20% efficiency rate.  Take g = 9.8 ms−2 :

Option 1)

2.45×10−3 kg

 Option 2)

 6.45×10−3 kg
Option 3)

 9.89×10−3 kg

 Option 4)

12.89×10−3 kg

 
Read More

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1)

2,000 \; J - 5,000\; J

 Option 2)

200 \, \, J - 500 \, \, J
Option 3)

2\times 10^{5}J-3\times 10^{5}J

 Option 4)

20,000 \, \, J - 50,000 \, \, J
Read More

A particle is projected at 600   to the horizontal with a kinetic energy K. The kinetic energy at the highest point

Option 1)

K/2\,

 Option 2)

\; K\;
Option 3)

zero\;

 Option 4)

K/4
Read More

In the reaction,

2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}

Option 1)

11.2\, L\, H_{2(g)}  at STP  is produced for every mole HCL_{(aq)}  consumed

 Option 2)

6L\, HCl_{(aq)}  is consumed for ever 3L\, H_{2(g)}      produced
Option 3)

33.6 L\, H_{2(g)} is produced regardless of temperature and pressure for every mole Al that reacts

 Option 4)

67.2\, L\, H_{2(g)} at STP is produced for every mole Al that reacts .
Read More

How many moles of magnesium phosphate, Mg_{3}(PO_{4})_{2} will contain 0.25 mole of oxygen atoms?

Option 1)

0.02

 Option 2)

3.125 × 10-2
Option 3)

1.25 × 10-2

 Option 4)

2.5 × 10-2
Read More

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1)

decrease twice

 Option 2)

increase two fold
Option 3)

remain unchanged

 Option 4)

be a function of the molecular mass of the substance.
Read More

With increase of temperature, which of these changes?

Option 1)

Molality

 Option 2)

Weight fraction of solute
Option 3)

Fraction of solute present in water

 Option 4)

Mole fraction.
Read More

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol-1) is

Option 1)

twice that in 60 g carbon

 Option 2)

6.023 × 1022
Option 3)

half that in 8 g He

 Option 4)

558.5 × 6.023 × 1023
Read More

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t2) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m2 , the number of rotations made by the pulley before its direction of motion if reversed, is

Option 1)

less than 3

 Option 2)

more than 3 but less than 6
Option 3)

more than 6 but less than 9

 Option 4)

more than 9
Read More

Colleges After 12th

Popular Course After 12th

Also Read

Applications for Admissions are open.

JEE Main Important Physics formulas
JEE Main Important Physics formulas
Apply

As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters

UPES School of Liberal Studies
UPES School of Liberal Studies
Apply

Ranked #52 Among Universities in India by NIRF | Up to 30% Merit-based Scholarships | Lifetime placement assistance

Aakash iACST Scholarship Test 2024
Aakash iACST Scholarship Test 2024
Apply

Get up to 90% scholarship on NEET, JEE & Foundation courses

JEE Main Important Chemistry formulas
JEE Main Important Chemistry formulas
Apply

As per latest 2024 syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters

PACE IIT & Medical, Financial District, Hyd
PACE IIT & Medical, Financial District, Hyd
Apply

Enrol in PACE IIT & Medical, Financial District, Hyd for JEE/NEET preparation

ALLEN JEE Exam Prep
ALLEN JEE Exam Prep
Apply

Start your JEE preparation with ALLEN

View All Application Forms
News and Notifications
Barmer teacher suspended for slapping class 3 student
01/05/2024, 17:07:10
Teacher arrested for `sexual exploitation' at Ujjain Ashram school
01/05/2024, 14:28:18
Delhi NCR schools get bomb threat; fire tender arrives, search underway
01/05/2024, 04:09:04
Education is greatest gift to society: Vice President Jagdeep Dhankhar
30/04/2024, 17:02:12
Jharkhand schools up to Class 8 closed till further orders due to heat wave
30/04/2024, 11:49:37
Delhi School EWS Admission 2024-25: Registration window opens at edudel.nic.in; eligibility
30/04/2024, 09:27:25
Education ministry asks NCERT to review, update textbooks on yearly basis
29/04/2024, 12:22:03
Back to top