NCERT Solutions for Class 9 Maths Chapter 7 Exercise 7.1 - Triangles

Q1 In quadrilateral $ABCD$ , $AC=AD$ and $AB$ bisects $\mathrm{\angle }A$ (see Fig.7.16). Show that $\mathrm{\Delta }ABC\cong \mathrm{\Delta }ABD$ . What can you say about $BC$ and $BD$ ?

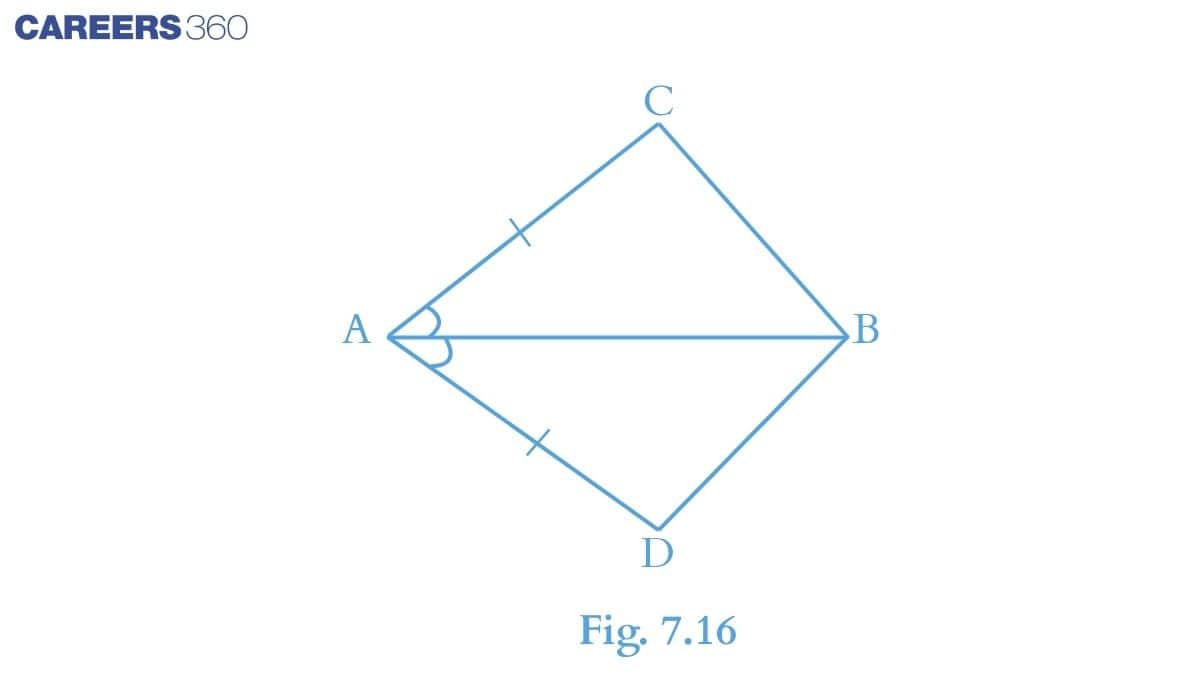
Solution:
In the given triangles, we are given that:-
In $\mathrm{\Delta }ABC$ and $\mathrm{\Delta }ABD$
(i) $AC=AD$
(ii) Further, it is given that AB bisects angle A.
Thus, $\mathrm{\angle }$ BAC $=\mathrm{\angle }$ BAD.
(iii) Side AB is common to both triangles. $AB=AB$
Hence by SAS congruence, we can say that: $\mathrm{\Delta }ABC\cong \mathrm{\Delta }ABD$
By C.P.C.T. (corresponding parts of congruent triangles are equal) we can say that $BC=BD$

Q2 (i) $ABCD$ is a quadrilateral in which $AD=BC$ and $\mathrm{\angle }DAB=\mathrm{\angle }CBA$ (see Fig. 7.17). Prove that $\mathrm{\Delta }ABD\cong \mathrm{\Delta }BAC$ .

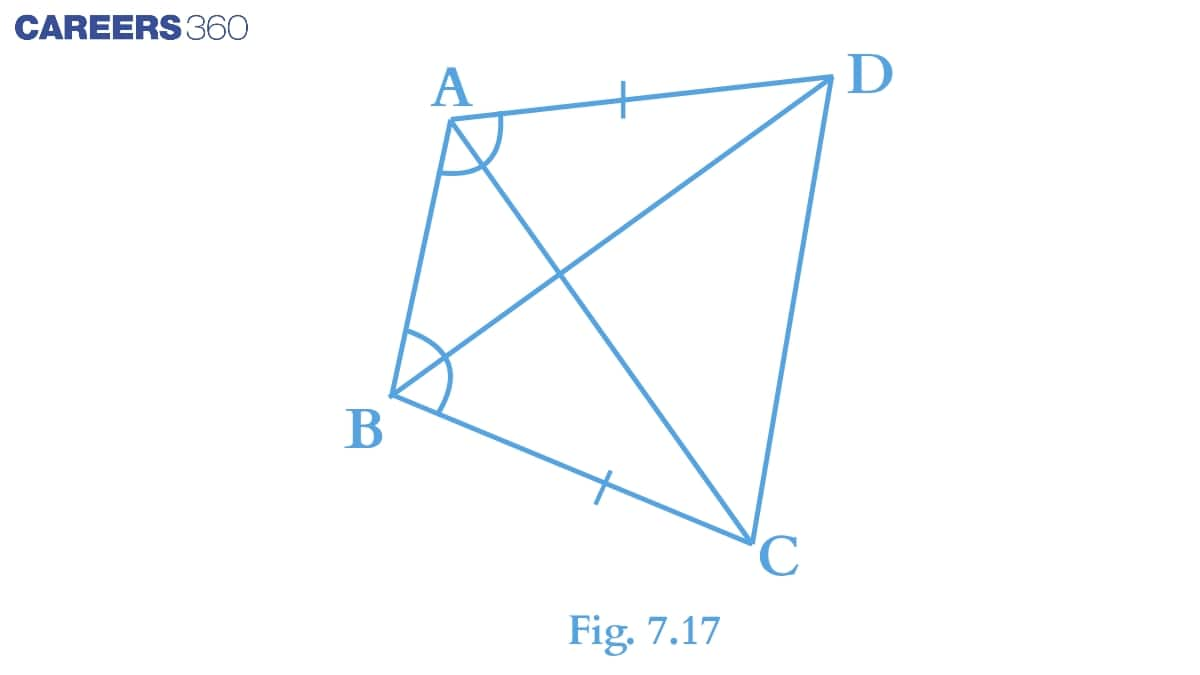
Solution:
In $\mathrm{\Delta }ABD$ and $\mathrm{\Delta }BAC$
(i) AD = BC
(ii) $\mathrm{\angle }DAB=\mathrm{\angle }CBA$
(iii) AB = AB (Side AB is common to both the triangles)
So, by SAS congruence, we can write :
$\mathrm{\Delta }ABD\cong \mathrm{\Delta }BAC$

Q2 (ii) ABC $ABCD$ is a quadrilateral in which $AD=BC$ and $\mathrm{\angle }DAB=\mathrm{\angle }CBA$ (see Fig.7.17). Prove that $BD=AC$

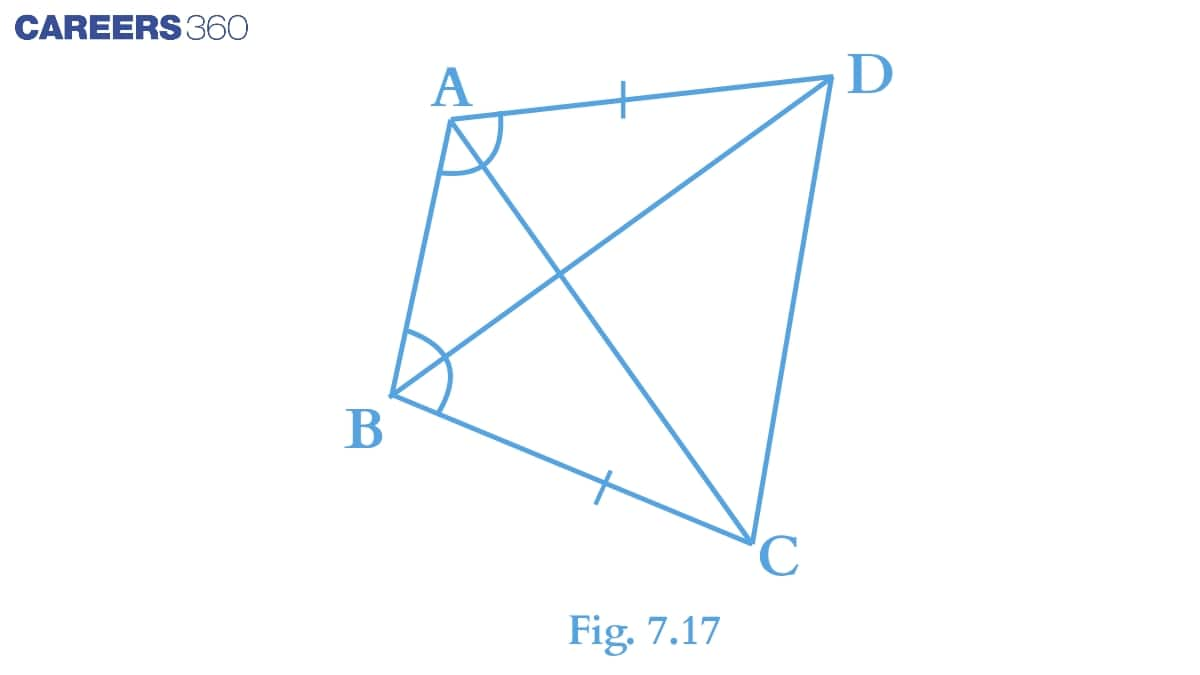
Solution:
In the previous part, we have proved that $\mathrm{\Delta }ABD\cong \mathrm{\Delta }BAC$.
Thus, by C.P.C.T., we can write: $BD=AC$

Q2 (iii) $ABCD$ is a quadrilateral in which $AD=BC$ and $\mathrm{\angle }DAB=\mathrm{\angle }BAC$ (see Fig.7.17). Prove that $\mathrm{\angle }ABD=\mathrm{\angle }BAC$ .

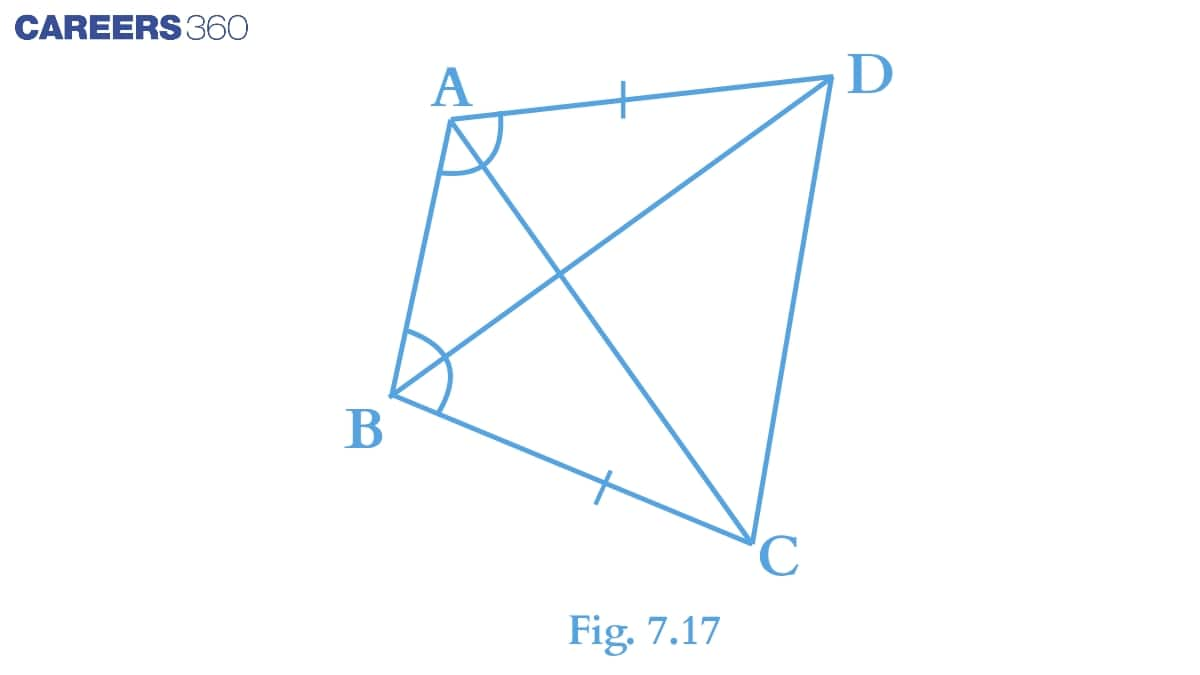
Solution:
In the first part we have proved that $\mathrm{\Delta }ABD\cong \mathrm{\Delta }BAC$.
Thus, by C.P.C.T., we can conclude,
$\mathrm{\angle }ABD=\mathrm{\angle }BAC$ ∠ABD=∠B

Q3. $AD$ and $BC$ are equal perpendiculars to a line segment $AB$ (see Fig.). Show that $CD$ bisects $AB$ .

Solution:
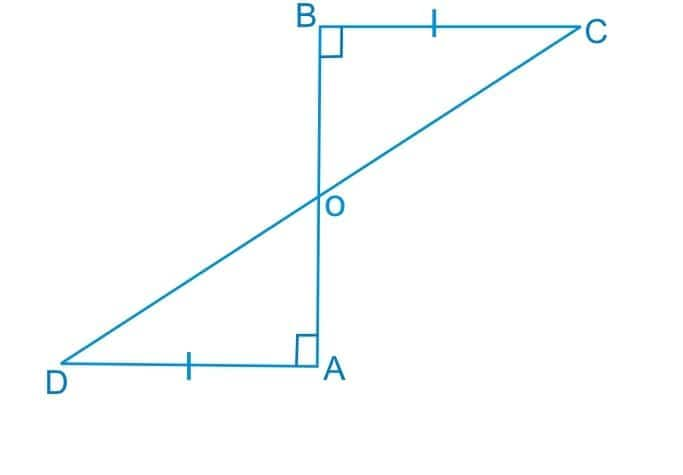
In the given figure consider $\mathrm{\Delta }$ AOD and $\mathrm{\Delta }$ BOC.
(i) AD = BC (Given)
(ii) $\mathrm{\angle }$ A = $\mathrm{\angle }$ B (Given that the line AB is perpendicular to AD and BC)
(iii) $\mathrm{\angle }$ AOD = $\mathrm{\angle }$ BOC (Vertically opposite angles).
Thus by AAS Postulate, we have
$\mathrm{\Delta }AOD\cong \mathrm{\Delta }BOC$
Hence, by C.P.C.T. we can write: $AO=OB$
Thus, CD bisects AB.

Q4. $l$ and $m$ are two parallel lines intersected by another pair of parallel lines $p$ and $q$ (see Fig.7.19 ). Show that $\mathrm{\Delta }ABC\cong \mathrm{\Delta }CDA$ .

Solution:
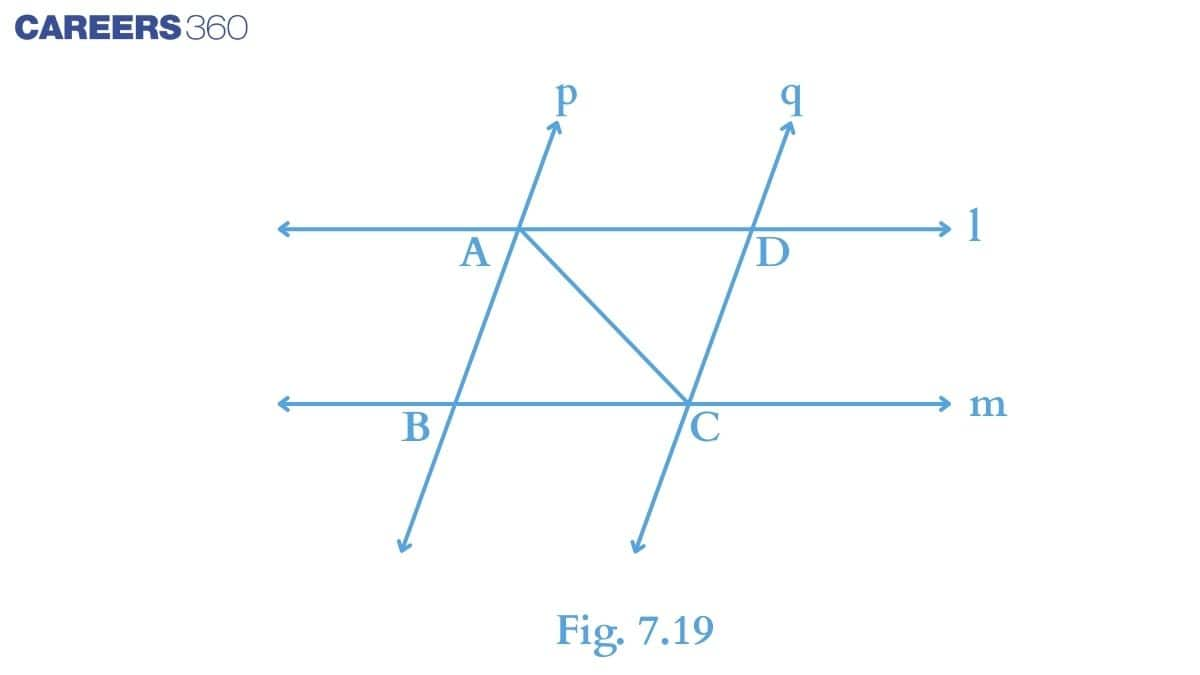
In the given figure, consider $\mathrm{\Delta }$ ABC and $\mathrm{\Delta }$ CDA :
(i) $\mathrm{\angle }BCA=\mathrm{\angle }DAC$ (Alternate interior angles)
(ii) $\mathrm{\angle }BAC=\mathrm{\angle }DCA$ (Alternate interior angles)
(iii) Side AC is common in both the triangles.
Thus by ASA congruence, we have:
$\mathrm{\Delta }ABC\cong \mathrm{\Delta }CDA$

ΔABC ≅ ΔCD

Q5 (i) Line $l$ is the bisector of an angle $\mathrm{\angle }A$ and B is any point on $l$ . $BP$ and $BQ$ are perpendiculars from $B$ to the arms of $\mathrm{\angle }A$ (see Fig. 7.20). Show that: $\mathrm{\Delta }APB\cong \mathrm{\Delta }AQB$

Solution:
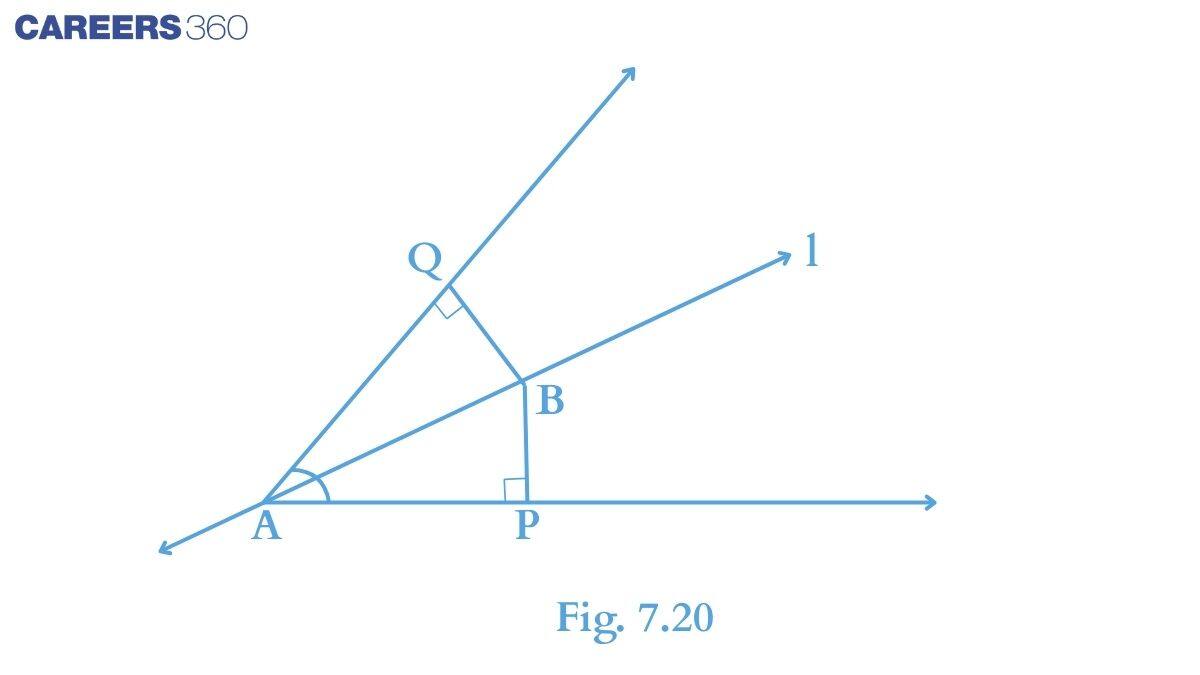
In the given figure consider $\mathrm{\Delta }APB$ and $\mathrm{\Delta }AQB$,
(i) $\mathrm{\angle }P=\mathrm{\angle }Q$ (Right angle)
(ii) $\mathrm{\angle }BAP=\mathrm{\angle }BAQ$ (Since it is given that I is bisector)
(iii) Side AB is common to both triangles.
Thus, AAS congruence, we can write:
$\mathrm{\Delta }APB\cong \mathrm{\Delta }AQB$

ΔAPB≅ΔAQ

Q5 (ii) Line $l$ is the bisector of an angle $\mathrm{\angle }A$ and $B$ is any point on $l$ . $BP$ and $BQ$ are perpendiculars from $B$ to the arms of $\mathrm{\angle }A$ (see Fig. 7.20). Show that: $BP=BQ$ or $B$ is equidistant from the arms of $\mathrm{\angle }A$ .

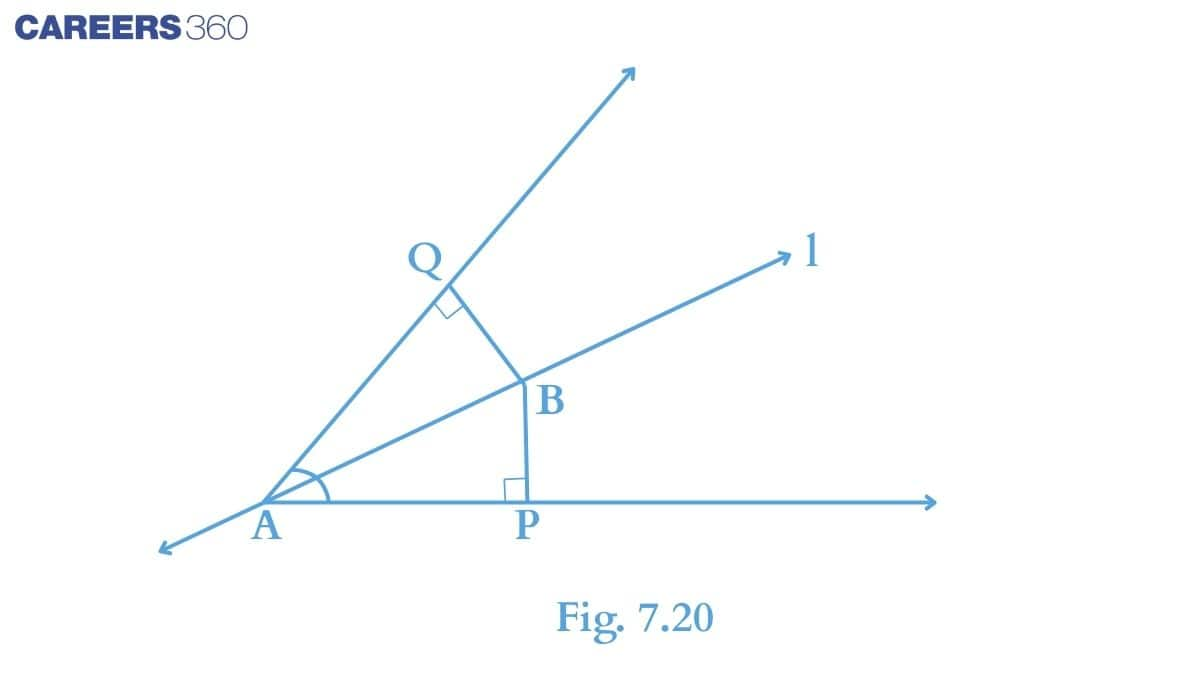
Solution:
In the previous part, we have proved that $\mathrm{\Delta }APB\cong \mathrm{\Delta }AQB$.
Thus, by C.P.C.T., we can write:
$BP=BQ$
Thus, B is equidistant from the arms of angle A.

Q6. In Fig, $AC=AE,AB=AD$ and $\mathrm{\angle }BAD=\mathrm{\angle }EAC$ . Show that $BC=DE$ .

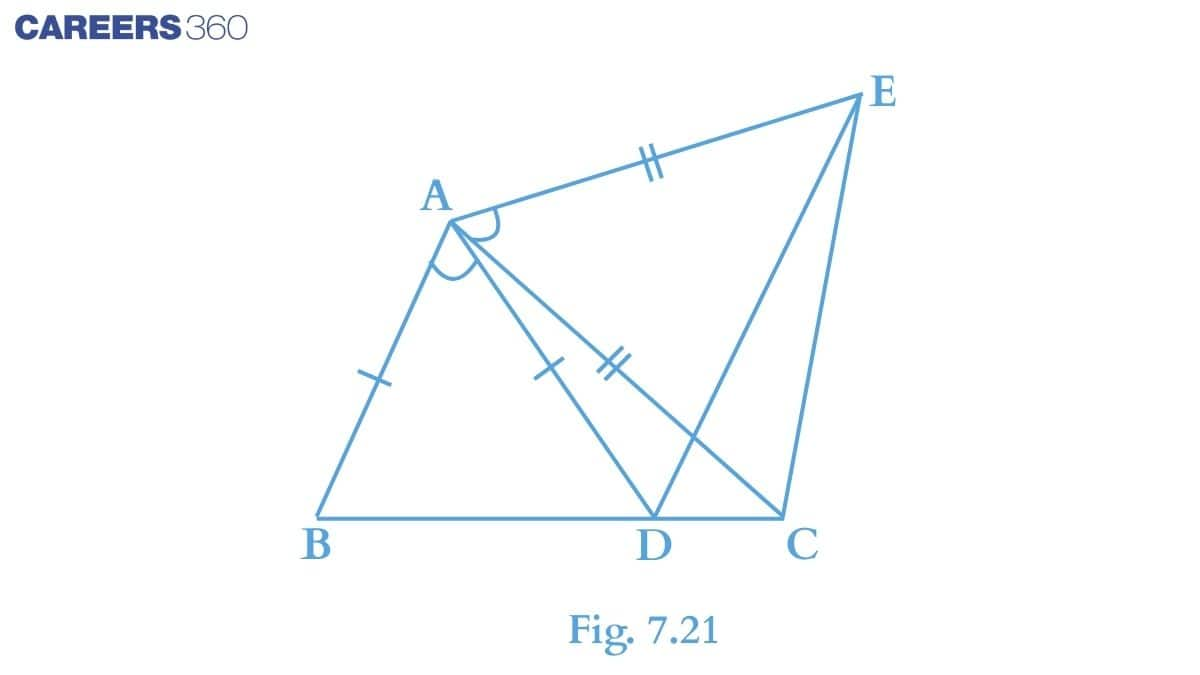
Solution:
From the given figure following result can be drawn:-
$\mathrm{\angle }BAD=\mathrm{\angle }EAC$
Adding $\mathrm{\angle }DAC$ to both sides, we get:
$\mathrm{\angle }BAD+\mathrm{\angle }DAC=\mathrm{\angle }EAC+\mathrm{\angle }DAC$
$\mathrm{\angle }BAC=\mathrm{\angle }EAD$
Now consider $\mathrm{\Delta }ABC$ and $\mathrm{\Delta }ADE$ , :-
(i) $AC=AE$ (Given)
(ii) $\mathrm{\angle }BAC=\mathrm{\angle }EAD$ (Proved above)
(iii) $AB=AD$ (Given)
Thus, by SAS congruence, we can say that :
$\mathrm{\Delta }ABC\cong \mathrm{\Delta }ADE$
Hence by C.P.C.T., we can say that : $BC=DE$

Q7 (i) $AB$ is a line segment and $P$ is its mid-point. $D$ and $E$ are points on the same side of $AB$ such that $\mathrm{\angle }BAD=\mathrm{\angle }ABE$ and $\mathrm{\angle }EPA=\mathrm{\angle }DPB$ (see Fig). Show that $\mathrm{\Delta }DAP\cong \mathrm{\Delta }EBP$

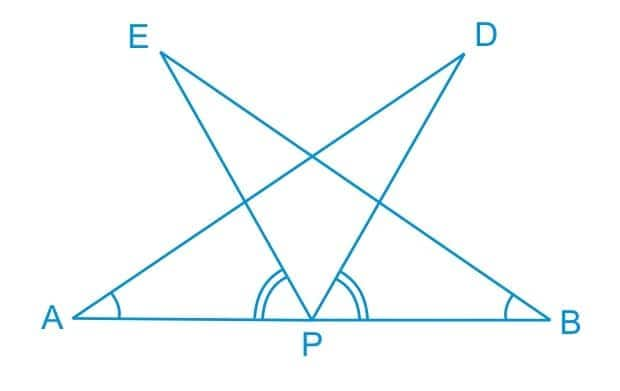
Solution:
From the figure, it is clear that :
$\mathrm{\angle }EPA=\mathrm{\angle }DPB$
Adding $\mathrm{\angle }DPE$ both sides, we get:
$\mathrm{\angle }EPA+\mathrm{\angle }DPE=\mathrm{\angle }DPB+\mathrm{\angle }DPE$
Or $\mathrm{\angle }DPA=\mathrm{\angle }EPB$
Now, consider $\mathrm{\Delta }DAP$ and $\mathrm{\Delta }EBP$ :
(i) $\mathrm{\angle }DPA=\mathrm{\angle }EPB$ (Proved above)
(ii) $AP=BP$ (Since P is the midpoint of line AB)
(iii) $\mathrm{\angle }BAD=\mathrm{\angle }ABE$ (Given)
Hence, by ASA congruence, we can say that:
$\mathrm{\Delta }DAP\cong \mathrm{\Delta }EBP$

Q7 (ii) AB is a line segment and P is its midpoint. D and E are points on the same side of AB such that $\mathrm{\angle }BAD=\mathrm{\angle }ABE$ and $\mathrm{\angle }EPA=\mathrm{\angle }DPA$ (see Fig). Show that $AD=BE$

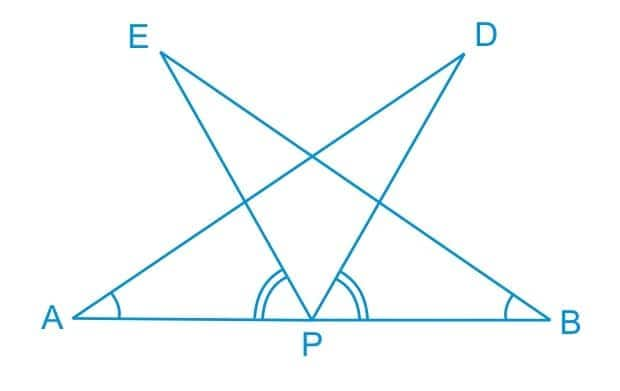
Solution:
In the previous part we have proved that $\mathrm{\Delta }DAP\cong \mathrm{\Delta }EBP$.
Thus, by C.P.C.T., we can say that:
$AD=BE$

Q8 (i) In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that $DM=CM$ . Point D is joined to point B (see Fig.7.23). Show that: $\mathrm{\Delta }AMC\cong \mathrm{\Delta }BMD$

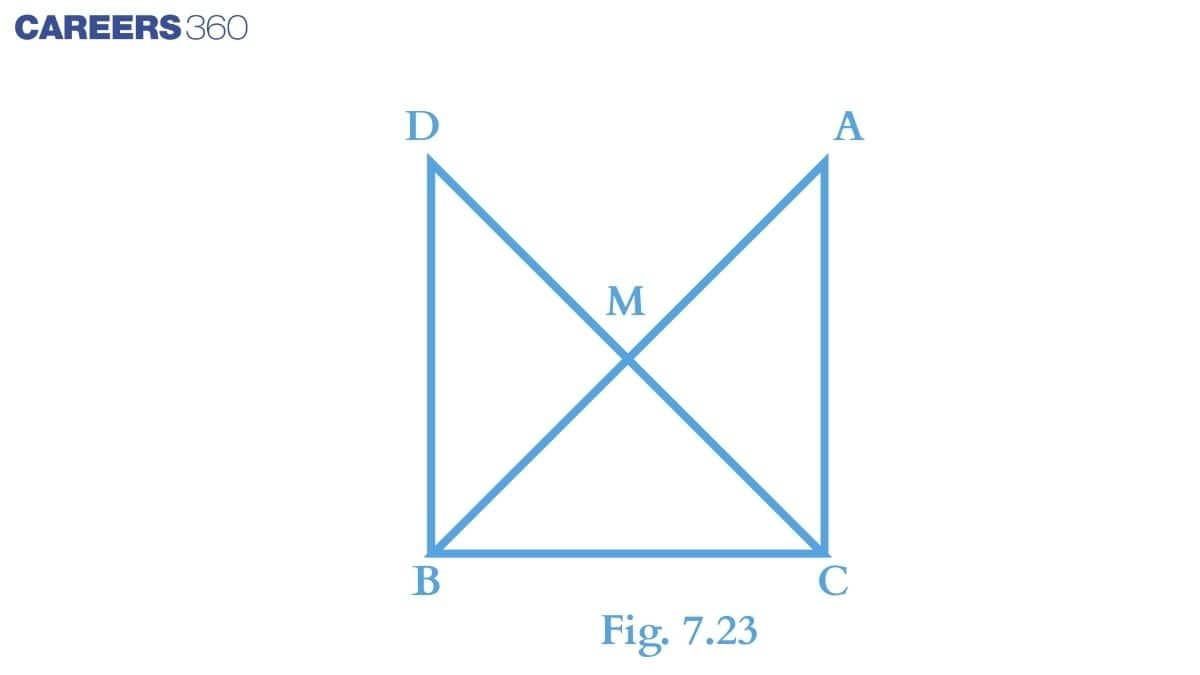
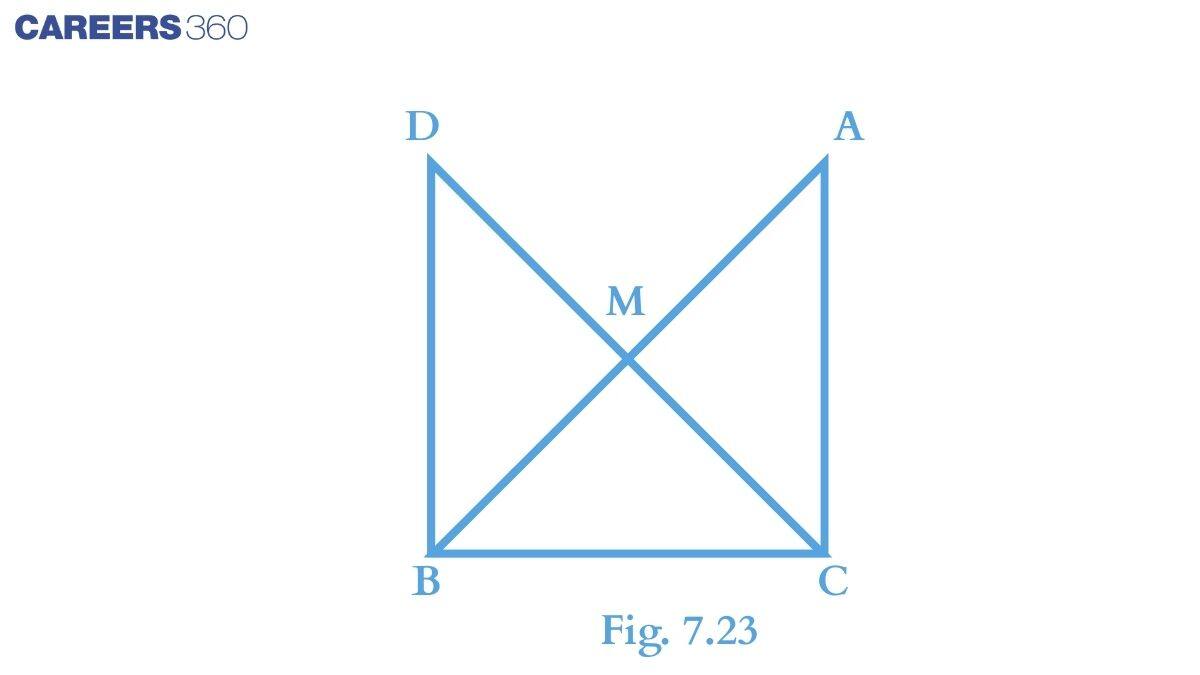
Solution:Consider $\mathrm{\Delta }AMC$ and $\mathrm{\Delta }BMD$,
(i) $AM=BM$ (Since M is the mid-point)
(ii) $\mathrm{\angle }CMA=\mathrm{\angle }DMB$ (Vertically opposite angles are equal)
(iii) $CM=DM$ (Given)
Thus, by SAS congruency, we can conclude that:
$\mathrm{\Delta }AMC\cong \mathrm{\Delta }BMD$

Q8 (ii) In right triangle ABC, right angled at C, M is the midpoint of the hypotenuse AB. C is joined to M and produced to a point D such that $DM=CM$ . Point D is joined to point B (see Fig.7.23). Show that: $\mathrm{\angle }DBC$ is a right angle.

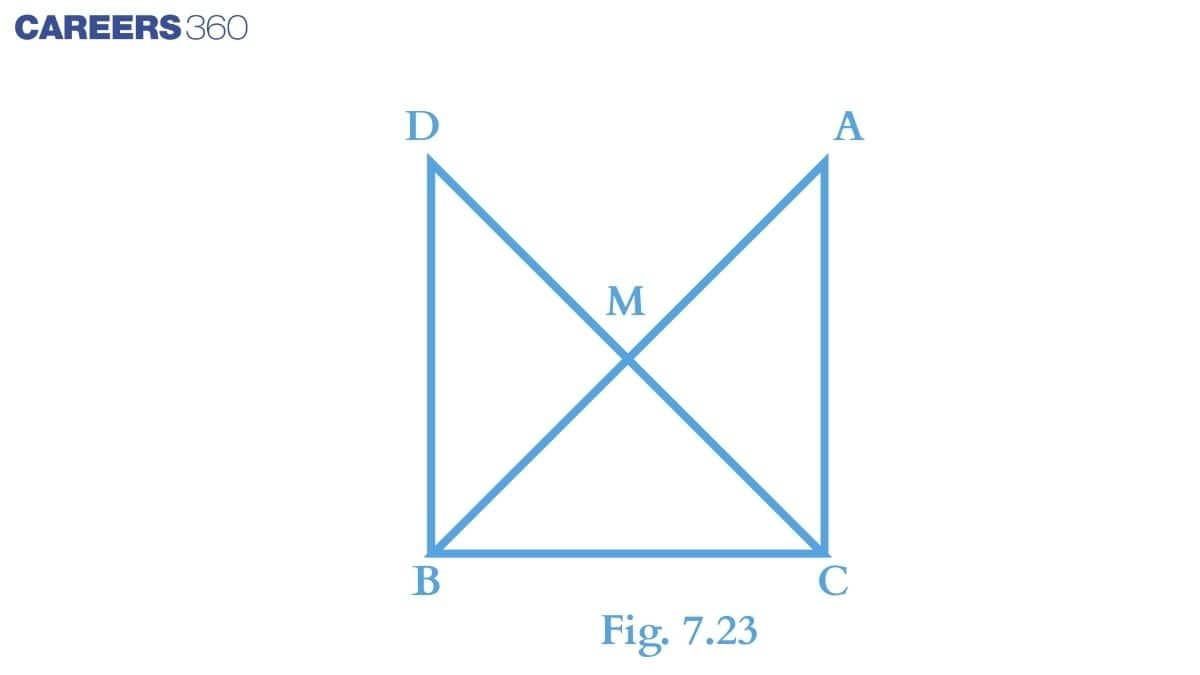
Solution:
In the previous part, we have proved that $\mathrm{\Delta }AMC\cong \mathrm{\Delta }BMD$.
By c.p.c.t. we can say that: $\mathrm{\angle }ACM=\mathrm{\angle }BDM$
This implies side AC is parallel to BD.
Thus, we can write : $\mathrm{\angle }ACB+\mathrm{\angle }DBC={180}^{\circ }$ (Co-interior angles)
And, ${90}^{\circ }+\mathrm{\angle }DBC={180}^{\circ }$
Or $\mathrm{\angle }DBC={90}^{\circ }$
Hence, $\mathrm{\angle }DBC$ is a right angle.

Q8 (iii) In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that $DM=CM$ . Point D is joined to point B (see Fig.7.23). Show that: $\mathrm{\Delta }DBC\cong \mathrm{\Delta }ACB$

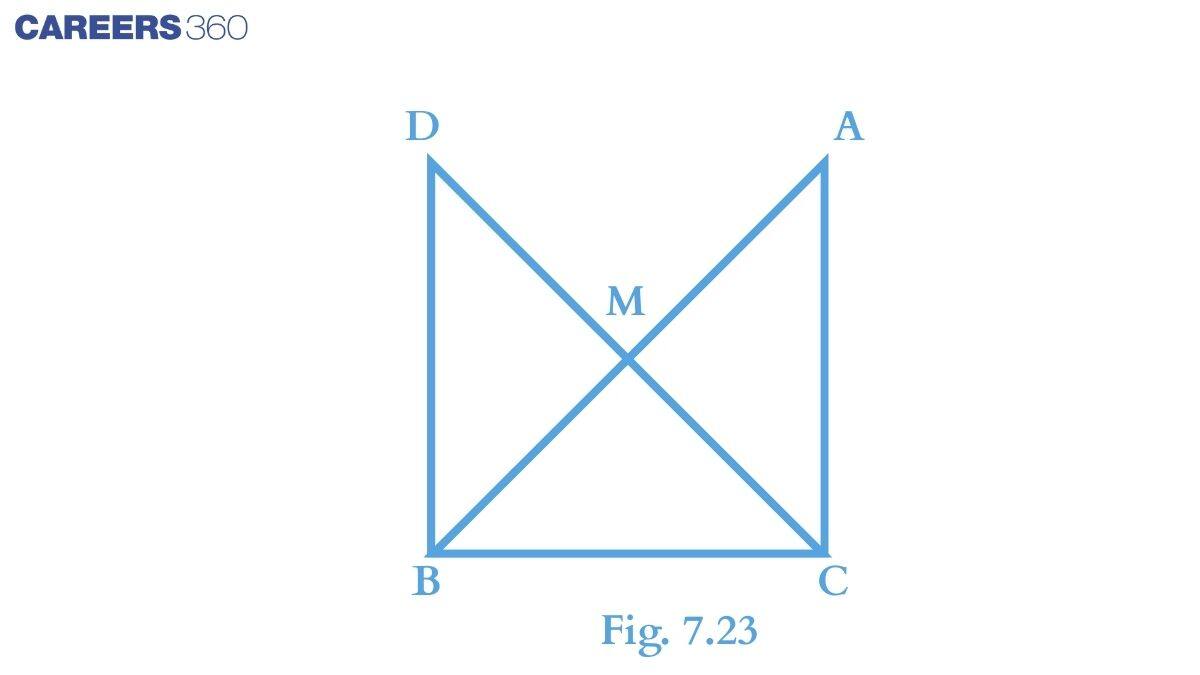
Solution:Consider $\mathrm{\Delta }DBC$ and $\mathrm{\Delta }ACB$,
(i) $BC=BC$ (Common in both the triangles)
(ii) $\mathrm{\angle }ACB=\mathrm{\angle }DBC$ (Right angle)
(iii) $DB=AC$ (By c.p.c.t. from part (a) of the question.)
Thus, SAS congruence we can conclude that:
$\mathrm{\Delta }DBC\cong \mathrm{\Delta }ACB$

Q8 (iv) In right triangle ABC, right angled at C, M is the midpoint of hypotenuse AB. C is joined to M and produced to a point D such that $DM=CM$ . Point D is joined to point B (see Fig.7.23). Show that: $CM=\frac{1}{2}AB$

Solution:

In the previous part, we have proved that

$\mathrm{\Delta }DBC\cong \mathrm{\Delta }ACB$.

Thus by c.p.c.t., we can write : $DC=AB$

$DM+CM=AM+BM$

Or $CM+CM=AB$ (Since M is the midpoint.)

Or $CM=\frac{1}{2}AB$.

Hence proved.