NCERT Solutions for Class 9 Maths Chapter 1 Exercise 1.2

NCERT Solutions for Class 9 Maths Chapter 1 Exercise 1.2 - Number Systems

Edited By Vishal kumar | Updated on May 15, 2025 02:38 PM IST

Through irrational numbers the number system extends into space occupied by values that cannot be classified as fractional numbers. The numeric representations √2 and π both possess extended decimal sequences that never end and never repeat digits. The understanding of different real numbers in the number line becomes clearer through visual representation of their placement. The fundamental number concepts prepared us to tackle additional topics involving roots and exponents together with equations.

New: Get up to 90% Scholarship on NEET/JEE Coaching from top Coaching Institutes

This Story also Contains

NCERT Solutions for Class 9 Maths Chapter 1 – Number Systems Exercise 1.2
Access Solution of Number Systems Class 9 Chapter 1 Exercise: 1.2
Topics covered in Chapter 1 Number System: Exercise 1.2
Also see-
NCERT Solutions of Class 9 Subject Wise
NCERT Exemplar Solutions of Class 9 Subject Wise

NCERT Solutions for Class 9 Maths Chapter 1 Exercise 1.2 - Number Systems

Students who use NCERT Solutions and NCERT Books have a proven method to prepare for irrational numbers and their properties along with operations. The resources simplify challenging concepts by providing solved illustrations and exercise structures which lead to easy subject comprehension. Students derive advantages from each step explained explanations because they strengthen fundamental concepts and improve understanding. Students who refer to these established learning materials will develop their analytical capabilities.

NCERT Solutions for Class 9 Maths Chapter 1 – Number Systems Exercise 1.2

Access Solution of Number Systems Class 9 Chapter 1 Exercise: 1.2

Q1 (i) State whether the following statements are true or false. Justify your answers. (i) Every irrational number is a real number.

Answer:

(i) True
The set of real numbers includes both rational and irrational numbers.

Q1 (ii) State whether the following statements are true or false. Justify your answers. (ii) Every point on the number line is of the form $\sqrt{m}$ , where m is a natural number.

Answer:

(ii) False

Many points on the number line are rational (like 2, $\frac{1}{2}$ , etc.) which are not in the form $\sqrt{m}$ .

Q1 (iii) State whether the following statements are true or false. Justify your answers. (iii) Every real number is an irrational number.

Answer:

(iii) False

Real numbers include both rational and irrational numbers. For example, 3 is a real number but not irrational.

Q2 Are the square roots of all positive integers irrational? If not, give an example of the square root of a number that is a rational number.

Answer:

No, the square roots of all positive integers are not irrational.

Example:

$\sqrt{4}$ = 2, which is a rational number.

Q3 Show how $\sqrt{5}$ can be represented on the number line.

Answer:

Construction method (simplified):

We know that

$\sqrt{5} = \sqrt{(2)^{2} + (1)^{2}}$

Now,

Let OA be a line on the number line that is 2 units long. Next, link OB and build AB of unit length perpendicular to OA.

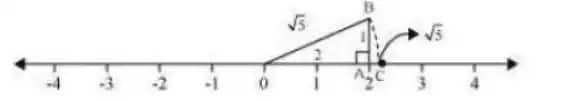
Now, in right angle triangle OAB, by Pythagoras theorem

OB = $\sqrt{(2)^{2} + (1)^{2}} = \sqrt{5}$

Now, draw an arc that intersects the number line at C using O as the center and OB as the radius.

Point C represent $\sqrt{5}$ on a number line.

Also Read:

Topics covered in Chapter 1 Number System: Exercise 1.2

Concept of irrational numbers: Numbers classified as irrational cannot be expressed as fractions with integer values p and q. Numerical forms of irrational numbers hold infinite decimal expansions without repeating patterns.
Difference between rational and irrational numbers: Any number expressed as a fraction will always represent a rational number while producing a terminating or repeating decimal result. Irrational numbers resist fraction representation and expand into endless sequences of digits without repeating patterns.
Properties of irrational numbers: Real numbers include irrationals which cannot be completed as decimal expansions. The combination of a rational number together with an irrational number usually produces an irrational outcome unless one is zero when multiplying.
Representation of irrational numbers on the number line: One can place irrational numbers on the number line through geometrically based methods. People can identify points through right triangle construction followed by a ruler and compass technique.
Use of Pythagoras theorem to construct irrational numbers: Using the Pythagoras theorem enables creation of irrational numbers. The hypotenuse in a right-angled triangle having legs of 1 unit measures the irrational value of $\sqrt{2}$ .

NEET/JEE Offline Coaching

Get up to 90% Scholarship on your NEET/JEE preparation from India’s Leading Coaching Institutes like Aakash, ALLEN, Sri Chaitanya & Others.

Apply Now

Also see-

NCERT Solutions of Class 9 Subject Wise

Students must check the NCERT solutions for class 9 of Mathematics and Science Subjects.

NCERT Exemplar Solutions of Class 9 Subject Wise

Students must check the NCERT Exemplar solutions for class 9 of Mathematics and Science Subjects.

Frequently Asked Questions (FAQs)

1. According to NCERT solutions for Class 9 Maths chapter 1 exercise 1.2 , How are real numbers classified?

Real numbers are the union of both rational and irrational numbers.

2. 1/3 is a ______ number

Thus 1/3 is a rational number.

3. Say true /false: On a number line, a number on the left is always greater than a number on the right.

The given statement is false. A number on the left is always less than a number on the right because the left side of zero was occupied by the negative numbers on the number line.

4. A number on the right is always _______ than a number on the left.

A number on the right is always greater than a number on the left. Since all positive numbers occupy the right side of the zero.

5. How many numbers can be represented in a number line?

Mathematically, number lines extend indefinitely at both ends. So an infinite number can be represented in a number line.

6. Integers are a subset of _______

Integers are a subset of rational numbers.

7. Say true or false: Rational Numbers are a subset of Real Numbers.

Yes, the given statement is true. Rational Numbers and irrational numbers are a subset of Real Numbers. Real numbers are the union of both rational and irrational numbers.

8. What is a number line?

The horizontal straight lines in which the integers are placed in equal intervals are known as number lines.

9. ___ is the middle point of the number line.

0 is the middle point of the number line.

10. In the NCERT solutions for Class 9 Maths chapter 1 exercise 1.2 , how many questions and what types of questions are there?

NCERT solutions for Class 9 Maths chapter 1 exercise 1.2 consists of 4 questions and the questions are based on the concept of rational and irrational numbers, differences regarding rational and irrational numbers and how to identify them, and also how to locate them on a number line.

Also Read

NCERT Solutions for Class 9 Maths Chapter 1 Exercise 1.4 - Number Systems

NCERT Solutions for Class 9 Maths Chapter 1 Exercise 1.3 - Number Systems

NCERT Solutions for Class 9 Maths Chapter 1 Exercise 1.2 - Number Systems

NCERT Solutions for Class 9 Maths Chapter 1 Exercise 1.1 - Number Systems

NCERT Solutions For Class 9 Science Chapter 1 Matter in Our Surroundings

NCERT Solutions for Class 9 Science Chapter 2 Is Matter Around Us Pure

NCERT Class 9 Science Notes - Download Chapter-wise PDFs

NCERT Class 9 Notes - Download Subject Wise PDF Notes

NCERT Class 9 Maths Chapter 5 Notes Introduction To Euclid’s Geometry - Download PDF

NCERT Class 9 Maths Chapter 1 Notes Number System - Download Free PDF

NCERT Class 9 Maths Chapter 6 Notes Lines and Angles - Download PDF

Triangles Class 9th Notes - Free NCERT Class 9th Maths Chapter 7 Notes - Download PDF

NCERT Exemplar Class 9 Solutions - Subject Wise Problems with Solutions

NCERT Exemplar Class 9 Maths Solutions Chapter 2 Polynomials

NCERT Exemplar Class 9 Maths Solutions Chapter 6 Lines and Angles

NCERT Exemplar Class 9 Maths Solutions Chapter 5 Introduction to Euclids Geometry

NCERT Exemplar Class 9 Science Solutions Chapter 15 Improvement in Food Resources

NCERT Exemplar Class 9 Science Solutions Chapter 14 Natural Resources

NCERT Books for Class 9 Maths 2025-26; Download Free PDF

NCERT Books for Class 9 All Subjects - Download Free PDF

NCERT Books for Class 9 Social Science 2025-26: Download PDF

NCERT Books for Class 9 Hindi 2025-26: Download PDF

NCERT Books for Class 9 English 2025-26: Download All Chapters PDF

NCERT Books for Class 9 Science 2025-26; Download PDF (All Chapters)

Articles

FYJC Mumbai Admission 2025: 11th Admission Process, Application Fee, Cut off List @11thadmission.org.in

May 14, 2025

SoSE Delhi School Admission Eligibility Criteria 2025: Requirements & Guidelines

May 10, 2025

SoSE Delhi School Admission 2025: All You Need to Know About Eligibility, Dates & Process

May 10, 2025

BHU SET Answer Key 2025: Download Solutions for Class 6, 9, and 11 Entrance Exam

May 09, 2025

BHU School Entrance Test Admit Card 2025 - Download Class 9, 11 SET Admit Card @ bhuonline.in

May 09, 2025

Medical Diploma Courses After 12th - Eligibility Criteria & Top Institutes

May 09, 2025

UPTAC Counselling 2025: Date, Registration, Seat Allotment, Choice Filling, Document Verification

May 08, 2025

NSSNET Admit Card 2025 Out, Navyug School Entrance NTA Hall ticket

May 05, 2025

Upcoming School Exams

Assam High School Leaving Certificate Examination

Application Date:01 April,2025 - 15 May,2025

Mock Test

Result

Admit Card

National Institute of Open Schooling 10th examination

Admit Card Date:02 April,2025 - 19 May,2025

Result

Application Process

Exam Pattern

National Institute of Open Schooling 12th Examination

Admit Card Date:02 April,2025 - 19 May,2025

Application Process

Exam Pattern

Admit Card

Goa Board Higher Secondary School Certificate Examination

Admit Card Date:17 April,2025 - 17 May,2025

Mock Test

Result

Admit Card

Andhra Pradesh Board of Intermediate Education Examination

Admit Card Date:06 May,2025 - 20 May,2025

Result

Admit Card

Mock Test

View All School Exams

Popular Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms^-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times. Assume that the potential energy lost each time he lowers the mass is dissipated. How much fat will he use up considering the work done only when the weight is lifted up ? Fat supplies 3.8×10⁷J of energy per kg which is converted to mechanical energy with a 20% efficiency rate. Take g = 9.8 ms⁻² :

Option 1)

2.45×10⁻³ kg

Option 2)

6.45×10⁻³ kg

Option 3)

9.89×10⁻³ kg

Option 4)

12.89×10⁻³ kg

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

A particle is projected at 60⁰ to the horizontal with a kinetic energy $K$ . The kinetic energy at the highest point

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

In the reaction,

$2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

How many moles of magnesium phosphate, $Mg_{3}(PO_{4})_{2}$ will contain 0.25 mole of oxygen atoms?

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

With increase of temperature, which of these changes?

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol^-1) is

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t²) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m² , the number of rotations made by the pulley before its direction of motion if reversed, is