ALLEN Coaching
ApplyRegister for ALLEN Scholarship Test & get up to 90% Scholarship
Have you noticed that the things surrounding you, like windows, doors, your notebook or tablet, all have a similar four-sided shape? Well, these four-sided shapes are called quadrilaterals. Quadrilaterals can be of various types, such as a Parallelogram, a Rhombus, a Trapezium, etc. From NCERT Class 9 Maths, the chapter Quadrilaterals contains the concepts of Properties of a Parallelogram, theorems related to angles and sides, the Mid-point Theorem, etc. These concepts will help the students grasp more advanced geometry concepts easily and enhance their problem-solving ability in real-world applications.
This article on NCERT solutions for class 9 Maths Chapter 8 Quadrilaterals offers clear and step-by-step solutions for the exercise problems in the NCERT Books for class 9 Maths. Students who are in need of Quadrilaterals class 9 solutions will find this article very useful. It covers all the important Class 9 Maths Chapter 8 question answers. These Quadrilaterals class 9 ncert solutions are made by the Subject Matter Experts according to the latest CBSE syllabus, ensuring that students can grasp the basic concepts effectively. NCERT solutions for class 9 maths and NCERT solutions for other subjects and classes can be downloaded from the NCERT Solutions.
Quadrilateral:
Sum of all angles = 360°
Parallelogram:
A diagonal of a parallelogram divides it into two congruent triangles.
In a parallelogram, the diagonals bisect each other.
In a parallelogram, opposite angles are equal.
In a parallelogram, opposite sides are equal.
Square:
Diagonals of a square bisect each other at right angles and are equal, and vice versa.
Triangle:
A line through the midpoint of a side of a triangle parallel to another side bisects the third side (Midpoint theorem).
The line segment joining the midpoints of two sides of a triangle is parallel to the third side and equal to half the third side.
Parallelogram Angle Bisectors:
In a parallelogram, the bisectors of any two consecutive angles intersect at a right angle.
If a diagonal of a parallelogram bisects one of the angles of a parallelogram, it also bisects the second angle.
Rectangle:
The angle bisectors of a parallelogram form a rectangle.
Each of the four angles of a rectangle is a right angle.
Rhombus:
The diagonals of a rhombus are perpendicular to each other.
Class 9 maths chapter 8 NCERT solutions - Exercise: 8.1
Page number: 110-111, Total questions: 7
Q1. If the diagonals of a parallelogram are equal, then show that it is a rectangle.
Answer:
Given: ABCD is a parallelogram with AC=BD.
To prove: ABCD is a rectangle.
Proof : In
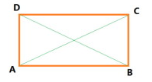
BC= AD (Opposite sides of parallelogram)
AC=BD (Given)
AB=AB (common)
and
Hence, it is a rectangle.
Q2. Show that the diagonals of a square are equal and bisect each other at right angles.
Answer:
Given : ABCD is a square i.e. AB=BC=CD=DA.
To prove : the diagonals of a square are equal and bisect each other at right angles i.e. AC=BD,AO=CO,BO=DO and
Proof : In
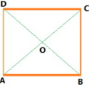
AD=BC (Given )
AB=AB (common)
BD=AC (CPCT)
In
AB=CD (Given )
AO=OC ,BO=OD (CPCT)
In
OB=OD (proved above)
AB=AD (Given )
OA=OA (COMMON)
2.
Hence, the diagonals of a square are equal and bisect each other at right angles.
Q3. (i) Diagonal AC of a parallelogram ABCD bisects
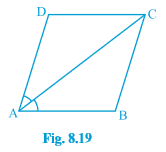
Answer:
Given:
From equation 1,2 and 3, we get
Hence, diagonal AC bisect angle C also.
Q3. (ii) Diagonal AC of a parallelogram ABCD bisects
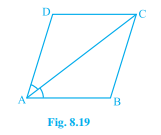
Answer:
Given:
From equations 1,2, and 3, we get
From 2 and 4, we get
In
AD=DC (In a triangle,sides opposite to equal angle are equal)
A parallelogram whose adjacent sides are equal , is a rhombus.
Thus, ABCD is a rhombus.
Q4. (i) ABCD is a rectangle in which diagonal AC bisects
Answer:
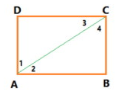
Given: ABCD is a rectangle with AB=CD and BC=AD
To prove: ABCD is a square.
Proof :
From 1 and 2,
In
DC=AD (In a triangle, sides opposite to equal angle are equal )
A rectangle whose adjacent sides are equal is a square.
Hence, ABCD is a square.
Q4. (ii) ABCD is a rectangle in which diagonal AC bisects
Answer: 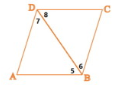
In
AD = AB (ABCD is a square)
From 1 and 2, we have
and
From 1 and 4, we get
Hence, from 3 and 5, diagonal BD bisects angles B as well as angle D.
Q5. (i) In parallelogram ABCD, two points P and Q are taken on diagonal BD such that
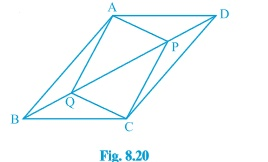
Answer:
Given: In parallelogram ABCD, two points P and Q are taken on diagonal BD such that
To prove :
Proof :
In
DP=BQ (Given )
AD=BC (opposite sides of a parallelogram)
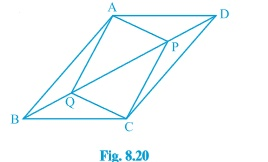
Q5. (ii) In parallelogram ABCD, two points P and Q are taken on diagonal BD such that
Answer:
Given: In parallelogram ABCD, two points P and Q are taken on diagonal BD such that
To prove :
Proof :
In
DP=BQ (Given )
AD=BC (opposite sides of a parallelogram)
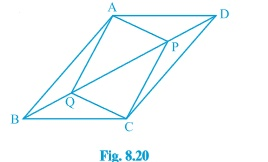
Q5. (iii) In parallelogram ABCD, two points P and Q are taken on diagonal BD such that
Answer:
Given: In parallelogram ABCD, two points P and Q are taken on diagonal BD such that
To prove :
Proof :
In
DP=BQ (Given )
AB=CD (opposite sides of a parallelogram)
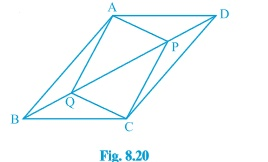
Q5. (iv) In parallelogram ABCD, two points P and Q are taken on diagonal BD such that
Answer:
Given: In parallelogram ABCD, two points P and Q are taken on diagonal BD such that
To prove :
Proof :
In
DP=BQ (Given )
AB=CD (opposite sides of a parallelogram)
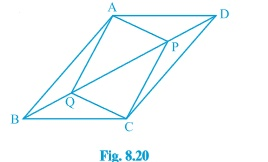
Answer:
Given: In parallelogram ABCD, two points P and Q are taken on diagonal BD such that
To prove: APCQ is a parallelogram
Proof :
In
DP=BQ (Given )
AD=BC (opposite sides of a parallelogram)
Also,
In
DP=BQ (Given )
AB=CD (opposite sides of a parallelogram)
From equation 1 and 2, we get
Thus, opposite sides of quadrilateral APCQ are equal so APCQ is a parallelogram.
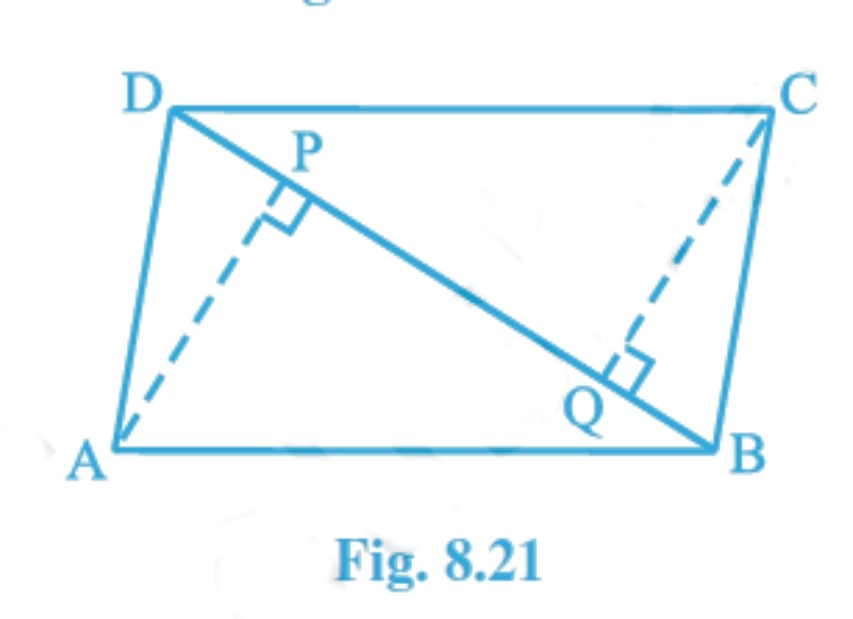
Answer:
Given: ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD.
To prove :
Proof: In
AB=CD (Opposite sides of a parallelogram )
Thus,
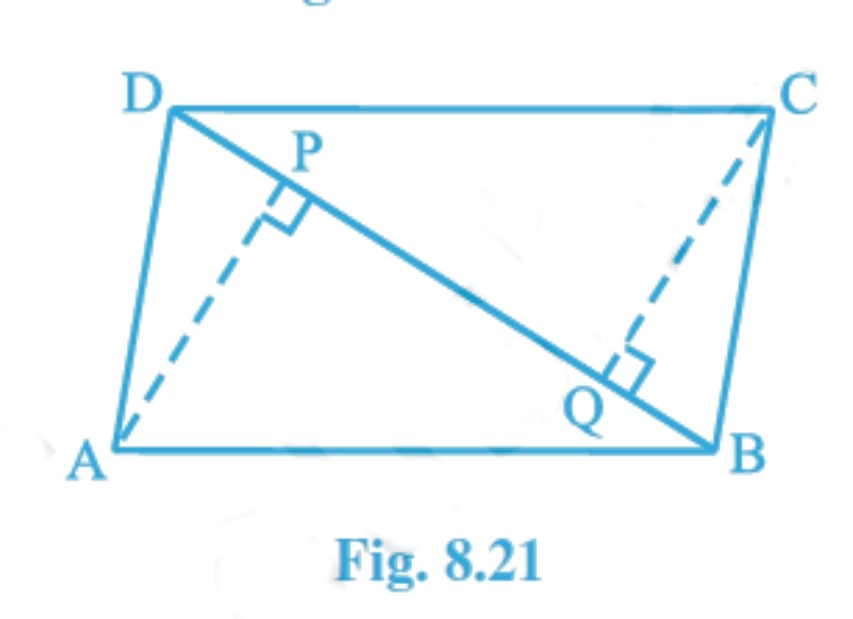
Answer:
Given: ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD.
To prove :
Proof: In
AB=CD (Opposite sides of a parallelogram )
Thus,
Q7. (i) ABCD is a trapezium in which
[Hint: Extend AB and draw a line through C parallel to DA intersecting AB produced at E.]
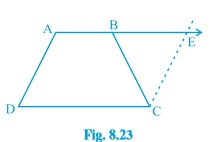
Answer:
Given: ABCD is a trapezium in which
To prove :
Proof: Let
In AECD,
AE||DC (Given)
AD||CE (By construction)
Hence, AECD is a parallelogram.
AD=CE...............1(opposite sides of a parallelogram)
AD=BC.................2(Given)
From 1 and 2, we get
CE=BC
In
From 4 and 5, we get
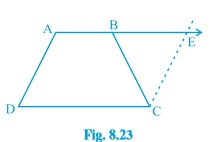
Q7. (ii) ABCD is a trapezium in which AB || CD and AD = BC (see Fig. 8.23). Show that
[Hint: Extend AB and draw a line through C parallel to DA intersecting AB produced at E.]
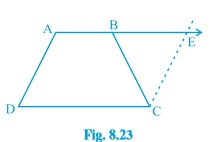
Answer:
Given: ABCD is a trapezium in which
To prove :
Proof: Let
Thus,
Q7. (iii) ABCD is a trapezium in which
[Hint: Extend AB and draw a line through C parallel to DA intersecting AB produced at E.]
Answer:
Given: ABCD is a trapezium in which
To prove :
Proof: In
BC=AD (Given )
AB=AB (Common )
Thus,
Q7. (iv) ABCD is a trapezium in which
[Hint: Extend AB and draw a line through C parallel to DA intersecting AB produced at E.]
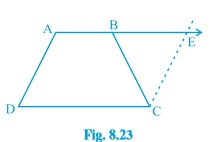
Answer:
Given: ABCD is a trapezium in which
To prove: diagonal AC
Proof: In
BC=AD (Given )
AB=AB (Common )
Thus,
diagonal AC
Class 9 maths chapter 8 question answer - Exercise: 8.2
Page Number: 113-114, Total Questions: 6
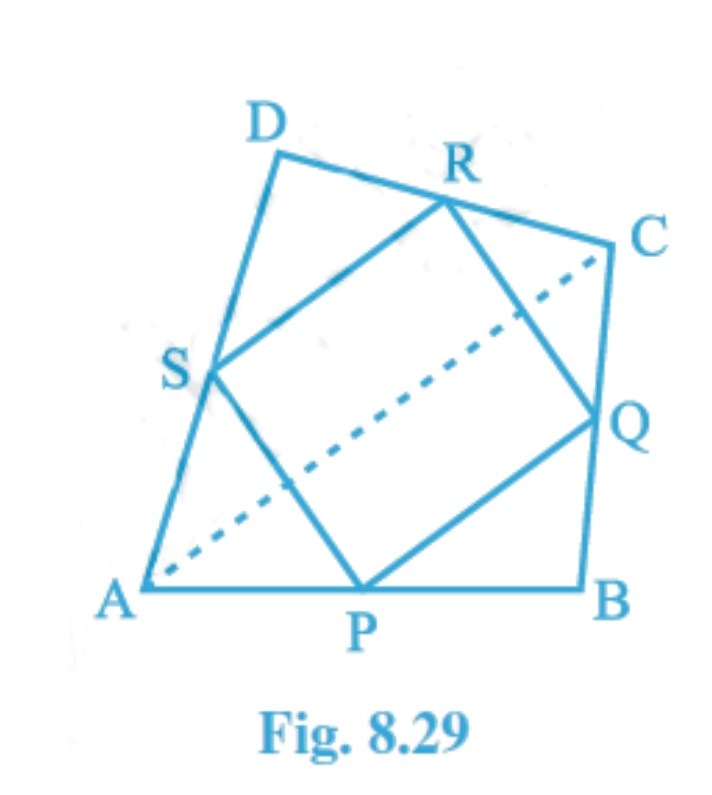
Answer:
Given: ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see Fig.
To prove :
Proof: In
S is the midpoint of DA. (Given)
R is the midpoint of DC. (Given)
By midpoint theorem,
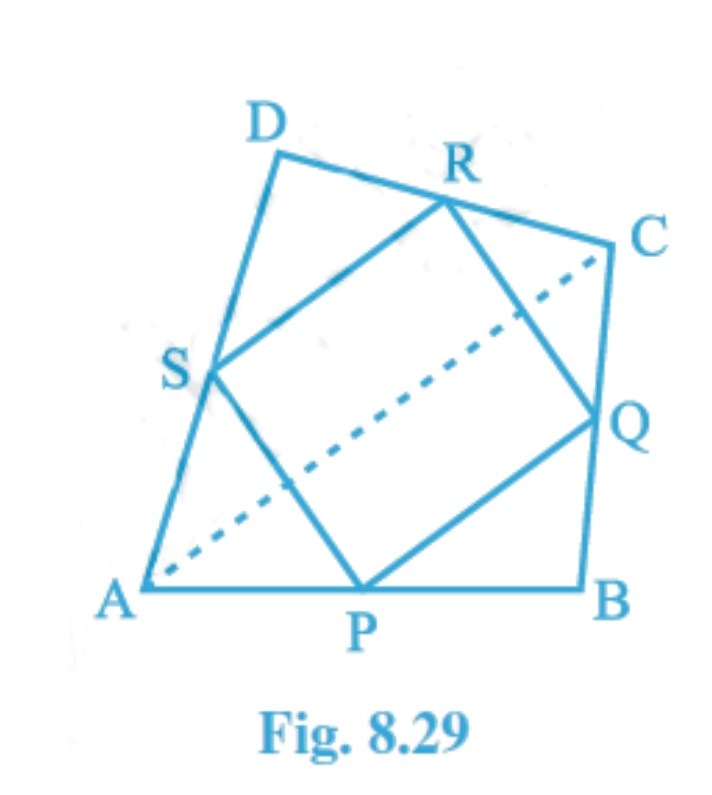
Answer:
Given: ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see Fig.
To prove :
Proof : In
S is mid point of DA. (Given)
R is mid point of DC. (Given)
By mid-point theorem,
In
P is mid point of AB. (Given)
Q is mid point of BC. (Given)
By mid-point theorem,
From 1 and 2,we get
Thus,
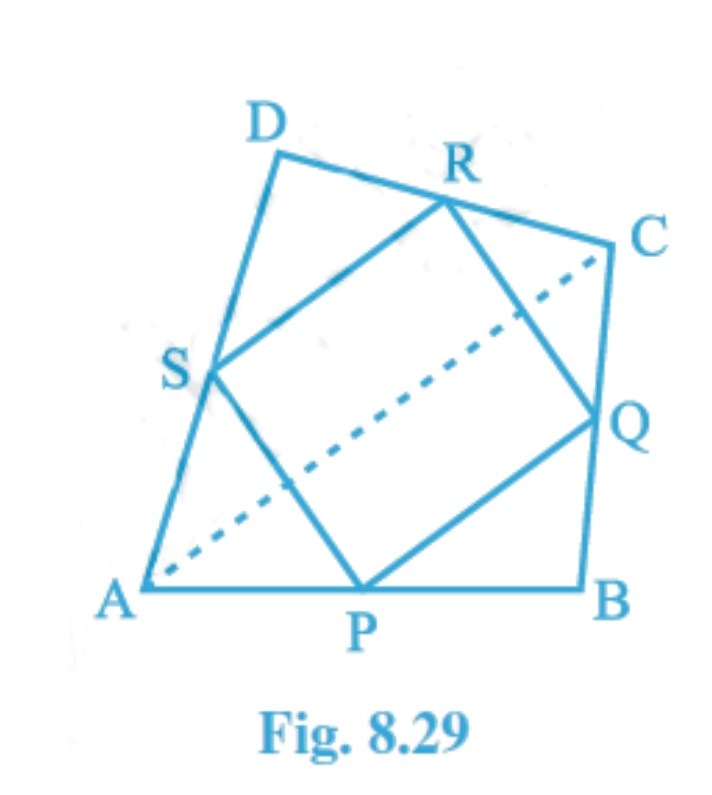
Answer:
Given : ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see Fig
To prove : PQRS is a parallelogram.
Proof : In PQRS,
Since,
So,PQRS is a parallelogram.
Answer:
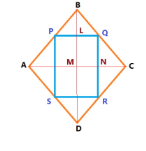
Given: ABCD is a rhombus in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA . AC, BD are diagonals.
To prove: the quadrilateral PQRS is a rectangle.
Proof: In
S is midpoint of DA. (Given)
R is midpoint of DC. (Given)
By midpoint theorem,
In
P is midpoint of AB. (Given)
Q is mid point of BC. (Given)
By mid point theorem,
From 1 and 2,we get
Thus,
So,the quadrilateral PQRS is a parallelogram.
Similarly, in
Q is mid point of BC. (Given)
R is mid point of DC. (Given)
By mid point theorem,
So, QN || LM ...........5
LQ || MN ..........6 (Since, PQ || AC)
From 5 and 6, we get
LMPQ is a parallelogram.
Hence,
But,
so,
Thus, a parallelogram whose one angle is a right angle is a rectangle.
Hence, PQRS is a rectangle.
Answer:
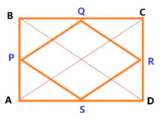
Given: ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively.
To prove: the quadrilateral PQRS is a rhombus.
Proof :
In
S is the midpoint of DA. (Given)
R is the midpoint of DC. (Given)
By midpoint theorem,
In
P is the midpoint of AB. (Given)
Q is the midpoint of BC. (Given)
By midpoint theorem,
From 1 and 2, we get
Thus,
So, the quadrilateral PQRS is a parallelogram.
Similarly, in
Q is the midpoint of BC. (Given)
R is the midpoint of DC. (Given)
By midpoint theorem,
AC = BD.......................6(diagonals )
From 2, 5 and 6, we get
PQ=QR
Thus, a parallelogram whose adjacent sides are equal is a rhombus. Hence, PQRS is a rhombus.
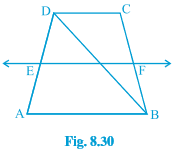
Answer:
Given: ABCD is a trapezium in which
To prove: F is the mid-point of BC.
In
E is the midpoint of AD. (Given)
EG || AB (Given)
By converse of midpoint theorem,
G is the midpoint of BD.
In
G is mid point of BD. (Proved above)
FG || DC (Given)
By converse of midpoint theorem,
F is the midpoint of BC.
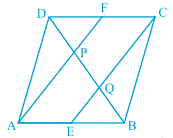
Answer:
Given: In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively
To prove: the line segments AF and EC trisect the diagonal BD.
Proof : In quadrilatweral ABCD,
AB=CD (Given)
In quadrilateral AECF,
AE=CF (Given)
AE || CF (Opposite sides of a parallelogram)
Hence, AECF is a parallelogram.
In
F is the midpoint of DC. (given )
FP || CQ (AECF is a parallelogram)
By converse of midpoint theorem,
P is the mid point of DQ.
DP= PQ....................1
Similarly,
In
E is the midpoint of AB. (given )
EQ || AP (AECF is a parallelogram)
By converse of midpoint theorem,
Q is the midpoint of PB.
OQ= QB....................2
From 1 and 2, we have
DP = PQ = QB.
Hence, the line segments AF and EC trisect the diagonal BD.
Answer:
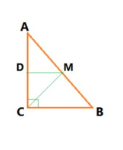
Given: ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D.
To prove :D is mid point of AC.
Proof: In
M is mid point of AB. (Given)
DM || BC (Given)
By converse of mid point theorem,
D is the mid point of AC.
Answer:
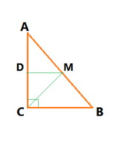
Given: ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallels to BC intersects AC at D.
To prove :
Proof :
Hence,
Answer:
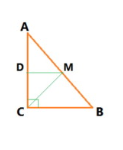
Given: ABC is a triangle right angled at C. A line through the mid-point M of the hypotenuse AB and parallel to BC intersects AC at D.
To prove :
Proof : In
M is the midpoint of AB. (Given)
DM || BC (Given)
By the converse of the midpoint theorem,
D is the midpoint of AC i.e. AD = DC.
In
AD = DC (proved above)
DM = DM (Common)
AM = CM (CPCT)
But ,
Hence,
Students can practice Class 9 Maths Chapter 8 question answers using the exercise link given below.
Here are the subject-wise links for the NCERT solutions of class 9:
Given below are some useful links for NCERT books and the NCERT syllabus for class 9:
Angle sum property of a quadrilateral, properties of the parallelogram, another condition for a quadrilateral to be a parallelogram, and mid-point theorem are the important topics covered in class 9th quadrilateral solution. students can practice these NCERT solutions for class 9 to command the concepts.
NCERT solutions are not only helpful for the students if they stuck while solving NCERT problems but also it will provide them new ways to solve the problems. These solutions are provided in a very detailed manner which will give them conceptual clarity.
Quadrilateral class 9 solutions define a quadrilateral as a two-dimensional shape that has four sides or edges and four corners or vertices. Quadrilaterals are commonly recognized by their standard shapes, such as rectangle, square, trapezoid, and kite, but they can also have irregular and undefined shapes.
Here you will get the detailed NCERT solutions for class 9 by clicking on the link. for ease students can study quadrilateral class 9 pdf both online and offline mode.

Register for ALLEN Scholarship Test & get up to 90% Scholarship

Get up to 90% Scholarship on Offline NEET/JEE coaching from top Institutes

This ebook serves as a valuable study guide for NEET 2025 exam.

This e-book offers NEET PYQ and serves as an indispensable NEET study material.
As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
As per latest 2024 syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters