NCERT Solutions for Exercise 12.1 Class 9 Maths Chapter 12

NCERT Solutions for Exercise 12.1 Class 9 Maths Chapter 12 - Heron's Formula

Edited By Vishal kumar | Updated on Oct 11, 2023 08:09 AM IST

NCERT Solutions for Class 9 Maths Chapter 12: Heron's Formula Exercise 12.1- Free PDF Download

NCERT Solutions for Class 9 Maths Chapter 12: Heron's Formula Exercise 12.1- Heron's Formula will be unlocked in 9th class maths exercise 12.1 answers. When you know the lengths of a triangle's sides, you can use this formula to get its area.

To begin, do class 9 maths chapter 12 exercise 12.1, which is similar to the first stage in this adventure. It tests you with tasks that require you to compute triangle areas using Heron's Formula. What's more, guess what? The solutions to these problems are available in PDF format for free. This means you can access them even when you're not connected to the internet, making your journey through triangles and Heron's Formula even more accessible.

Don't Miss: JEE Main 2027: Narayana Scholarship Test Preparation Kit for Class 9

This Story also Contains

NCERT Solutions for Class 9 Maths Chapter 12: Heron's Formula Exercise 12.1- Free PDF Download
Download PDF of NCERT Solutions for Class 9 Maths Chapter 12 – Heron’s Formula Exercise 12.1
Access Heron's Formula C lass 9 Chapter 12 Exercise: 12.1
More Information About NCERT Solutions for Class 9 Maths Exercise 12.1
Benefits of NCERT Solutions for Class 9 Maths Exercise 12.1
Key Features of Exercise 12.1 Class 9 Maths
Also, see-
NCERT Solutions of Class 10 Subject Wise
Subject Wise NCERT Exemplar Solutions

NCERT Solutions for Exercise 12.1 Class 9 Maths Chapter 12 - Heron's Formula

NCERT Solutions for Class 9 Maths chapter 12 exercise 12.1 is about what the heron formula is and to use and apply heron formula in questions and problems. The basic idea of NCERT solutions for exercise 12.1 class 9 maths is that If the quadrilaterals are broken into triangular portions, Heron's formula may also be used to calculate the area of a quadrilateral. Apply Heron's formula to find the area of individual triangular portions once the quadrilaterals have been separated into triangular shapes. After you've calculated the areas of each triangle portion, add them all up to get the quadrilateral's area.

Heron's formula Exercise 12.2

**As per the CBSE Syllabus for 2023-24, please note that this chapter has been renumbered as Chapter 10. So, when referring to this chapter, consider it as Chapter 10 - Heron's Formula.

Download PDF of NCERT Solutions for Class 9 Maths Chapter 12 – Heron’s Formula Exercise 12.1

Download PDF

Access Heron's Formula C lass 9 Chapter 12 Exercise: 12.1

Q1 A traffic signal board, indicating ‘SCHOOL AHEAD’, is an equilateral triangle with side ‘ a ’. Find the area of the signal board, using Heron’s formula. If its perimeter is 180 cm, what will be the area of the signal board?

Answer:

Given the perimeter of an equilateral triangle is 180cm.

So, $3a = 180\ cm$ or $a = 60\ cm$ .

Hence, the length of the side is 60cm.

Now,

Calculating the area of the signal board by the Heron's Formula:

$A = \sqrt{s(s-a)(s-b)(s-c)}$

Where, s is the half-perimeter of the triangle and a, b and c are the sides of the triangle.

Therefore,

$s = \frac{1}{2}Perimeter = \frac{1}{2}180cm = 90cm$

$a =b=c = 60cm$ as it is an equilateral triangle.

Substituting the values in the Heron's formula, we obtain

$\implies A = \sqrt{90(90-60)(90-60)(90-60)} = 900\sqrt{3}\ cm^2$ .

Q2 The triangular side walls of a flyover have been used for advertisements. The sides of the walls are 122 m, 22 m and 120 m (see Fig. 12.9). The advertisements yield an earning of Rs. 5000 per m ² per year. A company hired one of its walls for 3 months. How much rent did it pay?

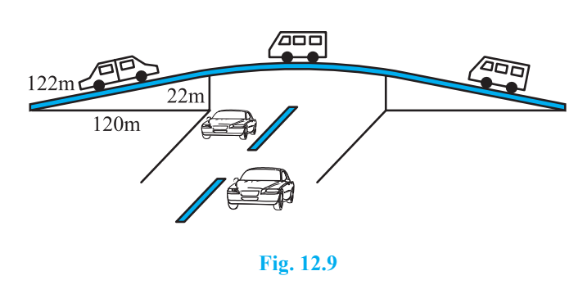
1640600558297 Answer:

From the figure,

The sides of the triangle are:

$a = 122m,\ b = 120m\ and\ c = 22m$

The semi perimeter, s will be

$s = \frac{a+b+c}{2} = \frac{122+120+22}{2} = \frac{264}{2} = 132m$

Therefore, the area of the triangular side wall will be calculated by the Heron's Formula,

$A = \sqrt{s(s-a)(s-b)(s-c)}$

$= \sqrt{132(132-122)(132-120)(132-22)}\ m^2$

$= \sqrt{132(10)(12)(110)}\ m^2$

$= \sqrt{(12\times11)(10)(12)(11\times10)}\ m^2 = 1320\ m^2$

Given the rent for 1 year (i.e., 12 months) per meter square is Rs. 5000.

Rent for 3 months per meter square will be:

$Rs.\ 5000\times \frac{3}{12}$

Therefore, for 3 months for 1320 m ² :

$Rs.\ 5000\times \frac{3}{12}\times 1320 = Rs.\ 16,50,000.$

Q3 There is a slide in a park. One of its side walls has been painted in some colour with a message “KEEP THE PARK GREEN AND CLEAN” (see Fig. 12.10 ). If the sides of the wall are 15 m, 11 m and 6 m, find the area painted in colour.

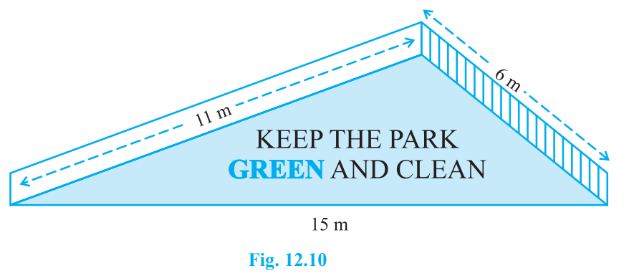
1640600584782 Answer:

Given the sides of the triangle are:

$a = 15 m,\ b= 11m\ and\ c= 6m.$

So, the semi perimeter of the triangle will be:

$s = \frac{a+b+c}{2} = \frac{15+11+6}{2} = \frac{32}{2} = 16m$

Therefore, Heron's formula will be:

$A = \sqrt{s(s-a)(s-b)(s-c)}$

$= \sqrt{16(16-15)(16-11)(16-6)}$

$= \sqrt{16(1)(5)(10)}$

$= \sqrt{(4\times 4)(1)(5)(5\times 2 )}$

$= 4\times 5 \sqrt2 = 20\sqrt2\ m^2$

Hence, the area painted in colour is $20\sqrt2\ m^2$ .

Q4 Find the area of a triangle two sides of which are 18cm and 10cm and the perimeter is 42cm.

Answer:

Given the perimeter of the triangle is $42cm$ and the sides length $a= 18cm$ and $b= 10cm$

So, $a+b+c = 42cm$

Or, $c = 42 - 18-10 = 14cm$

So, the semi perimeter of the triangle will be:

$s = \frac{P}{2} = \frac{42cm}{2} = 21cm$

Therefore, the area given by the Heron's Formula will be,

$A = \sqrt{s(s-a)(s-b)(s-c)}$

$= \sqrt{21(21-18)(21-10)(21-14)}$

$= \sqrt{(7\times3 )(3)(11)(7)}$

$= 21\sqrt{11}\ cm^2$

Hence, the area of the triangle is $21\sqrt{11}\ cm^2.$

Q5 Sides of a triangle are in the ratio of 12 : 17 : 25 and its perimeter is 540cm. Find its area.

Answer:

Given the sides of a triangle are in the ratio of $12:17:25$ and its perimeter is $540cm$

Let us consider the length of one side of the triangle be $a = 12x$

Then, the remaining two sides are $b = 17x$ and $c = 25x.$

So, by the given perimeter, we can find the value of x:

$Perimeter = a+b+c = 12x+17x+25x = 540cm$

$\implies 54x = 540cm$

$\implies x = 10$

So, the sides of the triangle are:

$a = 12\times10 =120 cm$

$b = 17\times10 =170 cm$

$c = 25\times10 =250 cm$

So, the semi perimeter of the triangle is given by

$s = \frac{540cm}{2} = 270cm$

Therefore, using Heron's Formula, the area of the triangle is given by

$A = \sqrt{s(s-a)(s-b)(s-c)}$

$= \sqrt{270(270-120)(270-170)(270-250)}$

$= \sqrt{270(150)(100)(20)}$

$= \sqrt{81000000} = 9000cm^2$

Hence, the area of the triangle is $9000cm^2$ .

Q6 An isosceles triangle has perimeter 30 cm and each of the equal sides is 12 cm. Find the area of the triangle.

Answer:

The perimeter of an isosceles triangle is 30 cm (Given).

The length of the sides which are equal is12 cm.

Let the third side length be 'a cm'.

Then, $Perimeter = a+b+c$

$\Rightarrow 30= a+12+12$

$\Rightarrow a = 6cm$

So, the semi-perimeter of the triangle is given by,

$s= \frac{1}{2}Perimeter =\frac{1}{2}\times30cm = 15cm$

Therefore, using Herons' Formula, calculating the area of the triangle

$A = \sqrt{s(s-a)(s-b)(s-c)}$

$= \sqrt{15(15-6)(15-12)(15-12)}$

$= \sqrt{15(9)(3)(3)}$

$= 9\sqrt{15}\ cm^2$

Hence, the area of the triangle is $9\sqrt{15}cm^2.$

Benefits of NCERT Solutions for Class 9 Maths Exercise 12.1

Class 9 Mathematics chapter 12 activity 12.1 offers the basics of the knowledge we'll need to go on to Class 9 Maths chapter 12 exercise 12.2.
Heron's formula for calculating the perimeter or area of a triangle does not rely on the area formula that uses base and height.
Heron's formula is also useful in trigonometry, as it can be used to calculate the law of cosines or the law of cotangents, among other things.
In contrast to previous triangle area calculations, the heron formula does not need you to compute angles or other distances in the triangle first.

Key Features of Exercise 12.1 Class 9 Maths

Introduction to Heron's Formula: Class 9 maths ex 12.1 introduces students to Heron's Formula, a vital concept for finding the area of triangles when the lengths of their sides are known.
Step-by-Step Solutions: NCERT Solutions for class 9 ex 12.1 provide detailed, step-by-step explanations for each problem, helping students understand and apply Heron's Formula effectively.
Practical Application: The ex 12.1 class 9 offers problems that require students to calculate the areas of triangles, providing real-world applications of the formula.
Clear and Understandable Language: Class 9 ex 12.1 solutions are written in clear and understandable language to make the concepts accessible to students.
PDF Format: Solutions are available in PDF format for free download, allowing students to access them offline, enhancing learning flexibility.
Preparation for Advanced Geometry: Heron's Formula is a fundamental concept that prepares students for more advanced topics in geometry they will encounter in higher classes.

Also, see-

NCERT Solutions of Class 10 Subject Wise

Subject Wise NCERT Exemplar Solutions

Frequently Asked Questions (FAQs)

1. How many types of triangle are there used in NCERT solutions for Class 9 Maths exercise 12.1 ?

There are three types of triangle

Right-angled triangle
Isosceles triangle
Scalene triangle

2. How do we find the area of a right-angled triangle according to NCERT solutions for Class 9 Maths exercise 12.1?

When the triangle is right-angled, we may use two sides containing the right angle as the base and height to apply the formula directly. these two sides are base and perpendicular

Area of a right-angled triangle = 0.5 × perpendicular × base

3. How do we find the area of isosceles triangle according to NCERT solutions for Class 9 Maths exercise 12.1?

As two sides of an isosceles triangle are equal, we find the midpoint of the non-equal side of the triangle. Then we connect that midpoint to the vertex above it, and the isosceles triangle is divided into two right-angle triangles. We can then get the area of each right-angle triangle and sum them to get the area of the isosceles triangle.

4. How do we find the area of a equilateral triangle triangle according to NCERT solutions for Class 9 Maths exercise 12.1?

As the equilateral triangle has all sides equal so it is easy to find the midpoint of the base side. Then we join that midpoint with the vertex above and this is how equilateral triangles get separated into two right-angle triangles then we can find the area of both right-angle triangles and then add them so as to get the area of an equilateral triangle

5. Give some real life example used throughout exercise 12.1 Class 9 Maths.

Making the traffic board

Creating posters for advertisement

Area of a slide to get the quantity of paint required

6. What is the unit of measurement for length or breadth are taken in exercise 12.1 Class 9 Maths?

The metre (m) or centimetre (cm) are the units of measurement for length and width, respectively.

7. What is the unit of measurement for unit of measurement for area of any plane are taken in exercise 12.1 Class 9 Maths?

The area of any flat figure is measured in square metres (m^2), square centimetres (cm^2)

8. What kinds of questions do NCERT solutions for Class 9 Maths exercise 12.1 cover?

Exercise 12.1 Class 9 Maths covers all types of basic questions in which we have to apply the heron formula on triangles making real-life examples out of the question that make them interesting to solve but basic premise to apply formula only.

Also Read

NCERT Solutions for Class 9 - Subject-wise PDFs Updated for 2024-25

NCERT Solutions for Class 9 Science

NCERT Solutions for Class 9 Maths

NCERT Syllabus for Class 9 Maths 2023 - Download Syllabus Pdf

NCERT Solutions For Class 9 Science Chapter 1 Matter in Our Surroundings

NCERT Solutions for Exercise 2.5 Class 9 Maths Chapter 2 Polynomials

NCERT Class 9 Maths Notes - Download Chapter Wise PDFs

NCERT Class 9 Maths Chapter 8 Notes Quadrilaterals - Download PDF

NCERT Class 9 Maths Chapter 2 Notes Polynomials - Download PDF

NCERT Class 9 Maths Chapter 1 Notes Number System - Download Free PDF

NCERT Class 9 Maths Chapter 5 Notes Introduction To Euclid’s Geometry - Download PDF

NCERT Class 9 Maths Chapter 6 Notes Lines and Angles - Download PDF

NCERT Exemplar Class 9 Maths Solutions - Chapter Wise Problems with Solutions

NCERT Exemplar Class 9 Solutions - Subject Wise Problems with Solutions

NCERT Exemplar Class 9 Science Solutions Chapter 15 Improvement in Food Resources

NCERT Exemplar Class 9 Science Solutions Chapter 14 Natural Resources

NCERT Exemplar Class 9 Science Solutions Chapter 13 Why do we fall ill?

NCERT Exemplar Class 9 Science Solutions Chapter 12 Sound

Articles

How to Make APAAR ID via DigiLocker and School: A Complete Guide

Dec 17, 2024

Sainik School Entrance Exam 2025: Application, Exam Date, How to Apply, Syllabus

Dec 17, 2024

MVPP Scholarship Exam 2024: Mukhyamantri Vigyan Pratibha Pariksha Registration, Exam Date

Dec 16, 2024

Silverzone ISSO Answer Key 2024-25: Download PDF

Dec 16, 2024

Silverzone IOEL Answer Key 2024 – Download International Olympiad of English Language Solutions

Dec 16, 2024

Silverzone Olympiad IOM Answer Key 2024-25: Qualifying Marks, Score

Dec 16, 2024

Silverzone STEM Olympiad Answer Key 2024-25: Check Answer Key for all Classes

Dec 16, 2024

Silverzone ABHO Answer Key 2024 | Download Akhil Bhartiya Hindi Olympiad Solutions

Dec 16, 2024

Upcoming School Exams

Bihar Open Board 10th Examination

Application Date:11 November,2024 - 10 January,2025

Admit Card

Result

Bihar Open Board 12th Examination

Application Date:11 November,2024 - 10 January,2025

Admit Card

Result

National Means Cum-Merit Scholarship

Admit Card Date:27 November,2024 - 22 December,2024

Eligibility Criteria

Application Process

Exam Pattern

Gujarat Board Higher Secondary School Certificate

Late Fee Application Date:13 December,2024 - 22 December,2024

Exam Pattern

Result

Admit Card

Madhya Pradesh State Open School 10th Examination

Admit Card Date:13 December,2024 - 31 December,2024

Admit Card

Result

View All School Exams

Get answers from students and experts

Popular Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms^-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times. Assume that the potential energy lost each time he lowers the mass is dissipated. How much fat will he use up considering the work done only when the weight is lifted up ? Fat supplies 3.8×10⁷J of energy per kg which is converted to mechanical energy with a 20% efficiency rate. Take g = 9.8 ms⁻² :

Option 1)

2.45×10⁻³ kg

Option 2)

6.45×10⁻³ kg

Option 3)

9.89×10⁻³ kg

Option 4)

12.89×10⁻³ kg

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

A particle is projected at 60⁰ to the horizontal with a kinetic energy $K$ . The kinetic energy at the highest point

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

In the reaction,

$2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

How many moles of magnesium phosphate, $Mg_{3}(PO_{4})_{2}$ will contain 0.25 mole of oxygen atoms?

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

With increase of temperature, which of these changes?

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol^-1) is

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t²) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m² , the number of rotations made by the pulley before its direction of motion if reversed, is