NCERT Solutions for Exercise 13.3 Class 9 Maths Chapter 13

NCERT Solutions for Exercise 13.3 Class 9 Maths Chapter 13 - Surface Area and Volumes

Edited By Vishal kumar | Updated on Oct 13, 2023 11:26 AM IST

NCERT Solutions for Class 9 Maths Exercise 13.3 Chapter 13 Surface Areas and Volumes- Download Free PDF

NCERT Solutions for Class 9 Maths exercise 13.3 deals with the concept of the right circular cone and it’s surface areas . A three-dimensional shape which narrows smoothly from a flat base to a point is known as cone. Mathematically, there are two types of cones namely right circular cone and oblique cone. In exercise 13.3 Class 9 Maths of NCERT Solution, a type of cone whose axis falls perpendicular on the plane of the base is known as the right circular cone. The distance from the vertex or apex to the point on the outer line of the circular base of the cone is known as slant height which is derived from the Pythagoras Theorem. The formula for calculating the slant height of right circular cone is l²=r²+h², from the formula l can be calculated. The surface area of a right circular cone is the area covered by the surface of the right circular cone. Surface area can be divided into two categories. They are

Don't Miss: JEE Main 2027: Narayana Scholarship Test Preparation Kit for Class 9

This Story also Contains

NCERT Solutions for Class 9 Maths Exercise 13.3 Chapter 13 Surface Areas and Volumes- Download Free PDF
Download the PDF of NCERT Solutions for Class 9 Maths Chapter 13 Surface Areas and Volumes Exercise 13.3
Access Surface Area and Volumes Class 9 Chapter 13 Exercise: 13.3
More About NCERT Solutions for Class 9 Maths Exercise 13.3: Surface Area and Volumes – right circular cone
Benefits of NCERT Solutions for Class 9 Maths Exercise 13.3 :
Key Features of 9th Class Maths Exercise 13.3 Answers
NCERT Solutions of Class 10 Subject Wise

NCERT Solutions for Exercise 13.3 Class 9 Maths Chapter 13 - Surface Area and Volumes

Area of Lateral Surface
Area of Total Surface

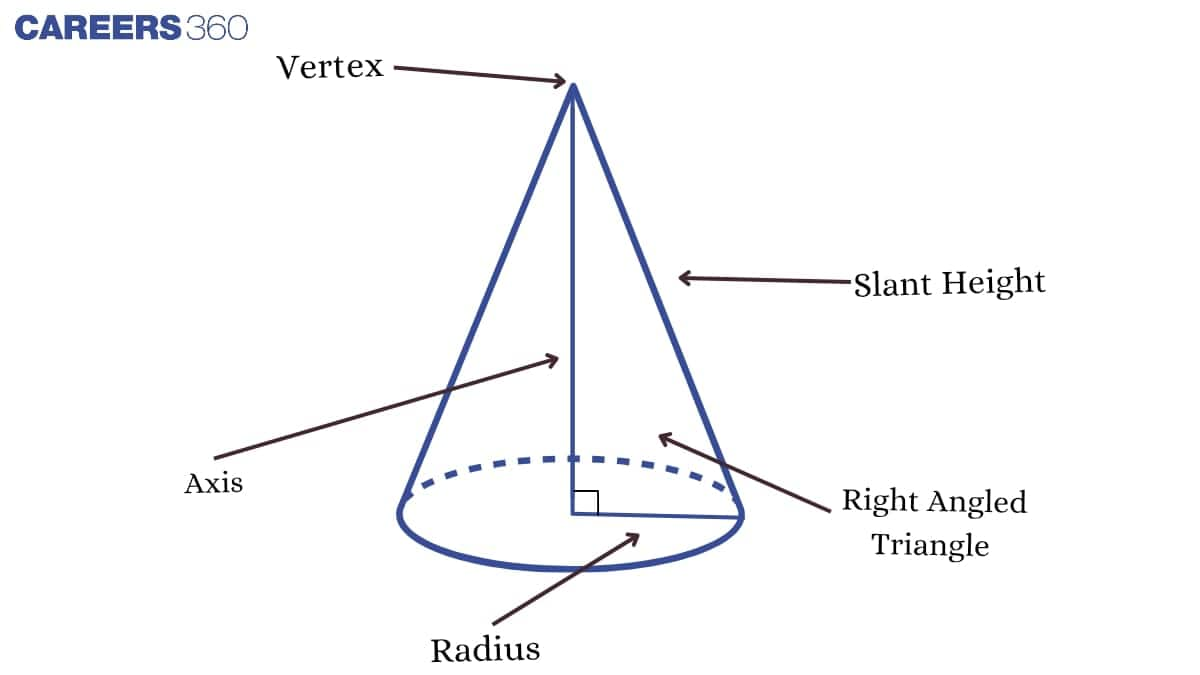
The curved surface area of the right circular cone, also known as the lateral surface area of the right circular cone, is the area covered by the curved surface of the cone. The total surface area of the right circular cone is the area occupied by the complete cone . NCERT solutions for Class 9 Maths chapter 13 exercise 13.3 include eight questions, seven of which are simple and the remaining one may take some time to complete . This Class 9 Maths chapter 13 exercise 13.3 thoroughly explains the concepts of surface area and volume. Along with class 9 maths chapter 13 exercise 13.3 the following exercises are also present.

** As per the CBSE Syllabus for 2023-24, please note that this chapter has been renumbered as Chapter 11.

Download the PDF of NCERT Solutions for Class 9 Maths Chapter 13 Surface Areas and Volumes Exercise 13.3

Download PDF

Access Surface Area and Volumes Class 9 Chapter 13 Exercise: 13.3

Q1 Diameter of the base of a cone is $\small 10.5 \hspace{1mm}cm$ and its slant height is $\small 10 \hspace{1mm}cm$ . Find its curved surface area.

Answer:

Given,

Base diameter of the cone = $d=10.5\ cm$

Slant height = $l=10\ cm$

We know, Curved surface area of a cone $= \pi r l$

$\therefore$ Required curved surface area of the cone=

$\\ = \frac{22}{7}\times \frac{10.5}{2}\times10 \\ \\ = 165\ cm^2$

Q2 Find the total surface area of a cone, if its slant height is $\small 21\hspace{1mm}m$ and diameter of its base is $\small 24\hspace{1mm}m$ .

Answer:

Given,

Base diameter of the cone = $d=24\ m$

Slant height = $l=21\ cm$

We know, Total surface area of a cone = Curved surface area + Base area

$= \pi r l + \pi r^2 = \pi r (l + r)$

$\therefore$ Required total surface area of the cone=

$\\ = \frac{22}{7}\times\frac{24}{2}\times(21+12) \\ \\ =\frac{22}{7}\times\frac{24}{2}\times33 \\ = 1244.57 \ m^2$

Q3 (i) Curved surface area of a cone is $\small 308\hspace{1mm}cm^2$ and its slant height is 14 cm. Find radius of the base .

Answer:

Given,

The curved surface area of a cone = $\small 308\hspace{1mm}cm^2$

Slant height $= l = 14\ cm$

(i) Let the radius of cone be $r\ cm$

We know, the curved surface area of a cone= $\pi rl$

$\therefore$ $\\ \pi rl = 308 \\ \\ \Rightarrow \frac{22}{7}\times r\times14 = 308 \\ \Rightarrow r = \frac{308}{44} = 7$

Therefore, the radius of the cone is $7\ cm$

Q3 (ii) Curved surface area of a cone is $\small 308\hspace{1mm}cm^2$ and its slant height is $\small 14\hspace{1mm}cm$ . Find total surface area of the cone.

Answer:

Given,

The curved surface area of a cone = $\small 308\hspace{1mm}cm^2$

Slant height $= l = 14\ cm$

The radius of the cone is $r =$ $7\ cm$

(ii) We know, Total surface area of a cone = Curved surface area + Base area

$= \pi r l + \pi r^2$

$\\ = 308+\frac{22}{7}\times 7^2 \\ = 308+154 = 462\ cm^2$

Therefore, the total surface area of the cone is $462\ cm^2$

Q4 (i) A conical tent is 10 m high and the radius of its base is 24 m. Find slant height of the tent.

Answer:

Given,

Base radius of the conical tent = $r=24\ m$

Height of the conical tent = $h=10\ m$

$\therefore$ Slant height = $l=\sqrt{h^2+r^2}$

$\\ =\sqrt{10^2+24^2} \\ = \sqrt{676} \\ = 26\ m$

Therefore, the slant height of the conical tent is $26\ m$

Q4 (ii) A conical tent is 10 m high and the radius of its base is 24 m. Find cost of the canvas required to make the tent, if the cost of $\small 1\hspace{1mm}m^2$ canvas is Rs 70.

Answer:

Given,

Base radius of the conical tent = $r=24\ m$

Height of the conical tent = $h=10\ m$

$\therefore$ Slant height = $l=\sqrt{h^2+r^2} = 26\ m$

We know, Curved surface area of a cone $= \pi r l$

$\therefore$ Curved surface area of the tent

$\\ = \frac{22}{7}\times24\times26 \\ \\ =\frac{13728}{7}\ m^2$

Cost of $1\ m^2$ of canvas = $Rs.\ 70$

$\therefore$ Cost of $\frac{13728}{7}\ m^2$ of canvas =

$Rs.\ (\frac{13728}{7}\times70) = Rs.\ 137280$

Therefore, required cost of canvas to make tent is $Rs.\ 137280$

Q5 What length of tarpaulin 3 m wide will be required to make conical tent of height 8 m and base radius 6 m? Assume that the extra length of material that will be required for stitching margins and wastage in cutting is approximately 20 cm (Use $\small \pi =3.14$ ).

Answer:

Given,

Base radius of the conical tent = $r =6\ m$

Height of the tent = $h =8\ m$

We know,

Curved surface area of a cone = $\pi rl = \pi r\sqrt{h^2 + r^2}$

$\therefore$ Area of tarpaulin required = Curved surface area of the tent

$\\ =3.14\times6\times \sqrt{8^2+ 6^2} \\ = 3.14\times6\times 10 \\ = 188.4\ m^2$

Now, let the length of the tarpaulin sheet be $x\ m$

Since $20\ cm$ is wasted, effective length = $x - 20 cm = (x - 0.2)\ m$

Breadth of tarpaulin = $3\ m$

$\\ \therefore [(x - 0.2) \times 3] = 188.4 \\ \Rightarrow x - 0.2 = 62.8 \\ \Rightarrow x = 63\ m$

Therefore, the length of the required tarpaulin sheet will be 63 m.

Q6 The slant height and base diameter of a conical tomb are 25 m and 14 m respectively. Find the cost of white-washing its curved surface at the rate of Rs 210 per $\small 100\hspace{1mm}m^2$ .

Answer:

Given, a conical tomb

The base diameter of the cone = $d =14\ m$

Slant height $= l = 25\ m$

We know, Curved surface area of a cone $= \pi r l$

$\\ = \frac{22}{7}\times\frac{14}{2}\times25 \\ \\ = 22\times25 \\ = 550\ m^2$

Now, Cost of whitewashing per $\small 100\hspace{1mm}m^2$ = $\small Rs.\ 210$

$\therefore$ Cost of whitewashing per $\small 550\hspace{1mm}m^2$ = $\small \\ Rs. (\frac{210}{100}\times550 )$

$\small \\ = Rs.\ (21\times55 ) = Rs.\ 1155$

Therefore, the cost of white-washing its curved surface of the tomb is $\small Rs.\ 1155$ .

Q7 A joker’s cap is in the form of a right circular cone of base radius 7 cm and height 24 cm. Find the area of the sheet required to make 10 such caps.

Answer:

Given, a right circular cone cap (which means no base)

Base radius of the cone = $r=7\ cm$

Height $= h = 24\ cm$

$\therefore l = \sqrt{h^2+r^2}$

We know, Curved surface area of a right circular cone $= \pi r l$

$\therefore$ The curved surface area of a cap =

$\\ = \frac{22}{7}\times7\times\sqrt{24^2+7^2} \\ \\ = 22\times\sqrt{625} \\ = 22\times25\ \\ = 550\ cm^2$

$\therefore$ The curved surface area of 10 caps = $550\times10 = 5500\ cm^2$

Therefore, the area of the sheet required for 10 caps = $5500\ cm^2$

Q8 A bus stop is barricaded from the remaining part of the road, by using 50 hollow cones made of recycled cardboard. Each cone has a base diameter of 40 cm and height 1 m. If the outer side of each of the cones is to be painted and the cost of painting is Rs 12 per $\small m^2$ , what will be the cost of painting all these cones? (Use $\small \pi =3.14$ and take $\small \sqrt{1.04}=1.02$ )

Answer:

Given, hollow cone.

The base diameter of the cone = $d = 40\ cm = 0.4\ m$

Height of the cone = $h = 1\ m$

$\therefore$ Slant height = $l = \sqrt{h^2+r^2}$ $= \sqrt{1^2+0.2^2}$

We know, Curved surface area of a cone = $\pi r l = \pi r\sqrt{h^2+r^2}$

$\therefore$ The curved surface area of 1 cone = $3.14\times0.2\times\sqrt{1.04} = 3.14\times0.2\times1.02$

$= 0.64056\ m^2$

$\therefore$ The curved surface area of 50 cones $= (50\times0.64056)\ m^2$

$= 32.028\ m^2$

Now, the cost of painting $\small 1\ m^2$ area = $\small Rs.\ 12$

$\therefore$ Cost of the painting $32.028\ m^2$ area $= Rs.\ (32.028\times12)$

$= Rs.\ 384.336$

Therefore, the cost of painting 50 such hollow cones is $Rs.\ 384.34\ (approx)$

Benefits of NCERT Solutions for Class 9 Maths Exercise 13.3 :

• NCERT solutions for Class 9 Maths exercise 13.3 will help us to easily identify the object which resembles the cone shape example: an ice cream cone.

•NCERT book Exercise 13.3 Class 9 Maths, clearly explained the formula for calculating the curved surface area of the right circular cone step by step for our better understanding.

• By completing the NCERT syllabus Class 9 Maths chapter 13 exercise 13.3 exercises, we can build a solid foundation of mathematical knowledge as well as gain confidence in approaching new topics in our higher classes.

Key Features of 9th Class Maths Exercise 13.3 Answers

Step-by-Step Solutions: The NCERT Solutions for class 9 maths chapter 13 exercise 13.3 provide detailed, step-by-step explanations for each problem, simplifying complex calculations and enhancing students' understanding.
Practical Application: This exercise 13.3 class 9 maths challenges students with problems that require the calculation of surface areas and volumes of intricate 3D shapes, allowing them to apply their knowledge to real-world scenarios.
Clear and Understandable Language: Class 9 maths ex 13.3 solutions are written in clear and understandable language to ensure that students can grasp the concepts with ease.
PDF Format: Students can access the ex 13.3 class 9 solutions in PDF format for free download, enabling offline use and flexible learning.

Also See:

NCERT Solutions of Class 10 Subject Wise

Frequently Asked Questions (FAQs)

1. According to NCERT solutions for Class 9 Maths chapter 13 exercise 13.3 , define the right circular cone .

According to NCERT solutions for Class 9 Maths chapter 13 exercise 13.3 , A type of cone whose axis falls perpendicular on the plane of the base is known as the right circular cone.

2. The point formed at the end of the cone is known as _______

The point formed at the end of the cone is known as the apex .

3. How many apexes does the cone have?

A cone has only one apex .

4. The total surface area of the cone is _________

The total surface area of the cone is πr(l + r)

5. The number of surfaces in the right cone is ______

In the right cone, there are two surfaces. There are two types of surfaces: base and slanted surfaces .

6. How is the total surface area of the right circular cone determined from the lateral surface area and the area of the circle?

The total surface area of a closed right circular cone is computed by adding the area of the lateral surface and the area of the circular base.

Also Read

NCERT Solutions for Class 9 - Subject-wise PDFs Updated for 2024-25

NCERT Solutions for Class 9 Science

NCERT Solutions for Class 9 Maths

NCERT Syllabus for Class 9 Maths 2023 - Download Syllabus Pdf

NCERT Solutions For Class 9 Science Chapter 1 Matter in Our Surroundings

NCERT Solutions for Exercise 2.5 Class 9 Maths Chapter 2 Polynomials

NCERT Class 9 Maths Notes - Download Chapter Wise PDFs

NCERT Class 9 Maths Chapter 8 Notes Quadrilaterals - Download PDF

NCERT Class 9 Maths Chapter 2 Notes Polynomials - Download PDF

NCERT Class 9 Maths Chapter 1 Notes Number System - Download Free PDF

NCERT Class 9 Maths Chapter 5 Notes Introduction To Euclid’s Geometry - Download PDF

NCERT Class 9 Maths Chapter 6 Notes Lines and Angles - Download PDF

NCERT Exemplar Class 9 Maths Solutions - Chapter Wise Problems with Solutions

NCERT Exemplar Class 9 Solutions - Subject Wise Problems with Solutions

NCERT Exemplar Class 9 Science Solutions Chapter 15 Improvement in Food Resources

NCERT Exemplar Class 9 Science Solutions Chapter 14 Natural Resources

NCERT Exemplar Class 9 Science Solutions Chapter 13 Why do we fall ill?

NCERT Exemplar Class 9 Science Solutions Chapter 12 Sound

Articles

AMU Class 1 Admission 2025: Application Process, Eligibility & Key Dates

Dec 30, 2024

Jamia Millia Islamia School Admission 2025: Application Process, Eligibility & Dates

Dec 30, 2024

SoSE Delhi School Admission 2025: All You Need to Know About Eligibility, Dates & Process

Dec 30, 2024

DBSE Result 2025, Check Delhi Board 10th, 12th Result

Dec 30, 2024

NMMS Chhattisgarh Result 2024-25: Check Merit List, Cut Off Marks

Dec 28, 2024

CBSE Scholarship 2024-25: Check CBSE Scholarships for Class 10, 12

Dec 27, 2024

HOS 12th Date Sheet 2025 - Haryana Open School Class 12 Exam Schedule

Dec 27, 2024

AMU School Admission 2025 - Know Application Process, Eligibility & Important Dates

Dec 27, 2024

Upcoming School Exams

Madhya Pradesh State Open School 10th Examination

Admit Card Date:13 December,2024 - 31 December,2024

Admit Card

Result

Madhya Pradesh State Open School 12th Examination

Admit Card Date:13 December,2024 - 06 January,2025

Admit Card

Result

National Institute of Open Schooling 10th examination

Late Fee Application Date:21 December,2024 - 31 December,2024

Application Process

Exam Pattern

Mock Test

National Institute of Open Schooling 12th Examination

Late Fee Application Date:21 December,2024 - 31 December,2024

Application Process

Exam Pattern

Admit Card

All India Sainik Schools Entrance Examination

Application Date:24 December,2024 - 13 January,2025

Exam Pattern

Answer Key

Result

View All School Exams

Get answers from students and experts

Popular Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms^-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times. Assume that the potential energy lost each time he lowers the mass is dissipated. How much fat will he use up considering the work done only when the weight is lifted up ? Fat supplies 3.8×10⁷J of energy per kg which is converted to mechanical energy with a 20% efficiency rate. Take g = 9.8 ms⁻² :

Option 1)

2.45×10⁻³ kg

Option 2)

6.45×10⁻³ kg

Option 3)

9.89×10⁻³ kg

Option 4)

12.89×10⁻³ kg

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

A particle is projected at 60⁰ to the horizontal with a kinetic energy $K$ . The kinetic energy at the highest point

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

In the reaction,

$2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

How many moles of magnesium phosphate, $Mg_{3}(PO_{4})_{2}$ will contain 0.25 mole of oxygen atoms?

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

With increase of temperature, which of these changes?

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol^-1) is

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t²) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m² , the number of rotations made by the pulley before its direction of motion if reversed, is