Careers360 Logo
School
Search Colleges, Exams, Schools & more
NCERT Solutions for Exercise 13.4 Class 9 Maths Chapter 13 - Surface Area and Volumes

NCERT Solutions for Exercise 13.4 Class 9 Maths Chapter 13 - Surface Area and Volumes

Edited By Vishal kumar | Updated on Oct 16, 2023 09:01 AM IST

NCERT Solutions for Class 9 Maths Exercise 13.4 Chapter 13 Surface Areas And Volumes- Download Free PDF

9th class maths exercise 13.4 answers is a significant component of Chapter 13. It consists of nine questions, each with multiple parts. These solutions have been meticulously crafted by subject matter experts from Careers360, ensuring they are presented in simple and detailed language for enhanced understanding. Furthermore, students can conveniently access the PDF version of these class 9 maths chapter 13 exercise 13.4 solutions, allowing them to study offline at their convenience without the need for an internet connection.

This Story also Contains
  1. NCERT Solutions for Class 9 Maths Exercise 13.4 Chapter 13 Surface Areas And Volumes- Download Free PDF
  2. Access Surface Area and Volumes Class 9 Chapter 13 Exercise: 13.4
  3. More About NCERT Solutions for Class 9 Maths Exercise 13.4
  4. Benefits of NCERT Solutions for Class 9 Maths Exercise 13.4 :
  5. Key Features of Class 9 Maths Ex 13.4
  6. NCERT Solutions of Class 10 Subject Wise
NCERT Solutions for Exercise 13.4 Class 9 Maths Chapter 13 - Surface Area and Volumes
NCERT Solutions for Exercise 13.4 Class 9 Maths Chapter 13 - Surface Area and Volumes

exercise 13.4 class 9 maths deals with the concept of the sphere, hemisphere, and it's surface area. A three-dimensional object which is round in shape is known as a sphere. Radius is the distance between the surface and centre of the sphere and diameter is the distance from one point to another point on the surface of the sphere, passing through the centre. The diameter of the sphere is given by 2r where r is the radius of the sphere.

In exercise 13.4 Class 9 Maths, The total area covered by the surface of a sphere in a three-dimensional space is known as the surface area of the sphere. The amount of space occupied by the sphere is known as the volume of the sphere . The nine questions in NCERT solutions for Class 9 Maths Chapter 13 Exercise 13.4 are based on the notion of surface areas and volumes of spheres. Class 9 Maths chapter 13 exercise 13.4 thoroughly explains the concepts of surface area and volume. The following activities are included along with Class 9 Maths chapter 13 exercise 13.4 .

**As per the CBSE Syllabus 2023-24, this chapter has been renumbered as Chapter 11.

Download the PDF of NCERT Solutions for Class 9 Maths Chapter 13 Surface Areas and Volumes Exercise 13.4

Download PDF


Access Surface Area and Volumes Class 9 Chapter 13 Exercise: 13.4

Q1 (i) Find the surface area of a sphere of radius: \small 10.5\hspace{1mm}cm .

Answer:

We know,

The surface area of a sphere of radius r = 4\pi r^2

\therefore Required surface area = 4\times\frac{22}{7} \times(10.5)^2

\\ =88\times1.5\times10.5 \\ = 1386\ cm^2

Q1 (ii) Find the surface area of a sphere of radius: \small 5.6\hspace{1mm}cm

Answer:

We know,

The surface area of a sphere of radius r = 4\pi r^2

\therefore Required surface area = 4\times\frac{22}{7} \times(5.6)^2

\\ =88\times0.8\times5.6 \\ = 394.24\ cm^2

Q1 (iii) Find the surface area of a sphere of radius: \small 14\hspace{1mm}cm

Answer:

We know,

The surface area of a sphere of radius r = 4\pi r^2

\therefore Required surface area = 4\times\frac{22}{7} \times(14)^2

\\ = 88\times2\times14 \\ = 2464 \ cm^2

Q2 (i) Find the surface area of a sphere of diameter: 14 cm

Answer:

Given,

The diameter of the sphere = 14\ cm

We know,

The surface area of a sphere of radius r = 4\pi r^2

\therefore Required surface area = 4\times\frac{22}{7} \times\left (\frac{14}{2} \right )^2

= 4\times\frac{22}{7} \times\frac{14}{2}\times\frac{14}{2}

\\ = 22\times2\times14 \\ = 616\ cm^2

Q2 (ii) Find the surface area of a sphere of diameter: 21 cm

Answer:

Given,

The diameter of the sphere = 21\ cm

We know,

The surface area of a sphere of radius r = 4\pi r^2

\therefore Required surface area = 4\times\frac{22}{7} \times\left (\frac{21}{2} \right )^2

= 4\times\frac{22}{7} \times\frac{21}{2}\times\frac{21}{2}

\\ = 22\times3\times21 \\ = 1386\ cm^2

Q2 (iii) Find the surface area of a sphere of diameter: \small 3.5\hspace{1mm}m

Answer:

Given,

The diameter of the sphere = 3.5\ m

We know,

The surface area of a sphere of radius r = 4\pi r^2

\therefore Required surface area = 4\times\frac{22}{7} \times\left (\frac{3.5}{2} \right )^2

= 4\times\frac{22}{7} \times\frac{3.5}{2}\times\frac{3.5}{2}

\\ = 22\times0.5\times3.5 \\ = 38.5\ m^2

Q3 Find the total surface area of a hemisphere of radius 10 cm. (Use \small \pi =3.14 )

Answer:

We know,

The total surface area of a hemisphere = Curved surface area of hemisphere + Area of the circular end

= 2\pi r^2 + \pi r^2 = 3\pi r^2

\therefore The required total surface area of the hemisphere = 3\times3.14\times(10)^2

\\ = 942\ cm^2

Q4 The radius of a spherical balloon increases from 7 cm to 14 cm as air is being pumped into it. Find the ratio of surface areas of the balloon in the two cases.

Answer:

Given,

r_1 = 7\ cm

r_2 = 14\ cm

We know,

The surface area of a sphere of radius r = 4\pi r^2

\therefore The ratio of surface areas of the ball in the two cases = \frac{Initial}{Final} = \frac{4\pi r_1^2}{4\pi r_2^2}

= \frac{r_1^2}{r_2^2}

\\ = \left (\frac{7}{14} \right )^2 \\ \\ = \left (\frac{1}{2} \right )^2 \\ \\ = \frac{1}{4}

Therefore, the required ratio is 1:4

Q5 A hemispherical bowl made of brass has inner diameter \small 10.5\hspace{1mm}cm . Find the cost of tin-plating it on the inside at the rate of Rs 16 per \small 100\hspace{1mm}cm^2 .

Answer:

Given,

The inner radius of the hemispherical bowl = r = \frac{10.5}{2}\ cm

We know,

The curved surface area of a hemisphere = 2\pi r^2

\therefore The surface area of the hemispherical bowl = 2\times\frac{22}{7}\times\left (\frac{10.5}{2} \right )^2

=11\times1.5\times10.5

= 173.25 \ cm^2

Now,

Cost of tin-plating \small 100\hspace{1mm}cm^2 = Rs 16

\therefore Cost of tin-plating \small 33\hspace{1mm}cm^2 = \small \\ Rs. \left (\frac{16}{100}\times173.25 \right )

\small = Rs. 27.72

Therefore, the cost of tin-plating it on the inside is \small Rs. 27.72

Q6 Find the radius of a sphere whose surface area is \small 154\hspace{1mm}cm^2 .

Answer:

Given,

The surface area of the sphere = \small 154\hspace{1mm}cm^2

We know,

The surface area of a sphere of radius r = 4\pi r^2

\therefore 4\pi r^2 = 154

\\ \Rightarrow 4\times\frac{22}{7}\times r^2 = 154

\\ \Rightarrow r^2 = \frac{154\times7}{4\times22} = \frac{7\times7}{4}

\\ \Rightarrow r = \frac{7}{2}

\\ \Rightarrow r = 3.5\ cm

Therefore, the radius of the sphere is 3.5\ cm

Q7 The diameter of the moon is approximately one fourth of the diameter of the earth. Find the ratio of their surface areas.

Answer:

Let diameter of Moon be d_m and diameter of Earth be d_e

We know,

The surface area of a sphere of radius r = 4\pi r^2

\therefore The ratio of their surface areas = \frac{Surface\ area\ of\ moon}{Surface\ area\ of\ Earth}

= \frac{4\pi \left (\frac{d_m}{2} \right )^2}{4\pi \left (\frac{d_e}{2} \right )^2}

= \frac{d_m^2}{d_e^2}

=\left ( \frac{\frac{1}{4}d_e}{d_e} \right )^2

= \frac{1}{16}

Therefore, the ratio of the surface areas of the moon and earth is = 1:16

Q8 A hemispherical bowl is made of steel, \small 0.25\hspace{1mm}cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.

Answer:

Given,

The inner radius of the bowl = r_1 = 5\ cm

The thickness of the bowl = \small 0.25\hspace{1mm}cm

\therefore Outer radius of the bowl = (Inner radius + thickness) =

r_2 = 5+0.25 = 5.25\ cm

We know, Curved surface area of a hemisphere of radius r = 2\pi r^2

\therefore The outer curved surface area of the bowl = 2\pi r_2^2

= 2\times\frac{22}{7}\times (5.25)^2

= 2\times\frac{22}{7}\times5.25\times5.25 = 173.25\ cm^2

Therefore, the outer curved surface area of the bowl is 173.25\ cm^2

Q9 (i) A right circular cylinder just encloses a sphere of radius \small r (see Fig. \small 13.22 ). Find surface area of the sphere,

1640847480239

Answer:

Given,

The radius of the sphere = r

\therefore Surface area of the sphere = 4\pi r^2

Q9 (ii) A right circular cylinder just encloses a sphere of radius \small r (see Fig. \small 13.22 ). Find curved surface area of the cylinder,

1640847500491

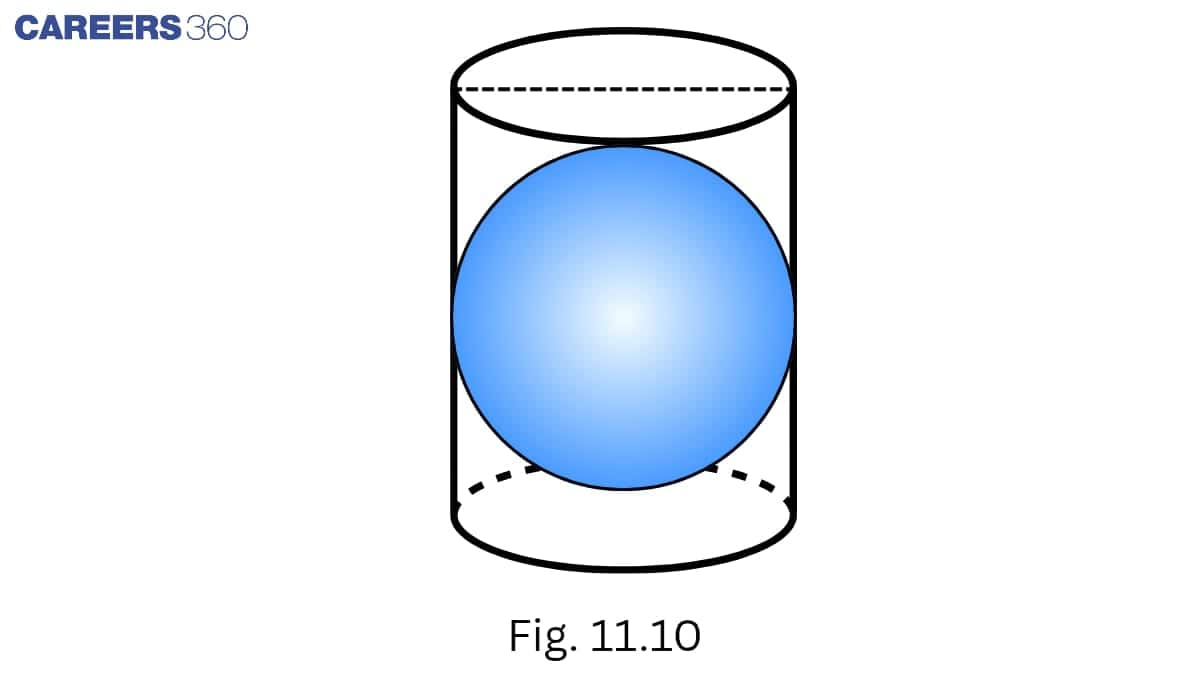
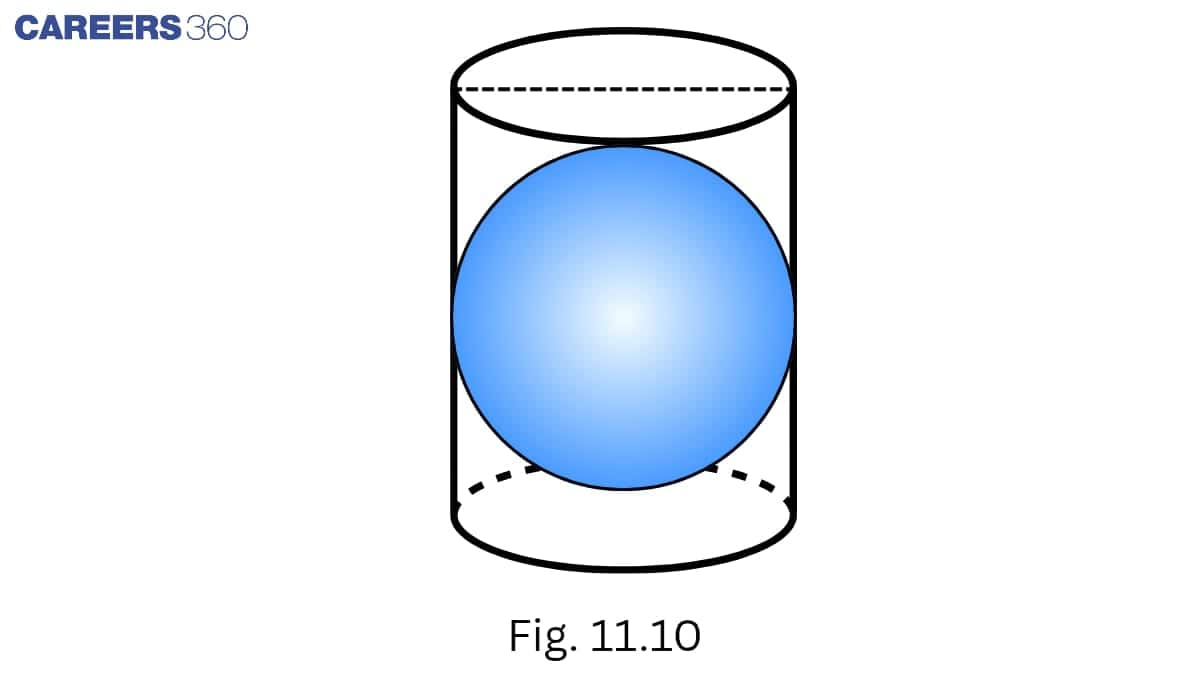
Answer:

Given,

The radius of the sphere = r

\therefore The surface area of the sphere = 4\pi r^2

According to the question, the cylinder encloses the sphere.

Hence, the diameter of the sphere is the diameter of the cylinder.

Also, the height of the cylinder is equal to the diameter of the sphere.

We know, the curved surface area of a cylinder = 2\pi rh

= 2\pi r(2r) = 4\pi r^2

Therefore, the curved surface area of the cylinder is 4\pi r^2

Q9 (iii) A right circular cylinder just encloses a sphere of radius \small r (see Fig. \small 13.22 ). Find ratio of the areas obtained in (i) and (ii).

1640847519663

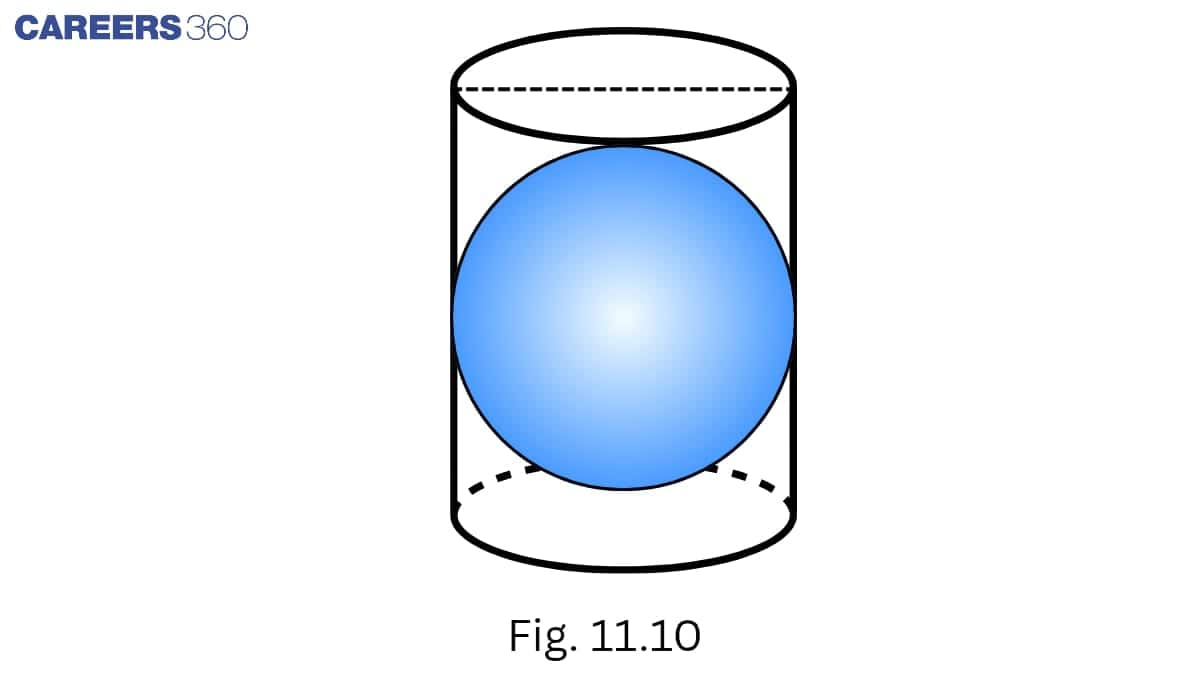
Answer:

The surface area of the sphere = 4\pi r^2

And, Surface area of the cylinder = 4\pi r^2

So, the ratio of the areas = \frac{4\pi r^2}{4\pi r^2} = 1



More About NCERT Solutions for Class 9 Maths Exercise 13.4

The NCERT solutions for Class 9 Maths exercise 13.4 is mainly focused on the surface area and the volume of the sphere. The surface area of the sphere is calculated by the product of four times the area of the circle. A=4πr2. The volume of the sphere is equal to 4Πr3/3. An exact half of a sphere is known as the hemisphere. When a sphere is cut at the exact centre along its diameter which leaves two equal hemispheres. There are two types of surface area. They are

  • Lateral Surface Area

  • Total Surface Area

The total surface area of hemisphere is equal to 3Ď€r2 whereas the Lateral surface area of hemisphere is equal to 2Ď€r2. In NCERT solutions for Class 9 Maths exercise 13.4, the formulas for computing surface areas and volume for the sphere and hemisphere are thoroughly explored.

Also Read| Surface Areas And Volumes Class 9 Notes

Benefits of NCERT Solutions for Class 9 Maths Exercise 13.4 :

• NCERT solutions for Class 9 Maths exercise 13.4, helps in finding the radius and diameter of a sphere and hemisphere by using the formula of total surface area of sphere and hemisphere respectively.

• NCERT book Exercise 13.4 Class 9 Maths, the questions are explained clearly with proper geometric figures and explanations in a step-by-step procedure for our good understanding that will help us to secure more marks.

• By answering the NCERT syllabus Class 9 Maths chapter 13 exercise 13.4 exercises, we may improve our grades in the first and second terms, and the formulae for calculating surface area and volume can aid us in solving competitive problems, therefore we should remember them.

Key Features of Class 9 Maths Ex 13.4

  1. Comprehensive Solutions: These 9th class maths exercise 13.4 answers cover and clarify all questions in Exercise 13.4.

  2. Sphere and Hemisphere Calculations: They assist in determining the radius of a sphere and the total surface area of a hemisphere.

  3. Alignment with NCERT Guidelines: The ex 13.4 class 9 solutions adhere to NCERT guidelines, aiding students in their exam preparation.

  4. Stepwise Expert Solutions: Subject matter experts have provided step-by-step solutions, helping students understand and score better.

Also See:

NCERT Solutions of Class 10 Subject Wise

Frequently Asked Question (FAQs)

1. Define sphere, according to NCERT solutions for Class 9 Maths chapter 13 exercise 13.4 .

A three-dimensional object with a round shape is called a sphere, according to NCERT solutions for Class 9 Maths chapter 13 exercise 13.4 .

2. Define radius and diameter.

Radius is the distance between surface and centre of the sphere whereas the diameter is the distance from one point to another point on the surface of the sphere, passing through the centre. 

3. The surface area of the sphere is _________

The sphere has a surface area of 4πr^2 . 

4. What are the different kinds of surface areas?

Surface area can be divided into two categories. They  are.

  • Area of Lateral Surface 

  • Area of Total Surface 

5. The total surface area of hemisphere is _________

The total surface area of hemisphere is 3πr^2 

6. The Lateral surface area of the hemisphere is __________ .

The Lateral surface area of hemisphere is 2πr^2 .

7. According to NCERT solutions for Class 9 Maths chapter 13 exercise 13.4 , How hemispheres are formed from a sphere ?

According to NCERT solutions for Class 9 Maths chapter 13 exercise 13.4 , When a sphere is cut at the exact centre along its diameter which leaves two equal hemispheres. 

Also Read

Articles

Upcoming School Exams

National Institute of Open Schooling 12th Examination

Admit Card Date:28 March,2024 - 22 May,2024

National Institute of Open Schooling 10th examination

Admit Card Date:28 March,2024 - 22 May,2024

Bihar Board 12th Examination

Admit Card Date:19 April,2024 - 11 May,2024

Madhya Pradesh Board 10th Examination

Application Date:01 May,2024 - 20 May,2024

Madhya Pradesh Board 12th Examination

Application Date:01 May,2024 - 20 May,2024

View All School Exams
Get answers from students and experts

Popular Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1)

0.34\; J

 Option 2)

0.16\; J
Option 3)

1.00\; J

 Option 4)

0.67\; J
Read More

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times.  Assume that the potential energy lost each time he lowers the mass is dissipated.  How much fat will he use up considering the work done only when the weight is lifted up ?  Fat supplies 3.8×107 J of energy per kg which is converted to mechanical energy with a 20% efficiency rate.  Take g = 9.8 ms−2 :

Option 1)

2.45×10−3 kg

 Option 2)

 6.45×10−3 kg
Option 3)

 9.89×10−3 kg

 Option 4)

12.89×10−3 kg

 
Read More

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1)

2,000 \; J - 5,000\; J

 Option 2)

200 \, \, J - 500 \, \, J
Option 3)

2\times 10^{5}J-3\times 10^{5}J

 Option 4)

20,000 \, \, J - 50,000 \, \, J
Read More

A particle is projected at 600   to the horizontal with a kinetic energy K. The kinetic energy at the highest point

Option 1)

K/2\,

 Option 2)

\; K\;
Option 3)

zero\;

 Option 4)

K/4
Read More

In the reaction,

2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}

Option 1)

11.2\, L\, H_{2(g)}  at STP  is produced for every mole HCL_{(aq)}  consumed

 Option 2)

6L\, HCl_{(aq)}  is consumed for ever 3L\, H_{2(g)}      produced
Option 3)

33.6 L\, H_{2(g)} is produced regardless of temperature and pressure for every mole Al that reacts

 Option 4)

67.2\, L\, H_{2(g)} at STP is produced for every mole Al that reacts .
Read More

How many moles of magnesium phosphate, Mg_{3}(PO_{4})_{2} will contain 0.25 mole of oxygen atoms?

Option 1)

0.02

 Option 2)

3.125 × 10-2
Option 3)

1.25 × 10-2

 Option 4)

2.5 × 10-2
Read More

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1)

decrease twice

 Option 2)

increase two fold
Option 3)

remain unchanged

 Option 4)

be a function of the molecular mass of the substance.
Read More

With increase of temperature, which of these changes?

Option 1)

Molality

 Option 2)

Weight fraction of solute
Option 3)

Fraction of solute present in water

 Option 4)

Mole fraction.
Read More

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol-1) is

Option 1)

twice that in 60 g carbon

 Option 2)

6.023 × 1022
Option 3)

half that in 8 g He

 Option 4)

558.5 × 6.023 × 1023
Read More

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t2) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m2 , the number of rotations made by the pulley before its direction of motion if reversed, is

Option 1)

less than 3

 Option 2)

more than 3 but less than 6
Option 3)

more than 6 but less than 9

 Option 4)

more than 9
Read More

Colleges After 12th

Popular Course After 12th

Also Read

Applications for Admissions are open.

JEE Main Important Physics formulas
JEE Main Important Physics formulas
Apply

As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters

Aakash iACST Scholarship Test 2024
Aakash iACST Scholarship Test 2024
Apply

Get up to 90% scholarship on NEET, JEE & Foundation courses

JEE Main Important Chemistry formulas
JEE Main Important Chemistry formulas
Apply

As per latest 2024 syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters

PACE IIT & Medical, Financial District, Hyd
PACE IIT & Medical, Financial District, Hyd
Apply

Enrol in PACE IIT & Medical, Financial District, Hyd for JEE/NEET preparation

ALLEN JEE Exam Prep
ALLEN JEE Exam Prep
Apply

Start your JEE preparation with ALLEN

ALLEN NEET Coaching
ALLEN NEET Coaching
Apply

Ace your NEET preparation with ALLEN Online Programs

View All Application Forms
News and Notifications
Barmer teacher suspended for slapping class 3 student
01/05/2024, 17:07:10
Teacher arrested for `sexual exploitation' at Ujjain Ashram school
01/05/2024, 14:28:18
Delhi NCR schools get bomb threat; fire tender arrives, search underway
01/05/2024, 04:09:04
Education is greatest gift to society: Vice President Jagdeep Dhankhar
30/04/2024, 17:02:12
Jharkhand schools up to Class 8 closed till further orders due to heat wave
30/04/2024, 11:49:37
Delhi School EWS Admission 2024-25: Registration window opens at edudel.nic.in; eligibility
30/04/2024, 09:27:25
Education ministry asks NCERT to review, update textbooks on yearly basis
29/04/2024, 12:22:03
Back to top