NCERT Solutions for Exercise 13.1 Class 9 Maths Chapter 13

NCERT Solutions for Exercise 13.1 Class 9 Maths Chapter 13 - Surface Area and Volumes

Edited By Vishal kumar | Updated on Oct 12, 2023 01:15 PM IST

NCERT Solutions for Class 9 Maths Exercise 13.1 Chapter 13 Surface Area And Volume- Download Free PDF

NCERT Solutions for Class 9 Maths Chapter 13: Surface Areas and Volumes Exercise 13.1- NCERT Solutions for Class 9 Maths Exercise 13.1 deal with the concept of surface areas cuboid and cube. The cuboid is a three-dimensional object defined by six rectangular planes with varied magnitudes of length, breadth, and height. It has eight vertices and twelve edges, and opposite sides are always equal . In exercise 13.1 Class 9 Maths, The area of a cuboid is referred to as the surface area since the cuboid is a three-dimensional solid. There are two types of surface area of the cuboid . They are

Don't Miss: JEE Main 2027: Narayana Scholarship Test Preparation Kit for Class 9

This Story also Contains

NCERT Solutions for Class 9 Maths Exercise 13.1 Chapter 13 Surface Area And Volume- Download Free PDF
Download PDF of NCERT Solutions for Class 9 Maths Chapter 13 – Surface Areas and Volumes Exercise 13.1
More About NCERT Solutions for Class 9 Maths Exercise 13.1 : Surface Area And Volumes
Benefits of NCERT Solutions for Class 9 Maths Exercise 13.1 :
Key Features of 9th Class Maths Exercise 13.1 Answers
NCERT Solutions of Class 10 Subject Wise

NCERT Solutions for Exercise 13.1 Class 9 Maths Chapter 13 - Surface Area and Volumes

Total Surface Area
Lateral Surface Area

The total surface area of the cuboid can be calculated by adding the areas of the 6 rectangular faces where the area is found by multiplying the length and breadth of each surface. TSA = 2(lb + bh + hl) . The lateral surface area of a cuboid can be calculated by adding the 4 planes of a rectangle, leaving the upper and the lower surface. LSA = 2h(l + b) . NCERT solutions for Class 9 Maths chapter 13 exercise 13.1 consists of 8 questions in which 6 of them are short and the remaining 2 might require some extra time to solve. The concepts related to the surface area are well explained in this 9th class maths exercise 13.1 answers . Along with exercise 13.1 class 9 maths the following exercises are also present in the NCERT book.

**As per the CBSE Syllabus for 2023-24, please note that this chapter has been renumbered as Chapter 11.

Download PDF of NCERT Solutions for Class 9 Maths Chapter 13 – Surface Areas and Volumes Exercise 13.1

Download PDF

Access Surface Area and Volumes Class 9 Maths Chapter 13 Exercise: 13.1

Q1 (i) A plastic box $\small 1.5\hspace {1mm}m$ long, $\small 1.25\hspace {1mm}m$ wide and $\small 65\hspace {1mm}cm$ deep is to be made. It is opened at the top. Ignoring the thickness of the plastic sheet, determine: The area of the sheet required for making the box.

Answer:

Given, dimensions of the plastic box

Length, $l = 1.5\ m$

Width, $b = 1.25\ m$

Depth, $h = 65\ cm = 0.65\ m$

(i) The area of the sheet required for making the box (open at the top)= Lateral surface area of the box. + Area of the base.

= $2(bh+hl)+lb$

$\\ = 2(1.25\times0.65+0.65\times1.5)+1.5\times1.25 \\ = 2(0.8125+ 0.975)+1.875= 5.45$

The required area of the sheet required for making the box is $5.45\ m^2$

Q1 (ii) A plastic box $\small 1.5\hspace {1mm} m$ long, $\small 1.25\hspace {1mm} m$ wide and $\small 65\hspace {1mm} cm$ deep is to be made. It is opened at the top. Ignoring the thickness of the plastic sheet, determine: The cost of sheet for it, if a sheet measuring $\small 1m^2$ costs Rs 20.

Answer:

Given, dimensions of the plastic box

Length, $l = 1.5\ m$

Width, $b = 1.25\ m$

Depth, $h = 65\ cm = 0.65\ m$

We know, area of the sheet required for making the box is $5.45\ m^2$

(ii) Cost for $\small 1m^2$ of sheet = Rs 20

$\therefore$ Cost for $5.45\ m^2$ of sheet = $5.45\times20 = 109$

Required cost of the sheet is $Rs.\ 109$

Q2 The length, breadth and height of a room are $\small 5\hspace {1mm}m$ , $\small 4\hspace {1mm}m$ and $\small 3\hspace {1mm}m$ respectively. Find the cost of white washing the walls of the room and the ceiling at the rate of $\small Rs \hspace {1mm} 7.50$ per $\small m^2$ .

Answer:

Given,

Dimensions of the room = $5\ m\times4\ m\times3\ m$

Required area to be whitewashed = Area of the walls + Area of the ceiling

= $2(lh+bh) + lb$

$\\ = 2(5\times4+4\times3)+ 5\times4 \\ = 2(32)+20 = 74\ m^2$
Cost of white-washing per $\small m^2$ area = $\small Rs \hspace {1mm} 7.50$
Cost of white-washing $74\ m^2$ area = $Rs (74 \times 7.50)$
$= Rs.\ 555$

Therefore, the required cost of whitewashing the walls of the room and the ceiling is $Rs.\ 555$

Q3 The floor of a rectangular hall has a perimeter $\small 250\hspace {1mm} m$ . If the cost of painting the four walls at the rate of Rs 10 per $\small m^2$ is Rs 15000, find the height of the hall. [ Hint : Area of the four walls $\small =$ Lateral surface area.]

Answer:

Given,

The perimeter of rectangular hall = $\small 250\hspace {1mm} m$

Cost of painting the four walls at the rate of Rs 10 per $\small m^2$ = Rs 15000

Let the height of the wall be $h\ m$

$\therefore$ Area to be painted = $Perimeter\times height$

$= 250h\ m^2$

$\therefore$ Required cost = $250h\times10\ m^2 = 15000\ m^2$

$\\ \implies 2500h = 15000 \\ \implies h = \frac{150}{25} = 6\ m$

Therefore, the height of the hall is $6\ m$

Q4 The paint in a certain container is sufficient to paint an area equal to $\small 9.375\hspace{1mm} m^2$ . How many bricks of dimensions $\small 22.5\hspace {1mm}cm \times 10\hspace {1mm}cm \times 7.5\hspace {1mm}cm$ can be painted out of this container?

Answer:

Given, dimensions of the brick = $\small 22.5\hspace {1mm}cm \times 10\hspace {1mm}cm \times 7.5\hspace {1mm}cm$

We know, Surface area of a cuboid = $2(lb+bh+hl)$

$\therefore$ The surface area of a single brick = $2(22.5\times10+10\times7.5+7.5\times22.5)$

$= 2(225+75+166.75) = 937.5\ cm^2 = 0.09375\ m^2$

$\therefore$ Number of bricks that can be painted = $\frac{Total\ area\ the\ container\ can\ paint}{Surface\ area\ of\ a\ single\ brick}$

$= \frac{9.375}{0.09375} = 100$

Therefore, the required number of bricks that can be painted = 100

Q5 (i) A cubical box has each edge $\small 10 \hspace{1mm}cm$ and another cuboidal box is $\small 12.5 \hspace{1mm}cm$ long, $\small 10 \hspace{1mm}cm$ wide and $\small 8 \hspace{1mm}cm$ high.

Which box has the greater lateral surface area and by how much?

Answer:

Given,

Edge of the cubical box = 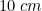$\small 10 \hspace{1mm}cm$

Dimensions of the cuboid = $12.5\cm \times 10\ cm \times 8\ cm$

(ii) The total surface area of the cubical box = $6\times(10\times10)\ cm^2 = 600\ cm^2$

The total surface area of the cuboidal box = 2[lh + bh+lb]

$\\ = [2(12.5 × 8 + 10 × 8 + 12.5\times10)]\ cm^2 \\ = 610\ cm^2$

Clearly, the total surface area of a cuboidal box is greater than the cubical box.

Difference between them = $610-600 = 10\ cm^2$

Q5 (ii) A cubical box has each edge $\small 10 \hspace {1mm}cm$ and another cuboidal box is $\small 12.5 \hspace {1mm}cm$ long, $\small 10 \hspace {1mm}cm$ wide and $\small 8 \hspace {1mm}cm$ high.

Which box has the smaller total surface area and by how much?

Answer:

Given,

Edge of the cubical box = $\small 10 \hspace{1mm}cm$

Dimensions of the cuboid = $12.5\cm \times 10\ cm \times 8\ cm$

(ii) The total surface area of the cubical box = $6\times(10\times10)\ cm^2 = 600\ cm^2$

The total surface area of the cuboidal box = 2[lh + bh+lb]

$\\ = [2(12.5 × 8 + 10 × 8 + 12.5\times10)]\ cm^2 \\ = 610\ cm^2$

Clearly, the total surface area of a cuboidal box is greater than the cubical box.

Difference between them = $610-600 = 10\ cm^2$

Q6 (i) A small indoor greenhouse (herbarium) is made entirely of glass panes (including base) held together with tape. It is $\small 30 \hspace{1mm}cm$ long, $\small 25 \hspace{1mm}cm$ wide and $\small 25 \hspace{1mm}cm$ high.What is the area of the glass?

Answer:

Given, dimensions of the greenhouse = $30\ cm \times25\ cm \times25\ cm$

Area of the glass = $2[lb + lh + bh]$
$\\ = [2(30 \times 25 + 30 \times 25 + 25 \times 25)] \\ = [2(750 + 750 + 625)] \\ = (2 \times 2125) \\ = 4250\ cm^2$
Therefore, the area of glass is $4250\ cm^2$

Q6 (ii) A small indoor greenhouse (herbarium) is made entirely of glass panes (including base) held together with tape. It is 30 cm long, 25 cm wide and 25 cm high. How much of tape is needed for all the 12 edges?

Answer:

Given, dimensions of the greenhouse = $30\ cm \times25\ cm \times25\ cm$

(ii) Tape needed for all the 12 edges = Perimeter = $4(l+b+h)$

$4(30+25+25) = 320\ cm$

Therefore, $320\ cm$ of tape is needed for the edges.

Q7 Shanti Sweets Stall was placing an order for making cardboard boxes for packing their sweets. Two sizes of boxes were required. The bigger of dimensions $\small 25\hspace {1mm}cm \times 20\hspace {1mm}cm \times 5 \hspace {1mm}cm$ , and the smaller of dimensions $\small 15\hspace {1mm}cm \times 12\hspace {1mm}cm \times 5 \hspace {1mm}cm$ . For all the overlaps, $\small 5\%$ of the total surface area is required extra. If the cost of the cardboard is Rs 4 for $\small 1000\hspace {1mm}cm^2$ , find the cost of cardboard required for supplying 250 boxes of each kind.

Answer:

Given,

Dimensions of the bigger box = $\small 25\hspace {1mm}cm \times 20\hspace {1mm}cm \times 5 \hspace {1mm}cm$ ,

Dimensions of smaller box = $\small 15\hspace {1mm}cm \times 12\hspace {1mm}cm \times 5 \hspace {1mm}cm$

We know,

Total surface area of a cuboid = $2(lb+bh+hl)$

$\therefore$ Total surface area of the bigger box = $2(25\times20+20\times5+5\times25)$

$= 2(500+100+125) = 1450\ cm^2$

$\therefore$ Area of the overlap for the bigger box = $5\%\ of\ 1450\ cm^2 = \frac{5}{100}\times1450 = 72.5\ cm^2$

Similarly,

Total surface area of the smaller box = $2(15\times12+12\times5+5\times15)$

$= 2(180+60+75) = 630\ cm^2$

$\therefore$ Area of the overlap for the smaller box = $5\%\ of\ 630\ cm^2 = \frac{5}{100}\times630 = 31.5\ cm^2$

Since, 250 of each box is required,

$\therefore$ Total area of carboard required = $250[(1450+72.5)+(630 + 31.5)] = 546000\ cm^2$

Cost of $\small 1000\hspace {1mm}cm^2$ of the cardboard = Rs 4

$\therefore$ Cost of $\small 546000\hspace {1mm}cm^2$ of the cardboard = $\small Rs. (\frac{4}{1000}\times546000) = Rs. 2184$

Therefore, the cost of the cardboard sheet required for 250 such boxes of each kind is $\small Rs.\ 2184$

Q8 Parveen wanted to make a temporary shelter for her car, by making a box-like structure with tarpaulin that covers all the four sides and the top of the car (with the front face as a flap which can be rolled up). Assuming that the stitching margins are very small, and therefore negligible, how much tarpaulin would be required to make the shelter of height $\small 2.5\hspace {1mm}m$ , with base dimensions $\small 4\hspace {1mm}m\times 3\hspace {1mm}m$ ?

Answer:

Given, Dimensions of the tarpaulin = $4\ m\times 3\ m \times2.5\ m$

The required amount of tarpaulin = Lateral surface area of the shelter + Area of top

= $2(lh + bh )+ lb$ Required

$\\ = 2(4\times2.5 + 3\times2.5 )+ 4\times3 \\ = 2(10+7.5) + 12 = 47\ m^2$

Therefore, $47\ m^2$ tarpaulin is required.

Benefits of NCERT Solutions for Class 9 Maths Exercise 13.1 :

• NCERT solutions for Class 9 Maths Exercise 13.1 enabled us to develop our basic concepts regarding the cuboid and its surface areas.

• By solving the NCERT solution for Class 9 Maths chapter 13 exercise 13.1 exercises, it helps us to score good marks in the first and second term examinations.

• Exercise 13.1 Class 9 Maths, helps us to simplify complex forms by reducing them down into smaller, easier-to-understand individual objects.

Key Features of 9th Class Maths Exercise 13.1 Answers

Step-by-Step Solutions: NCERT Solutions for class 9 maths ex 13.1 provide detailed, step-by-step explanations for each problem, simplifying the process of calculating surface areas and volumes.
Practical Application: This ex 13.1 class 9 presents problems that require students to compute surface areas and volumes of different 3D shapes, enabling practical applications of the concepts.
Clear and Understandable Language: Class 9 ex 13.1 Solutions are written in clear and understandable language to make the concepts accessible to students.
PDF Format: Students can access the 9th class maths exercise 13.1 answers in PDF format for free download, facilitating offline use and flexible learning.
Preparation for Advanced Geometry: Exercise 13.1 equips students with the foundational knowledge required to tackle more complex geometry topics in higher classes.

Also See:

NCERT Solutions of Class 10 Subject Wise

Frequently Asked Questions (FAQs)

1. Define cuboid, according to NCERT solutions for Class 9 Maths chapter 13 exercise 13.1 .

Solution :

A three-dimensional object circumscribed by six rectangular planes, each with a different magnitude of length, breadth, and height, is known as a cuboid, according to NCERT solutions for Class 9 Maths chapter 13 exercise 13.1 .

2. If the length, breadth, and height of the cuboid are equal, then it is known as _______.

Solution :

A cube is defined as a cuboid that has the same length, width, and height.

3. A cuboid's lateral surface area is ________

Solution :

A cuboid's lateral surface area is 2h(l + b)

4. The surface area of the cube with the edge x is ______

Solution :

The surface area of the cube with the edge x is 6x2

5. The total surface area of cuboid is _______

Solution :

The total surface area of cuboid is TSA = 2(lb + bh + hl) .

6. The surface area of the cube is measured in terms of _______ units.

Solution :

The surface area of the cube is measured in terms of square units.

7. What is the surface area of the cube of it’s edge is 4 cm ?

Solution :

The surface area of the cube with the edge a is 6a^2

The surface area of the cube with the edge 4 is 64^2=6×16=96 cm2 .

8. According to NCERT solutions for Class 9 Maths chapter 13 exercise 13.1 , define cube .

Solution :

A three dimensional shape which has six faces, eight vertices and twelve edges is known as a cube.

9. According to NCERT solutions for Class 9 Maths chapter 13 exercise 13.1 , Why is the area of the cuboid referred to as the surface area?

Solution :

The area of a cuboid is referred to as the surface area since the cuboid is a three dimensional solid.

Also Read

NCERT Solutions for Class 9 - Subject-wise PDFs Updated for 2024-25

NCERT Solutions for Class 9 Science

NCERT Solutions for Class 9 Maths

NCERT Syllabus for Class 9 Maths 2023 - Download Syllabus Pdf

NCERT Solutions For Class 9 Science Chapter 1 Matter in Our Surroundings

NCERT Solutions for Exercise 2.5 Class 9 Maths Chapter 2 Polynomials

NCERT Class 9 Maths Notes - Download Chapter Wise PDFs

NCERT Class 9 Maths Chapter 8 Notes Quadrilaterals - Download PDF

NCERT Class 9 Maths Chapter 2 Notes Polynomials - Download PDF

NCERT Class 9 Maths Chapter 1 Notes Number System - Download Free PDF

NCERT Class 9 Maths Chapter 5 Notes Introduction To Euclid’s Geometry - Download PDF

NCERT Class 9 Maths Chapter 6 Notes Lines and Angles - Download PDF

NCERT Exemplar Class 9 Maths Solutions - Chapter Wise Problems with Solutions

NCERT Exemplar Class 9 Solutions - Subject Wise Problems with Solutions

NCERT Exemplar Class 9 Science Solutions Chapter 15 Improvement in Food Resources

NCERT Exemplar Class 9 Science Solutions Chapter 14 Natural Resources

NCERT Exemplar Class 9 Science Solutions Chapter 13 Why do we fall ill?

NCERT Exemplar Class 9 Science Solutions Chapter 12 Sound

Articles

IGNOU Assignment Result 2024

Dec 21, 2024

DBSE Time Table 2025, Check Delhi Board Exam Schedule for Class 10 & 12

Dec 20, 2024

How to Make APAAR ID via DigiLocker and School: A Complete Guide

Dec 17, 2024

Sainik School Entrance Exam 2025: Application, Exam Date, How to Apply, Syllabus

Dec 17, 2024

MVPP Scholarship Exam 2024: Mukhyamantri Vigyan Pratibha Pariksha Registration, Exam Date

Dec 16, 2024

Silverzone ISSO Answer Key 2024-25: Download PDF

Dec 16, 2024

Silverzone IOEL Answer Key 2024 – Download International Olympiad of English Language Solutions

Dec 16, 2024

Silverzone Olympiad IOM Answer Key 2024-25: Qualifying Marks, Score

Dec 16, 2024

Upcoming School Exams

Bihar Open Board 10th Examination

Application Date:11 November,2024 - 10 January,2025

Admit Card

Result

Bihar Open Board 12th Examination

Application Date:11 November,2024 - 10 January,2025

Admit Card

Result

National Means Cum-Merit Scholarship

Admit Card Date:27 November,2024 - 22 December,2024

Eligibility Criteria

Application Process

Exam Pattern

Gujarat Board Higher Secondary School Certificate

Late Fee Application Date:13 December,2024 - 22 December,2024

Exam Pattern

Result

Admit Card

Madhya Pradesh State Open School 10th Examination

Admit Card Date:13 December,2024 - 31 December,2024

Admit Card

Result

View All School Exams

Get answers from students and experts

Popular Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms^-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times. Assume that the potential energy lost each time he lowers the mass is dissipated. How much fat will he use up considering the work done only when the weight is lifted up ? Fat supplies 3.8×10⁷J of energy per kg which is converted to mechanical energy with a 20% efficiency rate. Take g = 9.8 ms⁻² :

Option 1)

2.45×10⁻³ kg

Option 2)

6.45×10⁻³ kg

Option 3)

9.89×10⁻³ kg

Option 4)

12.89×10⁻³ kg

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

A particle is projected at 60⁰ to the horizontal with a kinetic energy $K$ . The kinetic energy at the highest point

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

In the reaction,

$2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

How many moles of magnesium phosphate, $Mg_{3}(PO_{4})_{2}$ will contain 0.25 mole of oxygen atoms?

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

With increase of temperature, which of these changes?

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol^-1) is

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t²) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m² , the number of rotations made by the pulley before its direction of motion if reversed, is