ALLEN Coaching
ApplyRegister for ALLEN Scholarship Test & get up to 90% Scholarship
NCERT Solutions for exercise 14.2 Class 9 Maths chapter 14 statistics is another important portion exercise of NCERT solutions for Class 9 Mathematics. As from prior exercise, we know that statistics is nothing a data analysis an area of Mathematics that comes with collecting and presenting amounts of numerical data over one or more places. Talking about exercise 14.2 Class 9 Maths is an exercise followed by exercise 14.1 of the chapter given that consists of introductory numerical problems of collection of data. In this exercise 14.2, we will be studying all those concepts which we talk about in the previous exercise one by one. In chapter 14 of Class 9 NCERT Mathematics, the primary concept of arrangements of students and numerical data will be discussed using examples mentioned with problems.
The Class 9 Maths chapter 14 exercise 14.2 lists basic level numerical problems to learn the concepts of statistics mentioned as terms like frequency mean etc. The class 9th Maths Chapter 14 exercise 14.2 covers Initial topics with examples mentioned and numerical to be solved. Many real-life scenario questions will also be discussed in NCERT solutions for NCERT book Class 9 Maths chapter 14 exercise 14.2. Along with NCERT syllabus Class 9 Maths Chapter 1 exercise 14.2 the following exercises are also present.
Q1 The blood groups of 30 students of Class VIII are recorded as follows:
A, B, O, O, AB, O, A, O, B, A, O, B, A, O, O,
A, AB, O, A, A, O, O, AB, B, A, O, B, A, B, O.
Represent this data in the form of a frequency distribution table. Which is the most common, and which is the rarest, blood group among these students?
Answer:
The representation of the given data in the form of a frequency distribution table is as follows.
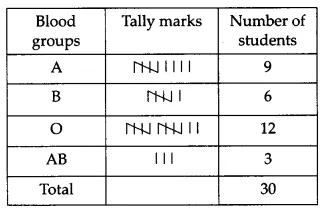
From the table we can see that O is the most common and AB is the rarest blood group.
Q2 The distance (in km) of
5 3 10 20 25 11 13 7 12 31
19 10 12 17 18 11 32 17 16 2
7 9 7 8 3 5 12 15 18 3
12 14 2 9 6 15 15 7 6 12
Construct a grouped frequency distribution table with class size
Answer:
As the minimum and maximum distances of an engineer from his place of work is 2 and 32 respectively the class intervals with class size 5 would be the following.
0 - 5, 5 - 10, 10 - 15, 15 - 20, 20 - 25, 25 - 30, 30 - 35
The representation of the given data in the form of a grouped frequency distribution table is as follows
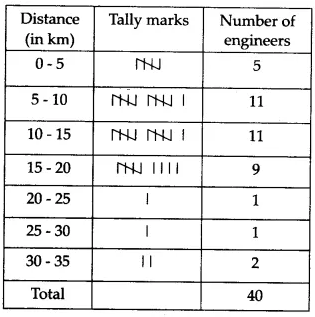
Frequencies of the class intervals 5 - 10 and 10 - 15 are maximum and equal to 11 each and frequencies of the class intervals 20 - 25 and 125 - 30 are minimum and equal to 1 each.
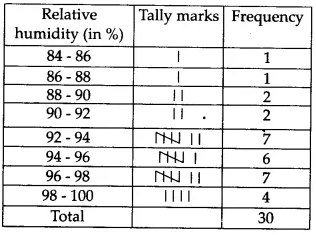
Q3 (i) The relative humidity (in
98.1 98.6 99.2 90.3 86.5 95.3 92.9 96.3 94.2 95.1
89.2 92.3 97.1 93.5 92.7 95.1 97.2 93.3 95.2 97.3
96.2 92.1 84.9 90.2 95.7 98.3 97.3 96.1 92.1 89
Construct a grouped frequency distribution table with classes 84 - 86, 86 - 88, etc.
Answer:
Q3 (ii) The relative humidity (in
98.1 98.6 99.2 90.3 86.5 95.3 92.9 96.3 94.2 95.1
89.2 92.3 97.1 93.5 92.7 95.1 97.2 93.3 95.2 97.3
96.2 92.1 84.9 90.2 95.7 98.3 97.3 96.1 92.1 89
Which month or season do you think this data is about?
Answer:
As from the table we can see relative humidity in most of the days is above 92% we can conclude the data is from a month of the rainy season. The leaast humidity recorded is 84.9% which also is prettry high.
Q3 (iii) The relative humidity (in
98.1 98.6 99.2 90.3 86.5 95.3 92.9 96.3 94.2 95.1
89.2 92.3 97.1 93.5 92.7 95.1 97.2 93.3 95.2 97.3
96.2 92.1 84.9 90.2 95.7 98.3 97.3 96.1 92.1 89
What is the range of this data?
Answer:
Range of a given data = Highest observation - Lowest Observation
Highest recorded humidity = 99.2%
Lowest recorded humidity = 84.9%
Therefore range of the given data = 99.2 - 84.9 = 14.3%
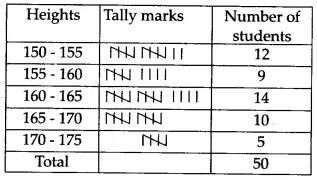
Q4 (i) The heights of 50 students, measured to the nearest centimetres, have been found to be as follows:
161 150 154 165 168 161 154 162 150 151
162 164 171 165 158 154 156 172 160 170
153 159 161 170 162 165 166 168 165 164
154 152 153 156 158 162 160 161 173 166
161 159 162 167 168 159 158 153 154 159
Represent the data given above by a grouped frequency distribution table, taking the class intervals as 160 - 165, 165 - 170, etc.
Answer:
The highest recorded height of a student is 173 cm.
The lowest recorded height of a student is 150 cm.
The class intervals would therefore be 150 -155, 155 - 160, 160 - 165, 165 - 170, 170 - 175
The representation of the given data in the form of a grouped frequency distribution table is as follows.
Q4 (ii) The heights of 50 students, measured to the nearest centimetres, have been found to be as follows:
161 150 154 165 168 161 154 162 150 151
162 164 171 165 158 154 156 172 160 170
153 159 161 170 162 165 166 168 165 164
154 152 153 156 158 162 160 161 173 166
161 159 162 167 168 159 158 153 154 159
What can you conclude about their heights from the table?
Answer:
From the table we can conclude that maximum students have height in the range 160 - 165 cm and more than half of the students are shorter than 165 cm.
0.03 0.08 0.08 0.09 0.04 0.17
0.16 0.05 0.02 0.06 0.18 0.20
0.11 0.08 0.12 0.13 0.22 0.07
0.08 0.01 0.10 0.06 0.09 0.18
0.11 0.07 0.05 0.07 0.01 0.04
Make a grouped frequency distribution table for this data with class intervals as 0.00 - 0.04, 0.04 - 0.08, and so on.
Answer:
The lowest value of the concentration of sulphur dioxide in the air is 0.01 ppm
The highest value of the concentration of sulphur dioxide in the air is 0.22 ppm
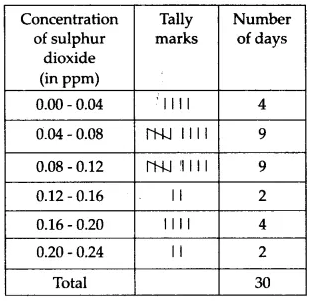
The representation of the given data in the form of a frequency distribution table is as follows.
0.03 0.08 0.08 0.09 0.04 0.17
0.16 0.05 0.02 0.06 0.18 0.20
0.11 0.08 0.12 0.13 0.22 0.07
0.08 0.01 0.10 0.06 0.09 0.18
0.11 0.07 0.05 0.07 0.01 0.04
For how many days, was the concentration of sulphur dioxide more than 0.11 parts per million?
Answer:
From the frequency distribution table, we can see the concentration of sulphur dioxide was more than 0.11 ppm for 8 days.
It was in the range 0.12 - 0.16 for 2 days, 0.16 - 0.20 for 4 days and 0.20 - 0.24 for 2 days.
0 1 2 2 1 2 3 1 3 0
1 3 1 1 2 2 0 1 2 1
3 0 0 1 1 2 3 2 2 0
Prepare a frequency distribution table for the data given above
Answer:
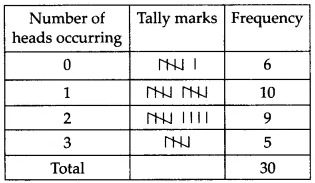
A frequency distribution table for the data given above is as follows.
Q7 (i) The value of
3.14159265358979323846264338327950288419716939937510
Make a frequency distribution of the digits from 0 to 9 after the decimal point.
Answer:
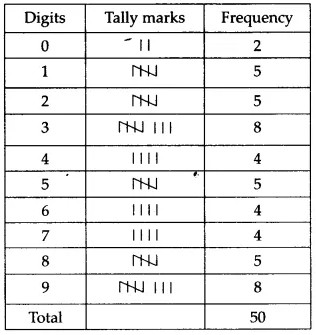
The representation of the given data in the form of a frequency distribution table is as follows.
Q7 (ii) The value of
3.14159265358979323846264338327950288419716939937510
What are the most and the least frequently occurring digits?
Answer:
The most frequently occurring digits are 3 and 9 with a frequency of 8.
1 6 2 3 5 12 5 8 4 8
10 3 4 12 2 8 15 1 17 6
3 2 8 5 9 6 8 7 14 12
Make a grouped frequency distribution table for this data, taking class width 5 and one of the class intervals as 5 - 10.
Answer:
The highest number of hours for which a child watched TV = 17
The lowest number of hours for which a child watched TV = 1
The class intervals with class width 5 would, therefore, be 1 - 5, 5 - 10, 10 - 15, 15 - 20
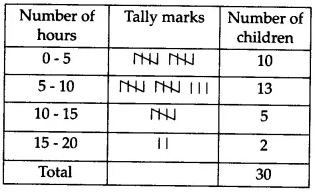
The representation of the given data in the form of a frequency distribution table is as follows.
1 6 2 3 5 12 5 8 4 8
10 3 4 12 2 8 15 1 17 6
3 2 8 5 9 6 8 7 14 12
How many children watched television for 15 or more hours a week?
Answer:
2 children watched television for 15 or more hours a week as we can see from the frequency distribution table. Frequency of the class interval 15 - 20 is 2.
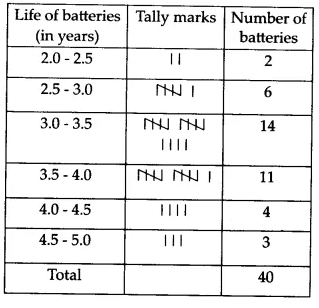
2.6 3.0 3.7 3.2 2.2 4.1 3.5 4.5
3.5 2.3 3.2 3.4 3.8 3.2 4.6 3.7
2.5 4.4 3.4 3.3 2.9 3.0 4.3 2.8
3.5 3.2 3.9 3.2 3.2 3.1 3.7 3.4
4.6 3.8 3.2 2.6 3.5 4.2 2.9 3.6
Construct a grouped frequency distribution table for this data, using class intervals of size 0.5 starting from the interval 2 - 2.5.
Answer:
The least value of life of a battery recorded = 2.2
The highest value of life of a battery recorded = 4.6
The class intervals with interval size 0.5 would therefore be 2.0 - 2.5, 2.5 - 3.0, 3.0 - 3.5, 3.5 - 4.0, 4.0 - 4.5, 4.5 - 5.0
The representation of the given data in the form of a frequency distribution table is as follows.
There are only 8-9 questions that cover the theory as well as practical aspects of exercise and chapter too as mentioned in exercise 14.2 Class 9 Maths. The initial questions give a basic idea of data analysis and working that can be collected from daily life. As the number increases the level of questions also increases in NCERT solutions for Cass 9 Maths chapter 14 exercise 14.2. In last of Class 9 Maths chapter 14 exercise 14.2 gives the briefing of all the introductory topics and examples discussed over chapter. The Class 9 Maths chapter 14 exercise 14.2 is to simplify mathematical terms for students to work on their problem-solving skills.
Also Read| Statistics Class 9 Notes
Benefits of NCERT Solutions for Class 9 Maths Chapter 14 Exercise 14.2
The solved example given in exercise 14.2 Class 9 Maths and the NCERT solutions for Class 9 Maths chapter 14 exercise 14.2 are important as it covers questions from the frequency of random and distributed data and questions related to grouping and ungrouping of data.
If students can solve each and over the most question of this exercise 14.2 Class 9 Maths they will be able to enjoy statistics initial to another level that is given in Class 9 Maths chapter 14 exercise 14.2
One last benefit of solving Class 9 Maths chapter 1 exercise 14.2 is students understand the formulas methods by heart instead of just Cramming with no prior knowledge and practice.
Also, see-
Ans: The frequency (or absolute frequency) of an event in statistics is the number of times the event happened in an experiment or research.
Ans:. A statistical data set's (or population's) distribution is a list or function that shows all of the potential values (or ranges) of the data and how frequently they occur. When categorical data is grouped, you may see the number or percentage of people in each group
Ans: Yes we can find the frequency of Undistributed data by the exact observation or by means of counting in general.
Ans: Grouping of data is a statistical term used in data analysis. Raw data may be structured in a table by grouping together related measurements. This frequency table is sometimes referred to as grouped data.
Ans: Grouping is accomplished by creating a set of ranges and then calculating how many of the data points fall inside each range.
Ans: Grouped data refers to information (or data) presented in the form of class intervals such as 0-20, 20-40, and so on.
Ans: Ungrouped data is described as information presented in the form of individual points (i.e. values or numbers) such as 15, 63, 34, 20, 25, and so on.
Ans: Range of data = Max value of data – Min value of data

Register for ALLEN Scholarship Test & get up to 90% Scholarship

Get up to 90% Scholarship on Offline NEET/JEE coaching from top Institutes

This ebook serves as a valuable study guide for NEET 2025 exam.

This e-book offers NEET PYQ and serves as an indispensable NEET study material.
As per latest 2024 syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
As per latest 2024 syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters