NCERT Solutions for Class 8 Maths Chapter 10 Visualizing Solid Shapes

Edited By Ramraj Saini | Updated on Mar 01, 2024 06:34 PM IST

Visualizing Solid Shapes Class 8 Questions And Answers provided here. These NCERT Solutions are created by expert team at Craeers360 keeping in mind of the latest syllabus and pattern of CBSE 2023-23. In this NCERT solutions for Class 8 Maths chapter 10 Visualizing Solid Shapes you will study solid objects like cubes, cuboids, cones, spheres, hemispheres, etc. You must have noticed that 3-dimensional objects look different when you look from different positions or different angles. To simplify its study there are defined viewpoints (front view, side view, top view) for the 3D object.

New: ALLEN TALLENTEX. Get Scholarships & Cash prizes. Register Now

This Story also Contains

Visualizing Solid Shapes Class 8 Questions And Answers PDF Free Download
Visualizing Solid Shapes Class 8 Solutions - Important Formulae
Visualizing Solid Shapes Class 8 NCERT Solutions (Intext Questions and Exercise)
Picture (object)
Shape
(i) An agricultural field
NCERT Solutions for Class 8 Maths Chapter 10 Visualizing Solid Shapes - Topics
NCERT Solutions for Class 8 Maths - Chapter Wise
Key Features Of NCERT Solutions for Class 8 Maths Chapter 10 Visualizing Solid Shapes
NCERT Solutions for Class 8 - Subject Wise
NCERT Books and NCERT Syllabus

NCERT Solutions for Class 8 Maths Chapter 10 Visualizing Solid Shapes

In NCERT solutions for Class 8 Maths chapter 10 Visualizing Solid Shapes, maximum questions are related to finding front view, side view, and top view of the 3D geometry. Important topics like views of 3D shapes, mapping space around us, faces, edges, and vertices of the objects are covered in this chapter. NCERT solutions for Class 8 Maths chapter 10 Visualizing Solid Shapes are very helpful in engineering drawing, locating the place and will build a basic understanding of the 3D shapes. Here you will get the detailed NCERT Solutions for Class 8 Maths by clicking on the link.

Visualizing Solid Shapes Class 8 Questions And Answers PDF Free Download

Download PDF

Visualizing Solid Shapes Class 8 Solutions - Important Formulae

Polyhedron: A solid object bordered by polygons (platonic solid).
Euler's Formula: For a polyhedron with F faces, V vertices, and E edges:

F + V - E = 2

Prism: A solid with parallelogram side faces and congruent parallel polygon ends (bases). A prism consists of two triangular faces, three rectangular faces, six vertices, and nine edges.
Pyramid: A polyhedron with a polygonal base and additional triangular faces converging at a common vertex. A pyramid typically consists of one square face, four triangular faces, five vertices, and eight edges.
Tetrahedron: A type of triangular pyramid where the base is a triangle.
Dimensions of a Solid: Three-dimensional objects have three measurements (length, width, and height), while two-dimensional objects have two measurements (length and breadth or depth).
Mapping: Maps are different from photographs, as they show the relationships between objects and locations using symbols and a set scale.
Faces, Vertices, and Edges: In a polyhedron, faces are polygonal sections, edges are line segments connecting faces, and vertices are the points where edges intersect.

Free download NCERT Solutions for Class 8 Maths Chapter 10 Visualizing Solid Shapes for CBSE Exam.

Visualizing Solid Shapes Class 8 NCERT Solutions (Intext Questions and Exercise)

Visualizing solid shapes class 8 NCERT solutions - Topic 10.1 Introduction

Question:1 Match the following: (First one is done for you)

download11121

Answer:

Matched items are:

16700_s

Question:2 Match the following pictures (objects) with their shapes:

Picture (object)		Shape
(i) An agricultural field		Two rectangular cross paths inside a rectangular park.
(ii) A groove		A circular path around a circular ground.
(iii) A toy		A triangular field adjoining a square field.
(iv) A circular park		_{A cone has been taken out of a cylinder.}
(v) A cross path		A hemisphere surmounted on a cone.

Answer:

Matched objects are: 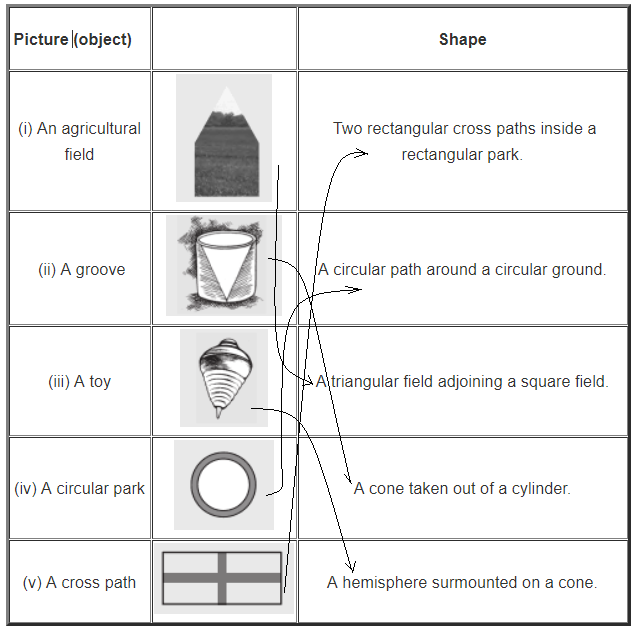1643869494833

Class 8 maths chapter 10 question answer - exercise: 10.1

Question:1 For each of the given solid, the two views are given. Match for each solid the
corresponding top and front views. The first one is done for you.

Object Side view Top view

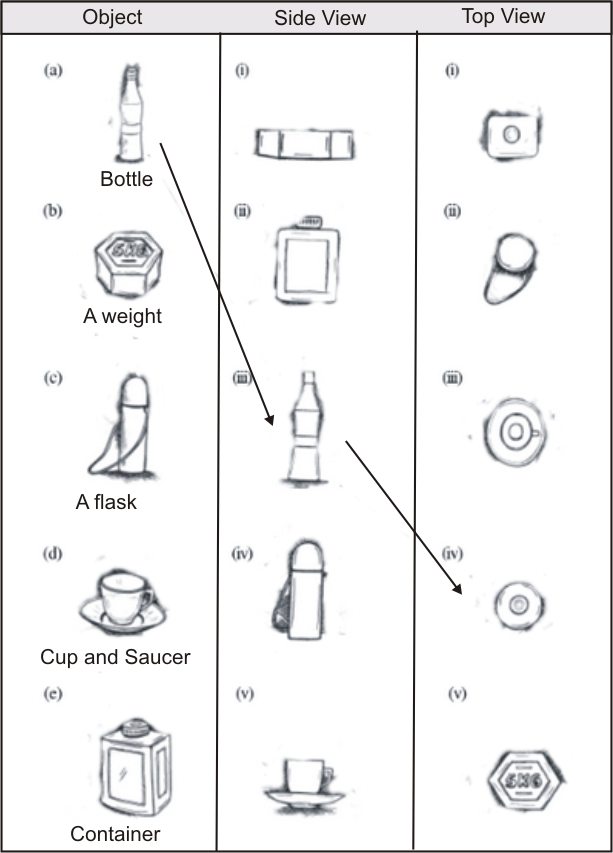
1643869538109

Answer:

Matched objects are:

(1) →iii)→iv)

(2) →i) →v)

(3) →iv)→ii)

(4) →v) →iii)

(5) →ii) →i)

16712_s

Question:2(a) For each of the given solid, the three views are given. Identify for each solid the corresponding top,
front and side views.

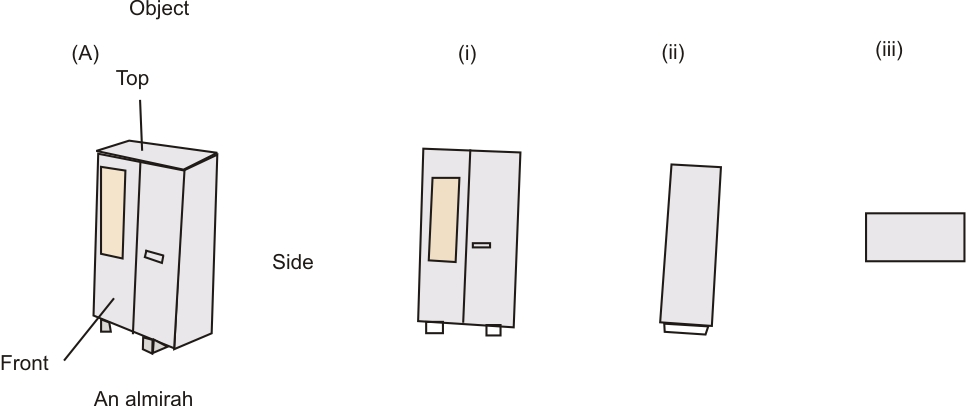1643869561496 Answer:

(a) Different views have given: (i) Front, (ii) Side, (iii) Top.

16714

Question:2(b) For each of the given solid, the three views are given. Identify for each solid the corresponding top,
front and side views. 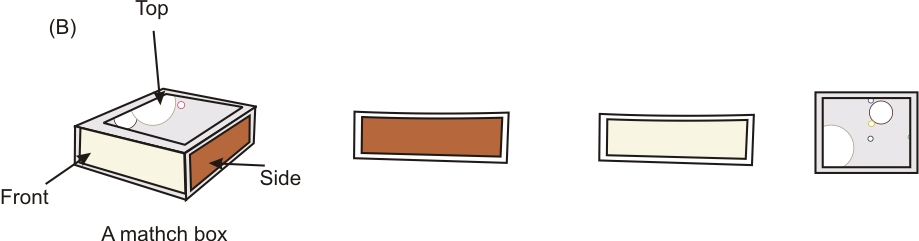1643869613151

Answer:

(b) (i) Side view, (ii) Front view, (iii) Top view.

Question:2(c) For each of the given solid, the three views are given. Identify for each solid the corresponding top,
front and side views. 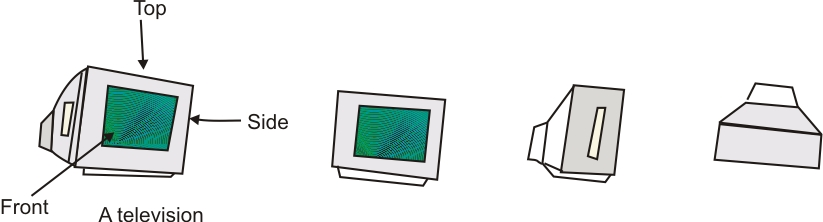1643869662372

Answer:

(c) (i) Front view, (ii) Side view, and (iii) Top view.

Question:2(d) For each of the given solid, the three views are given. Identify for each solid the corresponding top,
front and side views.

1643869695086

Answer:

(d) (i) Front view, (ii) Side view, and (iii) Top view.

Question:3(a) For each given solid, identify the top view, front view and side view.

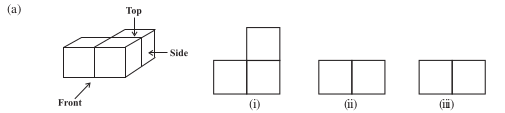
1643869721444

Answer:

(a) (i) Top view, (ii) Front/ Side view, and (iii) Side/ Front view.

Question:3(b) For each given solid, identify the top view, front view and side view.

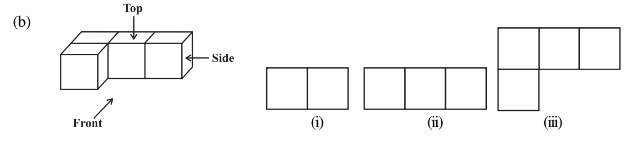
1643869773489

Answer:

(b) (i) Side view, (ii) Front view, and (iii) Top view.

Question:3(c) For each given solid, identify the top view, front view and side view.

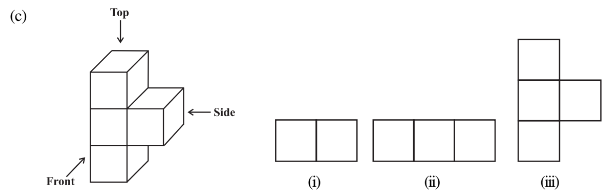
1643869839369

Answer:

(c) (i) Top view, (ii) Side view, and (iii) Front view.

16773_s

Question:3(d) For each given solid, identify the top view, front view and side view.

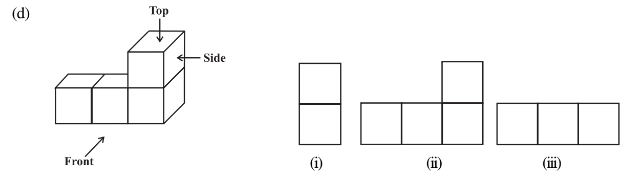
1643869879724

Answer:

(d) (i) Side view, (ii) Front view, and (iii) Top view.

Question:3(e) For each given solid, identify the top view, front view and side view.

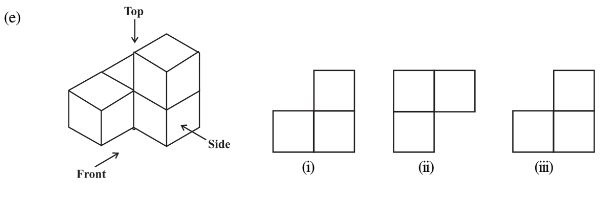
1643869919192

Answer:

(e) (i) Front view, (ii) Top view, and (iii) Side view.

16775_s

Question:4(a) Draw the front view, side view and top view of the given objects.

A military tent 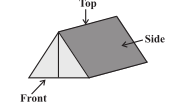1643869950891

Answer:

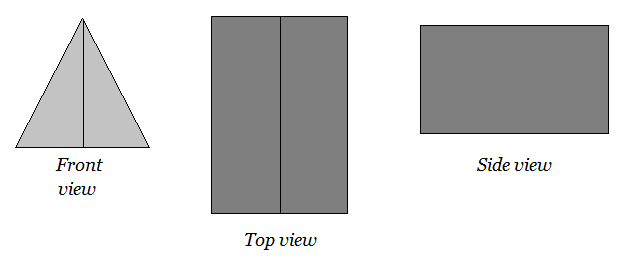
The front view, top view, and side view of a tent are shown below:

1643869991481

Question:4(b) Draw the front view, side view and top view of the given objects.

A table

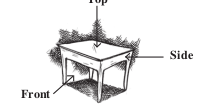
1643870029742

Answer:

The front view, top view, and Side view of the table are shown below: 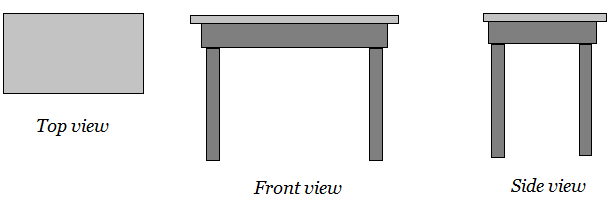1643870062495

Question:4(c) Draw the front view, side view and top view of the given objects.

A nut 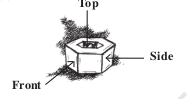1643870094182

Answer:

The front, top, and side views of the nut are:

download111

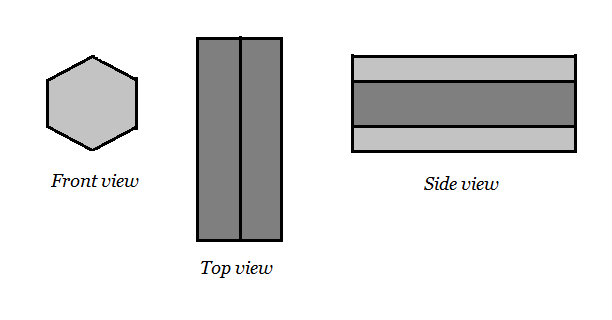
Question:4(d) Draw the front view, side view and top view of the given objects.

A hexagonal block 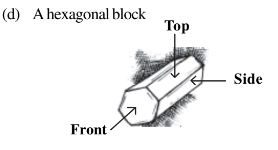1643870119298

Answer:

The front view, side view and top view of the hexagonal block are shown below:

1643870138302

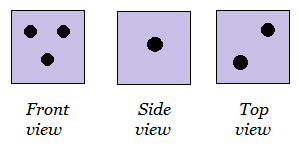
Question:4(e) Draw the front view, side view and top view of the given objects. 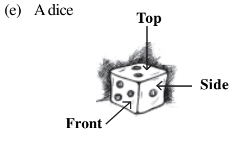1643870164279

Answer:

The front view, side view and top view of the dice as shown below:

1643870188777

Question:4(f) Draw the front view, side view and top view of the given objects.

A solid

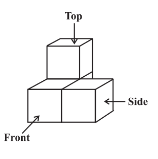
1643870213151

Answer:

The front view, side view and top view of the given solid.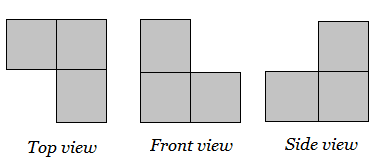

Class 8 maths chapter 10 ncert solutions - exercise: 10.2

Question:1(a) Look at the given map of a city.

1643870256704

Answer the following.

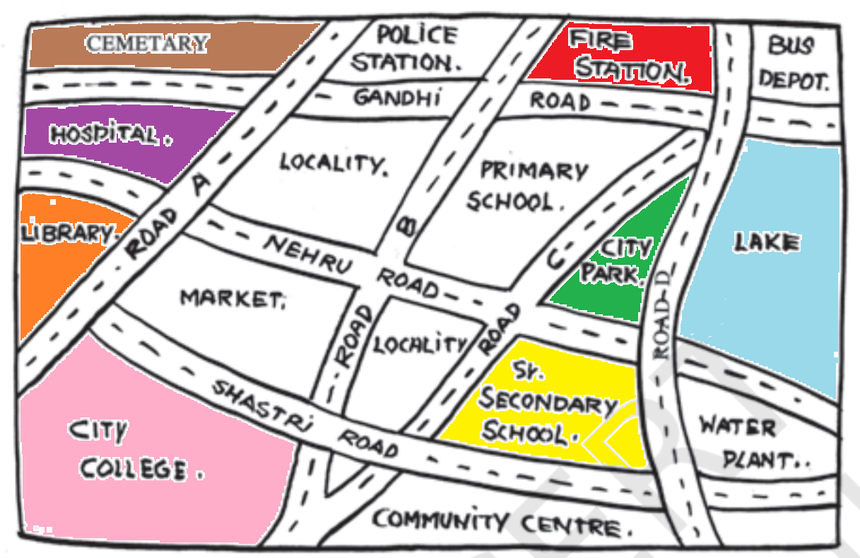
Colour the map as follows: Blue-water, red-fire station, orange-library, yellow
schools, Green - park, Pink - College, Purple - Hospital, Brown - Cemetery.

Answer:

(a) Coloured map shown:

1643870273523

Question:1(b) Look at the given map of a city.

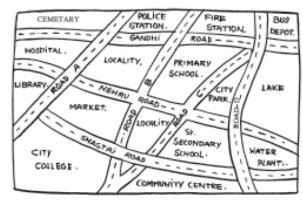
1643870298293

Answer the following.

Mark a green ‘X’ at the intersection of Road ‘C’ and Nehru Road, Green ‘Y’ at the intersection of Gandhi Road and Road A.

Answer:

(b) marked points of intersections "X" and "Y":

16789_s

Question:1(c) Look at the given map of a city. 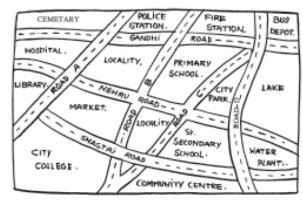1643870330068

Answer the following.

In red, draw a short street route from Library to the bus depot.

Answer:

Route from Library to bus depot is shown:

12362565

Question:1(d) Look at the given map of a city.

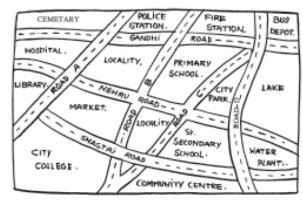
1643870366137

Answer the following.

Which is further east, the city park or the market?

Answer:

(d) The city park is further east.

Question:1(e) Look at the given map of a city. 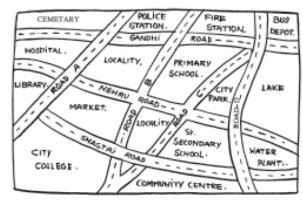1643870416760

Answer the following.

Which is further south, the primary school or the Sr. Secondary School?

Answer:

(e) Sr. Secondary School is further south.

Question:2 Draw a map of your class room using proper scale and symbols for different objects.

Answer:

Do it yourself.

Question:3 Draw a map of your school compound using proper scale and symbols for various features like play ground main building, garden etc.

Answer:

Do it with your own imagination.

Question:4 Draw a map giving instructions to your friend so that she reaches your house without any difficulty.

Answer:

Do it with your own imagination.

NCERT Solutions for Class 8 Maths Chapter 10 Visualizing Solid Shapes - Topic 10.4 Faces, Edges and Vertices

Question:1 Tabulate the number of faces, edges and vertices for the following polyhedrons: (Here ‘V’ stands for number of vertices, ‘F’ stands for number of faces and ‘E’ stands for number of edges).What do you infer from the last two columns? In each case, do you find, i.e., $F + V ï¿½ E = 2$ ? This relationship is called Euler’s formula. In fact this formula is true for any polyhedron.

Solid	F	V	E	F+V	E+2
Cuboid
Triangular pyramid
Triangular prism
Pyramid with square base
Prism with square base

Answer:

Solid	F	V	E	F+V	E+2
Cuboid	6	8	12	14	14
Triangular pyramid	4	4	6	8	8
Triangular prism	5	6	9	11	11
Pyramid with square base	5	5	8	10	10
Prism with square base	6	8	12	14	14

Class 8 visualizing solid shapes ncert solutions - exercise: 10.3

Question:1(i) Can a polyhedron have for its faces

3 triangles?

Answer:

(i) No, a polyhedron cannot have 3 triangles for its faces .

Question:1(ii) Can a polyhedron have for its faces

4 triangles?

Answer:

(ii) Yes, a polyhedron having 4 triangles is pyramid on triangular base.

Question:1(iii) Can a polyhedron have for its faces

a square and four triangles?

Answer:

(iii) Yes, a polyhedron can have faces of a square and four triangles which makes a pyramid on square base.

Question:2 Is it possible to have a polyhedron with any given number of faces? (Hint: Think of a pyramid).

Answer:

Yes , it is possible if the number of faces is four or more than four.

Question:3(i) Which are prisms among the following?

1643870498088

Answer:

(i) a nail is not a prism.

Question:3(ii) Which are prisms among the following? 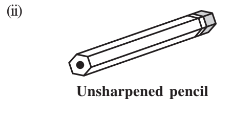1643870519515

Answer:

(ii) The unsharpened pencil is a prism.

Question:3(iii) Which are prisms among the following?

1643870544274

Answer:

(iii) Table weight is not a prism.

Question:3(iv) Which are prisms among the following?

1643870563257

Answer:

(iv) Given box is a prism.

Question:4(i) How are prisms and cylinders alike?

Answer:

(i) A prism is a polyhedron whose base and top are congruent polygons and whose other faces, i.e., lateral faces are parallelograms in shape. Also, the cylinder has base on top and bottom and faces are parallel.

Question:5 Is a square prism same as a cube? Explain.

Answer:

No , not always, because it can be a cuboid also when extended.

Question:6(i) Verify Euler’s formula for these solids.

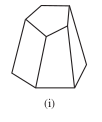
1643870598721

Answer:

(i) Euler formula F+V = E + 2.

So, Number of faces (F) = 7, Number of vertices (V) = 10 and number of edges (E) = 15.

Hence putting in the formula; we get,

7 + 10 = 15 + 2

17 = 17 hence, it satisfies the Euler formula.

Question:6(ii) Verify Euler’s formula for these solids. 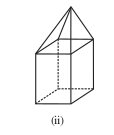1643870635063 Answer:

(ii) Euler formula F+V = E + 2.

So, Number of faces (F) = 9, Number of vertices (V) = 9 and number of edges (E) = 16.

Hence putting in the formula; we get,

9 + 9 = 16 + 2

18 = 18 hence, it satisfies the Euler formula.

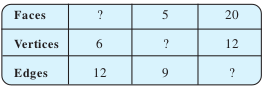
Question:7 Using Euler’s formula find the unknown.

1643870659232 Answer:

We know the Euler Formula:

F + V = E + 2 :

hence we can easily find the unknown let say 'F, V, and E'

So, For Ist case:

F + 6 = 12 + 2 or F = 8, hence the number of faces in Ist case is 8.

For IInd Case;

5 + V = 9 + 2 or V = 6, hence the number of vertices in IInd case is 6.

For IIIrd case;

20 + 12 = E + 2 or E = 30, hence the number of Edges in the IIIrd case is 30.

Faces	8	5	20
Vertices	6	6	12
Edges	12	9	30

Question:8 Can a polyhedron have 10 faces, 20 edges and 15 vertices?

Answer:

Checking using Euler's Formula:

F + V = E +2;

Given that F = 10, V = 15 and E = 20 so,

Putting in equation: 10 + 15 = 35 and 20 +2 = 22

Hence, given polyhedron having 10 faces, 20 edges and 15 vertices is not possible.

NCERT Solutions for Class 8 Maths Chapter 10 Visualizing Solid Shapes - Topics

Views of 3D-Shapes
Mapping Space Around Us
Faces, Edges, and Vertices

NCERT Solutions for Class 8 Maths - Chapter Wise

Chapter -1	Rational Numbers
Chapter -2	Linear Equations in One Variable
Chapter-3	Understanding Quadrilaterals
Chapter-4	Practical Geometry
Chapter-5	Data Handling
Chapter-6	Squares and Square Roots
Chapter-7	Cubes and Cube Roots
Chapter-8	Comparing Quantities
Chapter-9	Algebraic Expressions and Identities
Chapter-10	Visualizing Solid Shapes
Chapter-11	Mensuration
Chapter-12	Exponents and Powers
Chapter-13	Direct and Inverse Proportions
Chapter-14	Factorization
Chapter-15	Introduction to Graphs
Chapter-16	Playing with Numbers

Key Features Of NCERT Solutions for Class 8 Maths Chapter 10 Visualizing Solid Shapes

Comprehensive Coverage: Solutions for maths chapter 10 class 8 cover all topics and concepts related to visualizing solid shapes as per the Class 8 syllabus.

Step-by-Step Solutions: Detailed, step-by-step explanations for each problem, making it easy for students to understand and apply mathematical concepts related to three-dimensional shapes.

Illustrations and Diagrams: These class 8 maths ch 10 question answer includes diagrams, figures, and illustrations to visually explain the properties, views, and representations of solid shapes.

NCERT Solutions for Class 8 - Subject Wise

In NCERT solutions for class 8 maths chapter 10 visualizing solid shapes only one formula has been used know as Euler’s formula.

For any polyhedron-

$F+V-E=2$

where F - Number of faces

V - Number of vertices

E - Number of edges

As the name suggests this chapter is more about visualizing the shapes and drawing the front view, side view, and top view. In exercise 1 and 2 of NCERT solutions for class 8 maths chapter 10 visualizing solid shapes, most questions are related to visualizing shape and mapping which are explained with the help of drawing and graphs.

NCERT Books and NCERT Syllabus

Frequently Asked Questions (FAQs)

1. What are the important topics of NCERT book chapter Visualizing Solid Shapes ?

Views of the 3D-shapes, faces, edges and vertices are the important topics of this chapter.

2. How many chapters are there in the NCERT syllabus CBSE class 8 maths ?

There are 16 chapters starting from rational number to playing with numbers in the CBSE class 8 maths.

3. Does CBSE provide NCERT solution for class 8 ?

No, CBSE doesn't provide NCERT solutions for any class and subject.

4. Where can I find the complete solutions of NCERT for class 8 ?

Here you will get the detailed NCERT solutions for class 8 by clicking on the link.

5. Where can I find the complete solutions of NCERT for class 8 maths ?

Here you will get the detailed NCERT solutions for class 8 maths by clicking on the link.

6. How does the NCERT solutions are helpful ?

NCERT solutions are provided in a very detailed manner so it will give the conceptual clarity to students as well it helps them in their homework.

Also Read

NCERT Solutions for Class 8 Science Chapter 11 Force and Pressure

NCERT Solutions for Class 8 Science Chapter 10 Reaching The age of adolescence

NCERT Solutions for Class 8 Science Chapter 9 Reproduction in Animals

NCERT Solutions for Class 8 Science Chapter 7 Conservation of Plants and Animals

NCERT Solutions for Class 8 Science Chapter 3 Synthetic Fibres and Plastics

NCERT Solutions for Class 8 Science Chapter 2 Microorganisms Friend and Foe

NCERT Books for Class 8 English 2024 - Download PDF

NCERT Syllabus for Class 8 2024-25 - Download All Subjects PDF

Articles

Top Special Children School List 2025: A Guide for Parents

Dec 06, 2024

Christmas Day - Celebrations, How Schools Celebrate, Significance

Dec 06, 2024

Silverzone IOEL Answer Key 2024 – Download International Olympiad of English Language Solutions

Dec 03, 2024

Silverzone Olympiad IOM Answer Key 2024-25: Qualifying Marks, Score

Dec 03, 2024

How to Prepare for Science Olympiads: The Ultimate Study Plan

Dec 03, 2024

Silverzone STEM Result 2024-25 – Check STEM Olympiad Results Online

Dec 02, 2024

Silverzone STEM Olympiad Answer Key 2024-25: Check Answer Key for all Classes

Dec 02, 2024

Silverzone Commerce Olympiad Answer Key 2024: ICSI Question Paper Solution

Dec 02, 2024

Upcoming School Exams

Gujarat Board Higher Secondary School Certificate

Application Date:24 October,2024 - 09 December,2024

Exam Pattern

Result

Admit Card

National Means Cum-Merit Scholarship

Application Date:05 November,2024 - 07 December,2024

Eligibility Criteria

Application Process

Exam Pattern

Manipur Board 12th Examination

Application Date:11 November,2024 - 11 December,2024

Exam Pattern

Admit Card

Result

Bihar Open Board 10th Examination

Application Date:11 November,2024 - 10 December,2024

Admit Card

Result

Bihar Open Board 12th Examination

Application Date:11 November,2024 - 10 December,2024

Admit Card

Result

View All School Exams

Get answers from students and experts

Popular Questions

A block of mass 0.50 kg is moving with a speed of 2.00 ms^-1 on a smooth surface. It strikes another mass of 1.00 kg and then they move together as a single body. The energy loss during the collision is

Option 1) $0.34\; J$	Option 2) $0.16\; J$
Option 3) $1.00\; J$	Option 4) $0.67\; J$

A person trying to lose weight by burning fat lifts a mass of 10 kg upto a height of 1 m 1000 times. Assume that the potential energy lost each time he lowers the mass is dissipated. How much fat will he use up considering the work done only when the weight is lifted up ? Fat supplies 3.8×10⁷J of energy per kg which is converted to mechanical energy with a 20% efficiency rate. Take g = 9.8 ms⁻² :

Option 1)

2.45×10⁻³ kg

Option 2)

6.45×10⁻³ kg

Option 3)

9.89×10⁻³ kg

Option 4)

12.89×10⁻³ kg

An athlete in the olympic games covers a distance of 100 m in 10 s. His kinetic energy can be estimated to be in the range

Option 1) $2,000 \; J - 5,000\; J$	Option 2) $200 \, \, J - 500 \, \, J$
Option 3) $2\times 10^{5}J-3\times 10^{5}J$	Option 4) $20,000 \, \, J - 50,000 \, \, J$

A particle is projected at 60⁰ to the horizontal with a kinetic energy $K$ . The kinetic energy at the highest point

Option 1) $K/2\,$	Option 2) $\; K\;$
Option 3) $zero\;$	Option 4) $K/4$

In the reaction,

$2Al_{(s)}+6HCL_{(aq)}\rightarrow 2Al^{3+}\, _{(aq)}+6Cl^{-}\, _{(aq)}+3H_{2(g)}$

Option 1) $11.2\, L\, H_{2(g)}$ at STP is produced for every mole $HCL_{(aq)}$ consumed	Option 2) $6L\, HCl_{(aq)}$ is consumed for ever $3L\, H_{2(g)}$ produced
Option 3) $33.6 L\, H_{2(g)}$ is produced regardless of temperature and pressure for every mole Al that reacts	Option 4) $67.2\, L\, H_{2(g)}$ at STP is produced for every mole Al that reacts .

How many moles of magnesium phosphate, $Mg_{3}(PO_{4})_{2}$ will contain 0.25 mole of oxygen atoms?

Option 1) 0.02	Option 2) 3.125 × 10^-2
Option 3) 1.25 × 10^-2	Option 4) 2.5 × 10^-2

If we consider that 1/6, in place of 1/12, mass of carbon atom is taken to be the relative atomic mass unit, the mass of one mole of a substance will

Option 1) decrease twice	Option 2) increase two fold
Option 3) remain unchanged	Option 4) be a function of the molecular mass of the substance.

With increase of temperature, which of these changes?

Option 1) Molality	Option 2) Weight fraction of solute
Option 3) Fraction of solute present in water	Option 4) Mole fraction.

Number of atoms in 558.5 gram Fe (at. wt.of Fe = 55.85 g mol^-1) is

Option 1) twice that in 60 g carbon	Option 2) 6.023 × 10²²
Option 3) half that in 8 g He	Option 4) 558.5 × 6.023 × 10²³

A pulley of radius 2 m is rotated about its axis by a force F = (20t - 5t²) newton (where t is measured in seconds) applied tangentially. If the moment of inertia of the pulley about its axis of rotation is 10 kg m² , the number of rotations made by the pulley before its direction of motion if reversed, is