Aakash Repeater Courses
ApplyTake Aakash iACST and get instant scholarship on coaching programs.
Children often wonder about the geometry around them—"What shape is my book?", "How were the pyramids measured?", or even "How much wax is needed to make a candle for my school project?" and "What shape is a Christmas tree?" All these curious questions relate directly to the concept of Mensuration. They will clearly understand these shapes in detail after completing the Mensuration chapter, which is Class 8 Maths NCERT Chapter 9. In this section of Mathematics, we discuss the length, volume, and surface areas of various types of quadrilaterals.
Excelling in these Class 8 Maths Chapter 9 solutions will help us in board exams and lay a smooth path to learning about these concepts in higher classes. Other competitive exams frequently include many questions from this chapter. These class 8 Mensuration solutions contain many images and diagrams clearly illustrating the shapes because visualization is often considered the best way to learn. Experienced Careers 360 experts have made these solutions following the latest CBSE guidelines
Mensuration Class 8 Solutions - Important Formulae
Perimeter: The length of the outline of any simple closed figure is known as the perimeter.
Curved Surface Area of a Cone: πrl, where 'r' is the base radius and 'l' is the slant height, l = r2+h2
Mensuration Class 8 NCERT Solutions (Exercise)
| NCERT Solutions for Class 8 Maths Chapter 0 Mensuration: Topic 9.2 Area Of A Polygon |
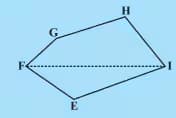
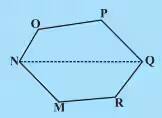
Question:(i) Divide the following polygons (Fig 11.17) into parts (triangles and trapezium) to find out its area.
![]()
![]()
FI is a diagonal of polygon EFGHI NQ is a diagonal of polygon MNOPQR
Answer:
(i)
Area of polygon EFGHI = area of △ EFI + area of quadrilateral FGHI
Draw a diagonal FH.
Area of polygon EFGHI = area of △ EFI + area of △ FGH + area of △ FHI
ii)
Area of polygon MNOPQR = area of quadrilateral NMRQ+area of quadrilateral NOPQ
Draw diagonals NP and NR.
Area of polygon MNOPQR = area of △ NOP + area of △ NPQ + area of △ NMR + area of △ NRQ
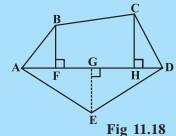
Area of ΔAFB=12×AF×BF=12×3×2=....
Area of trapezium FBCH
=FH×(BF+CH)2
=3×(2+3)2[FH=AH−AF]
Area of ΔCHD=12×HD×CH=.....
Area of ΔADE=12×AD×GE=...
So, the area of polygon ABCDE = ....
![]()
Answer:
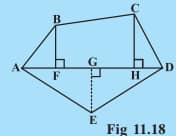
![]()
Area of Polygon ABCDE= area of △AFB+ area of trapezium BCHF + area of △CDH +area of △AED=(12×AF×BF)+(FH×(BF+CH)÷2)+(12×HD×CH)+(12×AD×EG)=(12×3×2)+(3×(2+3)÷2)+(12×2×3)+(12×8×2.5)=3+7.5+3+10=23.5 cm2
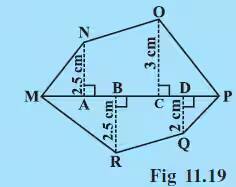
![]()
Answer:
the area of polygon MNOPQR
= area of △MAN+ area of trapezium ACON+area of △CPO+ area of △MBR+ area of trapezium BDQR+area of △DPQ=(12×2×2.5)+(4(2.5+3)÷2)+(12×3×3)+(12×4×2.5)+(3(2.5+2)÷2)+(12×2×2)=2.5+11+4.5+5+6.75+2=31.75 cm2
| Class 8 maths chapter 9 ncert solutions: Exercise: 9.1 Total Questions: 11 Page number: 105-106 |
Answer:
Area of table =
= 12 × sum of parallel sides × distance between parallel sides
= 12 ×(1+1.2)×0.8
= 0.4×2.2
= 0.88 m2
Answer:
Let the length of the other parallel side be x
area of a trapezium, 12×(10+x)×4=34
⇒ (10+x)=17
⇒ x = 17−10
⇒ x = 7 cm
Hence, the length of the other parallel side is 7 cm.
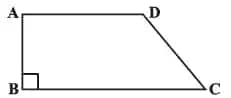
![]()
Answer:
BC=48m, CD=17m and AD=40m ,
Length of the fence of a trapezium-shaped field ABCD
= 120m = AB+BC+CD+DA
⇒ 120 = AB+48+17+40
⇒ AB = 15m
Area of trapezium
= 12 × sum of parallel sides × height
= 12×(40+48)×15
= 12×(88)×15
= (44)×15
= 660m2
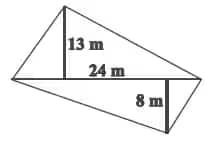
![]()
Answer:
The diagonal of a quadrilateral-shaped field is 24 m.
The perpendiculars are 8 m and 13 m.
the area of the field
= 12×d×(h1+h2)
= 12×24×(13+8)
= 12×21
= 252 m2
Question:5 The diagonals of a rhombus are 7.5 cm and 12 cm. Find its area.
Answer:
Area of rhombus =
12 × product of diagonals
= 12×7.5×12
= 7.5×6
= 45cm2
Answer:
A rhombus is a type of parallelogram, and the area of the parallelogram is the product of base and height.
So, Area of rhombus = base × height
= 5×4.8
= 24
Let the other diagonal be x
Area of rhombus =
= 12 × product of diagonals
⇒ 24 = 12×8×x
⇒ x = 6cm
Answer:
Area of 1 tile
= 12× product of diagonals
= 12×45×30
= 675 cm2
Area of 3000 tiles =
= 675 ×3000
= 202.5 m2
Total cost of polishing
= 202.5 × 4
= Rs. 810
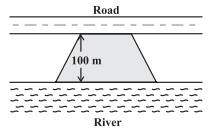
![]()
Answer:
Let the length of the side along the road be x m.
Then, according to the question, the length of the side along the river will be 2x m.
Area of trapezium = 12h(a+b)
So the equation becomes:
12100(x+2x)=10500
⇒ 3x = 210
⇒ x = 70
So the length of the side along the river is 2x = 140m.
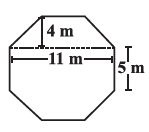
![]()
Answer:
Area of octagonal surface = Area of rectangular surface + 2(Area of trapezium surface)
Area of rectangular surface = 11×5 = 55 m2
Area of trapezium surface =
12 × 4 (11+5) = 2 × 16 = 32m2
So the total area of the octagonal surface = 55 + 2(32) = 55 + 64 = 119 m2
Find the area of this park using both ways. Can you suggest some other way of finding its area?
Answer:
Area of pentagonal park according to Jyoti's diagram:
=2( Area of trapezium )=2(12×152×45)=15×452=337.5 m2
Area of pentagonal park according to Kavita's diagram:
= Area of triangle + Area of square .
=12×15×15+15×15=112.5+225=337.5 m2
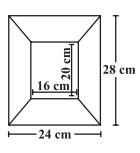
![]()
Answer:
Area of opposite sections will be the same.
So the area of horizontal sections,
= 12×4(16+24) = 2(40) =80 cm2
And area of vertical sections,
= 12×8(20+28) = 4(48) = 96 cm2
| NCERT Solutions for Class 8 Maths Chapter 9 Mensuration: Topic 9.4.1 Cuboid |
Question:1 Find the total surface area of the following cuboids (Fig 11.31):
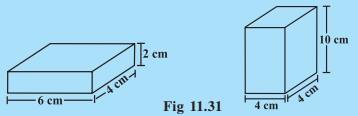
![]()
Answer:
(i) Total surface area
= 2(lb + bh + hl)
= 2(6×4+4×2+6×2)
= 2(24+8+12)
= 2(24+8+12)
= 88 cm2
(ii) Total surface area
= 2(lb + bh + hl)
= 2(4×4+4×10+10×4)
= 2(16+40+40)
= 2(96)
= 192 cm2
| NCERT Solutions for Class 8 Maths Chapter 9 Mensuration - Topic 9.4.2 Cube |
Question: Find the surface area of cube A and the lateral surface area of cube B (Fig 11.36).

![]()
Answer:
Surface area of cube A = 6l2
= 6(10)2 = 600 cm2
Lateral surface area of cube B = 4l2
= 4(8)2 = 4×64 = 256 cm2
| NCERT Solutions for Class 8 Maths Chapter 9 Mensuration: Topic 9.4.3 Cylinders |
Question:1 Find the total surface area of the cylinders following figure

![]()
Answer:
Total surface area of cylinder = 2πr (r + h)
(i) Area =
2π×14(14+8) = 2π×14(22) = 1935.22 cm2
(ii) Area = 2π×1(1+2) = 2π×1(3) =18.84 cm2
| Class 8 maths chapter 9 ncert solutions: Exercise: 9.2 Total Questions: 10 Page number: 114 |
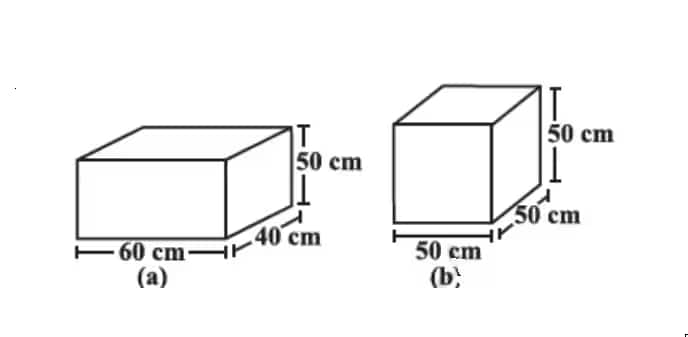
![]()
Answer:
Surface area of cuboid (a) =2(60×40+40×50+50×60)=14800 cm2
Surface area of cube (b) =6(50)2=15000 cm2
So, box (a) requires the lesser amount of material to make.
Answer:
Surface area of suitcase =2(80×48+48×24+24×80)=13824 cm2 .
Area of such 100 suitcases will be 1382400 cm2
So length of tarpaulin cloth = 1382400296 cm=14400 cm or 144m
Question:3 Find the side of a cube whose surface area is 600cm2.
Answer:
Surface area of cube = 6l2
So, 6l2 = 600
⇒ l2 = 100
⇒ l = 10 cm
Thus, the side of the cube is 10 cm.
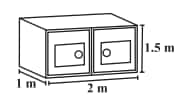
![]()
Answer:
Required area = Total area - Area of bottom surface
Total area =2(1×2+2×1.5+1.5×1)=13 m2
Area of bottom surface =1×2=2 m2
So, required area = 13−2 =11 m2
Answer:
Total area painted by Daniel :
= 2(15×10+10×7+7×15)−15×10 ( ∵ Bottom surface is excluded.)
So, Area
= 650−150 =500 m2
No. of cans of paint required
= 500100 = 5
Thus, 5 cans of paint are required.
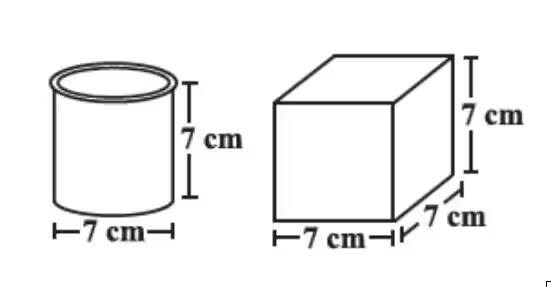
![]()
Answer:
The two figures have the same height. The difference between them is that one is a cylinder and another is a cube.
lateral surface area of cylinder = 2×π×r×h
= 2×π×3.5×7
= 154 cm2
lateral surface area of cube = 4×side2
=4×72
=4×49
=196 cm2
So, the cube has a larger lateral surface area.
Answer:
Total surface area of cylinder
= 2πr (h + r)
= 2π×7(7+3)
= 14π×10
= 440 m2
Answer:
Length of rectangular sheet
=422433=128 cm
So perimeter of rectangular sheet
= 2(l + b)
= 2(128+33)
= 322 cm
Thus perimeter of the rectangular sheet is 322 cm.
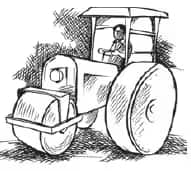
![]()
Answer:
Area in one complete revolution of roller = 2πrh
= 2π×0.42×1 = 2.638 m2
So, area of road = 2.638 m2 × 750 = 1979.20 m2
Area of label = 2πrh
=2π×(7)×(16)=703.71 cm2
Here, the height is found out as = 20-2-2 = 16 cm.
| NCERT class 8 maths ch 11 question answer: Topic 9.5.1 Cuboid |
Question:1 Find the volume of the following cuboids 
![]()
Answer:
(i) Volume of a cuboid is given as:
Volume of cuboid = length×breadth×height,
So, Given that Length = 8cm, Breadth = 3cm, and height = 2 cm so,
Its volume will be = 8cm×3cm×2cm = 48 cm3
Also, for the Given Surface area of a cuboid 24m2 and height = 3 cm,
We can easily calculate the volume:
Volume = Surface area×height
So, Volume = 24×3100 = 0.72m3
| NCERT Solutions for Class 8 Maths Chapter 9 Mensuration: Topic 9.5.2 Cube |
Question: Find the volume of the following cubes
(a) with a side 4 cm (b) with a side 1.5 m
Answer:
(a) The volume of a cube with a side equal to 4 cm will be
Volume = Side × side × side
⇒ Volume = 4cm × 4cm × 4cm = 64 cm3 .
(b) When having side length equal to 1.5m
then,
Volume = 1.5m × 1.5m × 1.5m = 3.375 m3
| NCERT Solutions for Class 8 Maths Chapter 9 Mensuration: Topic 9.5.2 Cube |
Question:1 Find the volume of the following cylinders. 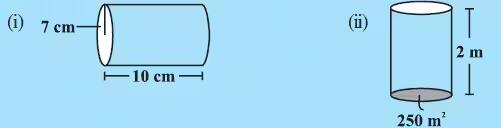
![]()
Answer:
(i) The volume of a cylinder given as = π × r2 × length
⇒ Given radius of cylinder = 7 cm and length of cylinder = 10 cm.
So, we can calculate the volume of the cylinder = π × (7cm)2 × 10cm
(Take the value of π=227 )
The volume of the cylinder = 1,540 cm3.
(ii) Given for the Surface area = 250 m2 and height = 2m
we have,
Volume of cylinder = 250 m2 × 2m = 500 m3
| Class 8 maths chapter 9 ncert solutions: Exercise: 9.3 Total Questions: 8 Page number: 119-120 |
Question: 1 Given a cylindrical tank, in which situation will you find surface area and in
which situation volume.
(a) To find how much it can hold.
Answer:
(a) To find out how much the cylindrical tank can hold we will basically find out the volume of the cylinder.
(b) Number of cement bags required to plaster it.
Answer:
(b) If we want to find out the cement bags required to plaster it means the area to be applied, we then calculate the surface area of the bags.
(c) To find the number of smaller tanks that can be filled with water from it.
Answer:
(c) We have to find out the volume.

![]()
Answer:
Given the diameter of cylinder A = 7cm and the height = 14cm.
Also, the diameter of cylinder B = 14cm and height = 7cm.
We can easily suggest whose volume is greater without doing any calculations:
Volume is directly proportional to the square of the radius of the cylinder and directly proportional to the height of the cylinder.
Hence,
B has more Volume as compared to A because B has a larger diameter.
Verifying:
Volume of A : π×r2×height=π × (72cm)2×14cm = 539 cm3
and Volume of B : π×r2×height=π×(142cm)2×7cm=1,078cm3 .
Hence, clearly we can see that the volume of cylinder B is greater than the volume of cylinder A.
The cylinder B has surface area of = 2×π×r(r+h)=2×π×14cm2(142+7)=616cm2 .
and the surface area of cylinder A = 2×π×r(r+h)=2×π×7cm2×(72+14)=385cm2 .
The cylinder with greater volume also has greater surface area.
Question:3 Find the height of a cuboid whose base area is 180 cm 2 and volume is 900 cm 3.
Answer:
Given the height of a cuboid whose base area is 180 cm2 and volume is 900 cm3
As the Volume of the cuboid = base area × height
So, we have the relation: 900 cm3 =180cm2 × height
⇒ Height = 5 cm
Answer:
So given the dimensions of a cuboid is 60 cm × 54 cm × 30 cm
Hence, its volume is equal to = 97,200 cm3
We have to make small cubes with a side of 6cm, which occupies the volume
= 6cm × 6cm × 6cm = 216 cm3
Hence, we now have one cube having a side length = 6 cm and volume = 216 cm3.
So, the total number of small cubes that can be placed in the given cuboid
=97200216=450
Hence, 450 small cubes can be placed in that cuboid.
Question:5 Find the height of the cylinder whose volume is 1.54 m 3 and diameter of the base is 140 cm?
Answer:
Given that the volume of the cylinder is 1.54 m3 and its diameter at the base = 140 cm
So, as the Volume of the cylinder = base area × height
Hence, putting in the relation, we get;
1.54 m3 = (π× (1.4 m2)2) × height
The height of the cylinder would be = 1 metre
Question:6 A milk tank is in the form of a cylinder whose radius is 1.5 m and length is 7 m. Find the quantity of milk in litres that can be stored in the tank.
Answer:
Volume of the cylinder = V = πr2h
⇒π(1.5)2×7=49.48 m3
So, the quantity of milk in litres that can be stored in the tank is 49500 litres.
(∵1 m3 = 1000 litres)
Question:7(i) If each edge of a cube is doubled, How many times will its surface area increase?
Answer:
The surface area of a cube = 6l2
So if we double the edge, l becomes 2l.
New surface area = 6(2l)2=24l2
Thus surface area becomes 4 times.
Question:7(ii): If each edge of a cube is doubled, how many times will its volume increase?
Answer:
Volume of cube = l3
Since l becomes 2l, so new volume is : (2l)3 = 8l3
Hence, the volume becomes 8 times.
Answer:
Given that the water is pouring into a cuboidal reservoir at the rate of 60 litres per minute.
The volume of the reservoir is 108 m3, then
The number of hours it will take to fill the reservoir will be:
As we know, 1m3 = 1000L
Then 108 m3 = 108,000 Litres
Time taken to fill the tank will be:
10800060=1800 minutes
1800 minutes = 180060=30 hours
NCERT Class 8 Maths chapter 9 solutions discuss some important 2D and 3D figures like cubes, cuboids, and cylinders. Here are the important features of these solutions.
NCERT Solutions for Class 8: Subject Wise
Students can use the following links to find the solutions for other subjects.
NCERT Books and NCERT Syllabus
During preparation, one of the most important tools is the latest syllabus. The following link will lead you to it. There are also links for some reference books, which are beneficial for students.
Addition and subtraction of the algebraic expression, multiplication of the algebraic expression, standard identities, and application of identities are important topics in this chapter.
CBSE class 8 maths is not tough at all. It teaches a very basic and simple maths.
There are 16 chapters starting from rational number to playing with numbers in the CBSE class 8 maths.
Here you will get the detailed NCERT solutions for class 8 by clicking on the link.
Here you will get the detailed NCERT solutions for class 8 maths by clicking on the link.
ncert.nic.in is the official website of the NCERT where you can get NCERT textbooks and syllabus from class 1 to 12.

Take Aakash iACST and get instant scholarship on coaching programs.

This ebook serves as a valuable study guide for NEET 2025 exam.

This e-book offers NEET PYQ and serves as an indispensable NEET study material.

As per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters

As per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
As per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE