NCERT Solutions for Class 8 Maths Chapter 10 Exponents and Powers
In this modern world with higher-level mathematics, finding the area of squares, calculating the compound interest or expressing larger data, nothing is possible without Exponents and Powers. This chapter on Exponents and Powers helps represent and work with larger numbers and calculations. This chapter lays the introduction to the rules and properties of exponents. None of the higher mathematical topics can be covered without this concept of Exponents and Powers. The NCERT Solutions for Class 8 Maths provide students with comprehensive step-by-step solutions to every exercise problem. These step-by-step solutions help students understand and apply the concepts effectively.
This Story also Contains
- Exponents and Powers Class 8 Questions And Answers PDF Free Download
- Exponents and Powers Class 8 NCERT Solutions (Exercise)
- NCERT Solutions for Class 8 Maths Chapter 10 Exponents and Powers - Topics
- Exponents and Powers Class 8 Solutions - Important Formulae
- NCERT Solutions for Class 8 Maths - Chapter Wise
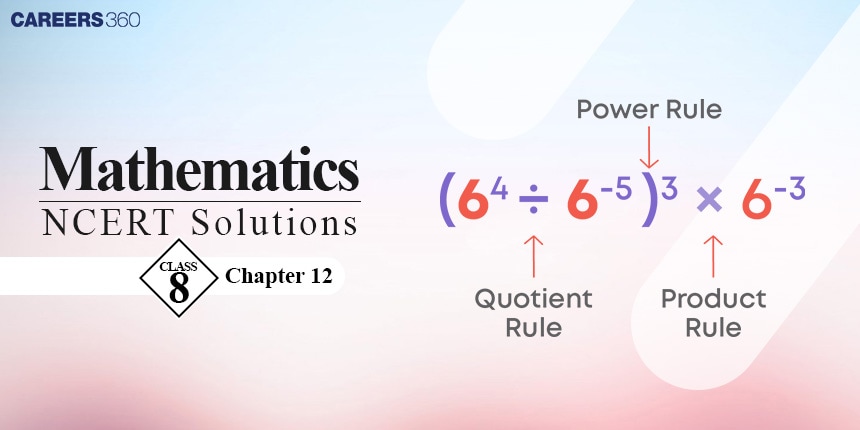
These NCERT Solutions are highly reliable as they are developed by the subject matter experts of Careers360. These solutions are of great help for the exam preparation as the students can practice the exercise problems and check the answers to evaluate their performance, and can focus on their weaker areas. Students can refer to the NCERT Solutions for Class 8 to access the subject-wise solutions for the Class.
Exponents and Powers Class 8 Questions And Answers PDF Free Download
Students who wish to access the NCERT solutions for Class 8 Maths Chapter 10, Exponents and Powers, can click on the link below to download the entire solutions in PDF format for free.
Exponents and Powers Class 8 NCERT Solutions (Exercise)
|
NCERT Solutions for Class 8 Maths Chapter 10 Exponents and Powers Exercise 10.1 Page Number: 125-126 Number of Questions: 7 |
Question:1 (i) Evaluate.
$3^{-2}$
Answer:
The detailed explanation for the above-written question is as follows,
We know that,
$a^{-m}=\frac{1}{a^{m}}$
So, here m =2 and a = 3
$3^{-2}=\frac{1}{3^{2}} = \frac{1}{3}\times \frac{1}{3} = \frac{1}{9}$
Question: 1(ii) Evaluate.
$(-4)^{-2}$
Answer:
The detailed explanation for the above-written question is as follows
We know that,
$a^{-m}= \frac{1}{a^{m}}$
So, here (a = -4) and (m = 2)
Then, according to the law of exponent
$(-4)^{-2}= \frac{1}{(-4)^{2}} = \frac{1}{(-4)}\times \frac{1}{(-4)} = \frac{1}{16}$ [ negative $\times$ negative = positive]
Question: 1(iii) Evaluate.
$\left(\frac{1}{2}\right )^{-5}$
Answer:
The detailed solution for the above-written question is as follows
We know that,
$(\frac{a}{b})^{m}=\frac{a^{m}}{b^{m}}$ $\&$ $a^{-m} = \frac{1}{a^{m}}$
So, here
a = 1 and b = 2 and m =-5
According to the law of exponent
$(\frac{1}{2})^{-5}=\frac{1^{-5}}{2^{-5}} = \frac{-1}{2^{-5}}$
$=(-1)\times -2\times -2\times -2\times -2\times -2 = 32$
Question: 2(i) Simplify and express the result in power notation with a positive exponent.
$(-4)^{5}\div (-4)^{8}$
Answer:
The detailed solution for the above-written question is as follows
We know the exponential formula
$\frac{a^{m}}{a_{n}} = a^{m-n}$ and $a^{-m}= \frac{1}{a^{m}}$
So according to this
a = -4, m = 5 and n = 8
$\frac{4^{5}}{4_{8}} = -4^{5-8} = -4^{-3}$
$= (-\frac{1}{4})\times -(\frac{1}{4})\times(-\frac{1}{4}) = -\frac{1}{64}$
Question: 2(ii) Simplify and express the result in power notation with a positive exponent.
$\left (\frac{1}{2^3} \right )^2$
Answer:
The detailed solution for the above-written question is as follows
We know the exponential formula
$\frac{a^{m}}{b^{m}} = (\frac{a}{b})^{m}$ and $a^{-m}= \frac{1}{a^{m}}$ and $(a^{m})^{n} = a^{mn}$
So, we have given
a = 1, b=2
By using the above exponential law,
$\frac{a^{m}}{b^{m}} = \frac{1}{(2^{3})^{2}} = \frac{1}{2^{6}}$
$= \frac{1}{2}\times \frac{1}{2}\times \frac{1}{2}\times \frac{1}{2}\times \frac{1}{2}\times \frac{1}{2} = \frac{1}{64}$
Question: 2(iii) Simplify and express the result in power notation with a positive exponent.
$(-3)^4\times \left(\frac{5}{3} \right )^{4}$
Answer:
The detailed solution for the above-written question is as follows,
We know the exponential formula
$(\frac{a}{b})^{m}= \frac{a^{m}}{b^{m}}$
So, $(-3)^{4}\times (\frac{5}{3})^{4}= (-3)^{4}\times \frac{5^{4}}{3^{4}} = \frac{625}{1}$
Question: 2(iv) Simplify and express the result in power notation with a positive exponent.
$(3^{-7}\div 3^{-10})\times 3^{-5}$
Answer:
The detailed explanation for the above-written question is as follows
As we know, the exponential form
$\frac{a^{m}}{b^{n}}= a^{m-n} \& (a^{m}\times a^{n})=a^{m+n}$
By using these two forms, we get,
$\frac{3^{-7}}{3^{-10}}\times (3)^{-5}=3^{-7-(-10)} \times (3)^{-5}$
$=3^{3} \times (3)^{-5}= 3^{-2}$
$=\frac{1}{3}\times \frac{1}{3}=\frac{1}{9}$
Question:2(v) Simplify and express the result in power notation with a positive exponent.
$2^{-3} \times (-7)^{-3}$
Answer:
The detailed solution for the above-written question is as follows,
we know the exponential forms
$a^{-m}=\frac{1}{a^{m}}$ & $a^{m}\times b^{m}= (ab)^{m}$
So, according to our data,
Here, initially, we use the first form and then the second one.
$2^{-3}\times (-7)^{-3}= \frac{1}{2^{3}}\times \frac{1}{(-7)^{3}}$
$= \frac{1}{(2\times -7)^{3}}= \frac{1}{(-14)^{3}}$
Question:3(i) Find the value of.
Answer:
The detailed explanation for the above-written question is as follows,
As we know that $a^{0}=1$
So, $3^{0}=1$
now,
$=(1+\frac{1}{4})\times 2^{2}$
$=\frac{5}{4}\times 2^{2}\Rightarrow \frac{5}{2^{2}}\times 2^{2}=5/1$
Question: 3(ii) Find the value of.
$(2^{-1}\times 4^{-1})\div 2^{-2}$
Answer:
The detailed explanation for the above-written question is as follows
Rewrite the equation
$(2^{-1}\times 4^{-1})\div 2^{-2}$ $= (2^{-1}\times 2^{-2})\div 2^{-2}$
$=(2^{-1+(-2)})\div 2^{-2}$ ................................. $a^{m}\times a^{n}= a^{(m+n)}$
$=(2^{-3})\div 2^{-2} = 2^{-3-(-2)}$ ........................ $a^{m}\div a^{n}= a^{(m-n)}$
$= 2^{-1}= \frac{1}{2}$
Question: 3(iii) Find the value of.
$\left (\frac{1}{2}\right)^{-2} + \left (\frac{1}{3}\right)^{-2} + \left (\frac{1}{4}\right)^{-2}$
Answer:
The detailed explanation for the above-written question is as follows,
This is the exponential form
$(a/b)^{m}= \frac{a^{m}}{b^{m}}$
So, $\left (\frac{1}{2}\right)^{-2} + \left (\frac{1}{3}\right)^{-2} + \left (\frac{1}{4}\right)^{-2}$
$=\frac{1}{2^{-2}}+\frac{1}{3^{-2}}+\frac{1}{4^{-2}}$ .......................using this form $a^{m}= \frac{1}{a^{m}}$
$=2^{2}+3^{2}+4^{2}$
= 4+9+16
= 29
Question: 3(iv) Find the value of.
$(3^{-1} + 4^{-1} + 5^{-1})^0$
Answer:
since we know that
$a^{0}=1$
$(3^{-1} + 4^{-1} + 5^{-1})^0$ $=1$
Question: 3(v) Find the value of.
$\left \{ \left (\frac{-2}{3} \right )^{-2} \right \}^{2}$
Answer:
The detailed explanation for the above-written question is as follows
$\left \{ \left (\frac{-2}{3} \right )^{-2} \right \}^{2}$
$=(-2/3)^{-2\times 2}$ .............. By using these form of exponential $(a^{m})^{n}=a^{mn}$
$(-2/3)^{-4}=(-3/2)^{4}$ ......... use this $a^{-m}=\frac{1}{a^{m}}$
$=\frac{81}{16}$
Question:4(i) Evaluate
$\frac{8^{-1}\times 5^{3}}{2^{-4}}$
Answer:
The detailed explanation for the above-written question is as follows
$\frac{8^{-1}\times 5^{3}}{2^{-4}}$
After rewriting the above equation, we get,
$\frac{2^{-3}\times 5^{3}}{2^{-4}}$ $=2^{-3-(-4)}\times 5^{3}$ ...........as we know that $\frac{a^{m}}{a^{n}}= a^{m-n}$
$\\=2^{1}\times 5^{3}\\ = 2\times 125 = 250$
An alternate method,
$= \frac{5^{3}}{2^{-4}\times 2^{3}}$
here you can use first $a^{-m}= \frac{1}{a^{m}}$ and after that use $a^{m}\times a^{n}= a^{m+n}$
Question: 4(ii) Evaluate
$(5^{-1}\times 2^{-1})\times 6^{-1}$
Answer:
The detailed explanation for the above-written question is as follows
We clearly see that this is in the form of $a^{-m}=\frac{1}{a^{m}}$
So, $(5^{-1}\times 2^{-1})\times 6^{-1}= (\frac{1}{5}\times \frac{1}{2})\times \frac{1}{6}$
$= \frac{1}{60}$
Question: 5 Find the value of $m$ for which
Answer:
We have,
$a^{m}\div a^{n}= a^{m-n}$
Here a = 5 and n =-3 and m-n = 5
therefore,
$5^{m}\div 5^{-3}= 5^{m+3} = 5^{5}$
By comparing both sides, we get,
m+3 = 5
m= 2
Question: 6(i) Evaluate
$\left \{\left (\frac{1}{3} \right )^{-1} - \left( \frac{1}{4} \right )^{-1} \right \}^{-1}$
Answer:
The detailed solution for the above-written question is as follows
$\left \{\left (\frac{1}{3} \right )^{-1} - \left( \frac{1}{4} \right )^{-1} \right \}^{-1}$
$= [(1\times 3)-(1\times 4)]^{-1}$ .............by using $a^{-m}= \frac{1}{a^{m}}$
$= [3-4]^{-1}$
$\\= [-1]^{-1}\\=-1$
Question: 6(ii) Evaluate
$\left ( \frac{5}{8} \right )^{-7}\times \left (\frac{8}{5}\right)^{-4}$
Answer:
The detailed solution for the above-written question is as follows
$\left ( \frac{5}{8} \right )^{-7}\times \left (\frac{8}{5}\right)^{-4}$
$=\frac{5^{-7}}{8^{-7}}\times \frac{8^{-4}}{5^{-4}}$ ................ using the form $\frac{a^{m}}{b^{m}}= (a/b)^{m}$
$=5^{-7+4}\times 8^{-4+7}$ ............using $a^{m} \div a^{n} = a^{m-n}$
$= 5^{-3}\times 8^{+3}$
$\\= \frac{8^{3}}{5^{3}}\\\\=\frac{512}{125}$
Question: 7(i) Simplify
$\frac{25\times t^{-4}}{5^{-3}\times 10\times t^{-8}}\;\;(t\neq 0)$
Answer:
The detailed solution for the above-written question is as follows
$\frac{25\times t^{-4}}{5^{-3}\times 10\times t^{-8}}\;\;(t\neq 0)$
we can write $25= 5^{2}$
So, after rewriting the equation,
$\frac{5^{2}\times t^{-4}}{5^{-3}\times 10\times t^{-8}}$
$= \frac{5^{2+3}\times t^{-4+8}}{10}$ .................using the form $[a^{m}\div a^{n}= a^{m-n}]$
$= \frac{5^{5}\times t^{4}}{10}$ .............(By expanding, we get,)
$= \frac{625t^{4}}{2}$
Question: 7(ii) Simplify.
$\frac{3^{-5}\times 10^{-5}\times 125}{5^{-7}\times6 ^{-5}}$
Answer:
The detailed solution for the above-written question is
$\frac{3^{-5}\times 10^{-5}\times 125}{5^{-7}\times6 ^{-5}}$
we can write 125 = $5^{3}$ and $6^{-5}$ can be written as $(2\times 3)^{-5}$
Now, rewriting the equation, we get
$=\frac{3^{-5}\times 10^{-5}\times 5^{3}}{5^{-7}\times(2\times 3) ^{-5}}$
$=\frac{3^{-5}\times 10^{-5}\times 5^{3+7}}{(2\times 3) ^{-5}}$ .............by using $[a^{m}\div a^{n}=a^{m-n}]$
$=\frac{ 10^{-5}\times 5^{10}}{(2) ^{-5}}$ .....................Use $[a^{m}\div a^{n}=a^{m-n}]$
$5^{10-5}= 5^{5}=3125$
As $[10^{-5} = (2\times 5)^{-5}=2^{-5}\times 5^{-5}]$ .
$2^{-5}$ can be cancelled out with the denominator $2^{-5}$
|
NCERT Solutions for Class 8 Maths Chapter 10 Exponents and Powers Exercise 10.2 Page Number: 128 Number of Questions: 4 |
Question: 1(i) Express the following numbers in standard form.
0.0000000000085
Answer:
The standard form is $8.5\times 10^{-12}$
Question: 1(ii) Express the following numbers in standard form.
0.00000000000942
Answer:
The standard form is $9.42\times 10^{-12}$
Question: 1(iii) Express the following numbers in standard form.
6020000000000000
Answer:
The standard form is $6.02\times 10^{15}$
Question: 1(iv) Express the following numbers in standard form.
0.00000000837
Answer:
The standard form of the given number is $8.37\times10^{-9}$
Question: 1(v) Express the following numbers in standard form.
31860000000
Answer:
The standard form is $3.186\times10^{10}$
Question: 2(i) Express the following numbers in the usual form.
$3.02\times 10^{-6}$
Answer:
$3.02\times 10^{-6}$
$=\frac{3.02}{1000000}$
$=0.00000302$
This is the usual form.
Question: 2 (ii) Express the following numbers in the usual form.
$4.5 \times 10^4$
Answer:
$4.5 \times 10^4=4.5\times 10000$
$=45000$
This is the usual form.
Question: 2(iii) Express the following numbers in the usual form.
$3\times 10^{-8}$
Answer:
$3\times 10^{-8}$
$\frac{3}{100000000} = 0.000000030$
This is the usual form.
Question: 2(iv) Express the following numbers in the usual form.
$1.0001\times 10^9$
Answer:
$1.0001\times 10^9$
$=1.0001\times 100000000$
$=1000100000$
This is the usual form.
Question: 2(v) Express the following numbers in the usual form.
Answer:
$5.8\times 10^{12}$
$=5.8\times 100000000000$
$=5800000000000$
This is the usual form.
Question: 2(vi) Express the following numbers in the usual form.
Answer:
$3.61492 \times 10^6$
$= 3.61492\times 1000000$
$= 3614920$
17155
Question: 3(i) Express the number appearing in the following statements in standard form.
1 micron is equal to $\frac{1}{1000000}m$.
Answer:
1 micron is equal to
$\frac{1}{1000000}m$
$= 1\times 10^{-6}$
Question: 3(ii) Express the number appearing in the following statements in standard form.
The charge of an electron is 0.000,000,000,000,000,000,16 coulomb.
Answer:
The charge of an electron is 0.000,000,000,000,000,000,16 coulomb.
$=1.6\times 10^{-19}$ coulomb.
Question: 3(iii) Express the number appearing in the following statements in standard form.
The size of a bacteria is 0.0000005 m.
Answer:
The size of a bacteria is 0.0000005 m
$\frac{5}{10000000}=5\times 10^{-7}m$
Question: 3(iv) Express the number appearing in the following statements in standard form.
The size of a plant cell is 0.00001275 m.
Answer:
The size of a plant cell is 0.00001275 m
$=\frac{1275}{10000}=1.275\times10^{-5}m$
Question: 3(v) Express the number appearing in the following statements in standard form.
The thickness of a thick paper is 0.07 mm.
Answer:
The thickness of a thick paper is 0.07
$=\frac{7}{100}=7\times 10^{-2}mm$
Answer:
The thickness of each book = 20mm
So, the thickness of 5 books = $(5\times 20)=100 mm$
The thickness of one paper sheet =0.016mm
So, the thickness of 5 paper sheet = $(5\times 0.016)=0.08 mm$
The total thickness of the stack = (100+0.08)mm
=100.08 mm or $(1.008\times10^{-2}mm)$
NCERT Solutions for Class 8 Maths Chapter 10 Exponents and Powers - Topics
The topics discussed in the NCERT Solutions for class 8, chapter 10, Exponents and Powers, are:
- Powers with Negative Exponents
- Laws of Exponents
- Use of Exponents to Express Small Numbers in Standard Form
- Comparing very large and very small numbers
Exponents and Powers Class 8 Solutions - Important Formulae
Here are some important formulas or laws that will help students to solve problems related to Exponents and Powers.
-
Law of Product: $a^m \times a^n=a^{(m+n)}$
-
Law of Quotient: $\frac{a^m}{a^n}=a^{(m-n)}$
-
Law of Zero Exponent: $a^{0} = 1$
-
Law of Negative Exponent: $a^{(-m)}= \frac{1}{ a^m}$
-
Law of Power of a Power: $\left(a^m\right)^n=a^{\left(mn\right)}$
-
Law of Power of a Product: $(a b)^n=a^{n} b^n$
-
Law of Power of a Quotient: $(\frac{a}{b})^m= \frac{a^m}{b^m}$
NCERT Solutions for Class 8 Maths - Chapter Wise
Given below are all the chapter-wise NCERT class 8 maths solutions given in one place for ease of access:
NCERT Solutions for Class 8 - Subject Wise
The NCERT solutions for class 8 Maths is one of the essential study materials for students in exam preparation. Check out the Subject-wise NCERT Solutions for Class 8 below.
NCERT Books and NCERT Syllabus
It is very important to focus on other study resources like NCERT Books and NCERT Syllabus to get an overview of the topics covered in this academic year. Students can use the below links to access the NCERT Syllabus and book PDFs.
Popular Questions
Courses After 12th
Applications for Admissions are open.
This ebook serves as a valuable study guide for NEET 2025 exam.
NEET Previous 10 Year Questions
Get nowThis e-book offers NEET PYQ and serves as an indispensable NEET study material.
JEE Main Important Physics formulas
Get nowAs per latest syllabus. Physics formulas, equations, & laws of class 11 & 12th chapters
JEE Main Important Chemistry formulas
Get nowAs per latest syllabus. Chemistry formulas, equations, & laws of class 11 & 12th chapters
JEE Main high scoring chapters and topics
Get nowAs per latest 2024 syllabus. Study 40% syllabus and score upto 100% marks in JEE
JEE Main Important Mathematics Formulas
Get nowAs per latest syllabus. Maths formulas, equations, & theorems of class 11 & 12th chapters
