NCERT Solutions for Class 7 Maths Chapter 9 Perimeter and Area

A perimeter gives the length of the boundary of a shape, which can be calculated by adding the lengths of the boundary. An area gives the space covered within the boundaries of the shape. This chapter covers all essential formulas like the perimeter and areas of a square, a rectangle, a parallelogram, a triangle, and a circle. The NCERT Solutions in this article give detailed step-by-step solutions to help students understand these essential concepts used in our daily lives.

Latest: NCERT Class 7 Maths: Chapterwise Important Formulas with Examples and Points

This Story also Contains

1. NCERT Solutions for Class 7 Maths Chapter 9 Perimeter and Area - Important Formulae
2. NCERT Solutions for Maths Chapter 9 Perimeter and Area Class 7 - Download PDF
3. NCERT Solution for Class 7 Maths Chapter 9 (Exercise)
4. Perimeter And Area Class 7 Maths Chapter 11- Topics
5. NCERT Solutions for Class 7 Maths Chapter 9 Perimeter and Area - Points to Remember
6. NCERT Solutions for Class 7 Maths Chapter Wise
7. NCERT Solutions for Class 7 Subject Wise

The NCERT Solutions for Class 7 Maths are solved by the subject matter experts at Careers360, providing accurate and reliable study material for the students in a very easily understandable and accessible format. These solutions help students practice more problems and evaluate their own performance to work more effectively in their weaker areas, making it a greater help for exam preparation.

NCERT Solutions for Class 7 Maths Chapter 9 Perimeter and Area - Important Formulae

Perimeter: The total length of the boundary of a two-dimensional shape.

Circumference: The distance around the boundary of a circle.

Area: The measure of the space enclosed by a two-dimensional shape.

Congruent: Two shapes are congruent if they have the same size and shape.

Base: The bottom side of a two-dimensional shape, like a parallelogram or triangle.

Height: The perpendicular distance between the base and the opposite side in a two-dimensional shape.

Diameter: The longest chord (line segment connecting two points on a circle) passing through the centre of a circle.

Radius: The distance from the centre of a circle to any point on its boundary.

Unit Conversions: Converting measurements from one unit to another, based on their relationships.

Unit Conversions:

$\begin{array}{rl}& 1{\mathrm{cm}}^2=100{\mathrm{mm}}^2\\ & 1{\mathrm{m}}^2=10000{\mathrm{cm}}^2\\ & 1\text{ hectare }=10000{\mathrm{m}}^2\end{array}$

Formulas:

Perimeter of a Triangle = Side1 + Side2 + Side3

Perimeter of a Square = 4 × side of the square

Perimeter of a Rectangle = 2 × ( Length + Breadth or Width )

Circumference or Perimeter of a Circle$=\pi d=2\pi r$ where d = Diameter of circle = 2r, r = Radius of circle

Area of a Square = Side × Side

Area of a rectangle = Length × Breadth

Area of a parallelogram = Base × Height

Area of a Triangle = ($\frac{1}{2}$) × ( Base) × ( Height)

Area of a circle $=\pi r^2$ where, r = Radius of circle

NCERT Solutions for Maths Chapter 9 Perimeter and Area Class 7 - Download PDF

NCERT Solution for Class 7 Maths Chapter 9 (Exercise)

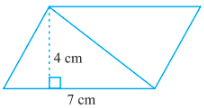
Question: 1(a)Find the area of the following parallelograms:

Answer: We know that

Area of parallelogram $=base\times height$

Here,

Base of parallelogram = 7cm

Height of parallelogram = 4 cm

$\Rightarrow 7\times 4=28c{m}^2$

Therefore, the area of the parallelogram is $28c{m}^2$

Question: 1(b) Find the area of the following parallelograms:

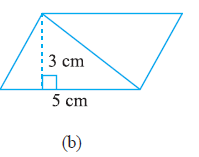
Answer:

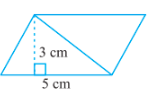
We know that

Area of parallelogram $=base\times height$

Here,

Base of parallelogram = 5cm

and

Height of parallelogram = 3 cm

$\Rightarrow 3\times 5=15c{m}^2$

Therefore, the area of the parallelogram is $15c{m}^2$

Question: 1(c) Find the area of the following parallelograms:

Answer: We know that

Area of parallelogram $=base\times height$

Here,

Base of parallelogram = 2.5cm

Height of parallelogram = 3.5 cm

$\Rightarrow 3.5\times 2.5=8.75c{m}^2$

Therefore, the area of the parallelogram is $8.75c{m}^2$

Question: 1(d) Find the area of the following parallelograms:

Answer: We know that

Area of parallelogram $=base\times height$

Here,

Base of parallelogram = 5cm

Height of parallelogram = 4.8 cm

$\Rightarrow 5\times 4.8=24c{m}^2$

Therefore, the area of a parallelogram is $24c{m}^2$

Question: 1(e) Find the area of the following parallelograms:

Answer: We know that

Area of parallelogram $=base\times height$

Here,

Base of parallelogram = 2 cm

Height of parallelogram = 4.4 cm

$\Rightarrow 2\times 4.4=8.8c{m}^2$

Therefore, the area of a parallelogram is $8.8c{m}^2$

Question: 2(a) Find the area of each of the following triangles:

Answer: We know that

Area of triangle $=\frac{1}{2}\times base\times height$

Here,

Base of triangle = 4 cm

and

Height of triangle =3 cm

$\Rightarrow \frac{1}{2}\times 4\times 3=6c{m}^2$

Therefore, the area of the triangle is $6c{m}^2$

Question: 2(b) Find the area of the following triangles:

Answer: We know that

Area of triangle $=\frac{1}{2}\times base\times height$

Here,

Base of triangle = 5 cm

Height of triangle =3.2 cm

$\Rightarrow \frac{1}{2}\times 5\times 3.2=8c{m}^2$

Therefore, the area of the triangle is $8c{m}^2$

Question: 2(c) Find the area of the following triangles:

Answer: We know that

Area of triangle $=\frac{1}{2}\times base\times height$

Here,

Base of triangle = 3 cm

Height of triangle =4 cm

$\Rightarrow \frac{1}{2}\times 3\times 4=6c{m}^2$

Therefore, the area of the triangle is $6c{m}^2$

Question: 2(d) Find the area of the following triangles:

Answer: We know that

Area of triangle $=\frac{1}{2}\times base\times height$

Here,

Base of triangle = 3 cm

Height of triangle =2 cm

$\Rightarrow \frac{1}{2}\times 2\times 3=3c{m}^2$

Therefore, the area of the triangle is $3c{m}^2$

Question: 3 Find the missing values:

Answer: We know that

Area of parallelogram $=base\times height$

a) Here, the base and area of the parallelogram are given

$\Rightarrow 246=20\times height$

$\Rightarrow height=\frac{246}{20}=12.3cm$

b) Here height and area of the parallelogram are given

$\Rightarrow 154.5=15\times base$

$\Rightarrow base=\frac{154.5}{15}=10.3cm$

c) Here height and area of the parallelogram are given

$\Rightarrow 48.72=8.4\times base$

$\Rightarrow base=\frac{48.72}{8.4}=5.8cm$

d) Here base and area of the parallelogram are given

$\Rightarrow 16.38=15.6\times height$

$\Rightarrow height=\frac{16.38}{15.6}=1.05cm$

Question: 4 Find the missing values:

Answer: We know that

Area of triangle $=\frac{1}{2}\times base\times height$

a) Here, the base and area of the triangle is given

$\Rightarrow 87=\frac{1}{2}\times 15\times height$

$\Rightarrow height=\frac{87}{7.5}=11.6cm$

b) Here height and area of the triangle are given

$\Rightarrow 1256=\frac{1}{2}\times 31.4\times base$

$\Rightarrow base=\frac{1256}{15.7}=80mm$

c) Here base and area of the triangle is given

$\Rightarrow 170.5=\frac{1}{2}\times 22\times height$

$\Rightarrow height=\frac{170.5}{11}=15.5cm$

Question: 5(a) $PQRS$ is a parallelogram (Fig 11.23). $QM$ is the height from $Q$ to $SR$ and $QN$ is the height from $Q$ to $PS$ . If $SR=12cm$ and

$QM=7.6cm.$ Find: the area of the parallelogram $PQRS$

Answer: We know that

Area of parallelogram $=base\times height$

Here,

Base of parallelogram = 12 cm

Height of parallelogram = 7.6 cm

$\Rightarrow 12\times 7.6=91.2c{m}^2$

Therefore, the area of the parallelogram is $91.2c{m}^2$

Question: 5(b) $PQRS$ is a parallelogram (Fig 11.23). $QM$ is the height from $Q$ to $SR$ and $QN$ is the height from $Q$ to $PS$ . If $SR=12cm$ and $QM=7.6cm.$ Find: $QN$ , if $PS=8cm$.

Answer: We know that

Area of parallelogram $=base\times height$

Here,

Base of parallelogram = 12 cm

Height of parallelogram = 7.6 cm

$\Rightarrow 12\times 7.6=91.2c{m}^2$

Now,

The area is also given by $QN\times PS$

$\Rightarrow 91.2=QN\times 8$

$\Rightarrow QN=\frac{91.2}{8}=11.4cm$

Therefore, the value of QN is $11.4cm$

Question: 6 $DL$ and $BM$ are the heights on sides $AB$ and $AD$ respectively, of parallelogram $ABCD$ (Fig 11.24). If the area of the parallelogram is $1470c{m}^2.$ $AB=35cm$ and $AD=49cm,$ find the length of $BM$ and $DL.$

Answer: We know that

Area of parallelogram $=base\times height$

Here,

Base of parallelogram(AB) = 35 cm

Height of parallelogram(DL) = h cm

$\Rightarrow 1470=35\times h$

$\Rightarrow h=\frac{1470}{35}=42cm$

Similarly,

The area is also given by $AD\times BM$

$\Rightarrow 1470=49\times BM$

$\Rightarrow BM=\frac{1470}{49}=30cm$

Therefore, the values of BM and DL are 30cm and 42cm, respectively

Question: 7 $\mathrm{\Delta }ABC$ is right-angled at $A$ (Fig 11.25). $AD$ is perpendicular to $BC$ . If $AB=5cm,$ $BC=13cm$ and $AC=12cm,$ Find the area of $\mathrm{\Delta }ABC$ . Also, find the length of $AD$.

Answer: We know that

Area of triangle $=\frac{1}{2}\times base\times height$

Now,

When base = 5 cm and height = 12 cm

Then, the area is equal to

$\Rightarrow \frac{1}{2}\times 5\times 12=30c{m}^2$

Now,

When base = 13 cm and height = AD area remains the same

Therefore,

$\Rightarrow 30=\frac{1}{2}\times 13\times AD$

$\Rightarrow AD=\frac{60}{13}cm$

Therefore, the value of AD is $\frac{60}{3}cm$ and the area is equal to $30c{m}^2$

Question: 8 $\mathrm{\Delta }ABC$ is isosceles with $AB=AC=7.5cm$ and $BC=9cm$ (Fig 11.26). The height $AD$ from $A$ to $BC,$ is $6cm,$ Find the area of $\mathrm{\Delta }ABC.$ What will be the height from $C$ to $AB$ i.e., $CE$ ?

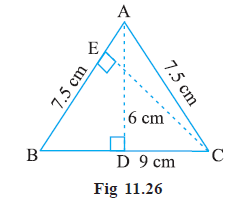
Answer: We know that

Area of triangle $=\frac{1}{2}\times base\times height$

Now,

When base(BC) = 9 cm and height(AD) = 6 cm

Then, the area is equal to

$\Rightarrow \frac{1}{2}\times 9\times 6=27c{m}^2$

Now,

When base(AB) = 7.5 cm and height(CE) = h , area remain same

Therefore,

$\Rightarrow 27=\frac{1}{2}\times 7.5\times CE$

$\Rightarrow CE=\frac{54}{7.5}=7.2cm$

Therefore, the value of CE is 7.2cm and the area is equal to $27c{m}^2$

Question: 1(a) Find the circumference of the circles with the following radius: (Take $\pi =\frac{22}{7}$ )

$14cm$

Answer: We know that

Circumference of a circle is $=2\pi r$

$\Rightarrow 2\pi r=2\times \frac{22}{7}\times 14=88cm$

Therefore, the circumference of the circle is 88 cm

Question: 1(b) Find the circumference of the circles with the following radius: (Take $\pi =\frac{22}{7}$ )

$28mm$

Answer: We know that

Circumference of circle is $=2\pi r$

$\Rightarrow 2\pi r=2\times \frac{22}{7}\times 28=176mm$

Therefore, the circumference of the circle is 176 mm

Question: 1(c) Find the circumference of the circles with the following radius: (Take $\pi =\frac{22}{7}$ )

$21cm$

Answer: We know that

Circumference of circle is $=2\pi r$

$\Rightarrow 2\pi r=2\times \frac{22}{7}\times 21=132cm$

Therefore, the circumference of the circle is 132 cm

Question: 2(a) Find the area of the following circles, given that:

$radius=14mm$ (Take $\pi =\frac{22}{7}$ )

Answer: We know that

Area of circle is $=\pi r^2$

$\Rightarrow \pi r^2=\frac{22}{7}\times (14{)}^2=616m{m}^2$

Therefore, the area of the circle is $616m{m}^2$

Question: 2(b) Find the area of the following circles, given that:

$diameter=49m$

Answer: We know that

Area of circle is $=\pi r^2$

$\Rightarrow \pi r^2=\frac{22}{7}\times {\left(\frac{49}{2}\right)}^2=1886.5m^2$

Therefore, the area of the circle is $1886.5m^2$

Question: 2(c) Find the area of the following circles, given that:

$radius=5cm$

Answer: We know that

Area of circle is $=\pi r^2$

$\Rightarrow \pi r^2=\frac{22}{7}\times {\left(5\right)}^2=\frac{550}{7}c{m}^2$

Therefore, the area of the circle is $\frac{550}{7}c{m}^2$

Question: 3 If the circumference of a circular sheet is $154m,$ find its radius. Also, find the area of the sheet. (Take $\pi =\frac{22}{7}$ )

Answer: It is given that the circumference of a circular sheet is 154 m

We know that

Circumference of circle is $=2\pi r$

$\Rightarrow 154=2\pi r$

$\Rightarrow 154=2\times \frac{22}{7}\times r$

$\Rightarrow r=\frac{49}{2}=24.5m$

Now,

Area of circle $=\pi r^2$

$\Rightarrow \frac{22}{7}\times {\left(24.5\right)}^2=1886.5m^2$

Therefore, the radius and area of the circle are 24.5 m and $1886.5m^2$ respectively

Question: 4 A gardener wants to fence a circular garden of diameter $21m$. Find the length of the rope he needs to purchase if he makes $2$ rounds of the fence. Also find the cost of the rope, if it costs $Rs.4permeter.$ (Take $\pi =\frac{22}{7}$ ).

Answer: It is given that the diameter of a circular garden is $21m$.

We know that

Circumference of circle is $=2\pi r$

$\Rightarrow 2\times \frac{22}{7}\times \frac{21}{2}=66m$

Now, the length of the rope required to make $2$ rounds of fence is

$\Rightarrow 2\times$ circumference of circle

$\Rightarrow 2\times 66=132m$

Now, cost of rope at $Rs.4permeter$ is

$\Rightarrow 132\times 4=528Rs$

Therefore, the length of the rope required to make $2$ rounds of fence is 132 m and the cost of rope at $Rs.4permeter$ is Rs 528

Question: 5 From a circular sheet of radius $4cm$, a circle of radius $3cm$ is removed. Find the area of the remaining sheet.(Take $\pi =3.14$ )

Answer: We know that

Area of circle $=\pi r^2$

Area of circular sheet with radius 4 cm $=3.14\times (4{)}^2=50.24c{m}^2$

Area of the circular sheet with radius 3 cm $=3.14\times (3{)}^2=28.26c{m}^2$

Now,

Area of remaining sheet = Area of the circle with radius 4 cm - Area of the circle with radius 3 cm

$=50.24-28.26=21.98c{m}^2$

Therefore, the Area of the remaining sheet is $21.98c{m}^2$

Question: 6 Saima wants to put lace on the edge of a circular table cover of diameter $1.5m$. Find the length of the lace required and also find its cost if one meter of the lace costs $Rs.15.$ (Take $\pi =3.14$ )

Answer: It is given that the diameter of a circular table is 1.5m.

We know that

Circumference of circle is $=2\pi r$

$\Rightarrow 2\times \frac{22}{7}\times \frac{1.5}{2}=4.71m$

Now, the length of the lace required is

$\Rightarrow$ circumference of circle $=4.71m$

Now, cost of lace at $Rs.15permeter$ is

$\Rightarrow 4.71\times 15=70.65Rs$

Therefore, the length of the lace required is 4.71 m and the cost of lace at $Rs.15permeter$ is Rs 70.65

Question: 7 Find the perimeter of the adjoining figure, which is a semicircle including its diameter.

Answer: It is given that the diameter of a semicircle is 10 cm.

We know that

Circumference of semi-circle is $=\pi r$

Circumference of a semi-circle with a diameter of 10 cm including diameter is

$\Rightarrow (\frac{22}{7}\times \frac{10}{2})+10=15.7+10=25.7cm$

Therefore, the Circumference of a semi-circle with a diameter of 10 cm including a diameter is 25.7 cm

Question: 8 Find the cost of polishing a circular table-top of diameter $1.6m$, if the rate of polishing is $Rs.15/m^2$. (Take $\pi =3.14$ )

Answer: It is given that the diameter of a circular table is 1.6m.

We know that

Area of circle is $=\pi r^2$

$\Rightarrow 3.14\times {\left(\frac{1.6}{2}\right)}^2=2.0096m^2$

Now, the cost of polishing at $Rs.15per{m}^2$ is

$\Rightarrow 2.0096\times 15=30.144Rs$

Therefore, the cost of polishing at $Rs.15per{m}^2$ is Rs 30.144

Question: 9 Shazli took a wire of length $44cm$ and bent it into the shape of a circle. Find the radius of that circle. Also, find its area. If the same wire is bent into the shape of a square, what will be the length of each of its sides? Which figure encloses more area, the circle or the square? (Take $\pi =\frac{22}{7}$ )

Answer: It is given that the length of the wire is 44 cm

Now, we know that

Circumference of the circle (C) = $2\pi r$

$\Rightarrow 44=2\pi r$

$\Rightarrow r=\frac{44\times 7}{2\times 22}=7cm$

Now,

Area of circle (A) = $\pi r^2$

$\Rightarrow \pi r^2=\frac{22}{7}\times (7{)}^2=154c{m}^2$ - (i)

Now,

Perimeter of square(P) = $4a$

$\Rightarrow 44=4a$

$\Rightarrow a=\frac{44}{4}=11cm$

Area of sqaure = $a^2$

$\Rightarrow a^2=(11{)}^2=121c{m}^2$ -(ii)

From equations (i) and (ii) we can clearly see that the area of the circular-shaped wire is more than the square-shaped wire

Question: 10 From a circular card sheet of radius $14cm$, two circles of radius $3.5cm$ and a rectangle of length $3cm$ and breadth $1cm$ are removed.(as shown in the adjoining figure). Find the area of the remaining sheet. (Take $\pi =\frac{22}{7}$ )

Answer: It is given that the radius of the circular card sheet is $14cm$

Now, we know that

Area of circle (A) = $\pi r^2$

$\Rightarrow \pi r^2=\frac{22}{7}\times (14{)}^2=616c{m}^2$ - (i)

Now,

The area of the circle with a radius of 3.5 cm is

$\Rightarrow \pi r^2=\frac{22}{7}\times (3.5{)}^2=38.5c{m}^2$

Area of two such circle is = $38.5\times 2=77c{m}^2$ -(ii)

Now, Area of rectangle = $l\times b$

$\Rightarrow l\times b=3\times 1=3c{m}^2$ -(iii)

Now, the remaining area is (i) - [(ii) + (iii)]

$\Rightarrow 616-[77+3]\Rightarrow 616-80=536c{m}^2$

Therefore, the area of the remaining sheet is $536c{m}^2$

Question: 11 A circle of radius $2cm$ is cut out from a square piece of an aluminium sheet of side $6cm$. What is the area of the leftover aluminium sheet?

(Take $\pi =3.14$ )

Answer: It is given that the radius of the circle is $2cm$

Now, we know that

Area of the circle (A) = $\pi r^2$

$\Rightarrow \pi r^2=3.14\times (2{)}^2=12.56c{m}^2$ - (i)

Now,

Now, the Area of square = $a^2$

$\Rightarrow a^2=(6{)}^2=36c{m}^2$ -(ii)

Now, the remaining area is (ii) - (i)

$\Rightarrow 36-12.56=23.44c{m}^2$

Therefore, the area of the remaining aluminium sheet is $23.44c{m}^2$

Question: 12 The circumference of a circle is $31.4cm$. Find the radius and the area of the circle. (Take $\pi =3.14$ )

Answer: It is given that the circumference of the circle is $31.4cm$

Now, we know that

Circumference of circle is = $2\pi r$

$\Rightarrow 31.4=2\pi r$

$\Rightarrow 31.4=2\times 3.14\times r$

$\Rightarrow r=5cm$

Now, the Area of the circle (A) = $\pi r^2$

$\Rightarrow \pi r^2=3.14\times (5{)}^2=78.5c{m}^2$

Therefore, the radius and area of the circle are $5cmand78.5c{m}^2$ respectively

Question: 13 A circular flower bed is surrounded by a path $4m$ wide. The diameter of the flower bed is $66m$. What is the area of this path? $(\pi =3.14)$

Answer: It is given that the diameter of the flower bed is $66m$

Therefore, $r=\frac{66}{2}=33m$

Now, we know that

Area of the circle (A) = $\pi r^2$

$\Rightarrow \pi r^2=3.14\times (33{)}^2=3419.46m^2$ -(i)

Now, Area of outer circle with radius(r ') = 33 + 4 = 37 cm is

$\Rightarrow \pi r^{\prime 2}=3.14\times (37{)}^2=4298.66m^2$ -(ii)

The area of the path is equation (ii) - (i)

$\Rightarrow 4298.66-3419.46=879.2m^2$

Therefore, the area of the path is $879.2m^2$

Question: 14 A circular flower garden has an area of $314m^2$. A sprinkler at the centre of the garden can cover an area that has a radius of $12m$. Will the sprinkler water the entire garden? (Take $\pi =3.14$ )

Answer: It is given that the radius of the sprinkler is $12m$

Now, we know that

Area of the circle (A) = $\pi r^2$

The area covered by sprinkles is

$\Rightarrow \pi r^2=3.14\times (12{)}^2=452.16m^2$

And the area of the flower garden is $314m^2$

Therefore, the sprinkler waters the entire garden

Question: 15 Find the circumference of the inner and the outer circles, shown in the adjoining figure. (Take $\pi =3.14$ )

Answer: We know that

Circumference of circle = $2\pi r$

Now, the circumference of the inner circle with radius (r) = $19-10=9m$ is

$\Rightarrow 2\pi r=2\times 3.14\times 9=56.52m$

And the circumference of the outer circle with radius (r ') = 19 m is

$\Rightarrow 2\pi r=2\times 3.14\times 19=119.32m$

Therefore, the circumference of the inner and outer circles are $56.52mand119.32m$ respectively

Question: 16 How many times a wheel of radius $28cm$ must rotate to go $352m$? (Take $\pi =\frac{22}{7}$ )

Answer: It is given that the radius of the wheel is $28cm$

Now, we know that

Circumference of circle = $2\pi r$

$\Rightarrow 2\pi r=2\times 3.14\times 28=175.84cm$

Now, the number of rotations done by the wheel to go 352 m is

$\Rightarrow \frac{352m}{175.84cm}=\frac{35200}{175.84}\cong 200$

Therefore, the number of rotations done by the wheel to go 352 m is 200

Question: 17 The minute hand of a circular clock is $15cm$ long. How far does the tip of the minute hand move in $1$ hour? (Take $\pi =3.14$ )

Answer: It is given that the minute hand of a circular clock is $15cm$ long i.e. ( r = 15 cm)

Now, we know that one hour means a complete circle of minute hand

Now,

Circumference of circle = $2\pi r$

$\Rightarrow 2\pi r=2\times 3.14\times 15=94.2cm$

Therefore, the distance covered by a minute hand in one hour is 94.2 cm

Perimeter And Area Class 7 Maths Chapter 11- Topics

• Squares And Rectangles
• Triangles As Parts Of Rectangles
• Area Of A Parallelogram
• Area Of A Triangle
• Circles
• Circumference Of A Circle
• Area Of a Circle

NCERT Solutions for Class 7 Maths Chapter 9 Perimeter and Area - Points to Remember

Area of a triangle: If the base length and height of a triangle are given

$Area=\frac{1}{2}\times base\times height$

The perimeter of the triangle: The perimeter will be equal to the sum of the sides of the triangle

$\text{Perimeter}=a+b+c$

a- First side of the triangle

b- Second side of the triangle

c- Third side of the triangle

Area of Circles:

$Area=\pi r^2$

r- Radius of the circle

Circumference of Circle:

$\text{Circumference }=2\pi r$

r- Radius of the circle

Area of a parallelogram:

$Area=b\times h$

b- base length

h- height

NCERT Solutions for Class 7 Maths Chapter Wise

NCERT Solutions for Class 7 Subject Wise

Careers360 also provides solutions for all other subjects of Class 7. These subject-wise solutions contain all the solutions for all the chapters, subject-wise. Check out the links below to access the subject-wise Class 7 solutions.

• NCERT Solutions for Class 7 Maths
• NCERT Solutions for Class 7 Science

Students can also check the NCERT Books and the NCERT Syllabus here:

• NCERT Syllabus Class 7 Maths
• NCERT Books Class 7
• NCERT Syllabus Class 7