NCERT Solutions for Class 7 Maths Chapter 5 Parallel and Intersecting Lines

NCERT Solutions for Class 7 Maths Chapter 5 Parallel and Intersecting Lines: Download Free PDF

Careers360 brings you NCERT Class 7 Maths Chapter 5 Parallel and Intersecting Lines solutions, carefully prepared by subject experts to simplify your studies and help in exams. A downloadable PDF is available — click the link below to access it.

Download PDF

NCERT Solutions for Class 7 Maths Chapter 5 Parallel and Intersecting Lines Solutions: Exercise

NCERT Class 7 Maths Chapter 5 Parallel and Intersecting Lines question answers with detailed explanations are provided below.

Figure it Out

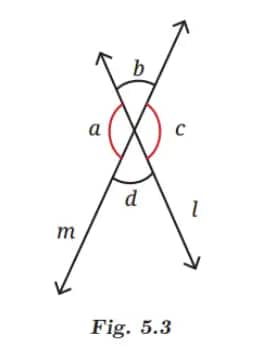
List all the linear pairs and vertically opposite angles you observe in Fig. 5.3:

Answer:

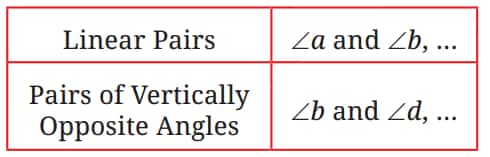
Linear pair angles: ∠a and ∠b; ∠b and ∠c; ∠c and ∠d; ∠a and ∠d.

Vertically opposite angles: ∠a and ∠c; ∠b and ∠d.

Figure it Out

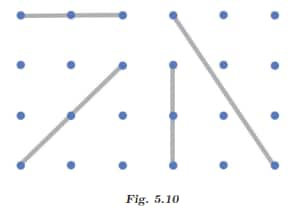
Q1: Draw some lines perpendicular to the lines given on the dot paper in Fig. 5.10.

Answer:

Draw lines that intersect the given lines at 90° angles, using a set square or protractor to ensure right angles. Do it Yourself.

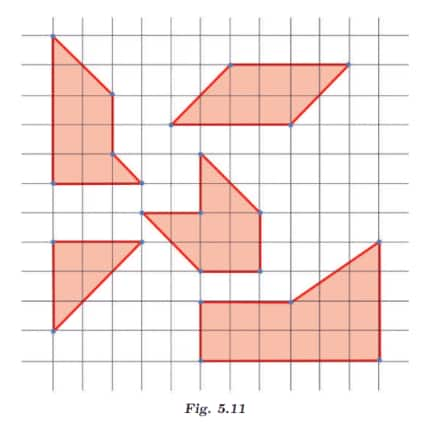
Q2: In Fig. 5.11, mark the parallel lines using the notation given above (single arrow, double arrow, etc.). Mark the angle between perpendicular lines with a square symbol.

(a) How did you spot the perpendicular lines?

(b) How did you spot the parallel lines?

Answer:

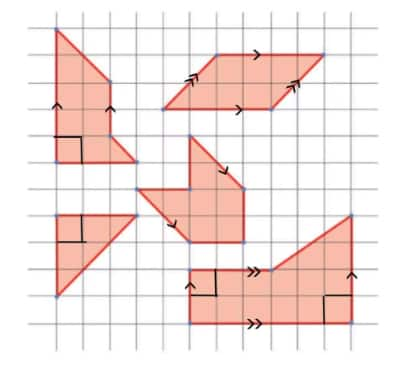
(a) Lines that intersect at a 90° angle are perpendicular.

(b) Lines that do not meet, no matter how far they are extended, are parallel.

Q3: In the dot paper following, draw different sets of parallel lines. The line segments can be of different lengths, but should have dots as endpoints.

Answer:

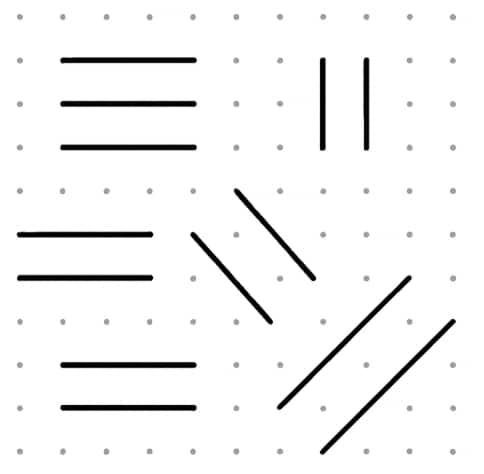
Q4: Using your sense of how parallel lines look, try to draw lines parallel to the line segments on this dot paper.

(a) Did you find it challenging to draw some of them?

(b) Which ones?

(c) How did you do it?

Answer:

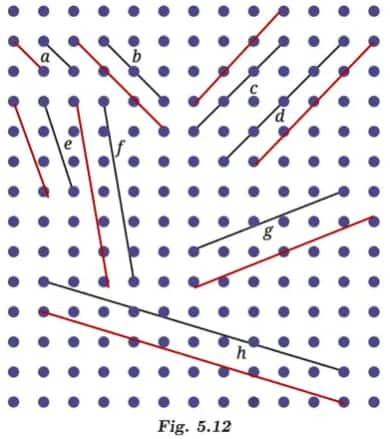
(a) Yes, some line segments are a little more difficult to draw than others.

(b) Line segments e, f, h, and g.

(c) Parallel lines are drawn by keeping them equidistant from the given lines.

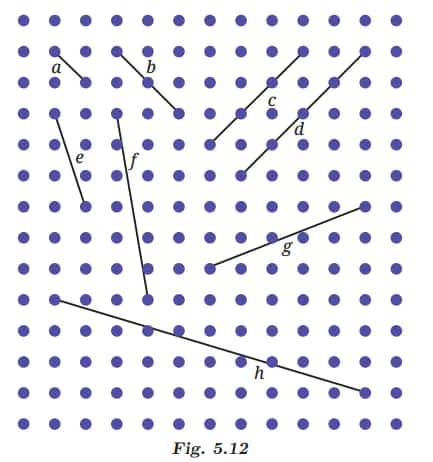
Q5: In Fig. 5.13, which line is parallel to line a – line b or line c? How do you decide this?

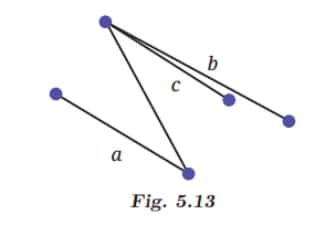
Answer:

In the given figure, line a is parallel to line c because these two lines are always the same distance apart and never meet, no matter how far they go.

Figure it Out

Can you draw a line parallel to l, that goes through point A? How will you do it with the tools from your geometry box? Describe your method.

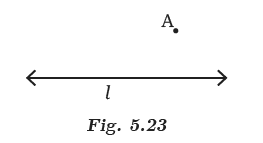
Answer:

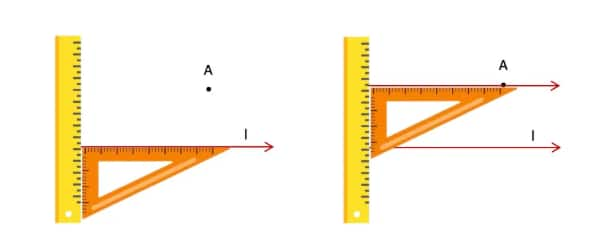
Step-by-step procedure:

• Align one edge of the set square with line l.

• Place a ruler along the other perpendicular edge.

• Slide the set square along the ruler until it reaches point A.

• Draw a line through A along the set square’s edge.

• This is the required line parallel to l.

Figure it Out

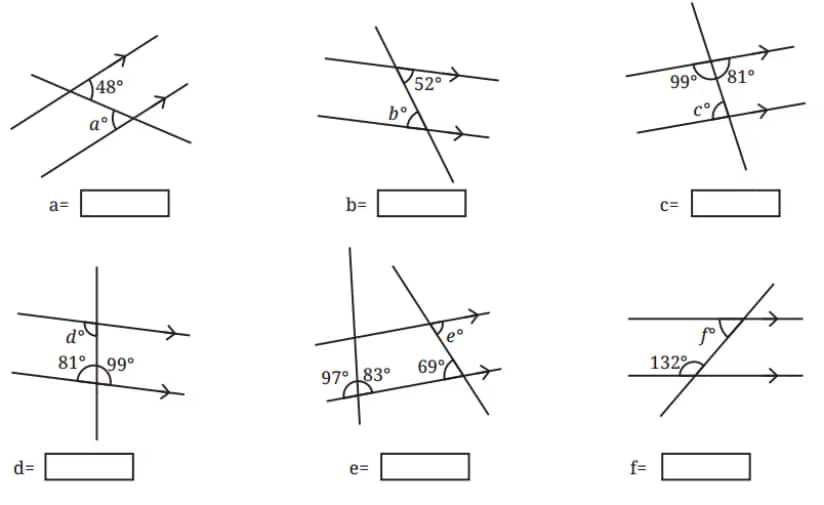
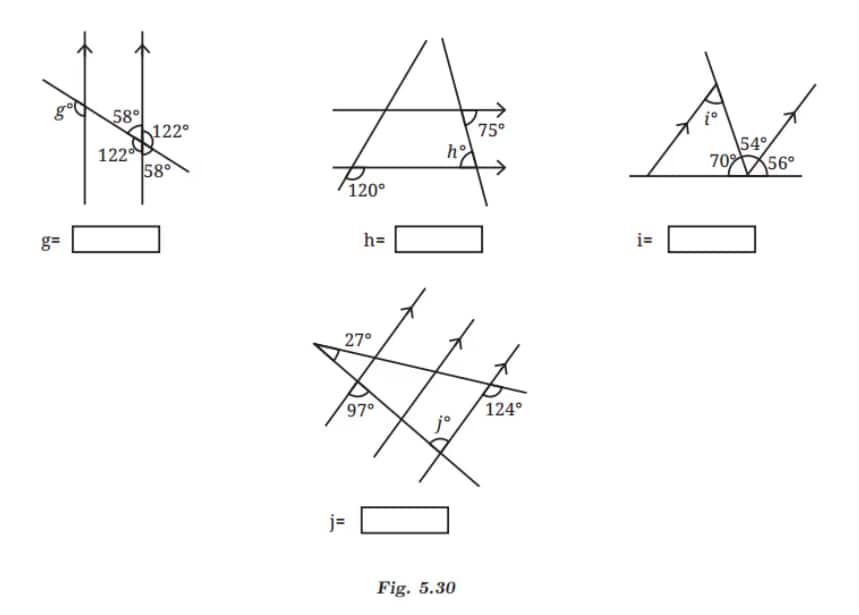
Q1: Find the angles marked below.

Answer:

(i) Since alternate interior angles formed by a transversal intersecting a pair of parallel lines are equal, ∠a = 48°.

(ii) Since alternate interior angles formed by a transversal intersecting a pair of parallel lines are equal, ∠b = 52°.

(iii) Since alternate interior angles formed by a transversal intersecting a pair of parallel lines are equal, ∠c = 81°.

(iv) Since alternate interior angles formed by a transversal intersecting a pair of parallel lines are equal, ∠d = 99°.

(v) Since alternate interior angles formed by a transversal intersecting a pair of parallel lines are equal, ∠e = 69°.

(vi) Since the sum of interior angles on the same side of a transversal is always equal to 180°. Therefore, ∠f + 132° = 180°
∠f = 180° – 132°
∠f = 48°.

(vii) Since corresponding angles formed by a transversal intersecting a pair of parallel sides are equal, ∠g = 122°.

(viii) Since alternate interior angles formed by a transversal intersecting a pair of parallel lines are equal, ∠h = 75°.

(ix) Since alternate interior angles formed by a transversal intersecting a pair of parallel lines are equal, ∠i = 54°.

(x) Since alternate interior angles formed by a transversal intersecting a pair of parallel lines are equal, ∠j = 97°.

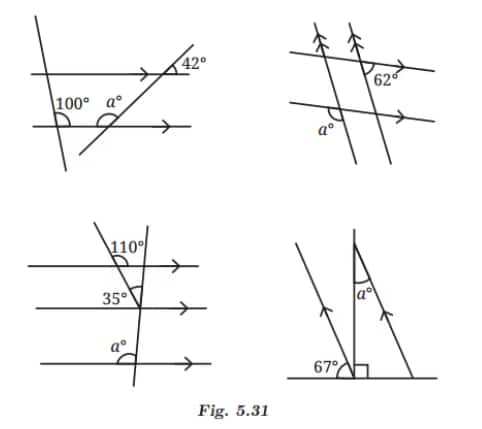
Q2: Find the angle represented by a.

Answer:

(i)

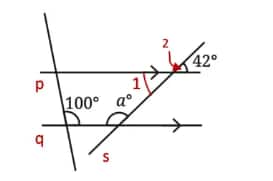
∠1 = ∠2 = 42° ……….(Vertically opposite angles)

Line p is parallel to q, and s is a transversal, then

∠a + ∠1 = 180° …………….(Sum of interior angles on the same side of the transversal)

⇒ ∠a + 42° = 180°

⇒ ∠a = 180° − 42°

⇒ ∠a = 138°

(ii)

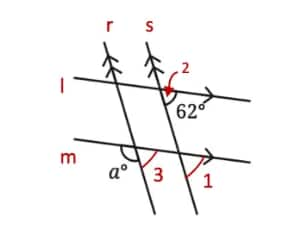
Line l is parallel to line m, and s is a transversal, then

∠1 = ∠2 = 62° ……………(Corresponding angles)

Line r is parallel to line s, and m is a transversal, then

∠1 = ∠3 = 62° ……………(Corresponding angles)

∠a + ∠3 = 180° …………..(Linear pair angles)

⇒ ∠a + 62° = 180°

⇒ ∠a = 180° − 62°

⇒ ∠a = 118°

(iii)

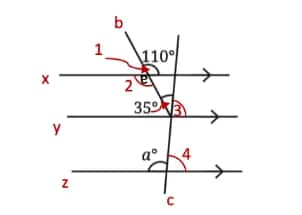
∠1 = ∠2 = 110° …… (Vertically opposite angles)

Line x is parallel to line y, and b is a transversal, then

∠2 = 35° + ∠3 ……… (Alternate interior angles)

⇒ 110° = 35° + ∠3

⇒110° – 35° = ∠3

⇒ ∠3 = 75°.

Line y is parallel to line z, and c is the transversal, then

∠3 = ∠4 = 75° ……. (Corresponding angles)

∠a + ∠4 = 180° ……… (Linear pair angles)

⇒ ∠a + 75° = 180°

⇒ ∠a = 180° – 75°

⇒ ∠a = 105°

(iv)

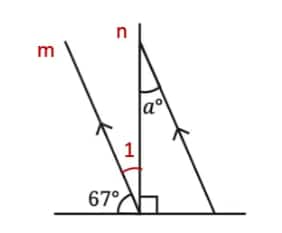
∠1 + 67° + ∠2 = 180° ……… (Sum of angles on a straight line)

⇒ ∠1 + 67° + 90° = 180°

⇒ ∠1 + 157° = 180°

⇒ ∠1 = 180° – 157°

⇒ ∠1 = 23°.

⇒ ∠1 = ∠a = 23° …… (Alternate interior angles).

Q3: In the figures below, what angles do x and y stand for?

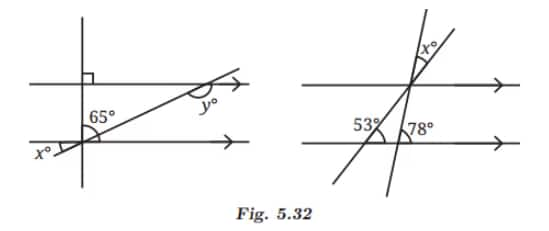
Answer:

(i)

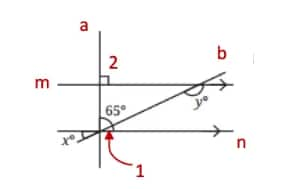
Line m is parallel to line n, and a is a transversal, then

∠2 = 65° + ∠1 ………(Corresponding angles)

⇒ 90° = 65° + ∠1

⇒ ∠1 = 90° – 65°

⇒ ∠1 = 25°.

⇒ ∠1 = x = 25° ………..(Vertically opposite angles)

Line m is parallel to line n, and b is a transversal, then

∠1 + ∠y = 180° ……………(Sum of interior angles on the same side of the transversal)

⇒ 25° + ∠y = 180°

⇒ ∠y = 180° – 25°

⇒ ∠y = 155°.

(ii)

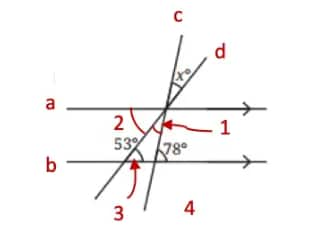
Line a is parallel to line b, and line d is a transversal, then

∠2 = ∠3 = 53° …………..(Alternate interior angles)

Also, line a is parallel to line b, and line c is a transversal, then

∠1 + ∠2 = ∠4 …………. (Alternate interior angles)

⇒ ∠1 + 53° = 78°

⇒ ∠1 = 78° – 53°

⇒ ∠1 = 25°.

Therefore, ∠1 = x = 25° ………….(Vertically opposite angles).

Q4: In Fig. 5.33, ∠ABC = 45° and ∠IKJ = 78°. Find angles ∠GEH, ∠HEF, and ∠FED.

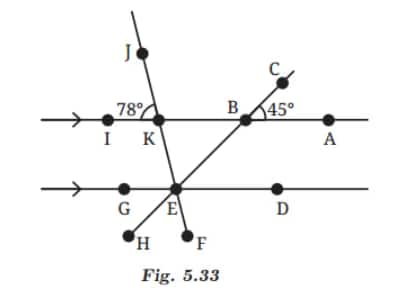
Answer:

∠GEH = 45° (Exterior alternate angles)

∠FED = 78° (Exterior alternate angles)

So, ∠GEH + ∠HEF + ∠FED = 180° (all lie on a straight line)

⇒ 45° + ∠HEF + 78° = 180°

⇒ ∠HEF = 57°

Q5: In Fig. 5.34, AB is parallel to CD, and CD is parallel to EF. Also, EA is perpendicular to AB. If ∠BEF = 55°, find the values of x and y.

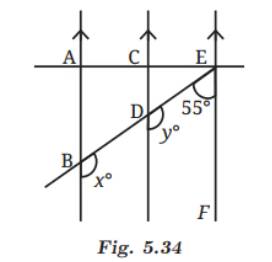
Answer:

y° + 55° = 180° (Co-interior angles)

⇒ y° = 180° - 55° = 125°

Also, x° = y° ( Corresponding angles)

⇒ x° = y° = 125°.

Q6: What is the measure of angle ∠NOP in Fig. 5.35?

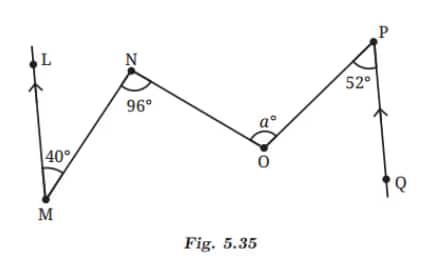
Answer:

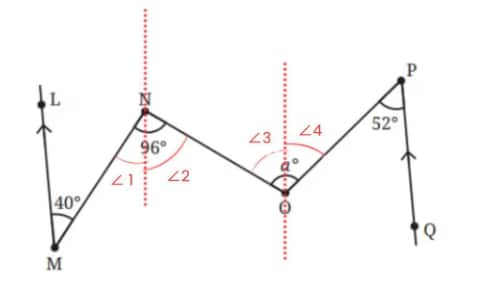
∠1 = 40° (Alternate angles are equal)

∠2 = 90° - 40° = 56°

∠3 = 56° (Alternate angles are equal)

∠4 = 52° (Alternate angles are equal)

So, ∠NOP = ∠a = 56° + 52° = 108°.

NCERT Class 7 Maths Chapter 5 Parallel and Intersecting Lines: Topics

Topics you will learn in NCERT Class 7 Maths Chapter 5 Parallel and Intersecting Lines include:

• Across the Line
• Perpendicular Lines
• Between Lines
• Parallel and Perpendicular Lines in Paper Folding
• Transversals
• Corresponding Angles
• Alternate Angles
• Consecutive Angle
• Parallel Illusions

Class 7 Maths Chapter 5 Parallel and Intersecting Lines Solutions: Extra Question

Question:

A line $l$ intersects two parallel lines $m$ and $n$. If one of the corresponding angles is $65^{\circ}$, find all the other corresponding angles.

Solution:

When a transversal cuts two parallel lines, the corresponding angles are equal.
So, if one corresponding angle is equal to $65^ {\circ}$, then all corresponding angles formed will also be $65^{\circ}$.

Thus, there are 4 corresponding angles, and each measures: $65^{\circ}$

Hence, the correct answer is $65^{\circ}$.

Parallel and Intersecting Lines Class 7 NCERT Solutions: Notes

Careers360 has prepared these Class 7 Parallel and Intersecting Lines Notes to make your revision smoother and faster. Additionally, these notes will help students to understand the Parallel and Intersecting Lines NCERT solutions and solve them on their own from next time.

Definitions

• Line: A straight path that can go infinitely in both directions.
• Line Segment: A portion of a line with two proper endpoints.
• Ray: A section of a line that starts from a point and extends indefinitely in one direction.
• Intersecting Lines: Lines which can intersect or cross one another at a point.
• Point of Intersection: The point where two lines meet each other.
• Parallel Lines: Lines that are on the same plane but never intersect with each other, regardless of how far out you draw them.
• Perpendicular Lines: Lines that meet each other and form a right angle (90°) at the point where they intersect.

Properties

• Lines that intersect each other meet at a point.
• Parallel lines never intersect and are always equidistant from each other.
• Perpendicular lines intersect each other at right angles.

Example:

• Examples of Parallel Lines are: Railway lines, lines in a notebook, and sides of a ruler.
• Examples of Intersecting Lines are: Scissors blades, crossed wires, and intersecting roads.
• Examples of Perpendicular Lines are: The corners of a square table, where walls meet the floor, and more.

Representation:

• Parallel lines are represented as: AB || CD
• Perpendicular lines are represented as: AB ⊥ CD
  

Parallel and Intersecting Lines Class 7 NCERT Solutions: Points to Remember

Check the following important points to understand and solve Class 7 Maths Chapter 5 Parallel and Intersecting Lines question answers effectively.

• Every line is a straight line that extends indefinitely in both directions.
• A line segment is defined by its two endpoints.
• Lines intersect at precisely one point.
• Parallel lines never cut at any point and always remain at equal distances.
• A transversal is a line that intersects two or more lines.
• Lines that intersect create four angles at the intersection point.
• Vertically opposite angles are always congruent.
• The sum of two adjacent angles of two intersecting lines is 180°.
• If a transversal intersects two parallel lines, then it creates eight angles.

Why are Class 7 Maths Chapter 5 Parallel and Intersecting Lines question answers important?

The fifth chapter of Class 7, Parallel and Intersecting Lines, is important in Geometry because it builds foundational geometry concepts that students will use in higher classes. Some important concepts include perpendicular lines, corresponding angles, transversals, consecutive angles, alternate angles, and many more. Here’s why the Class 7 Maths Parallel and Intersecting Lines question answers are important.

• This chapter builds the base for advanced topics in Classes 8, 9, and 10 geometry, especially for chapters like Triangles, Coordinate Geometry, and Lines and Angles.
• Parallel lines and intersecting lines are used in real life, like railway tracks, doors, windows, roads, and architectural designs, and solving questions improves logical understanding and connects math to real-world situations.
• Questions from this chapter often appear in school exams and Olympiads. So, checking these solutions will help students to improve their confidence and scoring ability.

NCERT Solutions for Class 7 Maths Chapter Wise

Given below are the chapter-wise NCERT solutions for class 7 mathematics, provided in one place:

NCERT Books and NCERT Syllabus

Download NCERT books and check the syllabus for Class 7 Maths from the links given below.

• NCERT Syllabus Class 7 Maths
• NCERT Books for Class 7 Maths
• NCERT Books Class 7
• NCERT Syllabus Class 7